1.
Introduction
As two important kinds of additive categories, abelian categories and triangulated categories are ubiquitous in mathematics. A natural problem is to consider the relationship between them. It is well known that homotopy categories and derived categories of abelian categories are triangulated categories.
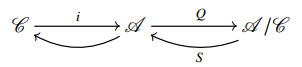
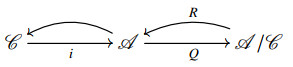
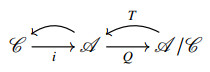
Let us recall some background. For details, we refer the reader to the online notes [1] and we will recall the relevant notions in Section 2. Recall that for an abelian category A, a full additive subcategory C of A is called a Serre subcategory if C is closed under taking subobjects, quotients and extensions. In this case, we can form the the Serre quotient of A by C by inverting each morphism f in A such that its kernel and cokernel belong to C. The Serre quotient A/C is also an abelian category and the quotient functor Q:A→A/C is an exact functor. In this case, we will say that
is a short exact sequence of abelian categories, where i:C→A is the inclusion functor.
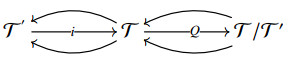
Similarly one can define short exact sequences of triangulated categories. Let T be a triangulated category. A triangulated subcategory T′ of T is called a thick subcategory if it is closed under taking direct summands ([2] and [3,Proposition 1.3]). Then we can define the Verdier quotient T/T′, which is the localization of T by inverting all morphisms f in T whose cones lie in T′. The Verdier quotient T/T′ is still a triangulated category, and the quotient functor Q:T→T/T′ is a triangle functor. In this case, we will say that
is a short exact sequence of triangulated categories, where i:T′→T is the inclusion functor.
It is a natural question to see whether a short exact sequence of abelian categories (1.1) gives rise to short exact sequences of triangulated categories by taking their homotopy categories or derived categories.
Let us introduce some notations. For ∗=+,−,b,∅, we denote by C∗(A) the category of left bounded, right bounded, bounded and unbounded complexes of A, respectively. Let K∗(A) and D∗(A) be the corresponding versions for homotopy categories and derived categories. Note that for unbounded versions, we usually delete ∅ in the notations, although K∅(A) has a different meaning in [2].
A naive question is whether (1.1) induces short exact sequences of derived categories
for ∗=+,−,b or ∅. It is easy to see by examples that this naive version is wrong and one reason is that D∗(C) is NOT necessarily a triangulated subcategory of D∗(A). We should replace D∗(C) by D∗C(A), i.e. the full subcategory of D∗(A) consisting of complexes whose cohomology groups belong to C. In [4,Theorem 3.2], J. Miyachi provided such a short exact sequence for left bounded, right bounded and bounded derived categories, respectively.
Theorem 1.1. [4,Theorem 3.2] Let 0⟶Ci⟶AQ⟶A/C⟶0 be a short exact sequence of abelian categories. For each ∗∈{+,−,b}, we have an induced short exact sequence of triangulated categories
where D∗C(A)={X∙∈D∗(A) | ∀i∈Z,Hi(X∙)∈C}.
The objective of this paper is to refine the above result of J. Miyachi, more precisely, we want to consider the corresponding versions for complex categories, homotopy categories and unbounded derived categories.
It reveals that the key point is to produce short exact sequences of complexes categories.
Theorem 1.2 (Theorems 3.1 and 3.5). A short exact sequence of abelian categories
induces short exact sequences of abelian categories
for ∗∈{+,−,b,∅}.
The left bounded /right bounded/bounded versions of the above result and their proofs have been implicit in the work of J. Miyachi [4]. It is surprising to notice that for the unbounded version, we do NOT need any extra conditions.
Based on Theorem 1.2, we obtain short exact sequences of homotopy categories.
Theorem 1.3 (Theorem 4.3). Let 0⟶Ci⟶AQ⟶A/C⟶0 be a short exact sequence of abelian categories. We have induced short exact sequences of triangulated categories
for ∗∈{+,−,b,∅}.
It seems that there is no natural description of the kernel of the functor Q∗:K∗(A)→K∗(A/C), although we provide such one in Proposition 4.2.
Now we can easily deduce Miyachi's result and its unbounded version.
Theorem 1.4 (Theorem 5.2). Let 0⟶Ci⟶AQ⟶A/C⟶0 be a short exact sequence of abelian categories. We have induced short exact sequences of triangulated categories
for ∗∈{+,−,b,∅}.
Notice that based on the induced short exact sequences of complex categories, the proofs for homotopy categories and derived categories are rather direct.
Next we consider the question when the natural functor D∗(C)→D∗C(A) is an equivalence. D. Yao showed that the fullness implies that dense property for bounded derived categories [5,Theorem 2.1]. We consider the left/right bounded cases and the unbounded case under the following conditions (★1) and (★2) on the inclusion functor i:C→A:
(★1) A has countable coproducts, C is closed under countable coproducts in A and countable coproducts of exact sequences are exact;
(★2) A has countable products, C is closed under countable products in A and countable products of exact sequences are exact.
We also show that the dense property also implies the fully faithfulness, as far as we assume a slighter stronger dense property; see Proposition 6.7.
This paper is organised as follows. Section 2 contains some preliminaries, including basic notions about calculi of fractions, and we also recall basic facts about localisations of abelian and triangulated categories as well as exact sequences, (co)localisation sequences and recollements. Section 3 is the core part of this paper, in which we study the induced short exact sequences of complex categories. It contains much technical details. In Sections 4 and 5, we consider induced short exact sequences for homotopy categories and derived categories, respectively. In particular, we show Theorem 5.2 which is an unbounded version of the result of J. Miyachi. In Section 6, we continue the line of research begun by D. Yao. We consider several criterions for the natural functor D∗(C)→D∗C(A) to be an equivalence. In the last Section 7, we present some applications and examples, some known, while others unknown.
In this paper, we don't care about set theoretical difficulties, that is, we always assume that the categories involved exist.
2.
Preliminaries
2.1. Calculi of fractions
In this subsection, we recall basic facts about calculi of fractions. For an introduction to localisations of categories, we refer the reader to [6].
Definition 2.1. [6,Section 2.2] Let A be a category. A class of morphisms S in A admits a calculus of left fractions (or is a left localizing class) if it satisfies the following conditions (morphisms in S will be denoted by ⟹):
(i) For every object C∈A, the identity morphism idC belongs to S;
(ii) the composition of two morphisms in S is again an element of S, whenever they are composable;
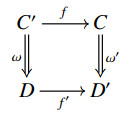
(iii) every diagram Cf⟵C′ω⟹D with ω∈S can be completed to a commutative square
with ω′∈S;
(iv) if for two morphisms f, g in A and ω in S such that f∘ω=g∘ω, then there exists ϑ∈S such that ϑ∘f=ϑ∘g.
Dually, we define calculi of right fractions or right localising classes. A class of morphisms which admits both a left and right calculus of fractions is called a multiplicative system (or a localising class).
Let S be a left localising class of a category A and X,Y∈A. A left fraction (s,b) in A from X to Y is a diagram
with s∈S. Two left fractions (s,b) and (r,a) from X to Y are equivalent, denoted by (s,b)∼(r,a), if there exists a commutative diagram in A
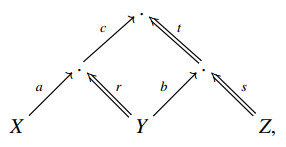
This is an equivalence relation. We denote the equivalence class of (s,b) by s−1b. Let r−1a be an equivalence class of left fractions from X to Y, s−1b be an equivalence class of left fractions from Y to Z. Then their composition is defined as
which can be illustrated by the following diagram:
where the new morphisms t and c are constructed using Definition 2.1 (iii). It is not difficult to see that the definition of compositions does not depend on the choices of t and c.
We define a new category, denoted by [S−1]A, as follows. Its objects are the same as A, the morphisms from one object X to another one Y are the equivalence classes of left fractions from X to Y, and compositions of morphisms are defined above. Moreover, there exists a natural quotient functor
sending X to X and f:X→Y to 1−1Yf. It can be shown that [S−1]A is the localisation of A with respect to S. Similarly, given a right localisation class S in A, one can define the category A[S−1], which is the localisation of A with respect to S.
2.2. Exact sequences, (co)localisation sequences and recollements of abelian categories
Basic references of this subsection are [6,Section 3.6] and [1].
Let A be an abelian category. A full additive subcategory C of A is called a Serre subcategory if for every short exact sequence 0→X→Y→Z→0 in A, the following condition holds: X,Z∈C if and only if Y∈C. It is easy to see that the class of morphisms
is a multiplicative system, where Ker(f) and Coker(f) denote the kernel and the cokernel of f, respectively.
We denote by A/C the localization of A with respect to MC(A) and call it the quotient category of A by C. The quotient category A/C is an abelian category and the quotient functor Q:A→A/C is an exact functor. We denote by i:C→A the inclusion functor.
In this case, we say that
is a short exact sequence of abelian categories.
If we assume that the inclusion functor i:C→A satisfies the condition (★1), then A/C has countable coproducts and the exact functor Q:A→A/C commutes with countable coproducts [7,Lemma A.2.21]. Dually, if the condition (★2) holds for the inclusion functor i:C→A, then A/C has countable products and the exact functor Q:A→A/C commutes with countable products.
Let F:A→B be an exact functor between abelian categories. Then
is a Serre subcategory of A and so F induces an exact functor ¯F:A/Ker(F)→B. This functor ¯F is necessarily faithful, whose simple proof is left to the reader.
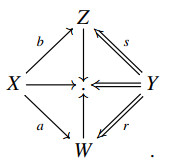
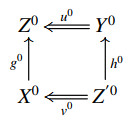
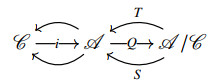
Given a short exact sequence of abelian categories
if Q has a right adjoint S (or equivalently i has right adjoint; cf [6]), the diagram
is called a localisation sequence (or a right recollement) of abelian categories. Obviously, S is left exact. However, it is generally not an exact functor.
Dually, if Q has a left adjoint R (or equivalently i has left adjoint), the diagram
is called a colocalisation sequence (or a left recollement) of abelian categories. Obviously, R is right exact. However, it is generally not an exact functor.
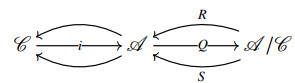
When Q has both a left adjoint R and a right adjoint S (or equivalently so does i), the diagram
will be called a recollement of abelian categories.
There also exists a generalisation of the above notions, say, ladders of abelian categories; see, for instance, [8].
2.3. Exact sequences, (co)localisation sequences and recollements of triangulated categories
Let T be a triangulated category with shift functor Σ. A triangulated subcategory T′ of T is called a thick subcategory if it is closed under taking direct summands; see [2] and [3,Proposition 1.3]. Then the Verdier quotient T/T′ is defined as the localization of T by the multiplicative system
where Cone(f) is the third object appearing in the distinguished triangle
The Verdier quotient T/T′ is still a triangulated category and the quotient functor Q:T→T/T′ is a triangle functor. In this case, let i:T′→T be the inclusion functor. We say that T′i→TQ→T/T′ is a short exact sequence of triangulated categories.
Given a short exact sequence of triangulated categories T′i→TQ→T/T′, if i (or Q) has a left adjoint, then the diagram
is called a colocalisation sequence (or a left recollement) of triangulated categories. Dually, given a short exact sequence of triangulated categories T′i→TQ→T/T′, if i (or Q) has a right adjoint, then the diagram
is called a localisation sequence or a right recollement of triangulated categories.
When i (or Q) has a left adjoint and a right adjoint, the diagram
is called a recollement of triangulated categories.
There exists a generalisation of the above notions, say, ladders of triangulated categories; see [9].
The main goal of this paper is to produce short exact sequences, left recollements, right recollements, recollements of homotopy categories and derived categories from short exact sequences, left recollements, right recollements, recollements of abelian categories, respectively.
3.
Constructing short exact sequences of abelian categories of complexes
In this section, we will show that a short exact sequence of abelian categories gives rise to short exact sequences of various complex categories.
Let A be an abelian category. Recall that for ∗=+,−,b,∅, we denote by C∗(A) the category of left bounded, right bounded, bounded or unbounded complexes, respectively.
Theorem 3.1. A short exact sequence of abelian categories
induces short exact sequences of abelian categories
for ∗∈{+,−,b}.
We split the proof of the above result into several lemmas.
The first lemma shows that it suffices to show that Q∗:C∗(A)→C∗(A/C) is dense and full. This lemma is the result for abelian categories analogous to [4,Lemma 3.1], which itself deals with triangulated categories.
Lemma 3.2. Let F:C→D be an exact functor between abelian categories. Suppose that F is dense and full. Then the induced functor
is an equivalence, where Ker(F):={X∈C | F(X)≃0}.
Proof It is obvious that ¯F is full and dense, so it suffices to show that ¯F is faithful. Given a morphism s−1f in C/Ker(F) presented by a left fraction Xf→Y′s⇐Y. Suppose that it is sent to zero by ¯F.
Then ¯F(s−1f)=F(s)−1F(f)=0 and thus F(f)=0. Since F is exact, F(Im(f))=Im(F(f))=0. Hence, Im(f) belongs to Ker(F). As f can be written as the composition X→Im(f)→Y′, it becomes the zero morphism in C/Ker(F).
Lemma 3.3. [10,Tag 06XL] Given a short exact sequence of abelian categories
then for ∗∈{+,−,b}, the induced functor Q∗:C∗(A)→C∗(A/C) is dense.
Let us remark that the above result and also the following one have been implicit in the proof of [4,Theorem 3.2].
Lemma 3.4. Let C be a Serre subcategory of an abelian category A and let ∗∈{+,−,b}. Then the induced functor Q∗:C∗(A)/C∗(C)→C∗(A/C) is full.
Proof. The result follows obviously from the following statement.
For a chain map f:X∙→Y∙ in C∗(A/C), there exist a complex Z∙ in C∗(A) and chain maps
in C∗(A) such that Q∗(u)∘f=Q∗(g) and that Q∗(u) is an isomorphism in C∗(A/C).
Now we prove this statement. By Lemma 3.3, the functor Q∗:C∗(A)→C∗(A/C) is dense. We can assume that X∙ and Y∙ are complexes in C∗(A).
(1) The left bounded case.
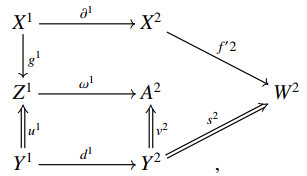
Suppose that f=(fi)i∈Z has the following representation diagram
with si∈MC(A), ∀i∈Z.
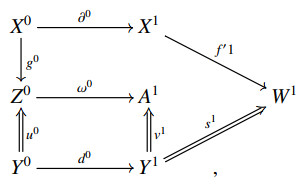
When i<0, let Zi=0. For i=0, let Z0=W0, g0=f′0 and u0=s0. Since s0∈MC(A), by Definition 2.1 (iii), we have the following commutative diagram in A
where v1:Y1→A1 belongs to MC(A).
Since f is a chain map, there exist r1:A1→Z1 and k1:W1→Z1 in MC(A) such that the following diagram commute in A
Let ∂′0=r1∘ω0, g1=k1∘f′1 and u1=k1∘s1. Then we get the following commutative diagram in A:
Thus, we have constructed the object Z1, the maps u1, g1 and the differential ∂′0. Moreover, we have
Since s1∈MC(A), by Definition 2.1 (iii), we have a commutative diagram in A
where v2:Y2→A2 belongs to MC(A). Since f is a chain map, there exist r2:A2→W′2 and k2:W2→W′2 belonging to MC(A) such that the following diagram commutes in A
We set ∂″1=r2∘ω1, g′2=k2∘f′2 and u′2=k2∘s2. Then the following diagram commutes in A
It is easy to see that ∂″1∘∂′0∘u0=u′2∘d1∘d0=0=0∘u0.
Since u0∈MC(A), by Definition 2.1 (iv), there exists a:W′2⇒Z2 in MC(A) such that a∘∂″1∘∂′0=0.
Let u2=a∘u′2,g2=a∘g′2 and ∂′1=a∘∂″1. So ∂′1∘∂′0=0. Thus, we have constructed Z2, u2, g2 and the differential ∂′1. Moreover, we have f2=(u2)−1g2.
Repeating this process, we construct the complex Z∙∈C+(A) and the chain map g:X∙→Z∙, u:Y∙→Z∙ in C+(A) such that Q+(u)∘f=Q+(g) and that Q+(u) is isomorphism in C+(A).
(2) The right bounded case.
In this situation, we use right fractions to construct the complex Z∙ and the chain maps g and u.
(3) The bounded case.
We use left fractions (or right fractions) to construct the complex Z∙ and the chain maps g and u. It is easy to see that we can choose Zn=0 for |n|≫0.
Hence, our statement is proved.
Proof of Theorem 3.1 Let Ker(Q∗) be the kernel of Q∗:C∗(A)→C∗(A/C). It is obvious that Ker(Q∗)=C∗(C). The above three lemmas show that the induced functor C∗(A)/C∗(C)→C∗(A/C) is an equivalence.
Now we consider the unbounded case.
Theorem 3.5. Given a short exact sequence of abelian categories
it induces another short exact sequence of abelian categories of unbounded complexes
By Lemma 3.2, this theorem follows from the two lemmas below.
Lemma 3.6. [10,Tag 06XL] The induced functor Q:C(A)→C(A/C) in Theorem 3.5 is dense.
Lemma 3.7. The induced functor Q:C(A)→C(A/C) in Theorem 3.5 is full.
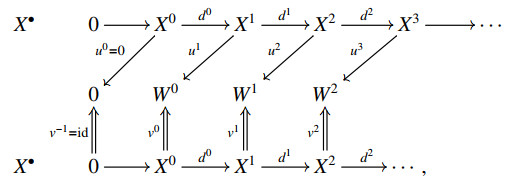
Proof Let f:X∙→Y∙ be a chain map in C(A/C). By Lemma 3.6, we can assume that X∙ and Y∙ are in C(A). By Lemma 3.4, we construct a positive complex (Z∙,∂′∙)∈C+(A) such that the following diagram commute
where Q(ui)−1Q(gi)=fi for all i⩾0.
Dually, by Lemma 3.4, we construct a negative complex (Z∙,d′∙) such that the following diagram commutes
where Q(hi)Q(vi)−1=fi for all i⩽0. An interesting point is that the morphisms v0 and h0 are constructed by using the commuting square
whose existence is guaranteed by (the dual) of Definition 2.1 (iii). This will not influence the proof of Lemma 3.4.
Observe that, by construction, the following two complexes
and
are in C(A).
Thus, we have the following commutative diagram in A
Therefore, we have chain maps f′:M∙→N∙, v:(M∙,∂′∙)→(X∙,∂∙) and u:(Y∙,d∙)→(N∙,d′∙) in C(A). It is easy to see that v and u are isomorphisms in C(A)/C(C). Then Q(u)−1Q(f′)Q(v)−1=f. So the induced functor Q:C(A)/C(C)→C(A/C) is full.
4.
Constructing short exact sequences of homotopy categories
In this section, we show that a short exact sequence of abelian categories gives rise to short exact sequences of homotopy categories.
We begin with an interesting observation which should be well known, but we could not find it in the literature. Let A be an abelian category. Let A∈A and n∈Z. Denote by Dn(A) the elementary contractible complex
which concentrates in degrees n and n+1. Elementary contractible complexes are contractible as shown by the following commutative diagram
Proposition 4.1. A complex X∙ is contractible if and only if X∙ is a direct summand of direct sums of elementary contractible complexes. More precisely, X∙ is a direct summand of ⊕n∈ZDn(Xn).
Proof The if-part is obvious. We now prove the only-if-part. Let (X∙,d∙) be a chain complex. Suppose that it is contractible. Then there exist a family of morphisms {sn:Xn→Xn−1|n∈Z} such that for each n∈Z,
Let Y∙ be be the direct sum of elementary contractible complexes ⊕n∈ZDn(Xn). For each n, we have Yn=Xn⊕Xn−1 and the differential of Y∙ has the form (0010).
For each n, let
Then f∙∘g∙=1X∙. Thus, X∙ is a direct summand of ⊕n∈ZDn(Xn).
Notice that when X∙∈C∗(A) for ∗∈{+,−,b,∅}, we still have ⊕n∈ZDn(Xn)∈C∗(A).
Let C be a Serre subcategory of A. For ∗∈{+,−,b,∅}, we will describe the kernel of Q∗:K∗(A)→K∗(A/C). We say that a complex (X∙,d∙X) in C∗(A) is null homotopic modulo C if there exist chain maps
in C∗(A) and a collection of morphisms h={hi:Zi→Wi−1,i∈Z} in A such that si,vi∈MC(A) and hi∂i+δi−1hi−1=visi for all i∈Z.
Proposition 4.2. Let A be an abelian category and C a Serre subcategory of A. Then a complex X∙ belongs to Ker(Q∗) if and only if X∙ is null homotopic modulo C.
Proof The condition is obviously sufficient, so we only need to show the necessity. We deal with the left, right bounded cases and the unbounded case, respectively.
(1) The left bounded case.
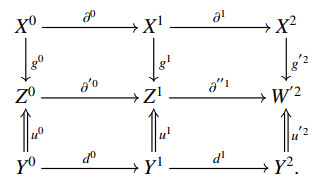
Suppose that a complex (X∙,d∙) lies in Ker(Q+). Without loss of generality, we assume that
Since X∙ is null homotopic in C+(A/C), we have the following representation diagram in A:
where vi,∀i∈Z, is in MA(C) and satisfy Q(di−1)Q(vi−1)−1Q(ui)+Q(vi)−1Q(ui+1)Q(di)=idXi.
Next we will construct a complex (Y∙,∂∙):⋯→0→Y0→Y1→Y2→⋯ together with morphisms si:Xi→Yi−1 and ti:Xi→Yi∈MA(C), ∀i∈Z, such that the following diagram commutes in A
For all i∈Z, we have formulas
When i⩽0, let si=0, ti−1=id0 and ∂i−1=0. For i=0, since Q(v0)−1Q(u1)Q(d0)=idX0, we take Y0=W0 and let s1=u1, t0=v0. Then the desired conditions hold obviously.
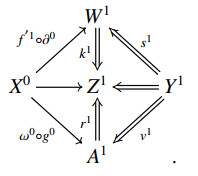
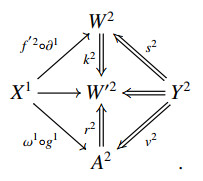
For i=1, by Definition 2.1 (iii), we can obtain two commutative squares
with f0∈MC(A) and
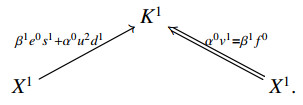
with α0∈MC(A). Therefore, the sum Q(d0)Q(v1)−1Q(u1)+Q(v2)−1Q(u2)Q(d1) can be represented as the following diagram in A
Since we have formula
there exist morphisms b1:K1→Y1 and a1:X1→Y1 in MA(C) such that the following diagram commutes in A
We set s2=b1α0u2,t1=b1α0v1 and ∂0=b1β1e0. Then we have
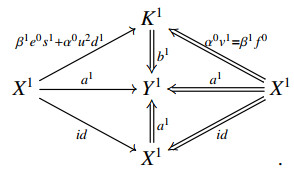
For i=2, by the same construction in the case i=0, we construct the following commutative diagram in A
However, the map σ1∂0 maybe not 0. As σ1∂0t0=ρ2d1d0=0 and t0∈MA(C), by Definition 2.1 (iv), there exists a map w1:K2→Y2 in MA(C) such that w1σ1∂0=0.
We set ∂1=w1σ1, s3=w1j3 and t2=w1ρ2. Then Y2, s3 and t2 satisfy the conditions that we need. Repeating this same process, we construct the desired complex (Y∙,∂∙). Therefore, X∙ is null homotopic modulo C.
(2) The right bounded case.
In this situation, we should use right fractions to construct the right bounded complex (Z∙,δ∙) and maps {ti:Zi+1→Xi|i∈Z⩽0}
such that for each i in Z, the map tiδi+di−1ti−1=ui belongs to MA(C) and uiδi−1=di−1ui−1. Therefore, the complex X∙ is null homotopic modulo C.
(3) The unbounded case.
Suppose (X∙,d∙) is in Ker(Q). We assume that
By the proof of the right and left bounded cases, we can construct the following diagram in A:
such that tiδi+di−1ti−1=ui∈MA(C) for i⩽0 and sjdj+∂−(j+1)s−(j+1)=αj∈MA(C) for j⩾0.
The morphisms u0 and t−1 are constructed by using the commuting square
Its existence is guaranteed by (the dual of) Definition 2.1 (iii).
Observe that the following complexes
and
are in C(A).
Thus, we have the following diagram in C(A)
where u and α are morphisms of chain complexes in MA(C) and {hi:Zi→Wi−1 | i∈Z} are a collection of maps. Moreover, we have
for each i∈Z. Therefore, X∙ is null homotopic modulo C.
Theorem 4.3. Let 0⟶Ci⟶AQ⟶A/C⟶0be an short exact sequence of abelian categories. Then for each ∗∈{+,−,b,∅}, the induced triangle functor Q∗:K∗(A)→K∗(A/C) is fully faithful and we have an induced short exact sequence of triangulated categories
Proof By [4,Lemma 3.1], it suffices to show that the induced functor Q∗:K∗(A)/Ker(Q∗)→K∗(A/C) is full and dense.
The dense property is clear by Lemma 3.6. It is enough to show that
is full. We give the proof for the case ∗=∅, the other cases ∗=+,−,b being similar.
For any morphism f:M∙→N∙∈K(A/C), by the statement in the proof of Lemma 3.7, there exist chain maps
in MC(C)(C(A)) and chain map f′:M∙→N∙in C(A) such that the chain map u−1f′v−1 in C(A)/C(C) is sent to f in C(A/C). Obviously Q:K(A)/Ker(Q∗)→K(A/C) sends its corresponding morphism in K(A)/Ker(Q∗) to f.
5.
An unbounded version of Miyachi's theorem
Let A be an abelian category and C a Serre subcategory of A. For ∗∈{+,−,b,∅}, recall that D∗C(A) denotes the full subcategory of D∗(A) whose objects are complexes X∙ such that the n-th homology Hn(X) belongs to C for all n∈Z.
J. Miyachi showed the following result, which is the starting point of this paper.
Theorem 5.1. [4,Theorem 3.2] Let 0⟶Ci⟶AQ⟶A/C⟶0 be an short exact sequence of abelian categories. Then we have induced short exact sequences of triangulated categories
for ∗∈{+,−,b}.
We would like to generalize Miyachi's result to unbounded derived categories without imposing any other condition.
Theorem 5.2. Let 0⟶Ci⟶AQ⟶A/C⟶0 be a short exact sequence of abelian categories. Then we have an induced short exact sequence of unbounded derived categories
Proof We still denote by Q:D(A)→D(A/C) the derived functor induced by the exact quotient functor Q:A→A/C. Note that Ker(Q)=DC(A).
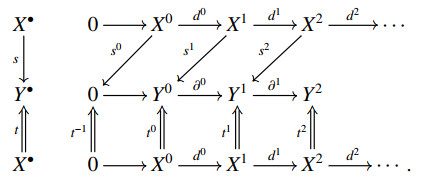
By [4,Lemma 3.1], it suffices to show that the induced functor ¯Q:D(A)/DC(A)→D(A/C) is full and dense.
As in the case of homotopy categories, the dense property is clear by Lemma 3.6. We need to show that ¯Q:D(A)/DC(A)→D(A/C) is full.
Let f:X∙→Y∙ be a morphism in D(A/C). It has a presentation by left fraction
where f′ and t are morphisms in K(A/C) and t is a quasi-isomorphism.
By Theorem 4.3, there exist morphisms s1,s2,t1 in K(A) such that the quotient functor
sends the left fractions s−11f1 and s−12t1 in K(A)/KerQ to f′ and t respectively. Since D(A)/DC(A) is a quotient of K(A), the image of (s−12t1)−1(s−11f1) under the functor ¯Q:D(A)/DC(A)→D(A/C) is t−1f′.
We are done.
Next, we consider the cases when the given short exact sequence of abelian categories is in fact part of a (co)localization sequence, or even a recollement.
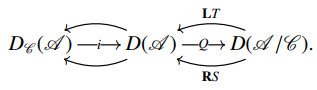
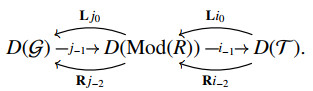
Theorem 5.3. Let
be a localization sequence of abelian categories. Assume that the right total derived functor RS:D(A/C)→D(A) of S exists (for instance, when A/C has enough injectives and satisfies the axiom AB4∗, i.e. it has arbitrary small products which preserve exactness). Then there exists a localization sequence of unbounded derived categories:
Similarly, one can show the following two results.
Theorem 5.4. Let
be a colocalization sequence of abelian categories. Assume that the left total derived functor LT:D(A/C)→D(A) of T exists (for instance, when A/C has enough projectives and satisfies the axiom AB4, i.e. it has arbitrary small coproducts which preserve exactness). Then there exists a colocalisation sequence of unbounded derived categories
Theorem 5.5. Let
be a recollement of abelian categories. Assume that the right total derived functor RS:D(A/C)→D(A) of S and the left total derived functor LT:D(A/C)→D(A) of T exist. Then there exists a recollement of unbounded derived categories
6.
Criterions for the canonical functor D∗(C)→D∗C(A) to be an equivalence
Let C be a Serre subcategory of an abelian category A. It is obvious that the triangle functor D∗(C)→D∗(A) factors through D∗(C)→D∗C(A), which is still denoted by i∗ by abuse of notations. In this section, we consider the question when the natural functor
is an equivalence with ∗∈{+,−,b,∅}.
An interesting observation is that the fullness of the functor i∗ implies its dense property. This has been obtained by D. Yao [5] for bounded derived categories.
Proposition 6.1. [5,Theorem 2.1] Let A be an abelian category and C a Serre subcategory of A. If the canonical functor ib:Db(C)→DbC(A) is full, then it is dense.
Remark that the canonical functor ib:Db(C)→DbC(A) is also faithful in Proposition 6.1. In fact, since i:C→A is fully faithful and exact, ib:Db(C)→DbC(A) takes any non-zero object to non-zero object. By the following lemma, it is faithful as well.
Lemma 6.2. [11,pp.446] Any full triangle functor between triangulated categories is faithful as long as it does not take any non-zero object to zero.
We generalise Yao's result to left bounded/right unbounded/unbounded cases.
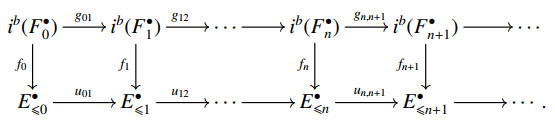
Proposition 6.3 (The left bounded case). Let A be an abelian category and C a Serre subcategory of A. Suppose that the inclusion functor i:C→A satisfies the condition (★1). If the canonical functor i+:D+(C)→D+C(A) is full, then it is faithful and dense, hence an equivalence.
Proof Obviously, the functor ib:Db(C)→DbC(A) is full. By Proposition 6.1, ib:Db(C)→DbC(A) is dense. Let E∙∈D+C(A) and suppose that E∙ can be represented as follows:
Suppose that the condition (★1) holds on the inclusion functor i:C→A. We know that
where
is the right mild truncation of E∙ at the n-th place together with canonical morphisms un,n+1:E∙⩽n→E∙⩽n+1.
Since E∙⩽n belongs to DbC(A), then there exists a complex F∙n∈Db(C) together with an isomorphism fn:ib(F∙n)→E∙⩽n in DbC(A). Define gn,n+1=f−1n+1∘un,n+1∘fn in DbC(A) for each n∈Z⩾0 and we have the following commutative diagram in D+C(A):
Since i+:D+(C)→D+C(A) is full, there exists hn,n+1:F∙n→F∙n+1 in D+(C) such that i+(hn,n+1)=gn,n+1 for each n≥0.
By our assumption, the subcategory C has countable direct sums. One can form the homotopy colimit
where the restriction of h to F∙i is id−hi,i+1.
The condition (★1) implies that i+ commutes with countable direct sums. So we have the following morphism of distinguished triangles in D+C(A)
Since countable direct sums of quasi-isomorphisms are still quasi-isomorphisms, θ is an isomorphism in D+C(A). We are done.
By using a similar method, we have the following results.
Proposition 6.4 (The right bounded case). Let A be an abelian category and C a Serre subcategory of A. Suppose that the inclusion functor i:C→A satisfies the condition (★2). If the canonical functor i−:D−(C)→D−C(A) is full, then it is faithful and dense, hence an equivalence.
Proposition 6.5 (The unbounded case). Let A be an abelian category and C a Serre subcategory of A. Suppose that the inclusion functor i:C→A satisfies the condition the conditions (★1) and (★2). If the canonical functor i:D(C)→DC(A) is full, then it is faithful and dense, hence an equivalence.
Now we consider the inverse problem whether the dense property implies that i∗ is fully faithful. We could only show that this holds under slightly stronger property. We need a well known criterion.
Proposition 6.6. [12,Proposition 1.6.5] Let C be a category and C′ a full subcategory of C. Let S be a multiplicative system in C, and let S′ be the family of morphisms of C′ which belong to S. Assume that one of the following conditions holds:
(1) whenever f:X→Y is a morphism in S, with Y∈Ob(C′), there exists g:W→X, with W∈Ob(C′) and f∘g∈S,
(2) the same as (1) with the arrows reversed.
Then the localisation C′[S′−1] is a full subcategory of C[S−1].
Proposition 6.7. Let A be an abelian category and C a Serre subcategory of A. Suppose that the canonical functor i∗:D∗(C)→D∗C(A) is dense "with fixed direction", i.e. one of the following conditions holds:
(1) for any X∙∈D∗C(A), there exists a quasi-isomorphism X∙→Y∙ with Y∙∈D∗(C);
(2) for any X∙∈D∗C(A), there exists a quasi-isomorphism Y∙→X∙ with Y∙∈D∗(C).
Then i∗ is fully faithful, hence an equivalence.
Proof This follows immediately from Proposition 6.6.
Recall that a full abelian subcategory C of an abelian category A is thick if it is closed under extensions. The next result is another criterion, which is in fact a special case of [12,Proposition 1.7.11].
Proposition 6.8. Let A be an abelian category with enough injective objects and C a thick subcategory of A. Suppose that each object C∈C can be embedded into an object I of C which is an injective object of A. Then the natural functor i∗:D∗(C)→D∗C(A) is an equivalence for ∗=+,b. This is also true for ∗=∅, if we suppose that the inclusion functor i:C→A satisfies the condition (★1) or (★2).
Proof The cases for ∗=+,b are exactly [13,Proposition 2.42]. In fact, by [12,Proposition 1.7.11], for each complex X∙∈D+C(A), one can find a quasi-isomorphism X∙→Y∙ with Y∙∈D+(C).
For the unbounded case, let X∙ be a complex in DC(A). Under the condition (★1) we realise X∙ as a direct limit of brutal truncations and then use the homotopy colimits, or under the condition (★2) realise X∙ as an inverse limit of mild truncations and use homotopy limits. The details are left to the reader.
Similarly, the dual statement of the above proposition is also true.
Proposition 6.9. Let A be an abelian category with enough projective objects and C a thick subcategory of A. Suppose that any C in C is a quotient of an object A in C which is projective as an object of A. Then the natural functor i∗:D∗(C)→D∗C(A) is an equivalence for ∗=−,b. This is also true for ∗=∅, if we suppose that the inclusion functor i:C→A satisfies the condition (★1) or (★2).
7.
Examples
In this section, we will present some examples, most of which come from [17] and [8].
Example 7.1. [14,pp.205] Let A be an abelian category. Recall that an additive contravariant functor F from A to the category of abelian groups Ab is finitely presented if it fits into an exact sequence
for some morphism Xf→Y in A. Denote by mod(A) the category of all finitely presented contravariant functors from A to Ab.
Introduce a functor ω:mod(A)→A by imposing
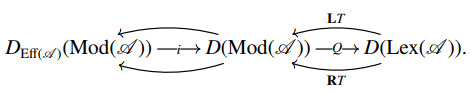
for a finitely presented contravariant functor F:Aop→Ab with a presentation (7.1). The functor ω is exact and is left adjoint to the Yoneda functor Y:A→mod(A). Moreover there exists a localization sequence of abelian categories
with eff(A):=Ker(ω).
By Theorems 5.1 and 5.2, we obtain the following exact sequences of triangulated categories
for ∗=b,−,+,∅.
It would be very interesting to determine when i∗:D∗(eff(A))→D∗eff(A)(mod(A)) is an equivalence.
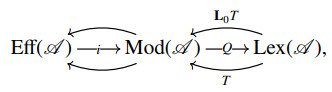
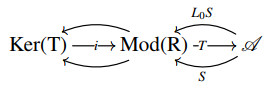
Example 7.2. Let A be a small abelian category. Denote by Mod(A)=Add(Aop,Ab) the category of all additive contravariant functor from A to Ab and by Lex(A) the full subcategory of Mod(A) consisting of all left exact functors. By [15,Theorem 2.3], there exists a localisation sequence of abelian categories
where i is the inclusion functor and Eff(A):=Ker(Q) which can be seen as the ind-completion of eff(A) [16].
It is easy to see that the adjunction pair (Q,T) satisfies the conditions in [17,Theorem 10]. Then the above localisation sequence can be extended to be a recollement of abelian categories
where L0T is the 0-th left derived functor of T.
By Theorem 5.1, we obtain the following exact sequence of derived categories
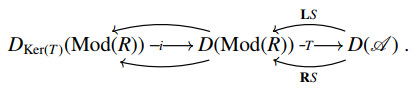
Since A is small, Lex(A) is a Grothendieck category [16,Theorem 8.6.5]. Hence, by Theorem 5.3, 5.4 and 5.5, we have the following right recollement, left recollement and recollement of triangulated categories:
and
It would be very interesting to determine when i∗:D∗(Eff(C))→D∗Eff(C)(Mod(C)) is an equivalence.
Example 7.3. [17,Section 4.7] Let C be a finitely accessible additive category. We denote by fpC the full subcategory of C consisting of all finitely presented objects and recall that mod(C) denotes the category of finitely presented functors as in Example 7.1. The category mod(C) is an abelian category with all small colimits. Any additive functor G∈Mod(fpC) can be extended to a unique functor ←G:Cop→Ab which preserves inverse limits. Therefore, there is a functor
which is right adjoint to the restriction functor
By [17,Corollary 20], we have in fact a recollement of abelian categories
where S:=←− and L0S is the 0-th left derived functor of S.
By Theorems 5.1, we have the following exact sequence of derived categories
Since Mod(fpC) has enough projectives and enough injectives, by Theorems 5.3, 5.4 and 5.5, we obtain the following right recollement, left recollement and recollement of triangulated categories:
It would be very interesting to determine when i∗:D∗(Ker(R))→D∗Ker(R)(mod(C)) is an equivalence.
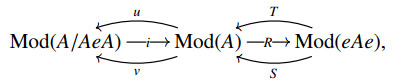
Example 7.4. Let A be an associative ring with unit and e∈A an idempotent. Then there exists a recollement of abelian categories
where i is the inclusion, u=−⊗AA/AeA, v=HomA(A/AeA,−), R=HomA(eA,−), T=−⊗eAeeA, S=HomeAe(Ae,−).
By Theorem 5.1, we have an exact sequence of triangulated categories
By Theorems 5.3, 5.4 and 5.5, we obtain the following right recollement, left recollement and recollement of triangulated categories:
and
The natural functor i∗:D∗(Ker(R))→D∗Ker(R)(Mod(A)) is an equivalence if and only if the surjection A→A/AeA is a homological epimorphism; see [18].
Example 7.5. Let A be a right Noetherian ring. Denote by Mod(A) the category of all A-modules and by mod(A) the full subcategory of finitely generated modules. Then mod(A) is a Serre subcategory of Mod(A). We have an exact sequence of abelian categories
where i and Q are the canonical inclusion functor and the quotient functor respectively. Notice that by [8,Section 4.2], this short exact sequence can NOT be extended to a left or right recollement.
By [16,Theorem 15.3.1], we know that
are equivalences. Thus, by Theorem 5.1 and 5.2, we have the following exact sequences of triangulated categories:
for ∗=b,−,
and
It would be very interesting to see when i+:D+(mod(A))→D+mod(A)(Mod(A)) and i:D(mod(A))→Dmod(A)(Mod(A)) are equivalences; for recent progress, see [19].
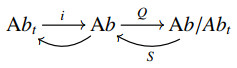
Example 7.6. Let Abt be the category of all torsion abelian groups and Abf the category of torsion-free abelian groups. Then (Abt,Abf) is a torsion pair in Ab and Abt is a Serre subcategory of Ab. We get a right recollement of abelian categories
which can not be extended further; see [8,Section 4.3].
By Theorem 5.1, we have the following short exact sequences of triangulated categories
for ∗=b,−.
It is easy to see that the quotient category Ab/Abt has enough injectives. By Theorem 5.3, we obtain the following right recollements of triangulated categories:
It would be very interesting to see whether i∗:D∗(Abt)→D∗Abt(Z) is an equivalence.
Example 7.7. Let us recall the Gabriel-Popescu Theorem; see for example [20]. Let A be a Grothendieck category, G a generator of A and R the ring of all endomorphisms of G. Let S be the functor from A to Mod(R) defined by S(X)=HomA(G,X). Then S is fully faithful. Moreover, S has an exact left adjoint functor T, i.e. we have the following right recollement of abelian categories
By Theorem 5.1, we obtain the following exact sequences of derived categories
for ∗=−,b.
Since A is a Grothendieck category which has enough injectives, by Theorems 5.3, we have the following right recollement of triangulated categories
with ∗=+,∅.
We can strengthen the Gabriel-Popescu Theorem as follows.
Claim: Suppose that A has enough projectives. Assume further that G is self-small, that is, for each set X, we have a natural bijection
Then there exists a recollement of abelian categories
and a recollement of triangulated categories
The proof of this claim follows easily from [17,Theorem 10] and Theorem 5.5, which is left to the reader. It would be very interesting to see when i∗:D∗(Ker(T))→D∗Ker(T)(Mod(R)) is an equivalence.
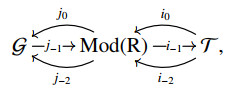
Example 7.8. Let K be a field, R=∏ i=1Ki and I=⊕∞i=1Ki with each Ki=K. Then R is a commutative ring and I is an idempotent ideal of R. We set
and
By [8,Example 4.3], we get the following recollement of abelian categories
where i0 is the fully faithful inclusion, j0=−⊗RR/I, j−1=HomR/I(R/I,−)≅R/I⊗R/I−, j−2=HomR(R/I,−), respectively.
Notice that for each ∗∈{+,−,b,∅}, the functor j∗−1:D∗(G)→D∗(ModR) is fully faithful, and that j−1:G→Mod(R) satisfies the the conditions (★1) and (★2).
Hence, by Propositions 6.1, 6.3, 6.4 and 6.5, we have triangle equivalences
for ∗∈{+,−,b,∅}.
By Theorem 5.1, we obtain an exact sequence of derived categories
By Theorems 5.3, 5.4 and 5.5, we have the following right recollement, left recollement and recollement of derived categories:
and
Example 7.9. Let R and S be rings, M an S-R-bimodule, and Λ=(R0MS) the triangular matrix ring. A right Λ-module is identified with a triple (X,Y)f, where X is a right R-module, Y a right S-module, and f:Y⊗SM→X a right R-map. In case of no confusion, we write (X,Y) instead of (X,Y)f. A left Λ-module is identified with a triple (UV)g, where U is a left R-module, V a left S-module, and g:M⊗RU→V a left S-map. In case of no confusion, we write (UV) instead of (UV)g.
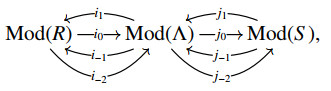
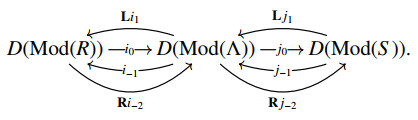
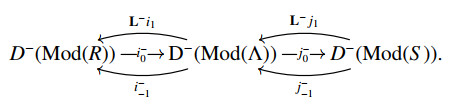
Let e1=(1000) and e2=(0001). By [8,Section 4.5], there is a ladder of abelian categories
where
For each ∗∈{+,−,b,∅}, the functors i∗0:D∗(Mod(R))→D∗(Mod(Λ)) and j∗−1:D∗(Mod(S))→D∗(Mod(Λ)) are fully faithful.
Notice that i0:Mod(R)→Mod(Λ) and j−1:Mod(S)→Mod(Λ) satisfy the conditions (★1) and (★2). Thus, by Propositions 6.1, 6.3, 6.4 and 6.5, we have triangle equivalences
for ∗∈{+,−,b,∅}.
By Theorem 5.1, we obtain an exact sequence of derived categories
By Theorem 5.5, we have the following ladder of derived categories
Similarly, we obtain the following recollements of derived categories:
Acknowledgments
While preparing this paper, the authors were supported by NSFC (No. 12071137) and by STCSM (No. 18dz2271000).
The authors would like to express sincere gratitude to the referees for their careful reading of this manuscript and for their many useful comments which lead to an essential improvement of the presentation of this paper.
Conflict of interest
The authors declare there is no conflicts of interest.









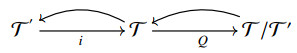
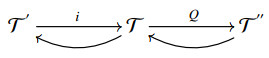
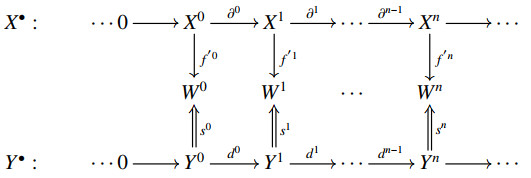


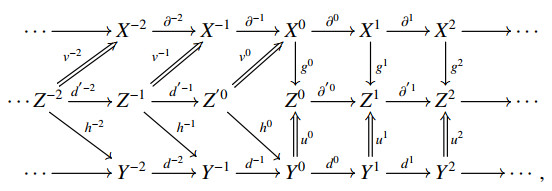

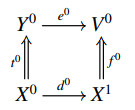


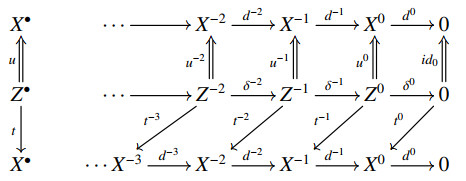
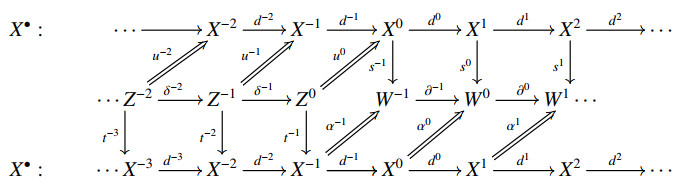
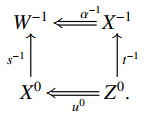


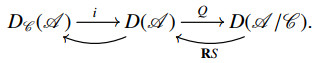
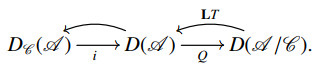

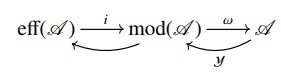
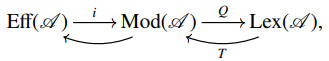
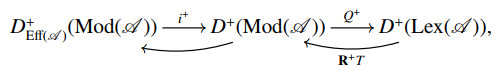
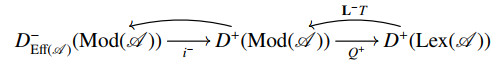
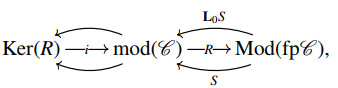
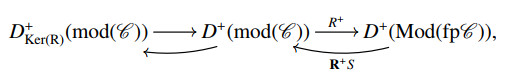
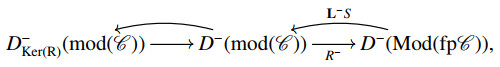
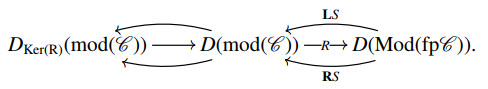
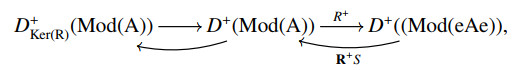


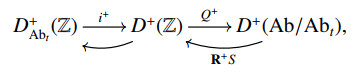
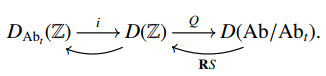
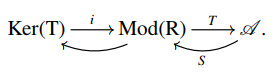
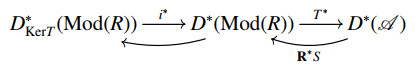
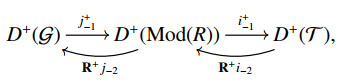

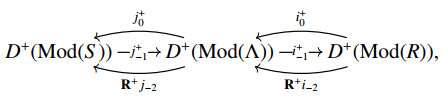



 DownLoad:
DownLoad: