In this paper, we define and study quasi-monoidal comonads on a monoidal category. It generalize the (Hom type) coquasi-bialgebras to a non-braided setting. We investigate their corepresentations and their coquasitriangular structures. We also discuss their gauge equivalence relations.
Citation: Dingguo Wang, Xiaohui Zhang. On quasi-monoidal comonads and their corepresentations[J]. Electronic Research Archive, 2022, 30(8): 3153-3171. doi: 10.3934/era.2022160
| [1] | Shengxiang Wang, Xiaohui Zhang, Shuangjian Guo . The Hom-Long dimodule category and nonlinear equations. Electronic Research Archive, 2022, 30(1): 362-381. doi: 10.3934/era.2022019 |
| [2] | Yongjie Wang, Nan Gao . Some properties for almost cellular algebras. Electronic Research Archive, 2021, 29(1): 1681-1689. doi: 10.3934/era.2020086 |
| [3] | Xing Zhang, Xiaoyu Jiang, Zhaolin Jiang, Heejung Byun . Algorithms for solving a class of real quasi-symmetric Toeplitz linear systems and its applications. Electronic Research Archive, 2023, 31(4): 1966-1981. doi: 10.3934/era.2023101 |
| [4] | Shuguan Ji, Yanshuo Li . Quasi-periodic solutions for the incompressible Navier-Stokes equations with nonlocal diffusion. Electronic Research Archive, 2023, 31(12): 7182-7194. doi: 10.3934/era.2023363 |
| [5] | Natália Bebiano, João da Providência, Wei-Ru Xu . Approximations for the von Neumann and Rényi entropies of graphs with circulant type Laplacians. Electronic Research Archive, 2022, 30(5): 1864-1880. doi: 10.3934/era.2022094 |
| [6] | Jincheng Shi, Shuman Li, Cuntao Xiao, Yan Liu . Spatial behavior for the quasi-static heat conduction within the second gradient of type Ⅲ. Electronic Research Archive, 2024, 32(11): 6235-6257. doi: 10.3934/era.2024290 |
| [7] | Wenjie Zuo, Mingguang Shao . Stationary distribution, extinction and density function for a stochastic HIV model with a Hill-type infection rate and distributed delay. Electronic Research Archive, 2022, 30(11): 4066-4085. doi: 10.3934/era.2022206 |
| [8] | Jianxing Du, Xifeng Su . On the existence of solutions for the Frenkel-Kontorova models on quasi-crystals. Electronic Research Archive, 2021, 29(6): 4177-4198. doi: 10.3934/era.2021078 |
| [9] | Xuerong Hu, Yuxiang Han, Junyan Lu, Linxiang Wang . Modeling and experimental investigation of quasi-zero stiffness vibration isolator using shape memory alloy springs. Electronic Research Archive, 2025, 33(2): 768-790. doi: 10.3934/era.2025035 |
| [10] | Zayd Hajjej . Asymptotic stability for solutions of a coupled system of quasi-linear viscoelastic Kirchhoff plate equations. Electronic Research Archive, 2023, 31(6): 3471-3494. doi: 10.3934/era.2023176 |
In this paper, we define and study quasi-monoidal comonads on a monoidal category. It generalize the (Hom type) coquasi-bialgebras to a non-braided setting. We investigate their corepresentations and their coquasitriangular structures. We also discuss their gauge equivalence relations.
The theory of (co)monads can be used as a tool in various fields of mathematics such as algebra, logic or operational semantics, and theoretical computer science. Note that in algebra theory, there are two different "bimonads". On the one hand, bimonads and Hopf monads without monoidal structures were introduced in [1], and developed in [2,3,4]. On the other hand, bimonads on monoidal categories were introduced in [5]. In 2002, Moerdijk used an opmonoidal monad to define a bimonad. This bimonad F is both a monad and an opmonoidal functor satisfying the multiplication and the unit of F are all monoidal natural transformations (see [5] for details). Although Moerdijk called his bimonad "Hopf monad", the antipode was not involved in his definition. In 2007, A. Bruguières and A. Virelizier introduced the notion of Hopf monad with antipode in the rigid categories in [6], and then put it in the non-dual monoidal categories [7]. We refer to [7,8,9,10,11] for the recent research on A. Bruguières and A. Virelizier's bimonads.
Quasi-bialgebras were introduced by V. G. Drinfel'd in [12]. The dual definition, a k-coquasi-bialgebra H (or a Majid algebra), was introduced by S. Majid in [13]. The associativity of the multiplication are replaced by a weaker property, called coquasi-associativity. The multiplication is associative up to conjugation by a convolution invertible linear form ω∈(H⊗H⊗H)∗, called the coassociator. Note that the definition of a coquasi-bialgebra is not selfdual, and the category of (left or right) comodules over a coquasi-bialgebra is a monoidal category with nontrivial associativity constraint and nontrivial unit constraints. Coquasi-bialgebras in a braided monoidal category also have been studied in [14].
Taking into account the results proved A. Bruguières and A. Virelizier in [6], it is now very natural to ask how to extend coquasi-bialgebras to the non-braided setting. This is the main motivation of the present paper.
In this paper, we present a dual version of the second author's results about quasi-bimonads which appeared in [15]. We mainly provide a generalization of coquasi-bialgebras by introducing the notion of quasi-monoidal comonad. Actually, a quasi-monoidal comonad F is both a comonad and a quasi-monoidal functor such that its corepresentations is a non-strict monoidal category. The notion of quasi-monoidal comonad is very general. For example, the tensor functor of a (Hom-type) coquasi-bialgebras and bicomonads are all special cases of quasi-monoidal comonads.
The paper is organized as follows. In Section 2 we recall some notions of comonads, quasi-monoidal functors, π-categories and so on. In Section 3, we introduce the definition of quasi-monoidal comonads and discuss their corepresentations. In Section 4, we mainly investigate the coquasitriangular structures of a quasi-monoidal comonad. At last, we introduce the gauge equivalent relation on quasi-monoidal comonads.
Throughout the paper, we let k be a fixed field and char(k)=0 and Veck be the category of finite dimensional k-spaces. All the algebras and coalgebras, modules and comodules are supposed to be in Veck. For the comultiplication Δ of a k-space C, we use the Sweedler-Heyneman's notation: Δ(c)=∑c1⊗c2 for any c∈C.
Let (C,⊗,I,a,l,r) and (C′,⊗′,I′,a′,l′,r′) be two monoidal categories. Recall that a quasi-monoidal functor from C to C′ is a triple (F,F2,F0), where F:C→C′ is a functor, F2:F⊗′F→F⊗ is a natural transformation, and F0:I′→FI is a morphism in C′.
Furthermore, if the following equations hold for any X,Y,Z∈C:
| F2(X,Y⊗Z)∘(idFX⊗′F2(Y,Z))∘a′FX,FY,FZ=F(aX,Y,Z)∘F2(X⊗Y,Z)∘(F2(X,Y)⊗′idFZ), | (2.1) |
| F(lX)∘F2(I,X)∘(F0⊗′idFX)=l′FX, | (2.2) |
| F(rX)∘F2(X,I)∘(idFX⊗′F0)=r′FX, | (2.3) |
then F=(F,F2,F0) is called a monoidal functor.
Let C be a category, F: C→C be a functor. Recall from [16] or [17] that if there exist natural transformations δ: F→FF and ε: F→idC, such that the following identities hold
| Fδ∘δ=δF∘δ,andidF=Fε∘δ=εF∘δ, |
then we call the triple (F,δ,ε) a comonad on C.
Let X∈C, and (F,δ,ε) a comonad on C. If there exists a morphism ρX: X→FX, satisfying
| FρX∘ρX=δX∘ρX,andεX∘ρX=idX, |
then we call the couple (X,ρX) an F-comodule.
A morphism between F-comodules g: X→X′ is called F-colinear, if g satisfies: Fg∘ρX=ρX′∘g. The category of F-comodules is denoted by CF.
Let (C,⊗,I,a,l,r) be a monoidal category, (F,δ,ε) be a comonad on C, and (F,F2,F0):C→C be a monoidal functor. Then recall from [18] or [19] that F is called a monoidal comonad (or a bicomonad) on C if δ and ε are both monoidal natural transformations, i.e. the following compatibility conditions hold for any X,Y∈C:
{(C1)F(F2(X,Y))∘F2(FX,FY)∘(δX⊗δY)=δX⊗Y∘F2(X,Y),(C2)εX⊗Y∘F2(X,Y)=εX⊗εY,(C3)F(F0)∘F0=δI∘F0,(C4)εI∘F0=idI.
Given a category C and a positive integer n, we denote Cn=C×C×⋯×C the n-tuple cartesian product of C. If F is a comonad on C, then F×n (the n-tuple cartesian product of F) is a comonad on Cn, and we have CnF×n=(CF)n.
Assume that U:CF→C is the forgetful functor and P,Q:Cn→D are functors. Then from [[9], Proposition 4.1], we have the following results.
Lemma 2.1. There is a canonical bijection:
| Nat(PU×n,QU×n)≅Nat(PF×n,Q). |
Proof. Define ?♭:Nat(PU×n,QU×n)→Nat(PF×n,Q), f↦f♭, by
| f♭(X1,⋯,Xn):P(FX1×⋯×FXn)f(FX1,⋯,FXn)→Q(FX1×⋯×FXn)Q(εX1,⋯,εXn)→Q(X1×⋯×Xn), |
and ?♯:Nat(PF×n,Q)→Nat(PU×n,QU×n), α↦α♯, by
| α♯(M1,⋯,Mn):P(M1×⋯×Mn)P(ρM1,⋯,ρMn)→P(FM1×⋯×FMn)α(M1,⋯,Mn)→Q(M1×⋯×Mn), |
for any f∈Nat(PU×n,QU×n), α∈Nat(PF×n,Q) and Xi∈C, (Mi,ρMi)∈CF. It is easy to check that ?♭ and ?♯ are well defined and are inverse with each other.
Let P,Q,R:Cn→D be functors. For any α∈Nat(PF×n,Q) and β∈Nat(QF×n,R), define their convolution product β∗α∈Nat(PF×n,R) by setting, for any objects X1,⋯,Xn in C,
| β∗αX1,⋯,Xn=βX1,⋯,Xn∘αFX1,⋯,FXn∘P(δX1,⋯,δXn). |
We say that α∈Nat(PF×n,Q) is ∗-invertible if there exists β∈Nat(QF×n,P) such that β∗α=P(ε×n)∈Nat(PF×n,P) and α∗β=Q(ε×n)∈Nat(QF×n,Q). We denote β by α∗−1.
Proposition 2.2. The ∗-invertible elements in Nat(PF×n,Q) are in corresponding with the natural isomorphisms in Nat(PU×n,QU×n).
Proof. Suppose that f∈Nat(PU×n,QU×n) is a natural isomorphism. Then we immediately get that (f♭)∗−1=(f−1)♭.
Conversely, if α∈Nat(PF×n,Q) is ∗-invertible, then α♯−1=(α∗−1)♯.
Suppose that (C,⊗,I,a,l,r) is a monoidal category, F:C→C is a functor, (F,δ,ε) is a comonad and (F,F2,F0) is a quasi-monoidal functor.
Lemma 3.1. If we define the F-coaction on I by F0, anddefine the F-coaction on M⊗N (as the tensor product in C) for any (M,ρM),(N,ρN)∈CF by:
| ρM⊗N:M⊗NρM⊗ρN→FM⊗FNF2(M,N)→F(M⊗N), |
then (I,F0) and (M⊗N,ρM⊗N) are all objects in CF if and only ifthe compatibility conditions Eqs (C1)–(C4) hold.
Proof. It is straightforward to check that Eqs (C1) and (C2) hold if and only if (M⊗N,ρM⊗N)∈CF, Eqs (C3) and (C4) hold if and only if (I,F0)∈CF.
From now on, we always assume that the compatibility conditions Eqs (C1)–(C4) hold.
We suppose that there are natural transformations ϑ:(_⊗_)⊗_∘F×3⇒_⊗(_⊗_):C×3→C, and ι:I⊗F_⇒_:C→C, κ:F_⊗I⇒_:C→C. From Lemma 2.1, for any objects (M,ρM),(N,ρN),(P,ρP)∈CF, ϑ,ι,κ can induce the following natural transformations
| AM,N,P=ϑ♯M,N,P,LM=ι♯M,RM=κ♯M. |
Conversely, if there are natural transformations A:(_⊗_)⊗_⇒_⊗(_⊗_):C×3→C and L:I⊗_⇒id:C→C, R:_⊗I⇒id:C→C, then from Lemma 2.1, for any X,Y,Z∈C, they can induce natural transformations
| ϑX,Y,Z=A♭X,Y,Z,ιX=L♭X,κX=R♭X. |
Next, we will discuss when A is the associativity constraint and L,R are the unit constraints in CF.
Lemma 3.2. A, L and R are isomorphisms if and only if ϑ, ι and κ are ∗-invertible.
Proof. Straightforward from Proposition 2.2.
Lemma 3.3. A is F-colinear if and only if ϑ satisfies
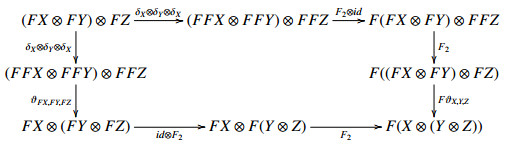
|
(3.1) |
for any X,Y,Z∈C.
Proof. ⇐): Since the following diagram
|
is commutative for any M,N,P∈CF, AM,N,P is F-colinear.
⇒): Notice that AFX,FY,FZ is F-colinear for any X,Y,Z∈C, then it follows
| F(εX⊗εY⊗εZ)∘FAFX,FY,FZ∘ρ(FX⊗FY)⊗FZ=F(εX⊗εY⊗εZ)∘ρFX⊗(FY⊗FZ)∘AFX,FY,FZ. |
After a direct computation, we obtain (3.1).
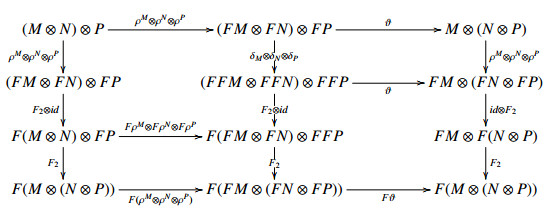
Lemma 3.4. A satisfies the Pentagon Axiom in CF if and only if ϑ satisfies
| (id⊗ϑX,Y,Z)∘ϑW,FX⊗FY,FZ∘(id⊗F2⊗id)∘(ϑFW,FFX,FFY⊗id)∘(δW⊗δ2X⊗δ2Y⊗δZ)=ϑW,X,Y⊗Z∘(id⊗id⊗F2)∘ϑFW⊗FX,FY,FZ∘(F2⊗id⊗id)∘(δW⊗δX⊗δY⊗δZ) | (3.2) |
for any W,X,Y,Z∈C.
Proof. ⇐): Since we have
| (id⊗ϑN,P,Q)∘(id⊗ρN⊗ρP⊗ρQ)∘ϑM,N⊗P,Q∘(id⊗F2⊗id)∘(ρM⊗ρN⊗ρP⊗ρQ)∘(ϑM,N,P⊗id)∘(ρM⊗ρN⊗ρP⊗id)=(id⊗ϑN,P,Q)∘ϑM,FN⊗FP,FQ∘(id⊗F(ρN⊗ρP)⊗ρQ)∘(id⊗F2⊗id)∘(ϑFM,FN,FP⊗id)∘(FρM⊗FρN⊗FρP⊗ρQ)∘(ρM⊗ρN⊗ρP⊗id)=(id⊗ϑN,P,Q)∘ϑM,FN⊗FP,FQ∘(id⊗F2⊗id)∘(ϑFM,FFN,FFP⊗id)∘(δM⊗δ2N⊗δ2P⊗δQ)∘(ρM⊗ρN⊗ρP⊗ρQ)=ϑM,N,P⊗Q∘(id⊗id⊗F2)∘ϑFM⊗FN,FP,FQ∘(F2⊗id⊗id)∘(δM⊗δN⊗δP⊗δQ)∘(ρM⊗ρN⊗ρP⊗ρQ)=ϑM,N,P⊗Q∘(id⊗id⊗F2)∘(ρM⊗ρN⊗ρP⊗ρQ)∘ϑM⊗N,P,Q∘(F2⊗id⊗id)∘(ρM⊗ρN⊗ρP⊗ρQ) |
for any M,N,P,Q∈CF, A satisfies the Pentagon Axiom.
⇒): For any W,X,Y,Z∈C, we have cofree F-comodules FW,FX,FY,FZ. Consider the following Pentagon Axiom:
| AFW,FX,FY⊗FZ∘AFW⊗FX,FY,FZ=(id⊗AFX,FY,FZ)∘AFW,FX⊗FY,FZ∘(AFW,FX,FY⊗id). |
Applying εW⊗εX⊗εY⊗εZ to both sides of the above identity, we get Diagram (3.2).
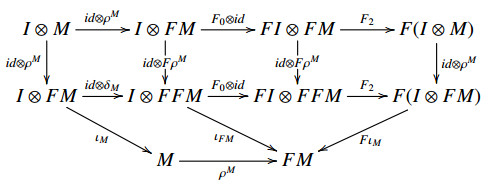
Lemma 3.5. For any X∈C,
(1) L is F-colinear if and only if ι satisfies
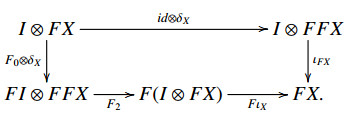
|
(3.3) |
(2) R is F-colinear if and only if κ satisfies

|
(3.4) |
Proof. We only prove (1).
⇐): From the following commutative diagram
|
for any M∈CF, LM is F-colinear.
⇒): Conversely, since FX is an F-comodule and LFX is F-colinear for any X∈C, it is directly to get Diagram (3.3).
Lemma 3.6. A, L and R satisfy the Triangle Axiom in CF if and only if ϑ, ι and κ satisfy
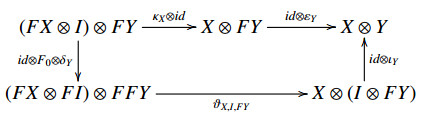
|
(3.5) |
for any X,Y,Z∈C.
Proof. ⇐): For any M,N∈CF, we compute
| (idM⊗ιN)∘(idM⊗idI⊗ρN)∘ϑM,I,N∘(ρM⊗F0⊗ρN)=(idM⊗ιN)∘ϑM,I,FN∘(idFM⊗F0⊗δN)∘(ρM⊗idI⊗ρN)=(idM⊗εN)∘(κM⊗idFN)∘(ρM⊗idI⊗ρN)=(κM⊗idN)∘(ρM⊗idI⊗idN) |
thus the Triangle Axiom in CF holds.
⇒): Conversely, for any X,Y∈C, since we have
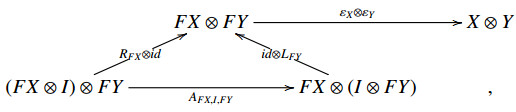
|
it is a direct computation to get Diagram (3.5).
Definition 3.7. Let (C,⊗,I,a,l,r) be a monoidal category on which (F,δ,ε) is a monad and (F,F2,F0) is a quasi-monoidal functor such that the compatible conditions Eqs (C1)–(C4) are satisfied. If there are ∗-invertible natural transformations ϑ, ι and κ satisfying (3.1)–(3.5), then we call (F,δ,ε,F2,F0,ϑ,ι,κ) a quasi-monoidal comonad on C,
Then by Lemma 3.1–3.6, one gets the following result.
Theorem 3.8. Let (C,⊗,I,a,l,r) be a monoidal category on which (F,δ,ε) is a monad and (F,F2,F0) is a quasi-monoidal functor such that the compatible conditions Eqs (C1)–(C4) is satisfied. Then there exist natural transformations ϑ, ι and κ such that (F,δ,ε,F2,F0,ϑ,ι,κ) is a quasi-monoidal comonad if and only if there are natural transformations A, L and R such that (CF,⊗,I,A,L,R) is a monoidal category.
Example 3.9. Let (C,⊗,I,a,l,r) be a monoidal category on which (F,δ,ε) is a monad and (F,F2,F0) is a quasi-monoidal functor such that the compatible conditions Eqs (C1)–(C4) are satisfied. If we define
| ϑX,Y,Z=a♭X,Y,Z,ιX=l♭X,κX,Y,Z=r♭X,Y,Z |
for any X,Y,Z∈C, then Eq (3.2) holds because of the Pentagon Axiom of a; Eq (3.5) holds because of the Triangle Axiom of a,l,r; Eqs (3.1), (3.3) and (3.4) hold if and only if (F,F2,F0) is a monoidal functor. That means, the quasi-monoidal comonad (F,δ,ε,F2,F0,ϑ,ι,κ) is exactly a monoidal comonad.
Example 3.10. Recall from [9] or [10], we consider the following monoidal category ¯Hi,j(Veck) for any i,j∈Z:
∙ the objects of ¯Hi,j(Veck) are pairs (X,αX), where X∈Veck and αX∈Autk(X);
∙ the morphism f:(X,αX)→(Y,αY) in ¯Hi,j(Veck) is a k-linear map from X to Y such that αY∘f=f∘αX;
∙ the monoidal structure is given by
| (X,αX)⊗(Y,αY)=(X⊗Y,αX⊗αY), |
and the unit is (k,idk);
∙ the associativity constraint a, the unit constraints l and r are given by
| aX,Y,Z:(x⊗y)⊗z↦αi+1X(x)⊗(y⊗α−j−1Z(z));lX(1k⊗x)=αj+1X(x),rX(x⊗1k)=αi+1X(x),∀X∈Veck. |
Now assume that (H,αH) is an object in ¯Hi,j(Veck), mH:H⊗H→H (with notation mH(a⊗b)=ab), ηH:k→H (with notation ηH(1k)=1H), and ΔH:H→H⊗H (with notation ΔH(h)=h1⊗h2), and εH:H→k are all morphisms in ¯Hi,j(Veck). Further, we write
| ¨H=_⊗H:¯Hi,j(Veck)→¯Hi,j(Veck),(X,αX)↦(X⊗H,αX⊗αH) |
for the right tensor functor of H.
If we define the following structures on ¨H:
∙ δ:¨H→¨H¨H and ϵ:¨H→id¯Hi,j(Veck) are defined by
| δX:x⊗h↦(αX(x)⊗h1)⊗α−1H(h2),ϵX:x⊗h↦εH(h)α−1X(x); |
∙ ¨H2:¨H⊗¨H→¨H⊗ and ¨H0:k→¨H(k) are given by
| ¨H2(X,Y):(x⊗a)⊗(y⊗b)↦(x⊗y)⊗αiH(a)αjH(b),¨H0(1k)=1k⊗1H, |
for any X,Y∈¯Hi,j(Veck). Then obviously ¨H=(¨H,δ,ϵ) forms a comonad on ¯Hi,j(Veck) if and only if (H,αH,ΔH,εH) is a Hom-coalgebra over k, Eqs (C1)–(C4) hold if and only if mH and ηH are all morphisms of Hom-coalgebras.
Suppose that there are αH-invariant convolution invertible linear forms ω∈(H⊗H⊗H)∗ and p,q∈H∗, then we can define the following ∗-invertible natural transformations
| ϑX,Y,Z:((x⊗a)⊗(y⊗b))⊗(z⊗c)↦ω(α2iH(a),αi+jH(b),αj−1H(c))(αiX(x)⊗(α−1Y(y)⊗α−j−2Z(z))),ιX:1k⊗(x⊗a)↦p(a)αjX(x),κX:(x⊗a)⊗1k↦q(a)αiX(x), |
where a,b,c∈H, x∈X, y∈Y, z∈Z and X,Y,Z∈Veck. Thus we immediately get that ϑ satisfies Eq (3.1) if and only if ω satisfies
| ∑αH(a1)(b1c1)ω(a2,b2,c2)=∑ω(a1,b1,c1)(a2b2)αH(c2); | (3.6) |
ϑ satisfies Eq (3.2) if and only if ω satisfies
| ∑ω(αH(a1),αH(b1),c1d1)ω(a2b2,αH(c2),αH(d2))=∑ω(b1,c1,αH(d1))ω(αH(a1),α−1H(b21)α−1H(c21),αH(d2))ω(αH(a2),b22,c22); | (3.7) |
ι satisfies Eq (3.3) and κ satisfies Eq (3.4) if and only if p,q satisfy
| ∑p(a1)1Ha2=αH(a1)p(a2),∑q(a1)a21H=αH(a1)q(a2); | (3.8) |
ϑ,ι and κ satisfy Eq (3.5) if and only if ω, p and q satisfy
| ω(a,1H,b)=q(a)p∗−1(b). | (3.9) |
This means, ¨H=(¨H,δ,ϵ,¨H2,¨H0,ϑ,ι,κ) forms a quasi-monoidal comonad on ¯Hi,j(Veck) if and only if H=(H,αH,mH,ηH,ΔH,εH,ω,p,q) forms a Hom-coquasi-bialgebra over k (see [20] for the dual definition). Further, from Theorem 3.10, one get that Corep(H)=(¯Hi,j(Veck))¨H, the category of right H-Hom-comodules, is a monoidal category and its associativity constraint, unit constraints are given as follows:
| AM,N,P((m⊗n)⊗p)=∑ω(α2i(m1),αi+j(n1),αj−1(p1))αiM(m0)⊗(α−1N(n0)⊗α−j−2P(p0)),LM(1k⊗m)=∑p(m1)αjM(m0),RM(m⊗1k)=∑q(m1)αiM(m0), |
where m∈M, n∈N, p∈P, M,N,P∈Corep(H).
Example 3.11. Under the consideration of Example 3.10, if all the Hom-structure maps α are identity maps, then the Hom-coquasi-bialgebra is exactly the Majid algebra (also called a Majid algebra, see [13] for details) over k.
Example 3.12. Let B=(B,μ,1B,Δ,ε) be a bialgebra over k, αB:B→B be an endo-isomrophism. Recall that a k-linear form g∈B∗ is called
(1) dual central if g(x1)x2=x1g(x2) for any x∈B;
(2) dual group-like if it is convolution invertible and satisfies g(xy)=g(x)g(y) for any x,y∈B;
(3) αB-invariant if g(αB(x))=g(x).
Now suppose that p,q∈B∗ are all dual central dual group-like and αB-invariant linear forms. Define a k-linear form ω:B⊗B⊗B→k by
| ω(x,y,z)=p(x)ε(y)q∗−1(z),foranyx,y,z∈B, |
define the new multiplication μαB and comultiplication ΔαB by
| μαB=αB∘μ,ΔαB=Δ∘αB. |
Then it is a direct calculation to check that αB,ω,p,q satisfy Eqs.(3.6) - (3.9) (under μαB and ΔαB), hence Bp,qαB=(B,αB,μαB,1B,ΔαB, ε,ω,p,q) forms a nontrivial Hom-coquasi-bialgebra.
Recall that a braiding in a monoidal category (C,⊗,I,a,l,r) is a natural isomorphism τ: ⊗⇒⊗op:C×C→C such that the following identities hold
| aY,Z,X∘τX,Y⊗Z∘aX,Y,Z=(idY⊗τX,Z)∘aY,X,Z∘(τX,Y⊗idZ), | ( |
| a−1Z,X,Y∘τX⊗Y,Z∘a−1X,Y,Z=(τX,Z⊗idY)∘a−1X,Z,Y∘(idX⊗τY,Z) | ( |
for any X,Y,Z∈C.
Now let F be a quasi-monoidal comonad on C. Suppose that there is a natural transformation σ: ⊗∘(F×F)⇒⊗op:C×2→C. From Lemma 2.1, for any objects M,N in CF, σ can induce a natural transformation
| τM,N=σ♯M,N:M⊗NρM⊗ρN→FM⊗FNσM,N→N⊗M. |
Conversely, if there exists τ:⊗⇒⊗op:C×C→C, then from Lemma 2.1, for any X,Y∈C, τ can induce the following
| σX,Y=τ♭X,Y:FX⊗FYτFX,FY→FY⊗FXεY⊗εX→Y⊗X. |
Next we will discuss when τ is a braiding in CF.
Lemma 4.1. τ is an isomorphism if and only if σ is ∗-invertible.
Proof. Straightforward from Proposition 2.2.
Lemma 4.2. τ is F-colinear if and only if σ satisfies
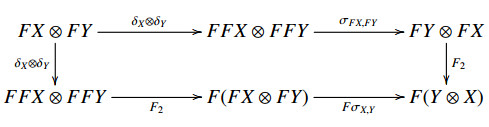
|
(4.1) |
for any X,Y∈C.
Proof. ⇐): We compute
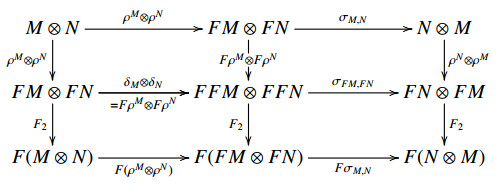
|
for any M,N∈CF. Hence τM,N is F-colinear.
⇒): Conversely, notice that τFX,FY is F-colinear for any X,Y∈C, we have
| F(εY⊗εX)∘FτFX,FY∘ρFX⊗FY=F(εY⊗εX)∘ρFY⊗FX∘τFX,FY, |
which implies Diagram (4.1) holds.
Lemma 4.3. Diagram (B1) holds in CF if and only if σ satisfies
| ϑY,Z,X∘σFX,FY⊗FZ∘(id⊗F2)∘ϑFFX,FFY,FFZ∘(δ2X⊗δ2Y⊗δ2Z)=(id⊗σX,Z)∘ϑY,FX,FZ∘(σFFX,FY⊗id)∘(δ2X⊗δY⊗δZ) | (4.2) |
for any X,Y,Z∈C.
Proof. ⇐): Take X=M, Y=N, Z=P for any F-comodules M,N,P. Multiplied by ρM⊗ρN⊗ρP right on both sides of Eq (4.2), we immediately get Diagram (B1).
⇒): Since Diagram (B1) is commutative for any FX,FY,FZ∈C, multiplied by ε⊗ε⊗ε left on both sides of the above equation, we get Eq (4.2).
Lemma 4.4. For any X,Y,Z∈C, Diagram (B2) holds in CF if and only if σ satisfies
| ϑ∗−1Z,X,Y∘σFX⊗FY,FZ∘(F2⊗id))∘ϑ∗−1FFX,FFY,FFZ∘(δ2X⊗δ2Y⊗δ2Z)=(σX,Z⊗id)∘ϑ∗−1FX,FZ,Y∘(id⊗σFY,FFZ)∘(δX⊗δY⊗δ2Z), | (4.3) |
where ϑ∗−1 means the ∗-inverse of ϑ.
Proof. The proof is similar to Lemma 4.3.
Definition 4.5. Let (F,δ,ε,F2,F0,ϑ,ι,κ) be a quasi-monoidal comonad on a monoidal category C. If there is a ∗-invertible natural transformation σ∈Nat(F⊗F,⊗op), satisfying Eqs (4.1)–(4.3) for any X,Y,Z∈C, then σ is called a coquasitriangular structure of F, and (F,σ) is called a coquasitriangular quasi-monoidal comonad.
Combining Lemma 4.1–Definition 4.5, we obtain the following result.
Theorem 4.6. Let (F,δ,ε,F2,F0,ϑ,ι,κ) be a quasi-monoidal comonad on a monoidal category C. Then CF is a braided monoidal category if and only if there exists a natural transformationσ:F⊗F→⊗op such that (F,σ) is a coquasitriangular quasi-monoidal comonad. Further, the braiding in CFis given by τ=σ♯.
Corollary 4.7. Let (F,σ) be a coquasitriangular quasi-monoidal comonad on a monoidal category C. Then for any X,Y,Z∈C, σ satisfies the following generalized Yang-Baxter equation:
| (id⊗σX,Y)∘ϑZ,FX,FY∘(σFFX,FZ⊗id)∘ϑ∗−1F3X,FFZ,FFY∘(id⊗σF3Y,F3Z)∘ϑF4X,F4Y,F4Z∘(δ4X⊗δ4Y⊗δ4Z)=ϑZ,Y,X∘(σFY,FZ⊗id)∘ϑ∗−1FFY,FFZ,FX∘(id⊗σFFX,F3Z)∘ϑF3Y,F3X,F4Z∘(σF4X,F4Y⊗id)∘(δ4X⊗δ4Y⊗δ4Z). |
Proof. Straightforward.
Example 4.8. If F is a monoidal comonad on C, and σ:⊗∘F×2⇒⊗op is a ∗-invertible natural transformation satisfying Eqs (4.1)–(4.3), then (F,σ) is exactly a coquasitriangular monoidal comonad (see [9], Definition 4.12).
Example 4.9. With the notations in Example 3.10, if Q∈(H⊗H)∗ is αH-invariant and convolution invertible, then we have the following ∗-invertible natural transformation
| σX,Y:¨HX⊗¨HY→Y⊗X,(x⊗a)⊗(y⊗b)↦Q(αiH(a),αjH(b))αj−i−1Y(y)⊗αi−j−1X(x), |
where x∈X, y∈Y and X,Y∈¯Hi,j(Veck). Thus we immediately get that σ satisfies Eq (4.1) if and only if Q satisfies
| ∑Q(a1,b1)a2b2=∑b1a1Q(a2,b2), |
σ satisfies Eqs (4.2) and (4.3) if and only if Q satisfies
| ∑ω(b1,c1,a1)Q(αH(a21),b21c21)ω(a22,b22,c22)=∑Q(a1,c1)ω(b1,α−1H(a21),c2)Q(α−1H(a22),b2),∑ω∗−1(c1,a1,b1)Q(a21b21,αH(c21))ω∗−1(a22,b22,c22)=∑Q(a1,c1)ω∗−1(a2,α−1H(c21),b1)Q(b2,α−1H(c22)), |
where a,b,c∈H. That is, (¨H,σ) forms a coquasitriangular quasi-monoidal comonad if and only if (H,Q) is a coquasitriangular Hom-coquasi-bialgebra. Further, from Theorem 4.6, one get that Corep(H)=(¯Hi,j(Veck))¨H is a braided monoidal category.
Example 4.10. With the notations in Example 3.12, if p∈B∗ is a dual central dual group-like αB-invariant k-linear form on a bialgebra B, then we get a coquasi-bialgebra Bp,pαB. Now suppose that Q∈(B⊗B)∗ is the coquasitriangular structure over B. If Q∘(αB⊗αB)=Q, then after a straightforward compute we get that Q is also a coquasitriangular structure over the Hom-coquasi-bialgebra Bp,pαB.
Let F=(F,δ,ε,F2,F0) be a quasi-monoidal comonad on a monoidal category (C,⊗,I,a,l,r).
Definition 5.1. A gauge transformation on F is a ∗-invertible natural transformation ξ:F⊗F⇒⊗.
Using a gauge transformation ξ on F, we can build a new quasi-monoidal comonad Fξ as follows.
Firstly, as a functor, Fξ=F:C→C.
Secondly, the comonad structure of Fξ is Fξ=F=(F,δ,ε).
Thirdly, the quasi-monoidal functor structure of Fξ is given by:
∙ for any X,Y∈C, Fξ2:F⊗F⇒F⊗ is defined as follows
| Fξ2(X,Y):FX⊗FYδ2X⊗δ2Y→F3X⊗F3Yξ→FFX⊗FFYF2→F(FX⊗FY)F(ξ∗−1X,Y)→F(X⊗Y) | (5.1) |
where ξ∗−1 means the ∗-inverse of ξ;
∙ Fξ0=F0:FI→I.
Proposition 5.2. With the above notations, δ and ε are both monoidal natural transformations
Proof. We only need to show the compatible conditions Eqs (C1)–(C4) hold.
To prove Eq (C1), we compute

|
for any X,Y∈C. The rest are straightforward.
For any X,Y∈C, define the natural transformation ϑξ:(F⊗F)⊗F⇒_⊗(_⊗_) by
| ϑξX,Y,Z=(id⊗ξ∗−1Y,Z)∘ξ∗−1X,FY⊗FZ∘(id⊗F2)∘ϑFX,FFY,FFZ∘(ξFFX⊗F3Y,F3Z)∘(F2⊗id)∘(ξF3X,F4Y⊗id)∘(δ3X⊗δ4Y⊗δ3Z), | (5.2) |
and define the followings natural transformations:
| ιξX:I⊗FXF0⊗δX→FI⊗FFXξ→I⊗FXιX→X, | (5.3) |
and
| κξX:FX⊗IδX⊗F0→FFX⊗FIξ→FX⊗IκX→X. | (5.4) |
It is easy to get that ϑξ, ιξ and κξ are all ∗-invertible. Further, we have the following properties.
Lemma 5.3. With the above notations, ϑξ satisfies Eqs (3.1) and (3.2).
Proof. We only prove Eq (3.1). For any X,Y,Z∈C, we compute
| F(ϑξX,Y,Z)∘Fξ2∘(Fξ2⊗id)∘(δX⊗δY⊗δZ)=F(id⊗ξ∗−1Y,Z)∘F(ξ∗−1X,FY⊗FZ)∘F(id⊗F2)∘F(ϑFX,FFY,FFZ)∘F(ξFFX⊗F3Y,F3Z)∘F(F2⊗id)∘F(ξF4X,F3Y⊗id)∘F(δ3X⊗δ4Y⊗δ3Z)∘F(ξ∗−1FX⊗FY,FZ)∘F2∘(δFX⊗FY⊗δFZ)∘ξF(FX⊗FY),FFZ∘(δFX⊗FY⊗δFZ)∘(F(ξ∗−1FX,FY)⊗id)∘(F2⊗id)∘(δFX⊗δFY⊗id)∘(ξFFX,FFY⊗id)∘(δFX⊗δFY⊗id)∘(δX⊗δY⊗δZ)=F(id⊗ξ∗−1Y,Z)∘F(ξ∗−1X,FY⊗FZ)∘F(id⊗F2)∘F(ϑFX,FFY,FFZ)∘F(ξFFX⊗F3Y,F3Z)∘F(F2⊗id)∘F(δFX⊗δ2FY⊗δ3Z)∘F(ξ∗−1FFX⊗FFY,FZ)∘F2∘(δFFX⊗FFY⊗δFZ)∘ξF(FFX⊗FFY),FFZ∘(δFFX⊗FFY⊗δFZ)∘(F2⊗id)∘(δFX⊗δFY⊗id)∘(ξFFX,FFY⊗id)∘(δ2X⊗δ2Y⊗δZ)=F(id⊗ξ∗−1Y,Z)∘F(ξ∗−1X,FY⊗FZ)∘F(id⊗F2)∘F(ϑFX,FFY,FFZ)∘F2∘(F2⊗id)∘(δ2FX⊗δ2FY⊗δ2FZ)∘ξF3X⊗F3Y,FFZ∘(F2⊗id)∘(δFX⊗δ2FY⊗δFZ)∘(ξFFX,FFY⊗id)∘(δ2X⊗δ2Y⊗δZ)=F(id⊗ξ∗−1Y,Z)∘F(ξ∗−1X,FY⊗FZ)∘F(id⊗F2)∘F2∘(id⊗F2)∘(δX⊗δ2Y⊗δ2Z)∘ϑFX,FY,FZ∘ξFFX⊗FFY,FFZ∘(F2⊗id)∘(ξF3X,F3Y⊗id)∘(δ3X⊗δ3Y⊗δ2Z)=F(ξ∗−1X,Y⊗Z)∘F(id⊗F(ξ∗−1Y,Z))∘F2∘(δX⊗δFY⊗FZ)∘(id⊗F2)∘(id⊗δY⊗δZ)∘(εFX⊗εFY⊗εFZ)∘ϑFFX,FFY,FFZ∘ξF3X⊗F3Y,F3Z∘(F2⊗id)∘(ξF4X,F4Y⊗id)∘(δ4X⊗δ4Y⊗δ3Z)=F(ξ∗−1X,Y⊗Z)∘F2∘(δX⊗δY⊗Z)∘ξFX,F(Y⊗Z)∘ξ∗−1FFX,FF(Y⊗Z)∘(FδX⊗FδY⊗Z)∘(id⊗FF(ξ∗−1Y,Z))∘(id⊗F(F2))∘(id⊗F(δY⊗δZ))∘(id⊗F2)∘ϑFFX,FFY,FFZ∘ξF3X,F3Y⊗F3Z∘(F(id⊗FFεFY)⊗FFFεFZ)∘(F2⊗id)∘(ξF4X,F5Y⊗id)∘(δ4X⊗δ5Y⊗δ4Z)=F(ξ∗−1X,Y⊗Z)∘F2∘(δX⊗δY⊗Z)∘ξFX,F(Y⊗Z)∘(δX⊗δY⊗Z)∘(F(id⊗ξ∗−1Y,Z))∘(id⊗F2(FY,FZ))∘(id⊗δY⊗δZ)∘(id⊗ξFX,FY)∘(id⊗ξ∗−1FFY,FFZ)∘(id⊗δFY⊗δFZ)∘ξ∗−1FX,FFY⊗FFZ∘(id⊗F2(FFY⊗FFZ))∘ϑFFX,F3Y,F3Z∘ξF3X,F4Y⊗F4Z∘(F2⊗id)∘(ξF4X,F5Y⊗id)∘(δ4X⊗δ5Y⊗δ4Z)=Fξ2(FX,FY⊗FZ)∘(id⊗Fξ2(FY,FZ))∘ϑξFX,FY,FZ∘(δX⊗δY⊗δZ). |
Thus the conclusion holds.
Lemma 5.4. With the above notations, ιξ satisfies Eq (3.3) and κξ satisfies Eq (3.4).
Proof. We only prove Eq (3.3). For any X∈C, we have
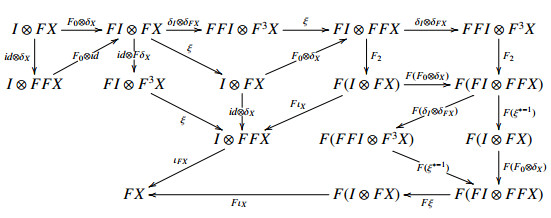
|
which implies Eq (3.3).
Lemma 5.5. With the above notations, ϑξ and ιξ, κξ satisfy Eq (3.5).
Proof. For any X,Y∈C, we obtain
| (id⊗ιξY)∘(ϑξX,I,FY)∘(id⊗F0⊗δY)=(id⊗ιY)∘(id⊗ξI,FFY)∘(id⊗F0⊗δY)⊗(id⊗ξ∗−1I,FY)∘ξ∗−1X,FI,FFY∘(id⊗F2)∘ϑFX,FFI,F3Y∘ξFFX⊗F3I,F4Y∘(F2⊗id)∘(ξF3X,F4I⊗id)∘(δ3X⊗δ4I⊗δ3FY)⊗(δX⊗F0⊗δY)=ξ∗−1X,Y∘(id⊗FιY)∘(id⊗F2)∘ϑFX,FI,FFY∘ξFFX⊗FFI,F3Y∘(F2⊗id)∘(ξF3X,F3I⊗id)∘(δ3X⊗δ3I⊗δ2FY)∘(δX⊗F0⊗δY)=ξ∗−1X,Y∘(id⊗ιFY)∘ϑFX,I,FFY∘(id⊗F0⊗δY)∘ξFFX⊗I,FFY∘(F2⊗id)∘(ξF3X,FI⊗id)∘(δ3X⊗δI⊗δ2Y)∘(δX⊗F0⊗id)=(id⊗εY)∘ξ∗−1X,FY∘(κFX⊗id)∘ξFFX⊗I,FFY∘(F2⊗id)∘(δFX⊗F0⊗id)∘(ξFFX,I⊗id)∘(δ2X⊗F0⊗δ2Y)=(id⊗εY)∘ξ∗−1X,FY∘ξFX,FFY∘(κFFX⊗id)∘(δFX⊗id⊗id)∘(ξFFX,I⊗id)∘(δ2X⊗F0⊗δ2Y)=(id⊗εY)∘(κX⊗id)∘(ξFX,I⊗id)∘(δX⊗F0⊗id)=(id⊗εY)∘(κξX⊗id) |
hence Eq (3.5) holds.
Theorem 5.6. Fξ=(F,δ,ε,Fξ2,F0,ϑξ,ιξ,κξ) is a quasi-monoidal comonad.
Remark 5.7. (CFξ,⊗,I,Aξ,Lξ,Rξ) is a monoidal category, where Aξ=(ϑξ)♯, Lξ=(ιξ)♯, Rξ=(κξ)♯.
Now consider a coquasitriangular quasi-monoidal comonad (F,σ). For any gauge transformation ξ on F, for any X,Y∈C, define
| σξX,Y:FX⊗FYδ2⊗δ2→F3X⊗F3Yξ→FFX⊗FFYσ→FY⊗FXξ∗−1→Y⊗X. | (5.5) |
Proposition 5.8. With the above notations, σξ is a coquasitriangular structure of Fξ. Thus Fξ is a coquasitriangular quasi-monoidal comonad. Hence CFξ is a braided monoidal category with the braiding τξ=(σξ)♯.
Proof. Firstly, it is straightforward to get that σξ is ∗-invertible.
Secondly, to prove Eq (4.1), for any X,Y∈C, we compute
| Fξ2∘σξFX,FY∘(δX⊗δY)=F(ξ∗−1Y,X)∘F2∘(δY⊗δX)∘ξFY,FX∘ξ∗−1FFY,FFX∘(δ2Y⊗δ2X)∘σFX,FY∘ξFFX,FFY∘(δ2X⊗δ2Y)=F(ξ∗−1Y,X)∘F2∘σFFX,FFY∘ξF3X,F3Y∘(δ3X⊗δ3Y)=F(ξ∗−1Y,X)∘F(σFX,FY)∘F(ξFFX,FFY)∘F(ξ∗−1F3X,F3Y)∘F(δ3X⊗δ3Y)∘F2∘(δX⊗δY)=F(ξ∗−1Y,X)∘F(σFX,FY)∘F(ξFFX,FFY)∘F(δ2X⊗δ2Y)∘F(ξ∗−1FX,FY)∘F2∘(δFX⊗δFY)∘ξFFX,FFY∘(δ2X⊗δ2Y)=F(σξY,X)∘Fξ2(FX,FY)∘(δX⊗δY). |
Thirdly, for Eq (4.2), we have
| ϑξY,Z,X∘σξFX,FY⊗FZ∘(id⊗Fξ2)∘ϑξFFX,FFY,FFZ∘(δ2X⊗δ2Y⊗δ2Z)=(id⊗ξ∗−1Z,X)∘ξ∗−1Y,FZ⊗FX∘(id⊗F2)∘ϑFY,FFZ,FFX∘ξFFY⊗F3Z,F3X∘(F2⊗id)∘(ξF3Y⊗F4Z⊗id)∘(δ3Y⊗δ4Z⊗δ3X)∘ξ∗−1FY⊗FZ,FX∘σFFX,F(FY⊗FZ)∘ξF3X,FF(FY⊗FZ))∘(δ2FX⊗δ2FY⊗FZ)∘(id⊗F(ξ∗−1FY,FZ))∘(id⊗F2)∘(id⊗ξF3Y,F3Z)∘(id⊗δ2FY⊗δ2FZ)∘(id⊗ξ∗−1F2Y,F2Z)∘ξ∗−1FFX,F3Y⊗F3Z∘(id⊗F2)∘ϑF3X,F4Y,F4Z∘ξF4X⊗F5Y,F5Z∘(F2⊗id)∘(ξF5X,F6Y⊗id)∘(δ5X⊗δ6Y⊗δ5Z)=(id⊗ξ∗−1Z,X)∘ξ∗−1Y,FZ⊗FX∘(id⊗F2)∘ϑFY,FFZ,FFX∘σF3X,FFY⊗F3Z∘(id⊗F2)∘ϑF4X,F3Y,F4Z∘(δ2FFX⊗δ2FY⊗δ2FFZ)∘ξF3X⊗FFY,F3Z∘(F2⊗id)∘(ξF4X,F3Y⊗id)∘(δ4X⊗δ3Y⊗δ3Z)=(id⊗ξ∗−1Z,X)∘(id⊗σFX,FZ)∘ξ∗−1Y,FFX⊗FFZ∘(id⊗F2)∘ϑFY,F3X,F3Z∘(σF4X,FFY⊗id)∘(δ2FFX⊗δFY⊗δFFZ)∘ξF3X⊗FFY,F3Z∘(F2⊗id)∘(ξF4X,F3Y⊗id)∘(δ4X⊗δ3Y⊗δ3Z)=(id⊗ξ∗−1Z,X)∘(id⊗σFX,FZ)∘ξ∗−1Y,FFX⊗FFZ∘(id⊗F2)∘ϑFY,F3X,F3Z∘ξF2Y⊗F4X,F4Z∘(F(σF4X,FFY)⊗id)∘(F2⊗id)∘(δ4FX⊗δ3FY⊗δFY)∘(ξFFX,FFY⊗id)∘(id⊗δ2Z⊗δ2Z)=(id⊗σξX,Z)∘ϑξY,FX,FZ∘(σξFFX,FF⊗id)∘(δ2X⊗δY⊗δZ). |
At last, we can prove Eq (4.3) in a similar way. Thus the conclusion holds.
Now consider the corepresentations of F and Fξ.
Theorem 5.9. CF and CFξ are isomorphic as monoidal categories.Further, if F is a coquasitriangular quasi-monoidal comonad, then CF and CFξare braided isomorphic.
Proof. For any morphism f and objects M,N in C, the monoidal functor is defined as follows
| E=(E,Eξ2,E0):(CF,⊗,I,A,L,R)→(CFξ,⊗,I,Aξ,Lξ,Rξ), |
where
| E(M):=MasanF−comodule,E(f):=f,E0=idI, |
and Eξ2(M,N):E(M)⊗E(N)→E(M⊗N) is given by
| Eξ2(M,N)=ξ♯:M⊗NρM⊗ρN→FM⊗FNξM,N→M⊗N. |
Obviously E is well-defined.
Now we will check relation (2.1). Indeed, we have
| Eξ2(M,N⊗P)∘(id⊗Eξ2(N,P))∘AξM,N,P=ξM,N⊗P∘(id⊗F2)∘(ρM⊗ρN⊗ρP)∘(id⊗ξN,P)∘(id⊗ρN⊗ρP)∘(id⊗ξ∗−1N,P)∘ξ∗−1M,FN⊗FP∘(id⊗F2)∘ϑFM,FFN,FFP∘ξFFM⊗F3N,F3P∘(F2⊗id)∘(ξF3M,F4N⊗id)∘(δ3M⊗δ4N⊗δ3P)∘(ρM⊗ρN⊗ρP)=ξM,N⊗P∘(ρM⊗F2)∘ξ∗−1M,FN⊗FP∘(id⊗F2)∘ϑFM,FFN,FFP∘ξFFM⊗F3N,F3P∘(F2⊗id)∘(ξF3M,F4N⊗id)∘(δ3M⊗δ4N⊗δ3P)∘(ρM⊗ρN⊗ρP)=ξM,N⊗P∘ξ∗−1FM,F(N⊗P)∘(δM⊗δN⊗P)∘(id⊗F2)∘ϑFM,FN,FP∘ξFFM⊗FFN,FFP∘(F2⊗id)∘(ξF3M,F3N⊗id)∘(δ3M⊗δ3N⊗δ2P)∘(ρM⊗ρN⊗ρP)=ϑM,N,P∘ξFM⊗FN,FP∘(F2⊗id)∘(ξF2M,F2N⊗id)∘(δ2M⊗δ2N⊗δP)∘(ρM⊗ρN⊗ρP)=E(AM,N,P)∘Eξ2(M⊗N,P)∘(Eξ2(M,N)⊗id), |
which implies Eq (2.1).
Further, we can obtain (2.2) and (2.3) by straightforward computation. Hence the conclusion holds.
Moreover, if σ is a coquasitriangular structure of F, then from Theorem 5.6, (Fξ,σξ) is also a coquasitriangular quasi-monoidal comonad. Then we have
| Eξ2(N,M)∘τξM,N=ξN,M∘(ρN⊗ρM)∘ξ∗−1N,M∘σFM,FN∘ξFFM,FFN∘(δ2M⊗δ2N)∘(ρM⊗ρN)=(εN⊗εM)∘σFM,FN∘ξFFM,FFN∘(δ2M⊗δ2N)∘(ρM⊗ρN)=σFM,FN∘(ρN⊗ρM)∘ξM,N∘(ρM⊗ρN)=E(τM,N)∘Eξ2(M,N), |
which implies (E,Eξ2,E0) is a braided monoidal functor.
Example 5.10. With the notations in Example 3.10, if there is a convolution invertible linear form χ∈(H⊗H)∗ satisfying χ∘(αH⊗αH)=χ, then we have the following ∗-invertible natural transformation in ¯Hi,j(Veck)
| ξX,Y:¨HX⊗¨HY→X⊗Y,(x⊗a)⊗(y⊗b)↦χ(αiH(a),αjH(b))α−1X(x)⊗α−1Y(y), |
where a,b∈H, x∈X, y∈Y and X,Y∈¯Hi,j(Veck). It is not hard to check that ¨Hξ2, ϑξ, ιξ and κξ in Eqs (5.1)–(5.4) are deduced from the following
| mχ(a⊗b)=∑χ∗−1(a1,b1)α−2H(a21)α−2H(b21)χ(a22,b22), |
where χ∗−1 means the convolution inverse of χ, and
| ωχ(a,b,c)=∑χ∗−1(b11,c11)χ∗−1(αH(a11),α−1H(b121)c12)ω(a12,α−1H(b122),c21)χ(a21b21,αH(c22))χ(a22,b22),pχ(a)=∑p(a1)χ(1H,a2),qχ(a)=∑q(a1)χ(a2,1H), |
respectively. Thus from Example 3.10 and Theorem 5.6, Hχ=(H,αH,mχ,1H,Δ,ε,ωχ,pχ,qχ) is also a Hom-coquasi-bialgebra.
Example 5.11. With the notations in Example 3.12, note that the BαB=(B,αB,αB∘μ,1B,Δ∘αB,ε) is a Hom-bialgebra, and it can be seen as a Hom-coquasi-bialgebra BαB=(B,αB,αB∘μ,1H,Δ∘αB,ε,ε⊗ε⊗ε,ε,ε). If there are αB-invariant and dual central dual group-like k-linear forms p,q∈B∗, then we have the following gauge transformation χ∈(B⊗B)∗ by
| χ(a,b)=q∗−1(a)p(b),where a,b∈B. |
Obviously BχαB=Bp,qαB.
The work was partially supported by the National Natural Science Foundation of China (No. 11801304, 11871301), and the Taishan Scholar Project of Shandong Province (No. tsqn202103060).
The authors declare there is no conflict of interest.
| [1] |
B. Mesablishvili, R. Wisbauer, Bimonads and Hopf monads on categories, J. K-Theory, 7 (2011), 349–388. https://doi.org/10.1017/is010001014jkt105 doi: 10.1017/is010001014jkt105

|
| [2] |
M. Livernet, B. Mesablishvili, R. Wisbauer, Generalised bialgebras and entwined monads and comonads, J. Pure Appl. Algebra, 219 (2015), 3263–3278. https://doi.org/10.1016/j.jpaa.2014.10.013 doi: 10.1016/j.jpaa.2014.10.013

|
| [3] |
B. Mesablishvili, R. Wisbauer, Galois functors and generalised Hopf modules, J. Homotopy Relat. Struct. 9 (2014), 199–222. https://doi.org/10.1007/s40062-013-0072-1 doi: 10.1007/s40062-013-0072-1

|
| [4] |
B. Mesablishvili, R. Wisbauer, The fundamental theorem for weak braided bimonads, J. Algebra, 490 (2017), 55–103. https://doi.org/10.1016/j.jalgebra.2017.06.023 doi: 10.1016/j.jalgebra.2017.06.023

|
| [5] |
I. Moerdijk, Monads on tensor categories, J. Pure Appl. Algebra, 168 (2002), 189–208. https://doi.org/10.1016/S0022-4049(01)00096-2 doi: 10.1016/S0022-4049(01)00096-2

|
| [6] |
A. Bruguières, A. Virelizier, Hopf monads, Adv. Math., 215 (2007), 679–733. https://doi.org/10.1016/j.aim.2007.04.011 doi: 10.1016/j.aim.2007.04.011

|
| [7] |
A. Bruguières, S. Lack, A. Virelizier, Hopf monads on monoidal categories, Adv. Math., 227 (2011), 745–800. https://doi.org/10.1016/j.aim.2011.02.008 doi: 10.1016/j.aim.2011.02.008

|
| [8] |
G. Böhm, S. Lack, R. Street, Weak bimonads and weak Hopf monads, J. Algebra, 328 (2011), 1–30. https://doi.org/10.1016/j.jalgebra.2010.07.032 doi: 10.1016/j.jalgebra.2010.07.032

|
| [9] |
X. H. Zhang, L. H. Dong, Braided mixed datums and their applications on Hom-quantum groups, Glasgow Math. J., 60 (2018), 231–251. https://doi.org/10.1017/S0017089517000088 doi: 10.1017/S0017089517000088

|
| [10] |
X. H. Zhang, W. Wang, X. F. Zhao, Smash coproducts of monoidal comonads and Hom-entwining structures, Rocky Mountain J. Math., 49 (2019), 2063–2105. https://doi.org/10.1216/RMJ-2019-49-6-2063 doi: 10.1216/RMJ-2019-49-6-2063

|
| [11] |
H. X. Zhu, The crossed structure of Hopf bimodules, J. Algebra Appl., 17 (2018), 1850172. https://doi.org/10.1142/S0219498818501724 doi: 10.1142/S0219498818501724

|
| [12] | V. G. Drinfel'd, Quasi-Hopf algebras, Leningrad Math. J., 1 (1990), 1419–1457. |
| [13] |
M. Gerstenhaber, J. Stasheff, Deformation theory and quantum groups with applications to mathematical physics, Contemp. Math., 134 (1992), 219–232. https://doi.org/10.1090/conm/134/1187289 doi: 10.1090/conm/134/1187289

|
| [14] |
I. Angiono, A. Ardizzoni, C. Menini, Cohomology and coquasi-bialgebras in the category of Yetter-Drinfeld modules, Ann. Sc. Norm. Super. Pisa Cl. Sci., 17 (2017), 609–653. https://doi.org/10.2422/2036-2145.201509_018 doi: 10.2422/2036-2145.201509_018

|
| [15] |
X. H. Zhang, X. F. Zhao, W. Wang, Quasi-bimonads and their representations. J. Pure Appl. Algebra, 225 (2021), 106459. https://doi.org/10.1016/j.jpaa.2020.106459 doi: 10.1016/j.jpaa.2020.106459

|
| [16] |
J. Beck, Distributive laws, Lect. Notes Math., 80 (1969), 119–140. https://doi.org/10.1007/BFb0083084 doi: 10.1007/BFb0083084

|
| [17] |
R. Street, The formal theory of monads, J. Pure Appl. Algebra, 2 (1972), 149–168. https://doi.org/10.1016/0022-4049(72)90019-9 doi: 10.1016/0022-4049(72)90019-9

|
| [18] | D. Chikhladze, S. Lack, R. Street, Hopf monoidal comonads, Theory Appl. Categ., 24 (2010), 554–563. |
| [19] |
X. H. Zhang, D. G. Wang, Cotwists of Bicomonads and BiHom-bialgebras, Algebra Repr. Theory, 23 (2020), 1355–1385. https://doi.org/10.1007/s10468-019-09888-2 doi: 10.1007/s10468-019-09888-2

|
| [20] |
M. Elhamdadi, A. Makhlouf, Hopf algebras and tensor categories, Contemp. Math., 585 (2013), 227–245. https://doi.org/10.1090/conm/585/11617 doi: 10.1090/conm/585/11617

|
| [21] |
A. Bruguières, A. Virelizier, Quantum double of Hopf monads and categorical centers, Trans. Amer. Math. Soc., 364 (2012), 1225–1279. https://doi.org/10.1090/S0002-9947-2011-05342-0 doi: 10.1090/S0002-9947-2011-05342-0

|
| [22] |
G. Böhm, T. Brzeziński, R. Wisbauer, Monads and comonads on module categories, J. Algebra, 322 (2009), 1719–1747. https://doi.org/10.1016/j.jalgebra.2009.06.003 doi: 10.1016/j.jalgebra.2009.06.003

|
| [23] |
X. L. Fang, Gauge transformations for quasitriangular quasi-Turaev group coalgebras, J. Algebra Appl., 17 (2018), 1850080. https://doi.org/10.1142/S0219498818500809 doi: 10.1142/S0219498818500809

|
| [24] |
S. H. Wang, A. Van Daele, Y. H. Zhang, Constructing quasitriangular multiplier Hopf algebras by twisted tensor coproducts, Comm. Algebra, 37 (2009), 3171–3199. https://doi.org/10.1080/00927870902747894 doi: 10.1080/00927870902747894

|