On the existence of solutions for the Frenkel-Kontorova models on quasi-crystals

1.   Introduction

We consider the Frenkel-Kontorova models with quasi-periodic potentials. One may refer to [6] for several physical interpretations of such models.

In order to give a unified picture of the known results, let's take the one-dimensional quasi-crystals case for example (see [11] for more advanced studies on general quasi-crystals). Frenkel-Kontorova models describe the dislocations of particles deposited over a substratum given by the quasi-crystals (see [6]). That is, we model the position of the $i$-th particle by $x_i\in\mathbb{R}$ and the total energy is formally composed of the summation over all $i\in \mathbb{Z}$ of spring potential $\frac{1}{2} (x_{i}-x_{i+1})^2$ between the nearest neighbors $i$-th and the $(i+1)$-th particles, and the potential $V(x_i)$ from interaction of the $i$-th particle with the quasi-periodic substratum:

In this article, we are concerned with the case that the potential function $V$ is a pattern equivariant function (see Definition 2.11 for details) and aim to discuss existing approaches to prove the existence of equilibria. Related problems of finding equilibria generated by other types of quasi-periodic functions are discussed in [13,14] and references therein. [10] presents an example to show the non-existence of bounded correctors in the setting of homogenization.

The aperiodicity will bring us difficulties such as loss of compactness and less results are known for the quasi-periodic case than for the periodic one. We now recall the existing results in the literature. In [3,16], under the assumptions that the potential function is large enough, the authors use the idea of the anti-integrable limits to obtain multiple equilibria with any prescribed rotation number or without any rotation numbers. This approach also works for higher dimensional generalization of the quasi-periodic Frenkel-Kontorova model but requires that the corresponding system is far away from the integrable case.

In another direction, [7] considers the one-dimensional Fibonacci quasi-crystals without extra assumption on whether the system is integrable or not, and mainly uses topological methods to establish the existence of the minimal configurations with any given rotation number. Here it is rather essential that the configuration space is one dimension. Note that the minimal configuration is a special equilibrium with extra properties. One may refer to [8] for other methods to search minimal configurations.

In this article, we construct an explicit potential $V$ driven by the Fibonacci quasi-crystals. Our goal here is to provide several approaches to tackle variational problem for the Frenkel-Kontorova models. More precisely, we aim to find minimal configurations and non-minimal equilibrium configurations of the Frenkel-Kontorova model with quasi-periodic potentials.

In order to give a simple and self-contained proof, we fix at the beginning a positive number $\theta = (3\tau+1)/2$ where $\tau = (\sqrt{5}+1)/2 = 1.618\cdots$. We state the main result as follows:

Theorem 1.1. For the Frenkel-Kontorova model with the potential $V$ (which is defined in Section 2.3),

(i) there exists a minimal configuration with the rotation number $\theta$,

(ii) there exists a non-minimal equilibrium configuration with the rotation number $\theta$.

Remark 1. $(1)$ We choose $\theta = (3\tau+1)/2$ for computational convenience. In fact, Theorem 1.1 is valid for any $\theta\in\mathbb{R}$.

$(2)$ Related results could also hold for other one-dimensional quasi-periodic tilings.

But so far, analogues of the above result in higher dimensions are not yet known. We hope to get some inspiration from the specific example and corresponding numerical simulations. For numerical computations of minimal configurations, one may refer to [2,15,5].

Organization of the article. In Section 2, we introduce some necessary fundamentals about quasi-crystals and the variational problem for the Frenkel-Kontorova models. In particular, we give an example of the quasi-crystals, the Fibonacci chain, and an example of the Frenkel-Kontorova models with quasi-periodic potentials.

Section 3 is devoted to the proof of Theorem 1.1. Numerical simulations searching for equilibrium configurations are provided here.

In fact, in Section 3.1, we obtain the minimal configurations for the Frenkel-Kontorova models on one-dimensional Fibonacci quasi-crystals with slight modifications compared with those in [7]. Thus we finish the proof of item (i) in Theorem 1.1. Of course, the KAM circles are minimizers.

We apply the idea of anti-integrable limits to prove the existence of equilibrium configurations of type $h$ in Section 3.2. Then we prove that these equilibrium configurations are non-minimal, which completes the proof of item (ii) in Theorem 1.1.

2.   Preliminaries

We recall several standard notions of quasi-crystals in Section 2.1. In Section 2.2, we introduce the minimal and equilibrium configurations of the variational problem for the Frenkel-Kontorova models. Particularly, in Section 2.3, we state the definition of pattern equivariant potential which we take as the potential in the variational problem, and finally an example is provided.

2.1.   Quasi-crystals

In the $d$-dimensional Euclidean space $\mathbb{R}^d$, the open ball centered at $x$ with radius $r$ is denoted by $B_r(x)$. The closure of a set $A$ and its cardinality are denoted by $\overline{A}$ and $\text{Card}(A)$, respectively. The Lebesgue measure of a Lebesgue-measurable set $A$ is denoted by $\lambda(A)$. Countable subsets in $\mathbb{R}^d$ are called point sets.

A point set $\Lambda\subset \mathbb{R}^d$ is uniformly discrete if there exists $r>0$ such that $(x+B_r(0))\cap(y+B_r(0)) = \emptyset$ holds for all distinct $x,y\in\Lambda$, where the sign "$+$" is the Minkowski sum. A point set $\Lambda$ is relative dense if there exists $R>0$ such that $\Lambda+\overline{B_R(0)} = \mathbb{R}^d$.

Definition 2.1 (Delone sets). A point set $\Lambda\subset \mathbb{R}^d$ is Delone if it is both uniformly discrete and relative dense.

A cluster of the point set $\Lambda$ is the intersection $K\cap \Lambda$ for some compact set $K\subset\mathbb{R}^d$. In particular, if the compact set $K$ is convex, we call such cluster $K\cap \Lambda$ a patch. Two clusters $P_1$ and $P_2$ are said to be equivalent if there exists a vector $v\in\mathbb{R}^d$ such that $P_1+v = P_2$.

Definition 2.2 (finite local complexity). A point set $\Lambda\subset \mathbb{R}^d$ is of finite local complexity if for any $M>0$, the point set $\Lambda$ possesses only finitely many equivalence classes of clusters with diameters smaller than $M$, where the diameter of a subset $A\subset \mathbb{R}^d$ is $\sup_{x,y\in A}|x-y|.$

Definition 2.3 (repetitive). A point set $\Lambda\subset \mathbb{R}^d$ is repetitive if for any cluster $P\subset \Lambda$, there exists $R>0$ such that any ball with radius $R$ contains a cluster equivalent to $P$.

Definition 2.4 (non-periodic). A point set $\Lambda\subset\mathbb{R}^d$ is non-periodic if $t+\Lambda\ne \Lambda$ for any $t\in\mathbb{R}^d\backslash\{0\}$.

Definition 2.5 (quasi-crystal). A point set $\Lambda\subset\mathbb{R}^d$ is called a quasi-crystal if it is repetitive, non-periodic and of finite local complexity.

Let $K\subset\mathbb{R}^d$ be a compact set and fix a cluster $P = \Lambda\cap K$ of a point set $\Lambda$. Given a ball $B_r(a)$, we consider the quantity

If the above quantity converges as $r\rightarrow +\infty$ with $a\in\mathbb{R}^d$ fixed, we call the limit the absolute frequency of the cluster $P$ in $\Lambda$ at $a$ and denote it by $\text{Freq}_a(P)$. If the convergence is uniform in $a$, the limit is called the uniform absolute frequency of $P$ and is denoted by $\text{Freq}(P)$.

2.1.1.   The Fibonacci chain as a specific one-dimensional quasi-crystal

In this section, we will provide the Fibonacci chain as an example of one-dimensional quasi-crystals whose construction will be divided into the following three steps.

Step 1. Construct a one-sided Fibonacci word. Consider a two-letter alphabet $\{a,b\}$ and the free group $\langle a,b\rangle$ generated by letters $a$ and $b$. A substitution rule $\rho$ on $\{a,b\}$ is an endomorphism of $\langle a,b\rangle$. Specifically, we consider the substitution rule

and define a sequence $(u^{(i)})_{i\in\mathbb{Z}^+}$ of finite words by

The iterating process can be illustrated as follows:

It can be directly derived from the definition by induction that

Thus the sequence $(u^{(i)})_{i\in\mathbb{Z}^+}$ converges to an infinite word

as $i\rightarrow +\infty$ in the product topology of $\{a,b\}^{\mathbb{Z}^+}$, where $u_0, u_1, \dots, u_j, \dots\in\{a,b\}$. The limit $u$ is a fixed point of $\rho$ and is called the one-sided Fibonacci word.

Remark 2. Notice that the length of $u^{(i)}$ is the Fibonacci number $f_i$ defined by $f_1 = 1$, $f_2 = 2$ and $f_{i+2} = f_{i+1}+f_{i}$ for all $i\geq 1$. As a convention, let $f_0 = 1, f_{-1} = 0$ and $\tau = (1+\sqrt{5})/{2}$. It is easy to show that

Furthermore, the number of times the letter $a$ appears in $u^{(i)}$ is $f_{i-1}$ and the number of times the letter $b$ appears in $u^{(i)}$ is $f_{i-2}$.

Step 2. Construct a two-sided Fibonacci word. Define a sequence $(w^{(i)})_{i\in\mathbb{Z}^+}$ of finite words by $w^{(i)} = u^{(i)}|u^{(i)}$:

where the vertical line indicates the reference point. In fact, we can get palindromes by eliminating the last two letters of $u^{(i)}$ for all $i\geq 3$, see [1]. Thus we have

for all $i\geq 3$, where $\widetilde{u^{(i)}}$ denotes the reversal of $u^{(i)}$. Furthermore $(w^{(i)})_{i\in\mathbb{Z}^+}$ has two limit points that are 2-periodic points under the substitution $\rho$:

That is, the two bi-infinite words are fixed points of $\rho^2$. Here we only consider the first bi-infinite word whose underlined position is $\underline{ba}$, and we denote it by

where $w_j\in\{a,b\}$ for all $j\in\mathbb{Z}$.

Step 3. Obtain a point set by the bi-infinite word as a quasi-crystal. Let $w_{[k,l]}$ be the finite subword of $w$ from position $k$ to $l$ where $k,l\in\mathbb{Z}$ and $k\leq l$. Note that $w_{[k, k]} = w_{k}\in \{a, b\}$. We then define an assignment function $|\cdot|$ on any finite word of alphabet $\{a,b\}$ by

with $|a| = \tau$, $|b| = 1$. Note that this is a cocycle in the free group.

Then we can recursively define a bi-infinite sequence $S: = (S_i)_{i\in\mathbb{Z}}\in\mathbb{R}^\mathbb{Z}$ by $S_i = S_{i-1}+|w_{i-1}|$ with $S_0: = 0\in\mathbb{R}$ and $w_{i-1}\in \{a, b\}$ constructed in Step 2. The range of the sequence $S:\mathbb{Z}\to\mathbb{R}$, also denoted by $S$, is called the Fibonacci chain.

Remark 3. Our discussion will concern three kinds of words - geometric words, symbolic words and interval words. We will use the following example to illustrate the relationship among these three kinds of words. The geometric word $S\cap[-\tau, \tau+1]$ is the point set $\{-\tau, 0,\tau, \tau+1\}$ and its corresponding symbolic word is $a|ab$ which is more visually appealing. It is obvious that geometric and symbolic words can be transformed into each other. The corresponding interval word is the left-closed and right-open interval $[-\tau,\tau+1)$. The geometric and symbolic words can be transformed into the interval words but not vice versa.

Lemma 2.6. The Fibonacci chain $S$ is a quasi-crystal, so we also call $S$ the Fibonacci quasi-crystal.

Proof. We will prove the lemma by checking the definition of quasi-crystals.

(i). We will first show that $S$ is repetitive. Suppose that $P = K\cap S$ is a cluster of $S$ where $K$ is a compact set in $\mathbb{R}$. Then $P$ is a finite subset of $S$ and we denote the corresponding index set by $I = \{ i\in \mathbb{Z} : S_i\in P \}$. Consider the finite word $w^P: = w_{[\min I-1,\max I]}$ corresponding to $P$. There exists $i\in\mathbb{Z}^+$ such that $w^P$ is a subword of $w^{(i)}$. Since $w^{(1)}$ is a subword of $u^{(4)}$, by induction, $w^{(i)}$ is a subword of $u^{(i+3)}$. The word $bb$ never occurs in $w$ since $b$ only appears in $ab$ as an image of $\rho$. Thus any $2$-letter subword of $w$ contains $a$. Any $6$-letter subword of $w$ contains two images of some letter under $\rho$ and so contains $\rho(a)$. By induction, any $(2^{i+3}-2)$-letter subword of $w$ contains $\rho^{i+2}(a) = u^{(i+3)}$ and thus contains $w^P$. Any ball of radius $2^{i+2}\tau$ in $\mathbb{R}$ contains at least $2^{i+3}$ points in $S$ and the corresponding $(2^{i+3}-1)$-letter subword of $w$ contains $w^P$. Hence $P$ is contained in any ball of radius $2^{i+2}\tau$.

(ii). Obviously, $S$ is of finite local complexity since $|S_i-S_{i-1}|$ ranges on $\{\tau,1\}$ for all $i\in\mathbb{Z}$ and there are only a limited number of arrangements of intervals in a bounded range.

(iii). If $S$ has non-zero period, then $w = \lim\limits_{n\rightarrow +\infty} x^n$ for some finite word $x$. Then the frequency of the letter $a$ in $w$ would be the quotient of the number of letter $a$ in $x$ divided by the length of $x$, which is rational. But on the other hand, the frequency of the letter $a$ is

and is irrational, which is a contradiction.

In conclusion, we have constructed the Fibonacci quasi-crystal $S$ which is a geometric word associated with the bi-infinite symbolic word $w$.

2.2.   The variational framework for the Frenkel-Kontorova model

We consider the space $\mathbb{R}^\mathbb{Z}$ of bi-infinite real-valued sequences with the product topology. An element $x\in\mathbb{R}^\mathbb{Z}$ is denoted by $(x_i)_{i\in\mathbb{Z}}$ and is sometimes called a configuration.

Given a function $H:\mathbb{R}^2\rightarrow \mathbb{R}$ we extend $H$ to arbitrary finite segments $(x_j, \dots, x_k), j<k$, of configuration $x\in\mathbb{R}^\mathbb{Z}$ by

We say that the segment $(x_j, \dots, x_k)$ is minimal with respect to $H$ if

for all $(x_j^*, \dots, x_k^*)$ with $x_j = x_j^*$ and $x_k = x_k^*$.

Definition 2.7 (minimal configuration). A configuration $x\in\mathbb{R}^\mathbb{Z}$ is minimal if every finite segment of $x$ is minimal.

Definition 2.8 [stationary configuration] If $H$ is $C^1$, we say that $x\in \mathbb{R}^\mathbb{Z}$ is a stationary configuration or an equilibrium configuration if

Obviously, each minimal configuration is a stationary configuration.

In this article, we take the function $H$ as follows:

Then (4) becomes

In order to further describe the configurations, we introduce the following two notions.

Definition 2.9 (rotation number). Let $\rho\in\mathbb{R}$. A configuration $x\in\mathbb{R}^\mathbb{Z}$ has a rotation number $\rho$ if the limit

Definition 2.10 (type-$h$ configurations). Let $h:\mathbb{Z}\rightarrow \mathbb{R}$. A configuration $x\in\mathbb{R}^\mathbb{Z}$ is of type-$h$ if

It is easy to see that the notion of the type-$h$ configurations is more general than that of the rotation number. Taking $h(i) = \rho i$ for instance, any configuration of type-$h$ has a rotation number $\rho$.

2.3.   The equivariant potential

In this section, we aim to build some special equivariant potential generated by the Fibonacci quasi-crystal. We will first introduce the following notion of "equivariant" in our settings.

Definition 2.11 For any point set $\Lambda\subset\mathbb{R}^d$, we say a continuous function $f:\mathbb{R}^d\rightarrow \mathbb{R}$ is $\Lambda$-equivariant if there exists $R>0$ such that if $x,y\in\mathbb{R}^d$ satisfy

then $f(x) = f(y)$.

Given $x\geq 0$, we denote

It is easy to see that both $\alpha$ and $\beta$ are right-continuous step functions.

As is explained in Remark 3, for all $i\in\mathbb{Z}^+$, we can regard $u^{(i)}$ defined in (1) as a left-closed and right-open interval in $\mathbb{R}$, and one can always find some $i(x)\in\mathbb{Z}^+$ such that $x\in u^{(i(x))} \setminus u^{(i(x)-1)}$ with $u^{(0)} = \emptyset$. Or, in other words, due to (3), we have

and so we get either

Let us consider the later case, and we have $0\leq x-\tau^{n_1}< \tau^{n_1-1}$. Therefore, considering $x-\tau^{n_1}$ instead of $x$, we get either

Hence, we could repeat the above procedure finitely many times and obtain either (i) $0\leq x< \tau$ or (ii) there exist finitely many $n_1,n_2, \dots, n_r \in \mathbb{Z}^+$ such that

This means that $x$ is covered either by the associated closed interval of $u^{(1)} = a$ or $u^{(n_1)}u^{(n_2)}\cdots u^{(n_r)}v$ where $v$ is an unknown word. By (7), it would be easy to see that $v$ could be $a$, $b$, $ba$ and $bb$.

Based on the discussion above, we could obtain the following facts.

Lemma 2.12. If $n_r > 1$, then $v = a$. If $n_r = 1$, then $v = b$. Moreover, we have

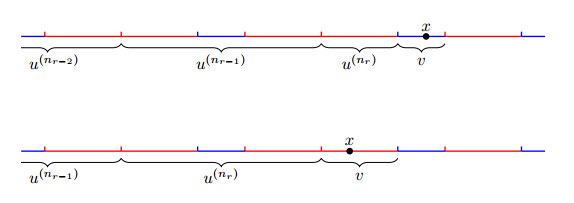
Proof. One could immediately have the expression of $\alpha(x)$. It then suffices to analyze the position of $x$ in the quasi-crystal into the following two cases (see Figure 1):

Case 1.: If $n_r = 1$ and so $n_r+1 = 2$, we have either $x-\tau^{n_1}-\cdots-\tau^{n_{r-1}}-\tau^{n_r} = 0$, that is $x$ is just both the right end point of the associated interval of $a$ and the left end point of the associated interval of $b$ or $0< x-\tau^{n_1}-\cdots-\tau^{n_{r-1}}-\tau^{n_r} <\tau$, that is $x$ is inside the associated interval of $b$. In both cases, we have $\beta(x) - \alpha(x) = 1$.

Case 2.: If $n_r > 1$, we obtain $u^{(n_r +1)} = u^{(n_r)} u^{(n_r -1)}$ due to (2), that is, $x$ is covered by $u^{(n_1)}\cdots u^{(n_r-1)}u^{(n_r+1)}$ but not $u^{(n_1)}\cdots u^{(n_r-1)}u^{(n_r)}$. Therefore, $v$ must be a front part of $u^{(n_r-1)}$. Then the first letter of $v$ is $a$ and $v = a$ and so $\beta(x) - \alpha(x) = \tau$.

Notice that we have defined $\alpha(x)$ and $\beta(x)$ only for $x\geq0$. Since $w = \widetilde{u}ba|u$, we can extend $\alpha(x)$ and $\beta(x)$ on the entire real line $\mathbb{R}$ by

Let $\zeta:\mathbb{R}\rightarrow \mathbb{R}$ be a function (see Figure 2 below) given by

It is easy to check that $\zeta$ is $C^1$ everywhere and $C^2$ except at $x = \pm1/3$. The potential $V:\mathbb{R}\rightarrow \mathbb{R}$ of the interaction (see Figure 3 below) is defined by

Lemma 2.13. The potential $V$ is $S$-equivariant with range $1$.

Proof. Fix $x,y\in\mathbb{R}$. if

then

If $\alpha(x)-x>-1$, then $\alpha(x)\in S\cap B_1(x)$. Hence $\alpha(y)\in S\cap B_1(y)$ and $\alpha(x)-x = \alpha(y)-y$.

If $\alpha(x)-x\leq-1$, then $\beta(x)-\alpha(x)>x-\alpha(x)\geq1$. Since $\alpha(x), \beta(x)\in S$, we have $\beta(x)-\alpha(x) = \tau$. Similarly, we also have $\beta(y)-\alpha(y) = \tau$. Thus $\beta(x)-x = \alpha(x)-x+\tau\leq\tau-1<1$. Then $\beta(x)-x = \beta(y)-y$. Hence $\alpha(x)-x = (\beta(x)-x)-(\beta(x)-\alpha(x)) = (\beta(y)-y)-(\beta(y)-\alpha(y)) = \alpha(y)-y$.

Hence we always have $\alpha(x)-x = \alpha(y)-y$. Similarly, $\beta(x)-x = \beta(y)-y$. Therefore, by the definition of the function $V$, we have $V(x) = V(y)$.

Lemma 2.14. The potential $V$ is not periodic.

Proof. The potential $V(x)$ reaches the maximum $160/27$ if and only if $x\in S$. If the potential $V$ had a positive period $T$, then we must have $S+T\subset S$, which is impossible since $S$ is non-periodic.

So far, we have constructed the interaction function $H$ in (5) where the potential $V$ is given by (8).

3.   The explicit one-dimensional example and its multiple solutions

Our goal in this section is to present the one-dimensional Fibonacci Frenkel-Kontorova model, i.e. the interaction function $H$ given in the above section, for which we can obtain minimal configurations, equilibrium configurations and multiple equilibria with or without any rotation number.

3.1.   Minimal configurations

In this section, we aim to find a minimal configuration with rotation number $(3\tau+1)/2$ where such minimal configurations exist according to [7]. Here we will give a more concrete construction.

3.1.1.   Local shapes of the Fibonacci quasi-crystal

For any $x\in\mathbb{R}$, the translation $S-x$ of $S$ is still a quasi-crystal. Let $S+\mathbb{R} = \{S-x\mid x\in\mathbb{R}\}$ be the collection of all translations of $S$.

Let $P$ be a given patch of quasi-crystal $S$ and $U$ be a subset in $\mathbb{R}$. The cylinder set $\Omega_{P,U}$ is the set of all quasi-crystals in $S+\mathbb{R}$ that contain a copy of $P$ translated by an element of $U$, that is,

In particular, we denote $\Omega_P: = \Omega_{P,\{0\}}$. It is easy to check that $\Omega_{P, U} = \bigcup_{u\in U}(\Omega_P-u)$.

For any integer $l\geq 1$, let

We also use the corresponding symbolic words to represent these patches, for example:

Let $C_l: = \Omega_{c_l}$, $\mathcal{E}_{l,1}: = \Omega_{\varepsilon_{l,1}}$ and $\mathcal{E}_{l,2}: = \Omega_{\varepsilon_{l,2}}$. Obviously, $\mathcal{E}_{l,1}$ and $\mathcal{E}_{l,2}$ are subsets of $C_l$.

Remark 4. The definitions of patches $c_l,\varepsilon_{l,1}$ and $\varepsilon_{l,2}$ above are motivated as follows. Consider the function

The patches translated from $c_l$ are distributed over the real line and we use the function $\mathcal{L}^l$ to measure the distance between every pair of adjacent patches. In the following Lemma 3.1, we will show that the distance can only take two values $\tau^{2l+1}$ and $\tau^{2l+2}$, i.e., the range of $\mathcal{L}^l$ is $\{\tau^{2l+1},\tau^{2l+2}\}$. Then, it is easy to see that $\varepsilon_{l,1}$ and $\varepsilon_{l,2}$ are two different patches which both start and end with $c_l$ and do not have any other $c_l$ in between.

For any integer $l\geq 1$, we define the following point sets of $\mathbb{R}$:

For any $x\in\mathbb{R}$, let $\alpha_l(x)$ be the largest number in $S^l$ such that $\alpha_l(x)\leq x$ and $\beta_l(x)$ be the least number in $S^l$ such that $\beta_l(x)>x$.

Lemma 3.1. For each $l\geq1$,

(i) $C_l = \mathcal{E}_{l,1}\sqcup\mathcal{E}_{l,2}$,

(ii) $S+\mathbb{R} = \Omega_{\varepsilon_{l,1},[0,\tau^{2l+1})}\sqcup\Omega_{\varepsilon_{l,2},[0,\tau^{2l+2})}$.

Proof. (1). Firstly, we show that the range of $\mathcal{L}^l$ is $\{\tau^{2l+1},\tau^{2l+2}\}$. The Fibonacci quasi-crystal $S$ is the corresponding geometric word of the symbolic word $\omega$ in Section 2.1.1. Then the substitution rule $\rho^2$ defined on any subword of $\omega$ can be also defined on any patches of $S$ by the connection between geometric words and symbolic words. Since $\rho^2(w) = w$, the image of a patch of $S$ under $\rho^2$ is still a patch of $S$. In particular, we have

that is, $c_{l+1} = \rho^2(c_l)$. Hence $\mathcal{L}^{l+1}(\mathcal{T}) = \tau^{2}\mathcal{L}^{l}(\mathcal{T})$ for all $\mathcal{T}\in C_{l+1}$. We only need to show the claim when $l = 1$. Notice that $\varepsilon_{1,1} = ba|abaab = c_1aab = ba|ac_1$, we have $\mathcal{L}^{1}(\mathcal{E}_{1,1}) = \{\tau^3\}$. It is also easy to see that $\mathcal{L}^{1}(\mathcal{E}_{1,2}) = \{\tau^4\}$. We claim that

In fact, for any $t\in(0, \tau^3)$ and any $\mathcal{T}\in C_l$, if $\mathcal{T}-t\in C_l$, then the corresponding symbolic word of $\mathcal{T}-t$ must be $\cdots ba|ab\cdots$. Then the corresponding symbolic word of $\mathcal{T}$ could only be $\cdots baab|\cdots$ or $\cdots baa|b\cdots$, which is impossible since $\mathcal{T}\in C_l$. For $t\in(\tau^3,\tau^4)$, in the same way, the corresponding symbolic word of $\mathcal{T}$ could only be $\cdots baab\cdot\cdot|\cdots$ or $\cdots baab\cdots|\cdots$. Furthermore, the word $\cdots baab\cdots|\cdots$ must be $\cdots baabaaa|\cdots$, which is impossible since $aaa$ is not a subword of $w$. Hence the corresponding symbolic word of $\mathcal{T}$ could only be $\cdots baab\cdot\cdot|\cdots$. Since $\mathcal{T}\in C_l$, its corresponding symbolic word is $\cdots baabba|ab\cdots$, which is impossible since $bb$ is not a subword of $w$. If $t\in (\tau^4, +\infty)$, the corresponding symbolic word of $\mathcal{T}$ could only be $\cdots baabPba|ab\cdots$. the subword $P$ formed by more than one letters must be like $ababa\cdots aba$ since $bb$ and $aa$ are not subwords of $P$. In fact, if $aa$ is a subword of $P$, then $baab$ is a subword of $\cdots P\cdots$, which contradicts that $\mathcal{T}-s\not\in C_l, \ \forall s\in(0,t)$. Then the word $\cdots baabPba|ab\cdots$ has subword $ababab$ which is impossible since $aaa$ is not a subword of $\omega$ and $\rho(aaa) = ababab$. Hence the range of $\mathcal{L}^1$ is $\{\tau^3,\tau^4\}$. It is obvious that

Since $\varepsilon_{l+1,i} = \rho^2(\varepsilon_{l,i})$, $i\in\{1,2\}$, by induction, we get (i).

(2). Recall the above definition of $\alpha_l$ and $\beta_l$. We have proved that $\beta_l(x)-\alpha_l(x)$ is $\tau^{2l+1} \text{ or } \tau^{2l+2}$. If $\beta_l(x)-\alpha_l(x) = \tau^{2l+1}$, then

If $\beta_l(x)-\alpha_l(x) = \tau^{2l+2}$, then

For any $\mathcal{T} = S-x\in S+\mathbb{R}$, we have $\mathcal{T}-(x-\alpha_l(x)) = S-\alpha_l(x)$ and thus

The proof of the opposite inclusion is obvious and so we complete the proof of (ii).

3.1.2.   The frequencies of the local shapes

If $\beta_l(x)-\alpha_l(x) = \tau^{2l+1}$, then we denote the corresponding symbolic word of $S\cap[\alpha_l(x),\beta_l(x)]$ by $A_l$. If $\beta_l(x)-\alpha_l(x) = \tau^{2l+2}$, then we denote the corresponding symbolic word of $S\cap[\alpha_l(x),\beta_l(x)]$ by $B_l$. For example,

Notice that $A_2 = A_1B_1$ and $B_2 = A_1B_1B_1$. Since $\varepsilon_{l+1,i} = \rho^2(\varepsilon_{l,i})$ for $i\in\{1,2\}$, we have $A_{l+1} = \rho^2(A_l)$ and $B_{l+1} = \rho^2(B_l)$. Hence we have $A_{l+1} = A_l B_l$ and $B_{l+1} = A_l B_l B_l$ for all $l\geq 1$ by induction. Let

then

Consider the two limits

where $|\cdot|$ is the assignment function defined in Section 2.1.1. Since the symbolic word of $S\cap[-\tau^4,\tau^3]$ is $B_1|A_1$, the absolute frequency of $A_l$ at $0$ is the limit $1/(\sqrt{5}\tau^{2l+2})$ above. In the same way, the absolute frequency of $B_l$ at $0$ is $1/(\sqrt{5}\tau^{2l+1})$.

3.1.3.   The construction of a minimal configuration

For any integer $l\geq 1$, let $\mathcal{B}_l$ be an oriented 1-dimensional branched manifold (see Figure 4 below) in $\mathbb{R}^2$, which consists of two circles $\gamma_{l,1}$ and $\gamma_{l,2}$ that are tangent to one another at the tangent point $R_l$. In fact, this branched manifold is the Anderson-Putnam complex [11]. The circumferences of $\gamma_{l,1}$ and $\gamma_{l,2}$ are $\tau^{2l+1}$ and $\tau^{2l+2}$, respectively. Given two points $\xi$ and $\eta$ on the same circle, the oriented length of the arc from $\xi$ to $\eta$ is denoted by $d(\xi,\eta)$. Let $m_{l,i}(x)$ denote the point on $\gamma_{l,i}$ such that $d(R_l,m_{l,i}(x)) = x$, $i\in\{1,2\}$.

For any $l\geq1$, we define the map $\kappa_l:\mathcal{B}_{l+1}\rightarrow \mathcal{B}_l$ which is illustrated by Figure 5 below:

And the projection $\pi_l: \mathbb{R}\rightarrow \mathcal{B}_l$ is defined by

On the one hand, since $A_{l+1} = A_l B_l$ and $B_{l+1} = A_l B_l B_l$ for all $l\geq 1$, we have $\kappa_l\circ\pi_{l+1} = \pi_l$. On the other hand, since the projection $\pi_l$ is a covering map from $\mathbb{R}$ to $\mathcal{B}_{l}$ and $\pi_l(S^l) = \{R_l\}$, the preimage $(\pi_l)^{-1}(y)$ of each given point $y$ is a point set in $\mathbb{R}$, and $S\cap \overline{B_1(x)}$ is the same patch up to translations for any point $x$ in $(\pi_l)^{-1}(y)$. Therefore, the value of $V$ is the same on $(\pi_l)^{-1}(y)$ by Lemma 2.13. Such property of $V$ allows us to define the potential on $\mathcal{B}_l$ by $\widehat{V}: = V\circ(\pi_l)^{-1}:\mathcal{B}_l\rightarrow \mathbb{R}$ and the function $\widehat{H}:\gamma_{l,1}\times\gamma_{l,1}\cup\gamma_{l,2}\times\gamma_{l,2}\rightarrow \mathbb{R}$ which maps $(\xi,\eta)$ to $\frac{1}{2}d(\xi,\eta)^2+\widehat{V}(\xi).$

Now we construct the minimal configuration.

Step 1. Fix $l = 1$. For each $i\in\{1,2\}$, let $b_{1,i}$ be a point of $\gamma_{1,i}\backslash \{R_1\}$, and as in Section 2.2 we extend $\widehat{H}$ acting on the triple segment $(R_1, b_{1,i}, R_1)$ and obtain:

One can easily see that $\widehat{H}(R_1, b_{1,i}, R_1)$ reaches its minimum at $b_{1,i}^*: = m_{1,i}(\tau^{2+i})$ which is the antipodal point of $R_1$ on $\gamma_{1,i}$ since $d(R_1,b_{1,i}^*) = \tau^{2+i}/2$ and the nonnegative potential $V$ vanishes at $\tau^{2+i}/2$. We can then construct a bi-infinite increasing sequence $(\theta_{1,n})_{n\in\mathbb{Z}}$ of $\mathbb{R}$ with $\theta_{1,0} = 0$ such that

This is because the pre-image of $\{R_1,b_{1,1}^*, b_{1,2}^*\}$ under $\pi_1$ is a discrete countable subset of $\mathbb{R}$. We thus obtain a configuration $(\theta_{1,n})_{n\in\mathbb{Z}}$ on $\mathbb{R}$ which is minimal on each segment $[\alpha_1(x),\beta_1(x)]$.

Step 2. For any $l\geq 1$ and each $i\in\{1,2\}$, we first define the number $N_{l,i}$ by iteration

Note that $(N_{l,1}, N_{l,2}) = (2f_{2l-2},2f_{2l-1})$, where $\{f_i\}$ is the Fibonacci number defined in Section 2.1.1.

We now extend $\widehat{H}$ acting on the segment $(R_l, b_{l,i,1}, \dots, b_{l,i,N_{l,i}-1}, R_l)$ where $b_{l,i,1}, b_{l,i,2},\dots, b_{l,i,N_{l,i}-1}$ are $N_{l,i}-1$ different points in $\gamma_{l,i}\backslash\{R_l\}$, and we require that the subscript $j$ of the point $b_{l,i,j}$ increases along the orientation of the circle $\gamma_{l,i}$. Then $\widehat{H}(R_l, b_{l,i,1}, \dots, b_{l,i,N_{l,i}-1}, R_l)$ reaches its minimum at $(b_{l,i,1}, \dots, b_{l,i,N_{l,i}-1} ) = (b_{l,i,1}^*, \dots, b_{l,i,N_{l,i}-1}^*)$.

Similarly as before, since pre-image $(\pi_l)^{-1}(\{R_l,b_{l,i,j}^*\mid 1\leq j\leq N_{l,i}-1\})$ is a discrete countable subset of $\mathbb{R}$, we can obtain a bi-infinite increasing sequence $(\theta_{l,n})_{n\in\mathbb{Z}}$ on $\mathbb{R}$ with $\theta_{l,0} = 0$ which is minimal on each segment $[\alpha_l(x),\beta_l(x)]$.

Step 3. By the two steps above we get a sequence of configurations $(\theta^m)_{m\in \mathbb{Z}^+} = ((\theta_{m,n})_{n\in\mathbb{Z}})_{m\in\mathbb{Z}^+}$ (see Figure 6 for numerical simulations of equilibrium configurations with $m = 5$). In the subsequent two sections, we will show that this sequence is in a compact subset of $\mathbb{R}^\mathbb{Z}$ and its accumulation point with respect to $m\rightarrow + \infty$ is a minimal configuration with rotation number $(3\tau+1)/2$.

3.1.4.   Combinatorics of minimal configurations

Proposition 1 ([4]). Let $(\theta_1, \dots,\theta_n)$ be a minimal segment and let $I$ be an interval in $[\theta_1,\theta_n]$, then there exists an integer $m\in \mathbb{Z}^{+}\cup\{0\}$ such that for any pair of disjoint intervals $I_1 = I+u_1$ and $I_2 = I+u_2$ in $[\theta_1,\theta_n]$ which satisfy that for each $\theta$ in $I$ and $k = 1, 2$:

the cardinality $\mathit{{Card}}(I_k\cap (\theta_1, \dots,\theta_n))\in \{m,m+1,m+2\}$ for $k = 1,2$.

Corollary 1. For each $l\geq 1$ and $i = 1,2$, let $u\in \mathbb{R}^\mathbb{Z}$ be a minimal configuration(resp. let $(u_p, \dots, u_q)$ be a minimal segment), then for any two connected component $I_1, I_2$ of $\pi_{l}^{-1}(\gamma_{l,i})$(resp. any two connected component $I_1, I_2$ of $\pi_{l}^{-1}(\gamma_{l,i})$ which does not intersect $(-\infty, u_p]\cup[u_q, +\infty)$), we have

(resp.

3.1.5.   The proof of item (i) of Theorem 1.1

Lemma 3.2. For each $l\geq 1$, $(\theta_{l,n})_{n\in\mathbb{Z}}$ has rotation number $(3\tau+1)/2$.

Proof. Let $n_{l,i}$ be the number of times $\pi_l([\theta_{l,0},\theta_{l,n}])$ covers completely the circle $\gamma_{l,i}$. Then

and

Thus

Then the rotation number $\rho_l$ of $(\theta_{l,n})_{n\in\mathbb{Z}}$ is the limit (if exists):

When $n$ goes to $+\infty$ the quantity

goes to the absolute frequency of $A_{l}$ if $i = 1$ or of $B_{l}$ if $i = 2$. Hence

Lemma 3.3. There exists $M>0$ such that for any $l\geq 1$, $n\in\mathbb{Z}$, we have

Proof. Let $M(m) = 2|\mathcal{B}_m| = 2\tau^{2m+3}$, where $m\geq 2$. Suppose by contradiction that there exist $l(m)$ and $n(m)$ such that

Then there exists minimal segment

such that

Let $n_{l(m),i}$ be the number of times $\pi_{m-1}([\theta_{l(m),0},\theta_{l(m),n}])$ covers completely the circle $\gamma_{m-1,i}$. Since $\kappa_{m-1}(\gamma_{m,i}) = \mathcal{B}_{m-1}$, let $\kappa_{m-1}$ act on each side then we have

By Corollary 1, for each connected component $I_{m-1,i}$ of $\pi_{m-1}^{-1}(\gamma_{m-1,i})$, we have

Since $\theta_{l(m)}$ has rotation number $(3\tau+1)/2$, for all $m\geq 2$, we have

Notice that $\lim_{n\to+\infty}\frac{n_{l(m),1}+\tau n_{l(m),2}}{2n_{l(m),1}+2 n_{l(m),2}+4}$ equals either $1/2$ or $\tau/2$ and so the right side of the inequality goes to $+\infty$ when $m\rightarrow +\infty$, which is impossible.

By Lemma 3.3, for each $l\geq1$, the distance $|\theta_{l,n+1}-\theta_{l,n}|$ is bounded for all $n\in\mathbb{Z}$. Therefore, all the configurations $((\theta_{m,n})_{n\in\mathbb{Z}})_{m\in\mathbb{Z}^+}$ are contained in a compact subset of $\mathbb{R}^\mathbb{Z}$, which guarantees that there exists at least one accumulation point as $m\rightarrow +\infty$. We denote by $(\theta_{\infty,n})_{n\in\mathbb{Z}}$ any of these accumulation points.

Theorem 3.4. The configuration $(\theta_{\infty,n})_{n\in\mathbb{Z}}$ is a minimal configuration with rotation number $(3\tau+1)/2$.

Proof. It is easy to show that $(\theta_{\infty,n})_{n\in\mathbb{Z}}$ is minimal. In fact, for any finite segment $(\theta_{\infty,j}, \cdots, \theta_{\infty,k}), k>j$ which is a limit point of minimal segments of $(\theta_{m,n})_{n = j}^k$ as $m\rightarrow +\infty$, it is straightforward to show that this segment is also minimal.

We just need to show its rotation number is $(3\tau+1)/2$. Let $n_{\infty,l,i}$ be the number of times $\pi_l([\theta_{\infty,0},\theta_{\infty,n}])$ covers completely the circle $\gamma_{l,i}$. Then

and by Corollary 1, we have

Thus

When $n$ goes to $+\infty$, the quantity

goes to the absolute frequency of $A_{l}$ if $i = 1$ and of $B_{l}$ if $i = 2$. Then for every $l\geq 1$, the rotation number $\rho_\infty$ of $(\theta_{\infty,n})_{n\in\mathbb{Z}}$ satisfies

Let $l\rightarrow +\infty$, and we obtain

3.2.   Equilibrium configurations close to the anti-integrable limit

In this section, we will first find an equilibrium configuration $(u_i)_{i\in\mathbb{Z}}$ with rotation number $(3\tau+1)/2$ satisfying

where $\Delta$ denotes the discrete Laplacian. Then a specific calculation of such configuration will be given.

To start with, let $h:\mathbb{Z}\rightarrow \mathbb{R}$ be defined by

and let $g:\mathbb{Z}\rightarrow \mathbb{R}$ be defined by

Since the distance between any pair of adjacent points in $S$ is $1$ or $\tau$, the closed ball with diameter $\tau$ centered at $h(i)$ must contain some point of $S$, which means that $|g(i)-h(i)|\leq\tau/2$ for all $i\in\mathbb{Z}$. Now we start to find an equilibrium configuration in our context. First we reduce the existence of equilibria to a fixed point of a contraction mapping on a closed neighborhood of $(g(i))_{i\in\mathbb{Z}}$ with the metric $\delta(u, u^{\prime}): = \sup _{i \in \mathbb{Z}}|u_{i}-u_{i}^{\prime}|$. More precisely, we consider a space $\Pi$ defined by

and a mapping $\Phi$ on $\Pi$ defined by

Notice that for all $u\in\Pi$, we have

Thus, we get $|\Phi(u)_{i}-g(i)| = \frac{1}{128}|(\Delta u)_i|\leq\frac{\tau}{62}$, so the mapping $\Phi$ is well defined. Moreover, $\Phi$ is a contraction mapping since

Hence by the contraction mapping principle, $\Phi$ has a unique fixed point $u$ satisfying

Now it only remains to show that formula (10) and (9) are equivalent. In fact, the above fixed point $u$ satisfies $\min\{|u_i-x|\mid x\in S\} = |u_i-g(i)|\leq \tau/62\leq 1/4$ for all $i\in \mathbb{Z}$, and hence $V'(u_i) = \frac{\text{d}}{\text{d} x}(-64(x-g(i))^2+160/27)|_{x = u_i} = -128(u_i-g(i))$. Then we can obtain (9) by multiplying by $128$ on both sides of (10), by which we can conclude that $u$ is an equilibrium configuration. What's more, since $|u_i-g(i)|\leq\frac{\tau}{62}$ and $|g(i)-h(i)|<\tau/2$ hold for all $i\in\mathbb{Z}$, the rotation number of $u$ is $(3\tau+1)/2$, the same as the slope of $h$.

In conclusion, we have obtained

Theorem 3.5. The configuration $u$ is an equilibrium configuration with rotation number $\theta$.

Next, let's calculate the equilibrium configuration $u$ we constructed. Let $\alpha = 1/128$, then (10) is equivalent to

Let $T$ be a tridiagonal operator defined by

where $\{e_i:i\in\mathbb{Z}\}$ is the orthonormal basis of the sequence space. Consider the truncation matrix

which is invertible by [9][Theorem 3.1]. And $\left\{\left\|T_{n}^{-1} e_{n}\right\|\right\}$, $\left\{\left\|T_{n}^{*^{-1}} e_{n}\right\|\right\}$ are bounded due to [4][Corollary 6.2]. Then using [12][Theorem 3.1], we have

where $y_n = (g(-n),\dots,g(0),\dots,g(n))^T$ is the truncation of $(g(i))_{i\in\mathbb{Z}}$.

Remark 5. $(i)$ In fact, the method of finding equilibrium configurations in this article can be applied to all $h$ satisfying $|(\Delta h)_i|<\infty$. For example, let

In this case, $\lim\limits_{i\to\pm\infty} h_1(i)/i = \pm\infty$ and hence the equilibrium configuration has no rotation number.

$(ii)$ See Figure 7 for numerical simulations of equilibrium configurations with or without rotation numbers.

The following theorem is a special case of [16][Theorem 1].

Theorem 3.6. Let $h:\mathbb{Z}\rightarrow \mathbb{R}$ satisfy $|(\Delta h)_i|<\infty\mathit{{for all}} i\in\mathbb{Z}.$ Then there exists a $\lambda_0$ such that for any $\lambda>\lambda_0$, there exists an equilibrium configuration $u$ of type $h$ with respect to

Remark 6. The original paper [3] considers the notion of anti-integrable limits for the classical periodic Frenkel-Kontorova models and obtains the same types of equilibrium configurations. The authors also show the chaotic properties of these "exotic" equilibrium configurations.

Moreover, in our context, we could show that there also exist non-minimal equilibrium configurations:

Theorem 3.7. Let $h:\mathbb{Z}\rightarrow \mathbb{R}$ satisfy $|(\Delta h)_i|<\infty\mathit{{for all}} i\in\mathbb{Z}.$ Then there exists $\lambda_0, \lambda_1\in \mathbb{R}$ satisfying $\lambda_1>\lambda_0$ such that for any $\lambda>\lambda_0$, there exists an equilibrium configuration $u$ of type $h$ with respect to $H_{\lambda}(\xi,\eta).$ In particular, if $\lambda>\lambda_1$, the equilibrium configuration obtained above is non-minimal.

To show Theorem 3.7, we just need a lemma:

Lemma 3.8. For any $\lambda>-4/\zeta''(0)$, if $u$ is an equilibrium configuration with respect to $H_{\lambda}$ and each component $u_i$ lies in the quadratic part of $V$ (that is, for each $u_i$, there exists a neighborhood $U$ of $u_i$ such that $V|_{U}$ is a quadratic function on $U$), then $u$ is non-minimal.

Remark 7. For the function $V$ defined in Section 2.3, it is easy to see that a point $x$ lies in the quadratic part of $V$ if and only if $\min_{y\in S}|x-y|<1/4$, where $S$ is the Fibonacci quasicrystal.

Proof. Fix $\lambda$ and $u$ that meet the conditions. Since each component $u_i$ lies in the quadratic part of $V$, we can regard the sequence $g(i)$ as the closest local maximum point of $V$ to $u_i$. Notice that

and $V'(g(i)) = 0$ for all $i\in\mathbb{Z}$, then there exists some index $i_0$ such that $u_{i_0}\ne g(i_0)$. Otherwise $(\Delta u)_i = \lambda V'(u_i) = \lambda V'(g(i)) = 0$ and thus $u_{i+1}-u_i$ is a positive constant for all $i\in\mathbb{Z}$. Since each $u_i = g(i)$ as a local maximum point of $V$ is contained in the Fibonacci chain $S$, and the distance between any two points in $S$ has the form $m\cdot\tau+n\cdot 1$, we suppose that $u_{i+1}-u_i = m\cdot \tau+n\cdot 1$ for some nonnegative integers $m$ and $n$. That is, the corresponding symbolic word of the geometric word $S\cap [u_i,u_{i+1}]$ contains $m$ $a$-letters and $n$ $b$-letters. Then the frequency of the letter $a$ in $w$ is equal to $m/(m+n)$, which is rational. However, the frequency of the letter $a$ in $S$ is $1/\tau$, which was calculated in the proof of Lemma 2.6. Without loss of generality, suppose that $i_0 = 0$. In order to show that $u$ is non-minimal, we show that $(u_{-1}, u_0, u_1)$ is non-minimal. That is, we find a $u_0': = u_0'(\lambda,u)$ such that

Before we give the value of $u_0'$, there are some relations among $u_0, g(0)$ and $\bar{u}: = (u_{-1}+u_1)/2$. Since $u_0$ lies in the quadratic part of $V$, from Taylor's formula and the condition of $\lambda$, we have

Then from (12) and (14), we know that

That is,

And from (15) we can see that the sign of $u_0-g(0)$ is different from the sign of $\bar{u}-g(0)$, thus we have

Now, we discuss three different cases and give the value of $u_0'$ in each case.

Case 1.: If $|\bar{u}-g(0)|\leq 1/2$, where $1/2$ is half of the minimal distance between two adjacent local maximum points of $V$. Let $u_0' = \bar{u}$. Consider the function $F(x) = \frac{1}{2}(u_{-1}-x)^2+\frac{1}{2}(x-u_1)^2$. $F(x)$ is a quadratic function and takes minimum at $\bar{u}$. Hence,

Since $|\bar{u}-g(0)|\leq 1/2$ and $V$ is increasing on the interval $[g(0)-1/2, g(0)]$, decreasing on the interval $[g(0), g(0)+1/2]$, and especially, strictly monotone on its quadratic parts, by (16), we have

Multiply both sides of the inequality (19) by $\lambda$ and add the inequality (18), then we have (13).

Case 2.: If $\bar{u}-g(0)> 1/2$. Let $u_0' = g(0)+ 1/3$, where $1/3$ is the radius of each bump of $V$. Then

By (17), we have

Then using the monotonicity of $F(x)$, we have

Multiply both sides of the inequality (20) by $\lambda$ and add the inequality (21), then we have (13).

Case 3.: If $\bar{u}-g(0)< -1/2$, let $u_0' = g(0)- 1/3$. The rest of the proof is the same as the one in Case 2.

Proof of Theorem 3.7. For any $h$ with $|(\Delta h)_i|<\infty\text{ for all } i\in\mathbb{Z}$, Theorem 3.6 ensures the existence of equilibrium configuration of type $h$ with respect to $H_\lambda$ for any $\lambda$ larger than some $\lambda_0$. Let $\lambda_{\frac{1}{2}} = \max\{\lambda_0, -4/\zeta''(0)\}$. Then for any $\lambda>\lambda_{\frac{1}{2}}$, the equilibrium configuration $u$ whose existence is proved in Theorem 3.6 is non-minimal by Lemma 3.8, as long as we have that each $u_i$ lies in the quadratic part of $V$.

To solve the problem of whether each $u_i$ can lie in the quadratic part of $V$, let us see the proof of Theorem 3.6 carefully. In our example of $V$, a sufficient condition is that the radius of the space that the contraction mapping is smaller than $1/4$. A possible construction of the contraction is

For all $u\in\Pi_\lambda$, since

we get

which means that $\Phi_\lambda$ is well-defined. $\Phi_\lambda$ is a contraction mapping because

Let the radius of $\Pi_\lambda$ be smaller than $1/4$, and we get a restriction on $\lambda$:

Hence, let

and then for any $\lambda>\lambda_1$, there exists a non-minimal equilibrium configuration of type $h$ with respect to $H_\lambda$.

Proof of item (ii) of Theorem 1.1. By Theorem 3.5, to show the item (ii) of Theorem 1.1, it suffices to show that $u$ is non-minimal with respect to $H_\lambda$ in (11) when $\lambda = 1$.

In fact, by the definition of the function $\zeta$, we have $-4/\zeta''(0) = 1/32<1$. Due to Theorem 3.5, we have that $u$ is an equilibrium configuration with respect to $H_{1} = H$. Since $u\in \Pi = \left\{u :|u_{i}-g(i)| \leq \frac{\tau}{62} \text { for all } i \in \mathbb{Z}\right\}$, we know that each component $u_i$ lies in the quadratic part of $V$. Using Lemma 3.8, $u$ is non-minimal.

Acknowledgments

The authors would like to thank the anonymous referees for the valuable comments and suggestions on the manuscript. We also thank Prof. R. de la Llave for a very careful reading of the manuscript and many suggestions which helped improve a lot the presentation and exposition.