Path-connectedness in global bifurcation theory

1.   Introduction

Krasnosel'skii [17] considered non-linear eigenvalues in the form

where $X$ is a real Banach space, the linear operator $L:X \to X$ is compact, and the non-linear $R:{\mathbb R} \times X \to X$ is continuous, compact, and satisfies

Since $R$ is continuous, (1.1b) implies that $R(\lambda,0) = 0$, and hence $x = 0$ is a solution of (1.1a), for all $\lambda \in {\mathbb R}$. Let $\mathcal{T} = \{(\lambda,0):\lambda \in {\mathbb R}\}$ denote the set of trivial solutions of (1.1a) and $\mathcal{S}$ the set of solutions that are not trivial. In all that follows, $L$ is linear and compact and $R$ is nonlinear, continuous and compact. The first observation is that under these hypotheses $\mathcal{S}$ may be empty.

Example 1.1. Let $X = {\mathbb R}^2$, $L(x,y) = (x+y,y)$ and $R(x,y) = (0,-x^3)$. Then $L$ is linear, (1.1b) holds, and equation (1.1a) is satisfied if and only if

which implies that $x\big( (\lambda-1)^2 + x^2\big) = 0$. Hence $x = 0$ and, by the first equation, $y = 0$, which shows $\mathcal{S} = \emptyset$.

According to Krasnosel'skii [17,Ch. Ⅳ,p. 181], a point $\lambda_0 \in {\mathbb R}$ is a bifurcation point for (1.1a) if there exists a sequence $\{(\lambda_n, x_n)\}\subset \mathcal{S}$ such that

In this definition there is no mention of curves, paths or connected sets in $\mathcal{S}$, bifurcating from $\mathcal{T}$ at $(\lambda_0,0)$. (A path in $\mathcal{S}$ is a set $\{\gamma(t): t \in [0,1]\}$ where $\gamma:[0,1]\to \mathcal{S} \subset {\mathbb R}\times X$ is continuous and a path is non-trivial if $\gamma$ is not constant. A curve is a smooth 1-dimensional manifold.)

In this paper it is shown by example that even when the operators in (1.1) are smooth, if they are not real-analytic the non-trivial solution sets predicted by classical theories may not be path-connected, and indeed may contain no paths at all.

1.1.   Bifurcation theory background

A necessary condition for bifurcation. The following necessary criterion for $\lambda_0$ to be a bifurcation point, when $L$ is compact and $R$ satisfies (1.1b) in a Banach space $X$, is well known [17,Ch. Ⅳ,§2,Lem. 2.1]. A real number $\lambda_0\neq 0$ is a bifurcation point only if $\lambda_0$ is an eigenvalue of $L$. If $X$ is finite-dimensional and $\lambda_0 = 0$ is a bifurcation point, then 0 is an eigenvalue of $L$. From Example 1.1 a real eigenvalue of $L$ need not be a be bifurcation point.

Multiplicities. The geometric multiplicity of an eigenvalue $\lambda_0$ of $L$ is the dimension of the eigenspace $\ker(\lambda_0I-L)$, and its algebraic multiplicity is the dimension of $\cup_{k\in {\mathbb N}}\ker (\lambda_0I-L)^k$. When the algebraic multiplicity is one, and either $\lambda_0\neq 0$ or $X$ is finite-dimensional, $\lambda_0$ is called simple. Both the multiplicities of all non-zero eigenvalues of compact operators are finite.

Bifurcation results by classical analysis. Many seemingly different bifurcation phenomena were studied in ad hoc situations before being recognised by Crandall & Rabinowitz [6] as special cases of the following overarching result, which is a corollary of the Implicit Function Theorem.

Theorem 1.2. Bifurcation from a simple eigenvalue [6] Suppose that $\lambda_0$ is a simple eigenvalue of $L$, that $R:{\mathbb R} \times X \to X$ is continuously differentiable, and that $\partial_{x\lambda}R$ exists and is continuous in a neighbourhood of $(\lambda_0,0)$. Then there is an injective, continuous function $\gamma:(-1,1) \to {\mathbb R} \times X$ such that $\gamma (0) = (\lambda_0,0)$ and a neighbourhood $U$ of $(\lambda_0,0)$ such that $U \cap \mathcal{S} = \{\gamma(s):s\in (-1,0)\cup (0,1)\}$. If $\partial_{xx}R$ is also continuous in the neighbourhood of $(\lambda_0,0)$, then $\gamma$ is $C^1$.

Remark. In Example 1.1 the nonlinearity $R$ satisfies the hypotheses in Theorem 1.2, and the only eigenvalue of $L$ is $\lambda_0 = 1$ which has geometric multiplicity 1, but $\lambda_0 = 0$ is not a bifurcation point. However Theorem 1.2 does not apply because the algebraic multiplicity of $\lambda_0$ is 2. Henceforth the word multiplicity always refers to algebraic multiplicity.

Bifurcation results by topological methods. In his D.Sc thesis (Kiev 1950 [16]), Krasnosel'skii used Leray-Schauder degree theory to prove, under the hypotheses of (1.1), that every non-zero eigenvalue of $L$ with odd multiplicity is a bifurcation point [15,Thm. 2], [17,Ch. Ⅳ,Thm. 2.1]. Then, in 1971, Rabinowitz reached the ground-breaking conclusion that this bifurcation is not local: indeed, under Krasnosel'skii's hypotheses, he showed a global connected set of non-trivial solutions bifurcates in ${\mathbb R} \times X$ from $\mathcal{T}$ at an eigenvalue of odd multiplicity.

Theorem 1.3. Global bifurcation at eigenvalues of odd multiplicity. [18,Thm. 1.3]. Suppose $L$ and $R$ are as in (1.1) and $\lambda_0$ is a non-zero eigenvalue of $L$ of odd multiplicity. Then $\lambda_0$ is a bifurcation point and there exists a connected subset $\mathcal C$ of $\mathcal{S}$ such that $(\lambda_0,0)\in \overline{ \mathcal{C}}$ and either $\mathcal{C}$ is unbounded in ${\mathbb R} \times X$ or there exists $(\lambda_1,0) \in \overline{ \mathcal{C}}$ where $\lambda_1 \neq \lambda_0$ is also an eigenvalue of odd multiplicity of $L$. (If $X$ is finite-dimensional, the result holds when $\lambda_0 = 0$ is an eigenvalue of odd multiplicity.)

Related Results. Dancer [9] used topological obstruction arguments to extend the topological account of bifurcation at simple eigenvalues and eigenvalues of geometric multiplicity one. Krasnosel'skii [17,Ch. Ⅳ.5,p.232 ff.] also studied bifurcation at eigenvalues of even multiplicity, when the nonlinearity $R$ is non-degenerate in a certain sense. Under his hypotheses, the methods introduced by Rabinowitz [18] lead easily to global bifurcation at eigenvalues of even multiplicity for certain nonlinearities [21]. The methods of Section 2 are relevant in that context also.

Bifurcation results by variational methods. To justify linearisation without reference to multiplicity of eigenvalues, Krasnoselskii [17,§Ⅵ] developed a variational approach to bifurcation theory in Hilbert space. (For an up-to-date account in Banach spaces, see [19].) Let $X$ be a real Hilbert space with inner product $\langle\cdot, \cdot\rangle$ and let $h:X \to {\mathbb R}$ be differentiable with derivative $Dh[x]:X \to {\mathbb R}$ at $x \in X$. Then $Dh[x]$ is a bounded linear operator on $X$ and, by the Riesz Representation Theorem, there exists a unique $x^* \in X$ such $Dh[x]y = \langle x^*, y\rangle$ for all $y \in X$. Hence $\nabla h(x) = x^*$ defines an operator $\nabla h:X \to X$, called the gradient of $h$, and an operator $H:X \to X$ is said to have gradient structure if $H = \nabla h$ for some differentiable $h:X \to {\mathbb R}$.

It is easily seen that a bounded linear operator $L:X \to X$ has gradient structure if and only if $\langle Lx,y\rangle = \langle x,Ly\rangle$ for all $x,y \in X$. In other words $L$ is a gradient if and only if it is self adjoint, in which case $Lx = \nabla \ell (x)$ where $\ell(x) = { {\frac12}} \langle Lx,x\rangle,\,x\in X$. Note that when $L$ is self-adjoint, $(L-\lambda I)^2 x = 0$ implies

and hence algebraic multiplicity and geometric multiplicity of eigenvalues coincide for self-adjoint operators. Obviously the identity operator $I$ on $X$ has gradient structure $I = \nabla \iota$ where $\iota(x) = { {\frac12}} \|x\|^2$. Finally, a function $g:X \to {\mathbb R}$ is weakly continuous if $g(x_k) \to g(x_0)$ in ${\mathbb R}$ for every weakly convergent sequence $x_k \rightharpoonup x_0$ in $X$. Vainberg proved [22,Thm. 8.2] that $g$ is weakly continuous when its gradient is a compact operator. In this setting, a special case of (1.1a) is

where $X$ is a real Hilbert space, $L:X \to X$ is self-adjoint, and $R$ satisfies (1.1b) with gradient structure independent of $\lambda$:

Krasnosel'skii proved [17,Ch. Ⅵ,§6,Thm. 2.2,p. 332] that for this class of problems bifurcation occurs at all non-zero eigenvalues of $L$, independent of multiplicity. The following version of his theorem replaces his hypothesis that "$R$ is uniform differentiable" near 0 with Vainberg's characterisation [22,Thm. 4.2,p. 45] of uniform differentiability in terms of the bounded uniform continuity of its Fréchet derivative.

Theorem 1.4. Variational theory of bifurcation at any eigenvalue. If $R$ in (1.2) has Fréchet derivative $x \mapsto dR[x]$ bounded and uniformly continuous in a neighbourhood of 0 in $X$, all eigenvalues $\lambda_0\neq 0$ of $L$ are bifurcation points. (When $X$ is finite-dimensional, the condition $\lambda_0 \neq 0$ is not needed.)

Remark 1.5. While Rabinowitz's theory of global bifurcation yields connected sets $\mathcal{C} \subset \mathcal{S}$ bifurcating from $\mathcal{T}$ at eigenvalues of odd multiplicity, Böhme's example [3,§6] showed that no such connectedness is guaranteed by Theorem 1.4.

Bifurcation results by real-analyticity. So far in this summary of bifurcation theory, Theorem 1.2 is the only result which guarantees the existence of a path of non-trivial solutions of equation (1.1), and even then it is localized to a neighbourhood $(\lambda_0,0)$, where $\lambda_0$ is the bifurcation point. However, in his Ph.D thesis (Cambridge 1972) Dancer [7,8] showed, among many other things, that when the operators in (1.1a) are real-analytic (infinitely differentiable and equal to the sum of the Taylor series in a neighbourhood of every point), there bifurcates from a simple eigenvalue a global path of solutions which, at every point, has a local real-analytic re-parametrization. More precisely, Dancer showed that the global continuum, which by Theorem 1.3 bifurcates from the trivial solutions at a simple eigenvalue, contains a path $\mathcal K = \{(\Lambda(s), \kappa (s)): s \in [0,\infty)\}\subset {\mathbb R} \times X$ with the following properties.

(i) $\Lambda(0) = \lambda_0\in {\mathbb R}$, $\kappa(0) = 0 \in X$ and $\mathcal K\setminus \{(\lambda_0,0)\}$ is a real-analytic curve in a neighbourhood of $(\lambda_0,0)$ [4,Thm. 8.3.1].

(ii) $\mathcal K$ is either unbounded or forms a closed loop in ${\mathbb R} \times X$.

(iii) For each $s^* \in (0,\infty)$ there exists $\rho^*:(-1,1) \rightarrow {\mathbb R}$ (a re-parametrisation) which is continuous, injective, and

This does not imply that $\mathcal K$ is locally a smooth curve. (The map $\sigma:(-1,1) \rightarrow {\mathbb R}^2$ given by $\sigma (t) = (t^2,t^3)$ is real-analytic and its image is two curves forming a cusp.) Nor does it preclude the possibility of secondary bifurcation points on $\mathcal K$. In particular, since $(\Lambda,\kappa):[0,\infty) \rightarrow {\mathbb R} \times X$ is not required to be globally injective; self-intersection of $\mathcal K$ (as in a figure eight) is not ruled out.

(iv) Secondary bifurcation points and points where the bifurcating branch is not smooth, if any, are isolated.

Under these hypotheses the real-analytic implicit function theorem [4,§4.5] can be used as in the proof of Theorem 1.2 to obtain a real-analytic curve of solutions which intersects the trivial solutions at $(\lambda_0,0)$. Dancer used the theory of real-analytic varieties to show that this observation has a global extension: when the operators are real-analytic there bifurcates from a simple eigenvalue a global path of solutions which is a real-analytic curve except possibly at a discrete set of points. This path is unique in the sense that it has a pre-determined continuation through secondary bifurcation points, or even through points where it intersects higher-dimensional manifolds of solutions. See [4] for an introductory account.

2.   Lack of path-connectedness - three examples

The following three examples are designed to illustrate how the situation may differ from Dancer's theory when the hypotheses of Theorems 1.2, 1.3 and 1.4 are satisfied by operators that are infinitely differentiable but not real-analytic. The main conclusion is that the non-trivial solution set $\mathcal{S}$ may contain global connected sets while having no path-connected components except singletons. Since no two non-trivial solutions in such a connected set can be joined by a path in $\mathcal{S}$, this possibility has serious implications for applications. The paper ends with a simple criterion for a connected set to contain a path joining two of its points. Thus Theorem 6.5 gives an insight into the lack of path-connectedness in connected sets.

In the first two examples of (1.1), $X = {\mathbb R}$, $L = 0$, $\lambda_0 = 0$ is the only eigenvalue of $L$ and is simple, and $R = r:{\mathbb R}^2 \to {\mathbb R}$, where $r$ is at least continuously differentiable and satisfies (1.1b). Under these hypotheses (1.1a) has the form

The first example concerns the global connected sets of solutions of (2.1) that, by Theorem 1.3, bifurcate at the simple eigenvalue $\lambda_0 = 0$, although Theorem 1.2 does not apply because $\partial_{\lambda x}r$ is not continuous at $(0,0)$.

Example A. There is a $C^1$-function $r:{\mathbb R}^2 \to {\mathbb R}$ which is infinitely differentiable on ${\mathbb R}^2\setminus \{(0,0)\}$ for which the non-trivial solution set $\mathcal{S}$ of (2.1) has no path-connected components except singletons. However by Theorem 1.3 it has an unbounded global connected set of non-trivial solutions which bifurcates at $\lambda_0 = 0$.

The second example illustrates the possible behaviour of solutions which bifurcate at $\lambda_0 = 0$ when simultaneously Theorem 1.2 yields the local bifurcation of a smooth curve, and Theorem 1.3 yields global bifurcation of an unbounded connected set, of non-trivial solutions of (2.1).

Example B. For an infinitely differentiable function $r:{\mathbb R}^2 \to {\mathbb R}$ let $\overline {\mathcal{C}}$ denote the closure of the connected sets of non-trivial solutions of (2.1) which by Theorems 1.2 and 1.3 bifurcate at $\lambda_0 = 0$. In this example $\overline {\mathcal{C}}$ is the union $\mathcal L \cup \mathcal{C}^+ \cup \mathcal{C}^-$ of three disjoint connected sets: $\mathcal L$ is the smooth curve $\{0\}\times (- { {\frac12}}, { {\frac12}})$ and $\mathcal{C}^\pm$ are closed, unbounded, connected sets in the first and third quadrants, respectively, $(\pm { {\frac12}},0) \in \mathcal{C}^\pm$ and all path-connected components of $\mathcal{C}^+\cup \mathcal{C}^-$ are singletons. (The only non-trivial paths in $\overline {\mathcal{C}}$ are subsets of the closure of $\mathcal L$.)

Although Böhme [3] showed the non-trivial solution set of (1.2) given by Theorem 1.4 when $R$ has gradient structure need not be connected, he did not exclude the possibility of it having non-trivial connected components. The next example shows that in any case all the path-connected components may be singletons.

Example C. In this example of problem (1.2), $X = {\mathbb R}^2$, $R = \nabla r$ where $r:{\mathbb R}^2 \to {\mathbb R}$ is infinitely differentiable, and $L$ is the zero operator which has only one eigenvalue, namely 0 with multiplicity 2. Then (1.2a) has the form

and the existence of non-trivial solutions with $\big(\lambda,( x,y)\big)$ near $\big(0,(0,0)\big)$ is given by Theorem 1.4. Example C shows, in addition to not forming a connected set, that all path-connected components of the non-trivial solution set may be singletons.

3.   Preliminaries

The construction of these examples depends crucially on classical results of Whitney in analysis and Knaster in point-set topology.

Theorem. (Whitney) For any closed set $G \subset{\mathbb R}^n$ there is an infinitely differentiable, globally Lipschitz continuous function $h$ such that $G = \{x \in {\mathbb R}^n: h(x) = 0\}$, and all the derivatives of $h$ are zero at every point of $G$.

Proof. Let $u:[0,\infty) \to [0,1]$ be a $C^\infty$-function with

For a closed set $G$, let the open set ${\mathbb R}^n\setminus G$ be the union of a countable collection of open balls $\{B_{r_j}(a_j):j \in {\mathbb N}\}$, with radius $r_j\in (0,1)$ centred at $a_j \in{\mathbb R}^n$, and put

Then $u_j$ is infinitely differentiable on ${\mathbb R}^n$ and positive on $B_{r_j}(a_j)$. Now, see [10,§2.7], let Lin$^k({\mathbb R}^n,{\mathbb R})$ denote the linear space of all $k$-linear maps from $({\mathbb R}^n)^k \to {\mathbb R}$ and let $\|A\|_k$ denote the norm of $A \in$ Lin$^k({\mathbb R}^n,{\mathbb R})$. Then $D^k u_j(x) \in$ Lin$^k({\mathbb R}^n,{\mathbb R})$ where $D^ku_j(x)$ is the $k^{th}$ derivative of $u_j$ at $x\in {\mathbb R}^n$, and $D^ku_j(x) = 0$ for $x \in G$ and $j\in {\mathbb N}$. Moreover, since $u_j$ is supported on $\overline{B_{r_j}(a_j)}$,

Therefore, since both series are convergent, uniformly in $x$, in their respective spaces,

and $h:{\mathbb R}^n \to [0,\infty)$ is $C^\infty$, $G = \{x\in {\mathbb R}^n: h(x ) = 0\}$, and $D^kh(x) = 0$ for all $k \in {\mathbb N}$ and $x \in G$.

A deep result in point-set topology due to Knaster (1922) concerns the possible structure of compact connected sets in metric spaces.

Definition. A continuum, which is a compact, connected set in a metric space, is indecomposable if it is not a union of two proper sub-continua, and hereditarily indecomposable if every sub-continuum is indecomposable. (See [2,5,12,13].)

Theorem. (Knaster) [14] In ${\mathbb R}^2$ there exists a hereditarily indecomposable $Q$.

Remark 3.1. Since a non-trivial path in $Q$ would be a decomposable sub-continuum, there are no non-trivial paths in $Q$. In other words, although $Q$ is compact and connected in ${\mathbb R}^2$, all its path-connected components are singletons.

A hereditarily indecomposable continuum which is snake-like (Definition 6.4) is called a pseudo-arc and all pseudo-arcs are homeomorphic [1,Thm. 1]. Since, by construction, Knaster's $Q$ is snake-like, it is in this sense the unique pseudo-arc. But all that is important here is that $Q$ is compact, connected and has no paths.

Preliminaries. Let $Q$ be a pseudo-arc and without loss of generality suppose

Now let $P = \{(\lambda, x\sin \lambda) \in {\mathbb R}^2: (\lambda,x) \in Q\}$. Then $P \subset [0,\pi] \times [- { {\frac14}}, { {\frac14}}]$,

and $P$ is a connected set (being the continuous image of a connected set) which contains no non-constant paths (since $Q$ is hereditarily indecomposable).

Since $P$ is connected, by Proposition 6.1 and Corollary 6.3, for any $\epsilon>0$ there exists an ordered set, $\{p^ \epsilon_i:1 \le i \le n_ \epsilon\} \subset P$ such that

and the union $L^ \epsilon$, of the straight line segments which join the points in order, is a continuous, piecewise-linear, non-self-intersecting path joining $(0,0)$ to $(\pi,0)$. Now define subsets of ${\mathbb R}^2$ by

and note that $\widetilde L^ \epsilon$ is an unbounded, piecewise linear, connected set which separates the plane, and each point of $\widetilde L^ \epsilon$ is within distance $\epsilon$ of a point of $\widetilde P$.

Now let $\widetilde P_c^\pm$ denote the connected components of ${\mathbb R}^2\setminus \widetilde P$ which contain the half spaces $\{(\lambda,x): \lambda\in {\mathbb R},\; \pm x > { {\frac14}}\}$, respectively.

Lemma 3.2. In the plane, $\widetilde P \subset {\mathbb R} \times \left[- { {\frac14}}, { {\frac14}}\right]$ is an unbounded, connected subset of a double cone centred on the horizontal axis with opening angle $\theta <\pi/6$. Moreover $\widetilde P$ contains no non-trivial paths, $(0,0)\in\widetilde P$, and $\widetilde P_c^+ \cap\widetilde P_c^- = \emptyset$.

Proof. From the definition, $(0,0) \in\widetilde P$ and $\widetilde P \subset {\mathbb R} \times \left[- { {\frac14}}, { {\frac14}}\right]$ is unbounded. Since $P = \{(\lambda,x\sin \lambda) \in {\mathbb R}^2: (\lambda,x) \in Q\}$ and $|x|\le { {\frac14}}$, $\widetilde P$ lies in a cone with opening angle less than $2\arctan ( { {\frac14}}) <\pi/6$. Moreover $\widetilde P$ is connected because $P_k$ is connected and $P_k\cap P_{k+1} = \{ ((k+1)\pi,0)\}$ for all $k$. Since each $P_k$ contains no paths, a non-trivial path in $\widetilde P$ must contain points $(\lambda_i,x_i)$ with $\lambda_i$ in the open intervals $(k_i\pi,(k_i+1)\pi)$, $i = 1,2$, where $k_1 \neq k_2$. However, this implies that these $P_{k_i}$ contain non-trivial paths, which is false. Hence $\widetilde P$ contains no non-trivial paths.

Now suppose $\widetilde P_c^+ \cap\widetilde P_c^- \neq \emptyset$. Then, since $\widetilde P_c^+ \cup\widetilde P_c^-$ is open and connected, it is path-connected. Therefore there exists a path $\gamma \subset \widetilde P_c^+ \cup\widetilde P_c^-$ joining $(0,-2)$ to $(0,2)$ with, since $\gamma$ is continuous, $\gamma[0,1]\subset [-K,K] \times [-K, K]$ for some $K >0$. Since, for all $\epsilon>0$, $\widetilde L^ \epsilon$ in (3.1) separates the plane, there exists

Therefore, by compactness, for a sequence $0< \epsilon_j \to 0$,

which is false since $\gamma \subset \widetilde P_c^+ \cup\widetilde P_c^-$. Hence $\widetilde P_c^+ \cap\widetilde P_c^- = \emptyset$.

4.   Construction of Examples A and B

General considerations. For $0<\alpha<\beta<\infty$, let

a double cone in the first and third quadrants. Then there exists $\omega:{\mathbb R}^2 \to {\mathbb R}$ with the following properties:

(a) $\omega(\lambda,x) = 0$ if $|x| \ge \alpha|\lambda|/2$, in particular, $\omega = 0$ on $C(\alpha,\beta)$;

(b) $\lambda\, \omega(\lambda,x) \ge 0$ on ${\mathbb R}^2$;

(c) $\omega(\lambda,0) = \lambda,\quad \lambda \in {\mathbb R}$;

(d) $\omega$ is infinitely differentiable on ${\mathbb R}^2\setminus \{(0,0)\}$;

(e) $\omega$ is globally Lipschitz continuous ${\mathbb R}^2$.

To see this, let $\varpi:{\mathbb R} \to {\mathbb R}$ be an infinitely differentiable even function which is non-increasing on $[0,\infty)$ with $\varpi(0) = 1$ and $\varpi (r) = 0$ for all $r \ge \alpha/2$. Then, for $x\in {\mathbb R}$, let

That $\omega$ satisfies (a)-(d) follows immediately from the definition and the properties of $\varpi$. Moreover, the partial derivatives at $(\lambda,x)$ are

since $\omega(\lambda,x) = 0$ when $|\lambda| \le |2|x|/\alpha$. For future reference note that

Since $\varpi'(r) = 0,\,|r|\ge \alpha/2$, the partial derivatives of $\omega$ are uniformly bounded in ${\mathbb R}^2\setminus \{(0,0)\}$, and property (e) follows.

Remark 4.1. It follows from (4.1b) and (4.1c) that $\partial_\lambda \omega$ is not continuous at $(0,0)$ and from (4.1a) and (4.1c) that $\partial_x \omega$ is not continuous at $(0,0)$. However, $(\lambda,x) \mapsto x\, \omega(\lambda,x)$ is continuously differentiable on ${\mathbb R}^2$. Note also, from the intermediate value theorem, that for any $\rho \in (0,1)$ there exists $s \in (0, \alpha/2)$ such that $\varpi(s) -s\varpi'(s)-s^2\varpi''(s) = \rho$ and hence, from (4.1d) with $x = s\lambda$, $\lambda \neq 0$, that

Thus, although $\partial_{\lambda x}(x \omega)(0,0) = \partial_{x\lambda}(x \omega)(0,0) = 0$, the mixed partial derivatives $\partial_{\lambda x} \big(x\, \omega (\lambda,x)\big)$ and $\partial_{x\lambda } \big(x\, \omega (\lambda,x)\big)$ are not continuous at $(0,0)$.

Let $D^+$ and $D^-$ denote the two disjoint components of the complement of $C(\alpha,\beta)$ which contain the positive and negative $\lambda$-axes respectively.

Definition ($\mathcal{H}$). A set $G \subset C(\alpha,\beta)$ satisfies hypothesis $( \mathcal{H})$ if it is closed and connected, its intersections with both half planes, $\{ \lambda \ge 0\}$ and $\{\lambda \le 0\}$ are unbounded, and $H^+ \cap H^- = \emptyset$, where $H^\pm$ are the connected components of ${\mathbb R}^2 \setminus G$ with $D^\pm\subset H^\pm$.

Lemma 4.2. If $G$ satisfies $( \mathcal{H})$, then $\omega \ge 0$ on $H^+$ and $\omega \le 0$ on $H^-$

Proof. This is immediate from properties (a) and (b) of $\omega$.

Lemma 4.3. When $G$ satisfies $( \mathcal{H})$ there is a locally Lipschitz continuous function $g: {\mathbb R}^2 \to {\mathbb R}$ which is infinitely differentiable on ${\mathbb R}^2 \setminus \{(0,0)\}$, with the property that $g(\lambda,0) = \lambda$ for all $\lambda \in {\mathbb R}$, and $g(\lambda,x) = 0 \mathit{\text{if and only if}} (\lambda,x) \in G.$

Proof. Since $G$ is closed, by Whitney's lemma there exists a non-negative, infinitely differentiable function $h:{\mathbb R}^2 \to [0,\infty)$ such that $h(\lambda,x) = 0$ if and only if $(\lambda,x) \in G$, and every derivative of $h$ is zero at every point of $G$. Let $\hat h:{\mathbb R}^2 \to {\mathbb R}$ be defined by

In particular, $\hat h(\lambda,x) = \pm h(\lambda,x)$, $(\lambda,x) \in H^\pm$, $\hat h$ is infinitely differentiable on ${\mathbb R}^2$, and $\hat h(\lambda,x) = 0$ if and only if $(\lambda,x) \in G$. Now with $\omega$ satisfying (a)-(e) above, let

It follows from (4.1) that $g$ is infinitely differentiable on ${\mathbb R}^2\setminus \{(0,0)\}$ and by Lemma 4.2 $g$ satisfies the conclusions of the Lemma.

Proposition 4.4. For $G$ satisfying $( \mathcal{H})$, there is a continuously differentiable function $r:{\mathbb R}^2 \to {\mathbb R}$ with $r\in C^\infty\big({\mathbb R}^2\setminus \{(0,0)\}\big)$, such that $|r(\lambda,x)|/|x| \to 0 \mathit{\text{as}}0\neq |x| \to 0$ uniformly for $\lambda$ in bounded intervals, and $G\setminus \{(0,0)\}$ is the set of non-trivial solutions of $\lambda x = r(\lambda,x)$.

Proof. For $G$ satisfying $( \mathcal{H})$ and the corresponding function $g$ in Lemma 4.3, let

Then the smoothness of $\hat h$ and the properties of $\omega$ in (4.1) imply that $g$ is infinitely differentiable on ${\mathbb R}^2\setminus\{(0,0)\}$ and, by Remark 4.1, $xg$ is continuously differentiable on ${\mathbb R}^2$ with $|r(\lambda,x)|/|x| \to 0 \text{ as }0\neq |x| \to 0$ uniformly for $\lambda$ in bounded intervals. Moreover, by construction, non-trivial solutions of (2.1) are the zeros of $g$ with $x \neq 0$. So, by Lemma 4.3, $G\setminus \{(0,0)\}$ is the set of non-trivial solutions of $\lambda x = r(\lambda,x)$ in ${\mathbb R}^2$. This completes the proof.

Remark. Since, from Remark 4.1, the mixed partial derivative $\partial_{\lambda x}r$ is not continuous at $(0,0)$, Theorem 1.2 does not apply to equation $\lambda x = r(\lambda,x)$ in this situation.

Construction of Example A of (2.1). Let $\widetilde P$ be the unbounded connected set defined in (3.1) and let $G$ denote $\widetilde P$ rotated counter-clockwise about the origin through an angle $\pi/4$. By Lemma 3.2, $G$ is connected, contains no non-trivial paths, and satisfies $( \mathcal{H})$ with $\alpha = \tan(\pi/6)$ and $\beta = \tan(\pi/3)$. With this choice of $G$, Example A is a special case of Proposition 4.4.

Construction of Example B of (2.1). This example shows that the global connected set $\mathcal{C}$ given by Theorem 1.3 need not be path connected even when all the operators involved are infinitely differentiable on ${\mathbb R}^2$ and locally, by Theorem 1.2, a smooth curve of solutions bifurcates from the trivial solutions at a simple eigenvalue.

With $\widetilde P$ defined in (3.1) let three disjoint connected sets be defined by

Then $\mathcal L$ is the smooth curve $\{0\}\times (- { {\frac12}}, { {\frac12}})$, and $\mathcal{C}^\pm$ are closed, unbounded, connected sets in the first and third quadrants respectively with $(0,\pm { {\frac12}}) \in \mathcal{C}^\pm$ and all path-connected components of $\mathcal{C}^+\cup \mathcal{C}^-$ are singletons. Let $\overline {\mathcal{C}}$ be their union

Now let $E^-$ and $E^+$ denote the connected components of ${\mathbb R}^2 \setminus \overline { \mathcal{C}}$ which contains $(-\infty,0]\times \{0\}$ and $[0, \infty)\times \{0\}$, respectively and note, from the argument for Lemma 3.2, that $E^+ \cap E^- = \emptyset$. By Whitney's result there exists a non-negative, infinitely differentiable function $h$ on ${\mathbb R}^2$ which is zero only on the closed set $\overline { \mathcal{C}}$, and at each point of $\overline { \mathcal{C}}$ all the derivatives of $h$ are zero. Let

so that $\tilde h \ge 0$ on $E^+$.

Now let $\tilde \omega: {\mathbb R} \to {\mathbb R}$ be an infinitely differentiable even function with $\tilde \omega(0) = 1$, $\tilde \omega$ decreasing on $[0, 1/4]$ and $\tilde \omega(x) = 0$ when $|x|\ge 1/4$, let $\tilde g(\lambda,x) = x^2 \tilde h(\lambda, x)+ \lambda \tilde \omega (x)$. Finally let $r(\lambda,x) = x(\lambda - \tilde g(\lambda,x)).$ Then the set of non-trivial solutions of $\lambda x = r(\lambda,x )$ coincide with the non-trivial solution set of $\tilde g(\lambda,x) = 0$ which is the set $\overline { \mathcal{C}}\setminus \{(0,0)\}$. This completes the justification of Example B.

5.   Construction of Example C of (2.2)

Example C is a simplified version of Böhme's example [3] with added structure to ensure that all path-connected sets of non-trivial solutions are singletons.

According to Bing [1,Ex. 2,p. 48] there exists a hereditarily indecomposable continuum, $H$ say, which separates the plane. Let $\Omega$ be a non-empty bounded component of ${\mathbb R}^2 \setminus H$ and $\partial \Omega$ its boundary. Then $\partial \Omega \subset H$, since points which are not in $H$ (which is closed) are interior points of their connected component in ${\mathbb R}^2 \setminus H$.

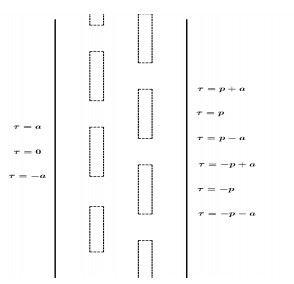
Without loss of generality, suppose that in the $(\varsigma,\tau)$-plane

Denote by $S$ the strip $[-\pi,\pi]\times {\mathbb R}$ and, with $a<p<2a$, consider two parallel columns of copies of $\Omega$, arranged periodically with period $2p$ in the $\tau$ direction, centred on the lines $\varsigma = \pm \pi/2$, and with height $2a$, as illustrated in Figure 1. The copies of $\Omega$ in the right column are translates through $(\pi, p)$ of those on the left. (Apart from being open, connected and satisfying (5.1), nothing is known about the shape of $\Omega$, so the diagram is for illustration only.) Let $\widehat \Omega$ denote the union of all the copies of $\Omega$ in this arrangement. The key to what follows is the property of $\widehat \Omega$ that, for all $\tau \in {\mathbb R}$, the set $\{\varsigma: (\varsigma,\tau) \in \widehat \Omega\}$ has strictly positive measure.

Now by Whitney's result there exists $\psi: {\mathbb R}^2 \to {\mathbb R}$ which is infinitely differentiable, $\psi>0$ on ${\mathbb R}^2 \setminus \partial \widehat \Omega$, and $\psi$ and all its derivatives are zero on $\partial \widehat \Omega$. There is no loss of generality in assuming that $\psi$ is $2p$-periodic in $\tau$ and equals 1 in the two strips $[7\pi/8,\pi] \times {\mathbb R}$ and $[-\pi,-7\pi/8] \times {\mathbb R}$. Now, for $(\varsigma ,\tau) \in S$, let

Next define infinitely differentiable functions $\kappa^\pm$ which are $2p$-periodic in $\tau\in {\mathbb R}$ by

where $\kappa_-(\tau) <0<\kappa^+(\tau),\; \tau \in {\mathbb R}$, and let

Then $\varphi(\varsigma,\tau) = -\kappa^-(\tau)>0$ when $|\varsigma-\pi|<\pi/8$, $\varphi$ is infinitely differentiable, $\partial \widehat \Omega$ is the zero set of $\varphi$, and by (5.2)

If $\Phi:S \to {\mathbb R}$ is defined by

then by (5.3), for $\tau \in {\mathbb R}$,

With this in mind, an infinitely differentiable function $r:{\mathbb R}^2 \to {\mathbb R}$ can be defined by putting $r(0,0) = 0$ and, for $(x,y) = \rho(\cos \vartheta,\sin \vartheta)$ in polar coordinates, let

Then, since (2.2) is of the form

its non-trivial solutions satisfy

By (5.4) and (5.5), the second equation implies that

Therefore, from (5.6) and the construction of $\widehat \Omega$, it follows that in this example any non-trivial solutions $(\lambda, (x,y))\in {\mathbb R} \times {\mathbb R}^2$ of (2.2) has

Since $\partial \widehat \Omega$ is the union of an infinite set of disjoint translates of $\partial \Omega$, see (5.1), and since $\partial\Omega \subset H$ which is a hereditarily indecomposable continuum, all path-connected components of the non-trivial solution set of (2.2) are singletons. However, non-trivial solutions of (2.2) with $\big(\lambda,( x,y)\big)$ near to $\big(0,(0,0)\big)$ exist, by Theorem 1.4.

6.   Notes on connected sets

Proposition 6.1. In a metric space $(M,d)$ let $\mathcal{G} = \{G_\alpha: \alpha \in \mathcal{A}\}$ be an open cover of a connected set $A$. Then for $x,y \in A$ there is a set $\{G_{\alpha_1}, \cdots, G_{\alpha_n}\}\subset \mathcal{G}$ with

Proof. Fix $x \in A$ and let $B \subset A$ be the set of $y \in A$ such that (6.1) holds for an ordered finite subset of $\mathcal{G}$. Then $B \neq \emptyset$ because $x \in B$, and if $y \in B$ then by (6.1) $z \in B$ for all $z \in G_{{\alpha_n}}\cap A$. So $B$ is open in $A$. Now suppose $z$ is in the closure of $B$ in $A$. Then, since $\mathcal{G}$ covers $A$, there exists $G \in \mathcal{G}$ such that $z \in G$, and there exists $y \in B$ with $y\in G$. Since $y\in B \cap G$, there exists $\{G_{\tilde\alpha_1}, \cdots, G_{\tilde\alpha_m}\}\subset \mathcal{G}$ such that (6.1) holds. Let $k$ be the smallest element of $\{1,\cdots, m\}$ for which $G_{\tilde \alpha_k}\cap G \neq \emptyset$. Then $\{ G_{\tilde\alpha_{j}},1 \le j \le k\}\cup \{G\}$ satisfies (6.1) with $z$ instead of $y$. So $z \in B$, whence $B$ is both closed and open in $A$. Hence $B = A$ since $A$ is connected and $B \neq \emptyset$.

Corollary 6.2. For $\epsilon>0$ and $x,y \in A$, where $A$ is connected in $(M,d)$, there is a set $\{x_1,\cdots,x_{n}\} \subset A$ with

where $B_ \epsilon(a)\subset M$ is the open ball with radius $\epsilon$ centred at $a$.

Proof. For given $\epsilon >0$ and $x,y \in A$, by Proposition 6.1 with $\mathcal{G} = \{B_{ \epsilon}(a): a\in A\}$, there exist $\{a_j:1\le j \le p\}\subset A$ with

Let $q = \max\{j\ge 1: B_{ \epsilon}(x) \cap B_ \epsilon(a_j)\neq \emptyset\}$ and put

Then $B_ \epsilon(y_i) \cap B_ \epsilon(y_j) \neq \emptyset$ if and only $|i-j|\le 1$, $y_1 = x$ and $y \in B_ \epsilon(y_{r})$.

Now let $n = m + 1$ where $m = \min\{j\le r: B_{ \epsilon}(y) \cap B_ \epsilon(y_j)\neq \emptyset\},$ and put $x_i = y_i,\; 1 \le i\le n-1$, $x_{n} = y$, to achieve the required result.

Corollary 6.3. When $(M,d)$ is a normed linear space, let $L_i = \{t x_i+(1-t)x_{i+1}: t \in [0,1]\}$, $1 \le i \le n-1$, be straight line segments joining the centres of consecutive balls in Corollary 6.2. Then

Consequently, $L: = \cup_{i = 1}^{n-1}L_i$ is a continuous, piecewise-linear, non-self-intersecting path joining $x$ to $y$.

Proof. First suppose that $z\in L_i \cap L_{i+1}$ and $z\neq x_{i+1}$. Then

whence $t(x_i - x_{i+1}) = s(x_{i+2} - x_{i+1})$. So $s \neq t$ because $x_i \neq x_{i+2}$. If $s<t$,

a contradiction, and if $t<s$,

which is also false. This proves that $L_i \cap L_{i+1} = \{x_{i+1}\}$ for all $i$.

Suppose $z \in L_i\cap L_j$ for $i\ge 1$ and $i+1<j\le n-1$. Then, by (6.2),

and

Therefore $x_{i+1} +s(x_i-x_{i+1}) = x_{j+1} +t(x_j-x_{j+1})$, which implies

and hence $s+t > 1$. Also $x_i-x_j = s'(x_i-x_{i+1}) + t'(x_{j+1}-x_j)$ and hence

from which it follows that $s'+t'>1$, equivalently, $s+t<1$, which is a contradiction. Since different line segments $L_i$ joining centres of balls do not intersect, their union $L$ is a continuous, piecewise-linear, non-self-intersecting path joining $x_1$ to $x_n$.

Definition 6.4. In a metric space a linear chain $\mathcal{G}$ is an ordered, finite collection of open sets with $G_i \cap G_j \neq \emptyset$ if and only if $|i-j|\le 1$. The $G_i$, which may not be connected, are the links of $\mathcal{G}$ and an $\epsilon$-linear chain is a linear chain with links of diameter less that $\epsilon$. If, for all $\epsilon>0$, a set $A$ can be covered by an $\epsilon$-linear chain, $A$ is said to be snake-like. A snake-like hereditarily indecomposable compact connected set is called a pseudo-arc.

A criterion for the existence of paths in connected sets in Banach spaces. Suppose in Corollaries 6.2 and 6.3 that the metric space $(M,d)$ is a Banach space $(V,\|\cdot\|)$, that $A \subset V$ is closed and connected, and that closed bounded subsets of $A$ are compact. For fixed $x \neq y \in A$ and any $\epsilon>0$ let

Theorem 6.5. If $\ell^{ \epsilon}$ is bounded as $\epsilon \to 0$, there is a path in $A$ joining $x$ to $y$.

The proof depends on infinite-dimensional versions of two well-known theorems.

Theorem 6.6. [20,p. 179] (Ascoli-Arzelà) When $X$ is a separable topological space, $Y$ is a metric space and $\{h_k\}$ is an equi-continuous sequence of functions from $X$ to $Y$ with the property that the closure of $\{h_k(x): k\in {\mathbb N}\}$ is compact in $Y$ for each $x \in X$, there is a subsequence $\{h_{k_j}\}$ and a continuous function $h$ such that $h_{k_j}(x) \to h(x)$ pointwise, and uniformly on every compact subset of $X$.

Theorem 6.7. [11,Ch.Ⅴ §2.6] (Mazur) In a Banach space the closed convex hull ${\rm \overline{co}}(K)$ of a compact set $K$ is compact.

Proof of Theorem 6.5. Let $0< \epsilon_k \to 0$ and for each $k$ let $L^{ \epsilon_k}$ be a piecewise linear, non-self-intersecting path in $V$ joining $x$ to $y$, as in Corollary 6.3, with length $\gamma_k$ where

Since, for all $k$, the $x^{ \epsilon_k}_i$s are in $A$ which is closed, and since $\|x^{ \epsilon_k}_i-x\|$ is bounded independent of $i$ and $k$, there is a closed bounded subset $A^*$ of $A$ with $x^{ \epsilon_k}_i \in A^*$ for all $i$ and $k$. By hypothesis, $A^*$ is compact. Therefore, since each $L^{ \epsilon_k}$ is a union of straight-lines joining points of $A^*$, the paths $L^{ \epsilon_k} \subset {\rm \overline{co}}(A^*)$, where ${\rm \overline{co}}(A^*)$ is compact in the Banach space $V$ by Mazur's Theorem 6.7. Moreover, by hypothesis each $L^{ \epsilon_k}$ is rectifiable with length $\gamma_k$ bounded above independent of $k$. Since $L^{ \epsilon_k}$ is piecewise linear it can be parameterised by arc-length $s$, $L^{ \epsilon_k} = \{f_k( s): s \in [0, \gamma_k]\}$ say, where $f_k:[0,\gamma_k] \to {\rm \overline{co}}(A^*)\subset V$, $\|f_k'(s)\| = 1$ almost everywhere, $f_k(0) = x$ and $f_k(\gamma_k) = y$.

Now let $h_k(t) = f_k(\gamma_k t),\,t \in [0,1]$, so that $L^{ \epsilon_k} = \{ h_k( t): t \in [0, 1]\}\subset {\rm \overline{co}}(A^*)$ and $\{h_k:k\in {\mathbb N}\}$ is uniformly bounded and equi-continuous on $[0,1]$, because $\|h'_k(t)\| = \gamma_k$ for almost all $t \in [0,1]$ and $\gamma_k$ is bounded.

Since $[0,1]\subset {\mathbb R}$ and ${\rm \overline{co}}(A^*)\subset V$ are both compact, it follows from the Ascoli-Arzelà Theorem 6.6, with $X = [0,1]$ and $Y = {\rm \overline{co}}(A^*)$, that a subsequence $\{h_{k_j}\}$ converges uniformly on $[0,1]$ to a continuous $h:[0,1] \to {\rm \overline{co}}(A^*)$. Since, for $s \in [0,1]$ and $j \in {\mathbb N}$, there exists $x_i^{ \epsilon_{k_j}} \in A^*$ with $\|x_i^{ \epsilon_{k_j}}-h_{k_j}(s)\| \le \epsilon_{k_j}$,

Since $A^*$ is closed, $h:[0,1] \to A^* \subset A$ and $h$ is continuous. Finally note that $x = h_{k_j}(0)\to h(0)$, $y = h_{k_j}(1)\to h(1)$. So $h$ defines a path in $A$ joining $x$ to $y$.