Analytical models of threshold voltage and drain induced barrier lowering in junctionless cylindrical surrounding gate (JLCSG) MOSFET using stacked high-<i>k</i> oxide

Hakkee Jung; Hakkee Jung

doi:10.3934/electreng.2022007

AIMS Electronics and Electrical Engineering

2022, Volume 6, Issue 2: 108-123. doi: 10.3934/electreng.2022007

Previous Article Next Article

Research article

Analytical models of threshold voltage and drain induced barrier lowering in junctionless cylindrical surrounding gate (JLCSG) MOSFET using stacked high-k oxide

Hakkee Jung ^,

Department of Electronic Eng., Kunsan National University, Gunsan-si, Jeollabuk-do, 54150, Republic of Korea

Received: 26 December 2021 Revised: 28 February 2022 Accepted: 11 March 2022 Published: 16 March 2022

We proposed the analytical models to analyze shifts in threshold voltage and drain induced barrier lowering (DIBL) when the stacked SiO₂/high-k dielectric was used as the oxide film of Junctionless Cylindrical Surrounding Gate (JLCSG) MOSFET. As a result of comparing the results of the presented model with those of TCAD, it was a good fit, thus proving the validity of the presented model. It could be found that the threshold voltage increased, but DIBL decreased by these models as the high-k dielectric constant increased. However, the shifts of threshold voltage and DIBL significantly decreased as the high-k dielectric constant increased. As for the degree of reduction, the channel length had a greater effect than the thickness of the high-k dielectric, and the shifts of threshold voltage and DIBL were kept almost constant when the high-k dielectric constant was 20 or higher. Therefore, the use of dielectrics such as HfO₂/ZrO₂, La₂O₃, and TiO₂ with a dielectric constant of 20 or more for stacked oxide will be advantageous in reducing the short channel effect. In conclusion, these models were able to sufficiently analyze the threshold voltage and DIBL.

Keywords:

Citation: Hakkee Jung. Analytical models of threshold voltage and drain induced barrier lowering in junctionless cylindrical surrounding gate (JLCSG) MOSFET using stacked high-k oxide[J]. AIMS Electronics and Electrical Engineering, 2022, 6(2): 108-123. doi: 10.3934/electreng.2022007

Related Papers:

[1]	Hakkee Jung . Analysis of drain induced barrier lowering for junctionless double gate MOSFET using ferroelectric negative capacitance effect. AIMS Electronics and Electrical Engineering, 2023, 7(1): 38-49. doi: 10.3934/electreng.2023003
[2]	Hakkee Jung . Analytical model of subthreshold swing in junctionless gate-all-around (GAA) FET with ferroelectric. AIMS Electronics and Electrical Engineering, 2023, 7(4): 322-336. doi: 10.3934/electreng.2023017
[3]	Hakkee Jung . Analytical subthreshold swing model of junctionless elliptic gate-all-around (GAA) FET. AIMS Electronics and Electrical Engineering, 2024, 8(2): 211-226. doi: 10.3934/electreng.2024009
[4]	Suliana Ab-Ghani, Hamdan Daniyal, Abu Zaharin Ahmad, Norazila Jaalam, Norhafidzah Mohd Saad, Nur Huda Ramlan, Norhazilina Bahari . Adaptive online auto-tuning using Particle Swarm optimized PI controller with time-variant approach for high accuracy and speed in Dual Active Bridge converter. AIMS Electronics and Electrical Engineering, 2023, 7(2): 156-170. doi: 10.3934/electreng.2023009
[5]	José M. Campos-Salazar, Juan L. Aguayo-Lazcano, Roya Rafiezadeh . Non-ideal two-level battery charger—modeling and simulation. AIMS Electronics and Electrical Engineering, 2025, 9(1): 60-80. doi: 10.3934/electreng.2025004
[6]	Amleset Kelati, Hossam Gaber, Juha Plosila, Hannu Tenhunen . Implementation of non-intrusive appliances load monitoring (NIALM) on k-nearest neighbors (k-NN) classifier. AIMS Electronics and Electrical Engineering, 2020, 4(3): 326-344. doi: 10.3934/ElectrEng.2020.3.326
[7]	Shaswat Chirantan, Bibhuti Bhusan Pati . Integration of predictive and computational intelligent techniques: A hybrid optimization mechanism for PMSM dynamics reinforcement. AIMS Electronics and Electrical Engineering, 2024, 8(2): 265-291. doi: 10.3934/electreng.2024012
[8]	Gaurav Sharma, Guennadi A. Kouzaev . Miniature glass-metal coaxial waveguide reactors for microwave-assisted liquid heating. AIMS Electronics and Electrical Engineering, 2023, 7(1): 100-120. doi: 10.3934/electreng.2023006
[9]	Olayanju Sunday Akinwale, Dahunsi Folasade Mojisola, Ponnle Akinlolu Adediran . Mitigation strategies for communication networks induced impairments in autonomous microgrids control: A review. AIMS Electronics and Electrical Engineering, 2021, 5(4): 342-375. doi: 10.3934/electreng.2021018
[10]	Mamun Mishra, Bibhuti Bhusan Pati . A hybrid IDM using wavelet transform for a synchronous generator-based RES with zero non-detection zone. AIMS Electronics and Electrical Engineering, 2024, 8(1): 146-164. doi: 10.3934/electreng.2024006

Abstract

1. Introduction

As the channel length of the transistor decreases to sub-10 nm or less, the conventional transistor structure becomes increasingly difficult to use due to the short channel effects ^[1,2,3]. For high-speed operation, low power consumption, and improved productivity, the reduction of transistor size is becoming the greatest competitiveness of all integrated circuit manufacturers. Transistors of various structures have been developed and used to reduce the short channel effects that inevitably occurs due to the reduction in transistor size. In particular, FinFET is widely used in Qualcomm's communication chips. FinFET has a tri-gate structure and it has been shown to have excellent control of carriers in the channel even at channel length and fin width of sub-10 nm ^[4,5]. In order to improve the gate control capability, the four-gate structure called Omega-FET, was developed ^[6]. Omega-FET is a structure in which the gate is wrapped around a rectangular channel, so that the control capability by the gate terminal is improved than that of FinFET. However, the Omega-FET has a problem of the corner-effect that inevitably occurs in the square structure. The structure developed to solve this problem is a cylindrical surrounding gate (CSG) MOSFET. The CSG MOSFET has been developed to cope with the short channel effects more than the FinFET used in various application ranges ^[7,8]. The CSG MOSFET is a structure in which the gate terminal completely encloses the cylindrical channel. As it is known as the most effective structure for controlling the carriers in the channel by the gate terminal as well as removing the corner effect that occurs in the Omega-FET, many studies are being conducted ^[9,10].

As the channel length is shortened, not only the structural problem of the channel but also the doping technology are causing problems. In the junction-based 3D FET of the inversion type using the PN junction formed between the source/drain and the channel, the reduction of channel length makes the PN junction process difficult, and the channel formation difficult due to the depletion layer effect that occurs in the PN junction. The MOSFET developed to solve this problem is the junctionless MOSFET ^[11,12]. A lot of research has been carried out since this structure was presented by Colinge et al., and it is a structure that can solve the difficulty of the process because it is not necessary to form a PN junction between the cannel and source/drain region ^[13]. In particular, in many studies, it has been known that the short-channel effects are more significantly reduced in the accumulation type which is a junctionless MOSFET than in the inversion type MOSFET that is a junction-based MOSFET ^[14,15].

In addition to the above problems, there are many problems in the thickness of the gate oxide layer with the reduction of the channel length due to scaling effects. According to the scaling rule, the thickness of the gate oxide film must be reduced in proportion to the channel length. However, when the thickness of the gate oxide is decreased, not only a problem in the process but also another short channel effects occurs due to an increase in gate parasitic current. As the reduction of the gate oxide film hits its limit, many efforts are made to use a high-k oxide film as the gate oxide film to reduce the short channel effects and gate leakage current ^{[16,17,18,19,20,21,22]}. However, the high-k oxide film material has a limitation in reducing the gate parasitic current due to a small band offset, generating roughness of the interface with silicon used as a channel. A structure that has emerged to solve this problem is a stacked structure of a gate oxide film. In this structure, SiO₂ is used for the part in contact with the silicon channel and the high-k dielectric is used for the part in contact with the gate metal, thereby solving the short channel effect caused by the reduction of the gate oxide thickness. Rasol et al. analyzed the I_off current for a junctionless cylindrical surrounding gate(JLCSG) MOSFET using stacked high-k oxide by ATLAS, and Darwin et al. used HfO₂, HfSiO₄, Al₂O₃, Si₃N₄, etc. as high-k materials, but the short-channel effects of the junctionless cylindrical MOSFET was analyzed using only the parametric form of the potential distribution ^[23,24]. Also Kosmani et al. analyzed the short-channel effects by TCAD, using a high-k oxide film in a junction-based double gate and Gate-All-Around MOSFET ^[25]. S. Gupta et al. obtained the potential distribution of the dual gate metal junctionless cylindrical gate-all-around (JLC-GAA) MOSFET, using HfO₂ as a high-k material ^[26]. As such, many researchers are trying to reduce the short channel effect of JLCSG MOSFETs by using stacked high-k materials. In this study, in order to meet this purpose, the analytical threshold voltage and DIBL model were proposed using the definition of the threshold voltage and DIBL which are a kind of the short channel effects. We will prove the validity of the proposed models and analyze the threshold voltage and DIBL using the proposed models to consider the phenomenon of short-channel effects reduction in the case of using SiO₂/high-k gate oxide stacked on the JLCSG MOSFET. As a high-k dielectrics, we will use SiO₂ (ε_s = 3.9), Al₂O₃ (ε_s = 9), Y₂O (ε_s = 15), HfO₂/ZrO₂ (ε_s = 25), La₂O₃ (ε_s = 30), TiO₂ (ε_s = 80).

2. Threshold voltage and DIBL models for JLCSG MOSFET

2.1. Potential distributions in the channel of JLCSG MOSFET

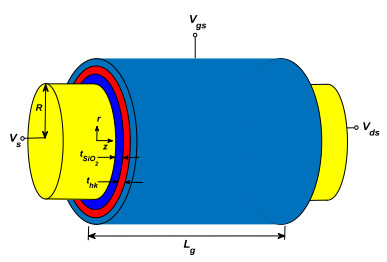
Figure 1 shows the schematic diagram of the JLCSG MOSFET used in this paper. The source, drain, and channel were doped with n-type of high concentration, and a metal with a work function of ϕ_m was used. L_g denotes the length of the channel, R the radius of the silicon. The t_SiO2 is the thickness of the SiO₂ oxide layer bonded to the silicon channel, and the t_hk the thickness of the high-k material, which is in contact with the gate metal, and these two dielectrics are stacked. The t_SiO2 used 1 nm, and the t_hk between 1 nm and 5 nm in this paper. The V_gs, V_ds, and V_s represent a gate voltage, a drain voltage, and a source voltage, respectively. At this time, the potential distribution of the JLCSG MOSFET was obtained using the following Poisson equation ^[24,26,27].

$\frac{1}{r}\frac{\partial }{{\partial r}}\left[ {r\frac{\partial }{{\partial r}}\phi (r, z)} \right] + \frac{{{\partial ^2}\phi (r, z)}}{{\partial {z^2}}} = - \frac{{q{N_d}}}{{{\varepsilon _{si}}}}$

(2.1)

Figure 1. Schematic cross-sectional diagram of the cylindrical surrounding gate (CSG) MOSFET.

DownLoad: Full-Size Img PowerPoint

Here, ε_si is the dielectric constant of silicon, and N_d is 10¹⁹/cm³ as the channel doping concentration. Using the superposition technique, the potential distributions in channel region are expressed as follows.

$\phi (r, z) = {\phi _1}(r) + {\phi _2}(r, z)$

(2.2)

Here, ϕ₁(r) is the one dimensional solution to Poisson's equation and ϕ₂(r, z) is the two dimensional solution to the homogenous Laplace equation, which is expressed as follows.

$\frac{1}{r}\frac{\partial }{{\partial r}}\left( {r\frac{{\partial {\phi _1}(r)}}{{\partial r}}} \right) = - \frac{{q{N_d}}}{{{\varepsilon _{si}}}}$

(2.3)

$\frac{{{\partial ^2}{\phi _2}(r, z)}}{{\partial {r^2}}} + \frac{1}{r}\frac{{\partial {\phi _2}(r, z)}}{{\partial r}} + \frac{{{\partial ^2}{\phi _2}(r, z)}}{{\partial z}} = 0$

(2.4)

To solve (2.3), the following boundary conditions are used ^[28].

$\begin{array}{l} {\left. {\frac{{\partial {\phi _1}\left( r \right)}}{{\partial r}}} \right|_{r = 0}} = 0\\ {\left. {\frac{{\partial {\phi _1}\left( r \right)}}{{\partial r}}} \right|_{r = R}} = \frac{{{C_{ox}}}}{{{\varepsilon _{si}}}}\left[ {{V_{gs}} - {\phi _{ms}} - {\phi _1}(R)} \right] \end{array}$

(2.5)

where V_gs is the applied voltage of the gate and ϕ_ms is the work function difference between gate metal and silicon. The C_ox is expressed as follows ^[29].

$\begin{array}{l} {C_{ox}} = \frac{{{\varepsilon _{Si{O_2}}}}}{{R\ln (1 + {t_{oxeff}}/R)}}\\ {t_{oxeff}} = {t_{Si{O_2}}} + ({\varepsilon _{Si{O_2}}}/{\varepsilon _{hk}}){t_{hk}} \end{array}$

(2.6)

At this time, the solution to (2.3) is as follows ^[30].

${\phi _1}(r) = - \frac{{q{N_d}}}{{4{\varepsilon _{si}}}}{r^2} + {V_{gs}} - {\phi _{ms}} + \frac{{q{N_d}R}}{{2{C_{ox}}}} + \frac{{q{N_d}{R^2}}}{{4{\varepsilon _{si}}}}$

(2.7)

Since the method of calculating ϕ₂(r, z) from (2.4) is independent of the doping distribution, using the variable separation method and Fourier-Bessel series by the method of C. Li et al. ^[30], ϕ₂(r, z) is as follows.

${\phi _2}(r, z) = \sum\limits_{n = 1}^\infty {\left[ {{C_n}\exp \left( {\frac{{{\alpha _n}z}}{R}} \right) + {D_n}\exp \left( { - \frac{{{\alpha _n}z}}{R}} \right)} \right]{J_0}\left( {\frac{{{\alpha _n}r}}{R}} \right)}$

(2.8)

where a_n is eigenvalue that satisfy the following equation.

$R{J_0}({\alpha _n}) - \frac{{{\varepsilon _{si}}}}{{{C_{ox}}}}{\alpha _n}{J_1}({\alpha _n}) = 0$

(2.9)

In (2.8), C_n and D_n are obtained using the following boundary conditions ^[28].

$\begin{array}{l} \phi (r, z = 0) = {V_F}\\ \phi (r, z = L) = {V_F} + {V_{ds}}\\ {V_F} = \frac{{kT}}{q}\ln \left( {\frac{{{N_d}}}{{{n_i}}}} \right) \end{array}$

(2.10)

At this time, because the first term dominates the series of (2.8) due to rapid decay of the Fourier-Bessel series, only C₁ and D₁ were used as follows.

$\begin{array}{l} {C_1} = \frac{{A\left[ {\exp \left( { - {\lambda _1}} \right) - 1} \right] - B}}{{2\sinh \left( { - {\lambda _1}} \right)}}\\ {D_1} = \frac{{A\left[ {1 - \exp \left( {{\lambda _1}} \right)} \right] + B}}{{2\sinh \left( { - {\lambda _1}} \right)}}\\ {\lambda _1} = \frac{{{\alpha _1}L}}{R}\\ A = \frac{{ - 2}}{{J_0^2({\alpha _1}) + J_1^2({\alpha _1})}}\frac{{{J_1}({\alpha _1})}}{{{\alpha _1}}}\left( {{V_{gs}} - {\phi _{ms}} + \frac{{q{N_D}R}}{{2{C_{ox}}}} + \frac{{q{N_D}{R^2}}}{{4{\varepsilon _{si}}}} - {V_F}} \right) \\ + \frac{{q{N_D}}}{{2{\varepsilon _{si}}}}\frac{1}{{J_0^2({\alpha _1}) + J_1^2({\alpha _1})}}\frac{{{\alpha _1}{J_1}({\alpha _1}) - 2{J_2}({\alpha _1})}}{{{{\left( {{\alpha _1}/R} \right)}^2}}}\\ B = \frac{{2{V_{ds}}}}{{J_0^2({\alpha _1}) + J_1^2({\alpha _1})}}\frac{{{J_1}({\alpha _1})}}{{{\alpha _1}}} \end{array}$

(2.11)

Substituting (2.7), (2.8), and (2.11) into (2.2), the potential distribution of the JLCSG MOSFET in the channel can be obtained.

2.2. The threshold voltage and DIBL of JLCSG MOSFET

The analytical threshold voltage model is derived using the definition of the threshold voltage and the distribution of electrostatic potential in the previous section. The junctionless MOSFET operates in accumulation mode, and the gate voltage is defined as the threshold voltage when the minimum value of the central potential distribution becomes V_F ^[31]. In other words, the gate voltage that satisfies

${\phi _{\min }} = \phi (0, {z_{\min }}) = {V_F}$

(2.12)

must be obtained. At this time, z_min represents the z value at which the central potential distribution becomes the minimum, and is given as ^[28].

${z_{\min }} = \left( {\frac{R}{{2{\alpha _1}}}} \right)\ln \left( {\frac{{{D_1}}}{{{C_1}}}} \right)$

(2.13)

S. K. Gupta et al. obtained the threshold voltage by the same method, but ignored the dependence of V_gs to the variables A, B, and z_min ^[32]. Therefore, in this paper, the following threshold voltage V_th can be obtained as in Appendix A if V_gs that satisfies (2.12) is obtained.

${V_{th}} = \frac{1}{{2\left[ {{a_1}/{{\sinh }^2}\left( { - {\lambda _1}} \right) - 1} \right]}}\\\left\{ { - \left[ {{a_2}/{{\sinh }^2}\left( { - {\lambda _1}} \right) - 2H} \right] + \sqrt {\begin{array}{*{20}{l}} {{{\left[ {{a_2}/{{\sinh }^2}\left( { - {\lambda _1}} \right) - 2H} \right]}^2} - }\\ {4\left[ {{a_1}/{{\sinh }^2}\left( { - {\lambda _1}} \right) - 1} \right]\left[ {{a_3}/{{\sinh }^2}\left( { - {\lambda _1}} \right) - {H^2}} \right]} \end{array}} } \right\}$

(2.14)

The constants a₁, a₂, a₃ and H are indicated in Appendix A. In order to prove the validity of (2.14), it was compared in Figure 2 with Hu's model ^[28].

Figure 2. Comparisons of the various threshold voltages with this model (2.14).

DownLoad: Full-Size Img PowerPoint

As seen in Figure 2, the threshold voltage obtained by the g_m/I_d method is overestimated. It can be seen that (2.14) suggested in this paper agrees well with Hu's model. However, it can be found that a difference between the threshold voltages occurs when the channel length is reduced to 10 nm.

The DIBL is a measure of the change of the threshold voltage to the drain voltage. In the previous paper ^[33,34,35], the change of the threshold voltage was calculated when the drain voltages are changed from V_ds = 0.1 V to V_ds = 0.5 V or 1.0 V to obtain the DIBL. However, in this paper, using the definition of the DIBL and (2.14), the following DIBL could be obtained through the same process as in Appendix B.

$DIBL = - \frac{1}{{2\left[ {{a_1} - {{\sinh }^2}\left( {{\lambda _1}} \right)} \right]}}\left\{ \begin{array}{l} {X^2}\left( {\exp \left( { - {\lambda _1}} \right) + \exp \left( {{\lambda _1}} \right) - 2} \right) + \\ \frac{{\left\{ \begin{array}{l} \left( {\frac{{{a_2}}}{{{{\sinh }^2}\left( {{\lambda _1}} \right)}} - 2H} \right)\left( { - {X^2}\left( {\exp \left( { - {\lambda _1}} \right) + \exp \left( {{\lambda _1}} \right) - 2} \right)} \right) - \\ 2\left( {\frac{{{a_1}}}{{{{\sinh }^2}\left( {{\lambda _1}} \right)}} - 1} \right)\left( { - XY\left( {\exp \left( { - {\lambda _1}} \right) + \exp \left( {{\lambda _1}} \right) - 2} \right) - 2{X^2}{V_{ds}}} \right) \end{array} \right\}}}{{\sqrt {{{\left[ {{a_2}/{{\sinh }^2}\left( {{\lambda _1}} \right) - 2H} \right]}^2} - 4\left[ {{a_1}/{{\sinh }^2}\left( {{\lambda _1}} \right) - 1} \right]\left[ {{a_3}/{{\sinh }^2}\left( {{\lambda _1}} \right) - {H^2}} \right]} }} \end{array} \right\}$

(2.15)

In (2.15), X and Y values are indicated in Appendix A. It can be shown in (2.15) that the DIBL changes according to the value of V_ds. That is, the DIBL value changes according to the V_ds value measuring DIBL. In order to prove the validity of (2.15), the DIBL values of other models in Figure 3 were compared under the same conditions. As a result, it can be observed that it fits well with the results of other models. However, it can be observed that the errors between models increase as the channel length decreases. As above, since the validity of the threshold voltage model (2.14) and DIBL model (2.15) presented in this paper has been verified, we will use these equations to observe the characteristics of the JLCSG MOSFET with the stacked oxide film of SiO₂ and high-k dielectrics. Device parameters used in this study are listed in Table 1.

Figure 3. Comparison of the various DIBLs with this model (2.15).

DownLoad: Full-Size Img PowerPoint

Table 1. Device parameters used in these models.

Device parameter	Symbol	Value
Channel length	L_g	10 - 30 nm
Silicon radius	R	5 nm
Doping concentration	N_d	10¹⁹/cm³
Thickness of SiO₂	t_SiO2	1 nm
Thickness of high-k dielectric	t_hk	1 - 5 nm
Permittivity of high-	ε_hk	3.9 - 80

| Show Table

DownLoad: CSV

3. Analysis of threshold voltage and DIBL for JLCSG MOSFET by these models

In the structure of Figure 1, the change of the threshold voltage with respect to the dielectric constant of the high-k material is calculated with the drain voltage as a parameter as shown in Figure 4. The difference in threshold voltage is indicated by arrows in Figure 4 when the drain voltage increases from 0.1 V to 1.1 V for a specific high-k material. As shown in Figure 4, as the dielectric constant of high-k material increases, the threshold voltage increases, but the threshold voltage is almost constant from the dielectric constant of 20. As shown in Figure 4, DIBL, which is the difference between the threshold voltage at the drain voltage of 0.1 V and the threshold voltage at 1.1 V, shows a larger value as the dielectric constant decreases, but gradually decreases as the dielectric constant of high-k increases. Therefore, the value is an almost constant at the dielectric constant of high-k of above 20.

Figure 4. Threshold voltages for dielectric constant of high-k with the drain voltage as a parameter.

DownLoad: Full-Size Img PowerPoint

Figure 5 shows the change of the DIBL calculated using (2.15) according to the high-k permittivity. As seen in Figure 5, the DIBL appears large when the high-k dielectric constant is small, and the DIBL decreases as the dielectric constant increases, and it can be observed that the DIBL appears constant when the high-k dielectric constant is 20 or higher. As seen in (2.15) and Figure 5, the DIBL changed according to the drain voltage, and the DIBL increased as the drain voltage increased. If the high-k dielectric constant is as small as 3.9 like SiO₂, the rate of change of DIBL for the change of the drain voltage (△DIBL/△V_ds) is about 14.6 mV/V² under the conditions given in Figure 5, but the changing rate of DIBL was maintained with 10 mV/V² when the high-k dielectric constant is 20 above.

Figure 5. DIBLs for dielectric constant of high-k with the drain voltage as a parameter.

DownLoad: Full-Size Img PowerPoint

The change of the threshold voltage with respect to the high-k dielectric constant as a parameter of the channel length is shown in Figure 6(a). As seen from Figure 2 and Figure 6(a), a threshold voltage shift occurs and the threshold voltage decreases when the channel length decreases. As the channel length decreased, the degree of reduction increased significantly according to the high-k dielectric constant. As described above, a constant threshold voltage was exhibited regardless of the channel length when the high-k dielectric constant is 20 or more. The shift of the threshold voltage is shown in Figure 6(b) when the channel length decreases from 30 nm to 10 nm. As seen from Figure 6(b), the shift of the threshold voltage decreases as the dielectric constant of high-k increases. In particular, the shift of the threshold voltage is almost constant when the dielectric constant of high-k is 20 or more.

Figure 6. (a) Threshold voltages and (b) threshold voltage shift for dielectric constant of high-k with the channel length as a parameter.

DownLoad: Full-Size Img PowerPoint

The change of DIBL according to the high-k dielectric constant as a parameter of the channel length is shown in Figure 7(a). The DIBL shows a large change according to the high-k dielectric constant when the channel length is very small such as about 10 nm. However, it can be observed that the change of high-k dielectric constant does not have a significant effect on DIBL when the channel length is increased to 30 nm. The change of DIBL (ΔDIBL) is shown in Figure 7(b) when the channel length changes from 30 nm to 10 nm. As seen from Figure 7(b), the change in DIBL was greatly reduced as the high-k dielectric constant increased. Like the threshold voltage, ΔDIBL was almost constant when the high-k dielectric constant was 20 or higher.

Figure 7. (a) DIBLs and (b) shift of DIBL for dielectric constant of high-k with the channel length as a parameter.

DownLoad: Full-Size Img PowerPoint

The reason for using high-k dielectric is to solve the difficulty in the process due to the reduction of the oxide film thickness. That is, the effective oxide thickness (EOT) can be increased by the ratio of the high-k dielectric constant and the SiO₂ dielectric constant of 3.9 ^[36]. Therefore, if the high-k oxide film is used, it will be possible to solve the difficulty in the process due to the reduction in the oxide film thickness. Here, we will observe the effect of the thickness of the high-k material on the threshold voltage. In Figure 8, the change of the threshold voltage with respect to the dielectric constant of high-k materials is shown with the thickness of the high-k material as a parameter. As shown in Figure 8(a), the threshold voltage increases as the dielectric constant of high-k material increases, but the rate of increase decreases rapidly, and the threshold voltage is maintained almost constant at a dielectric constant of above 20. In particular, if t_hk was decreased to 1 nm, even if the dielectric constant was 10 or more, the threshold voltage was maintained constantly regardless of the value of the high-k dielectric constant. Figure 8(a) shows that the threshold voltage changes sensitively to the change of dielectric constant as the thickness of high-k material increases, and the shift of threshold voltage decreases as the dielectric constant increases when the thickness of high-k material is changed from t_hk = 1 nm to t_hk = 5 nm as shown in Figure 8(b).

Figure 8. (a) Threshold voltages and (b) threshold voltage shift for dielectric constant of high-k with the thickness of high-k dielectric as a parameter.

DownLoad: Full-Size Img PowerPoint

Figure 9 shows the change of the DIBL according to the dielectric constant with the thickness of the high-k material as a parameter. Figure 9(a) shows that the DIBL decreased as the dielectric constant of the high-k material increased and the thickness decreased. As explained in Figure 8, it could be observed that if t_hk is decreased to 1 nm, the change in DIBL according to the dielectric constant is negligibly small even if the high-k dielectric constant is 10 or more. Figure 9(b) shows the change of DIBL according to the dielectric constant when the thickness of high-k is changed from t_hk = 1 nm to t_hk = 5 nm. The effect of the thickness change of the high-k material on DIBL is very small as the dielectric constant increases.

Figure 9. (a) DIBLs and (b) shift of DIBL for dielectric constant of high-k with the thickness of high-k dielectric as a parameter.

DownLoad: Full-Size Img PowerPoint

Comparing Figure 6 and Figure 8, the change in the threshold voltage according to the dielectric constant of the high-k material has a greater influence on the channel length than the change in the thickness of the high-k material. That is, the threshold voltage shift of about 0.26 V appeared when the channel length changed from 30 nm to 10 nm for the high-k dielectric of 20 or more, but the threshold voltage shift of about 0.05 V was only occurring when the thickness of the high-k dielectric changed from 1 nm to 5 nm. As seen from the comparison between Figure 7 and Figure 9, DIBL shift of about 380 mV/V appears when the channel length changes from 30 nm to 10 nm with a high-k dielectric constant of 20 or more. However, only the change of the DIBL of about 20 mV/V occurred when the dielectric thickness changes from 1 nm to 5 nm.

4. Conclusions

In this study, the analytical models of threshold voltage and DIBL were proposed to analyze the change in the threshold voltage and DIBL among the short channel effects of JLCSG MOSFETs using a stacked high-k dielectric as an oxide layer. They matched very well as a result of comparison with other papers. As scaling progresses, problems such as difficulty in process and short channel effects occur due to a reduction in the thickness of the oxide film according to the channel length. In this paper, the change of the threshold voltage and DIBL according to the dielectric constant of the high-k materials was observed using the proposed model with the channel length and thickness of the high-k dielectric as parameters. The shifts of threshold voltage and DIBL are 0.39 V and 577 mV/V, respectively, when the channel length decreases from 30 nm to 10 nm if SiO₂ is used as a high-k material, whereas it can be seen that those decrease to 0.27 V and 403 mV/V, respectively when the dielectric constant of the stacked high-k material is increased to 20. In addition, considering the changes in the thickness of the high-k dielectric, the shifts of threshold voltage and DIBL are 0.33 V and 97.0 mV/V, respectively when the thickness of the high-k dielectric decreases from 1 nm to 5 nm if SiO₂ is used as a high-k material. On the other hand, it was found that those decreased to 0.086 V and 20.8 mV/V, respectively when the dielectric constant of the high-k material increased to 20. As described above, the short-channel effect can be reduced in JLCSG MOSFETs by using a stacked high-k dielectric as the gate oxide. It was found that the proposed models excellently analyzed the threshold voltage and DIBL.

Conflict of interest

All authors declare no conflicts of interest in this paper.

Appendix

A. Analytical threshold voltage

If $H = - {\phi _{ms}} + \frac{{q{N_d}R}}{{2{C_{ox}}}} + \frac{{q{N_d}{R^2}}}{{4{\varepsilon _{si}}}} - {V_F}$ ,

$\begin{align} & {{\phi }_{\min }} = \phi (0, {{z}_{\min }}) = {{V}_{gs}}+H+{{C}_{1}}\exp \left( \frac{{{\alpha }_{1}}{{z}_{\min }}}{R} \right)+{{D}_{1}}\exp \left( -\frac{{{\alpha }_{1}}{{z}_{\min }}}{R} \right) \\ & = {{V}_{gs}}+H+{{C}_{1}}\exp \left( \frac{{{\alpha }_{1}}}{R}\frac{R}{2{{\alpha }_{1}}}\ln \left( \frac{{{D}_{1}}}{{{C}_{1}}} \right) \right)+{{D}_{1}}\exp \left( -\frac{{{\alpha }_{1}}}{R}\frac{R}{2{{\alpha }_{1}}}\ln \left( \frac{{{D}_{1}}}{{{C}_{1}}} \right) \right) \\ & = {{V}_{gs}}+H+2\sqrt{{{C}_{1}}{{D}_{1}}} \\ & = {{V}_{gs}}+H+\left( 1/\sinh \left( -{{\lambda }_{1}} \right) \right)\sqrt{\left[ A\left( \exp \left( -{{\lambda }_{1}} \right)-1 \right)-B \right]\left[ A\left( 1-\exp \left( {{\lambda }_{1}} \right) \right)+B \right]} \\ & = {{V}_{gs}}+H+\left( 1/\sinh \left( -{{\lambda }_{1}} \right) \right)\sqrt{\left[ (X{{V}_{gs}}+Y)\left( \exp \left( -{{\lambda }_{1}} \right)-1 \right)-B \right]\left[ (X{{V}_{gs}}+Y)\left( 1-\exp \left( {{\lambda }_{1}} \right) \right)+B \right]} \\ & = {{V}_{gs}}+H+\left( 1/\sinh \left( -{{\lambda }_{1}} \right) \right)\sqrt{\begin{align} & {{X}^{2}}\left( \exp \left( -{{\lambda }_{1}} \right)-1 \right)\left( 1-\exp \left( {{\lambda }_{1}} \right) \right)V_{gs}^{2}+ \\ & \left( Y\left( 1-\exp \left( {{\lambda }_{1}} \right) \right)+B \right)\left( \exp \left( -{{\lambda }_{1}} \right)-1 \right)X{{V}_{gs}}+ \\ & \left( Y\left( \exp \left( -{{\lambda }_{1}} \right)-1 \right)-B \right)\left( 1-\exp \left( {{\lambda }_{1}} \right) \right)X{{V}_{gs}}+ \\ & \left( Y\left( \exp \left( -{{\lambda }_{1}} \right)-1 \right)-B \right)\left( Y\left( 1-\exp \left( {{\lambda }_{1}} \right) \right)+B \right) \\ \end{align}} \\ & = {{V}_{gs}}+H+\left( 1/\sinh \left( -{{\lambda }_{1}} \right) \right)\sqrt{{{a}_{1}}V_{gs}^{2}+{{a}_{2}}{{V}_{gs}}+{{a}_{3}}} = 0 \\ \end{align}$

(A-1)

$\begin{align} & X = \frac{-2}{J_{0}^{2}({{\alpha }_{1}})+J_{1}^{2}({{\alpha }_{1}})}\frac{{{J}_{1}}({{\alpha }_{1}})}{{{\alpha }_{1}}} \\ & Y = \left( -{{\phi }_{MS}}+\frac{q{{N}_{D}}R}{2{{C}_{ox}}}+\frac{q{{N}_{D}}{{R}^{2}}}{4{{\varepsilon }_{si}}} \right) +\frac{q{{N}_{D}}}{2{{\varepsilon }_{si}}}\frac{1}{J_{0}^{2}({{\alpha }_{1}})+J_{1}^{2}({{\alpha }_{1}})}\frac{{{\alpha }_{1}}{{J}_{1}}({{\alpha }_{1}})-2{{J}_{2}}({{\alpha }_{1}})}{{{\left( {{\alpha }_{1}}/R \right)}^{2}}} \\ & B = \frac{2{{V}_{ds}}}{J_{0}^{2}({{\alpha }_{1}})+J_{1}^{2}({{\alpha }_{1}})}\frac{{{J}_{1}}({{\alpha }_{1}})}{{{\alpha }_{1}}} = -X{{V}_{ds}} \\ & {{a}_{1}} = {{X}^{2}}\left( \exp \left( -{{\lambda }_{1}} \right)-1 \right)\left( 1-\exp \left( {{\lambda }_{1}} \right) \right) \\ & {{a}_{2}} = \left( Y\left( 1-\exp \left( {{\lambda }_{1}} \right) \right)+B \right)\left( \exp \left( -{{\lambda }_{1}} \right)-1 \right)X+\left( Y\left( \exp \left( -{{\lambda }_{1}} \right)-1 \right)-B \right)\left( 1-\exp \left( {{\lambda }_{1}} \right) \right)X \\ & {{a}_{3}} = \left( Y\left( \exp \left( -{{\lambda }_{1}} \right)-1 \right)-B \right)\left( Y\left( 1-\exp \left( {{\lambda }_{1}} \right) \right)+B \right) \\ \end{align}$

(A-2)

If you find V_gs using the quadratic formula of (A-1), this is V_th of (2.14).

B. Analytical DIBL

The DIBL is defined as follows.

$DIBL = -\frac{d{{V}_{th}}}{d{{V}_{ds}}}$

Therefore, it can be found by differentiating (14) by V_ds. In (14), the only variables for V_ds are a₂ and a₃ with B, so

$DIBL = -\frac{d{{V}_{th}}}{d{{V}_{ds}}} = \frac{1}{2\left[ \frac{{{a}_{1}}}{{{\sinh }^{2}}\left( {{\lambda }_{1}} \right)}-1 \right]}\\\left\{ \left( \frac{1}{{{\sinh }^{2}}\left( {{\lambda }_{1}} \right)} \right)\frac{d{{a}_{2}}}{d{{V}_{ds}}}-\frac{\left\{ \begin{align} & \left( \frac{{{a}_{2}}}{{{\sinh }^{2}}\left( {{\lambda }_{1}} \right)}-2H \right)\left( \frac{1}{{{\sinh }^{2}}\left( {{\lambda }_{1}} \right)} \right)\frac{d{{a}_{2}}}{d{{V}_{ds}}}- \\ & 2\left( \frac{{{a}_{1}}}{{{\sinh }^{2}}\left( {{\lambda }_{1}} \right)}-1 \right)\left( \frac{1}{{{\sinh }^{2}}\left( {{\lambda }_{1}} \right)} \right)\frac{d{{a}_{3}}}{d{{V}_{ds}}} \\ \end{align} \right\}}{\sqrt{\begin{align} & {{\left[ {{a}_{2}}/{{\sinh }^{2}}\left( {{\lambda }_{1}} \right)-2H \right]}^{2}}- \\ & 4\left[ {{a}_{1}}/{{\sinh }^{2}}\left( {{\lambda }_{1}} \right)-1 \right]\left[ {{a}_{3}}/{{\sinh }^{2}}\left( {{\lambda }_{1}} \right)-{{H}^{2}} \right] \\ \end{align}}} \right\}$

(B-1)

In (B-1), the derivative of a₂ and a₃ with respect to V_ds is obtained using (A-2) as followings.

$\begin{align} & \frac{d{{a}_{2}}}{d{{V}_{ds}}} = -{{X}^{2}}\left( \exp \left( -{{\lambda }_{1}} \right)+\exp \left( {{\lambda }_{1}} \right)-2 \right) \\ & \frac{d{{a}_{3}}}{d{{V}_{ds}}} = -XY\left( \exp \left( -{{\lambda }_{1}} \right)+\exp \left( {{\lambda }_{1}} \right)-2 \right)-2{{X}^{2}}{{V}_{ds}} \\ \end{align}$

(B-2)

If (B-2) is substituted for (B-1), the DIBL of (B-3) can be obtained.

$DIBL = -\frac{1}{2\left[ {{a}_{1}}-{{\sinh }^{2}}\left( {{\lambda }_{1}} \right) \right]}\\\left\{ \begin{align} & {{X}^{2}}\left( \exp \left( -{{\lambda }_{1}} \right)+\exp \left( {{\lambda }_{1}} \right)-2 \right)+ \\ & \frac{\left\{ \begin{align} & \left( \frac{{{a}_{2}}}{{{\sinh }^{2}}\left( {{\lambda }_{1}} \right)}-2H \right)\left( -{{X}^{2}}\left( \exp \left( -{{\lambda }_{1}} \right)+\exp \left( {{\lambda }_{1}} \right)-2 \right) \right)- \\ & 2\left( \frac{{{a}_{1}}}{{{\sinh }^{2}}\left( {{\lambda }_{1}} \right)}-1 \right)\left( -XY\left( \exp \left( -{{\lambda }_{1}} \right)+\exp \left( {{\lambda }_{1}} \right)-2 \right)-2{{X}^{2}}{{V}_{ds}} \right) \\ \end{align} \right\}}{\sqrt{{{\left[ {{a}_{2}}/{{\sinh }^{2}}\left( {{\lambda }_{1}} \right)-2H \right]}^{2}}-4\left[ {{a}_{1}}/{{\sinh }^{2}}\left( {{\lambda }_{1}} \right)-1 \right]\left[ {{a}_{3}}/{{\sinh }^{2}}\left( {{\lambda }_{1}} \right)-{{H}^{2}} \right]}} \\ \end{align} \right\}$

(B-3)

References

[1]	Afzalian A (2021) Ab initio perspective of ultra-scaled CMOS from 2D-material fundamentals to dynamically doped transistors. npj 2D Mater Appl 5: 1-13. https://doi.org/10.1038/s41699-020-00181-1 doi: 10.1038/s41699-020-00190-0
[2]	Xu K, Chen D, Yang F, et al. (2017) Sub-10 nm Nanopattern Architecture for 2D Material Field-Effect Transistor. Nano Lett 17: 1065-1070. https://doi.org/10.1021/acs.nanolett.6b04576 doi: 10.1021/acs.nanolett.6b04576
[3]	Menduratta N, Tripathi SL (2020) A review on performance comparison of advanced MOSFET structures below 45 nm technology node. J Semicond 41: 061401. https://doi.org/10.1088/1674-4926/41/6/061401 doi: 10.1088/1674-4926/41/6/061401
[4]	Lee J, Choi K (2016) Trend and issues of the bulk FinFET. Vacuum Magazine 3: 16-21. https://doi.org/10.5757/vacmac.3.1.16 doi: 10.5757/vacmac.3.1.16
[5]	Chiarella T, Kubicek S, Rosseel E, et al. (2016) Towards High Performance Sub-10 nm finW Bulk FinFET Technology. 2016 46th European Solid-State Device Research Conference (ESSDERC), Lausanne Switzerland, 131-134. https://doi.org/10.1109/ESSDERC.2016.7599605
[6]	Zhao D, Tian Z, Liu H, et al. (2020) Realizing an Omega-Shaped Gate MoS2 Field-Effect Transistor Based on a SiO2/MoS2 Core-Shell Heterostructure. ACS Appl Mater Inter 12: 14308-14314. https://doi.org/10.1021/acsami.9b21727 doi: 10.1021/acsami.9b21727
[7]	Agarwal A, Pradhan PC, Swain BP (2020) Corrected Drain Current Model for Schottky Barrier Cylindrical Gate All Around FET Considering Quantum Mechanical Effects. Telecommunications and Radio Engineering 79: 433-442. https://doi.org/10.1615/TelecomRadEng.v79.i5.60 doi: 10.1615/TelecomRadEng.v79.i5.60
[8]	Dargar A, Srivastava VM (2021) Performance Comparison of Stacked Dual-Metal Gate Engineered Cylindrical Surrounding Double-Gate MOSFET. Int J Electron Telec 67: 29-34.
[9]	Sharma S, Chaudhury K (2012) A Novel Technique for Suppression of Corner Effect in Square Gate All Around MOSFET. Electrical and Electronic Engineering 2: 336-341.
[10]	Lee J, Kang M (2021) TID Circuit Simulation in Nanowire FETs and Nanosheet FETs. Electronics 10: 956. https://doi.org/10.3390/electronics10080956 doi: 10.3390/electronics10080956
[11]	Colinge JP, Kranti A, Yan R, et al. (2011) Junctionless Nanowire Transistor (JNT): Properties and design guidelines. Solid-State Electronics 65: 33-37. https://doi.org/10.1016/j.sse.2011.06.004 doi: 10.1016/j.sse.2011.06.004
[12]	Nowbahari A, Roy A, Marchetti L (2020) Junctionless Transistors: State-of-the-Art. Electronics 9: 1174. https://doi.org/10.3390/electronics9071174 doi: 10.3390/electronics9071174
[13]	Lee C, Borne A, Ferain I, et al. (2010) High-Temperature Performance of Silicon Junctionless MOSFETs. IEEE T Electron Dev 57: 620-625. https://doi.org/10.1109/TED.2009.2039093 doi: 10.1109/TED.2009.2039093
[14]	Huda ARN, Arshad MKM, Othman N, et al. (2015) Impact of Size Variation in Junctionless vs Junction Planar SOI n-MOSFET Transistor. 2015 IEEE Regional Symposium on Micro and Nanoelectronics(RSM), Kuala Terengganu, 1-4. https://doi.org/10.1109/RSM.2015.7354983
[15]	Jung H (2019) Analysis of Threshold Voltage Roll-off and Drain Induced Barrier Lowering in Junction-Based and Junctionless Double Gate MOSFET. Journal of the Korean Institute of Electrical and Electronic Material Engineers 32: 104-109. https://doi.org/10.21660/2019.55.4510 doi: 10.21660/2019.55.4510
[16]	Jung H (2021) Analysis of subthreshold swing in junctionless double gate MOSFET using stacked high-k gate oxide. International Journal of Electrical and Computer Engineering 11: 240-248. https://doi.org/10.11591/ijece.v11i1.pp240-248 doi: 10.11591/ijece.v11i1.pp240-248
[17]	Manikanda S, Balamurugan NB, Nirmal D (2020) Analytical Model of Double Gate Stacked Oxide Junctionless Transistor Considering Source/Drain Depletion Effetcs for CMOS Low Power Application. Silicon 12: 2053-2063. https://doi.org/10.1007/s12633-019-00280-9 doi: 10.1007/s12633-019-00280-9
[18]	Haque M, Kabir H, Adnan MR (2021) Analytical modelling and verification of potential profile of DG JLFET with and without stack oxide. Int J Electron 108: 819-840. https://doi.org/10.1080/00207217.2020.1818842 doi: 10.1080/00207217.2020.1818842
[19]	Baral K, Singh PK, Kumar S, et al. (2020) Ultrathin body nanowire hetero-dielectric stacked asymmetric halo doped junctionless accumulation model MOSFET for enhanced electrical chracteristics and negative bias stability. Superlattice Microst 138: 106364. https://doi.org/10.1016/j.spmi.2019.106364 doi: 10.1016/j.spmi.2019.106364
[20]	Swain SK, Biswal SM, Das SK, et al. (2020) Performance Comparison of InAs Based DG-MOSFET with Respect to SiO2 and Gate Stack Configuration. Nanoscience & Nanotechnology-Asia 10: 419-424. https://doi.org/10.2174/2210681209666190919094434 doi: 10.2174/2210681209666190919094434
[21]	Panchanan S, Maity R, Maity NP (2021) Modeling, Simulation and Analysis of Surface Potential and Threshold Voltage: Application to High-K Material HfO₂ Based FinFET. Silicon 13: 3271-3289. https://doi.org/10.1007/s12633-020-00607-x doi: 10.1007/s12633-020-00607-x
[22]	Maity NP, Maity R, Maity S, et al (2019) A New Surface Potential and Drain Current Model of Dual Material Gate Short Channel Metal Oxide Semiconductor Field Effect Transistor in Subthreshold Regime: Application to High-K material HfO₂. J Nanoelectron Optoe 14: 868-876. https://doi.org/10.1166/jno.2019.2547 doi: 10.1166/jno.2019.2547
[23]	Rasol MFM, Hamid FKA, Johari Z, et al. (2019) Stacking SiO2/High-k Dielectric Material in 30 nm Junction-less Nanowire Transistor Optimized Using Taguchi Method for Lower Leakage Current. 2019 IEEE Regional Symposium on Micro and Nanoelectronics (RSM), Pahang, 1-4. https://doi.org/10.1109/RSM46715.2019.8943545
[24]	Darwin S, Samuel TSA, Vimala P (2020) Impact of two gate oxide with no junction metal oxide semiconductor field effect transistor- an analytical model. Physica E: Low-dimensional Systems and Nanostructures 118: 113803. https://doi.org/10.1016/j.physe.2019.113803 doi: 10.1016/j.physe.2019.113803
[25]	Kosmani NF, Mamid FA, Razali MA (2020) Effect of High-k Dielectric Materials on Electrical Performance on Double Gate and Gate-All-Around MOSFET. Int J Integ Eng 12: 81-88.
[26]	Gupta S, Pandey N., Gupta RS (2020) Investigation of Dual-Material Double Gate Junctionless Accumulation-Mode Cylindrical Gate All Around(DMDG-JLAM-CGAA) MOSFET with High-k Gate Stack for Low Power Digital Applications. 2020 IEEE 17th india Council International Conference (INDICON), New Delhi 1-4. https://doi.org/10.1109/INDICON49873.2020.9342380
[27]	Gupta V, Awasthi H, Kumar N et al. (2021) A Novel Approach to Model Threshold Voltage and Subthreshold Current of Graded-Doped Junctionless-Gate-All-Around(GD-JL-GAA) MOSFETs. Silicon 1-9. https://doi.org/10.1007/s12633-021-01084-6 doi: 10.1007/s12633-021-01084-6
[28]	Hu G, Xiang P, Ding Z, et al. (2014) Analytical Models for Electric Potential, Threshold Voltage, and Subthreshold Swing of Junctionless Surrounding-Gate Transistors. IEEE T Electron Dev 61: 688-695. https://doi.org/10.1109/TED.2013.2297378 doi: 10.1109/TED.2013.2297378
[29]	Maduagwu UA, Srivastava VM (2019) Analytical Performance of the Threshold Voltage and Subthreshold Swing of CSDG MOSFET. Journal of Low Power Electronics and Applications 9: 10. https://doi.org/10.3390/jlpea9010010 doi: 10.3390/jlpea9010010
[30]	Li C, Zhuang Y, Di S., et al. (2013) Subthreshold Behavior Models for Nanoscale Short-Channel Junctionless Cylindrical Surrounding-Gate MOSFETs. IEEE T Electron Dev 60: 3655-3662. https://doi.org/10.1109/TED.2013.2281395 doi: 10.1109/TED.2013.2281395
[31]	Duksh YS, Singh B, Gola D, et al. (2021) Subthreshold Modeling of Graded Channel Double Gate Junctionless FETs. Silicon 13: 1231-1238. https://doi.org/10.1007/s12633-020-00514-1 doi: 10.1007/s12633-020-00514-1
[32]	Gupta SK (2015) Threshold voltage model of junctionless cylindrical surrounding gate MOSFETs including fringing field effects. Supperlattices and Microstructures 88: 188-197. https://doi.org/10.1016/j.spmi.2015.09.001 doi: 10.1016/j.spmi.2015.09.001
[33]	Ehteshanuddin M, Loan SA, Rafat M (2017) Excellent DIBL Immunity in Junctionless Transistor on a High-k Buried Oxide. 2017 IEEE 14th india Council International Conference (INDICON), Roorke, 1-4. https://doi.org/10.1109/INDICON.2017.8487497
[34]	Bhagat K, Patil GC (2020) Engineering substrate doping in bulk planar junctionless transistor: Scalability and variability study. Engineering Research Express 2: 025028. https://doi.org/10.1088/2631-8695/ab91f7 doi: 10.1088/2631-8695/ab91f7
[35]	Lagraf F, Rechem D, Guergouri K, et al. (2019) Channel Length Effect on Subthreshold Characteristics of Junctionless Trial Material Cylindrical Surrounding-Gate MOSFET with High-k Gate Dielectrics. Journal of Nano- and Electronic Physics 11: 02011. https://doi.org/10.21272/jnep.11(2).02011 doi: 10.21272/jnep.11(2).02011
[36]	Clark RD (2014) Emerging Applications for High K Materials in VLSI Technology. Materials 7: 2913-2944. https://doi.org/10.3390/ma7042913 doi: 10.3390/ma7042913

This article has been cited by:

Suruchi Saini, Hitender Kumar Tyagi, 2024, Chapter 26, 978-981-97-0699-0, 337, 10.1007/978-981-97-0700-3_26

Reader Comments

Your name:*

Email:*
© 2022 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

AIMS Electronics and Electrical Engineering

2.4

Metrics

Article views(3142) PDF downloads(236) Cited by(1)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

Figures and Tables

Figures(9) / Tables(1)

AIMS Electronics and Electrical Engineering