Capacity drop and traffic control for a second order traffic model

1.   Introduction

The description of vehicular traffic flow based on systems of conservation or balance laws has been proposed by many researchers during the last decades, see for example [9,30] and the references therein. In contrast to first order traffic models consisting only of one scalar equation for the traffic density, second order models are characterized by a second equation for the evolution of traffic mean velocity. Among these, Aw and Rascle [2] proposed a model that overcomes the drawbacks of previous models, and is capable to capture some traffic characteristics linked to the anticipation behavior of drivers. Greenberg [12] presented an extension of the Aw-Rascle model including a relaxation term towards a preferred (or equilibrium) velocity. Since then many contributions have appeared on theoretical and numerical investigations [1,3,4,10], networked problems [8,13,15,16,26], and more recently phenomenological and data driven approaches [7,17,21,24,29].

In this work, we present results concerning the coupling of interlinked road networks to on-ramps. It is known that in such situations capacity drops occur for high traffic densities, see e.g. [13,17,21,23,29]. Capacity drop means that the measured outflow of the system is smaller than the expected maximal flow if the system is well utilized. The difference might be up to 10% or even higher [17,29]. This can be explained by a less efficient driving style when exiting a congested zone (upstream the on-ramp), which reduces the downstream flow compared to free-flow conditions [30].

The coupling of on-ramps to roads is similar to the modeling of a merge [18]. However, in contrast to the majority of already existing approaches, here we assume that the on-ramp is given by an ordinary differential equation, see [6,11]. For second order models, the challenge here is to find appropriate conditions to ensure the conservation of mass and momentum flow. Once these coupling conditions are defined, they can be integrated in a finite-volume type numerical scheme to compute the evolution of traffic conditions on the network. We use a first-order Godunov scheme combined with a time splitting to resolve the relaxation term. In particular, we observe that the model is able to reproduce the capacity drop effect for increasing inflows, similar to the study made in [13] and in contrast to [23], where the phenomenon is only observed for a short time period.

Furthermore, our approach gives the opportunity to consider questions of optimal control in a first-discretize-then-optimize framework. Typical control issues arising in the on-ramp context are speed limit and ramp metering. These kind of traffic flow control problems have been mainly studied in the context of first-order models based on the Lighthill-Whitham-Richards (LWR) equations [22], see e.g. [5,11,14,25]. To the best of our knowledge, speed limit and ramp meter control has been not considered for the Aw-Rascle model so far. We discuss the numerical results obtained from the adjoint calculus and compare them to the LWR equations.

The outline is as follows: In Section 2 we present the Aw-Rascle model with relaxation term and the coupling conditions at on-ramps. Our discretization scheme will be introduced in Section 3. Numerical results concerning the capacity drop and optimal control issues can be found in Section 4. Comparisons to the solution of the LWR model are also given for all experiments.

2.   Modeling

We introduce the modeling equations given by the Aw-Rascle (AR) model [2] including a relaxation term as originally introduced in [12]. The model consists of a $2\times2$ system of conservation laws for the density and a sort of generalized momentum derived from the (anticipate) acceleration equation.

In this work, our focus is on the coupling of the Aw-Rascle model to on-ramps whose dynamics are modeled by ordinary differential equations. We will derive appropriate coupling conditions that allow for the definition of boundary conditions at junctions. As we will see in the numerical experiments, our modeling approach covers the capacity drop effect and allows to solve optimal control problems such as speed limit and ramp metering, cf. Section 4.3.

2.1.   Road dynamics

We briefly recall the Aw-Rascle traffic flow model [2] and explain how it can be extended to the context of networks. Traffic states are described by the density $\rho_i(x,t)$ and the mean speed of vehicles $v_i(x,t)$ on each road $i$ at position $x$ and time $t$.

Given some initial state $\big( \rho_i(x,0),v_i(x,0) \big)$ on each road $i$, the dynamics for $x\in(0,L_i)$ and $t\in(0,T)$ are described by [2,12]

or in conservative form with $y_i = \rho_i w_i$

where $p_i(\rho)$ is a known pressure function satisfying $p_i'(\rho) > 0$ and $\rho p''_i(\rho) + 2p_i'(\rho)>0$ for all $\rho.$ The latter condition ensures that the curve $\{w_i(\rho,v) = v + p_i(\rho) \stackrel{!}{=} c\}$ for any constant $c > 0$ is strictly concave and passes through the origin of the $(\rho,\rho v)$-plane. Further, there exists a sonic point $\sigma_i(c)$ maximizing the flux $\rho v$ along the curve $\{w_i(\rho,v) = c\}.$ The relaxation term in the velocity equation includes the preferential speed function $V_i(\rho)$ which the drivers tend to adopt and a relaxation parameter $\delta$.

We consider the preferential velocity depending on the density,

and the pressure function (as in [13,23])

equipped with maximal density $\rho_i^{\max}>0$, maximal velocity $v_i^{\max}>0$, reference velocity $v_i^{\rm{ref}}>0$ and $\gamma_i>0$. Later, in Section 4.3, we will also consider time dependent velocities $v_i^{\max}(t)$ and $v_i^{\rm{ref}}(t)$ so that actually $p_i(\rho) = p_i(\rho,t)$, $V_i(\rho) = V_i(\rho,t)$ and $g_i(\rho,y) = g_i(\rho,y,t)$.

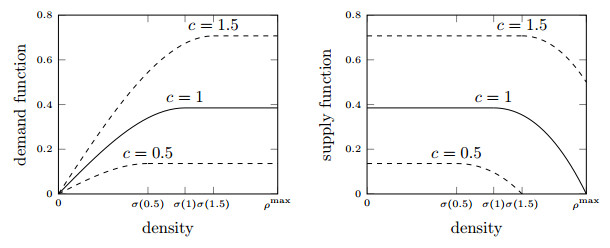
Note that above and in the following, we use $w_i = w_i(x,t)$ as space and time dependent state variable but also as function, e.g. in the form $w_i(\rho,v,t) = v + p_i(\rho,t)$. Analogously, we will use the notation $v_i(U,t) = w - p_i(\rho,t)$ for the velocity of a state $U = (\rho,\rho w)$. Similar to first order traffic models, we define the demand and supply functions for each road $i$ as follows: For a given constant $c$ (corresponding to a fixed value of $w$) we have

where

is the sonic point on the curve $\{w_i(\rho,v,t) = v + p_i(\rho,t) \stackrel{!}{=} c\}$ in the $(\rho,\rho v)$-plane. An illustration of the considered demand and supply functions is given in Figure 1. Supply and demand functions are needed to formulate the coupling of different roads or on-ramps. Note that due to the dependence on a given value of $w$, the value of the supply function at $\rho^{\max}$ may differ from zero. Even though this can be considered as a potential drawback of the applied model, the effect is compensated by the relaxation term to a great extent, since the latter forces the velocity and therewith the flux to zero when the density approaches $\rho^{\max}$.

2.2.   Coupling and boundary conditions

In the following, we present the serial coupling between single roads as well as the coupling with an on-ramp. In general, for well-posed coupling conditions in networks of hyperbolic conservation laws, one considers (half-)Riemann problems defining the set of admissible states for each road at the junction, i.e., states such that all waves produced by the Riemann problem have negative/positive speed if the considered road is an incoming/outgoing road (cf. [13]). Under these constraints, the flow at the junction is maximized, where additional conditions have to be posed to achieve uniqueness of the solution. Those conditions as well as the flow maximization are already included in the following description.

The considered coupling and boundary conditions can be given in terms of mass flow $q=\rho v$ and ''momentum flow'' $q w$. The computation of the actual states at a junction is not necessary (cf. the discretization in Section 3). Different to other coupling conditions [13,15,18,23,26], we assume that in the situation of a merge the priority parameter $P$ is independent of the demand of the ingoing roads. This guarantees the consistency of the corresponding Riemann solver, see [9,Definition 4.2.2].

1-to-1 junction. We use index 1 for the ingoing road, index 2 for the outgoing road, and consider the data $U_{i} = (\rho_{i}, \rho_{i} w_{i})$ at the adjacent boundaries of roads 1 and 2, respectively. Note that we omit the time dependency of the states $U_i$ and the fluxes $q_i$ in the following for a better reading, whereas we still indicate the explicit time dependency of the demand and supply functions.

Flow maximization at the junction over all admissible states leads to

where $\tilde\rho_2$ is either obtained by the intersection of the curves

or $\tilde \rho_2 = 0$. Note that for the considered pressure functions this problem can be solved explicitly:

Then, $q_1 = q_2 = \tilde q$ determines the mass flow out of road 1/into road 2. Further, with $\tilde w = w_{1}$ at the junction, the momentum flow is given by $\tilde q \tilde w$.

On-ramp at a 1-to-1 junction. We consider a 1-to-1 junction with an on-ramp. Here, we use index 1 for the ingoing road, index 2 for the on-ramp, index 3 for the outgoing road, and consider the data $U_{i} = (\rho_{i}, \rho_{i} w_{i})$ on roads 1 and 3, respectively, and the queue length $l_{2}$ at the on-ramp.

For the demand $D_2(l,t)$ at the on-ramp we apply

where $l$ is the length of the queue, $u_2(t)\in[0,1]$ is the (time-dependent) metering rate, $f_2^\text{in}(t)$ the ''inflow'' of cars arriving at the on-ramp and $f_2^{\max}$ the maximum flow onto the main road.

To get a unique solution, we assign the priority parameter $P$ to road 1, and apply

where $\tilde\rho_3$ is either obtained by the intersection of the curves

or $\tilde\rho_3 = 0$.

Again, the density $\tilde \rho_3$ can be computed explicitly:

As before, the momentum flow is computed by multiplication of $q_1$ and $q_3$ with $\tilde w = w_{1}$, while the formal momentum flow $\tilde w q_2$ from the on-ramp does not influence the state of the on-ramp and is therefore not computed.

The evolution of the queue at the on-ramp is given by

where we typically start with empty queues, i.e., $l_2(0) = 0$.

On-ramp at origin. We consider an on-ramp at a vertex with only one outgoing road. We use index 1 for the on-ramp with queue length $l_{1}$, and index 2 for the road with data $U_{2} = (\rho_{2}, \rho_{2} w_{2})$.

Similar to above we apply

for the demand at the on-ramp, where $l$ is the length of the queue, $u_1(t)\in[0,1]$ is the (time-dependent) metering rate, $f_1^\text{in}(t)$ the inflow of cars arriving at the on-ramp and $f_1^{\max}$ the maximum flow onto the road.

In this case there is no value for $w$ to evaluate the supply function of the road, which is needed to determine the actual flux onto the road $\tilde q$ in a similar fashion as in the case of a simple 1-to-1 junction, i.e., similar to (10). Therefore, we consider an auxiliary left state $U_{1}$ mimicking the desired inflow of the on-ramp. We assume that the velocity of the auxiliary state is at equilibrium with respect to the desired velocity of the road, i.e., we search for a state

where $\tilde w = w_2(\rho,V_2(\rho,t),t)$. Assuming that the flux $D_1(l,t) \le f_1^{\max}$ can be realized with the velocity function $V_2$, we get the solutions

Here we choose $\rho_-$, since this choice also fulfils

Finally, we apply

with $\tilde w = w_2(\rho_-,V_2(\rho_-,t),t)$, and $\tilde\rho_2$ is either obtained by the intersection of the curves

or $\tilde\rho_2 = 0$. The explicit representation is the same as (12).

Similar to above, the evolution of the queue at the on-ramp is given by

Outflow conditions. At nodes with only one ingoing road (with index 1) we consider absorbing boundary conditions up to a given maximum flow rate $f_1^{\rm{out}}(t)$:

The momentum flow is given by $q_1 w_{1}$.

3.   Discretization

For the numerical solution of the described model, a finite number of time points $t^n = n \Delta t$ is considered, where $\Delta t = T/Nt$. Further, each road $i$ is divided into $Nx_i$ cells of equal size $\Delta x_i = L_i / Nx_i$. To fulfill the CFL condition, we will claim (at least) $\Delta t\, v_i^{\max} \le \Delta x_i$ in all numerical examples.

3.1.   Roads

The underlying balance law (4) is discretized with a fractional step method: We use a first order Godunov scheme for the flux term and an implicit Euler method for the relaxation term. Due to the fundamental idea of the Godunov scheme, the computation of the actual states at junctions is not necessary and the coupling will be given in terms of fluxes only.Note that in the following $U_i=(\rho_i,\rho_i w_i)$ denotes again the state.

On each road $i$ the initial conditions (for $j \in \{1,\dots,Nx_i\}$) are given by the cell averages

Then, for $n\in\{0,\dots,Nt-1\}$ and $j\in\{1,\dots,Nx_i\}$ we apply

The flux terms $F_{i,0}^n$ and $F_{i,Nx_i}^n$ are given via coupling/boundary conditions (see next subsection) and for $j\in\{1,\dots,Nx_i-1\}$ we have

with

where $\tilde \rho_{i,j}^n$ is either obtained by the intersection of the curves

or $\tilde \rho_{i,j}^n = 0$. Note that this treatment is consistent with the treatment of a 1-to-1 junction where two roads with the same parameters meet.

3.2.   Coupling and boundary conditions

We complete the numerical scheme by the description of the coupling and boundary conditions according to the model equations from Section 2.2. As already mentioned, we only need the coupling fluxes here and not the actual states at the junctions.

1-to-1 junction. As before, we use index 1 for the ingoing road and index 2 for the outgoing road. According to (10) we apply for $n \in \{0, \dots, Nt-1\}$

where $\tilde \rho^n_{2,0}$ is either obtained by the intersection of the curves

or $\tilde \rho^n_{2,0} = 0$. Due to the choice of the pressure function (6), $\tilde \rho^n_{2,0}$ can be directly computed according to (12).Finally, we get

On-ramp at a 1-to-1 junction. We use index 1 for the ingoing road, index 2 for the on-ramp and index 3 for the outgoing road. According to (13) we apply

for the demand at the on-ramp. Then, using the abbreviations

we get from (14) and (15)

where $\tilde\rho_{3,0}^n$ is either obtained by the intersection of the curves

or $\tilde\rho_{3,0}^n = 0$. Again, in our case a direct computation is possible due to (18).Finally, we have

and apply

for the update of the queue length via the explicit Euler scheme. Note that we always achieve a non-negative queue length by our choice of the demand function (37). Alternatively, the time step $\Delta t$ could be adapted, cf. [6].

On-ramp at origin. We use index 1 for the on-ramp and index 2 for the road. Similar to above we apply

for the demand at the on-ramp. Next, following the idea introduced in Section 2.2, we compute

and

with $\tilde w^n = w_2(\rho^n_{1,-}, V_2(\rho^n_{1,-},t^n),t^n)$, and $\tilde\rho_{2,0}^n$ is either obtained by the intersection of the curves

or $\tilde\rho_{2,0}^n = 0$. Again, $\tilde\rho_{2,0}^n$ can be computed explicitly according to (12). Finally, we use

and apply

for the update of the queue length. Again, non-negativity of the queue length is ensured by our choice of the demand function (47).

Outflow conditions. At nodes with only one ingoing road (with index 1) we apply according to (27)

with

In the next section, we present a numerical study to show the characteristics of our modeling approach. We also comment on already existing examples from the literature, pointing out differences and similarities.

4.   Numerical results

Our numerical results emphasize on three different scenarios that our approach is able to deal with from a modeling and computational point of view. First, we show that, for a single road, the Aw-Rascle (AR) model with relaxation term numerically converges to the Lighthill-Whitham-Richards (LWR) model as expected [12]. The second example considers the capacity drop for a 1-to-1 junction with on-ramp. We will see that this phenomenon is not covered by the classical LWR model. The third example tackles speed control and coordinated ramp metering control issues. It will turn out that the control strategies considered individually do not lead to the best possible travel times, while the combination of both lead to satisfying results.

4.1.   Aw-Rascle towards LWR

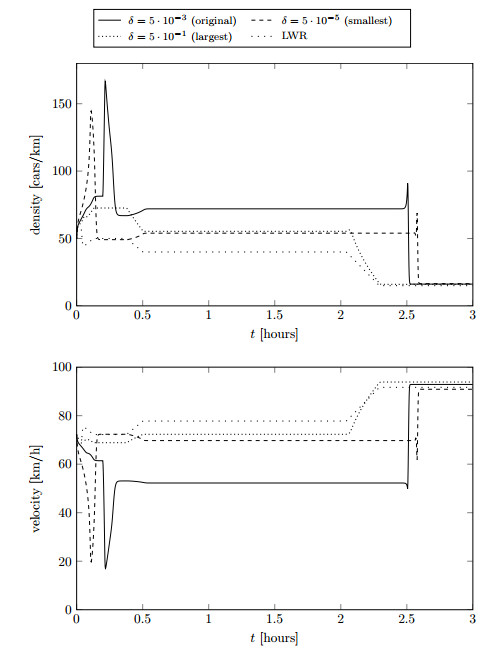
We consider a single road as depicted in Figure 6 with length $L = 1 \, km$, $\rho^{\max} = 200 \, \frac{\rm{cars}}{\rm{km}}$, $v^{\max} = v^{\rm{ref}} = 100 \, \frac{\rm{km}}{\rm{h}}$ and $\rho_0 = 80 \, \frac{\rm{cars}}{\rm{km}}$ as initial density. For the AR model we further use $\gamma = 2$, $v_0 = 60 \, \frac{\rm{km}}{\rm{h}}$ (in equilibrium state) and decreasing values for the relaxation parameter $\delta$ starting from a usual value of $\delta = 0.005$ hours (18 seconds, see e.g. [14]).

We consider a simulation time of $T = 36$ seconds and

as inflow profile at the origin ''in'' (in $\frac{\rm{cars}}{\rm{h}}$). As discretization parameters, we apply $\Delta x = 10$ meters and $\Delta t = 0.18$ seconds.

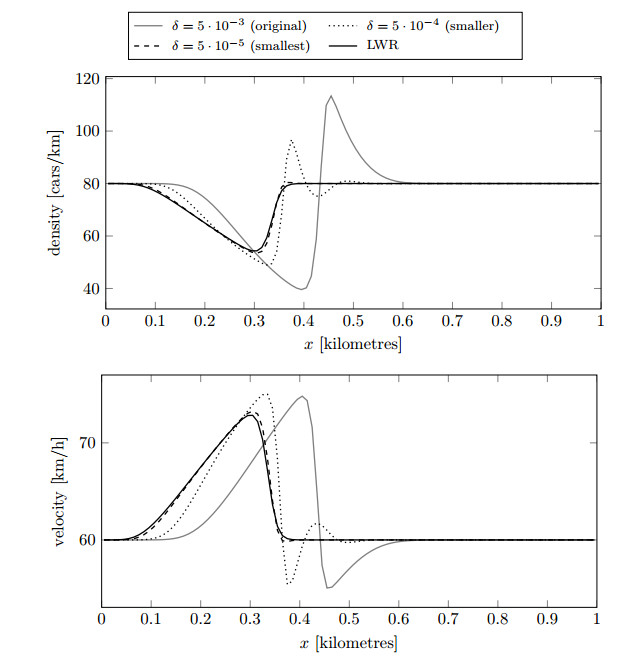
Figure 7 shows density and velocity profiles on the whole road after 36 seconds. As expected, the solution of the Aw-Rascle model with relaxation term tends towards the LWR model for decreasing relaxation parameter $\delta$. Moreover, decreasing densities are correlated with increasing velocities. Note that the application of larger values for the parameter $\gamma$ in the pressure term leads to higher peaks in the simulation results (not shown here).

4.2.   Capacity drop

Now we turn our attention to study the capacity drop effect, cf. [13]. We will see that increasing the inflow into the network depicted in Figure 8 will at a certain point lead to a decreasing outflow. Thus, a significant difference between the effective and maximal possible outflow occurs in the Aw-Rascle model. Conversely, this effect is not captured by the classical LWR model. This is due to the different modeling of the supply function. In the congested regime, the value of the supply function is at its maximum in the LWR model and therefore no capacity drop occurs. However, in the AR model, the supply function additionally depends on the ''incoming'' value of $w$ that allows for a different behavior.

We consider two roads of 1 km length with an on-ramp in between, see Figure 8. On both roads we use $\rho^{\max} = 180\,\frac{\rm{cars}}{\rm{km}}$, $v^{\max} = v^{\rm{ref}} = 100\,\frac{\rm{km}}{\rm{h}}$, $\gamma = 2$, $\delta = 0.005\,\text{h}$ and an initial density of $50 \,\frac{\rm{cars}}{\rm{km}}$. At the origin ''in'' we consider a constant (desired) inflow $f_1^{\rm{in}} = 3500\,\frac{\rm{cars}}{\rm{h}}$. At the on-ramp we consider different (desired) inflows, starting from $f_2^{\rm{in}} = 500\,\frac{\rm{cars}}{\rm{h}}$ up to $2500\,\frac{\rm{cars}}{\rm{h}}$ and down to $500\,\frac{\rm{cars}}{\rm{h}}$ again. As priority parameter at the on-ramp we use $P = 0.5$.

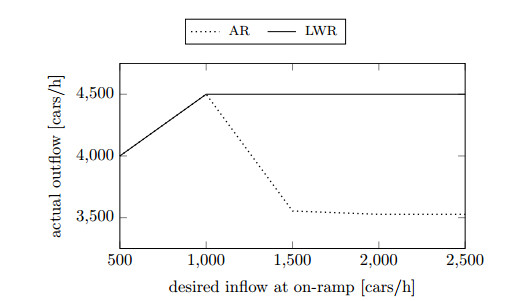
Table 1 and Figure 9 show the simulation results for the AR and the LWR model (with $\Delta x = 100$ metres and $\Delta t = 1.8$ seconds), i.e., the resulting stationary states. The first two columns report the desired and the actual inflow at the on-ramp. The following three values are the resulting density, velocity and the value of $w$ just upstream the on-ramp (end of the first road). The last two columns show the total outflow at the end of the second road.

We begin with the interpretation of the results obtained from the AR model: Obviously, up to an inflow of $1000\,\frac{\rm{cars}}{\rm{h}}$ at the on-ramp, the total inflow (of $4500\,\frac{\rm{cars}}{\rm{h}}$) is within the capacity of the given road. When the inflow at the on-ramp further increases, the resulting total flux cannot be realized at some point. From then on, the value of $w$ at the end of the first road directly influences the total flux entering the second road (compare equations (14) and (15) and the definition of the supply function (8)). As a consequence, the outflow at the end of the second road for the cases $f_2^{\rm{in}} \in \{1500\,\frac{\rm{cars}}{\rm{h}}, \, 2000\,\frac{\rm{cars}}{\rm{h}}, \, 2500\,\frac{\rm{cars}}{\rm{h}} \}$ is lower than the outflow for the cases $f_2^{\rm{in}} \in \{500\,\frac{\rm{cars}}{\rm{h}}, \, 1000\,\frac{\rm{cars}}{\rm{h}} \}$. Due to the choice of the priority parameter $P = 0.5$, the effect of a decreasing outflow while the desired inflow increases stagnates as soon as the fluxes from the first road and the on-ramp are equal, which is the case for $f_2^{\rm{in}} \in \{2000\,\frac{\rm{cars}}{\rm{h}}, \, 2500\,\frac{\rm{cars}}{\rm{h}} \}$.

Interestingly, even when the desired inflow at the on-ramp is lowered again, the original state for the same values of $f_1^{\rm{in}}$ and $f_2^{\rm{in}}$ is not reached. This is due to the fact that the outflow in the congested situation is below the accumulated desired inflows. Accordingly, the queue at the origin permanently increases in the final situation even though the capacity of the road could handle the desired inflows in the free flow situation.

Note that compared to the existing literature the capacity drop is investigated for different situations. In [13] no relaxation term is considered but a similar result is obtained.

The construction principle for the capacity drop is the same (decreasing value of $w$ for increasing flux), but in our investigations the possible equilibrium states are restricted due to the relaxation term so that higher densities on the incoming road lead to lower velocities (in difference to the equilibria 3 and 5 in [13,Table 3]). In [23], where a different junction model and also a different numerical scheme is used, the capacity drop effect for the model with relaxation term is only observed for short time intervals, whereas we identify a permanent drop, cf. Figure 9.

Finally, considering the same scenario with the LWR model (see [11] for the details), one observes that the outflow increases with the accumulated desired inflows until it reaches the maximum capacity of $4500\,\frac{\rm{cars}}{\rm{h}} = \frac{\rho^{\max}}{2} \cdot \frac{v^{\max}}{2}$ (see again Table 1 and Figure 9). According to the chosen priority $P=0.5$, the actual inflows at the origin and at the on-ramp are $2250\,\frac{\rm{cars}}{\rm{h}}$ in that situation. Any further increase of the desired inflow at the on-ramp does not have any effect on the situation on the roads. Further, unlike the AR model, a decrease of the desired inflow $f_2^{\rm{in}}$ below $1000\,\frac{\rm{cars}}{\rm{h}}$ leads back to the original situation (as soon as the queues have emptied).

4.3.   Coordinated speed control and ramp metering

For optimization purposes, we are finally interested in controlling maximal speed limits as well as on-ramp inflows to minimize the total travel time. As already introduced in equations (13) and (20), the ramp metering rate $u_i(t)$ is used to control the demand at the on-ramp. Similar to our recent work [11], we will use $v_i^{\max} = v_i^{\max}(t) \in [v_i^{\rm{low}}, v_i^{\rm{high}}]$ as another time-dependent (piecewise constant) control variable so that actually $V_i(\rho) = V_i(\rho,t)$ and $g_i(\rho,y) = g_i(\rho,y,t)$ for the relaxation term. Regarding the influence of the pressure term, we will consider two variants:

1. $v_i^{\rm{ref}}(t) = v_i^{\max}(t)$, i.e., the pressure term directly depends on the (controllable) speed limit and therefore $p_i(\rho) = p_i(\rho,t)$;

2. $v_i^{\rm{ref}}(t) = v_i^{\rm{high}}$, i.e., the pressure term is independent of the current speed limit in the sense that $\frac{\partial p_i}{\partial v_i^{\max}}=0$.

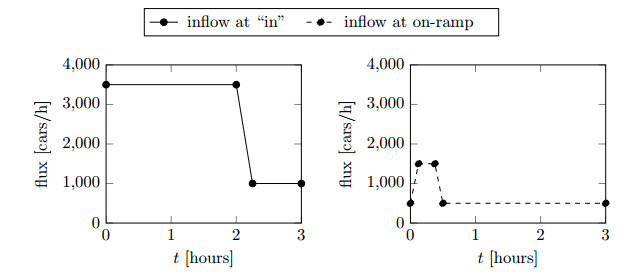
For our investigations, we consider the road network depicted in Figure 10 (adapted from [14]) with the road parameters of Table 2. The exponents in the pressure function are $\gamma_i = \gamma = 2$ for all roads and $\delta = 0.005\, \text{h}$ for the relaxation parameter. The priority parameter $P$ at the on-ramp equals 0.5 and $f^{\max} = 2000\,\frac{\rm{cars}}{\rm{h}}$. At the origin ''in'' we consider $f^{\max} = 4000\,\frac{\rm{cars}}{\rm{h}}$.

We consider a time horizon of $T=3.0$ hours and the boundary conditions shown in Figure 11. For the given scenario, we are interested in minimizing the total travel time

given an upper bound of 100 vehicles in the queue of the on-ramp. To solve this optimization task, we apply a first-discretize-then-optimize approach and adjoint calculus, see also [11]. Thus, for given control decisions (speed limits and metering rates), the discretization scheme described in Section 3 is always used to evaluate the objective function (55) (using the trapezoidal rule for quadrature). Further, sensitivity/gradient information with respect to the control decisions is computed based on the same discretization (we refer to [19,20] for more details). Finally, the SQP solver DONLP2 [27,28] is used for the optimization of the control decisions. The applied discretization parameters are $\Delta x = 250$ meters and $\Delta t = 7.2$ seconds.

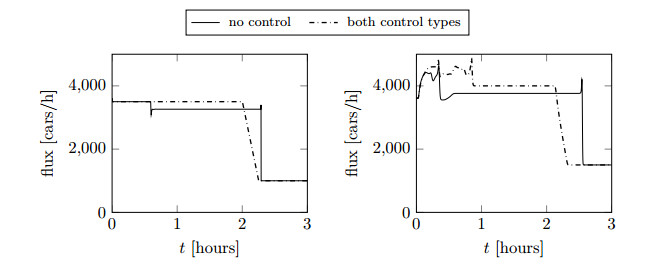
Table 3 shows the total travel times for the different models with and without optimization. The resulting queues for the AR model with scaling of the pressure function ($\frac{\partial p_i}{\partial v_i^{\max}}\ne0$) are shown in Figure 12. Without ramp metering and speed control the queue at the on-ramp stays empty whereas more than 300 cars accumulate in the queue at the origin. When only ramp metering is considered, the queue at the origin is reduced to zero, while up to 100 cars accumulate in the queue at the on-ramp. Obviously, the prescribed upper bound for the queue at the on-ramp is active, impeding further improvement of the objective function. When ramp metering and speed control are used, the upper bound of 100 cars is even never touched. In the case of speed control only, the queue at the on-ramp stays empty, whereas the queue at the origin cannot be kept empty during the complete time horizon.

Note that the results for the model without scaling of the pressure function ($\frac{\partial p_i}{\partial v_i^{\max}}=0$) are quite similar considering the queues and therefore are not plotted. Nevertheless the possible improvement with respect to total travel time is much larger in the case of speed control only (872.6 versus 1122.8). Concerning the LWR model, both queues stay empty during the whole time horizon already in the uncontrolled case and the optimization procedure terminates directly in the other cases -leading to the same result. The optimality of the uncontrolled case with respect to total travel time results from the fact that the classical LWR model does not capture the capacity drop effect (cf. Section 4.2).

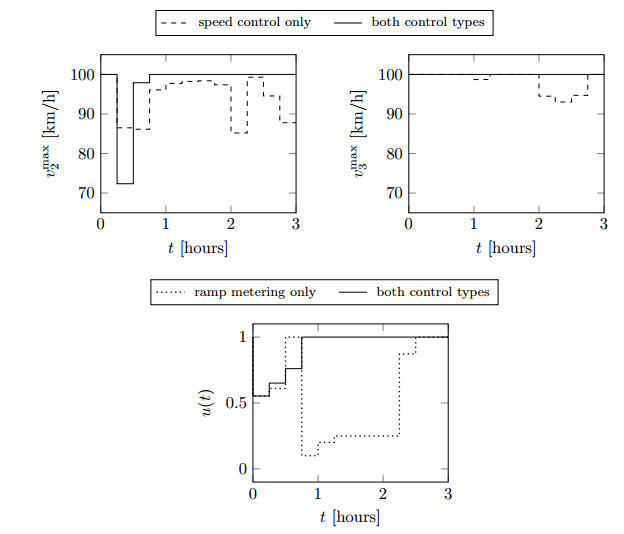
Figure 13 exemplarily shows where the improvement in total travel time comes from in the case of coordinated ramp metering and speed control in the model with scaling of the pressure function: The outflow of the system (plot on the right) in the optimized scenario is permanently above the outflow of the uncontrolled system until it drops to the low inflow level after two hours. Figure 14 shows the applied (optimal) controls.

As shown in Table 3, the uncontrolled case is already optimal for the LWR model, i.e., no ramp metering or speed control is required. Accordingly, we take a look at the influence of the relaxation parameter $\delta$ within the second order model, which regulates the acceleration towards the desired velocity $V(\rho,t)$ of the LWR model.

Table 4 shows the simulation and optimization results for a wide choice of $\delta$ (on all roads). Since smaller values of $\delta$ lead to larger values for the acceleration towards the desired velocity, one would expect that the results for small values of $\delta$ get close to the results of the LWR model (as in Section 4.1). Surprisingly, it is the other way around for the considered scenario.

First, one observes larger total travel times in the case of smaller values for $\delta$. These result from the faster reaction of the velocity on the increase in density behind the on-ramp at the beginning of the scenario, which in turn accelerates the increase in density compared to the other parameter choices (see Figure 15 where the results for the case $\frac{\partial p_i}{\partial v_i^{\max}}\ne0$ are plotted). For very large values of $\delta$ there is almost no reaction of the velocity on the density increase and no congestion. Qualitatively, the corresponding results are quite similar to the results of the LWR model (see again Figure 15).

5.   Conclusion

In this work, we have set up coupling conditions for the Aw-Rascle model linked to on-ramps described by ordinary differential equations. Applying a first order Godunov scheme, the presented approach allows for numerical investigations of the capacity drop effect and optimal control problems.

Future work will include extensions to on-ramps that are described by first order traffic models such as the LWR model. We will derive appropriate coupling conditions and focus on theoretical properties.