Matrix representations of Atkinson-type Sturm-Liouville problems with coupled eigenparameter-dependent conditions

1.   Introduction

Differential operators are a class of widely used unbounded linear operators. The theory of differential operators utilizes functional analysis to study differential equations, greatly expanding the theory of differential equations by viewing differential equations as mappings between function spaces. The classical representation of a differential operator is the Sturm-Liouville (S-L) operator, originating from the solution of heat conduction problems. By separating variables in the partial differential equations that describe the process of heat conduction, one can obtain the S-L equation. The main focus of S-L operator theory is its spectral theory, which finds extensive applications in areas such as mathematical physics and control theory, holding significant mathematical and physical significance ^[1,2]. For instance, the Schrödinger equation in quantum mechanics can be seen as a special S-L problem, and solving the Schrödinger equation yields the energy levels and wave functions of atoms ^[3,4]. Seismic wave propagation problems can be modeled as S-L problems, aiding in a better understanding of the internal structure of the earth ^[5,6]. Additionally, string vibration problems are closely related to S-L problems ^[7,8].

Over the past two centuries, S-L problems have seen significant development and have become a self-contained system ^[9,10,11]. In recent years, S-L problems with discontinuities within intervals have garnered attention due to their wide applications in mathematical physics and engineering, including heat conduction between different media, seismic wave propagation between different geological structures, and string vibration problems with additional point masses ^[12,13]. Furthermore, S-L problems with boundary conditions containing spectral parameters have also been extensively studied because the eigenvalues of S-L problems hold different physical meanings in various practical scenarios ^{[14,15,16,17]}. When real-world boundary conditions are influenced by spectral parameters, it becomes necessary to model practical problems as S-L problems with boundary conditions dependent on spectral parameters, such as heat conduction between solids and liquids and diffusion of water vapor through porous membranes ^[18,19,20]. Building upon this foundation, S-L problems with boundary conditions and transfer conditions simultaneously dependent on spectral parameters have piqued the interest of researchers. Therefore, aspects such as the asymptotic behavior of eigenvalues, inverse spectral theory, finite spectra, and oscillatory properties of eigenfunctions have been studied ^{[21,22,23,24]}. However, the transfer conditions considered in the aforementioned research have not been dependent on spectral parameters. Currently, there is limited research on S-L problems where transfer conditions are dependent on spectral parameters^{[25,26,27,28]}. In ^[25], the authors established the completeness formula of the operator theory of S-L problems with transfer conditions and the completeness of eigenfunctions. In ^[26], the authors investigated the asymptotic formula of eigenvalues for S-L problems with transfer conditions that are rationally dependent on spectral parameters. Additionally, ^[28] focused on the finite spectrum problem of S-L problems with transfer conditions dependent on spectral parameters.

It is well known that regular S-L problems have countably infinite eigenvalues and no finite accumulation points. However, Atkinson conjectured in his work that under certain conditions on the coefficients, the studied S-L problem may have a finite number of eigenvalues, but he did not give the proof ^[29]. Kong et al. confirmed this conjecture by constructing a class of S-L problems with a specified number of eigenvalues for any given positive integer ^[24]. These special problems are referred to as Atkinson-type S-L problems. Building upon this, Kong and others showed in a study that the finite spectrum problem of Atkinson-type S-L problems can be related to the eigenvalue problem of finite-dimensional matrices. They demonstrated that under specific circumstances, these two types of problems can be mutually transformed, providing diverse research approaches and deepening the understanding of finite spectrum problems in S-L theory. Matrix eigenvalue problems are known for their significant practical applications in various fields such as physics, engineering, computer science, and economics ^{[30,31,32,33]}. The practical relevance of matrix eigenvalue problems is one of the motivations for this study.

In recent years, Atkinson-type S-L problems have been extended to various types of differential operators, including Atkinson-type S-L problems with transfer conditions, Atkinson-type S-L problems with boundary conditions containing spectral parameters, and higher-order differential operators^[34,35,36]. In these studies, the authors proved that the discussed problems have a finite number of eigenvalues and provided corresponding equivalent matrix representations. In a recent paper, Ao and others considered S-L problems with transfer conditions separated depending on the spectral parameters and proved that their eigenvalues are finite, more precisely, they proved that the number of eigenvalues is at most $m+n+4$ ^[28].

To enhance the generality of the problem, in this paper, we consider the finite spectrum of S-L problems when the eigenparameter coupled appears in the transfer conditions. How does the number of eigenvalues of this problem change under these circumstances? This paper investigates the finite spectrum problem of Atkinson-type S-L problems, where both boundary conditions and transfer conditions are dependent on spectral parameters. Under certain conditions, it is proven that this problem has a finite number of eigenvalues, exploring the factors influencing the number of eigenvalues and providing criteria for determining the number of eigenvalues. We proved that the problem has at most $m+n+6$, which is different from ^[28]. The results indicate that the number of eigenvalues of this problem increases when the transfer conditions coupled depend on the spectral parameters. Building upon this, we consider the equivalence between Atkinson-type S-L problems and matrix eigenvalue problems and provide equivalent representations of these two types of problems. Finally, we give some examples to demonstrate the effectiveness of the results.

The rest of this paper is organized as follows: In Section 2, some notations and preliminaries are given. In Section 3, it is proved that the number of eigenvalues of the considered problem is finite, and the matrix representation is given in Section 4. In Section 5, for any given matrix eigenvalue problem of certain type and boundary and transfer conditions that both depend on eigenparameter, we construct a class of S-L problems with the same boundary and transfer conditions, which have the same eigenvalues.

2.   Notations and preliminaries

In this paper, we investigate the following S-L problem:

with boundary and transfer conditions

where

and $x = \xi$ is an inner discontinuity point, $Y(\xi^{+})$ denotes the right limit of $Y(x)$ at $\xi$, $Y(\xi^{-})$ denotes the left limit of $Y(x)$ at $\xi$, $\lambda \in \mathbb{C}$ is a spectral parameter, $\det(A_{\lambda})\neq0$, $\det(B_{\lambda})\neq0$ and $\det(C_{\lambda})\neq0$, $\det(D_{\lambda})\neq0$, let $\alpha_{i}, \beta_{i}, \delta_{i}, \gamma_{i}, \alpha_{i}^{\prime}, \beta_{i}^{\prime}, \delta_{i}^{\prime}, \gamma_{i}^{\prime} \in\mathbb{R}$, $i = 1, 2, 3, 4$, and

and

For convenience, let

then we suppose the coefficients satisfy the following conditions:

where $L^{1}(J, \, \mathbb{R})$ denotes the set of real-valued functions that are Lebesgue integrable on $J$.

Let $u = y$ and $v = py^{\prime}$, then

according to (2.1), there

so

We have the following equivalent system representation of Eq (2.1) on $J$:

Definition 2.1. ^[24] A trivial solution $y$ of (2.1) on some interval is a solution that is identically zero and whose quasi-derivative $v = py^{\prime}$ is also identically zero on this interval.

Let $\Phi(x, \lambda)$ and $\Psi(x, \lambda)$ be the fundamental solution matrices of system (2.9), where

determined by the initial condition

respectively.

The following result shows that the eigenvalues of problems (2.1)–(2.3) are the zeros of $\Delta(\lambda)$:

Lemma 2.1. $\Phi(x, \lambda)$ and $\Psi(x, \lambda)$ are defined as above. A complex number $\lambda$ is an eigenvalue of Eqs (2.1)–(2.3) if and only if $\Delta(\lambda) = 0$, where

there $A_{\lambda}$, $B_{\lambda}$ are defined as (2.4), and $C_{\lambda}$, $D_{\lambda}$ are defined as (2.5).

Proof. According to the existence uniqueness theorems of solutions, we can know

is the solution of (2.9) in $[a, \xi)$ satisfying the initial value $Y(a)$, so the value of $x = \xi^{-}$ is

similarly,

is the solution of (2.9) in $(\xi, b]$ satisfying the initial value $Y(b)$, so the value of $x = \xi^{-}$ is

Combining (2.11) and (2.12) with the transfer condition (2.3), then we have

Therefore, if a complex number $\lambda$ is an eigenvalue of (2.1)–(2.3), it satisfies

The system of homogeneous equations has a non-zero solution if and only if the determinant of the coefficients is zero, so

is an eigenvalue of (2.1)–(2.3). □

Definition 2.2. ^[24] The problems (2.1)–(2.3), or equivalently (2.2), (2.3), and (2.9), are said to be degenerate if either $\Delta(\lambda)\equiv 0$ for all $\lambda \in \mathbb{C}$ or $\Delta(\lambda)\neq 0$ for any $\lambda \in \mathbb{C}$ in (2.10).

3.   The finite spectrum problems of (2.1)–(2.3)

It is shown that the problems (2.1)–(2.3) have finite eigenvalues in this section. In the sequel, we always assume (2.8) holds and there exists a partition of $J$

for some positive integers $m\ge 1, n\ge 1$, such that

Definition 3.1. A S-L Eq (2.1) is said to be of Atkinson-type if it satisfies (3.1)–(3.3).

Definition 3.2. If there exists an Eq (2.1) of Atkinson-type, then the S-L problems (2.1)–(2.3) are said to be of Atkinson-type.

Definition 3.3. For convenience, we define the following notations:

Now, we determine the structure of the principal fundamental solution matrix of system (2.9).

Lemma 3.1. For $\Phi(x, \lambda)$ defined as above, we have

where

In general, for $1\le i\le m-1$, we have

particularly,

Proof. Observing Eq (3.2), we know that $u$ is constant on

by $r(x) = 0$ and $v$ is constant on $\cup_{i = 0}^{m-1}[a_{2i+1}, a_{2i+2}]$ by

So we can obtain the result by using the iterative method ^[24]. □

Lemma 3.2. Let $\tilde{\Psi}(x, \lambda)$ be the fundamental solution matrix of system (2.9), where

determined by the initial condition

Then we have

In general, for $1\le j\le n$,

Proof. The proof is similar to Lemma 3.1, so we omit the details. □

According to Lemmas 3.1 and 3.2, the following corollaries hold:

Corollary 3.1. For the fundamental matrix $\Phi(x, \lambda)$, we have

where

where

$\tilde{\varphi}_{11}(\lambda)$ is a polynomial in the form of

hence

deg denotes the degree of $\lambda$.

Corollary 3.2. For the fundamental matrix $\tilde{\Psi}(x, \lambda)$, we have

where

where

Lemma 3.3. For $\Psi(\xi^{+}, \lambda)$, $\tilde{\Psi}(b, \lambda)$ as above, we have

Proof. $\Psi(x, \lambda)$ and $\tilde{\Psi}(x, \lambda)$ are both the fundamental solution matrices of the system (2.9), where

So we have

let $x = \xi^{+}$. By initial condition $\tilde{\Psi}(\xi^{+}, \lambda)$, we have

Since the Wronskian is independent of $x$, we can obtain

therefore,

□

For convenience, we let

Theorem 3.1. Assume (2.8) holds, and by the above notations, we have

where

here,

$\tilde{\theta}_{1}(\lambda)$ is a polynomial in the form of

hence

Proof. According to Lemma 2.1, we know

then, by Corollaries 3.1 and 3.2, we can obtain the structure of $\Phi(\xi^{-})$ and $\Psi(\xi^{+})$. Plug them into the above equation, and by direct calculation we can obtain the conclusion. □

In the following, it turns out that the S-L problem with boundary and transfer conditions that both depend on spectrum has finite eigenvalues.

Theorem 3.2. Assume $\delta_{4}^{\prime}\gamma_{2}^{\prime}-\delta_{2}^{\prime}\gamma_{4}^{\prime}\neq0$. By (3.2)–(3.4). We have

(1) If $\alpha_{4}^{\prime}\beta_{2}^{\prime}-\alpha_{2}^{\prime}\beta_{4}^{\prime}\neq0$, then the problems (2.1)–(2.3) have exactly $m+n+6$ eigenvalues.

(2) If $\alpha_{4}^{\prime} \beta_{2}^{\prime}-\alpha_{2}^{\prime} \beta_{4}^{\prime} = 0$, but

then the problems (2.1)–(2.3) have exactly $m+n+5$ eigenvalues.

(3) If

but

then the problems (2.1)–(2.3) have exactly $m+n+4$ eigenvalues.

(4) If

but

then the problems (2.1)–(2.3) have exactly $m+n+3$ eigenvalues.

(5) If

but $\alpha_{1}\beta_{3}-\alpha_{3}\beta_{1} \neq 0$, then the problems (2.1)–(2.3) have exactly $m+n+2$ eigenvalues.

(6) If none of the above conditions hold in (3.5), then the problems (2.1)–(2.3) have exactly $l$ eigenvalues for $l\in\{1, 2, \cdots, m+n+1 \}$ or degenerate.

Proof. By Lemma 2.1, we know that the eigenvalues of the problem coincide with the zeros of $\Delta(\lambda).$ Hence, we only need to determine the degree of $\Delta(\lambda)$ as a polynomial of $\lambda$. Then we can obtian the number of eigenvalues of the problem using the algebraic fundamental theorem. To this end, we divide the problem into the above six cases according to the degree of $\Delta(\lambda)$ with respect to $\lambda$ under different conditions. Since

we have the degree of $\theta(\lambda)$ in $\lambda$ is $4$ by the expression of $h_1(\lambda)$. We only give the proof for (1) and (2) since the other cases can be treated similarly.

(1) If

the degree of $g_1(\lambda)$ as a polynomial of $\lambda$ is $2$. By Theorem 3.1, the degree of $\Theta_{1}(\lambda)$–$\Theta_{4}(\lambda)$ as a polynomial of $\lambda$ is $m+n+4, \; m+n+3, \; m+n+3, \; m+n+2$, respectively. Then we have that the degree of $\Delta(\lambda)$ as a polynomial of $\lambda$ is $m+n+6$ by (3.5). By the algebraic fundamental theorem, $\Delta(\lambda) = 0$ has exactly $m+n+6$ zeros; that is to say, the problem has exactly $m+n+6$ eigenvalues.

(2) If

and

Then we have that the degree of $g_1(\lambda)$ is $1$. By the assumption of the theorem, the degree of $h_1(\lambda)$ is still $2$. This implies that the degree of $\theta(\lambda)$ in $\lambda$ is $4$. And the degree of $\Theta_{1}(\lambda)$–$\Theta_{4}(\lambda)$ as a polynomial of $\lambda$ is $m+n+4, \; m+n+3, \; m+n+3, \; m+n+2$, respectively. Then we have that the degree of $\Delta(\lambda)$ as a polynomial of $\lambda$ is $m+n+5$ by (3.5). By algebraic fundamental theorem, $\Delta(\lambda) = 0$ has exactly $m+n+5$ zeros; that is to say, the problem has exactly $m+n+5$ eigenvalues. Conditions (3)–(6) can be treated similarly.

This completes the proof. □

Remark 3.1. In the above theorem, if

but

we can obtain the same conclusions. As a matter of fact, in this case, the highest degree of $\lambda$ in $\Delta(\lambda)$ is $m+n+5$. And then we can obtain the number of the eigenvalues is $m+n+5$, $m+n+4$, $m+n+3$, $m+n+2$, $m+n+1$, respectively.

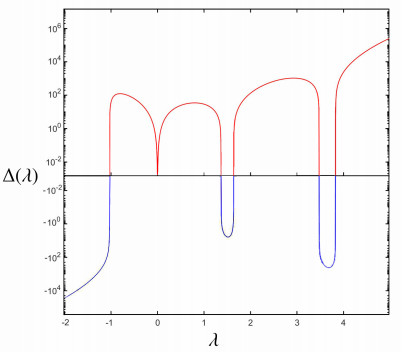
Example 3.1. We consider the following S-L problem:

where

We choose $m = 2, n = 1$ and $p(x), q(x), \omega(x)$ are piece-wise constant functions as follows:

By directly calculation, we have

then we can obtain the roots of $\Delta(\lambda)$ as follows:

The graph of the characteristic function is displayed in Figure 1.

4.   Matrix presentations of (2.1)–(2.3)

In this section, we discuss the matrix representation of the S-L problems (2.1)–(2.3), which has finite spectrum.

Definition 4.1. A S-L problem of Atkinson-type is said to be equivalent to a matrix eigenvalue problem if they have the exactly same eigenvalues.

For (2.1)–(2.3), we can construct matrix eigenvalue problems of the following form:

which have exactly the same eigenvalues as the corresponding S-L problem of Atkinson-type. Assume Eq (3.4) holds; moreover, we let

Remark 4.1. In accordance with (3.2)–(3.4), we know $p_{i}, w_{i}, \tilde{p}_{j}, \tilde{w}_{j}\in \mathbb{R} \setminus \left \{ 0 \right \}$ and no sign restrictions are imposed on them.

Besides, by (3.2)–(3.4), we see that for any solution $(u, v)$ of system (2.9), $u$ is constant on the sub-intervals where $r\equiv 0$; $v$ is constant on the sub-intervals where $q \equiv \omega \equiv 0$.

Let

and

Lemma 4.1. Suppose Eq (2.1) is of Atkinson-type. Then for any solution $(u, v)$ of system (2.9), we know that

Conversely, for any solution $u_{i}(i = 0, 1, \cdots, m), v_{i}(i = 0, 1, \cdots, m+1), \tilde{u}_{j}(j = 0, 1, \cdots, n),$ and $\tilde{v}_{j}(j = 0, 1, \cdots, n+1)$ of systems (4.4)–(4.7), there exists a unique solution $(u, v)$ of system (2.9) satisfying (4.2) and (4.3).

Theorem 4.1. Assume $\alpha_{i}, \beta_{i}, \delta_{i}, \gamma_{i}, \alpha_{i}^{\prime}, \beta_{i}^{\prime}, \delta_{i}^{\prime}, \gamma_{i}^{\prime}\in \mathbb{R}(i = 1, 2, 3, 4)$ and (2.6) and (2.7) hold. We define an $\, \, (m+n+6)\times (m+n+6)$ almost cyclic tridiagonal matrix in the case where $\delta_{4}^{\prime}\gamma_{2}^{\prime}-\delta_{2}^{\prime}\gamma_{4}^{\prime} \neq 0$

a diagonal matrix

and an almost diagonal matrix

Then S-L problems (2.1)–(2.3) are equivalent to matrix eigenvalue problems

where

Moreover, the eigenfunction $u(x)$ of S-L problems (2.1)–(2.3) and the corresponding eigenvector $U$ of the matrix eigenvalue problem (4.8) associated with the same eigenvalue $\lambda$ are related by ($4.2$).

Proof. There exists a one-to-one correspondence between the solutions of systems (4.4)–(4.7) and the following system:

Now, we assume that $u_{i}(i = 0, 1, \, \dots \, ,m)$ and $v_{i}(i = 0, 1, \, \dots \, ,m, m+1)$ are solutions of systems (4.4) and (4.5). Then (4.9)–(4.11) follow from (4.4) and (4.5). Similarly, (4.12)–(4.14) follow from (4.6) and (4.7) by assuming that $\tilde{u}_{j}(j = 0, 1, \, \dots \, ,n)$ and $\tilde{v}_{j}(j = 0, 1, \, \dots \, ,n)$ are solutions of systems (4.6) and (4.7).

On the other hand, assume $u_{i}(i = 0, 1, \, \dots \, ,m)$ is a solution of systems (4.9)–(4.11), $v_{0}$ and $v_{m+1}$ are determined by (4.9) and (4.11), respectively. Let $v_{i}(i = 1, \, \dots \, ,m)$ be defined by (4.4). Then, using (4.9) and by induction on (4.10), we obtain (4.5), similarly for (4.6) and (4.7).

Therefore, by Lemma 4.1, each solution of system (2.9), and hence of (2.1), is uniquely determined by a solution of systems (4.9)–(4.14). Note the first row of the matrix (4.8)

and the last row of the matrix (4.8)

substituting

into (4.15) and (4.16), we obtain the boundary condition (2.2). Similarly, from the $m+3$ row of matrix (4.8),

and the $m+4$ row of the matrix (4.8)

substituting

into (4.17) and (4.18), we obtain the transfer condition (2.3), and we choose the eigenvector $U$ as

Then the equivalence follows from (4.9)–(4.18). □

The next theorem illustrates the fact that an S-L problem of Atkinson-type is equivalent to an S-L problem with piecewise constant coefficients.

Theorem 4.2. Assume that Eq (2.1) is of Atkinson-type, and let $p_{i}(i = 1, 2, \dots, m), \tilde{p}_{j}(j = 1, 2, \dots, n), \\ q_{i}, \omega_{i}(i = 0, 1, \dots, m), \tilde{q}_{j}, \tilde{\omega}_{j}(j = 0, 1, 2, \dots, n)$ be given by (3.4) and (4.1). Define piecewise constant function $\bar{p}, \bar{q}, \bar{\omega}$ on $J$ by

Suppose that (2.2) and (2.3) hold. Then the eigenvalues of S-L problems (2.1)–(2.3) coincide with the eigenvalues of S-L problems

with (2.2) and (2.3).

Proof. It is observed that both the S-L problems (2.1)–(2.3) and (4.12), (2.2) and (2.3) determine the same

Thus, they are equivalent to the same matrix eigenvalue problem by Theorem 4.1. The results follow. □

Remark 4.2. ^[36] According to Theorem 4.2, we know that for fixed (2.2) and (2.3) on a given interval, there exists a family of S-L problems of the Atkinson-type, that have the same eigenvalues as S-L problems (2.2), (2.3), and (4.22). Such a family is called the equivalent family of S-L problems (4.22), (2.2), and (2.3).

5.   Sturm-Liouville representations of matrix eigenvalue problems

In the sequel, we will illustrate that matrix eigenvalue problems of the form

have representations as Atkinson-type S-L problems.

Theorem 5.1. Let $l\ge8$, $\delta_{i}, \gamma_{i}, \delta ^{\prime} _{i}, \gamma ^{\prime} _{i}(i = 1, 2, 3, 4)$ be real numbers associated with transfer condition (2.3) which satisfies $\det(C_{\lambda})\neq 0$ and $\det(D_{\lambda})\neq 0$. Let $A$ be an $l \times l$ almost cyclic tridiagonal matrix

where $a_{j, j+1}\neq 0\ (j = 2, 3, \dots, k+2, k+5, \dots, l-2), \ 2\le k \le l-6, \ a_{ij}\in \mathbb{R} (1\le i, j\le l), \ a_{21} = a_{k+5, k+4} = 1, \ a_{k+2, k+3} = a_{l-1, l} = -1$. Let $F$ be an $l\times l$ almost diagonal matrix

where $f_{jj}\neq 0$, $f_{jj}\in \mathbb{R}\, \, (j = 2, 3, \dots, k+2, k+5, \dots, l-1)$, and the elements of first and last row of $A$ and $F$ satisfy

the elements of $k+3$ and $k+4$ rows of $A$ and $F$ satisfy

Then (5.1) has representation as Atkinson-type S-L problems in the forms of (2.1)–(2.3). Furthermore, the S-L problems (2.2) and (2.3), and (4.22) have a unique representation when a fixed (3.1) of $J$ is given, with the notation in (3.4) and (4.1). All S-L representations of (3.1) are given by the corresponding equivalent families of S-L problems (2.2), (2.3), and (4.22).

Proof. Let $m = k$, $n = l-k-6$, $J = [a, \xi)\cup(\xi, b]$, $-\infty < a < b < \infty$. First, one defines the parameters in (2.2) and (2.3), let

and

For a given partition of $J$ by (3.1), one can define piecewise constant functions $\bar{p}, \bar{q}$ and $\bar{\omega}$ on interval $J$ satisfying (2.8), (3.2), and (3.3) as follows:

and

Next, define $\bar{p}, \bar{q}$ and $\bar{\omega}$ by (4.19)–(4.21), respectively. Such piecewise constant functions $\bar{p}, \bar{q}$ and $\bar{\omega}$ on interval $J$ satisfying (2.8), (3.2), and (3.3) are found and Eq (4.22) is of the Atkinson-type, (3.4) and (4.1) satisfy with $p, q,$ and $\omega$ replaced by $\bar{p}, \bar{q}$ and $\bar{\omega}$, respectively. Obviously, Eq (5.1) is of the same form as Eq (4.8). Therefore, the problem (5.1) is equivalent to the S-L problems (2.1)–(2.3) by Theorem 4.1. The last part is yield by Theorem 4.2. □

6.   Conclusions

In the present paper, we study the finite spectrum problems for a class of S-L problems with coupled eigenparameter-dependent boundary and transfer conditions. We proved that the problem under consideration has finite spectrum under certain conditions. Moreover, the corresponding matrix representation is also given. In our future research, we will study the corresponding inverse spectrum problem of Atkinson's type S-L problems.

Author contributions

Jinming Cai: writing–review & editing (equal); Shuang Li: writing–original draft (equal); Kun Li: supervision (equal), writing–review (equal). All authors read and approved the final manuscript.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

The authors thank the referees for their comments and detailed suggestions. These have significantly improved the presentation of this paper. This research is supported by the National Nature Science Foundation of China (No. 12401160), Natural Science Foundation of Shandong Province (Nos. ZR2023MA023, ZR2021MA047 and ZR2021QF041), Postdoctoral Foundation of China (No. 2020M682139) and the project of Youth Innovation Team of Universities of Shandong Province (No. 2022KJ314).

Conflict of interest

All authors declare no conflicts of interest in this paper.