Partial domination of network modelling

1.   Introduction

In this paper, all graphs considered are non-empty, finite, undirected and simple. Additionally, for standard graph theory terminology not given here, we refer to ^[1]. Let $G$ be a simple graph with the vertex set $V(G)$ and the edge set $E(G)$, and $|V(G)| = n$, $|E(G)| = m$. For any $v\in V(G)$, the $degree$ $d_G(v)$ of $v$ is the number of neighbors of $v$. The $minimum$ and $maximum$ $degree$ of $G$ are denoted by $\delta(G)$ and $\Delta(G)$, respectively. The $open$ $neighborhood$ $N_G(v)$ of $v$ is the set $N(v) = \{u\in V|\, uv\in E\}$ and the $closed$ $neighborhood$ of $v$ is the set $N_G[v] = N_G(v)\cup\{v\}$. For any $S\subseteq V(G)$, the $open$ $neighborhood$ of $S$ is the set $N_G(S) = \bigcup_{v\in S}N_G(v)$ and the $closed$ $neighborhood$ of $S$ is the set $N_G[S] = N_G(S)\cup S$. Furthermore, we define $N_S(v) = N_G(v)\cap S$ and $N_S[v] = N_S(v)\cup \{v\}$. The subgraph of $G$ induced by $S$ is denoted by $G[S]$. For a graph $H$, we say that $G$ is $H$-$free$ if $G$ does not contain $H$ as a subgraph. The $cycle$ and $clique$ on $n$ vertices are denoted as $C_n$ and $K_n$. Abbreviate $\{1, \, 2, \, \cdots, \, n\}$ to $[n]$ and say "$i$" is a symbol of $[n]$, where $n\in\mathbb{N^*}$ and $i\in[n]$.

In recent years, with the rapid development of information technology, the domination theory has been widely used in computer technology, cryptography, social network, communication network and many other subjects. In 1958, Claude Berge ^[2] first proposed the concept of the domination number. A vertex subset $S\subseteq V(G)$ is a $dominating$ $set$ of $G$ if $N_G[S] = V(G)$. The $domination$ $number$ $\gamma(G)$ of $G$ is the minimum cardinality of a dominating set of $G$, i.e $\gamma(G) = min\{|S|:\; S\; is\; a\; dominating\; set\; of\; G\}$.

In 2017, Caro and Hansberg ^[3] extended the domination to the partial domination, and proposed the concept of an $\mathcal{F}$-isolating set of a graph $G$ for the first time. Let $G = (V, E)$ be a graph and $\mathcal{F}$ be a family of graphs.

Definition 1.1. ^[3] A subset $S\subseteq V$ is called an $\mathcal{F}$-isolating set of $G$ if $G[V\backslash N_G[S]]$ does not contain $F$ as a subgraph for all $F\in\mathcal{F}$. The $\mathcal{F}$-$isolation$ $number$ of $G$ is the minimum cardinality of an $\mathcal{F}$-isolating set of $G$, denoted by $\iota(G, \mathcal{F})$.

In particalur, if $\mathcal{F} = \{K_k\}$, the set $S$ is called a $\{K_k\}$-$isolating$ $set$ of $G$ if $G[V\backslash N_G[S]]$ does not contain $K_k$ as a subgraph, and the $\{K_k\}$-$isolation$ $number$ of $G$ is the minimum cardinality of a $\{K_k\}$-isolating set of $G$, denoted by $\iota(G, \, k)$. For any positive integer $k$, if $\mathcal{F} = \{K_{1, k+1}\}$, the set $S$ is called a $k$-$isolating$ $set$ of $G$ if $G[V\backslash N_G[S]]$ does not contain $K_{1, k+1}$ as a subgraph, and the $k$-$isolation$ $number$ of $G$ is the minimum cardinality of a $k$-isolating set of $G$, denoted by $\iota_k(G)$. Especially, when $k = 0$, the set $S$ is called an $isolating$ $set$ of $G$, and the minimum cardinality of an isolating set of $G$ is the $isolation$ $number$ of $G$, denoted by $\iota(G)$.

With respect to this problem, Borg et al. ^[4] studied the $\iota(G, \, k)$ of a connected graph $G$ is at most $\frac{n}{k+1}$ unless $G\cong K_k$, or $k = 2$ and $G\cong C_5$. Caro and Hansberg ^[3] investigated that $\iota(G)\le\frac{n}{3}$ for $n\ge6$, $\iota _k(T)\le \frac{n}{k+3}$ of a tree $T$ which is different from $K_{1, k+1}$, $\iota _k(G)\le \frac{n}{4}$ of a maximal outerplanar graph $G$ with $n\ge4$ and so on. Tokunaga et al. ^[5] studied that if $G$ is a maximal outerplanar graph of order $n$ with $n_2$ vertices of degree $2$, then $\iota (G)\le \frac{n+n_2}{5}$ when $n_2\le \frac{n}{4}$, and $\iota (G)\le \frac{n-n_2}{3}$ otherwise. Borg and Kaemawichanurat ^[6] showed that if $G$ is a maximal outerplanar graph with $n\ge5$, then $\iota_1(G)\le \frac{n}{5}$, they also showed that $\iota_1(G)\le \frac{n+n_2}{6}$ when $n_2\le \frac{n}{3}$, and $\iota_1(G)\le \frac{n-n_2}{3}$ otherwise, where $n_2$ is the number of vertices of degree $2$. Borg and Kaemawichanurat ^[7] obtained that $\iota_{k}(G)\le min\{\frac{n}{k+4}, \, \frac{n+n_2}{5}, \, \frac{n-n_2}{3}\}$ for a maximal outerplanar graph $G$ and $k\ge0$, where $n_2$ is the number of vertices of degree $2$. Vazquez-Araujo ^[8] analyzed that $\iota(T) = \frac{n}{3}$ implies $\iota(T) = \gamma(T)$ for a tree $T$, and proposed simple algorithms to build these trees from the connections of stars.

For a $\{K_k\}$-isolating set $S$, in 2021, Favaron and Kaemawichanurat ^[9] restriced the induced subgraph $G[S]$ to be an independent set and introduced the concept of the independent $\{K_k\}$-isolation number of $G$. The vertex subset $S\subseteq V$ is said to be independent $\{K_k\}$-isolating if $S$ is a $\{K_k\}$-isolating set of $G$ and $G[S]$ has no edge. The independent $\{K_k\}$-isolation number of $G$ is the minimum cardinality of an independent $\{K_k\}$-isolating set of $G$. Based on the concept, we propose the following concepts.

Definition 1.2. A subset $S\subseteq V$ is called an $independent$ $\mathcal{F}$-$isolating$ $set$ of $G$ if $S$ is an $\mathcal{F}$-isolating set of $G$ and $S$ is an independent set. The $independent$ $\mathcal{F}$-$isolation$ $number$ of $G$ is the minimum cardinality of an independent $\mathcal{F}$-isolating set of $G$, denoted by $\iota_{I}(G, \mathcal{F})$.

Definition 1.3. A subset $S\subseteq V$ is called an $independent$ $isolating$ $set$ of $G$ if $S$ is an isolating set of $G$ and $G[S]$ has no edge. The $independent$ $isolation$ $number$ of $G$ is the minimum cardinality of an independent isolating set of $G$, denoted by $\iota_{I}(G)$.

In this paper, we investigate respectively the sharp bounds of the isolation number and the independent isolation number of the hypercube network and $n$-star network.

2.   Main results

2.1.   Isolation number of the hypercube network

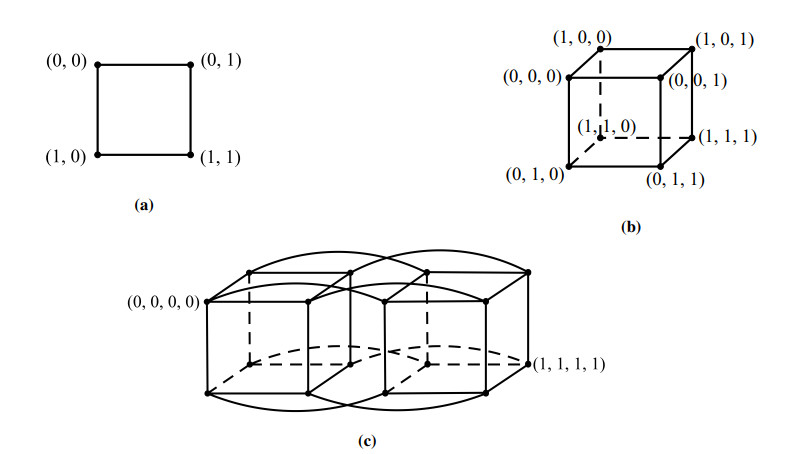
The hypercube network is the basic model for interconnection networks, and it is a popular network because of its attractive properties, including regularity, symmetry, small diameter, strong connectivity, recursive construction, partitionability and relatively low link complexity ^[10,11]. In general, a network model can be modeled as a graph. Let $n$ be a positive integer. The $hypercube$ $network$ of dimension $n$, denoted by $Q_n$, is the simple graph whose vertices are the $n$-tuples with entries in $\{0, 1\}$ and whose edges are the pairs of $n$-tuples that differ in exactly one position (see Figure 1). A $m$-$dimensional$ $subcube$ of $Q_n$ is isomorphic to $Q_m$ for any positive integer $1\le m\le n$. A vertex of $V(Q_n)$ is an $even$ $vertex$ if the number of $1$s is even in its all symbols. A vertex of $V(Q_n)$ is an $odd$ $vertex$ if the number of $1$s is odd in its all symbols.

Figure 1.  (a)$Q_2$; (b)$Q_3$; (c)$Q_4$.

DownLoad: Full-Size Img PowerPoint

The hypercube network have many classic and fascinating topological structural properties, such as those below.

Lemma 2.1. ^[1] Hypercube network satisfies the following properties:

(a) $|V(Q_n)| = 2^n$, $|E(Q_n)| = n\cdot 2^{n-1}$;

(b) Each edge of $Q_n$ has an even endvertex and an odd endvertex;

(c) $Q_n$ is a $n$-regular bipartite graph;

(d) Every perfect matching of $Q_n$ has $2^{n-1}$ edges;

(e) If $n\ge3$, then $Q_n$ has $2^{n-3}$ disjoint $3$-dimensional subcubes of $Q_n$.

By Lemma $2.1$, we obtain the following results.

Theorem 2.2. $\iota(Q_2) = 1$, $\iota(Q_3) = \iota(Q_4) = 2$.

Proof. According to the structure of $Q_n$, we know that $Q_2\cong C_4$, so $\iota(Q_2) = \iota(C_4) = 1$.

It is easy to know $\{(0, 0, 0), \, (1, 1, 1)\}$ is an isolating set of $Q_3$ (see Figure 1(b)), so $\iota(Q_3)\le2$. Let $S_1$ be a minimum isolating set of $Q_3$, clearly, $|S_1|\ge1$. Suppose that $|S_1| = 1$. If the vertex of $S_1$ is an even vertex, without loss of generality, let $S_1 = \{(1, \, 1, \, 0)\}$, then $V(Q_3)\backslash N_{Q_3}[S_1] = \{(0, \, 0, \, 0), \, (0, \, 0, \, 1), \, (1, \, 0, \, 1), \, (0, \, 1, \, 1)\}$. Hence, $V(Q_3)\backslash N_{Q_3}[S_1]$ is not an independent set of $Q_3$, which is a contradiction. If the vertex of $S_1$ is an odd vertex, without loss of generality, let $S_1 = \{(0, \, 1, \, 0)\}$, then $V(Q_3)\backslash N_{Q_3}[S_1] = \{(1, \, 0, \, 0), \, (0, \, 0, \, 1), \, (1, \, 0, \, 1), \, (1, \, 1, \, 1)\}$. Hence, $V(Q_3)\backslash N_{Q_3}[S_1]$ is not an independent set of $Q_3$, which is a contradiction. Thus, $|S_1|\neq 1$, furthermore, $\iota(Q_3) = |S_1|\ge2$. Hence, $\iota(Q_3) = 2$.

Additionally, it is easy to know $\{(0, 0, 0, 0), \, (1, 1, 1, 1)\}$ is an isolating set of $Q_4$ (see Figure 1(c)), so $\iota(Q_4)\le2$. Let $Q_3^1$ and $Q_3^2$ be two disjoint $3$-dimensional subcubes of $Q_4$ by Lemma $2.1(e)$, and $S_2$ be a minimum isolating set of $Q_4$. Obviously, $|S_2|\ge1$. If $|S_2| = 1$ and $S_2 = \{v\}$, then $v\in Q_3^i$ for any $i\in\{1, \, 2\}$. Since $Q_3^j \subset G[V(Q_4)\backslash N_{Q_4}[v]]$ for $j\in\{1, \, 2\}\backslash \{i\}$, $V(Q_4)\backslash N_{Q_4}[S_2]$ is not an independent set of $Q_4$, which is a contradiction. Thus, $|S_2|\neq 1$, furthermore, $\iota(Q_4) = |S_2|\ge2$. Hence, $\iota(Q_4) = 2$. □

Theorem 2.3. Let $n$ be a positive integer and $n\ge4$, then $\frac{2^{n-1}}{n}\le \iota(Q_n)\le 2^{n-3}$. Moreover, the bounds are sharp.

Proof. Let $S$ be a minimum $\{K_2\}$-isolating set of $Q_n$. Since every perfect matching of $Q_n$ has $2^{n-1}$ edges and $V(Q_n)\backslash N_{Q_n}[S]$ is an independent set, every edge of a perfect matching of $Q_n$ has at least one vertex in $N_{Q_n}(S)$, that is, $|N_{Q_n}(S)|\ge \frac{2^n}{2} = 2^{n-1}$. By Lemma $2.1(c)$, we have $|N_{Q_n}(S)|\le \Delta(Q_n) |S| = d(v)|S| = n|S|$ for any vertex $v\in S$. Thus, $\iota(Q_n) = |S|\ge \frac{|N_{Q_n}(S)|}{d(v)}\ge \frac{2^{n-1}}{d(v)} = \frac{2^{n-1}}{n}$.

By Lemma $2.1$, we know that $Q_n$ has $2^{n-3}$ disjoint $3$-dimensional subcubes of $Q_n$ for $n\ge4$ and each edge of $Q_n$ has one even endvertex and one odd endvertex, so each $Q_3$ has four odd vertices and four even vertices, and every even vertex is adjacent to three odd vertices in $Q_3$. Without loss of generality, let $x\in V(Q_3^1)$ be an even vertex and $y\in V(Q_3^1)\backslash N_{Q_3^1}[x]$ be an odd vertex of $Q_3^1$. Since $n\ge4$, there exists a $Q_3^i\, (2\le i\le 2^{n-3})$ such that $|N_{Q_n}(x)\cap V(Q_3^i)| = 1$. Let $N_{Q_n}(x)\cap V(Q_3^i) = \{x'\}$ and $y'\in V(Q_3^i)\backslash N_{Q_3^i}[x']$ be an even vertex of $Q_3^i$. By the structure of $Q_n$, we know that $N(x)\cap V(Q_3^i) = \{x'\}$, $N(y)\cap V(Q_3^i) = \{y'\}$ and $yy'$ is an edge, then all vertices of $(V(Q_3^1)\cup V(Q_3^i))\backslash N_{Q_n}[\{x, \, y'\}]$ are even vertices, and $\{x, \, y'\}$ is an isolating set of $Q_3^1\cup Q_3^i$. Choose one even vertex in each $Q_3^j\, (1\le j\le 2^{n-3})$ as the set $S$ such that all vertices of $V(Q_n)\backslash N_{Q_n}[S]$ are even vertices (In Figure 2, $a, \, b, \, c, \, d$ are even vertices of $V(Q_5)$ and $\{a, \, b, \, c, \, d\}$ is an isolating set of $Q_5$). Since each edge of $Q_n$ has an even endvertex and an odd endvertex, the set $V(Q_n)\backslash N_{Q_n}[S]$ is an independent set of $Q_n$, thus the set $S$ is an isolating set of $Q_n$. Since $|S\cap V(Q_3^j)| = 1$ for $1\le j\le 2^{n-3}$, we obtain $\iota(Q_n)\le |S| = 2^{n-3}$. In conclusion, $\frac{2^{n-1}}{n}\le \iota(Q_n)\le 2^{n-3}$ for $n\ge4$.

Figure 2.  $a=\{0,\,0,\,0,\,0,\,0\}$, $b=\{0,\,1,\,1,\,1,\,1\}$, $c=\{1,\,0,\,1,\,1,\,1\}$, $d=\{1,\,1,\,0,\,0,\,0\}$.

DownLoad: Full-Size Img PowerPoint

Especially, if $\frac{2^{n-1}}{n} = 2^{n-3}$, then $n = 4$. So the upper bound is equal to the lower bound if and only if $n = 4$. If $n = 4$, then $\iota(Q_n) = 2 = 2^{n-3} = \frac{2^{n-1}}{n}$ by Theorem $2.2$. Thus, the upper and lower bounds are sharp. □

Corollary 2.4. Let $n$ be a positive integer and $n\ge4$, then $\frac{2^{n-1}}{n}\le \iota_I(Q_n)\le 2^{n-3}$. Moreover, the bounds are sharp.

Proof. Let $S_I$ be a minimum independent isolaing set of $Q_n$. Obviously, $S_I$ is an isolating set of $Q_n$. Thus, by Theorem $2.2$, we have $\iota_I(Q_n)\ge \frac{2^{n-1}}{n}$. Let $\{Q_3^1, \, Q_3^2, \, \cdots, \, Q_3^{2^{n-3}}\}$ be the set of $2^{n-3}$ disjoint $3$-dimensional subcubes of $Q_n$. Choose one even vertex in each $Q_3^i\, (1\le i\le 2^{n-3})$ as the set $S$ such that all vertices of $V(Q_n)\backslash N_{Q_n}[S]$ are even vertices. According to the proof of Theorem $2.2$, the set $S$ is an isolating set of $Q_n$ and $\iota(Q_n)\le 2^{n-3}$. Since all vertices of $S$ are even vertices, the set $S$ is an independent isolating set of $Q_n$. Thus $\iota_I(Q_n)\le 2^{n-3}$. In conclusion, $\frac{2^{n-1}}{n}\le \iota_I(Q_n)\le 2^{n-3}$ for $n\ge4$.

Especially, if $n = 4$, then $\iota(Q_n) = 2 = 2^{n-3} = \frac{2^{n-1}}{n}$ by Theorem $2.2$. Thus, the upper and lower bounds are sharp. □

2.2.   Isolation number of the $n$-star network

The $n$-star network was proposed by Akers, Harel and Krishnamurthy ^[12] as an attractive alternative to the hypercube network for interconnecting processors on a parallel computer. For a positive integer $n$($n\ge2$), the $n$-$star$ $network$ on $n$ symbols, denoted by $S_n$, is the graph with $n!$ vertices, whose the vertex set $V(S_n)$ is all permutations of symbols $1, \, 2, \, \cdots, \, n$, and each vertex $v\in V(S_n)$ is connected to $n-1$ vertices which can be obtained by interchanging the first symbol of $v$ with the $i$th symbol of $v$ for $2\le i \le n$ ($S_4$ is shown as an example in Figure 3).

Figure 3.  $S_4$.

DownLoad: Full-Size Img PowerPoint

Lemma 2.5. ^[13,14] Let $G$ be an $n$-vertex graph with minimum degree $\delta(G)$. If $\delta(G)\ge1$, then $\gamma(G)\le \frac{n}{2}$.

Theorem 2.6. Let $n$ be a positive integer and $n\ge2$, then $\frac{n\cdot (n-2)!}{2}\le \iota(S_n)\le (n-1)!$. Moreover, the bounds are sharp.

Proof. Let $S$ be a minimum isolating set of $S_n$. According to the structure of $S_n$, we know that $S_n$ is a $(n-1)$-regular bipartite graph, and every perfect matching of $S_n$ has $\frac{n!}{2}$ edges. Note that $S$ is a minimum isolating set of $S_n$ and $V(S_n)\backslash N_{S_n}[S]$ is an independent set, then every edge of a perfect matching of $S_n$ has at least one endvertex in $N_{S_n}(S)$, that is, $|N_{S_n}(S)|\ge \frac{n!}{2}$. For any vertex $v\in S$, we have $|N_{S_n}(S)|\le \Delta(S_n)|S| = d(v)|S| = (n-1)|S|$. Thus, $\iota(S_n) = |S|\ge \frac{|N_{S_n}(S)|}{d(v)}\ge \frac{\frac{n!}{2}}{n-1} = \frac{n\cdot (n-2)!}{2}$.

Inspired by Alon and Spencer ^[15] and Caroa and Hansbergb ^[3], we show that $\iota(S_n)\le (n-1)!$ by the probabilistic method. Since $n\ge2$, $d(v) = n-1\ge 1$ for any vertex $v\in V(S_n)$. Let $p\in[0, \, 1]$, and we independently select a vertex subset $A\subset V(S_n)$ at random such that $P(v\in A) = p$. Let $I$ be the set of the isolated vertices of $V(S_n)\backslash A$. Meanwhile, let $B = V(S_n)\backslash(N_{S_n}[A]\cup I)$ and $D$ be a minimum dominating set of $G[B]$. Since there is no isolated vertice in $B$, $\delta (G[B])\ge1$, furthermore, $\gamma(G[B]) = |D| \le \frac{|B|}{2}$ by Lemma $2.5$. Thus, $A\cup D$ is an isolating set of $S_n$. Note that the expectated value $E[|D|]\le E[\frac{|B|}{2}] = \frac{1}{2}E[|B|]$. Hence, we have

Thus, we obtain that

Considering the function $f(p) = (p+\frac{1}{2}(1-p)^n)\cdot(n!)$ and its derivative $f'(p) = (1-\frac{1}{2}n(1-p)^{n-1}\cdot(n!)$, we can see that $f'(p) = 0$ when $p = 1-(\frac{2}{n})^{\frac{1}{n-1}}$. It follows that

Since $n\ge2$, we have $(\frac{2}{n})^{\frac{1}{n-1}}\le 1$. Then $\iota(S_n)\le (1-1+\frac{1}{2}(\frac{2}{n})\cdot(n!) = \frac{1}{n}\cdot(n!) = (n-1)!$.

In conclusion, $\frac{n\cdot (n-2)!}{2}\le \iota(S_n)\le (n-1)!$ for any positive integer $n\ge2$.

Especially, if $n = 2$, then $S_2\cong K_2$, and $\iota(K_2) = 1 = \frac{2\cdot 0!}{2} = \frac{n\cdot (n-2)!}{2}$. If $n = 3$, then $S_3\cong C_6$, and $\iota(C_6) = 2 = (3-1)! = (n-1)!$. Hence, the bounds are sharp. □

Corollary 2.7. Let $n$ be a positive integer and $n\ge2$, then $\frac{n\cdot (n-2)!}{2}\le \iota_I(S_n)\le (n-1)!$. Moreover, the bounds are sharp.

Proof. Let $S_I$ be a minimum independent isolaing set of $S_n$. Obviously, $S_I$ is also an isolating set of $S_n$. By Theorem $2.6$, we have $\iota_I(S_n)\ge \frac{n\cdot (n-2)!}{2}$. Let $V_1$ be the set of all vertices with the first symbol is $1$. Clearly, the set $V_1$ is an independent set of $S_n$. According the structure of $S_n$, we know that any vertex of $V_1$ has different $n-1$ neighbors, and the first symbol of these $n-1$ neighbors is $2, \, 3, \, \cdots, \, n-1, \, n$ respectively. Let $x$, $y\in V_1$ and $x\neq y$. If $N_{S_n}(x)\cap N_{S_n}(y)\neq \emptyset$, then there exists a vertex to be adjacent to $x$ and $y$, which contradicts the definition of $S_n$. Thus, $N_{S_n}(x)\cap N_{S_n}(y) = \emptyset$, that is, the neighborhoods of any two vertices of $V_1$ are disjoint. Hence, $|N_{S_n}[V_1]| = (n-1)\cdot |V_1|+|V_1| = n\cdot|V_1| = n\cdot(n-1)! = n! = |V(S_n)|$, this means that $G[V(S_n)\backslash N_{S_n}[V_1]]$ has no edge. Since $V_1$ is independent, the set $V_1$ is an independent isolating set of $S_n$. Then $\iota_I(S_n)\le |V_1| = (n-1)!$. In conclusion, $\frac{n\cdot (n-2)!}{2}\le \iota_I(S_n)\le (n-1)!$ for any positive integer $n\ge2$.

Especially, if $n = 2$, then $\{(1, \, 2)\}$ is an independent isolating set of $S_2$. Thus, $\iota_I(S_2) = 1 = \frac{2\cdot 0!}{2} = \frac{n\cdot (n-2)!}{2}$. If $n = 3$, then $\{(1, \, 2, \, 3), (1, \, 3, \, 2)\}$ is an independent isolating set of $S_3$. Thus, $\iota_I(S_3) = 2 = (3-1)! = (n-1)!$. Hence, the bounds are sharp. □

3.   Conclusions and problems

The hypercube network $Q_n$ and $n$-star network $S_n$ are both recursively constructed networks, and they have many known and attractive topological properties. This paper demonstrates the sharp bounds of the isolation number and the independent isolation number of the hypercube network and $n$-star network. In view of this research direction, there are still many academic issues worth studying:

Problem 1. Let $m\ge1$ be a positive integar and $\mathcal{F} = \{F_1, \, F_2, \, \cdots, \, F_m\}$. For any $F_i\in\mathcal{F}$, if $F_i\ncong K_2$, what $F_i$ can be used to explore the $\mathcal{F}$-isolation number of the hypercube network or $n$-star network?

Problem 2. Consider the $\mathcal{F}$-isolation number of some other network models.

For future work, it would be interesting and meaningful to probe and research the $\mathcal{F}$-isolation number of some other network models.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

This work is supported by the Science Found of Qinghai Province (No. 2021-ZJ-703), and the National Science Foundation of China (Nos.11661068, 12261074 and 12201335).

The authors are grateful to the reviewers for their helpful suggestions and comments.

Conflict of interest

All authors declare no conflicts of interest in this paper.