Pointwise error estimate of conservative difference scheme for supergeneralized viscous Burgers' equation

1.   Introduction

In this paper, we shall present a incisive analysis of a finite difference method for solving the following supergeneralized viscous Burgers' equation in the domain $[0, L] \times [0, T]$:

here $L$ and $T$ are positive constants, $\Psi(x)$ that satisfies $\Psi(0) = \Psi(L) = 0$ is smooth on $[0, L]$, $p \geq 1$ and $q \geq 0$ are two positive integers, and positive constant $\nu$ denotes the dynamic viscosity coefficient.

In the last few decades, Burgers' equation for the case of supergeneralized viscous Burgers' equation with $p = 1$ and $q = 0$ has attracted much attention from researchers. It is caused by numerous effective applications of Burgers' equation to many fields of science and engineering like shock wave theory, cosmology, gas dynamics, quantum field and traffic flow, see e.g., ^[2,3,4,5,6]. The supergeneralized viscous Burgers' equation is a typical evolution equation, and recently a series of numerical methods have been developed to solve it, e.g., finite difference method ^{[7,8,9,10,11]}, finite volume method ^[12,13,14], ADI method ^{[15,16,17,18]}, collocation method ^[19,20], two-grid method ^[21,22] and extrapolation method ^[23]. Meanwhile, as the other simplified form of supergeneralized viscous Burgers' equation with $p \geq 1$ and $q = 0$, the generalized Burgers' equation also plays an important role in applied mathematics and engineering, see e.g., ^{[24,25,26,27]}. Recently, Wang et al. ^[28] established two conservative fourth-order compact schemes for Burgers' equation. Zhang et al. ^[29,30] derived various efficient difference schemes for Burgers' type equations. Gao et al. ^[31] proposed a bounded high-order upwind scheme in the normalized-variable formulation for the modified Burgers' equations. Guo et al. ^[32] proposed a BDF3 finite difference scheme for the generalized viscous Burgers' equation. Hu et al. ^[33] considered an implicit difference scheme to study the local conservation properties for Burgers' equation. Pany et al. ^[34] investigated an $H^1$-Galerkin mixed finite element method to approximate the solution of the Burgers' equation. In addition, Jiwari et al. ^[35] studied a numerical scheme which is a composition of forward finite difference, quasilinearization process and uniform Haar wavelets for solving Burgers' equation. Wang et al. ^[36] used the weak Galerkin finite element method to study a class of time fractional generalized Burgers' equation. Wang et al. ^[37,38,39] presented an implicit robust difference method to solve the modified Burgers equation on graded meshes. Zhang et al. ^[40] provided a fourth-order compact difference scheme for time-fractional Burgers' equation. Zhang et al. ^[41] considered a conservative decoupled difference scheme for the rotation-two-component Camassa-Holm system. Sun et al. ^[42] obtained nonlinear discrete scheme for generalized Burgers' equation with the help of meshless method. Zhang et al. ^[1] constructed various difference schemes for generalized Burgers' equation only with one positive parameter $p\geq 1$.

The previous works are mainly concerned with the simple case of the parameter $p = 1$ for problem (1.1)–(1.3). Our scheme can extended the results in the previous work ^[1] with a positive integer $p\geq 1$. In this paper, the main contributions are as follows:

● We construct the discretization of the nonlinear term by a second-order operator in supergeneralized viscous Burgers' equation and provide complete theoretical analysis on the proposed scheme, including conservation, existence, uniqueness and convergence.

● We prove $L_2$-norm and $L_{\infty}$-norm convergence in pointwise sense by the cut-off function method, which doesn't have any step ratio restrictions. The $L_2$-norm and $L_{\infty}$-norm convergence are proved with separate and different ways, which is different from previous work in ^[1].

The rest of the paper is arranged as follows. We introduce some useful notations for discretization and construct our proposed scheme in Section 2. In Section 3, we present certain conclusions about conservative invariants and boundedness of the suggested numerical scheme, and we provide the proof of unique solvability and convergence. The numerical test in Section 4 is given to demonstrate the reliability of our analysis. A brief conclusion is followed in Section 5.

2.   Derivation of the three-level difference scheme

Firstly, for any integer $s$, we denote set $N_{s} = \{i| 1\leq i\leq s, i\in Z\}$ and $N_{s}^0 = \{i| 0\leq i\leq s, i\in Z\}$. For two positive integers $\tilde{m}$ and $\tilde{n}$, define the spatial step $h = \frac{L}{\tilde{m}}$, and the temporal step $\tau = \frac{T}{\tilde{n}}$. Denote$\, x_i = ih, \, i\in N_{\tilde{m}}^0;\ t_k = k\tau, \, k\in N_{\tilde{n}}^0$. We introduce the mesh $\tilde{\omega}_{LT} = \tilde{\omega}_L\times \tilde{\omega}_T,$ where $\tilde{\omega}_L = \{x_i\, |\, i\in N_{\tilde{m}}^0\}$, and $\tilde{\omega}_T = \{t_k\, |\, k\in N_{\tilde{n}}^0\}$. Denote $x_{i+\frac12} = \frac12(x_i+x_{i+1}), i\in N_{\tilde{m}-1}^0$ and $t_{k+\frac12} = \frac{1}{2}(t_k+t_{k+1}), k\in N_{\tilde{n}-1}^0$.

Let $\mathcal{J}_h = \{j\, |\, j = (j_0, j_1, \cdots, j_{\tilde{m}})\}$ and ${\mathop{\mathcal{J}}\limits^\circ}_h = \{j\, |\, j\in \mathcal{J}_h, j_0 = j_{\tilde{m}} = 0\}$ be the spaces of grid functions on $\tilde{\omega}_L$. For $d, j\in \mathcal{J}_h$, introducing the following notations:

Lemma 2.1. ^[28] Let $j\in \mathcal{J}_h$ and $r\in {\mathop{\mathcal{J}}\limits^\circ}_h,$ then

Lemma 2.2. ^[28] Set $j\in {\mathop{\mathcal{J}}\limits^\circ}_h$, then

Lemma 2.3. Suppose that $U = (U_0, U_1, \ldots, U_{\tilde{m}})$, $u = (u_0, u_1, \ldots, u_{\tilde{m}})\in \mathcal{J}_h$ and $g(u)$ is a second-order smooth function. Denote $e = (e_0, e_1, \ldots, e_{\tilde{m}})$ and $e_i = U_i-u_i$, $i\in N_{\tilde{m}}^0$. Then there are $\rho\in (0, 1)$ and $\zeta_i\in(y_i, r_i)$ such that

where

Proof. Using the mean value theorem, one has

Again, applying the mean value theorem, we have

The proof is finished.

In order to construct a three-level conservative numerical scheme for supergeneralized viscous Burgers' equation (1.1)–(1.3), we first turn problem (1.1) into an equivalent form as follows:

where $C_q^m$ is the binomial coefficient, $0\leq m\leq q$.

We denote $U^k_i = u(x_i, t_k)$, and let $u_i^k$ denote the nodal approximation to the exact solution computed at the mesh point $(x_i, t_k)$.

Considering (2.2) at the point $(x_i, t_k)$, $i\in N_{\tilde{m}-1}$, $k\in N_{\tilde{n}-1}$, one gets

By Taylor expansion, one gets

where $c_1$ is a positive constant.

We consider (1.1) at the point $(x_i, t_0)$, $i\in N_{\tilde{m}-1}$, noticing (1.2), and one gets

Denote

Considering (2.2) at the point $(x_i, t_{\frac{1}{2}})$, $i\in N_{\tilde{m}-1}$, one gets

and

Noticing (1.2) and (1.3), we get

Omitting the small terms $P_i^k$ in (2.3) and $P_i^0$ in (2.7), and replacing $U_i^k$ by $u_i^k$, and ${W_{(m)}}_i^k$ by ${w_{(m)}}_i^k$, $i\in N_{\tilde{m}-1}$, $k\in N_{\tilde{n}-1}$, respectively. Thus, we can obtain the three-level difference approximation for (1.1)–(1.3) as follows

Noticing that substituting (2.12) into (2.10), the three-level difference scheme only contains one variable $u^k_i$.

3.   The numerical analysis of three-level difference scheme

We now begin to consider the energy conservation and boundedness of solution of the three-level numerical scheme (2.10)–(2.14).

Theorem 3.1. Suppose that $\{u^k_i, {w_{(m)}}_i^k\, |\, i\in N_{\tilde{m}}^0, k\in N_{\tilde{n}}^0\}$ is the solution of (2.10)–(2.14), we get

where

Proof. 1) Taking the inner product of (2.11) with $u^{\frac12}$, one obtains

Since $u^{\frac12}\in {\mathop{\mathcal{J}}\limits^\circ}_h$, by Lemmas 2.1 and 2.2, one gets

Thus,

Namely,

2) Taking the inner product of (2.10) with $u^{\bar{k}}$, one gets

Since $u^{\bar{k}}\in {\mathop{\mathcal{J}}\limits^\circ}_h$, by Lemmas 2.1 and 2.2, we have

Thus,

Above equality can be rewritten as

Thus,

Corollary 3.2. Let $\{u^k_i, {w_{(m)}}_i^k\, |\, i\in N_{\tilde{m}}^0, k\in N_{\tilde{n}}^0\}$ represent the solution of (2.10)–(2.14). Then one has

Proof. According to Theorem 3.1,

Thus,

Corollary 3.3. Let $\{u^k_i, {w_{(m)}}_i^k\, |\, i\in N_{\tilde{m}}^0, k\in N_{\tilde{n}}^0\}$ represent the solution of (2.10)–(2.14). Then the computed solution $u^k_i$ can satisfy

Proof. From (3.4) and (3.5) in Theorem 3.1, we can get Corollary 3.3 directly. □

Furthermore, we will carry out the proof of existence and uniqueness of the solution of (2.10)–(2.14).

Theorem 3.4. The solution of (2.10)–(2.14) exists and it is unique.

Proof. According to (2.13) and (2.14), $u^0$ has been determined uniquely. From (2.11) and (2.14), establishing a linear system with respect to $u^1$, and considering the corresponding homogeneous system

Taking the inner product of (3.6) with $u^1$, one has

By Lemmas 2.1 and 2.2, one gets

Therefore,

It is easy to obtain

It implies that (2.11) and (2.14) determine $u^1$ uniquely.

Assume that $u^k$ and $u^{k-1}$ have been known. By (2.10), (2.12) and (2.14), we get the following linear homogeneous system of equations with respect to $u^{k+1}$:

Taking the inner product of (3.8) with $u^{k+1}$, one has

By Lemmas 2.1 and 2.2, one gets

Therefore,

It is easy to obtain

Consequently, it implies that $u^{k+1}$ solved by (2.10), (2.12) and (2.14) is unique.

Based on mathematical induction, (2.10)–(2.14) is uniquely solvable, and this completes the proof. □

In order to establish the convergence of (2.10)–(2.14), we will introduce the cut-off function method next.

Denote

Define a group of second-order smooth functions

where $0\leq m\leq q$.

Denote

Based on the cut-off function method, we construct a new difference scheme as follows:

For the above difference scheme, it is conservative.

Theorem 3.5. Suppose that $\{u^k_i, {w_{(m)}}_i^k\, |\, i\in N_{\tilde{m}}^0, k\in N_{\tilde{n}}^0\}$ represents the solution of (3.11)–(3.15), we get

where

Proof. The proof of (3.16) and (3.17) is similar to the proof of Theorem 3.1. □

Now we prove the $L_2$-norm and $L_{\infty}$-norm convergence of (3.11)–(3.15).

Theorem 3.6. Assume that $\{u^k_i, {w_{(m)}}_i^k\, |\, i\in N_{\tilde{m}}^0, k\in N_{\tilde{n}}^0\}$ is the solution of (3.11)–(3.15) and $\{U^k_i, {W_{(m)}}_i^k\, |\, i\in N_{\tilde{m}}^0, k\in N_{\tilde{n}}^0\}$ is the solution of (1.1)–(1.3), there exists a positive constant $c_{2}$ such that

Proof. Define

Since (3.10), we get

Subtracting (3.11)–(3.15) from (2.3), (2.7) and (2.9) follows

When $k = 0$, from (3.22) and (3.23), we get

Taking the inner product of (3.19) with $e^{\frac12}$, one gets

By Lemmas 2.1 and 2.2, we obtain

Substituting (3.26)–(3.28) into (3.25), we have

Thus,

When $\frac{\tau}{2}\leq \frac{1}{3}$, noticing (2.8), one gets

or

By (3.10) and Lagrange mean value theorem, one gets

Taking the inner product of (3.20) with $e^{\bar{k}}$, one gets

Using Lemma 2.2, one obtains

Substituting (3.33) and (3.34) into (3.32), above equality (3.32) becomes

where $a_0 = \max_{0\leq m\leq q} C^m_q$.

Noticing that

Thus, by Lemma 2.1, we have

Noticing (3.30), (3.31) and (3.10), we have

Substituting (3.37) into (3.35), (3.35) yields

Combining (2.4), above equality (3.38) becomes

where $c_{3} = \frac{a_0(1+q)\tilde{c}_1\hat{c}_1}{2(p+2)} + \frac{a_0^2(1+q)^2M^2\hat{c}_1^2}{4(p+2)^2\nu}$ and $c_{4} = \frac{a_0(1+q)\tilde{c}_1\hat{c}_1}{4(p+2)} +\frac{1}{4}$ are two positive constants.

Rearranging (3.39) to yield

For $k\in N_{\tilde{n}-1}$, when $4c_{4}\tau\leq \frac{1}{3}$, (3.40) yields

Therefore,

According to Gronwall's inequality, we obtain

Noticing (3.24) and (3.29), one gets

where $c_{2} = e^{6(c_{3}+2c_{4})T}\cdot[Lc^2_1 +\frac{Lc^2_1}{2(c_{3}+2c_{4})}]^{\frac{1}{2}}$.

Namely,

Theorem 3.7. Assume that $\{u^k_i, {w_{(m)}}_i^k\, |\, i\in N_{\tilde{m}}^0, k\in N_{\tilde{n}}^0\}$ is the solution of (3.11)–(3.15) and $\{U^k_i, {W_{(m)}}_i^k\, |\, i\in N_{\tilde{m}}^0, k\in N_{\tilde{n}}^0\}$ is the solution of (1.1)–(1.3), there exists positive constants $c_7$ and $c_8$ such that

Proof. We will use the mathematical induction to prove the result. When $k = 0$, from (3.22) and (3.23), we get

Therefore, the conclusion is valid for $k = 0$.

1) Taking the inner product of (3.19) with $\delta_te^{\frac12}$, one gets

Noticing that

then (3.46) becomes

Using Lemmas 2.1 and 2.2, we have

From (2.8), we get

When $\tau\leq2\nu$, one gets

or

2) Taking the inner product of (3.20) with $\Delta_te^k$, one gets

Suppose (3.43) and (3.44) hold for $0\leq k\leq s$ $(1\leq s\leq \tilde{n}-1)$.

From (3.10) and Lemma 2.2, one gets

When $c_7(\tau^2 + h^2)\leq 1$, one gets

Using Lemma 2.2, above equality (3.50) becomes

Noticing that

By Lagrange mean value theorem and the Lemma 2.3, we have

Thus, combining (3.54) and (3.55) yields

Using Lemma 2.2, combining (3.51), (3.52) and (3.56), it is easy to get

where $c_{9} = (L\hat{c}_1\tilde{c}_1 +\frac{1}{2}L\hat{c}_1\tilde{c}_1 +\frac{1}{2\sqrt{6}}L^2\hat{c}_2\tilde{c}_1^2)$ and $c_{10} = 2\hat{c}_0+\frac{1}{2}\sqrt{L}\hat{c}_1(\sqrt{L}\tilde{c}_1+1)$.

Thus, (3.53) becomes

where $a_0 = \max_{0\leq m\leq q}C^m_q$.

Noticing (2.4), (3.58) becomes

where $c_5 = \frac{a_0^2(q+1)^2c_{9}^2}{(p+2)^2}$ and $c_6 = \frac{a_0^2(q+1)^2c_{10}^2}{(p+2)^2}$ are two positive constants.

For $1\leq k\leq s$, rearranging (3.59) to yield

When $\frac{2c_6\tau}{\nu}\leq \frac{1}{3}$, (3.60) yields

Therefore,

According to Gronwall's inequality, (3.61) yields

Noticing (3.45) and (3.49), one gets

where $c_7 = e^{\frac{3c_5+3c_6}{\nu}T}\cdot[Lc^2_1 +\frac{Lc^2_1}{2(c_5+c_6)}]^{\frac{1}{2}}$.

Namely,

Consequently, (3.43) holds for $k = s+1$.

From Lemma 2.2, it is easy to get

Corollary 3.8. Let $\{u^k_i, {w_{(m)}}_i^k\, |\, i\in N_{\tilde{m}}^0, k\in N_{\tilde{n}}^0\}$ be the solution of (3.11)–(3.15). When $c_7(\tau^2+h^2)\leq 1$, there exists two constants $c_{11}$ and $c_{12}$ such that

Proof. When $c_7(\tau^2+h^2)\leq 1$, one has

By Lemma 2.2, we get $\|u^k\|_\infty \leq \frac{\sqrt{L}}{2} c_{10} \equiv c_{12}$.

This means the solution of (3.11)–(3.15) is bounded. □

In the end, for the proposed scheme (2.10)–(2.14), we can obtain the following convergence.

Corollary 3.9. Let $\{u^k_i, {w_{(m)}}_i^k\, |\, i\in N_{\tilde{m}}^0, k\in N_{\tilde{n}}^0\}$ be the solution of (2.10)–(2.14) and $\{U^k_i, {W_{(m)}}_i^k\, |\, i\in N_{\tilde{m}}^0, k\in N_{\tilde{n}}^0\}$ be the solution of (1.1)–(1.3). When $c_8(\tau^2+h^2)\leq 1$, one has

where $c_{13}$ is a constant.

Proof. Let $\{\hat{u}^k_i\, |\, i\in N_{\tilde{m}}^0, k\in N_{\tilde{n}}^0\}$ be the solution of (3.11)–(3.15). When $c_8(\tau^2+h^2)\leq 1$, one has

Thus, $g_m(\hat{u}_i^k) = (\hat{u}_i^k)^{p+m}$.

This means the difference scheme (2.10)–(2.14) is equivalent to (3.11)–(3.15). According to Theorems 3.6 and 3.7, we finish the proof of Corollary 3.9. □

4.   Numerical test

A numerical example is given to verify theoretical conclusions of the three-level difference scheme for supergeneralized viscous Burgers' equation.

Example 4.1. We consider (1.1)–(1.3) with $T = L = 1$, $\nu = 1$, $\Psi(x) = \sin(\pi x)$, and $p$, $q$ take some different integer values, respectively.

To describe the numerical errors in $L_{\infty}$-norm for the computed solution and corresponding convergence orders, we denote

and

where $h$ and $\tau$ are sufficiently small.

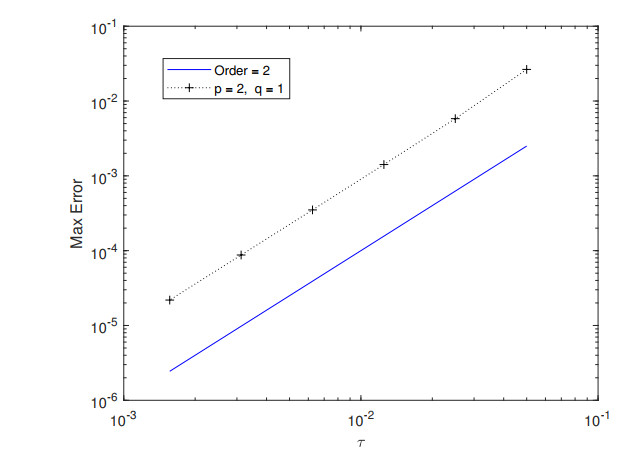
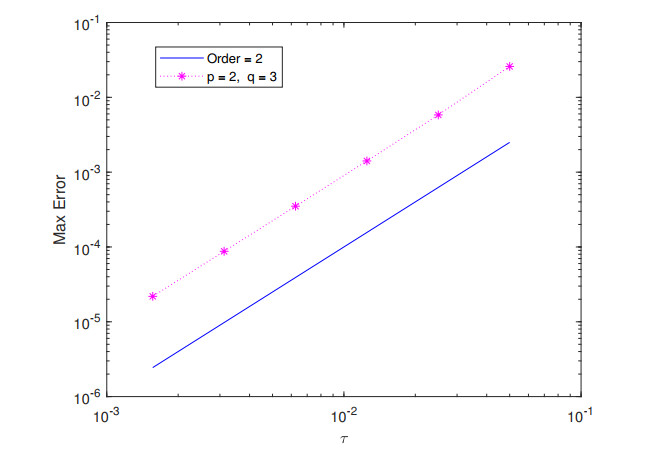
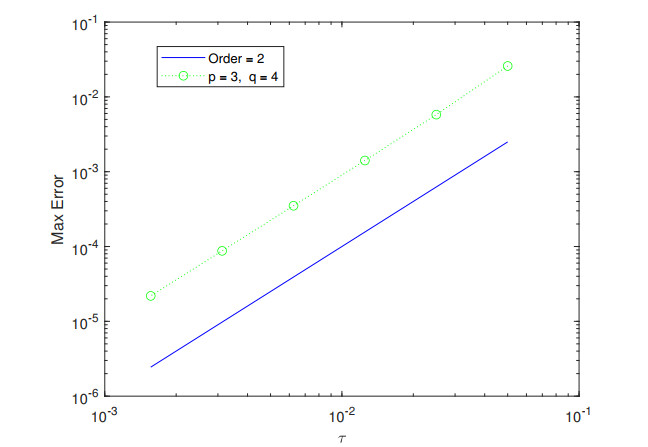
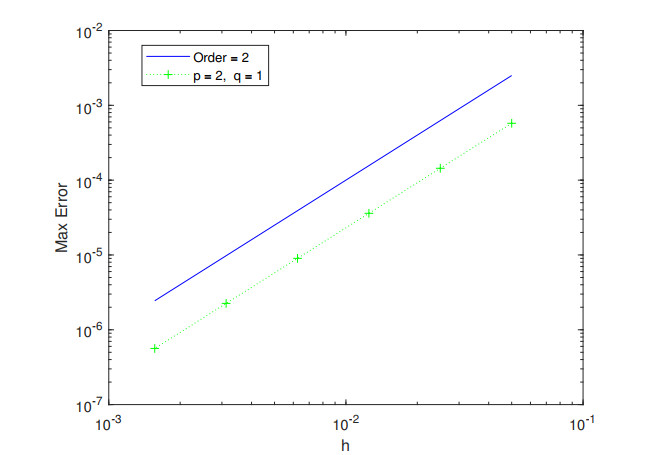
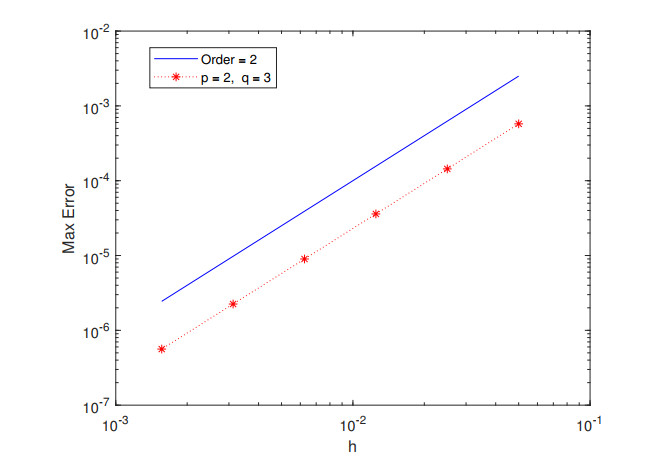
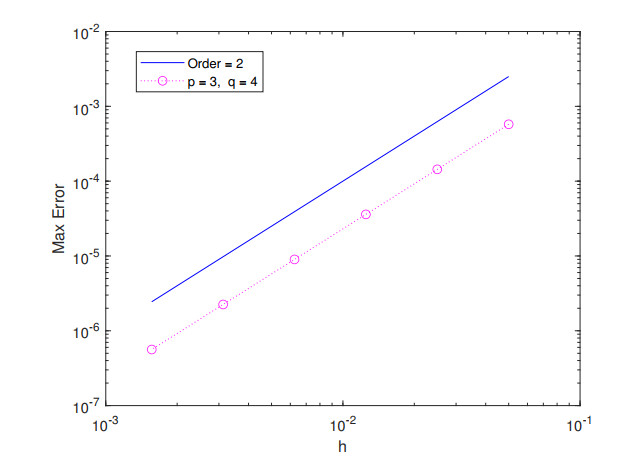
Table 1 lists the temporal convergence orders with $h = \frac{1}{64}$. We compute the spatial convergence orders with $\tau = \frac{1}{64}$ in Table 2. Table 3 presents the temporal and spatial errors and convergence orders with $\tau = h$. The corresponding error and convergence orders are presented in Figures 1–6. The results demonstrate (2.10)–(2.14) is convergent with the convergence order of two both in space and in time.

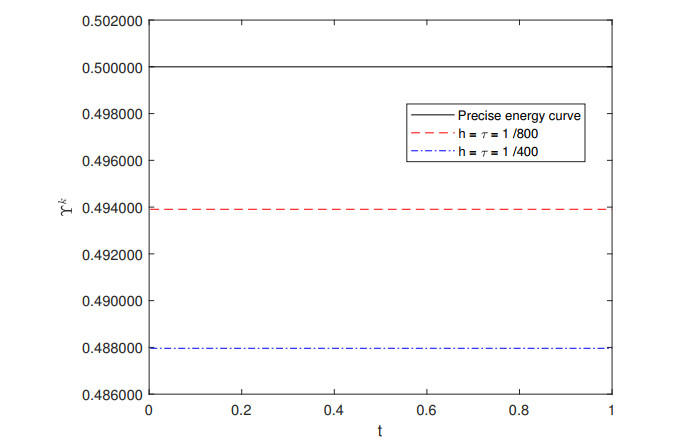
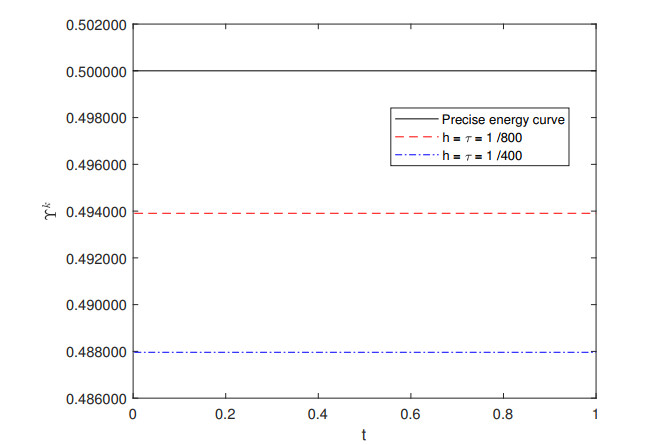
In Figures 7–9, we compute $\Upsilon^k$ in Theorem 3.1 to verify the conservativity of the difference scheme (2.10)–(2.14). The results demonstrate that difference scheme (2.10)–(2.14) is conservative.

5.   Conclusions

In this paper, a three-level linearized conservative scheme approximating supergeneralized viscous Burgers' equation is studied. We construct the discretization of the nonlinear term by a second-order operator in supergeneralized viscous Burgers' equation and prove the three-level scheme is uniquely solvable based on the mathematical induction. At last, the $L_2$-norm and $L_{\infty}$-norm convergence are proved with separate and different ways.

Use of AI tools declaration

The authors declare that they have not used Artificial Intelligence (AI) tools in the creation of this article.

Conflicts of interest

The authors declare no conflict of interest.