A novel Timoshenko beam model enriched to account for dissipation in cement-based materials was presented in this paper. The model introduced a new variable representing the relative sliding inside microcracks within the material. In the paper, the microcrack density was not supposed to increase, assuming a small deformation regime that implied no damage growth. The model utilized an expanded version of the principle of virtual work whose contributions came from external forces, internal elastic forces, and dissipation due to the microcrack's microstructure. The elastic energy included terms related to microcrack sliding and micro-macro interactions, accounting for nonlinearity in the material behavior. Numerical simulations, conducted using the finite element method, evaluated the mechanical properties of cement-based materials under three-point flexural tests and compression tests. These tests enabled the assessment of the material dissipative behavior under cyclic loading. Results showed dissipated energy cycles and mechanical responses influenced by the microcrack mechanics. Additionally, a parametric study, varying the friction force amplitude, revealed its impact on dissipated energy. The study highlighted a non-monotonic relationship between friction force amplitude and dissipated energy, with an optimal value maximizing dissipation. Overall, the model provided insights into the mechanics of cement-based materials, particularly regarding dissipation, which was essential for understanding their behavior in structural applications.
Citation: Giuliano Aretusi, Christian Cardillo, Larry Murcia Terranova, Ewa Bednarczyk. A dissipation model for concrete based on an enhanced Timoshenko beam[J]. Networks and Heterogeneous Media, 2024, 19(2): 700-723. doi: 10.3934/nhm.2024031
A novel Timoshenko beam model enriched to account for dissipation in cement-based materials was presented in this paper. The model introduced a new variable representing the relative sliding inside microcracks within the material. In the paper, the microcrack density was not supposed to increase, assuming a small deformation regime that implied no damage growth. The model utilized an expanded version of the principle of virtual work whose contributions came from external forces, internal elastic forces, and dissipation due to the microcrack's microstructure. The elastic energy included terms related to microcrack sliding and micro-macro interactions, accounting for nonlinearity in the material behavior. Numerical simulations, conducted using the finite element method, evaluated the mechanical properties of cement-based materials under three-point flexural tests and compression tests. These tests enabled the assessment of the material dissipative behavior under cyclic loading. Results showed dissipated energy cycles and mechanical responses influenced by the microcrack mechanics. Additionally, a parametric study, varying the friction force amplitude, revealed its impact on dissipated energy. The study highlighted a non-monotonic relationship between friction force amplitude and dissipated energy, with an optimal value maximizing dissipation. Overall, the model provided insights into the mechanics of cement-based materials, particularly regarding dissipation, which was essential for understanding their behavior in structural applications.
| [1] |
A. Misra, Stabilization characteristics of clays using class C fly ash, Transp. Res. Rec., 1611 (1998), 46–54. https://doi.org/10.3141/1611-06 doi: 10.3141/1611-06
|
| [2] |
D. A. S. Sclofani, L. Contrafatto, Experimental behaviour of polyvinyl-alcohol modified concrete, Adv. Mater. Res., 687 (2013), 155–160. https://doi.org/10.4028/www.scientific.net/AMR.687.155 doi: 10.4028/www.scientific.net/AMR.687.155
|
| [3] | L. Contrafatto, 8-Volcanic ash, in Sustainable Concrete Made with Ashes and Dust from Different Sources, (eds. R. Siddique and R. Belarbi), Elsevier, (2022), 331–418. https://doi.org/10.1016/B978-0-12-824050-2.00011-5 |
| [4] |
L. Contrafatto, R. Cosenza, R. Barbagallo, S. Ognibene, Use of recycled aggregates in road sub-base construction and concrete manufacturing, Ann. Geophys., 61 (2018), SE223. https://doi.org/10.4401/ag-7785 doi: 10.4401/ag-7785
|
| [5] |
G. Goel, P. Sachdeva, A. K. Chaudhary, Y. Singh, The use of nanomaterials in concrete: A review, Mater. Today Proc., 69 (2022), 365–371. https://doi.org/10.1016/j.matpr.2022.09.051 doi: 10.1016/j.matpr.2022.09.051
|
| [6] |
P. Franciosi, M. Spagnuolo, O. U. Salman, Mean Green operators of deformable fiber networks embedded in a compliant matrix and property estimates, Continuum Mech. Thermodyn., 31 (2019), 101–132. https://doi.org/10.1007/s00161-018-0668-0 doi: 10.1007/s00161-018-0668-0
|
| [7] |
M. Spagnuolo, Symmetrization of mechanical response in fibrous metamaterials through micro-shear deformability, Symmetry, 14 (2022), 2660. https://doi.org/10.3390/sym14122660 doi: 10.3390/sym14122660
|
| [8] |
M. Spagnuolo, P. Franciosi, F. Dell'Isola, A Green operator-based elastic modeling for two-phase pantographic-inspired bi-continuous materials, Int. J. Solids Struct., 188 (2020), 282–308. https://doi.org/10.1016/j.ijsolstr.2019.10.018 doi: 10.1016/j.ijsolstr.2019.10.018
|
| [9] |
I. Giorgio, N. L. Rizzi, E. Turco, Continuum modelling of pantographic sheets for out-of-plane bifurcation and vibrational analysis, Proc. R. Soc. A, 473 (2017), 1–21. https://doi.org/10.1098/rspa.2017.0636 doi: 10.1098/rspa.2017.0636
|
| [10] |
E. Turco, M. Golaszewski, I. Giorgio, F. D'Annibale, Pantographic lattices with non-orthogonal fibres: Experiments and their numerical simulations, Composites, Part B, 118 (2017), 1–14. https://doi.org/10.1016/j.compositesb.2017.02.039 doi: 10.1016/j.compositesb.2017.02.039
|
| [11] |
E. Turco, I. Giorgio, A. Misra, F. Dell'Isola, King post truss as a motif for internal structure of (meta) material with controlled elastic properties, R. Soc. Open Sci., 4 (2017), 171153. https://doi.org/10.1098/rsos.171153 doi: 10.1098/rsos.171153
|
| [12] |
P. P. Abhilash, D. K. Nayak, B. Sangoju, R. Kumar, V. Kumar, Effect of nano-silica in concrete; a review, Constr. Build. Mater., 278 (2021), 122347. https://doi.org/10.1016/j.conbuildmat.2021.122347 doi: 10.1016/j.conbuildmat.2021.122347
|
| [13] |
N. De Belie, E. Gruyaert, A. Al‐Tabbaa, P. Antonaci, C. Baera, D. Bajare, et al., A review of self-healing concrete for damage management of structures, Adv. Mater. Interfaces, 5 (2018), 1800074. https://doi.org/10.1002/admi.201800074 doi: 10.1002/admi.201800074
|
| [14] |
S. Sangadji, E. Schlangen, Mimicking bone healing process to self repair concrete structure novel approach using porous network concrete, Procedia Eng., 54 (2013), 315–326. https://doi.org/10.1016/j.proeng.2013.03.029 doi: 10.1016/j.proeng.2013.03.029
|
| [15] |
A. Casalotti, F. D'annibale, G. Rosi, Multi-scale design of an architected composite structure with optimized graded properties, Composite Structures, 252 (2020), 112608. https://doi.org/10.1016/j.compstruct.2020.112608 doi: 10.1016/j.compstruct.2020.112608
|
| [16] |
T. Lekszycki, F. Dell'Isola, A mixture model with evolving mass densities for describing synthesis and resorption phenomena in bones reconstructed with bio-resorbable materials, ZAMM Z. Angew. Math. Mech., 92 (2012), 426–444. https://doi.org/10.1002/zamm.201100082 doi: 10.1002/zamm.201100082
|
| [17] |
E. I. Bednarczyk, T. Lekszycki, W. Glinkowski, Effect of micro-cracks on the angiogenesis and osteophyte development during degenerative joint disease, Comput. Assisted Methods Eng. Sci., 24 (2018), 149–156. http://doi.org/10.24423/cames.191 doi: 10.24423/cames.191
|
| [18] |
I. Giorgio, F. Dell'Isola, U. Andreaus, F. Alzahrani, T. Hayat, T. Lekszycki, On mechanically driven biological stimulus for bone remodeling as a diffusive phenomenon, Biomech. Model. Mechanobiol., 18 (2019), 1639–1663. https://doi.org/10.1007/s10237-019-01166-w doi: 10.1007/s10237-019-01166-w
|
| [19] |
I. Giorgio, F. Dell'Isola, U. Andreaus, A. Misra, An orthotropic continuum model with substructure evolution for describing bone remodeling: an interpretation of the primary mechanism behind Wolff's law, Biomech. Model. Mechanobiol., 22 (2023), 2135–2152. https://doi.org/10.1007/s10237-023-01755-w doi: 10.1007/s10237-023-01755-w
|
| [20] |
M. Rajczakowska, K. Habermehl-Cwirzen, H. Hedlund, A. Cwirzen, Autogenous self-healing: A better solution for concrete, J. Mater. Civ. Eng., 31 (2019), 03119001. https://doi.org/10.1061/(ASCE)MT.1943-5533.0002764 doi: 10.1061/(ASCE)MT.1943-5533.0002764
|
| [21] |
M. E. Espitia-Nery, D. E. Corredor-Pulido, P. A. Castaño-Oliveros, J. A. Rodríguez-Medina, Q. Y. Ordoñez-Bello, M. S. Pérez-Fuentes, Mechanisms of encapsulation of bacteria in self-healing concrete, Dyna, 86 (2019), 17–22. https://doi.org/10.15446/dyna.v86n210.75343 doi: 10.15446/dyna.v86n210.75343
|
| [22] |
J. Xue, B. Briseghella, F. Huang, C. Nuti, H. Tabatabai, B. Chen, Review of ultra-high performance concrete and its application in bridge engineering, Constr. Build. Mater., 260 (2020), 119844. https://doi.org/10.1016/j.conbuildmat.2020.119844 doi: 10.1016/j.conbuildmat.2020.119844
|
| [23] |
L. Placidi, F. Dell'Isola, A. Kandalaft, R. Luciano, C. Majorana, A. Misra, A granular micromechanic-based model for Ultra High Performance Fiber-Reinforced Concrete (UHP FRC), Int. J. Solids Struct., 297 (2024), 112844. https://doi.org/10.1016/j.ijsolstr.2024.112844 doi: 10.1016/j.ijsolstr.2024.112844
|
| [24] |
V. Nguyen-Van, B. Panda, G. Zhang, H. Nguyen-Xuan, P. Tran, Digital design computing and modelling for 3-D concrete printing, Autom. Constr., 123 (2021), 103529. https://doi.org/10.1016/j.autcon.2020.103529 doi: 10.1016/j.autcon.2020.103529
|
| [25] |
A. Kezmane, B. Chiaia, O. Kumpyak, V. Maksimov, L. Placidi, 3D modelling of reinforced concrete slab with yielding supports subject to impact load, Eur. J. Environ. Civ. Eng., 21 (2017), 988–1025. https://doi.org/10.1080/19648189.2016.1194330 doi: 10.1080/19648189.2016.1194330
|
| [26] |
A. Casalotti, F. D'Annibale, G. Rosi, Optimization of an architected composite with tailored graded properties, Z. Angew. Math. Phys., 75 (2014), 126. https://doi.org/10.1007/s00033-024-02255-2 doi: 10.1007/s00033-024-02255-2
|
| [27] |
I. Giorgio, A. Ciallella, D. Scerrato, A study about the impact of the topological arrangement of fibers on fiber-reinforced composites: Some guidelines aiming at the development of new ultra-stiff and ultra-soft metamaterials, Int. J. Solids Struct., 203 (2020), 73–83. https://doi.org/10.1016/j.ijsolstr.2020.07.016 doi: 10.1016/j.ijsolstr.2020.07.016
|
| [28] |
A. Ciallella, F. D'Annibale, D. Del Vescovo, I. Giorgio, Deformation patterns in a second-gradient lattice annular plate composed of "spira mirabilis" fibers, Continuum Mech. Thermodyn., 35 (2023), 1561–1580. https://doi.org/10.1007/s00161-022-01169-6 doi: 10.1007/s00161-022-01169-6
|
| [29] |
T. Wangler, N. Roussel, F. P. Bos, T. A. M. Salet, R. J. Flatt, Digital concrete: A review, Cem. Concr. Res., 123 (2019), 105780. https://doi.org/10.1016/j.cemconres.2019.105780 doi: 10.1016/j.cemconres.2019.105780
|
| [30] |
N. Rezaei, E. Barchiesi, D. Timofeev, C. A. Tran, A. Misra, L. Placidi, Solution of a paradox related to the rigid bar pull-out problem in standard elasticity, Mech. Res. Commun., 126 (2022), 104015. https://doi.org/10.1016/j.mechrescom.2022.104015 doi: 10.1016/j.mechrescom.2022.104015
|
| [31] |
M. F. Funari, S. Spadea, F. Fabbrocino, R. Luciano, A moving interface finite element formulation to predict dynamic edge debonding in FRP-strengthened concrete beams in service conditions, Fibers, 8 (2020), 42. https://doi.org/10.3390/fib8060042 doi: 10.3390/fib8060042
|
| [32] |
W. Pietraszkiewicz, V. A. Eremeyev, On vectorially parameterized natural strain measures of the non-linear Cosserat continuum, Int. J. Solids Struct., 46 (2009), 2477–2480. https://doi.org/10.1016/j.ijsolstr.2009.01.030 doi: 10.1016/j.ijsolstr.2009.01.030
|
| [33] |
G. La Valle, A new deformation measure for the nonlinear micropolar continuum, Z. Angew. Math. Phys., 73 (2022), 78. https://doi.org/10.1007/s00033-022-01715-x doi: 10.1007/s00033-022-01715-x
|
| [34] | I. Giorgio, A. Misra, L. Placidi, Geometrically nonlinear Cosserat elasticity with chiral effects based upon granular micromechanics, in Sixty Shades of Generalized Continua, (eds. H. Altenbach, A. Berezovski, F. Dell'Isola and A. Porubov), Springer, 170 (2023), 273–292. https://doi.org/10.1007/978-3-031-26186-2_17 |
| [35] |
I. Giorgio, M. De Angelo, E. Turco, A. Misra, A Biot–Cosserat two-dimensional elastic nonlinear model for a micromorphic medium, Continuum Mech. Thermodyn., 32 (2020), 1357–1369. https://doi.org/10.1007/s00161-019-00848-1 doi: 10.1007/s00161-019-00848-1
|
| [36] |
E. Turco, F. Dell'Isola, A. Misra, A nonlinear Lagrangian particle model for grains assemblies including grain relative rotations, Int. J. Numer. Anal. Methods Geomech., 43 (2019), 1051–1079. https://doi.org/10.1002/nag.2915 doi: 10.1002/nag.2915
|
| [37] |
E. Turco, Forecasting nonlinear vibrations of patches of granular materials by elastic interactions between spheres, Mech. Res. Commun., 122 (2022), 103879. https://doi.org/10.1016/j.mechrescom.2022.103879 doi: 10.1016/j.mechrescom.2022.103879
|
| [38] |
D. Scerrato, I. Giorgio, A. Della Corte, A. Madeo, N. E. Dowling, F. Darve, Towards the design of an enriched concrete with enhanced dissipation performances, Cem. Concr. Res., 84 (2016), 48–61. https://doi.org/10.1016/j.cemconres.2016.03.002 doi: 10.1016/j.cemconres.2016.03.002
|
| [39] |
A. Scrofani, E. Barchiesi, B. Chiaia, A. Misra, L. Placidi, Fluid diffusion related aging effect in a concrete dam modeled as a Timoshenko beam, Math. Mech. Complex Syst., 11 (2023), 313–334. https://doi.org/10.2140/memocs.2023.11.313 doi: 10.2140/memocs.2023.11.313
|
| [40] |
I. Giorgio, D. Scerrato, Multi-scale concrete model with rate-dependent internal friction, Eur. J. Environ. Civ. Eng., 21 (2017), 821–839. https://doi.org/10.1080/19648189.2016.1144539 doi: 10.1080/19648189.2016.1144539
|
| [41] |
G. Jouan, P. Kotronis, F. Collin, Using a second gradient model to simulate the behaviour of concrete structural elements, Finite Elem. Anal. Des., 90 (2014), 50–60. https://doi.org/10.1016/j.finel.2014.06.002 doi: 10.1016/j.finel.2014.06.002
|
| [42] |
P. Germain, The method of virtual power in the mechanics of continuous media, Ⅰ: Second-gradient theory, Math. Mech. Complex Syst., 8 (2020), 153–190. https://doi.org/10.2140/memocs.2020.8.153 doi: 10.2140/memocs.2020.8.153
|
| [43] |
G. La Valle, B. E. Abali, G. Falsone, C. Soize, Sensitivity of a homogeneous and isotropic second-gradient continuum model for particle-based materials with respect to uncertainties, ZAMM Z. Angew. Math. Mech., 103 (2023), e202300068. https://doi.org/10.1002/zamm.202300068 doi: 10.1002/zamm.202300068
|
| [44] |
F. Dell'Isola, S. R. Eugster, R. Fedele, P. Seppecher, Second-gradient continua: From Lagrangian to Eulerian and back, Math. Mech. Solids, 27 (2022), 2715–2750. https://doi.org/10.1177/10812865221078822 doi: 10.1177/10812865221078822
|
| [45] |
F. Dell'Isola, U. Andreaus, L. Placidi, At the origins and in the vanguard of peridynamics, non-local and higher-gradient continuum mechanics: an underestimated and still topical contribution of Gabrio Piola, Math. Mech. Solids, 20 (2015), 887–928. https://doi.org/10.1177/1081286513509811 doi: 10.1177/1081286513509811
|
| [46] |
A. Berezovski, I. Giorgio, A. D. Corte, Interfaces in micromorphic materials: Wave transmission and reflection with numerical simulations, Math. Mech. Solids, 21 (2016), 37–51. https://doi.org/10.1177/1081286515572244 doi: 10.1177/1081286515572244
|
| [47] |
M. Golaszewski, R. Grygoruk, I. Giorgio, M. Laudato, F. D. Cosmo, Metamaterials with relative displacements in their microstructure: Technological challenges in 3D printing, experiments and numerical predictions, Continuum Mech. Thermodyn., 31 (2019), 1015–1034. https://doi.org/10.1007/s00161-018-0692-0 doi: 10.1007/s00161-018-0692-0
|
| [48] |
A. Ciallella, I. Giorgio, S. R. Eugster, N. L. Rizzi, F. Dell'Isola, Generalized beam model for the analysis of wave propagation with a symmetric pattern of deformation in planar pantographic sheets, Wave Motion, 113 (2022), 102986. https://doi.org/10.1016/j.wavemoti.2022.102986 doi: 10.1016/j.wavemoti.2022.102986
|
| [49] |
O. Szlachetka, I. Giorgio, Heat conduction in multi-component step-wise FGMs, Continuum Mech. Thermodyn., (2024), 1–19. https://doi.org/10.1007/s00161-024-01296-2 doi: 10.1007/s00161-024-01296-2
|
| [50] |
V. A. Eremeyev, W. Pietraszkiewicz, Material symmetry group and constitutive equations of micropolar anisotropic elastic solids, Math. Mech. Solids, 21 (2016), 210–221. https://doi.org/10.1177/1081286515582862 doi: 10.1177/1081286515582862
|
| [51] |
G. La Valle, S. Massoumi, A new deformation measure for micropolar plates subjected to in-plane loads, Continuum Mech. Thermodyn., 34 (2022), 243–257. https://doi.org/10.1007/s00161-021-01055-7 doi: 10.1007/s00161-021-01055-7
|
| [52] |
I. Giorgio, F. Dell'Isola, A. Misrav, Chirality in 2D Cosserat media related to stretch-micro-rotation coupling with links to granular micromechanics, Int. J. Solids Struct., 202 (2020), 28–38. https://doi.org/10.1016/j.ijsolstr.2020.06.005 doi: 10.1016/j.ijsolstr.2020.06.005
|
| [53] |
I. Giorgio, F. Hild, E. Gerami, F. Dell'Isola, A. Misra, Experimental verification of 2D Cosserat chirality with stretch-micro-rotation coupling in orthotropic metamaterials with granular motif, Mech. Res. Commun., 126 (2022), 104020. https://doi.org/10.1016/j.mechrescom.2022.104020 doi: 10.1016/j.mechrescom.2022.104020
|
| [54] |
G. La Valle, C. Soize, A higher-order nonlocal elasticity continuum model for deterministic and stochastic particle-based materials, Z. Angew. Math. Phys., 75 (2024). https://doi.org/10.1007/s00033-024-02196-w doi: 10.1007/s00033-024-02196-w
|
| [55] |
A. Madeo, F. Dell'Isola, F. Darve, A continuum model for deformable, second gradient porous media partially saturated with compressible fluids, J. Mech. Phys. Solids, 61 (2013), 2196–2211. https://doi.org/10.1016/j.jmps.2013.06.009 doi: 10.1016/j.jmps.2013.06.009
|
| [56] |
F. Dell'Isola, A. Madeo, P. Seppecher, Boundary conditions at fluid-permeable interfaces in porous media: A variational approach, Int. J. Solids Struct., 46 (2009), 3150–3164. https://doi.org/10.1016/j.ijsolstr.2009.04.008 doi: 10.1016/j.ijsolstr.2009.04.008
|
| [57] |
I. Giorgio, A variational formulation for one-dimensional linear thermoviscoelasticity, Math. Mech. Complex Syst., 9 (2022), 397–412. https://doi.org/10.2140/memocs.2021.9.397 doi: 10.2140/memocs.2021.9.397
|
| [58] |
E. Barchiesi, N. Hamila, Maximum mechano-damage power release-based phase-field modeling of mass diffusion in damaging deformable solids, Z. Angew. Math. Phys., 73 (2022). https://doi.org/10.1007/s00033-021-01668-7 doi: 10.1007/s00033-021-01668-7
|
| [59] |
A. Ramírez-Torres, R. Penta, A. Grillo, Effective properties of fractional viscoelastic composites via two-scale asymptotic homogenization, Math. Methods Appl. Sci., 46 (2023), 16500–16520. https://doi.org/10.1002/mma.9457 doi: 10.1002/mma.9457
|
| [60] |
L. Placidi, E. Barchiesi, Energy approach to brittle fracture in strain-gradient modelling, Proc. R. Soc. A, 474 (2018), 20170878. https://doi.org/10.1098/rspa.2017.0878 doi: 10.1098/rspa.2017.0878
|
| [61] |
F. Fabbrocino, M. F. Funari, F. Greco, P. Lonetti, R. Luciano, R. Penna, Dynamic crack growth based on moving mesh method, Composites, Part B, 174 (2019), 107053. https://doi.org/10.1016/j.compositesb.2019.107053 doi: 10.1016/j.compositesb.2019.107053
|
| [62] |
C. Comi, R. Fedele, U. Perego, A chemo-thermo-damage model for the analysis of concrete dams affected by alkali-silica reaction, Mech. Mater., 41 (2009), 210–230. https://doi.org/10.1016/j.mechmat.2008.10.010 doi: 10.1016/j.mechmat.2008.10.010
|
| [63] | A. Bilotta, A. Morassi, E. Turco, Simple convolutional neural networks for the damage identification in composite steel-concrete beams, in International Conference on Experimental Vibration Analysis for Civil Engineering Structures, Springer, 433 (2023), 422–431. https://doi.org/10.1007/978-3-031-39117-0_43 |
| [64] |
L. Placidi, A variational approach for a nonlinear one-dimensional damage-elasto-plastic second-gradient continuum model, Continuum Mech. Thermodyn., 28 (2016), 119–137. https://doi.org/10.1007/s00161-014-0405-2 doi: 10.1007/s00161-014-0405-2
|
| [65] |
L. Placidi, E. Barchiesi, A. Misra, A strain gradient variational approach to damage: A comparison with damage gradient models and numerical results, Math. Mech. Complex Syst., 6 (2018), 77–100. https://doi.org/10.2140/memocs.2018.6.77 doi: 10.2140/memocs.2018.6.77
|
| [66] |
L. Placidi, A. Misra, E. Barchiesi, Simulation results for damage with evolving microstructure and growing strain gradient moduli, Continuum Mech. Thermodyn., 31 (2019), 1143–1163. https://doi.org/10.1007/s00161-018-0693-z doi: 10.1007/s00161-018-0693-z
|
| [67] |
R. Luciano, A. Caporale, H. Darban, C. Bartolomeo, Variational approaches for bending and buckling of non-local stress-driven Timoshenko nano-beams for smart materials, Mech. Res. Commun., 103 (2020), 103470. https://doi.org/10.1016/j.mechrescom.2019.103470 doi: 10.1016/j.mechrescom.2019.103470
|
| [68] | Ciallella, A and Pasquali, D and Gołaszewski, M and D'Annibale, F and Giorgio, I, A rate-independent internal friction to describe the hysteretic behavior of pantographic structures under cyclic loads, Mech. Res. Commun., 116 (2021), 103761. |
| [69] |
A. Ciallella, D. Pasquali, M. Gołaszewski, F. D'Annibale, I. Giorgio, Shear rupture mechanism and dissipation phenomena in bias-extension test of pantographic sheets: Numerical modeling and experiments, Math. Mech. Solids, 27 (2022), 2170–2188. https://doi.org/10.1016/j.mechrescom.2021.103761 doi: 10.1016/j.mechrescom.2021.103761
|
| [70] |
I. Giorgio, L. Placidi, A variational formulation for three-dimensional linear thermoelasticity with 'thermal inertia', Meccanica, (2024). https://doi.org/10.1007/s11012-024-01796-0 doi: 10.1007/s11012-024-01796-0
|
| [71] |
A. Battista, L. Rosa, R. Dell'Erba, L. Greco, Numerical investigation of a particle system compared with first and second gradient continua: Deformation and fracture phenomena, Math. Mech. Solids, 22 (2017), 2120–2134. https://doi.org/10.1177/1081286516657889 doi: 10.1177/1081286516657889
|
| [72] |
D. Scerrato, I. Giorgio, A. Madeo, A. Limam, F. Darve, A simple non-linear model for internal friction in modified concrete, Int. J. Eng. Sci., 80 (2014), 136–152. https://doi.org/10.1016/j.ijengsci.2014.02.021 doi: 10.1016/j.ijengsci.2014.02.021
|
| [73] |
D. Scerrato, I. Giorgio, A. Della Corte, A. Madeo, A. Limam, A micro-structural model for dissipation phenomena in the concrete, Int. J. Numer. Anal. Methods Geomech., 39 (2015), 2037–2052. https://doi.org/10.1002/nag.2394 doi: 10.1002/nag.2394
|
| [74] | I. Giorgio, M. Spagnuolo, L. Greco, F. D'Annibale, A. Cazzani, A variational approach to address the problem of planar nonlinear beams, in Comprehensive Mechanics of Materials, (eds. V. Silberschmidt), Elsevier, 1 (2024), 67–97. https://doi.org/10.1016/B978-0-323-90646-3.00027-7 |
| [75] |
V. Pensée, D. Kondo, L. Dormieux, Micromechanical analysis of anisotropic damage in brittle materials, J. Eng. Mech., 128 (2002), 889–897. https://doi.org/10.1061/(ASCE)0733-9399(2002)128:8(889) doi: 10.1061/(ASCE)0733-9399(2002)128:8(889)
|
| [76] | B. R. Raveendra, G. S. Benipal, A. K. Singh, Constitutive modelling of concrete: An overview, Asian J. Civ. Eng., 6 (2005), 211–214. |
| [77] |
A. M. Bersani, P. Caressa, F. Dell'Isola, Approximation of dissipative systems by elastic chains: Numerical evidence, Math. Mech. Solids, 28 (2023), 501–520. https://doi.org/10.1177/10812865221081851 doi: 10.1177/10812865221081851
|
| [78] |
A. M. Bersani, P. Caressa, A. Ciallella, Numerical evidence for the approximation of dissipative systems by gyroscopically coupled oscillator chains, Math. Mech. Complex Syst., 10 (2022), 265–278. https://doi.org/10.2140/memocs.2022.10.265 doi: 10.2140/memocs.2022.10.265
|
| [79] |
A. Ciallella, D. Scerrato, M. Spagnuolo, I. Giorgio, A continuum model based on Rayleigh dissipation functions to describe a Coulomb-type constitutive law for internal friction in woven fabrics, Z. Angew. Math. Phys., 73 (2022). https://doi.org/10.1007/s00033-022-01845-2 doi: 10.1007/s00033-022-01845-2
|
| [80] |
L. Greco, M. Cuomo, L. Contrafatto, Two new triangular G1-conforming finite elements with cubic edge rotation for the analysis of Kirchhoff plates, Comput. Methods Appl. Mech. Eng., 356 (2019), 354–386. https://doi.org/10.1016/j.cma.2019.07.026 doi: 10.1016/j.cma.2019.07.026
|
| [81] |
L. Greco, D. Castello, M. Cuomo, An objective and accurate G1-conforming mixed Bézier FE-formulation for Kirchhoff–Love rods, Math. Mech. Solids, 29 (2024), 645–685. https://doi.org/10.1177/10812865231204972 doi: 10.1177/10812865231204972
|
| [82] |
I. Giorgio, A discrete formulation of Kirchhoff rods in large-motion dynamics, Math. Mech. Solids, 25 (2020), 1081–1100. https://doi.org/10.1177/1081286519900902 doi: 10.1177/1081286519900902
|
| [83] |
I. Giorgio, D. Del Vescovo, Energy-based trajectory tracking and vibration control for multilink highly flexible manipulators, Math. Mecha. Complex Syst., 7 (2019), 159–174. https://doi.org/10.2140/memocs.2019.7.159 doi: 10.2140/memocs.2019.7.159
|
| [84] |
D. Baroudi, I. Giorgio, A. Battista, E. Turco, L. A. Igumnov, Nonlinear dynamics of uniformly loaded elastica: Experimental and numerical evidence of motion around curled stable equilibrium configurations, ZAMM Z. Angew. Math. Mech., 99 (2019), e201800121. https://doi.org/10.1002/zamm.201800121 doi: 10.1002/zamm.201800121
|
| [85] |
E. Turco, Discrete is it enough? The revival of Piola–Hencky keynotes to analyze three-dimensional Elastica, Continuum Mech. Thermodyn., 30 (2018), 1039–1057. https://doi.org/10.1007/s00161-018-0656-4 doi: 10.1007/s00161-018-0656-4
|
| [86] |
R. Fedele, G. Maier, B. Miller, Identification of elastic stiffness and local stresses in concrete dams by in situ tests and neural networks, Struct. Infrastruct. Eng., 1 (2005), 165–180. https://doi.org/10.1080/15732470500030513 doi: 10.1080/15732470500030513
|
| [87] | R. Fedele, G. Maier, B. Miller, Image correlation-based identification of fracture parameters for structural adhesives, Tech. Mech. Eur. J. Eng. Mech., 32 (2012), 195–204. |
| [88] |
B. E. Abali, C. C. Wu, W. H. Müller, An energy-based method to determine material constants in nonlinear rheology with applications, Continuum Mech. Thermodyn., 28 (2016), 1221–1246. https://doi.org/10.1007/s00161-015-0472-z doi: 10.1007/s00161-015-0472-z
|
| [89] |
M. De Angelo, L. Placidi, N. Nejadsadeghi, A. Misra, Non-standard Timoshenko beam model for chiral metamaterial: Identification of stiffness parameters, Mech. Res. Commun., 103 (2020), 103462. https://doi.org/10.1016/j.mechrescom.2019.103462 doi: 10.1016/j.mechrescom.2019.103462
|
| [90] |
A. Ciallella, G. La Valle, A. Vintache, B. Smaniotto, F. Hild, Deformation mode in 3-point flexure on pantographic block, Int. J. Solids Struct., 265 (2023), 112129. https://doi.org/10.1016/j.ijsolstr.2023.112129 doi: 10.1016/j.ijsolstr.2023.112129
|
| [91] |
M. De Angelo, E. Barchiesi, I. Giorgio, B. E. Abali, Numerical identification of constitutive parameters in reduced-order bi-dimensional models for pantographic structures: Application to out-of-plane buckling, Arch. Appl. Mech., 89 (2019), 1333–1358. https://doi.org/10.1007/s00419-018-01506-9 doi: 10.1007/s00419-018-01506-9
|
| [92] |
N. Shekarchizadeh, B. E. Abali, E. Barchiesi, A. M. Bersani, Inverse analysis of metamaterials and parameter determination by means of an automatized optimization problem, ZAMM Z. Angew. Math. Mech., 101 (2021), e202000277. https://doi.org/10.1002/zamm.202000277 doi: 10.1002/zamm.202000277
|
| [93] |
I. Giorgio, P. Harrison, F. Dell'Isola, J. Alsayednoor, E. Turco, Wrinkling in engineering fabrics: A comparison between two different comprehensive modelling approaches, Proc. R. Soc. A, 474 (2018), 20180063. https://doi.org/10.1098/rspa.2018.0063 doi: 10.1098/rspa.2018.0063
|
| [94] |
R. Fedele, A. Ciani, L. Galantucci, V. Casalegno, A. Ventrella, M. Ferraris, Characterization of innovative CFC/Cu joints by full-field measurements and finite elements, Mater. Sci. Eng. A, 595 (2014), 306–317. https://doi.org/10.1016/j.msea.2013.12.015 doi: 10.1016/j.msea.2013.12.015
|
| [95] |
N. Cefis, R. Fedele, M. G. Beghi, An integrated methodology to estimate the effective elastic parameters of amorphous TiO2 nanostructured films, combining SEM images, finite element simulations and homogenization techniques, Mech. Res. Commun., 131 (2023), 104153. https://doi.org/10.1016/j.mechrescom.2023.104153 doi: 10.1016/j.mechrescom.2023.104153
|
Figures(10) / Tables(2)
Giuliano Aretusi, Christian Cardillo, Larry Murcia Terranova, Ewa Bednarczyk. A dissipation model for concrete based on an enhanced Timoshenko beam[J]. Networks and Heterogeneous Media, 2024, 19(2): 700-723. doi: 10.3934/nhm.2024031
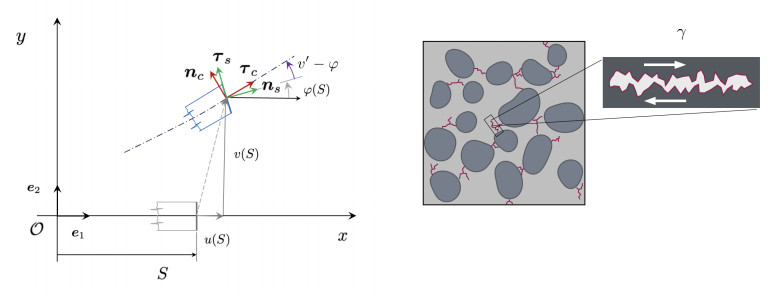
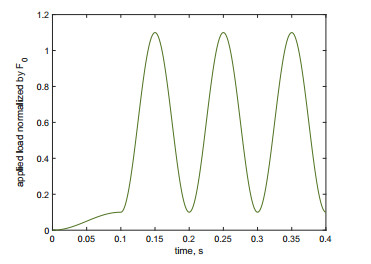
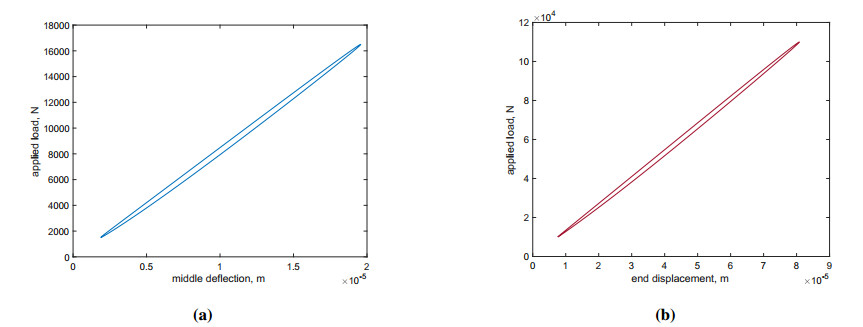
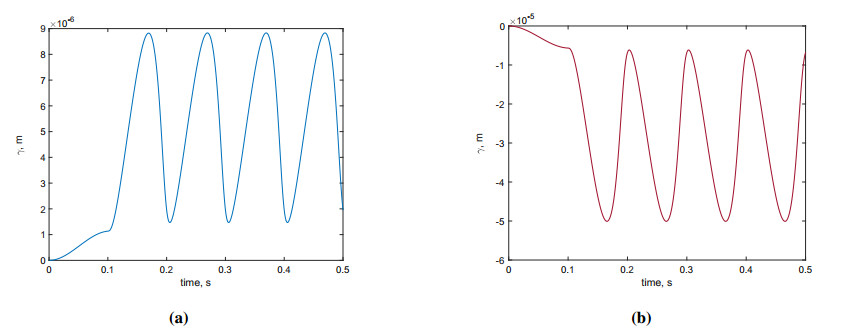
DownLoad: