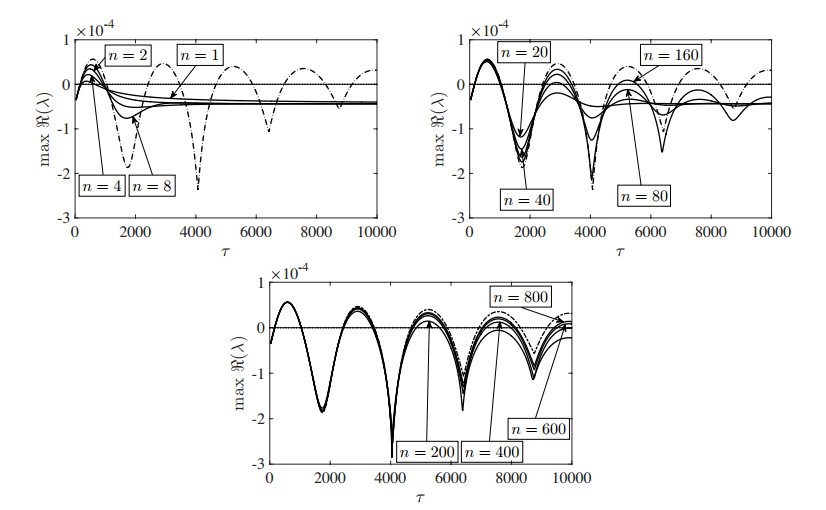
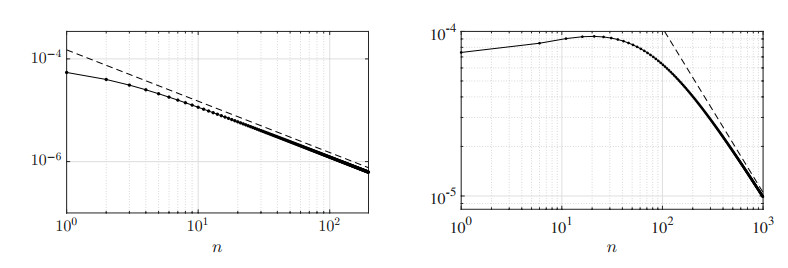
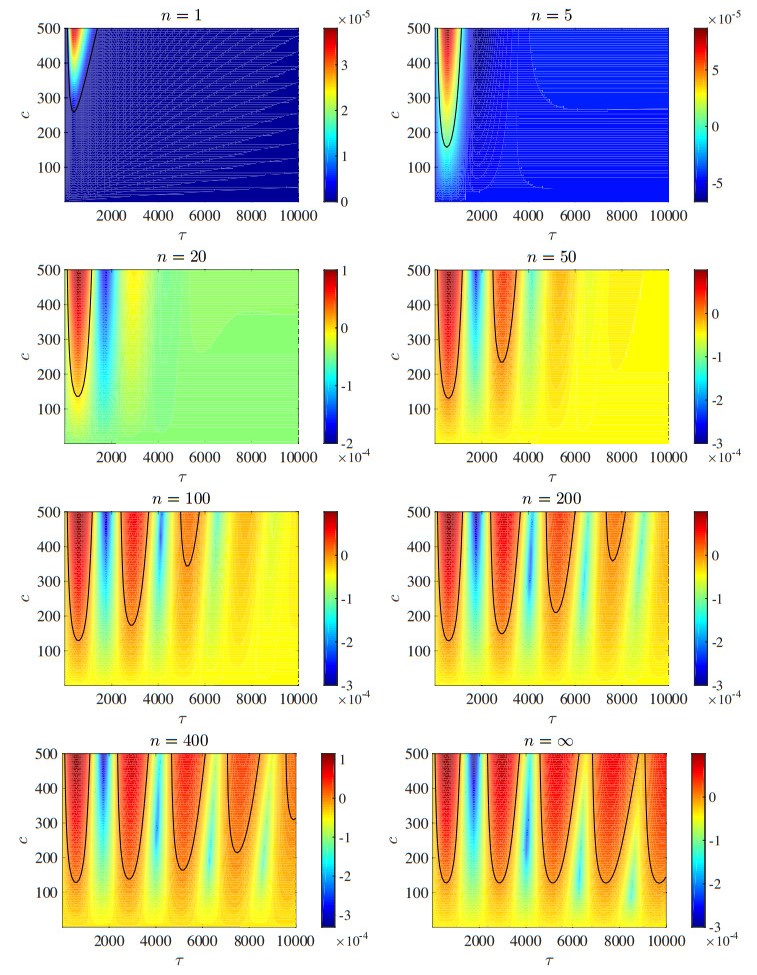
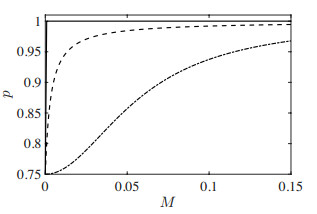
A prototype SIR model with vaccination at birth is analyzed in terms of the stability of its endemic equilibrium. The information available on the disease influences the parentso decision on whether vaccinate or not. This information is modeled with a delay according to the Erlang distribution. The latter includes the degenerate case of fading memory as well as the limiting case of concentrated memory. The linear chain trick is the essential tool used to investigate the general case. Besides its novel analysis and that of the concentrated case, it is showed that through the linear chain trick a distributed delay approaches a discrete delay at a linear rate. A rigorous proof is given in terms of the eigenvalues of the associated linearized problems and extension to general models is also provided. The work is completed with several computations and relevant experimental results.
1.
Introduction
In 1987, Karevia and Odell [1] first proposed the following one-predator and one-prey model with prey-taxis in order to explain that an area-restricted search creates the following predator aggregation phenomenon
where D>0 is the diffusivity coefficient of preys, d(w) denotes the motility function of predators, χ(w) represents the prey-taxis sensitivity coefficient, and the term −∇⋅(uχ(w)∇w) stands for the tendency of the predator moving towards the increasing direction of the prey gradient, and it is viewed as the prey-taxis term. The functions G1(u,w) and G2(u,w) describe the predator-prey interactions, which include both intra-specific and inter-specific interactions. Generally the predator-prey interaction functions G1(u,w) and G2(u,w) possess the following prototypical forms.
where γ>0 denotes the intrinsic predation rate, uF(w) represents the inter-specific interaction and uh(u) and f(w) stand for the intra-specific interaction. Specifically, F(w) is the functional response function accounting for the intake rate of predators as a function of prey density; it is often used in the following form in the literature [2,3,4]
with constants λ>0 and m>1; other types of functional response functions (e.g., Beddington-DeAngelis type in [5], Crowley-Martin type in [6]) and more predator-prey interactions can be found in [7,8,9,10]. The predator mortality rate function h(u) is typically of the form
where θ>0 accounts for the natural death rate and α≥0 denotes the rate of death resulting from the intra-specific competition, which is also called the density-dependent death [11]. The prey growth function f(w) is usually assumed to be negative for large w due to the limitation of resources (or crowding effect), and its typical forms are
where μ>0 is the intrinsic growth rate of prey, K>0 is called the carrying capacity and 0<k<K. Now there exist many interesting results about global existence, uniform boundedness, asymptotic behavior, traveling waves and pattern formation of solutions to System (1.1) or its variants in [11,12,13,14,15]. When d(w)=d>0 and χ(w)=χ>0, G1(u,v) and G2(u,v) have the forms of (1.2), Wu et al. [15] obtained the global existence and uniform persistence of solutions to (1.1) in any dimension provided that χ is suitably small. Then, Jin and Wang[13] derived the global boundedness and asymptotic stability of solutions for System (1.1) without the smallness assumption on χ in a two-dimensional bounded domain. Moreover, Wang et al. [16] studied the nonconstant positive steady states and pattern formation of (1.1) in a one-dimensional bounded domain. Under the conditions that d(w) and χ(w) are not constants and h(u) is given by (1.4), Jin and Wang[14] established the global boundedness, asymptotic behavior and spatio-temporal patterns of solutions for (1.1) under some conditions on the parameters in a two-dimensional smooth bounded domain. For more related results in predator-prey models, we refer the readers to [17,18,19,20,21,22,23,24,25,26,27,28,29] and the references therein.
However, all the aforementioned works are devoted to studying prey-taxis models with one-predator and one prey. Now let us mention some predator-prey models with two-predator and one-prey. Recently, the following general two-predator and one-prey model with prey-taxis has attracted a lot of attention.
as applied in a smooth bounded domain Ω⊂Rn, n≥1. Given d1(w)=d2(w)=1 and β1=β2=0, Wang et al. [30] derived the global boundedness, nonconstant positive steady states and time-periodic patterns of solutions for System (1.6). Wang and Wang [31] studied the uniform boundedness and asymptotic stability of nonnegative spatially homogeneous equilibria for (1.6) in any dimension. Given d1(w)=d2(w)=1, χi(w)=χi>0(i=1,2) and β1=β2=β>0, Mi et al. [32] obtained the global boundedness and stability of classical solutions in any dimension under suitable conditions of parameters. Under the conditions that d1(w) and d2(w) are non-constants and β1=β2=0, Qiu et al.[33] rigorously proved the global existence, uniform boundedness and stabilization of classical solutions in any dimension with suitable conditions on motility functions and the coefficients of the logistic source. However, when β1,β2≠0, the global existence and stabilization of solutions for (1.6) are still open. Given χi(w)=−d′i(w)≥0 if d′i(w)≤0(i=1,2), the diffusion-advection terms in (1.6) can respectively become the forms Δ(d1(w)u) and Δ(d2(w)v), which could be interpreted as "density-suppressed motility" in [34,35]. This means that the predator will reduce its motility when encountering the prey, which is a rather reasonable assumption that has very sound applications in the predator-prey systems. Since the possible degeneracy caused by the density-suppressed motility brings considerable challenges for mathematical analysis, many works have showed various interesting results, which can be found in [36,37,38,39,40,41,42,43,44]. Given χi(w)=−d′i(w)≥0(i=1,2), F1(w)=F2(w)=w, h1(u)=u,h2(v)=v and f(w)=μw(m(x)−w), System (1.6) can be simplified as
where the parameters D,μ,γi,βi(i=1,2) are positive, the dispersal rate functions di(w)(i=1,2) satisfy following the hypothesis: di(w)∈C2([0,∞)),d′i(w)≤0 on [0,∞) and di(w)>0. Wang and Xu [45] have found some interesting results for System (1.7) in a two-dimensional smooth bounded domain. More specifically, when D=1 and m(x)=1, System (1.7) has a unique globally bounded classical solution. By constructing appropriate Lyapunov functionals and using LaSalle's invariant principle, the authors proved that the global bounded solution of (1.7) converges to the co-existence steady state exponentially or competitive exclusion steady state algebraically as time tends to infinity in different parameter regimes. For a prey's resource that is spatially heterogeneous (i.e., m(x) is non-constant), the authors used numerical simulations to demonstrate that the striking phenomenon "slower diffuser always prevails" given in [46,47] fails to appear if the non-random dispersal strategy is employed by competing species (i.e., either d1(w) or d2(w) is non-constant) while it still holds if both d1(w) and d2(w) are constants. However, there are few results about global boundedness and large time behavior of solutions for (1.7) in the general form.
Inspired by the above works, this paper is concerned with the following two-species competitive predator-prey system with the following density-dependent diffusion
where Ω⊂R2 is a bounded domain with a smooth boundary ∂Ω, ∂∂ν denotes the derivative with respect to the outward normal vector ν of ∂Ω, and the parameters D and γi, βi (i=1,2) are positive. The unknown functions u=u(x,t) and v=v(x,t) denote the densities of two-competing species (e.g., predators), and w=w(x,t) represents the density of predators' resources (e.g., the prey) at a position x and time t>0. When d1(w)=d1>0 and d2(w)=d2>0, System (1.8) becomes the well-known diffusive predator-prey system, which has been widely studied in [48,49,50,51]. However, to the best of our knowledge, the results of the two-predator and one-prey system given by (1.8) with density-suppressed motility (i.e., d1(w) and d2(w) are non-constants) indicate that the competition and general predator mortality rate hi(u) are almost vacant. The main aim of this paper is to explore the influence of the predation interaction, competition and general predator mortality on the dynamical behavior of System (1.8). Throughout this paper, we assume that the functions di(s),Fi(s),hi(s),(i=1,2),f(s) and initial data (u0,v0,w0) mentioned in (1.8) satisfy the following hypotheses:
(H1) di(s)∈C2([0,∞)) with di(s)>0 and d′i(s)≤0, i=1,2 on [0,∞). The typical example is di(s)=1(1+s)κi or di(s)=exp(−κis) with κi>0, i=1,2.
(H2) Fi(s)∈C1([0,∞)), Fi(0)=0, Fi(s)>0 and F′i(s)>0, i=1,2 in (0,∞).
(H3) hi(s)∈C2([0,∞)) and there exist constants θi>0 and αi≥0 such that hi(s)≥θi and h′i(s)≥αi, i=1,2 for all s>0.
(H4) f(s)∈C1([0,∞)) with f(0)=0, and there exist positive constants μ and K such that f(s)≤μs for all s≥0, f(K)=0 and f(s)<0 for s>K.
(H5) (u0,v0,w0)∈(W1,p(Ω))3 with p>2 and u0,v0,w0≥0.
Here, we note that there are many candidates for the above functions Fi(s), hi(s) and f(s) as in (1.3)–(1.5). Due to the presence of the prey's density dependent diffusion coefficient, Model (1.8) is a cross-diffusion system and the parabolic comparison principle is no longer applicable. Moreover, when α1=α2=0, the key L2-spatiotemporal estimates of u and v cannot be directly derived; thus, the uniform boundedness of solutions is not an obvious result and needs to be justified. Based on the above hypotheses, the first main result of this paper asserts the global existence and boundedness of solutions for System (1.8) as follows.
Theorem 1.1. Let D,γi,βi>0(i=1,2), Ω⊂R2 be a smooth bounded domain and the hypotheses (H1)–(H5) hold. Then System (1.8) has a unique global nonnegative classical solution (u,v,w)∈(C(¯Ω×[0,∞))∩C2,1(¯Ω×(0,∞)))3, which is uniformly bounded in time, i.e., there exists a constant C>0 independent of t such that
In particular, one has 0≤w(x,t)≤K0 for all (x,t)∈Ω×(0,∞), where
Remark 1.1. For the special case F1(w)=F2(w)=w, h1(u)=u,h2(v)=v and f(w)=μw(1−w), the results of Theorem 1.1 have been obtained in [45]. However, since α1 and α2 may be equal to zero in the hypotheses of this paper, the L2-spatiotemporal estimates of u and v cannot be directly obtained by using the method in [45]. By means of the mechanism "density-suppressed motility", we invoke some ideas used in [14] and apply the self-adjoint realization of Δ+δ with some δ>0 in L2(Ω) to establish the key L2-spatiotemporal estimates of u and v.
The second main aim of this paper is to study the role of non-random dispersal and competition between two predators in the asymptotic properties of the nonnegative spatial homogeneous equilibria of System (1.8). For simplicity, we let F1(w)=F2(w)=w, h1(u)=θ1+α1u, h2(v)=θ2+α2v and f(w)=μw(1−w), where θ1=θ2=θ>0 and α1,α2,μ>0; then, System system (1.8) can be simplified as
Theorem 1.1 ensures that System (1.11) possesses a unique global bounded nonnegative classical solution (u,v,w) such that 0≤w(x,t)≤K0:=max{||w0||L∞(Ω),1} for all (x,t)∈Ω×(0,∞). Now we find some sufficient conditions of parameters so that (1.11) admits a positive constant solution (u∗,v∗,w∗) satisfying
i.e.,
where
If the determinant Φ of the coefficient matrix A in (1.13) does not equal zero, it follows from Cramer's rule that
where
When β1<α1 and β2<α2, it follows that Φ>0 and Φw>0, and thus we know w∗>0. Next, we shall discuss the sign of Φu and Φv. For convenience, we let
and
where
and
It is not difficult to see that u∗=ΦuΦ, v∗=ΦvΦ and w∗=ΦwΦ are positive, if γi>θ, i=1,2 and one of the following conditions holds:
(H6) γ1<γ2, l1>0, β1<min{α1,β∗1} and β2<min{α2,β∗2};
(H7) γ1>γ2, l2>0, β1<min{α1,β∗1} and β2<min{α2,β∗2};
(H8) γ1=γ2 and max{β1,β2}<min{α1,α2}.
Now, we give our main results on the asymptotic stability properties of the nonnegative spatial homogeneous equilibria of System (1.11) as follows.
Theorem 1.2. Let Ω⊂R2 be a smooth bounded domain and the parameters γi,αi,βi, (i=1,2), θ, μ and D be positive. Assume that d1(w) and d2(w) satisfy (H1), and that (u,v,w) is a global bounded classical solution of System (1.11). Suppose that γi>θ,i=1,2,
and
as well as one of the conditions (H6)–(H8) holds. Then for all initial data (u0,v0,w0) satisfying (H5), there exist positive constants C and λ such that
for all t>0, where (u∗,v∗,w∗) is given by (1.14).
Remark 1.2. From a biological point of view, it is well known that the change of the predators comes from predation, competition and mortality in System (1.11). The parameters γi, βi, αi(i=1,2) and θ respectively stand for the predation rate, competition strength, density-dependent death and natural death rate of the predators, which play a collective role in studying the dynamical behavior of (1.11). More specifically, when γi>θ, it is called strong predation; otherwise, it is weak predation. Hence the results of Theorem 1.2 can tell us that if the predations of two predators are strong and the prey diffusion coefficient D is suitably large, all species can reach a coexistence state.
Theorem 1.3. Let Ω⊂R2 be a smooth bounded domain and the parameters γi,αi,βi, (i=1,2), θ, μ and D be positive. Assume that d1(w) and d2(w) satisfy (H1), and the (u,v,w) is a global bounded classical solution of System (1.11). Suppose that we have (1.20) and
as well as one of the following conditions holds:
(i) γ1>γ2>θ, l2≤0, β1<min{α1,β∗1} and β2<α2;
(ii) γ1>γ2>θ, l2>0, β1<min{α1,β∗1} and β2∈[β∗2,α2);
(iii) γ1>θ≥γ2,
where
Then for all initial data (u0,v0,w0) satisfying (H5), one has
exponentially if θ>γ2¯w−β2¯u or algebraically if θ=γ2¯w−β2¯u.
Theorem 1.4. Let Ω⊂R2 be a smooth bounded domain and the parameters γi,αi,βi, (i=1,2), θ, μ and D be positive. Assume that d1(w) and d2(w) satisfy (H1), and the (u,v,w) is a global bounded solution of System (1.11). Suppose that we have (1.20) and
as well as one of the following conditions holds:
(i) γ2>γ1>θ, l1≤0, β2<min{α2,β∗2} and β1<α1;
(ii) γ2>γ1>θ, l1>0, β2<min{α2,β∗2} and β1∈[β∗1,α1);
(iii) γ2>θ≥γ1,
where
Then for all initial data (u0,v0,w0) satisfying (H5), one has
exponentially if θ>γ1˜w−β1˜v or algebraically if θ=γ1˜w−β1˜v.
Remark 1.3. It follows from Theorem 1.3 that if the predator u is superior over v in the competition and the prey diffusion coefficient D is suitably large, the semi-trivial equilibrium (¯u,0,¯w) is globally asymptotically stable. Similarly, we can obtain Theorem 1.4. Hence, we only give the conclusion of Theorem 1.4, without showing the details of the proof for brevity.
Theorem 1.5. Let Ω⊂R2 be a smooth bounded domain and the parameters γi,αi,βi, (i=1,2), θ, μ and D be positive. Assume that d1(w) and d2(w) satisfy (H1), and the (u,v,w) is a global bounded solution of System (1.11). Suppose that
Then for all initial data (u0,v0,w0) satisfying (H5), one has
exponentially if γi<θ, i=1,2 or algebraically if γi=θ, i=1,2.
Remark 1.4. It follows from Theorem 1.5 that if the capture rates of the two predators are low (i.e. γi≤θ, i=1,2), the prey-only steady state (0,0,1) is globally asymptotically stable regardless of the size of the prey diffusion coefficient D.
Remark 1.5. Compared with the previous results of [33] without competitive terms, the results of Theorems 1.2–1.5 indicate that the competition terms play a crucial role in the global stability of the constant steady states in (1.11). Moreover, under the condition of density-suppressed motility, our global stability results of Theorems 1.2–1.5 can also generalize the ranges of parameters αi and βi(i=1,2) for two dimensional cases in [32]. However, since the heat-semigroup estimates of u and v are no longer applicable due to the appearance of density-suppressed motility, the global stability in L∞-norm is still open in the higher-dimensional problem.
The rest of this paper is organized as follows. In Section 2, we first state the local existence of the classical solution to (1.8) and collect preliminary lemmas. In Section 3, we derive the global existence and boundedness of classical solutions for (1.8) and prove Theorem 1.1. Finally, we shall study the asymptotic stability of global bounded solutions for (1.11) and prove Theorems 1.2–1.5.
2.
Local existence and preliminaries
In this section, we shall give the local existence and some preliminary lemmas. Firstly, we state the local existence of the classical solution to (1.8), as obtained by means of the abstract theory of quasilinear parabolic systems in [52].
Lemma 2.1. Let D,γi,βi>0(i=1,2), Ω⊂R2 be a smooth bounded domain and the hypotheses (H1)–(H5) hold. Then, there exists a Tmax∈(0,∞] such that System (1.8) possesses a unique classical solution (u,v,w)∈(C(¯Ω×[0,Tmax))∩C2,1(¯Ω×(0,Tmax)))3 satisfying
In addition, the following extensibility criterion holds, i.e. if Tmax<∞, then
Proof. Let z=(u,v,w)T; then, System (1.8) can be rewritten as
where
According to the conditions that D>0 and di(w)>0(i=1,2), the matrix P(z) is positively definite for the given initial data z0, which asserts that System (1.8) is normally parabolic. Thus it follows from Theorem 7.3 of [53] that there exists a Tmax∈(0,∞] such that System (1.8) admits a unique classical solution (u,v,w)∈(C0(¯Ω×[0,Tmax))∩C2,1(¯Ω×(0,Tmax)))3. The nonnegativity of (u,v,w) directly comes from the maximum principle [14,45]. It similarly follows from Lemma 2.2 of [13] that w≤K0:=max{||w0||L∞(Ω),K}. Since P(z) is an upper triangular matrix, we can deduce from Theorem 5.2 of [54] that the extensibility criterion given by (2.2) holds. The proof of Lemma 2.1 is complete.
Lemma 2.2. Let Ω⊂Rn(n≥1) be a smooth bounded domain, D>0 and T∈(0,∞]. Assume that φ(x,t)∈C(¯Ω×[0,T))∩C2,1(¯Ω×(0,T)) satisfies
where ψ∈L∞((0,T);Lp(Ω)) with p≥1. Then there exists a positive constant C such that
where
Proof. This lemma directly comes from Lemma 1 of [55].
Now, we give the following lemma ([56], Lemma 3.4) to derive some a priori estimates for w.
Lemma 2.3. Let T>0,τ∈(0,T) and a,d>0, and assume that y:[0,T)→[0,∞) is absolutely continuous. If there exists a nonnegative function h∈L1loc([0,T)) satisfying
and
one has
Next, we give a basic property of the solution components u and v for System (1.8).
Lemma 2.4. Let the assumptions of Lemma 2.1 hold. Then there exists a constant C>0 such that
and
where τ=min{1,12Tmax}.
Proof. It follows from a direct computation for System (1.8) that
for all t\in (0, T_{\max}) , where we have applied ( H_{3} ), ( H_{4} ), \beta_{1}, \beta_{2} > 0 and (2.1).
Let \theta: = \min\{\theta_{1}, \theta_{2}\} , it follows from (2.1) that
which leads to (2.11) by Gronwall's inequality. If \alpha_{i} > 0 , i = 1, 2 , then integrating (2.14) over (t, t+\tau) , we have (2.12) directly. If \alpha_{i} = 0 , i = 1, 2 , we can also prove (2.12) by means of the idea used in [14]. For the readers' convenience, we give the sketch of the proof.
Let \mathcal{A} denote the self-adjoint realization of -\Delta+\delta under homogeneous Neumann boundary conditions in L^{2}(\Omega) , where \delta\in\left(0, \min\{\frac{\theta_{1}}{d_{1}(0)}, \frac{\theta_{2}}{d_{2}(0)}\}\right) . It follows from \delta > 0 that \mathcal{A} has an order-preserving bounded inverse \mathcal{A}^{-1} on L^{2}(\Omega) . Thus this allows us to obtain a positive constant c_{1} such that
and
By a simple calculation in (1.8), we have
which can be written as
where we have applied ( H_{1} ), ( H_{3} ), ( H_{4} ), (2.1) and \delta\in\left(0, \min\{\frac{\theta_{1}}{d_{1}(0)}, \frac{\theta_{2}}{d_{2}(0)}\}\right) . Hence, by multiplying (2.18) by \mathcal{A}^{-1}\left(\frac{1}{\gamma_{1}}u+\frac{1}{\gamma_{2}}v+w\right)\geq0 and integrating it over \Omega , we derive
According to the fact that 0 < d_{i}(K_{0})\leq d_{i}(w) , i = 1, 2 , due to ( H_{1} ) and (2.1), and by letting c_{3}: = \min\{d_{1}(K_{0}), d_{2}(K_{0}), D\} > 0 , we deduce
It follows from Hölder's and Young's inequality as well as (2.15) that
According to (2.16), we have
By combining (2.20)–(2.22), we derive
By the ordinary differential equations (ODE) argument, there exists a c_{4} > 0 such that
which implies that
The proof of Lemma 2.4 is complete.
Finally, we shall give the following key estimate of w , which plays a crucial role in the proof of Theorem 1.1.
Lemma 2.5. Let the assumptions of Lemma 2.1 hold. Then there exists a constant C > 0 such that
and
where \tau = \min\{1, \frac{1}{2}T_{\max}\} .
Proof. Multiplying the third equation of System (1.8) with -2\Delta w and integrating it by parts, we deduce from Young's inequality and (2.1) that
where we have used the hypotheses ( H_{2} ) and ( H_{4} ).
It follows from \frac{\partial w}{\partial\nu} = 0 , Young's inequality and (2.1) that
Let c_{5}: = \max\left\{\frac{4F_{1}^{2}(K_{0})}{D}, \frac{4F_{2}^{2}(K_{0})}{D}\right\} ; we infer from (2.28) and (2.29) that
where c_{6}: = \frac{2(\mu K_{0})^{2}}{D}|\Omega|+\frac{K_{0}^{2}}{2D}|\Omega| . It follows from Lemma 2.3 and Lemma 2.4 that (2.26) holds. Then integrating (2.30) over (t, t+\tau) , we can deduce from (2.12) and (2.26) that (2.27) holds.
3.
Global boundedness of solutions
In this section, we shall study the global existence and uniform boundedness of solutions for system (1.8) when n = 2 . To do this, we need the following lemmas.
Lemma 3.1. Let the conditions of Theorem 1.1 hold. Then the solution (u, v, w) of system (1.8) satisfies
and
for all q\in[1, \infty) and t\in(0, T_{\max}) , where C > 0 is a constant independent of t .
Proof. Multiplying the first equation of System (1.8) by 2u and integrating by parts, we deduce from Young's and Hölder's inequalities that
where \mathcal{K}_{1}: = \max_{w\in[0, K_{0}]}\frac{|d'_{1}(w)|^{2}}{d_{1}(w)} and we have applied ( H_{1} )–( H_{3} ) and (2.1).
By using the Gagliardo-Nirenberg inequality in two dimensions, there exists a C_{1} > 0 such that
According to Lemma 2.5 of [19] when n = 2 , it follows from Lemma 2.5 that
for all t\in(0, T_{\max}) , where C_{2}, C_{3} > 0 . Thus, by combining (3.4) with (3.5), we infer from Young's inequality that
where C_{4}, C_{5} are positive constants. Thus it follows from (3.3) and (3.6) that
where C_{6}: = \max\{C_{4}, C_{5}+2\gamma_{1}F_{1}(K_{0})\} .
By Lemma 2.4, we can find t_{0} = t_{0}(t)\in((t-\tau)_{+}, t) for any t\in(0, T_{\max}) such that there exists a C_{7} > 0 satisfying
where \tau is defined in Lemma 2.4. It follows from Lemma 2.5 that there exists a C_{8} > 0 such that
Therefore, integrating (3.7) over (t_{0}, t) , we deduce from t_{0} < t < t_{0}+\tau\leq t_{0}+1 , (3.8) and (3.9) that
for all t\in(0, T_{\max}) .
Similarly, we obtain
It follows from the third equation of System (1.8), we know that w solves the following problem
where g(u, v, w) = w-uF_{1}(w)-vF_{2}(w)+f(w) . According to ( H_{2} ), ( H_{3} ) and (2.1), we infer from (3.10) and (3.11) that
for all t\in(0, T_{\max}) . Hence, it follows from Lemma 2.2 in two dimensions that (3.2) holds. The proof of Lemma 3.1 is complete.
Next, we shall prove the boundedness of w in W^{1\infty}(\Omega) .
Lemma 3.2. Let the conditions of Theorem 1.1 hold. Then the solution component w of system (1.8) satisfies
for all t\in(0, T_{\max}) , where C > 0 is a constant independent of t .
Proof. Multiplying the first equation of System (1.8) by u^{2} and integrating by parts, we deduce from Young's and Hölder's inequalities that
for all t\in(0, T_{\max}) , where \mathcal{K}_{1} is defined in the proof of Lemma 3.1 and we have applied ( H_{1} )–( H_{3} ) and (2.1).
It follows from Lemma 3.1 that there exist positive constants C_{1} and C_{2} such that ||\nabla w||_{L^{4}(\Omega)}\leq C_{1} and ||u||_{L^{2}(\Omega)}\leq C_{2} for all t\in(0, T_{\max}) . Then by using the Gagliardo-Nirenberg inequality and Young's inequality, we can find positive constants C_{i} , i = 3, \cdot\cdot\cdot, 6 such that
and
for all t\in(0, T_{\max}) .
Combining (3.15)–(3.17), we have
for all t\in(0, T_{\max}) . By the ODE argument, we can derive
Similarly, we also derive the boundedness of ||v||_{L^{3}(\Omega)} . Then it follows from Lemma 2.2 in two dimensions that (3.14) holds.
By means of the boundedness of ||w(\cdot, t)||_{W^{1, \infty}(\Omega)} , it follows from the Moser iteration of [45] that we can obtain the boundedness of ||u(\cdot, t)||_{L^{\infty}(\Omega)} and ||v(\cdot, t)||_{L^{\infty}(\Omega)} for all t\in(0, T_{\max}) .
Lemma 3.3. Let the conditions of Theorem 1.1 hold. Then the solution component (u, v) of system (1.8) satisfies
for all t\in(0, T_{\max}) , where C > 0 is a constant independent of t .
Proof. Multiplying the first equation of System (1.8) by u^{p-1} with p\geq2 and integrating by parts, we deduce from Young's inequality that
for all t\in(0, T_{\max}) , where \mathcal{K}_{1} is defined in the proof of Lemma 3.1 and we have applied ( H_{1} )–( H_{3} ) and (2.1).
It follows from Lemma 3.2 that there exists a C_{1} > 0 such that ||\nabla w(\cdot, t)||_{L^{\infty}(\Omega)}\leq C_{1} for all t\in (0, T_{\max}) . Hence, we deduce from (3.21) that
for all t\in(0, T_{\max}) , where C_{2}: = \frac{\mathcal{K}_{1}C_{1}^{2}}{2}+\gamma_{1}F_{1}(K_{0})+1 is independent of p . The rest can be handled exactly as the Moser iteration in Lemma 2.7 of [45] to derive the boundedness of ||u(\cdot, t)||_{L^{\infty}(\Omega)} for all t\in(0, T_{\max}) . Similarly, we can obtain the boundedness of ||v(\cdot, t)||_{L^{\infty}(\Omega)} for all t\in(0, T_{\max}) . The proof of Lemma 3.3 is complete.
Proof of Theorem 1.1. Theorem 1.1 is a direct consequence of Lemma 2.1, Lemma 3.2 and Lemma 3.3.
4.
Large time behavior
In this section, we shall study the asymptotic stability of global bounded solutions for System (1.11) by constructing energy functionals used in [13,57]. To do this, we first give some regularity results of the solution (u, v, w) for System (1.11).
Lemma 4.1. Let (u, v, w) be a global bounded classical solution for (1.11) ensured in Theorem 1.1. Then there exist \sigma\in(0, 1) and C > 0 such that
Proof. This lemma can be verified by a similar argument in Lemma 4.1 of [14], so we omit the details here for brevity.
Lemma 4.2. Let (u, v, w) be a global bounded classical solution for (1.11) ensured in Theorem 1.1. Then there exists a C > 0 such that
Proof. This lemma can be verified by a similar argument in Lemma 3.6 of [45], so we omit the details here for brevity.
In order to prove the asymptotic stabilization of global bounded solutions for system (1.11), we provide the following lemma, which is proved in [57].
Lemma 4.3. Let \phi:(1, \infty)\rightarrow [0, \infty) be uniformly continuous such that \int_{1}^{\infty}\phi(t)dt < \infty . Then
4.1. Proof of Theorem 1.2
In this subsection, we are devoted to studying the stabilization of the coexistence steady state (u^{*}, v^{*}, w^{*}) for some parameters cases. Let us introduce the following functionals
and
where (u^{*}, v^{*}, w^{*}) is given by (1.14).
Lemma 4.4. Let the conditions of Theorem 1.2 hold. Then there exists a positive constant \varepsilon_{1} independent of t such that
and
Proof. Let
then \mathcal{E}_{1}(t) can be rewritten as
Step 1: We shall prove the nonnegativity of \mathcal{E}_{1}(t) for all t > 0 . Let H(\xi): = \xi-u^{*}\ln \xi for \xi > 0 ; it follows from Taylor's formula for all x \in \Omega and each t > 0 that there exists a \tau = \tau(x, t) \in(0, 1) such that
Hence, we immediately derive that I_{1}(t) = \int_{\Omega}\left(H(u)-H\left(u^{*}\right)\right)dx \geq 0 . Similarly, we know that I_{2}(t) \geq 0 and I_{3}(t) \geq 0 for all t > 0 . Thus, we know that (4.4) holds.
Step 2: Now, we further prove (4.5). By a series of simple calculations, we get
where we have used the fact that \theta = \gamma_{1}w^{*}-\alpha_{1}u^{*}-\beta_{1}v^{*} .
Similarly, it follows from the identities \theta = \gamma_{2}w^{*}-\beta_{2}u^{*}-\alpha_{2}v^{*} and \mu = u^{*}+v^{*}+\mu w^{*} that
and
Hence, by combining (4.6)–(4.8), we derive
where X_{1} = (u-u^{*}, v-v^{*}, w-w^{*}) and Y_{1} = \left(\frac{\nabla u}{u}, \frac{\nabla v}{v}, \nabla w\right) , as well as
It follows from (1.20) that
as well as
which implies that the matrix A_{1} is positive definite as according to Sylvester's criterion. Similarly, we deduce from (1.21) that
as well as
which implies that the matrix B_{1} is positive definite. Thus there exist positive constants \kappa_{1} and \kappa_{2} such that
for all x\in \Omega and t > 0 . Let \varepsilon_{1}: = \min\{\kappa_{1}, \kappa_{2}\} , we have
which implies that (4.5) holds. The proof of Lemma 4.4 is complete.
With the aid of Lemma 4.4, we shall give the following large time behavior of global solutions for system (1.11).
Lemma 4.5. Let the assumptions of Theorem 1.2 hold. Then the global bounded solution of (1.11) converges to the coexistence steady state (u^{*}, v^{*}, w^{*}) given by (1.14), i.e.,
as t\rightarrow \infty .
Proof. It follows from Lemma 4.4 and integration over (1, \infty) that
According to Theorem 1.1 and Lemma 4.1, the bounded solution u, v and w are Hölder continuous in \bar{\Omega} \times[t, t+1] with respect to t > 1 . Thus we conclude that \mathcal{F}_{1}(t) is uniformly continuous in (1, \infty) . Thus we infer from Lemma 4.3 that
as t \rightarrow \infty . By the Gagliardo-Nirenberg inequality in two dimensions, there exists a C_{1} > 0 such that
Moreover, it follows from Lemma 4.2 that u(\cdot, t)-u^{*} is bounded in W^{1, 4}(\Omega) ; thus, we conclude from (4.18) that u(\cdot, t) \rightarrow u^{*} in L^{\infty}(\Omega) as t \rightarrow \infty . By the similar arguments for v and w , we derive (4.17). The proof of Lemma 4.5 is complete.
Now, we give the convergence rate of the coexistence state (u^{*}, v^{*}, w^{*}) for System (1.11).
Lemma 4.6. Let the assumptions of Theorem 1.2 hold; the global bounded solution (u, v, w) of (1.11) exponentially converges to the coexistence state (u^{*}, v^{*}, w^{*}) , i.e. there exist C > 0 and \lambda > 0 such that
for all t > T_{1} , where T_{1} > 0 is some fixed time.
Proof. It follows from Lemma 4.5 that ||u-u^{*}||_{L^{\infty}(\Omega)}\rightarrow 0 as t\rightarrow \infty . Therefore, we apply L'Hôpital's rule to obtain
which implies that there exists a t_{1} > 0 such that
for all t > t_{1} . Similarly, we can find t_{2} > 0 satisfying
and
for all t > t_{2} . Let T_{1}: = \max\{t_{1}, t_{2}\} ; by means of the definitions of \mathcal{E}_{1}(t) and \mathcal{F}_{1}(t) , it follows from the second inequalities in (4.21)–(4.23) that there exists a C_{1} > 0 such that
By Lemma 4.4, we derive
which implies that there exist C_{2} > 0 and C_{3} > 0 such that
Thus we deduce from the first inequalities in (4.21)–(4.23) that there exists a C_{4} > 0 such that
It follows from the Gagliardo-Nirenberg inequality in two dimensions, Lemma 4.2 and Lemma 3.2 that there exist positive constants C_{5} and C_{6} such that
for all t > T_{1} . The proof of Lemma 4.6 is complete.
Proof of Theorem 1.2. The statement of Theorem 1.2 is a straightforward consequence of Lemma 4.6.
4.2. Proof of Theorems 1.3 and 1.4
In this subsection, we shall study the stabilization of the semi-trivial steady state (\overline{u}, 0, \overline{w}) or (0, \widetilde{v}, \widetilde{w}) for some parameters cases. Since the methods of the proofs of Theorem 1.3 and Theorem 1.4 are very similar, we only give the proof of Theorem 1.3 for brevity. To do this, let us introduce the following functionals
and
where \overline{u} = \frac{\mu(\gamma_{1}-\theta)}{\alpha_{1}\mu+\gamma_{1}}\; \; \text{and}\; \; \overline{w} = \frac{\alpha_{1}\mu+\theta}{\alpha_{1}\mu+\gamma_{1}} .
Lemma 4.7. Let the conditions of Theorem 1.3 hold. Then there exists a positive constant \varepsilon_{2} independent of t such that
and
Proof. Let
then \mathcal{E}_{2}(t) can be represented as
Firstly, we can prove the nonnegativity of \mathcal{E}_{2}(t) for all t > 0 by the similar arguments used in Step 1 in Lemma 4.4. For brevity, we omit the details here. Now, we shall prove (4.30). By a series of simple calculations, we get
where we have used the fact that \theta = \gamma_{1}\overline{w}-\alpha_{1}\overline{u} .
Similarly, we can derive
and
where we have used the fact that \mu = \overline{u}+\mu \overline{w} . Thus it follows from (4.31)–(4.33) that
where X_{2} = (u-\overline{u}, v, w-\overline{w}) and Y_{2} = \left(\frac{\nabla u}{u}, \nabla w\right) , as well as
It follows from (1.20) that
as well as
which implies that the matrix A_{2} is positive definite as according to Sylvester's criterion. Similarly, we deduce from (1.23) that
which implies that the matrix B_{2} is positive definite. Thus there exist positive constants \iota_{1} and \iota_{2} such that
for all x\in \Omega and t > 0 . Let \varepsilon_{2}\in (0, \min\{\iota_{1}, \iota_{2}\}) ; we obtain
which implies that (4.30) holds. The proof of Lemma 4.7 is complete.
With the help of Lemma 4.7, we shall give the following stabilization of the semi-trivial steady state (\overline{u}, 0, \overline{w}) for System (1.11).
Lemma 4.8. Let the assumptions of Theorem 1.3 hold. Then the global bounded solution (u, v, w) of (1.11) converges to the semi-trivial steady state (\overline{u}, 0, \overline{w}) given by (1.24), i.e.,
as t\rightarrow \infty .
Proof. The proof of this lemma is similar to that of Lemma 4.5; here we omit the details.
Now, we give the convergence rate of the semi-trivial steady state (\overline{u}, 0, \overline{w}) for System (1.11).
Lemma 4.9. Let the assumptions of Theorem 1.3 hold; then, there exist positive constants C and \lambda such that:
(a) when \theta = \gamma_{2}\overline{w}-\beta_{2}\overline{u} , then
(b) when \theta > \gamma_{2}\overline{w}-\beta_{2}\overline{u} , then
where T_{2} > 0 is some fixed time.
Proof. Let
then it follows from Lemma 4.7 that there exists a \varepsilon_{2} > 0 such that
We deduce from Lemma 4.8 that ||u(\cdot, t)-\overline{u}||_{L^{\infty}(\Omega)}+||v(\cdot, t)||_{L^{\infty}(\Omega)}+||w(\cdot, t)-\overline{w}||_{L^{\infty}(\Omega)}\rightarrow 0 as t\rightarrow \infty . Hence, we apply L'Hôpital's rule to obtain
which implies that there exists a t'_{1} > 0 such that
for all t > t'_{1} . Similarly, we can find t'_{2} > 0 satisfying
for all t > t'_{2} .
By using the fact that \lim_{s\rightarrow 0}\frac{s}{s^{2}+s} = 1 , it follows from ||v(\cdot, t)||_{L^{\infty}(\Omega)}\rightarrow 0 as t\rightarrow \infty that there exists a t'_{3} > 0 such that
for all t > t'_{3} .
(a) When \theta = \gamma_{2}\overline{w}-\beta_{2}\overline{u} , (4.45) can be turned into
Let T_{2}: = \max\{t'_{1}, t'_{2}, t'_{3}\} ; by means of the definitions of \mathcal{E}_{2}(t) and \mathcal{F}_{2}^{*}(t) , it follows from the second inequalities in (4.47) and (4.48) that there exist positive constants C_{1} and C_{2} such that
for all t > T_{2} , where we have used Hölder's inequality and the boundedness of (u, v, w) asserted by Theorem 1.1. Thus, we deduce from (4.50) that
which implies
with some positive constant C_{3} . Hence we infer from the first inequalities in (4.47)–(4.49) that there exists a C_{4} > 0 such that
It follows from the Gagliardo-Nirenberg inequality in two dimensions, Lemma 4.2 and Lemma 3.2 that there exist positive constants C_{5} and C_{6} such that
for all t > T_{2} .
(b) When \theta > \gamma_{2}\overline{w}-\beta_{2}\overline{u} , let T_{2}: = \max\{t'_{1}, t'_{2}, t'_{3}\} ; by means of the definitions of \mathcal{E}_{2}(t) and \mathcal{F}_{2}^{*}(t) , it follows from the second inequalities in (4.47) and (4.48) that there exists a positive constant C_{7} such that
for all t > T_{2} .
By combining (4.45) with (4.56), we have
Since \theta > \gamma_{2}\overline{w}-\beta_{2}\overline{u} , then we can select \varepsilon_{2}\leq \frac{1}{\gamma_{2}}(\theta-\gamma_{2}\overline{w}+\beta_{2}\overline{u}) such that
which means that there exist C_{8} > 0 and C_{9} > 0 satisfying
Thus we deduce from the first inequalities in (4.47)–(4.49) that there exists a C_{10} > 0 such that
It follows from the Gagliardo-Nirenberg inequality in two dimensions, Lemma 4.2 and Lemma 3.2 that there exist positive constants C_{11} and C_{12} such that
for all t > T_{2} . The proof of Lemma 4.9 is complete.
Proof of Theorem 1.3. The statement of Theorem 1.3 is a direct consequence of Lemma 4.9.
4.3. Proof of Theorem 1.5
In this subsection, we are devoted to discussing the asymptotic stability of the prey-only steady state (0, 0, 1) under some suitable parameters conditions. To do this, let us denote the following functionals
and
we can derive the following estimates of \mathcal{E}_{3}(t) and \mathcal{F}_{3}(t) .
Lemma 4.10. Let the conditions of Theorem 1.5 hold. Then there exists a \varepsilon_{3} > 0 independent of t such that
and
Proof. By the similar arguments as in the proofs of Lemma 4.4 and Lemma 4.7, we can derive (4.62) and
where we have used the fact that w\leq K_{0} = \max\{||w_{0}||_{L^{\infty}(\Omega)}, 1\} . Let \varepsilon_{3}\in \left(0, \min\{\frac{\alpha_{1}}{\gamma_{1}}, \frac{\alpha_{2}}{\gamma_{2}}, \mu, \frac{D}{K_{0}^{2}}\}\right) ; we obtain
for all t > 0 . The proof of Lemma 4.10 is complete.
With the help of Lemma 4.10, we shall give the following stabilization of the prey-only steady state for System (1.11).
Lemma 4.11. Let the assumptions of Theorem 1.5 hold. Then the global bounded solution of (1.11) converges to the prey-only steady state (0, 0, 1) , i.e.,
as t\rightarrow \infty .
Proof. The proof of this lemma is similar to that of Lemma 4.5; here we omit the details.
Now, we give the convergence rate of the prey-only steady state (0, 0, 1) for System (1.11).
Lemma 4.12. Let the assumptions of Theorem 1.5 hold; then there exist positive constants C and \lambda such that:
(a) when \gamma_{i} = \theta , i = 1, 2 , then
(b) when \gamma_{i} < \theta , i = 1, 2 , then
where T_{3} > 0 is some fixed time.
Proof. Let
then it follows from Lemma 4.10 that there exists a \varepsilon_{3} > 0 such that
By using the facts that \lim_{s\rightarrow 0}\frac{s}{s^{2}+s} = 1 and \lim_{s\rightarrow 1}\frac{s-1-\ln s}{(s-1)^{2}} = \frac{1}{2} , it follows from ||u(\cdot, t)||_{L^{\infty}(\Omega)}+||v(\cdot, t)||_{L^{\infty}(\Omega)}+||w(\cdot, t)-1||_{L^{\infty}(\Omega)}\rightarrow 0 as t\rightarrow \infty , as asserted in Lemma 4.11 that there exists a T_{3} > 0 such that
and
as well as
for all t > T_{3} .
(a) When \gamma_{i} = \theta , i = 1, 2 , (4.70) can be simplified as
By means of the definitions of \mathcal{E}_{3}(t) and \mathcal{F}_{3}^{*}(t) , it follows from the second inequality in (4.73) that there exist positive constants C_{1} and C_{2} such that
for all t > T_{3} , where we have used Hölder's inequality and the boundedness of (u, v, w) asserted by Theorem 1.1. Thus we deduce from (4.74) that
which implies
with some positive constant C_{3} . Hence we infer from the first inequalities in (4.71)–(4.73) that there exists a C_{4} > 0 such that
It follows from the Gagliardo-Nirenberg inequality in two dimensions, Lemma 4.2 and Lemma 3.2 that there exist positive constants C_{5} and C_{6} such that
for all t > T_{3} .
(b) When \gamma_{i} < \theta , i = 1, 2 , by means of the definitions of \mathcal{E}_{3}(t) and \mathcal{F}_{3}^{*}(t) , it follows from the second inequalities in (4.71)–(4.73) that there exists a positive constant C_{7} such that
for all t > T_{3} .
By combining (4.70) with (4.80), we derive
for all t > 0 . Since \gamma_{i} < \theta , i = 1, 2 , we can select \varepsilon_{3}\leq \min\left\{\frac{1}{\gamma_{1}}(\theta-\gamma_{1}), \frac{1}{\gamma_{2}}(\theta-\gamma_{2})\right\} such that
which means that there exist C_{8} > 0 and C_{9} > 0 satisfying
Thus we deduce from the first inequalities in (4.71)–(4.73) that there exists a C_{10} > 0 such that
It follows from the Gagliardo-Nirenberg inequality in two dimensions, Lemma 4.2 and Lemma 3.2 that there exist positive constants C_{11} and C_{12} such that
for all t > T_{3} . The proof of Lemma 4.12 is complete.
Proof of Theorem 1.5. The statement of Theorem 1.5 is a direct consequence of Lemma 4.12.
Acknowledgments
The author is grateful to the editor and three anonymous referees for their valuable comments, which greatly improved the exposition of our paper. The author is also deeply grateful to Professor Renjun Duan for the support and friendly hospitality in CUHK. The work was partially supported by the National Natural Science Foundation of China (Grant Nos: 11601053, 11526042), Natural Science Foundation of Chongqing (Grant No. cstc2019jcyj-msxmX0082), Chongqing Municipal Education Commission Science and Technology Research Project (Grant No. KJZD-K202200602), China-South Africa Young Scientist Exchange Project in 2020, The Hong Kong Scholars Program (Grant Nos: XJ2021042, 2021-005) and Young Hundred Talents Program of CQUPT from 2022–2024.
Conflict of interest
The author declares that there is no conflict of interest.















 DownLoad:
DownLoad: