In this paper, we propose and analyze a reaction-diffusion model for predator-prey interaction, featuring both prey and predator taxis mediated by nonlocal sensing. Both predator and prey densities are governed by parabolic equations. The prey and predator detect each other indirectly by means of odor or visibility fields, modeled by elliptic equations. We provide uniform estimates in Lebesgue spaces which lead to boundedness and the global well-posedness for the system. Numerical experiments are presented and discussed, allowing us to showcase the dynamical properties of the solutions.
1.
Introduction
The mathematical modeling of predator-prey interactions has a long and rich history. The basic dynamics are given by the system of Lotka–Volterra (here in nondimensional form)
where u(t)≥0 represents the predator and w(t)≥0 the prey at time t≥0. According to system (1.1), the predator population decreases exponentially in the absence of prey, while the prey follows a logistic growth law. Interactions between predators and prey are modeled by a mass-action law benefitting the predator and depleting the prey. The system's main feature is the global asymptotic stability of its only nontrivial steady state (u,w)=(α−1α,1α), which, for α>1, expresses a balance between predation, prey reproduction, and predators' natural death rate.
When spatial movement is expected to influence the dynamics, it is natural to consider predator and prey densities u(t,x) and w(t,x) depending on a spatial variable x in some physical domain Ω⊂R2. Then, one can introduce spatial diffusion and advection to model foraging movement, the spreading of the population in a territory, and/or movement in a preferred direction.
In particular, besides spatial diffusion, a reasonable assumption is that the prey tries to evade the predator, while the predator tries to chase the prey. This can be modeled by introducing advection terms into the equations. Thus, the predators advect towards regions of higher prey density, while prey advects away from regions of higher predator density. Variants of this idea have been considered, for instance, in [1,2,3,4,5,6,7,8,9,10,11,12,13,14].
Recently, indirect prey- and predator-taxis have been introduced as a mechanism allowing pursuit and evasion [3,4,10,15]. This supposes that the advection velocities are mediated by some indirect signal, which may be an odor, a chemical, a field of visual detection, or seen as a potential. In this spirit, following [3,4,10,15], we consider the predator-prey system with pursuit, evasion and non-local sensing (already written in a non-dimensional form)
for t>0, x in a bounded, open Ω⊂R2, supplemented with boundary conditions
and initial data u0(x),w0(x). Here, u(t,x) is the predator density, w(t,x) is the prey density, p(t,x) is the odor produced by the prey, q(t,x) is the odor produced by the predator, and α,β,Dw,Dp,Dq,δp,δq are non-negative non-dimensional constants --- see the Appendix for the physical meaning of the constants and details on the non-dimensionalisation procedure. System (1.2) states that the predator is attracted to the odor p of the prey w, which solves a (steady-state) diffusion equation with source proportional to w, while the prey is repelled by the odor q produced by the predator.
Notice that the equations for the odors of the prey and predator are elliptic, rather than parabolic. This is justified in cases where the diffusion of the odor happens in a much faster time scale than the movement of individuals, which is reasonable on a variety of ecological settings.
Note also that we refer to p and q as "odors" but these quantities do not necessarily model chemical odors. They may be more generally interpreted as potentials representing the chance of an animal being detected at a distance by, e.g., visual means.
We remark on the Neumann boundary conditions (1.3). They model the fact that in the time evolution of system (1.2) there is no flow of individuals across the border of the physical domain. Depending on the particular application, this may be a natural assumption, modeling for instance an enclosed area. However, different boundary conditions could be envisaged (e.g., Dirichlet or mixed-type) reflecting distinct natural settings. Here we limit ourselves to the analisys with (1.3). Still, in the numerical experiments presented below, we show an example with Dirichlet boundary conditions.
1.1. Main contributions and outline of the paper
In light of the recent mathematical results in [4,15], our main contributions are the introduction of the predator-prey Lotka-Volterra dynamics and the numerical simulations. As we will see, the interaction terms on the right-hand side make the analysis more involved, in particular with respect to the derivation of L∞ estimates. Indeed, while it is shown in the above-mentioned works that the system with no population or interaction dynamics does not originate finite time blow-up of the solutions, the predator equation dynamics introduces a quadratic term uw so it is not obvious that the boundedness property remains valid. We shall see that the attractive-repulsive nature of the advection terms continues to ensure boundedness of the solutions. Moreover, the property of instantaneous boundedness of the solution even for unbounded initial data, observed already in [4], remains valid in this setting.
An outline of the paper follows. In Section 2, we present our main well-posedness result. Next, in Section 3 we derive our main a priori estimates in Lγ spaces, for γ∈[1,∞]. This will allow us, in Section 4, to construct strong and then weak solutions to the system (1.2), completing the proof of our well-posedness result. Finally, in Section 5 we detail an implicit-explicit two-step finite volume method for the approximation of system (1.2) and present some numerical experiments.
2.
Main Results
The main results of this paper are concerned with the well-posedness and Lebesgue integrability of weak solutions of the system (1.2). We follow a strategy similar to [4,16], making use of fine a priori estimates in Lebesgue spaces, and a De Giorgi level-set method [17] to obtain boundedness of the solutions. Still, the character of the present system introduces several changes in the analysis with respect to the results in [4,16].
The system (1.2) is, mathematically, of chemotaxis type [18,19]. As is well known, such systems may exhibit blow-up of solutions in finite time, see for example the review [20]. Therefore, it is not obvious at first glance whether solutions might also possess blow-up behavior. As we shall see in the following analysis, the indirect nature of the sensing, as well as the attraction-repulsion behavior, prevent the densities from becoming infinite in finite time.
We say that the quadruple (u,w,p,q) is a weak solution of the system (1.2) if it satisfies:
1. (u,w)∈L2(0,T;H1(Ω)) and (∂tu,∂tw)∈L2(0,T,[H1(Ω)]∗), and
2. For any test function ξ∈C∞([0,∞)×Ω) compactly supported in [0,T)ׯΩ, we have
and
Note that while the biologically relevant regime is when α>1, the value of α>0 does not change the results, so we present most of our analysis with α, as well as all the remaining constants in system (1.2), set to 1.
We will suppose throughout the paper that the initial data (u0,w0) is non-negative and has finite mass
and that Ω⊂R2 is a bounded domain of class C2. The main results regarding the system (1.2) are collected here:
Theorem 2.1. Let the initial data u0,w0 be in Lα(Ω) for some α>2. Then, the system (1.2) has a unique non-negative weak solution. The following estimates are satisfied by the solutions for any 0≤t≤T<∞,
(ⅰ) For any γ∈[1,α], it holds
In particular, if u0,w0∈L∞(Ω), then
(ⅱ) Lγ−integrability: for any γ∈(α,∞], it holds
In particular, we have the L∞-integrability property
for some C independent of t>0.
3.
Analysis of the system (1.2)
In this section we provide a priori estimates which will be used in the well-posedness results. To establish the existence of a weak solution, we must first prove that strong, or classical, solutions exist. We say that (u,w,p,q) is a classical solution of the system (1.2) if it satisfies:
1. (u,w,p,q)∈C([0,T];L2(Ω)) and each of the terms in the system (1.2) are well defined functions in L2((0,T)×Ω),
2. The equations on (1.2) are satisfied almost everywhere, and
3. The initial data (u,w)|t=0=(u0,w0) and boundary conditions (1.3) are satisfied almost everywhere.
An essential feature of solutions of (1.2) is the following mass estimate:
Proposition 3.1 (Mass estimate). Let (u,w,p,q) be sufficiently smooth non-negative solutions of the system (1.2) with the boundary conditions (1.3). Then there exists a constant M depending on ‖u0‖1, ‖w0‖1, α, β and |Ω|, but not on t, such that for all t>0,
Proof. Integrating the first and second equations of (1.2) and using the Neumann boundary conditions (1.3), we find
From β(w−w2)≤(β+1)24β−w we get, with ζ(t):=∫Ωw+βαudx,
The conclusion of the proposition readily follows.
3.1. A priori estimates in Lγ
In this section we prove that data (u0,w0)∈L1(Ω) generate instantaneous Lγ-integrability, with γ>1, for classical non-negative solutions of (1.2).
Proposition 3.2 (A priori estimates in Lγ). Let (u,w,p,q) be sufficiently smooth nonnegative solutions of the system (1.2) with boundary condition (1.3) and integrable initial data, and let t>0 be arbitrary. Then, for any γ∈(1,∞), ϵ>0, we have the estimate
Moreover, if u0,w0∈Lγ(Ω), then actually
for some C>0 depending on M and Lγ−norms of the data, but independent of t.
Proof. We will frequently use the Gagliardo–Nirenberg–Sobolev (GNS) inequality in two dimensions (see e.g. [21]), holding for any α≥1,
as well as the interpolation inequality
We start by multiplying the second equation of (1.2) by wγ−1 and integrating, to find (discarding a nonpositive term)
Now multiply the fourth equation of (1.2) by wγ and integrate by parts to get
Also using
we find, discarding nonpositive terms,
Let us consider the terms on the right-hand side of the previous inequality. Take a small ϵ>0 to be specified later. We use the following consequence of Young's inequality, qwγ≤ϵwγ+1+ϵ−γqγ+1, and also the inequality
which is obtained from Young's inequality, the mass estimate (3.1), and the interpolation inequality (3.5). Therefore, for some constant C depending on γ, M, and ϵ,
This way, we find
and from the GNS inequality (3.4) and ϵ sufficiently small,
Again, from ∫Ωwγdx≤C+ε∫Ωwγ+1dx, we get
for some C depending on γ, M, the GNS constant, and the parameters of the system.
To deal with the last term on the right-hand side of (3.8), multiply the fourth equation of (1.2) by qγ−1 to get
From the GNS inequality (3.4) we deduce that
Choosing ϵ small, we find
In view of (3.9), the estimate (3.8) becomes
Note that the gain from w being the prey population, and thus having a repulsive behavior, is reflected in the lower power uγ.
In contrast, performing very similar computations using the first and third equations of the system (1.2), so that instead of (3.6) only
is valid, we find that for α≥2
Adding (3.10) and (3.11) gives
It is clear that to conveniently bound the terms on the right-hand side using the left-hand side, we should take α<γ<α+1.
Now, we make use of the interpolation inequalities
Recalling the mass estimate (3.1), we get
for C depending on γ,α, and M. Now, θ2(α+1)<γ+1 and θ1γ<α+1, so using Young's inequality with a sufficiently small ϵ allows the terms on the right-hand side to be absorbed into the left-hand side. This gives
for C depending on γ,α, and M. Next, use the inequality (3.5) to find
and so, from (‖u‖αα)αα−1≤(‖u‖αα)γγ−1 and convexity of the power function, we find, setting
that
Now use the ODE comparison result from [16, Corollary A.2] to conclude
In view of the definition of η, one finds
and, taking γ=α+ϵ,
for C depending on γ,α, and M. This proves the estimate (3.2). The uniform estimate (3.3) follows from the afore-mentioned ODE comparison results in [16]. This concludes the proof of Proposition 3.2.
3.2. L∞ estimates
In this section we prove two boundedness results adopting De Giorgi's energy method (see [17] and [4,16,22,23,24] for related applications of the method). Throughout this section, we will use the notation γ+ to denote an arbitrary fixed number in (γ,+∞).
First, we consider initial data u0 and w0 only in L1(Ω), and obtain an estimate of the type
for some constant C>0 depending on M, but not on T>0. Then, we will suppose that the initial data u0 and w0 are in L∞(Ω). Then we can upgrade the estimates above to
where C now depends also on the L∞ norms of initial data.
To further clarify the notation, let us spell out that, for instance, the estimate (3.12) precisely means that for any ϵ>0, it holds
with a constant C that may, however, depend on ϵ.
3.2.1. Initial data in L1
The main result in this section is the following:
Proposition 3.3. Let (u,w,p,q) be a sufficiently smooth non-negative solution of the system (1.2) with boundary conditions (1.3) and integrable, nonnegative initial data, and let T>0 be arbitrary. Then, for all t∈(0,T], we have the estimate
where the constant C is independent of T>0.
Let us begin by recording here the following L∞ estimates, which are a consequence of elliptic regularity for the last two equations of the system (1.2) and Proposition 3.2.
and
The first step in the proof of Proposition 3.3 is a boundedness result valid on each interval (t∗,T) with t∗>0.
Lemma 3.4. Let (u,w,p,q) be as in Proposition 3.3, and let t∗>0. Then, there exist constants M,N>0 depending on t∗,T, and u(t∗),w(t∗) such that 0≤u(t,x)≤M and 0≤w(t,x)≤N almost everywhere on (t∗,T)×Ω.
Proof. Although the structure of the proof is the similar to corresponding results in [4,16], we show the details since the rather involved calculations depend heavily on the structure of the system. Consider (u,p) a non-negative, sufficiently smooth solution to the general problem
in [0,T]×Ω with the boundary conditions
where n is the outward unit normal vector to ∂Ω, f and w are known, and w satisfies
Similarly, take a non-negative solution (w,q) to the problem
[0,T]×Ω with boundary conditions
g and u given and u satisfying
We denote Vλ={(t,x)∈Ω×[0,T],u(t,x)>λ} and define
Multiplying the first equation of (3.15) by uλ, integrating in space and using (3.16), we obtain
For the first term on the right-hand side note that using Young's inequality, we find
Thus,
Let M>0 be a constant to be defined later, let t∗>0, and set
for k=0,1,2,…. Define the energy functional
where we use the notation uk=uλk. Similarly, we define for w the energy functional
with the same definitions of λk (with N instead M) and tk, for some N>0 to be chosen later.
With λ=λk on (3.19), integrating over [s,t], we obtain
We use this relation with tk−1≤s≤tk≤t≤T to check that
Integrating with respect to s over [tk−1,tk], bearing in mind that tk−tk−1=t∗/2k and only the first term on the right-hand side of this inequality depends on s, we obtain
Now, we are going to introduce a series of estimates for I1, I2 and I3. For this, we will use the Gagliardo-Nirenberg interpolation inequality in Rn (see e.g. [25]) and a key estimate for 1Vk. Note that we are temporarily performing the analysis in n dimensions. We have
which holds for any α∈[0,1] and 1≤p≤∞. Choosing the parameter α∈[0,1] such that αp=2, it follows that p=2n+2n and
Observe also that uk>0 implies u>λk, therefore u−λk−1>λk−λk−1=M2k. We also have u−λk<u−λk−1, thus, which a simple computation,
holds for all a≥0.
● Estimate for I1
First, note that for a≥0 we have
We choose a in (3.24) such that 2+a=p=2n+2n, so a=4n. Thus,
Note that ∫Ttk−1(‖uk−1‖22+‖∇uk−1‖22)ds≤(T+1)Uk−1, which leads to
● Estimate for I2
We have that
Now, choosing a=p in (3.24), we obtain
where we need q>1. Thus,
where we used (3.23) with the relation (3.22). We choose α∈(0,1) satisfying αpq=2 to find
Observe that α=2qp implies α=qnn+2q, then
where q is such that 1<q<nn−2. Thus,
where in this last step we proceeded as in the estimate for I1. Therefore
● Estimates for I3
Proceeding as we did for the estimate of I2, using that uk≤u1Vk, we have
where α, p and q are the same of the estimate for I2. Since supt≥t∗2‖f+u‖q′≤supt≥t∗2{‖f+‖2q′‖u‖2q′}, we find
Combining (3.25), (3.26) e (3.27), we obtain
Now we are going to restrict our reasoning to the case n=2, where we have α=qq+1 and the advantage that we can take 1<q<∞, since 1<q<nn−2=∞. Using Proposition 3.2, we have
Likewise,
and particularizing to f+=uw,
Therefore,
Now, substituting these results in (3.28), we obtain
We are going to prove that there exists a constant a∈(0,1) depending only on q such that Uk≤akU0 for all k∈N. First, set Vk=akU0. Then applying the recurrence relation defined by the right-hand side of (3.31) to Vk gives
Now we choose a such that max{23a,22(q+1)qa1q}<1. So, the last line of (3.32) is bounded by
Now, choosing M so that
we find that
In other words, Vk is a supersolution of the recurrence relation defined by (3.31). By a comparison principle, we have Uk≤akU0⟶k→+∞0. Thus, we obtain
Fatou's lemma implies
which in turn implies 0≤u(t,x)≤M almost everywhere on (t∗/2,T)×Ω. For t∗/2<1 we can determine M explicitly via
Taking q↘1, we have
A very similar computation using (3.17), (3.21), particularizing to g+=w gives
Then, we obtain N>0 as before so that 0≤w(t,x)≤N almost everywhere on (t∗/2,T)×Ω and when 0<t∗/2<1, N can be estimated by
Renaming t∗/2 as t∗ concludes the proof of Lemma 3.4.
Proof of Proposition 3.3. In Lemma 3.4 we found M,N>0 such that 0≤u(t,x)≤M and 0≤w(t,x)≤N almost everywhere on (t∗,T)×Ω. From (3.33) and (3.34) we see that M and N depend directly on U0 and W0, respectively. First, we are going to obtain appropriate estimates for these terms, from which the L∞−bound for w and u in the time interval (t∗,1) follows. After this, we will extend the estimate for general large intervals. Proceeding as we did in (3.19) for the particular case f+=uw, we have
where, in the last step, we used
which follows from the first equation of (3.15). Observe that
Substituting this in (3.35), we obtain
Integrating over [s,t], we find
Observe that from this inequality we conclude
Now, via (3.33), using the estimates made previously, we get for t≥t∗
where we remember that 0<t∗<1. For W0, a similar computation with (3.17) and particularizing g=w leads to
Now, via (3.34), we conclude for t≥t∗
where we recall that 0<t∗<1.
These estimates are valid whenever 0<t∗≤t≤T=1, but the same reasoning can be applied for any T>0 and it would provide a bound depending on T. In order to justify that the same L∞-estimate can be made uniform with respect to T, we proceeding by extending this estimate in a similar way as was done in [16]: let t1∈(t∗,T−t∗) and note that the shifted functions wt1(t,x)=w(t+t1,x) and ut1(t,x)=u(t+t1,x) are still solutions of the same problem with initial data wt1(0,x)=w(t1,x) and ut1(0,x)=u(t1,x), and the appropriate right-hand side. Since the constant C doesn't change due to the Proposition 3.1, we pick t1∈(0,T) and repeat the same arguments to wt1 and ut1, which leads the same L∞-bounds (3.37) and (3.38) for w and u on the interval [t∗,T]. It means that (3.37) and (3.38) happen for [t∗+t1,T+t1], that is, we extend (3.37) and (3.38) over [t∗,T+t1]. We can repeat this procedure, completing the proof of Proposition 3.3.
3.2.2. Initial data in Lα, α>2
We suppose now we have initial data u0 and w0 in Lα(Ω), for some α>2. We will slightly modify the previous analysis in order to obtain better versions of the estimates in Propositions 3.2 and 3.3, as well as a uniform estimate in L∞ in the case of bounded initial data.
Proposition 3.5. Let u0 and w0 be initial data in Lα(Ω), for some α∈(2,∞]. The estimates (3.2) of Propositions 3.2 and 3.3 can be upgraded by adding to the constant C the dependence of Lα-norms on the initial data: for any γ>α, we have
and, in particular,
If u0,w0∈L∞(Ω), then we have the uniform estimate
where the constant C>0 is independent of T>0.
Proof. First of all, by Proposition 3.2, there exist a constant A>0 such that
We change the definition tk=(1−1/2k)t∗, and observe now that t0=0. Note first that (3.30) becomes
since ‖∇p(t)‖∞≤‖u(t)‖α≤A<∞. In this way, (3.31) becomes
with a constant C depending on M, A and T, but independent of t∗ and k. We are going to proceed as we did after (3.31), but now with (3.42), relying on the fact that here we have
where the constant ˜C depends on M, A and T. Let us now see that there exist a∈(0,1), M>0, so that Uk≤akU0, for all k. So, taking the right-hand side of (3.42) applied to Vk:=akU0, we find
Then, taking a so that 23a<1,
Choosing M>0 so that
we get that Vk is a supersolution of the recurrence defined by (3.42), and so Uk≤akU0⟶k→+∞0. Observe also that for t<1
Thus, we have 0≤u(t,x)≤M, whence it follows that
We get the same estimate for w proceeding exactly in the same way for Wk, which leads to
This proves (3.40). Estimate (3.39) follows by Lebesgue interpolation between estimates (3.44) and (3.45) and mass conservation.} From this point, the deduction of the uniform estimate of Proposition 3.5 using (3.44) and (3.45) is very similar to [16,Prop.3.2], so we refer the reader to that work for details.
4.
Construction of classical and weak solutions
The a priori estimates of the previous sections will now allow us to prove the global well-posedness of the system (1.2). The first step is to prove existence and uniqueness of classical solutions with smooth initial data. For this, we will use the Banach fixed-point theorem. A stability result for such solutions will be obtained and the existence of a weak solution follows as a consequence.
4.1. Construction of classical solutions
Let w0∈C∞c(Ω) and define the set
equipped with the norm
Note that Υ with the metric induced by ‖⋅‖Υ is a complete metric space. In this section we prove the following theorem:
Theorem 4.1. Let u0 and w0∈C∞c(Ω) be non-negative initial dada. Then, for all T>0 the system (1.2) supplemented with the boundary condition (1.3) admits a unique non-negative classical solution. This solution satisfies the estimates obtained in Propositions 3.2, 3.3 and 3.5.
The main step to prove this theorem is the use of the following lemma, whose proof is standard and can be found in [16,Thm. 3.1].:
Lemma 4.2. Let ψ be a smooth solution of
with b,B,∇⋅B∈L∞. Then,
Now let ϕ∈Υ and let p=p[ϕ] be the solution of
Linear theory guarantees that there exists a unique solution p∈H1(Ω), and, since ϕ(t)∈L∞(Ω) and Ω is smooth, we have p(t)∈H2(Ω) with ‖p(t)‖H2≤C‖ϕ(t)‖L2 almost everywhere in time. Therefore we have p(t),∇p(t) and Δp(t)∈L∞(Ω). Now we associate u=u[ϕ] the solution of
By linear theory, u is unique and such that u∈L2(0,T;H1(Ω))∩C([0,T],L2(Ω)). Linear theory still guarantees that there exists some constant C>0 depending on T and Ω such that ‖u‖L∞(0,T;H1(Ω))≤C‖u0‖H1(Ω). Now, we associate q=q[ϕ] the solution of
The same arguments lead to the existence of a unique solution q∈H1(Ω), which satisfies q(t)∈H2(Ω) with ‖q(t)‖H2≤C‖u(t)‖L2, then q(t),∇q(t),Δq(t)∈L∞(Ω). Finally, we associate w=w[ϕ] the solution of
where w[ϕ] is the only weak solution and such that w∈L2(0,T;H1(Ω))∩C([0,T],L2(Ω)).
Lemma 4.3. For T>0 small enough, w[ϕ]∈Υ.
Proof. Applying Lemma 4.2 for w[ϕ],
Note that all terms in the exponential can be bounded by C‖ϕ‖∞, with C>0 depending on the data of the problem. Thus, for T>0 small enough we have 0≤w(t,x)≤2‖w0‖∞, which means w[ϕ]∈Υ.
Lemma 4.4. Φ:ϕ∈Υ↦w[ϕ]∈Υ is a contraction on [0,T] for some T>0.
Proof. Let ϕ1,ϕ2∈Υ and define ¯w=w1−w2, where w1 and w2 are the respective associated solutions, and so forth. It's easy to check that
Multiplying by ¯w and integrating in space, we obtain
where we used the estimates for wi, ui and ϕi guaranteed by Lemma 4.2 and by the definition of Υ, respectively. Substituting
and
in (4.3), we find
By Gronwall's lemma, it follows that
for some constant K>0 which may change from line to line. Likewise, for ¯u=u1−u2, we get
Note that
Combining (4.5) and (4.6) with (4.4), we conclude that
for all t∈[0,T]. Thus, for T>0 small enough, Φ is a contraction.
This is enough to prove Theorem 4.1. We take ˜T>0 being the smallest guaranteed by Lemma 4.3 and Lemma 4.4. By the fixed point theorem, the result follows for small time. Extension to [0,T] is done in a standard way.
Let α>2. We say that the system of equations (1.2) is stable on L∞(0,T;Lα(Ω)) when, given two pairs of initial data u0,i,w0,i∈Lα(Ω), the respective classical solutions ui and wi admit C>0 depending only on M and on Lα−norms of the data such that
Proposition 4.5. Let α>2. The system (1.2) is stable (in the sense of (4.7)) on L∞(0,T;Lα(Ω)).
Proof. Let ¯u=u1−u2 and similarly for the other differences. The system for the differences reads
Multiplying (4.8) by ¯u and integrating in space, we get
It is easy to check using (3.40) that
Hölder inequality guarantees that
Observe that using
for q∈(1,∞), with ‖∇¯p(t)‖22q≤C‖¯p(t)‖2H2≤C‖¯w(t)‖22 and ‖u2(t)‖22q′≤‖u2(t)‖1+1q∞‖u2(t)‖1q′1 and (3.40), we find
Then,
Choosing q big enough, we have from (4.9) and the previous estimates that
for some small ϵ. A similar result is obtained for ¯w using the same arguments, where we get
Defining Z(t)=‖¯u‖22+‖¯w‖22, we have
Integration of this differential equation leads to the result.
4.2. Global well-posedness of weak solutions
Now we are ready to state and prove a well-posedness result for weak solutions:
Theorem 4.6. Fix an arbitrary T>0 and assume non-negative initial data u0,w0∈Lα(Ω), for some α>2. Then, there exists a unique non-negative weak solution for the system (1.2). This solution satisfies the estimates of Proposition 3.2 and 3.3–3.5.
Proof. Take a sequence of non-negative initial data u0,k,w0,k∈C∞c(Ω) with u0,k→u0 and w0,k→w0 both strongly in Lα(Ω). Theorem 4.1 guarantees sequences uk,wk,pk and qk in C([0,T],L2(Ω)) strong solutions of the system (1.2) for each pair of data u0,k,w0,k∈C∞c(Ω),
k∈N. The following convergence properties hold:
ⅰ) uk→u and wk→w strongly in L∞(0,T;L2(Ω)).
ⅱ) pk→p and qk→q strongly in L∞(0,T;H1(Ω)).
ⅲ) uk⇀u and wk⇀w weakly in L2(0,T;H1(Ω)).
ⅳ) ∂tuk→∂tu and ∂twk→∂tw weakly in L2(0,T;[H1(Ω)]∗).
ⅴ) uk∇pk→u∇p and wk∇qk→w∇q strongly in L1((0,T)×Ω).
In fact, estimate (3.3) guarantees that uk and wk are uniformly bounded in L∞(0,T;Lα(Ω)) with respect to k≥1. Therefore, Proposition 4.5 applied to the Cauchy differences uk−ul, wk−wl, k,l∈N guarantees that uk and wk are Cauchy sequences in L∞(0,T;L2(Ω)), giving ⅰ). Now, let ¯p=pk−pl with k,l∈N, and denote ¯w=wk−wl. Multiplying by ¯p the difference of the third equations of (1.2) and integrating in space, we get
Similarly, with the fourth equation we compute
Thus, using Proposition 4.5, we have
This means that pk and qk are Cauchy sequences in L∞(0,T;H1(Ω)), so we get ii). Next, multiply the first and second lines of (1.2) by uk and wk, respectively, and integrate in space. Using (3.13), (3.14), we find
Integrating in time gives that ∇uk,∇wk are in L2(0,T;L2(Ω)) uniformly in k. From this we find iii).
It is classical to check that ∂tuk(t)=∇⋅(∇uk−uk∇pk)+ukwk−uk∈(H1)∗ and ∂twk(t)=∇⋅(∇wk+wk∇qk)+wk−w2k−ukwk∈(H1)∗. For instance, if φ∈L2(0,T;H1), then we find (to take just the term involving ∇uk, and using that ∇uk is in L2(0,T;L2(Ω)) uniformly in k)
Treating the other terms similarly, we get that ∂tuk and ∂twk are bounded in L2(0,T;[H1(Ω)]∗), so iv) holds. The last convergence follows from the previous ones.
Thus, we can pass to the limit k→∞ in (4.10) to find that (u,w,p,q) is a weak solution of (1.2). The condition (u(0),w(0))=(u0,w0) is satisfied by continuity at t=0 which follows from the estimate on the time derivatives. Finally, using the approximating by classical solutions we can check that the stability result from Proposition 4.5 and estimate (4.11) hold for weak solutions. This can be used to prove uniqueness: using the notations in Proposition 4.5, if (ui,wi,pi,qi), i=1,2 are two weak solutions associated to the same initial data, then (4.7) proves that (u1,w1)=(u2,w2) a.e. on [0,T]×Ω. In turn, the estimate (4.11) (which, since it is a direct consequence of Proposition 4.5, also holds for weak solutions) gives uniqueness of p and q.
5.
Numerical experiments
In order to show some of the relevant features of the system, we provide in this section the details of a numerical simulation of (1.2). Our goal is to present an implicit-explicit finite volume scheme and showcase some numerical results exhibiting the system's main features, namely, evasive behavior of the prey, and chasing by the predator.
We consider the system (1.2) in a rectangular domain Ω=[0,Lx]×[0,Ly], where we introduce a cartesian mesh consisting of the cells Ii,j:=[xi−12,xi+12]×[yj−12,yj+12], which for the sake of simplicity, are assumed square with uniform size, so |Ii,j|:=h2 for all i and j. Consider a step size Δt>0 to discretize the time interval (0,T). Let N>0 the smallest integer such that NΔt≤T and set tn:=nΔt for n∈{0,N}. The cell average of a quantity v at time t is defined by
and define ¯vni,j:=¯vi,j(tn). Note that in this section we use x=(x,y) to denote the spatial variable. Let fk(u,w), k=1,2, be the reactive terms in the right-hand side of the first two equations in (1.2). Then, the terms
are approximated by fk,i,j:=fk(¯ui,j,¯wi,j),k=1,2. The Laplacian on a Cartesian grid is discretized via
The numerical fluxes in the x- and y- directions are respectively
and
and in a similar way for F2i+12,j(q) and F2i,j+12(q). Finally we incorporate a first-order Euler time integration for the u and w components. The diffusive terms are treated in an implicit form and an explicit form is used for the convective and reactive terms. The initial data are approximated by their cell averages,
To advance the numerical solution from tn to tn+1=tn+Δt, we use the following finite volume scheme: given un=(¯uni,j) and wn=(¯wni,j) for all cells Ii,j at time t=tn, the unknown values un+1 and wn+1 are determined by the following two steps implicit-explicit scheme:
Step 1 solve for p=(¯pi,j) and q=(¯qi,j)
Step 2 solve for un+1=(¯un+1i,j) and wn+1=(¯wn+1i,j)
where we have used the notation Δh=(Δi,j) to indicate the matrix of the discrete Laplacian operator, and I is the identity matrix.
Theorem 5.1. Suposse that f1(u,w)=u(αw−1) and f2(u,w)=βw(1−u−w) Then the solutions ¯pi,j, ¯qi,j and ¯un+1i,j, ¯wn+1i,j of the finite volume scheme (5.3a)–(5.3b) and (5.4a)–(5.4b) respectively, are nonnegatives for all i,j provided ¯uni,j, ¯wni,j are nonnegative for all i,j, and the following CFL-like condition is satisfied:
where
Proof. In (5.3a)–(5.3b), we have a linear system of algebraic equations for ¯pi,j and ¯qi,j which need to be solve in each time step tn. However, observe that the matrix of these linear systems are diagonally dominant, which guarantee the existence of solution and the positivity of pi,j and qi,j. Each system of equations (5.4a)–(5.4b) can be seen as a linear system for ¯un+1i,j and ¯wn+1i,j respectively, where the right side is positive according with the CFL condition (5.5) (see [26,27,28]) which guarantees the positivity of ¯un+1i,j and ¯wn+1i,j.
Each system of linear algebraic equations for ¯pi,j, ¯qi,j and ¯un+1i,j, ¯wn+1i,j can be solved by using an accurate and efficient linear algebraic solver.
5.1. Test 1: chasing and evasion
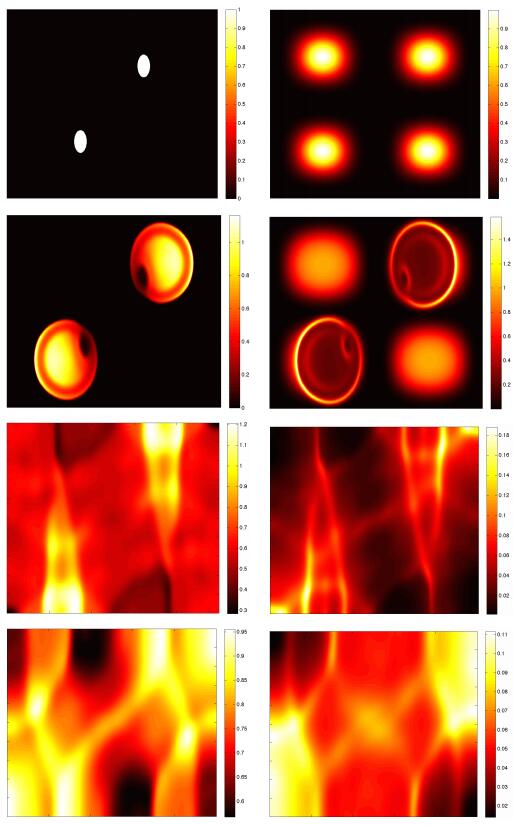
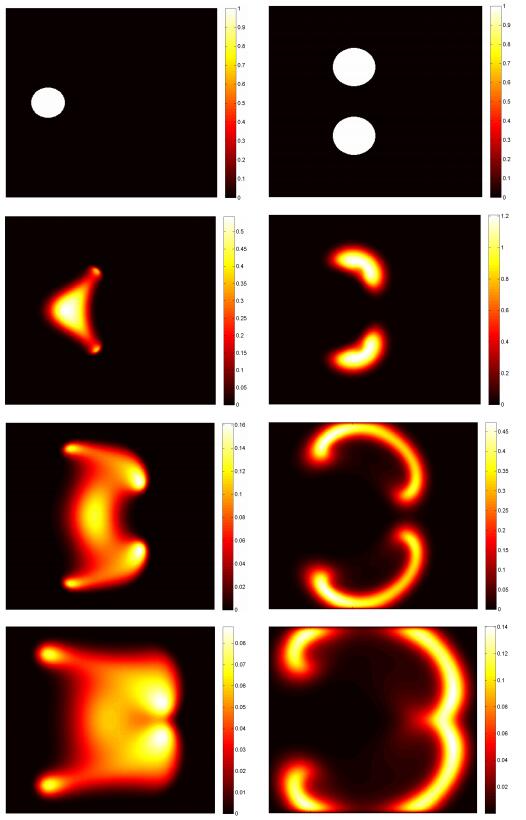
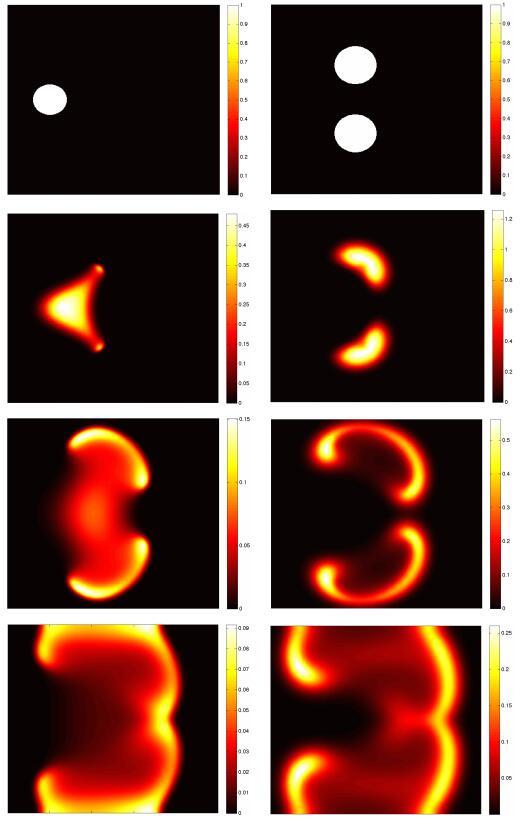
In this numerical test, shown in Figure 1, we suppress the terms on the right-hand side of (1.2), to ignore the population dynamics and emphasize the effect of the pursuit and evasion. We can see that the predator starts to chase the prey even though at first any direct contact with it would be very small (due to diffusion only). The prey takes evasive action immediately. Note that by choosing large δu,δw in this example, we see from Tables 1 and 2 that this may be interpreted as saying that the chemical sensitivity of the predator and prey are large compared to their diffusion rates. Therefore, we expect that the movement observed in Figure 1 is due to the attraction and repulsion terms and not so much to the diffusion. The numerical parameters are a 400 by 400 spatial cell grid, and a time step of 0.01.
5.2. Test 2: full dynamics
In this test, shown in Figure 2, we set some generic parameters in the system (1.2) in order to observe the full behavior. We can see now the predator-prey interaction taking place, as the densities of the two species fluctuate more widely in relation to the previous example, due to the predator's population growth from predation. After some time, the solution seems to exhibit wave-like interaction patterns with decreasing amplitudes, stabilizing around the values predicted by the equilibrium point (α−1α,1α)=(0.9,0.1). Although here we show the solution only until t=10, computation up to larger times, not shown here, confirm this behavior. The numerical parameters are a 400 by 400 spatial cell grid, and a time step of 0.01.
5.3. Test 3: Dirichlet boundary conditions
This test, which we show in Figure 3, implements a variant of the proposed method for system (1.2) with Dirichlet boundary conditions instead of the conditions (1.3). The parameters are the same as in Test 1. We can see that although the general dynamics remains similar, the boundary behavior affects the solution, in particular making the maximum density smaller. This in natural, since a Dirichlet condition corresponds, physically, to an absorption, or death, at the boundary.
One can also see, especially on the prey column, the formation of steep gradients along the border (boundary layers). This, again, is natural, since with the parameters of the simulation, the evasion dynamics of the prey should be dominant. Since evasion corresponds in (1.2) to an advection term, the formation of boundary layers when considering Dirichlet conditions is to be expected.
6.
Conclusions and outlook
In this work, we have studied analytically and numerically a system of parabolic-elliptic PDEs modeling predator-prey dynamics including prey- and predator-taxis, indirect detection by means of a "potential" or odor, diffusion, and Lotka–Volterra dynamics. We have seen that the system is well posed and established boundedness properties of the solution in Lebesgue spaces. Although these purely mathematical properties may not be of immediate biological relevancy, the reasonings and results establish that the model does not lead to non-biological phenomena such as blow-up in finite time – this being by no means a given property of systems of parabolic equations.
The Lotka–Volterra dynamics with logistic term featured in the right-hand side of our system was chosen since it is the simplest model not leading to unrealistic population explosions. Therefore, possible extensions of our work include the question of whether the results still hold for more realistic population dynamics, namely Holling-type, or after the inclusion of Allee effects, prey handling time, etc.
One other obvious extension to the system (1.2) would be to consider a fully parabolic system where the odor diffusion time scale is of the same order than the population diffusion time scale. In that case, the analysis is more involved. We plan to address this question in future work.
Acknowledgements
P.A. was partially supported by Faperj "Jovem Cientista do Nosso Estado" grant no. 202.867/2015, Capes by PRONEX, and CNPq grant no. 442960/2014-0. B.T. acknowledges the support from Capes via a doctoral grant from the Institute of Mathematics of UFRJ. L.M.V. was supported by CONICYT/PIA/Concurso Apoyo a Centros Científicos y Tecnológicos de Excelencia con Financiamiento Basal AFB170001. The authors would also like to thank the anonymous referee for their insightful comments.
Conflict of interest
The authors declare there is no confict of interest.
Appendix
The dimensional version of system (1.2) reads as follows:
supplemented with appropriate no-flux boundary conditions similar to (1.3).
In Table 1 we present the physical meaning of the parameters appearing in (6.1), and in Table 2 we show the dimensionless parameters appearing in system (1.2), obtained after a standard non-dimensionalization procedure applied to the system (6.1).









 DownLoad:
DownLoad: