Analysis of a generalized Fujikawa’s growth model

1.   Introduction

Growth models have traditionally been used for representing the time course of microbial concentration ^[1,2,3]. Also, there have been applications for representing the time course of other variables, namely: Colony diameter ^[4,5,6,7]; tumor size ^[8]; weight of plant seedlings ^[9]; dry weight of wheat grains ^[10]; cumulative epicotyl emergence (germination) of oaks ^[11]; body weight of Boer goats ^[12], weight of pigs ^[13], size of Desinognathus ^[14], pressure variation ^[15], among others.

The quality of growth models fitting may be assessed in terms of its capability for representing the slope at the inflection point ($k$) and the duration of the lag phase ($t_{lag}$), rather than the squared error (SSE) ^[16]. The lag phase duration indicates the time that the exponential phase begins. The inflection point characterizes the shape of the curve, and it allows to determine the maximum slope of the growth curve and the lag phase duration via the classical approach. In turn, the maximum growth rate is a measure of the growth speed during the exponential phase. Particularly, in the study of growth of undesired microorganisms on products, the lag phase duration indicates the initial time of the exponential phase of contaminants, and the maximum growth rate indicates its speed of growth ^[16,17].

One way to have growth models capable of generating the lag phase is through a differential equation, including a multiplicative term that suppresses the vector field for low values of the population size, i.e. during the lapse after the initial time. In the model proposed by Baranyi, Roberts and McClure ^[1], a time dependent adaptation function called adjustment function ($\alpha_t$) is incorporated into a logistic-type model, yielding a non-autonomous model and achieving satisfactory representation of the lag phase. However, autonomous differential equations also have a significant capability for representing the lag phase and the inflection point, some examples are: The Verhulst's logistic equation ^[18], the generalized Gompertz function ^[19], the Putter-Bertalanffy model ^[20], the generalized logistic model ^[19], the autonomous neoclassical model of Vadasz, Vadasz ^[21], and the model of Fujikawa, Kai, Morozumi ^[16]. Also, they allow performing equilibrium stability analysis ^[20]. Additional discussion is presented in section 2.

It is worth noticing that Tsoularis and Wallace ^[19] and Ohnishi, Yamakawa and Akamine ^[20] determined the analytical expression for the population at the inflection point, and assessed the effect of exponential parameter values on the existence or absence of the inflection point and lag phase, based on the curve of the growth rate versus population size. Tsoularis and Wallace ^[19] noticed that there is no logarithmic inflection point for certain parameter values. Also, they determined the limit values of the population at the inflection point for parameter values approaching zero or infinite. Ohnishi, Yamakawa and Akamine ^[20] observed that negative parameter values also lead to inflection point and lag phase, thus representing real life behavior. In contrast to these studies, in this work we consider the generalized Fujikawa's model which included the function $f_c = (1-N_{min}/N)^c$ that characterizes the original Fujikawa's model, the power law function $N^{\alpha}$ and a saturation term. We assess the effect of exponential parameters on the growth curve, considering separately the population $N$ and the logarithmic population $y = \ln(N/N_{to})$, with negative and positive parameter values. Indeed: ⅰ) we determine the ranges of parameter values that lead to existence or absence of inflection point, and those for the existence or absence of lag phase; ⅱ) we assess the effect of different combinations of positive and negative parameter ranges on the existence or absence of inflection point and lag phase; ⅲ) we determine the parameter ranges that lead to monotonic decreasing behavior of the slope of the growth rate curve; ⅳ) we propose an approach for the analytical determination of the lag phase duration. Numerical simulations illustrate the approach. In summary, the main contribution of this work is the determination of the capability of a generalized Fujikawa's model to capture all the features of real life monotonic growth behavior, including lag phase and inflection point.

The organization of the study is as follows. Section 2 presents some Preliminaries on the study of growth models. Section 3 presents the proposed model and the properties of the adaptation function. Section 4 presents the features of the regular growth model: Convergence and boundedness properties; determination of the regular inflection point and conditions for its existence; effect of model parameters on the existence of the inflection point and the lag phase; and properties of the regular inflection point, including population size of the inflection point under limiting values of the model parameters, and parameter values that lead to inflection point located at the mean value of the curve. Section 5 presents the features of the logarithmic growth model: Convergence and boundedness properties; determination of the logarithmic inflection point and conditions for its existence; effect of model parameters on the existence of the inflection point and the lag phase; and properties of the logarithmic inflection point, including population size of the logarithmic inflection point under limiting values of the model parameters, and parameter values that lead to inflection point located at the mean value of the curve. In section 6, an approach is proposed for determining the lag phase duration, for each the regular and the logarithmic growth curves. In section 7 the model is applied to experimental data, what includes the arrangement of the model and its properties in terms of the logarithmic measure, and a numerical fitting example. Finally, in section 8 the conclusions are drawn.

2.   Preliminaries

2.1.   Growth models study

In microbial culture studies, a typical monotonic growth curve comprises the following phases: Lag phase, exponential growth phase, and stationary phase, although the lag phase may not appear. Thus, monotonic growth does not comprise decay phase or oscillations ^[17,21,22]. The lag phase involves the physiological adaptation of microbial cells to the new environment, resulting in low or negligible growth rate. The exponential growth phase involves exponential reproduction of microorganisms. The stationary phase comprises constant population of microorganisms ^{[16,22,23,24]}. The maximum growth rate occurs in the exponential growth phase, at the so-called inflection point ^[17,24,25].

In growth model studies for microbial count data (cfu/mL), the logarithmic measure defined as $y = \ln(N/N_{to})$ or $y = \ln(N)$, is used as dependent variable for curve fitting and determination of the inflection point and lag phase duration ^[2,26,27].

The inflection point is characterized by the fact that the derivative of the dependent variable is maximum (the growth rate is maximum) ^[19,21]. The existence of the inflection point requires a convex shape in the growth rate versus population curve ^[17,25]. In turn, the existence of the lag phase requires the existence of the inflection point, and it is possible that the inflection point exists while the lag phase does not. In autonomous growth models the lag phase is generated if the initial population size ($N_{to}$) is sufficiently close to the unstable equilibrium point, thus yielding small value of the initial growth rate ($\left. dN/dt\right \rvert_{t = to}$). In this case, the escaping of the population from the neighborhood of the unstable equilibrium point takes more time ^[17,21].

The lag phase duration can be quantified via the classical approach, or based on the third derivative (maximum acceleration of the growth rate) or the maximum curvature ^[18,24]. The classical approach is based on the intersection of the tangent line at the inflection point with the horizontal line that meets the initial point ^[17,24].

2.2.   The model of Vadasz and Vadasz

The model of Vadasz and Vadasz ^[17,21] comprises a quadratic term, which defines a stable and an unstable equilibrium point. Certain values of the model parameters, corresponding to a specific region, render the model capable of representing the inflection point and the lag phase. For this parameter region, the value of the stable equilibrium point is higher than the unstable one, both being positive. The stable equilibrium point is the carrying capacity. The state variable behaves monotonically, escaping from the unstable equilibrium point towards the stable one. In addition, the model showed a high capacity to represent the lag phase and the inflection point for experimental data.

2.3.   The generalized logistic model

The generalized logistic model proposed by Tsoularis and Wallace ^[19] is:

Where $r$, $\alpha$, $\beta$, $\gamma$, $N_{max}$ are positive constants, $N$ is the population size, and $N_{max}$ is the limit value, usually called carrying capacity. This model combines the function $N^{\alpha}$, $\alpha > 0$, with the logistic type model.

Therein, model simulations with $\alpha = 1.5$ and $\alpha = 3$ indicated their capability for representing the lag phase. In addition, several growth models that are capable of representing the lag phase can be expressed in this form, namely: The Korf’s form, the hyperbolic form for regenerative growth, the Smith’s equation and the Richard model ^[19,25]. Moreover, the Smith’s equation has achieved satisfactory representation of the lag phase for experimental data ^[9].

2.4.   The Putter-Bertalanffy model

The Putter-Bertalanffy model analyzed by ^[20], can be expressed as

where $r$, $N_{max}$ and $\gamma$ are positive constants, and $N$ is the population size. It is a Savageau's model, which is in turn a particular case of the generalized logistic function analyzed by ^[19]. The possible ranges of $(1-ar)$, either positive, negative or zero, lead to different possibilities of the existence of the inflection point and the lag phase.

2.5.   Fujikawa's model

The model of Fujikawa, Kai and Morozumi is ^[16,28]:

where $N$ is the population size, $r$, $c$, $N_{min}$, $N_{max}$ are positive constants, $N_{max} > N_{min}$ and $N_{to} \in (N_{min}, \, \, N_{max})$. This model was constructed by adding the function $f_c = (1-N_{min}/N)^c$ to the logistic model

The function $f_c$ strongly suppresses the growth rate during the lag phase, whereas the term $(1-N/N_{max})$ strongly suppresses the growth rate during the stationary phase. The $f_c$ function is not mechanistically inspired. Fujikawa, Kai and Morozumi fitted the new model and the traditional model of Baranyi and Roberts ^[29] for data of E. coli culture. The $t_{lag}$ of the new model was similar to that of the Baranyi’s model, although slightly less ascertained. In contrast, the slope ($k$) was more ascertained than that of Baranyi’s model. In the fitting, it was found that a small $(N_{to} - N_{min})$ value is necessary for the estimated $c$ being less dependent on initial population. To this end, the value $N_{min} = (1-1\times 10^{-6})N_{to}$ was used, yielding appropriate representation of the lag phase

3.   Proposed model and basic properties

3.1.   Statement of the generalized Fujikawa's model

We propose the following modification of the Fujikawa’s model:

where $r$, $\alpha$, $\gamma$, $c$, $N_{min}$, $N_{max}$ are constants, and $r > 0$, $N_{max} > N_{min} > 0$; $N$ is the population size, and $N_{max}$ is the carrying capacity. In turn, the model (3.1) combines the term $f_c = (1-N_{min} /N)^c$ with the hyperlogistic function:

The difference of model (3.1) with the Fujikawa’s model (2.4) is the exponents $\alpha$ and $\gamma$. Indeed, Fujikawa’s model can be regarded as a simplified variant corresponding to $\alpha = 1$, $\gamma = 1$. The model coefficients are assumed constant in order to facilitate the study of model properties. Despite this assumption, the model parameters can be defined as function of growth variables in further studies.

Remark 3.1. The term $f_c = (1-N_{min}/N)^c$ with $c > 0$ slows the growth rate of the population size $N$ during the lag phase, provided small positive $(N_{to} – N_{min})$. In turn, this is related to the fact that $\left.dN/dt\right \rvert_{N = N_{to}}$ is proportional to $(N_{to} – N_{min})^{c}$. In contrast, a $c < 0$ value and a positive small $(N_{to} – N_{min})$ value imply: i) $\left. dN/dt\right \rvert_{N = N_{to}}$ values are high, so that the initial time period of the $N$ versus $t$ curve features large slope; ii) $\left.(1/N)(dN/dt)\right \rvert_{N = N_{to}}$ values are large, so that the initial time period of the $y$ versus $t$ curve features large slope.

Remark 3.2. In the generalized logistic form of Tsoularis and Wallace ^[19], Eq (2.1), and in the hyperlogistic form (3.2), the suppression of the growth rate during the lag phase is due to the term $N^{\alpha}$, with $\alpha > 0$, whereas in the proposed model (3.1), it is due to the term $f_c$ with $c > 0$.

Remark 3.3. The $c = 0$ value implies absence of the $f_c$ term in model (3.1), so that it reduces to the hyperlogistic model (3.2), which was studied by Tsoularis $&$ Wallace ^[19].

Remark 3.4. The shape of the growth curve and the existence of the lag phase are related to the equilibrium points of the system. The term $N^{\alpha}$, $\alpha > 0$ generates the equilibrium point $N^{eq} = 0$, whereas the term $(1-N/N_{max})^{\gamma}$, $\gamma > 0$ yields $N^{eq} = N_{max}$; and the term $(1-N_{min}/N)^c$, $c > 0$ yields $N^{eq} = N_{min}$. For $\gamma > 0$, $c > 0$ and $N_{to} > N_{min}$, the $N$ versus $t$ curve escapes from the equilibrium $N^{eq} = N_{min}$ and approaches equilibrium $N^{eq} = N_{max}$. The lag phase occurring at $N \approx N_{min}$ is related to a trajectory that begins close to $N_{min}$, so that it takes more time near $N_{min}$. Therefore, the existence of the lag phase occurring at $N \approx N_{min}$ requires: i) the existence of the equilibrium point $N^{eq} = N_{min}$, what in turn implies $c > 0$; ii) a $N_{to}$ value such that $N_{to} - N_{min}$ is small positive.

At what follows, we state the definitions of the growth rate curve related to the mean between lower and higher populations. The mean between $N_{min}$ and $N_{max}$ is:

The logarithmic population $y$, and its minimum and maximum values are defined as:

As a consequence of the above definitions, $\left. y\right \rvert_{t = to} = 0$. The mean between $y_{min}$ and $y_{max}$ is:

Substituting the definition of $y_{min}$ and $y_{max}$, that is, Eqs (3.5) and (3.6), into $y_{m}$, gives:

To obtain the value of $N$ corresponding to $y = y_m$, we use the definition $y = \ln(N/N_{to})$, what gives:

3.2.   Properties of the adaptation function

The adaptation function $f_c = (1 - N_{min}/N)^c$, $c > 0$, has the following properties:

Remark 3.5. Property ii implies that $f_c$ is monotonically increasing with respect to $N$, for $N \in (N_{min}, \, \, N_{max}]$

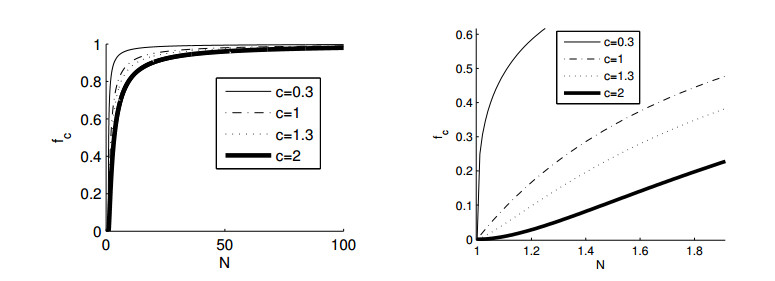
Remark 3.6. Properties ii, iii and iv imply that $f_c$ is lower for high $c$ values and for small positive values of $(N - N_{min})$. As a consequence, the $dN/dt$ value is suppressed for small positive values of $(N_{to} - N_{min})$.

Property $iv$ follows from:

Moreover, $f_c$ exhibits an inflection point with the following features:

Remark 3.7 The inflection point of $f_c$ leads to a steep change of the $f_c$ versus $N$ curve; and consequently a steep change of the $dN/dt$ versus $t$ curve for $c > 1$.

Figure 1 shows the $f_c$ versus $N$ curve for $N_{min} = (1 - 1 \times 10^{-6}) N_{to}$, $N_{to} = 1$ and several $c$ values.

4.   Features of the regular growth model

Proposition 4.1. Boundedness and convergence. Consider the growth model (3.1) with $\gamma > 0$, real values of $\alpha$ and $c$, and $N_{max} > N_{min} > 0$. If $N_{to} \in (N_{min}, \, \, N_{max})$, the population size $N$ exhibits the following properties: i) $N$ increases monotonically from $N = N_{to}$ to $N_{max}$, so that $\lim_{t \rightarrow \infty} N = N_{max}$; ii) $N \in [N_{to}, \, \, N_{max}) \, \, \, \forall t \geq to$.

Proof. The above proposition follows from the values of the growth rate $dN/dt$: ⅰ) $dN/dt = 0$ for $N = N_{max}$; ⅱ) $dN/dt > 0$ for $N \in (N_{min}, \, \, N_{max})$.

4.1.   Determination of the regular inflection point and existence conditions

In this study, the regular inflection point ($t^{\#}$, $N^{\#}$) is defined as the point of the $N$ versus $t$ curve where $dN/dt$ is maximum, being $\left. dN/dt\right \rvert_{N = N^{\#}}$ the maximum growth rate.

Proposition 4.2. Consider the model (3.1) with $\gamma > 0$; real values of $\alpha$ and $c$; $N_{max} > N_{min} > 0$; and $N_{to} \in (N_{min}, \, \, N_{max})$. With these conditions the regular inflection point population, $N^{\#}$, is provided by:

Proof. As the regular inflection point corresponds to a maximum of the $dN/dt$ versus $t$ curve, then

The condition $N_{to} \in (N_{min}, \, \, N_{max})$ implies that $N \in (N_{min}, \, \, N_{max}) \, \, \ \forall t \geq t_o$, according to Proposition 4.1. The condition $\left. dN/dt\right \rvert_{N = N^{\#}} > 0$ implies that $N^{\#} \neq N_{max}$. The above two results imply $N^{\#} \in (N_{min}, \, \, N_{max})$. This result and Eq (4.2) give

finally, applying condition (4.3) for the growth model (3.1), yields Eq (4.1).

Remark 4.1. The condition (4.3) implies that $N^{\#}$ corresponds to a maximum of the $dN/dt$ versus $N$ curve.

Proposition 4.3. Consider growth model (3.1) with real values of $\alpha$, $c$ and $\gamma$, $N_{max} > N_{min} > 0$ and $N_{to} \in (N_{min}, \, \, N_{max})$. If $N^{\#} < N_{to}$, then $N > N^{\#} \, \, \, \forall t \geq t_o$; that is, the $N$ versus $t$ curve exhibits no inflection point. If $N^{\#} = N_{to}$, then $N = N^{\#}$ for $t = t_o$; that is, the regular inflection point occurs at $t = t_o$, $N = N_{to}$.

Proof. Since the $N$ versus $t$ curve is monotonic and $N \geq N_{to} \, \ \forall t \geq t_o$, then $N > N^{\#} \, \, \forall t \geq t_o$ if $N^{\#} < N_{to}$.

Remark 4.2. Consider growth model (3.1), with $\gamma > 0$ and real values of $\alpha$ and $c$, and $N_{max} > N_{min} > 0$. The existence of the inflection point in the $N$ versus $t$ curve requires: i) the existence of a maximum in the $dN/dt$ versus $N$ curve, which is given by Eq (4.1), subject to $N^{\#} \in (N_{min}, \, \, N_{max})$, and ii) that $N_{to} < N^{\#}$ where $N_{to} > N_{min} > 0$. The existence of the lag phase requires the existence of the inflection point and a small value of $\left. dN/dt\right \rvert_{t = to}$. In turn, this implies a positive $c$ value and a small positive $(N_{to} - N_{min})$ value.

Proposition 4.4. Consider growth model (3.1) with real values of $\alpha$, $c$, $\gamma$, and $N_{max} > N_{min} > 0$ and $N_{to} \in (N_{min}, \, \, N_{max})$:

i) if

holds, then the $dN/dt$ versus $N$ curve exhibits a maximum at $N = N^{\#}$, $N^{\#} \in (N_{min}, \, \, N_{max})$.

ii) the inflection point population, $N^{\#}$, satisfies:

iii) if either

holds, the $dN/dt$ versus $t$ curve is monotonic decreasing for $N \in (N_{min}, \, \, N_{max})$.

Proof. Model (3.1) with $N_{max} > N_{min} > 0$ subject to parameter regime (4.4) gives:

Therefore, the $dN/dt$ versus $N$ curve exhibits a maximum at $N = N^{\#}$, with $N^{\#} \in (N_{min}, \, \, N_{max})$.

In addition, we notice from Eq (4.1) that the inflection point population $N^{\#}$ is provided by $\left. D_2 \right \rvert _{N = N ^{\#}}\; = 0$, where

The $D_2$ term exhibits vertical asymptotes at $N = N_{min}$ and at $N = N_{max}$. In addition, if parameter regime (4.4) holds, then

so that there is at least one $N$ value that belongs to range $(N_{min}, \, \, N_{max})$ and leads to $D_2 = 0$. Then, there exists a maximum in the $dN/dt$ versus $N$ curve, at $N = N^{\#}$, $N^{\#}$ provided by Eq (4.6), and $N^{\#} \in (N_{min}, \, \, N_{max})$.

For the second part, we recall that for model (3.1) with $N_{to} \in (N_{min}, \, \, N_{max})$, the inflection point of the $N$ versus $t$ curve satisfies Eq (4.2). As the inflection point corresponds to a maximum of the $dN/dt$ versus $t$ curve, then

according to ^[30]. Equivalently,

As $dN/dt > 0$ for $N \in (N_{min}, \, \, N_{max})$, real values of $\alpha$, $c$, $\gamma$ and $N_{max} > N_{min} > 0$, then the conditions $t < t^{\#}$, $t = t^{\#}$ and $t > t^{\#}$ are equivalent to $N < N^{\#}$, $N = N^{\#}$ and $N > N^{\#}$, respectively, for $N \in (N_{min}, \, \, N_{max})$. Therefore, expressions (4.13) lead to

For model (3.1), and $N \in (N_{min}, \, \, N_{max})$, we have

This and expressions (4.14) lead to

This and condition $N \in (N_{min}, \, \, N_{max})$ result in second part of Proposition.

For the third part, we notice that if $\gamma > 0$, and (4.7) holds, then

This and Eq (4.15) lead to

As $dN/dt > 0$ for $N \in (N_{min}, \, \, N_{max})$, then

thus $d^2 N / dt^2 < 0$ for $N \in (N_{min}, \, \, N_{max})$. Equivalently, the $dN/dt$ versus $t$ curve is decreasing.

Remark 4.3. In first part of Proposition 4.4 not only the values belonging to the parameter regime (4.4) lead to existence of a maximum in the $dN/dt$ versus $N$ curve. Indeed, certain negative $c$ values with $\gamma > 0$ also lead to a maximum. The third part of Proposition 4.4 corresponds to the parameter conditions for the absence of a maximum in the $dN/dt$ versus $N$ curve, hence absence of the regular inflection point.

4.2.   Effect of model parameters on the existence of the regular inflection point and lag phase

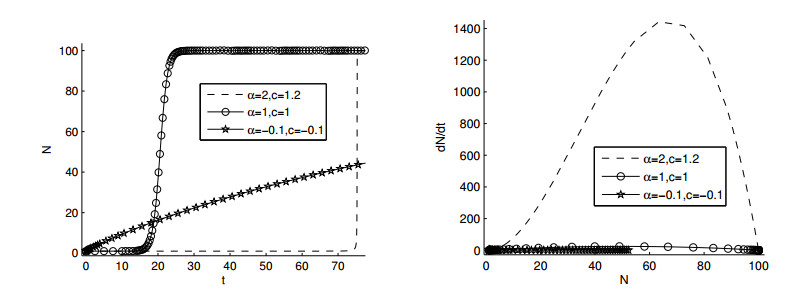
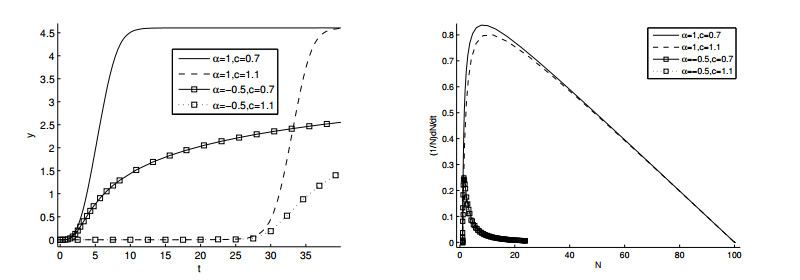
At what follows, the effect of model parameters on the existence of the inflection point and lag phase is assessed, on the basis of the growth rate versus population size. The effect of model parameters on the $dN/dt$ vs $N$ curve and the $N$ vs $t$ growth curve for the model (3.1) is illustrated in Figures 2–4. The following parameter values are used: $N_{max} = 100$, $\gamma = 1$, $N_{min} = (1-1\times 10^{-6})N_{to}$, $N_{to} = 1$. As the advantage of the proposed model is its capacity to represent the lag phase, it is assumed that $\gamma$ is positive, and its effect is not assessed. There are four different cases of the $\alpha$, $c$ values, yielding different possibilities of the existence of the lag phase and the inflection point:

● $\alpha > 0$, $c > 0$: Pⅰ) $dN/dt = 0$ for $N = N_{min}$; Pⅱ) $dN/dt = 0$ for $N = N_{max}$; Pⅲ) $dN/dt > 0$ for $N \in (N_{min}, \, \, N_{max})$. The first part of Proposition 4.4 applies, so that the inflection point occurs provided $N_{to} < N ^{\#}$ and the lag phase existence depends on the $(N_{to} - N_{min})$ value. Simulations are shown in Figure 2.

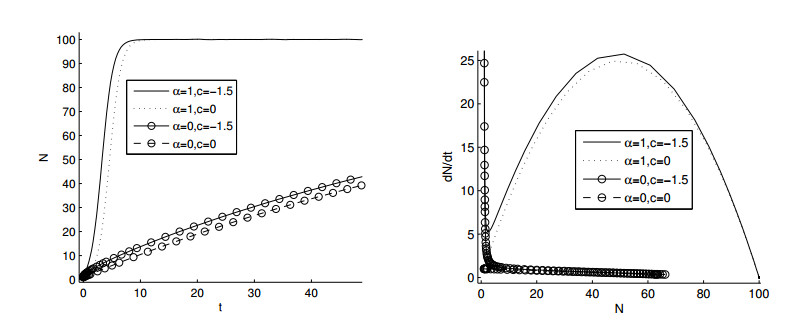
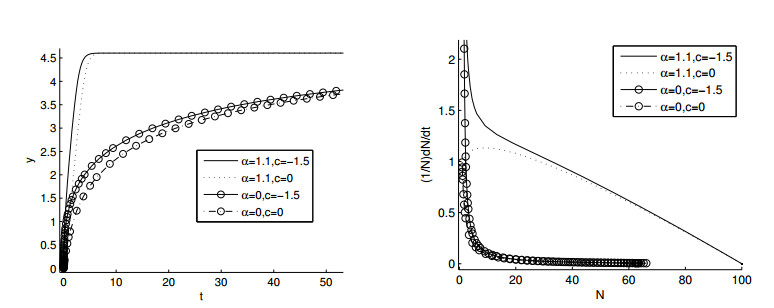
● $\alpha > 0$ and $c < 0$: Pⅰ) $dN/dt = \infty$ for $N = N_{min}$, that is, there is a vertical asymptote at $N = N_{min}$; Pⅱ) $dN/dt = 0$ for $N = N_{max}$; Pⅲ) $dN/dt > 0$ for $N \in (N_{min}, \, \, N_{max})$. Property Pi implies that $\left. dN/dt\right \rvert_{N = N_{to}}$ is overly high for low values of $(N_{to} - N_{min})$, so that there is no lag phase. The occurrence of the inflection point is determined by Eq (4.6) subject to $N^{\#} \in (N_{min}, \, \, N_{max})$, provided $N_{to} < N^{\#}$. The third part of Proposition 4.4 indicates that for $c \leq - \alpha (N_{max}/N_{min} - 1)$ values, there is no inflection point. Moreover, simulations show that for some parameter values there is a local minimum and a local maximum in the range $N \in (N_{min}, \, \, N_{max})$. As a consequence, there may be inflection point but there is no lag phase. Simulation shown in Figure 3 confirm the above facts, and also shows that the inflection point is owed to the term $N^{\alpha}$.

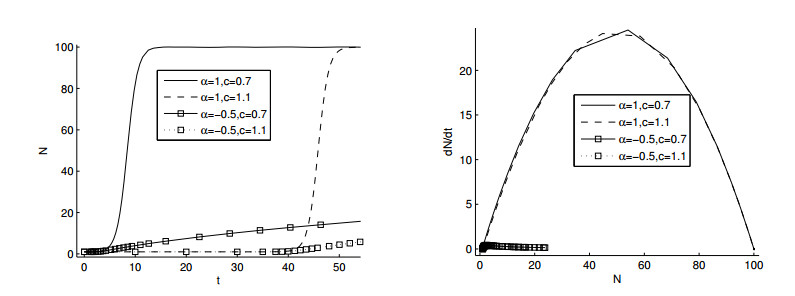
● $\alpha < 0$ and $c > 0$: The properties Pi to Piv of case $\alpha > 0$, $c > 0$ are fulfilled. The first part of Proposition 4.4 applies, so that the inflection point occurs provided $N_{to} < N ^{\#}$, and the lag phase existence depends on the $(N_{to} - N_{min})$ value. Simulation shown in Figure 4 confirms the above facts, and also show that: ⅰ) the effect of negative values of $\alpha$ on the lag phase duration is low; ⅱ) the maximum growth rate is overly low, so that the slope of the population at the exponential growth phase is overly low. This confirms the importance of the $N^{\alpha}$ term for the slope of the exponential growth phase.

● $\alpha < 0$ and $c < 0$: Pⅰ) $dN/dt = \infty$ for $N = N_{min}$, that is, there is a vertical asymptote at $N = N_{min}$; Pⅱ) $dN/dt = 0$ for $N = N_{max}$; Pⅲ) $dN/dt > 0$ for $N \in (N_{min}, \, \, N_{max})$. Proposition 4.4 applies, so that the $dN/dt$ versus $N$ curve is monotonically decreasing for $N \in (N_{min}, \, \, N_{max})$. Thus, no inflection point occurs, and therefore no lag phase occurs. Simulations in Figure 2 confirm the above properties.

Intermediate cases are:

● $\alpha > 0$ and $c = 0$: The term $f_c$ is absent, so that the model becomes $dN/dt = r N^{\alpha} (1-N/N_{max})^{\gamma}$, and: Pⅰ) $dN/dt > 0$ for $N = N_{min}$; Pⅱ) $dN/dt = 0$ for $N = N_{max}$; Pⅲ) $dN/dt > 0$ for $N \in (N_{min}, \, \, N_{max})$; Piv) $\left. dN/dt\right \rvert_{N = N_{to}}$ is positive and it is proportional to $N_{to}^{\alpha}$. Thus, the $dN/dt$ versus $N$ curve exhibits a maximum with $N^{\#} \in (N_{min}, \, \, N_{max})$, so that there is an inflection point provided $N_{to} < N^{\#}$, and it is owed to the term $N^{\alpha}$ with $\alpha > 0$. For general (not small) values of $N_{to}$, $\left. dN/dt \right \rvert_{t = to}$ is not small, so that there is no lag phase. Simulations are shown in Figure 3.

● $\alpha = 0$ and $c < 0$: The term $N^{\alpha}$ is absent, and the properties are similar to those of case $\alpha < 0$ and $c < 0$: Pⅰ) $dN/dt = \infty$ for $N = N_{min}$, that is, there is a vertical asymptote at $N = N_{min}$; Pⅱ) $dN/dt = 0$ for $N = N_{max}$; Pⅲ) $dN/dt > 0$ for $N \in (N_{min}, \, \, N_{max})$. According to Proposition 4.4, the $dN/dt$ versus $N$ curve exhibits monotonic decreasing behavior, so that no inflection point occurs, and therefore lag phase neither occurs, what is confirmed by simulation in Figure 3.

● $\alpha = 0$ and $c > 0$: The term $N^{\alpha}$ is absent, and the properties Pi to Piv of case $\alpha < 0$ and $c > 0$ are fulfilled. The first part of Proposition 4.4 applies, so that an inflection point occurs provided $N_{to} < N^{\#}$ and the lag phase existence depends on the $(N_{to} - N_{min})$ value. Although simulations are not shown, they indicate that the maximum occurs at low values of the population size ($N^{\#}$ is low), and the maximum growth rate is overly low, so that the slope of the growth curve at the exponential growth phase is overly low.

Remark 4.4. The above cases of $\alpha$ and $c$ values indicate that the $c$ parameter has a significant influence on the lag phase duration but the influence of the $\alpha$ value is low. Concerning the slope at the exponential growth phase, the $\alpha$ value has a significant influence, whereas the influence of the $c$ value is low.

Remark 4.5. The parameter region $\alpha < 0$ leads to low slope of the population at the exponential growth phase, whereas $c < 0$ implies that no lag phase occurs. Thus, the condition $c > 0$ is required for representing the lag phase, whereas $\alpha > 0$ is required for higher slopes of the population at the exponential growth phase. This is in accordance with the fact that the formulation of the adaptation function $f_c$ by Fujikawa, Kai and Morozumi ^[16] aimed at suppressing the growth rate during the lag phase, but without affecting the rest of the population growth behavior.

4.3.   Properties of the regular inflection point

Proposition 4.5. Consider the model (3.1) with $\gamma > 0$. The population at the inflection point, $N^{\#}$, is defined by:

where

And the discriminant is

so that no imaginary solutions are possible for $\gamma c \geq 0$. If $\bar{a} > 0$, $c > 0$, and $\bar{b}^2 – 4 \bar{a} \bar{c}$ is non-negative, only the positive square root can lead to $N^{\#} > N_{min}$.

Proof. The population at the regular inflection point is obtained by applying Eq (4.3) to the growth model (3.1), leading to

Rearranging yields the quadratic Eq (4.20). In order to determine whether the negative square root term can lead to $N^{\#} > N_{min}$ for $\bar{a} > 0$, we use Proposition presented in Appendix B. Using the definition of $\bar{a}$, $\bar{b}$, $\bar{c}$, we have: $\bar{c} + \bar{a} + \bar{b} = (-1) c (N_{max}/N_{min} - 1)$. Since $(N_{max}/N_{min}) > 1$, then $\bar{c} + \bar{a} + \bar{b} < 0$ for $c > 0$, so that the condition $\bar{c} + \bar{a} + \bar{b} > 0$ is not fulfilled for $c > 0$. Consequently, only the positive square root term leads to $N^{\#} / N_{min} > 1$. End of the proof.

Proposition 4.6. Limit values of $N^{\#}$. Consider model (3.1), with $\alpha > 0$, $\gamma > 0$, $c \geq 0$. The limit values of the regular inflection point, $N^{\#}$, are:

Proof. The above limit values are obtained by substituting $\gamma \rightarrow 0$, $\gamma \rightarrow \infty$, $c \rightarrow 0$, $c \rightarrow \infty$, $\alpha \rightarrow 0$, $\alpha \rightarrow \infty$ in Eq (4.23), and solving for $N^{\#}$.

Remark 4.6. The above proposition indicates that the limit values of $N^{\#}$ for $\alpha \rightarrow 0$ depends on the values of $c$ and $\gamma$; whereas the limit value of $N^{\#}$ for $c \rightarrow 0$ depends on the values of $\alpha$, $\gamma$.

Proposition 4.7. Conditions for $N^{\#} = N_{to}$. Consider the model (3.1). The population at the regular inflection point, $N^{\#}$, satisfies: $N^{\#} = N_{to}$ for

Proof. Using $N^{\#} = N_{to}$ in Eq (4.23) and arranging yields Eq (4.25).

Remark 4.7. The above proposition implies that in the $N$ versus $t$ curve, with $N_{to} \in (N_{min}, \, \, N_{max})$, the maximum $(dN/dt)$ occurs at $t = t_o$, $N = N_{to}$, if the $\alpha$, $\gamma$, $c$ values are such that Eq (4.25) holds true.

Proposition 4.8. Conditions for $N^{\#} = N_{mn}$. Consider model (3.1). The population at the regular inflection point, $N^{\#}$, satisfies $N^{\#} = N_{mn}$ for

Proof. Using $N^{\#} = N_{mn}$ in Eq (4.23) and arranging yields Eq (4.26).

Remark 4.8. The above proposition implies that in the $N$ versus $t$ curve, with $N_{to} \in (N_{min}, \, \, N_{mn})$, the maximum $dN/dt$ occurs at $N = N_{mn}$ where $N_{mn} = (N_{min}+N_{max})/2$, if the $\alpha$, $\gamma$, $c$ values are such that Eq (4.26) holds true.

Remark 4.9. The determination of the lag time of the regular growth curve via the classical approach requires the determination of the inflection point, as shown in Appendix A. To this end, the population at the inflection point ($N^{\#}$) can be computed using expressions (4.20), (4.21), and the inflection time $(t^{\#})$ must be computed numerically.

5.   Features of the logarithmic growth model

Proposition 5.1. Boundedness and convergence. Consider growth model (3.1) with $\gamma > 0$ and real values of $\alpha$, $c$. If $N_{to} \in (N_{min}, \, \, N_{max})$, equivalently $y_{to} \in (y_{min}, \, \, y_{max})$, then $y_{min} < 0 < y_{max}$, $y_{to} \in (y_{min}, y_{max})$ and the logarithmic population $y$ exhibits the following features: i) $y$ increases monotonically from $y = y_{to}$ to $y = y_{max}$, so that $\lim y _{t \rightarrow \infty} = y_{max}$; ii) $y \in [y_{to}, \, \, y_{max}) \, \, \, \forall t \geq to$.

Proof. For $y = \ln(N/N_{to})$ and the growth model (3.1), with $\gamma > 0$ and real values of $\alpha$, $c$, the specific growth rate $dy/dt = (1/N)(dN/dt)$ fulfills the following properties: ⅰ) $(dy/dt) = 0$ for $N = N_{max}$; ⅱ) $(dy/dt) > 0$ for $N \in (N_{min}, \, \, N_{max})$.

5.1.   Determination of the logarithmic inflection point and existence conditions

In this study, the logarithmic inflection point ($t^{\ast}$, $N^{\ast}$, $y^{\ast}$) is defined as the point of the $y$ versus $t$ curve where $dy/dt$ is maximum, being $[dy/dt]_{y^{\ast}}$ the maximum specific growth rate; and $N^{\ast}$ the value of $N$ corresponding to $y^{\ast}$, ie $N^{\ast} = N_{to} e^{y^{\ast}}$.

Proposition 5.2. Consider the growth model (3.1) with $\gamma > 0$; real values of $\alpha$, $c$; $N_{max} > N_{min} > 0$; and $N_{to} \in (N_{min}, \, \, N_{max})$, equivalently $y_{to} \in (y_{min}, \, \, y_{max})$: The logarithmic inflection point population, $N^{\ast}$, is provided by

Proof. As the logarithmic inflection point corresponds to a maximum of the $dy/dt = (1/N) (dN/dt)$ versus $t$ curve, then

The condition $N_{to} \in (N_{min}, \, \, N_{max})$ implies that $N \in (N_{min}, \, \, N_{max}) \, \, \, \forall t \geq t_{0}$, equivalently $y \in (y_{min}, \, \, y_{max})$ $\forall t \geq t_{0}$, according to Proposition 5.1. The condition $\left. dy/dt\right \rvert_{N = N^{\ast}} > 0$ implies that $N^{\ast} \neq N_{max}$ (equivalently $y^{\ast} \neq y_{max}$). The above two results imply that $N^{\ast} \in (N_{min}, \, \, N_{max})$, equivalently $y^{\ast} \in (y_{min}, \, \, \, y_{max})$. This result, Eq (5.2) and using $y = \ln(N/N_{to})$, yield

Applying this condition to the growth model (3.1), yields Eq (5.1).

Remark 5.1. The condition (5.3) implies that $N^{\ast}$ provided by Eq (5.1) corresponds to a maximum of the $(1/N)(dN/dt)$ versus $N$ curve ^[21].

Proposition 5.3. Consider the logarithmic measure $y = \ln(N/N_{to})$, being $N$ provided by growth model (3.1) with real values of $\alpha$, $c$, $\gamma$, and $N_{max} > N_{min} > 0$; and $N_{to} \in (N_{min}, \, \, N_{max})$. If $N^{\ast} < N_{to}$, (equivalently $y^{\ast} < y_{to}$) then $N > N^{\ast} \, \, \, \forall t \geq t_o$; that is, the $y$ versus $t$ curve exhibits no inflection point. If $N^{\ast} = N_{to}$, (equivalently $y^{\ast} = y_{to}$), then $N = N^{\ast}$ for $t = t_o$; that is, the logarithmic inflection point occurs at $t = t_o$, $N = N_{to}$.

Proof. Recall that the $N$ versus $t$ curve is monotonic and $N \geq N_{to} \, \, \forall t \geq t_o$. Then, $N > N^{\ast} \, \, \forall t \geq t_o$ for $N^{\ast} < N_{to}$. Consequently, $y > y^{\ast} \, \, \forall t \geq t_o$ for $y^{\ast} < y_{to}$.

Remark 5.2. Consider the logarithmic measure $y = \ln(N/N_{to})$ provided by growth model (3.1), with $\gamma > 0$, real values of $\alpha$, $c$, and $N_{max} > N_{min} > 0$, and $N_{to} \in (N_{min}, \, \, N_{max})$. The existence of the inflection point of the $y$ versus $t$ curve requires: i) the existence of a maximum in the $(1/N)(dN/dt)$ versus $N$ curve which is given by Eq (5.1) subject to $N^{\ast} \in (N_{min}, \, \, N_{max})$, and ii) that $N_{to} < N^{\ast}$ where $N_{to} > N_{min} > 0$. The existence of the lag phase requires the existence of the inflection point and a small value of $\left. (1/N)(dN/dt)\right \rvert_{t = to}$. In turn, this implies a positive $c$ value and a small positive $(N_{to} - N_{min})$ value.

Proposition 5.4. Consider the logarithmic measure $y = \ln(N/N_{to})$, being $N$ provided by growth model (3.1) with real values of $\alpha$, $c$, $\gamma$, and $N_{max} > N_{min} > 0$; and $N_{to} \in (N_{min}, \, \, N_{max})$:

i) If

holds, then the $(1/N)(dN/dt)$ versus $N$ curve exhibits a maximum at $N = N^{\ast}$, $N^{\ast} \in (N_{min}, \, \, N_{max})$.

ii) The logarithmic inflection point population $N^{\ast}$, satisfies:

iii) If either

then $(1/N)(dN/dt)$ versus $t$ curve is monotonic decreasing for $N \in (N_{min}, \, \, N_{max})$.

Proof. Model (3.1) with $N_{max} > N_{min} > 0$ subject to parameter regime (5.4) gives:

Therefore, the $(1/N)(dN/dt)$ versus $N$ curve exhibits a maximum at $N = N^{\ast}$, with $N^{\ast} \in (N_{min}, \, \, N_{max})$. In addition, we notice from Eq (5.1) that the inflection point population $N^{\ast}$ is provided by $\left. D_2 \right \rvert _{N = N ^{\ast}} = 0$, where

The $D_2$ term exhibits vertical asymptotes at $N = N_{min}$ and at $N = N_{max}$. In addition, if parameter regime (5.4) holds, then

so that there is at least one $N$ value that belongs to range $(N_{min}, \, \, N_{max})$ and leads to $D_2 = 0$. Then, there exists a maximum in the $(1/N)(dN/dt)$ versus $N$ curve, at $N = N^{\ast}$, $N^{\ast}$ provided by Eq (5.1), and $N^{\ast} \in (N_{min}, \, \, N_{max})$.

For the second part, we recall that for the model (3.1) with $N_{to} \in (N_{min}, \, \, N_{max})$, the inflection point of the $y$ versus $t$ curve satisfies Eq (5.2). As the inflection point corresponds to a maximum of the $dy/dt$ versus $t$ curve, then

according to ^[30]. As $y = \ln (N / N_{to})$, then

Combining this with Eq (5.12) yields:

As $(dN/dt) > 0$ for $N \in (N_{min}, \, \, N_{max})$, real values of $\alpha$, $c$, $\gamma$ and $N_{max} > N_{min} > 0$, then the conditions $t < t^{\ast}$, $t = t^{\ast}$ and $t > t^{\ast}$ are equivalent to $N < N^{\ast}$, $N = N^{\ast}$ and $N > N^{\ast}$, respectively, for $N \in (N_{min}, \, \, N_{max})$. Therefore, expression (5.14) leads to

For model (3.1), and $N \in (N_{min}, \, \, N_{max})$, we have

This and expressions (5.15) lead to

This and condition $N \in (N_{min}, \, \, N_{max})$ results in second part of Proposition.

For the third part, we notice that if (5.7) holds, then

This and Eq (5.16) lead to

As $(dN/dt) > 0$ for $N \in (N_{min}, \, \, N_{max})$, then

thus $d^2 y / dt^2 < 0$ for $N \in (N_{min}, \, \, N_{max})$. Equivalently, the $dy/dt$ versus $t$ curve is decreasing.

Remark 5.3. In first part of Proposition 5.4, not only the parameter values belonging to parameter regime (5.4) lead to existence of a maximum in the $(1/N)(dN/dt)$ versus $N$ curve. Indeed, certain negative $c$ values with $\gamma > 0$ also lead to a maximum. The third part of Proposition 5.4 corresponds to absence of a maximum in the $(1/N)(dN/dt)$ versus $N$ curve, therefore absence of the logarithmic inflection point.

5.2.   Effect of model parameters on the existence of the logarithmic inflection point and lag phase

At what follows, the effect of model parameters on the existence of the inflection point and lag phase is assessed, on the basis of the specific growth rate versus population size curve.

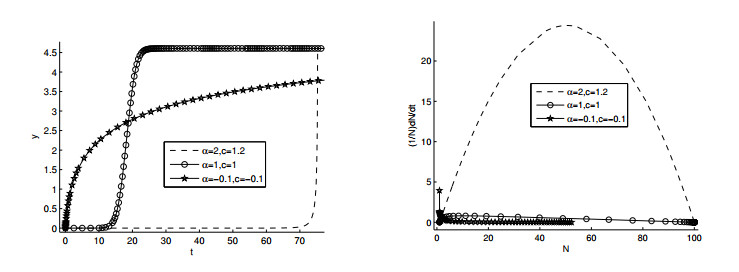
The effect of model parameters on the $(1/N)(dN/dt)$ vs $N$ curve and the $y$ vs $t$ curve is illustrated in Figures 5–7. The following parameter values are used: $N_{max} = 100$, $\gamma = 1$, $N_{min} = (1-1\times 10^{-6})N_{to}$, $N_{to} = 1$. As the advantage of the proposed model is its capacity to represent the lag phase, it is assumed that $\gamma$ is positive and its effect is not assessed. There are four different cases of the $\alpha$, $c$ values, yielding different possibilities of the existence of the lag phase and the inflection point in the $y$ versus $t$ curve:

● $\alpha > 1$, $c > 0$: Pⅰ) $(1/N)(dN/dt) = 0$ for $N = N_{min}$; Pⅱ) $(1/N)(dN/dt) = 0$ for $N = N_{max}$; Pⅲ) $(1/N)(dN/dt) > 0$ for $N \in (N_{min}, \, \, N_{max})$. The first part of Proposition 5.4 applies, so that the $(1/N)(dN/dt)$ versus $N$ curve exhibits a maximum at $N = N^{\ast}$, $N^{\ast} \in (N_{min}, \, \, N_{max})$. Thus, the inflection point occurs provided $N_{to} < N^{\ast}$, and the lag phase existence depends on the $(N_{to}-N_{min})$ value. Simulation is shown in Figure 5.

● $\alpha < 1$ and $c > 0$: The properties Pi to Piv of case $\alpha > 1$, $c > 0$ are fulfilled. The first part of Proposition 5.4 applies, so that the inflection point occurs provided $N_{to} < N ^{\ast}$, and the lag phase existence depends on the $(N_{to}-N_{min})$ value. Simulation is shown in Figure 6, confirming the above facts, and also shows that: ⅰ) the effect of $\alpha < 1$ values on the lag phase duration is low; ⅱ) the maximum growth rate is overly low, leading to low values of the slope of the population at the exponential growth phase. This confirms the importance of the $N^{\alpha}$ term for the slope of the exponential growth phase.

● $\alpha > 1$ and $c < 0$: Pⅰ) $(1/N)(dN/dt) = \infty$ for $N = N_{min}$, that is, there is a vertical asymptote at $N = N_{min}$; Pⅱ) $(1/N)(dN/dt) = 0$ for $N = N_{max}$; Pⅲ) $(1/N)(dN/dt) > 0$ for $N \in (N_{min}, \, \, N_{max})$. Property Pi implies that $\left. (1/N)(dN/dt)\right \rvert_{N = N_{to}}$ is overly high for low values of $(N_{to} - N_{min})$, so that there is no lag phase. The occurrence of inflection point is determined by Eq (5.6) subject to $N ^{\ast} \in (N_{min}, \, \, N_{max})$, and provided $N_{to} < N^{\ast}$. The third part of Proposition 5.4 indicates that for $c \leq (-1) (\alpha - 1) (N_{max} / N_{min} - 1)$ values there is no inflection point. Moreover, simulations show that for some parameter values there is a local minimum and a local maximum in the range $N \in (N_{min}, \, \, N_{max})$. As a consequence, there may be inflection point but there is no lag phase. Simulation in Figure 7 confirms the above facts.

● $\alpha < 1$ and $c < 0$: Pⅰ) $(1/N)(dN/dt) = \infty$ for $N = N_{min}$, that is, there is a vertical asymptote at $N = N_{min}$; Pⅱ) $(1/N)(dN/dt) = 0$ for $N = N_{max}$; Pⅲ) $(1/N) (dN/dt) > 0$ for $N \in (N_{min}, \, \, N_{max})$. Proposition 5.4 applies, so that the $(1/N)(dN/dt)$ versus $N$ curve is monotonically decreasing for $N \in (N_{min}, \, \, N_{max})$. Thus, no inflection point occurs, and therefore lag phase neither occurs. Simulation in Figures 5 and 7 confirms the above facts.

Intermediate cases are:

● $\alpha > 1$ and $c = 0$: The term $f_c$ is absent, so that the model becomes $(1/N)(dN/dt) = r N^{\alpha-1} (1-N/N_{max})^{\gamma}$, and: Pⅰ) $(1/N)(dN/dt) > 0$ for $N = N_{min}$; Pⅱ) $(1/N)(dN/dt) = 0$ for $N = N_{max}$; Pⅲ) $(1/N)(dN/dt) > 0$ for $N \in (N_{min}, \, \, N_{max})$; Piv) $\left. (1/N)(dN/dt)\right \rvert_{N = N_{to}}$ is positive and is proportional to $N_{to}^{\alpha - 1}$. Thus, the $(1/N)(dN/dt)$ versus $N$ curve exhibits a maximum with $N^{\ast} \in (N_{min}, \, \, N_{max})$, so that the inflection point occurs provided $N_{to} < N^{\ast}$ and it is owed to the term $N^{\alpha - 1}$ with $\alpha > 1$. For general (not small) values of $N_{to}$, $\left. (1/N)(dN/dt)\right \rvert_{t = to}$ is not small, so that there is no lag phase. Simulations are shown in Figure 7.

● $\alpha = 1$ and $c < 0$: The term $N^{\alpha-1}$ is absent, and the properties are similar to those of case $\alpha < 1$ and $c < 0$: Pⅰ) $(1/N)(dN/dt) = \infty$ for $N = N_{min}$, that is, there is a vertical asymptote at $N = N_{min}$; Pⅱ) $(1/N)(dN/dt) = 0$ for $N = N_{max}$; Pⅲ) $(1/N)(dN/dt) > 0$ for $N \in (N_{min}, \, \, N_{max})$. According to the third part of Proposition 5.4, the $(1/N)(dN/dt)$ versus $N$ curve exhibits monotonic decreasing behavior, so that no inflection point occurs, and therefore lag phase neither occurs.

● $\alpha = 1$ and $c > 0$: The term $N^{\alpha-1}$ is absent, and the properties Pi to Piv of case $\alpha < 1$ and $c > 0$, are fulfilled. The first part of Proposition 5.4 applies, so that the inflection point occurs provided $N_{to} < N^{\ast}$; and the lag phase existence depends on the $(N_{to} - N_{min})$ value. Simulation in Figure 5 confirms the above properties and: ⅰ) the maximum occurs at low values of the population size ($N^{\ast}$ is low); ⅱ) the maximum growth rate is overly low, so that the slope of the growth curve at the exponential growth phase is overly low.

Remark 5.4. The above cases of $\alpha$ and $c$ values indicate that the $c$ parameter has a significant influence on the lag phase duration but the influence of the $\alpha$ value is low. Concerning the slope at the exponential growth phase, the $\alpha$ value has a significant influence, whereas the influence of the $c$ value is low.

Remark 5.5. The parameter region $\alpha < 1$ leads to low slope of the population at the exponential growth phase, whereas $c < 0$ implies that no lag phase occurs. Thus, the condition $\alpha > 1$ is required for higher slopes of the population at the exponential growth phase, whereas $c > 0$ is required for representing the lag phase.

5.3.   Properties of the logarithmic inflection point

Proposition 5.5. Consider $y = \ln(N/N_{to})$, and the model (3.1) with $\gamma > 0$. The population at the logarithmic inflection point, $N^{\ast}$, is defined by

where

and the discriminant is

so that no imaginary solutions are possible for $\gamma c \geq 0$. If $\bar{a} > 0$, $c > 0$ and $\bar{b}^2 – 4 \bar{a} \bar{c}$ is non-negative, only the positive square root leads to $N^{\ast} > N_{min}$.

Proof. The population at the logarithmic inflection point, $N^{\ast}$, is obtained by applying Eq (5.3) to the growth model (3.1), leading to

Rearranging yields the quadratic Eq (5.21). In order to determine whether the negative square root term can lead to $N^{\ast} > N_{min}$ for $\bar{a} > 0$, $c > 0$ we use Proposition presented in Appendix B. Using the definition of $\bar{a}$, $\bar{b}$, $\bar{c}$, we have $\bar{c} + \bar{a} + \bar{b} = (-1) c (N_{max}/N_{min}-1)$. Since $(N_{max}/N_{min}) > 1$, then $\bar{c} + \bar{a} + \bar{b} < 0$ for $c > 0$. Hence, the condition $\bar{c} + \bar{a} + \bar{b} > 0$ is not fulfilled for $c > 0$. Consequently, only the positive square root term leads to $N^{\ast}/N_{min} > 1$.

Proposition 5.6. Limit values of $N^{\ast}$. Consider the model (3.1) with $\alpha - 1 > 0$, $\gamma > 0$, $c \geq 0$. The limit values of the population at the logarithmic inflection point, $N^{\ast}$, are:

Proof. The above limit values of $N^{\ast}$ are obtained by substituting $\gamma \rightarrow 0$, $\gamma \rightarrow \infty$, $c \rightarrow 0$, $c \rightarrow \infty$, $(\alpha -1) \rightarrow 0$, $(\alpha - 1) \rightarrow \infty$ in Eq (5.24) and solving for $N^{\ast}$.

Remark 5.6. The limit values of $y^{\ast}$ can be obtained straightforwardly by using $y^{\ast} = \ln(N^{\ast}/N_{to})$ and the definitions (3.5), (3.6).

Proposition 5.7. Conditions for $N^{\ast} = N_{to}$. Consider the model (3.1). The population at the logarithmic inflection point, $N^{\ast}$, satisfies: $N^{\ast} = N_{to}$ for

Proof. Using $N^{\ast} = N_{to}$ in Eq (5.24) and arranging yields Eq (5.26).

Remark 5.7. The above proposition implies that in the $y$ versus $t$ curve, with $N_{to} \in (N_{min}, \, \, N_{max})$, the maximum $dy/dt$ occurs at $t = t_o$, $N = N_{to}$, if the $\alpha$, $\gamma$, $c$ values are such that Eq (5.26) holds true.

Proposition 5.8. Conditions for $N^{\ast} = N_{my}$ (equivalently, $y^{\ast} = y_{m}$). Consider model (3.1). The population at the logarithmic inflection point, $N^{\ast}$, satisfies $N^{\ast} = N_{my}$ for

Proof. Using $N^{\ast} = N_{my}$, $N_{my} = (N_{min} N_{max})^{1/2}$ in Eq (5.24) and arranging yields Eq (5.27).

Remark 5.8. The above proposition implies that in the $y$ versus $t$ curve, with $N_{to} \in (N_{min}, \, \, N_{my})$, the maximum $dy/dt$ occurs at $N = N_{my}$; where $N_{my} = (N_{min} N_{max})^{1/2}$, if the $\alpha$, $\gamma$, $c$ values are such that Eq (5.27) holds true.

Remark 5.9. The determination of the lag time of the logarithmic growth curve via the classical approach requires the determination of the inflection point, as shown in Appendix A. To this end, the population at the inflection point $(N^{\ast})$ can be computed using expressions (5.21), (5.22), and the inflection time $(t^{\ast})$ must be computed numerically.

6.   Determination of the lag time using simplified models and the classical approach

In this section, we propose an approach for the analytical determination of the lag time, for each the regular and the logarithmic measures. Also, a numerical example is provided, using the proposed approach and the classical approach.

The classical measure of the lag time requires the determination of the inflection point, as shown in Appendix A. In turn, the regular and the logarithmic population at the inflection point ($N^{\#}$ and $N^{\ast}$) can be computed using the expressions (4.20), (4.21), (5.21), (5.22). The inflection times $(t^{\#}, t^{\ast})$ must be computed numerically.

The proposed approach is based on an application of the classical approach to a simplified growth model whose lag time value is quite similar to that of the original model. The simplified model is derived from proposed model (3.1) so as to fulfill the following properties: Rⅰ) its lag time value is quite similar to that of the original $N$ versus $t$ curve provided by model (3.1); Rⅱ) it allows to obtain an analytical expression of the population $N$; Rⅲ) it allows to apply the classical $t_{lag}$ approach. Thus, given a model simplified on the basis of the above facts, the $t_{lag}$ value of the simplified model can be considered as a $t_{lag}$ measure of the original model.

Disregarding the saturation term $(1-N/N_{N_{max}})$ simplifies the determination of the lag phase duration, as was used by Baranyi, Roberts and McClure ^[1]. For the proposed model (3.1), with given values of $\alpha$, $c$, $\gamma$, remarks 11, 20 indicate that: i) the effect of parameter $\alpha$ on the lag phase and the lag time is low; ii) during the lag phase and the early exponential growth phase, $N \approx N_{to}$, so that the effect of simplification $(1-N/N_{max})^{\gamma} = (1-N_{to}/N_{max})^{\gamma}$ on the lag time is low. Thus, a simplified form that accomplishes requirements Ri to Riii is:

where $k_2$ is a positive integer, and the $k_1$ option that is the nearest to the $\alpha$ value must be chosen. The $N_{s2}$ model is obtained from the proposed model (3.1) with the simplification $\alpha = k_1$ and $(1-N/N_{max})^{\gamma} = (1-N_{to}/N_{max})^{\gamma}$. The $N_{s2}$ curve has no inflection point, and it does not converge towards an equilibrium point, but its lag phase and the early part of the exponential growth phase are similar to those of the model (3.1). In the particular case $k_1 = c$, the analytical solution of Eq (6.1) is:

The application of the classical $t_{lag}$ approach is different for the regular and the logarithmic growth curves.

6.1.   Determination of the lag time for the regular population size

To apply the classical $t_{lag}$ approach on the $N_{s2}$ versus $t$ curve, we notice that this curve has no inflection point. Thus, we choose other point, located in the exponential growth phase, whose $N$ value, denoted as $N_{s2}^p$ equals the inflection point of the simplified $N$ versus $t$ curve provided by

so that $N_{s2}^p = N_{s1}^{\#}$. This curve involves the simplification $\alpha = k_1$, $k_1$ satisfying (6.2). In accordance with the classical $t_{lag}$ approach, the intersection of the line that is tangent to the $N_{s2}$ versus $t$ curve at $N_{s2} = N_{s1}^{\#}$, with the line $N_{s2} = N_{to}$ yields the $t_{lag}$ value. The $N_{s1}^{\#}$ value can be obtained via Eqs (4.20) and (4.21), with $\alpha = k_1$. In the particular case $k_1 = c$, the $N_{s1} ^{\#}$ value is obtained by applying Eq (4.3) to model (6.4) with $k_1 = c$:

The time for $N_{s2} = N_{s1} ^{\#}$ (denoted $t_s$) is obtained from the analytical solution of Eq (6.1), and using $N_{s2} = N_{s1} ^{\#}$. In the particular case $k_1 = c$, $t_s$ is obtained using Eq (6.3):

The $t_{lag}$ can be computed from

where $(dN_{s2}/dt)$ at $N_{s2} = N_{s1}^{\#}$ can be computed using Eq (6.1).

In summary, the application of the proposed approach for the regular growth curve involves the following steps:

● Recall the parameters of model (3.1) or obtain them by fitting to experimental data

● Select the $k_1$ option (6.2) that is the nearest to the $\alpha$ value

● Determine the analytical expression of the $N_{s2}$ curve by using Eq (6.1), and in the case $k_1 = c$ use Eq (6.3)

● Determine $N_{s1}^{\#}$ value using Eqs (4.20) and (4.21), with $\alpha = k_1$; in the particular case $k_1 = c$, use Eq (6.5)

● Compute the time for $N_{s2} = N_{s1}^{\#}$ (that is, $t_s$), using the analytical expression for $N_{s2}$.

● Compute the $t_{lag}$ value using Eq (6.7).

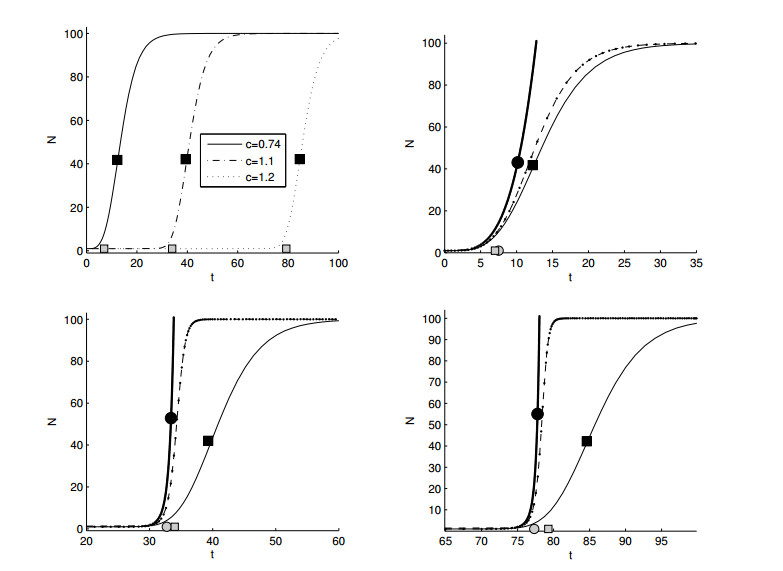
As a numerical example we consider model (3.1), with $r = 1$, $N_{min} = (1- 1 \times 10^{-6})N_{to}$, $N_{to} = 1$, $\gamma = 1$; $\alpha = 0.7$ and $c$ values $c = 0.74$, $c = 1.1$, $c = 1.2$. We apply the aforementioned procedure, choosing $k_1 = c$, and the results are shown in Figure 8. Upper left: The time course of $N$ for $c = 0.74$, $c = 1.1$, $c = 1.2$ (solid, dash-dotted and dotted lines), with the inflection points (black squares) and the lag time points (gray squares) determined via the classical approach. Each of the remaining figures comprises: The $N$ time course (thin solid line) with the inflection point (black square) and the lag time point (gray square) determined via the classical approach; the $N_{s1}$ time course (dash-dot line); and the $N_{s2}$ time course (thick solid line) with the point $N_{s2} = N_{s1} ^{\#}$ (black point), and the lag time point (gray point) determined via the proposed approach. The $c$ values used are $c = 0.74$ (upper right), $c = 1.1$ (lower left), and $c = 1.2$ (lower right).

6.2.   Determination of the lag time for the logarithmic population size

To apply the classical $t_{lag}$ approach on the $y_{s2} = \ln (N_{s2}/N_{to})$ versus $t$ curve, we notice that this curve has no inflection point. Thus, we choose other point, located in the exponential growth phase, whose $y_{s2}$ value, denoted as $y_{s2}^p$, results from the inflection point of the simplified $y$ versus $t$ curve provided by:

so that $y_{s2}^p = y_{s1}^{\ast}$. This curve involves the simplification $\alpha = k_1$, being $k_1$ chosen from options in (6.2). In accordance with the classical $t_{lag}$ approach, the intersection of the line that is tangent to the $y_{s2}$ versus $t$ curve at $y_{s2} = y_{s1}^{\ast}$, with the line $y_{s2} = y_{s2} \rvert_{t = to} = 0$ yields the $t_{lag}$ value.

The $y_{s1}^{\ast}$ value and the corresponding $N_{s1}^{\ast}$ value (which satisfies $y_{s1}^{\ast} = \ln (N_{s1}^{\ast}/N_{to})$) are obtained from Eqs (5.21) and (5.22) with $\alpha = k_1$. The time for $y_{s2} = y_{s1}^{\ast}$, equivalently $N_{s2} = N_{s1}^{\ast}$, denoted as $t_s$, is obtained from the analytical solution of Eq (6.1) using $N_{s2} = N_{s1}^{\ast}$. In the particular case $k_1 = c$, the time $t_s$ is obtained using Eq (6.3) with $N_{s2} = N_{s1}^{\ast}$:

The $t_{lag}$ value can be computed from

where $\left. dy_{s2}/dt \right \rvert_{Ns2 = N_{s1}^{\ast}}$ can be computed using $dy_{s2}/dt = (1/N_{s2})dN_{s2}/dt$, being $dN_{s2}/dt$ provided by Eq (6.1) with $N_{s2} = N_{s1}^{\ast}$.

In summary, the application of the proposed approach for he logarithmic growth curve involves the following steps:

● Recall the parameters of model model (3.1) or obtain them by fitting to experimental data

● Select the $k_1$ option (6.2) that is the nearest to the $\alpha$ value

● Determine the analytical expression of the $N_{s2}$ curve by using Eq (6.1), and in the case $k_1 = c$ use Eq (6.3).

● Determine the $y_{s1}^{\ast} = \ln (N_{s1}^{\ast}/N_{to})$ value, using Eqs (5.21) and (5.22) with $\alpha = k_1$.

● Compute the time for $y_{s2} = y_{s1}^{\ast}$ (that is, $t_s$), using the analytical expression for $N_{s2}$ with $N_{s2} = N_{s1}^{\ast}$.

● Compute the $t_{lag}$ value, using Eq (6.11)

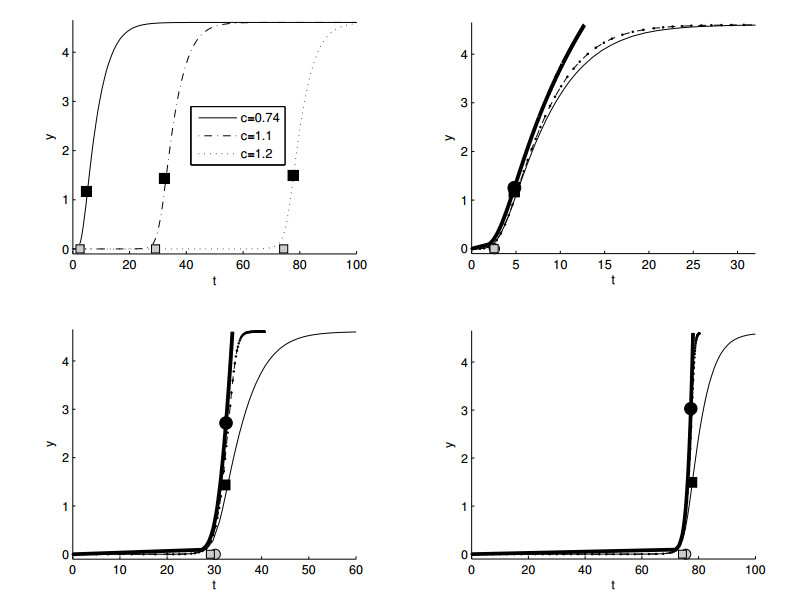
As a numerical example, we consider model (3.1), with $r = 1$, $N_{min} = (1- 1 \times 10^{-6})N_{to}$, $N_{to} = 1$, $\gamma = 1$; $\alpha = 0.7$, and $c$ values $c = 0.74$, $c = 1.1$, $c = 1.2$. We apply the aforementioned procedure, choosing $k_1 = c$, as shown in Figure 9. Upper left: Time course of $y$ for $c$ values $c = 0.74$, $c = 1.1$, $c = 1.2$ (solid, dash-dotted and dotted lines), with the inflection points (black squares) and lag time points (gray squares) determined via the classical approach. Each of the remaining figures comprise: The $y$ time course (thin solid line) with the inflection point (black square) and the lag time point (gray square) determined via the classical approach; the $y_{s1} = \ln (N_{s1} / N_{to})$ time course (dash-dot line); and the $y_{s2} = \ln (N_{s2} / N_{to})$ time course (thick solid line) with the point $y_{s2} = y_{s1} ^{\ast}$ (black point) and the lag time point (gray point) determined via the proposed approach. The $c$ values used are $c = 0.74$ (upper right), $c = 1.1$ (lower left), and $c = 1.2$ (lower right).

7.   Application of the proposed model to experimental data

In this section, the proposed model (3.1) is applied to experimental microbial growth curves.

7.1.   Arrangement of the proposed model for fitting to microbial growth curves

The differential Eq (3.1), the expression for the population at the inflection point $N^{\ast}$ and the expression for the lag time ($t_{lag}$) are rewritten in terms of the logarithmic measure $y = ln(N/N_{a})$ as follows, being $N_a$ a user defined positive constant. Using model (3.1), the above definition of $y$ instead of definition (3.4), and the definition

one obtains:

and its parameters are $\bar{r}$, $\alpha$, $\gamma$, $c$, $y_{min}$, $y_{max}$. Using definition (7.1), the term $N_{max}/N_{min}$ appearing in definitions (5.22) can be expressed as

Definition $y = ln(N/N_{a})$ implies $y^{\ast} = ln(N^{\ast} / N_{a})$. Using definition (7.1) and Eq (5.21), one further obtains $y^{\ast} = ln (e^{y_{min}} x)$, being $x$ obtained from Eq (5.21). The $t^{\ast}$ value can be computed numerically by using Eq (7.2), so as to obtain $y = y^{\ast}$. The lag time can be computed via the classical approach with

being $(dy/dt) \rvert_{y^{\ast}}$ obtained using Eq (7.2).

Remark 7.1. In summary, the model properties in terms of the logarithmic measure includes: i) the logarithmic population at the inflection point $(y^{\ast})$, which can be computed using expression $y^{\ast} = ln(e^{y_{min}} x)$, being $x$ obtained from Eqs (5.21) and (5.22) with $(N_{max}/N_{min}) = e^{y_{max} - y_{min}}$; ii) the inflection time $(t^{\ast})$, which can be computed numerically using Eq (7.2); and iii) the lag time $t_{lag}$ which can be computed by means of Eq (7.4), being $(dy/dt) \rvert_{y^{\ast}}$ obtained using Eq (7.2). In this way, the computation of $y^{\ast}$, $t^{\ast}$, $t_{lag}$ neither requires $N^{\ast}$ nor $N_{a}$.

7.2.   Survey of the model of Baranyi and Roberts and the Gompertz model

The model of Baranyi and Roberts ^[31] is:

its parameters are $\mu_{max}$, $m$, $y_{max}$, $q_o$, $v$; and the lag time is $t_{lag} = (1/v) Ln (1+1/q_o)$.

The Gompertz model is ^[32]:

its parameters are $\mu_{max}$, $y_{max}$, $\lambda$, $y_a$; and the lag time is $t_{lag} = \lambda$.

7.3.   Numerical fitting example

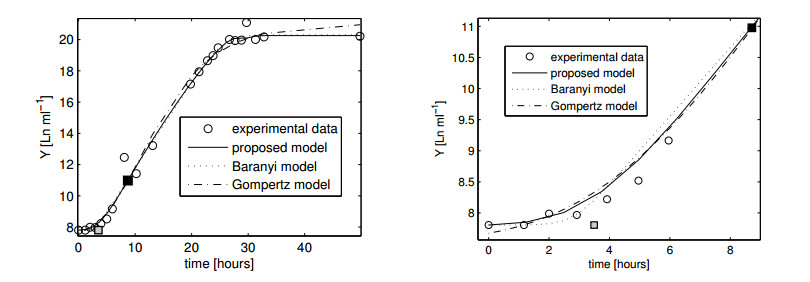
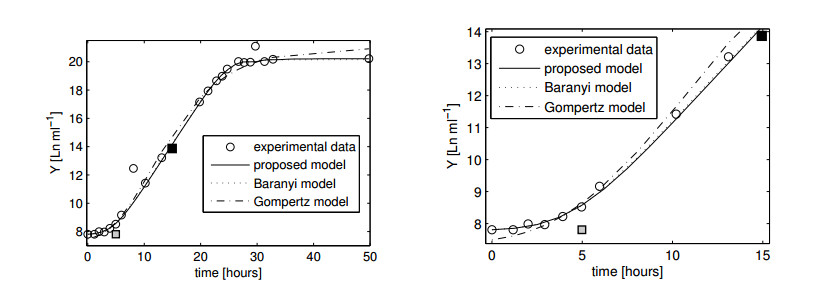
In order to assess the capability of the proposed model to simulate growth curves, it and also the model of Baranyi and Roberts and the Gompertz model were fitted to growth records of Salmonellae. The datasets were taken from ^[1], corresponding to constant temperature and pH. The proposed model, the inflection point and the lag time expressed in terms of the logarithmic measure $y$ are stated in subsection 7.1, whereas the model of Baranyi and Roberts and the Gompertz model are stated in subsection 7.2. The value $N_a = 1$ is used for the proposed model, in order to compare the simulation of $y = ln (N)$ for the three models.

Model simulation and parameter estimation were performed using Matlab software (the Math Works Inc., Natick, Mass.). The differential Eq (7.2) was numerically integrated using the ode45 routine. Parameter estimation was carried out by minimizing the sum of the squared deviations between the values calculated by the model and the experimental data. The $R^2$ value was used to evaluate the goodness of fit (see ^[33]), whereas the Akaike's Information Criterion (AIC) allows to compare the model acceptability on the basis of both fitting capability and complexity, being the complexity related to number of parameters ^[34,35]:

where $N$ is the number of data points, $N_{prm}$ is the number of model parameters, and RSS is the sum of the squared deviations between the values calculated by the model and the experimental data. The model with the lowest AIC value is the most acceptable. The fitting results are:

There is a good agreement between model simulation and experimental measurements (Figure 10), with a relatively high and similar $R^2$ value for all the models. The estimated $\alpha$, $\gamma$, $c$, are positive, and $\alpha$ is close to $1.0$. The lag time $t_{lag}$ values were not quite similar. In terms of the AIC value, the Baranyi model is the most acceptable. This is partially related to the number of parameters, indeed $N_{prm} = 6$, $N_{prm} = 5$, $N_{prm} = 4$ for the proposed model, the Baranyi's model and the Gompertz model, respectively. This worsens the AIC value of the proposed model in comparison with that of Baranyi's model. The fitting of the three models exhibit disagreement for the lag phase points (first seven points). In view of the above limitations, the fitting of the models to the lag phase points was improved, although at the cost of lowering the overall $R^2$ value; $\alpha = 1$ was used in the proposed model, what is a common value, see ^[16,28]; and the $R^2$ for the lag phase $(R^2_{lag})$ was calculated. The fitting results are:

The three models achieved satisfactory agreement between simulation and experimental data, with a high $R^2$ value (Figure 11). In terms of the AIC value, the Gompertz model is the most acceptable, but it exhibits significantly flawed fitting of several data points. The Baranyi model exhibits the second best AIC value. Both the Baranyi's model and the proposed model achieved proper fitting of the lag phase points, and the highest $R^2_{lag}$ was achieved by the proposed model.

The obtained results confirm the capability of the proposed model to simulate monotonic growth curves, mainly those involving lag phase.

8.   Conclusions

The studied generalized form of the Fujikawa’s growth model is autonomous, exhibits monotonic behavior of the population size and is capable of generating the common features of monotonic growth of microorganisms along time, including growth with decreasing slope without inflection point and growth with inflection point and lag phase. Indeed, either a large or a small lag time can be combined with either a large or a small slope of the exponential growth phase. Thus, the model is highly flexible and is suitable for different applications. Although Fujikawa, Kai and Morozumi ^[16,28] only employed positive values of $\alpha$, $c$, negative values lead to features of the $y$ versus $t$ curve that are possible in real situations.

A deep insight on the growth curves is obtained by analyzing the $dN/dt$ versus $N$ curve at $N = N_{min}$, $N = N_{max}$ and for $N \in (N_{min}, \, \, N_{max})$, in addition to assessing the presence of a maximum (which corresponds to an inflection point) or a minimum. A minimum and a maximum occur for $\alpha > 0$ and $c < 0$, what is not common in growth curves.

The study of the effect of $\alpha$ and $c$ values on the existence of the regular inflection point and lag phase indicates that: ⅰ) if both are positive, the inflection point occurs provided adequate $N_{to}$; ⅱ) if either $\alpha$ or $c$ is negative, the occurrence of the inflection point is possible; ⅲ) if both parameters are negative, there is no inflection point nor lag phase. The existence of lag phase depends on the value of the growth rate at initial time, what is proportional to $(N_{to} - N_{min})$.

The $\alpha$ and $c$ parameters also affect the lag time magnitude and the slope of the exponential growth phase: ⅰ) the $\alpha$ value has a significant influence on the slope at the exponential growth phase, whereas the influence of the $c$ value is low; ⅱ) the $c$ parameter has a significant influence on the lag phase duration but the influence of the $\alpha$ value is low. For adequate representation of a growth curve that exhibits lag phase and steep exponential growth phase, it is required that both the $\alpha$ and the $c$ values be positive.

The limit values of the inflection point population $N^{\#}$ confirms it great flexibility, due to the parameters $\alpha$, $c$, $\gamma$.

The numerical fitting example indicated the capability of the proposed model for fitting to experimental data of microbial growth. Indeed, the $R^2$ value is high and similar to that of the Baranyi model.

Acknowledgments

A Rincón was supported by Universidad Católica de Manizales.

Conflict of interest

The authors declare that there is no conflict of interests regarding the publications of this paper.

A.   Appendixes

A.1.   Appendix 1. Classical determination of the lag phase duration

For the regular measure ($N$), the tangent line at the inflection point ($t^{\#}, N^{\#}$) is

The lag phase duration ($t_{lag}$) is obtained from the intersection of this line with the horizontal line $N = N_{to}$, what gives

For the logarithmic measure $y = \ln(N/N_{to})$, the tangent line at the inflection point ($t^{\ast}, y^{\ast}$) is

where

The lag phase duration ($t_{lag}$) is obtained from the intersection of this line with the horizontal line $y = y_{to} = 0$, what gives

A.2.   Appendix 2. Conditions for selecting the solution of quadratic algebraic equations

Proposition A.1. Consider the quadratic algebraic equation

being $\bar{b}^2 – 4 \bar{a} \bar{c}$ non-negative. If $\bar{a}>0$, the solution with negative square root requires $\bar{c} + \bar{a} + \bar{b} >0$ for obtaining $x>1$.

Proof Consider the quadratic Eq (A.6), with $\bar{a}>0$, and the solution comprising the negative square root term. Accomplishment of condition $x>1$ implies

This expression implies that $2 \bar{a} + \bar{b} < 0$. Taking squares to Eq (A.7) and arranging yields $-\bar{c} < \bar{a} + \bar{b}$. Therefore, the solution with negative square root term, requires the condition $\bar{c} + \bar{a} + \bar{b} >0$ for achieving $x>1$.