Global dynamics of a model for treating microorganisms in sewage by periodically adding microbial flocculants

1.   Introduction and model formulation

With the improvement of people's living standards, people's demand for food, especially various meat product is growing. According to the statistics of the US Department of Agriculture, in 2014, the per capita consumption of red meat and chicken reached 100.4 pounds and 83.8 pounds, respectively. In 2015, these indicators reached 104.2 pounds and 89.3 pounds respectively, and these indicators increased year by year, by 2017, these indicators reached 108.2 pounds and 92.1 pounds respectively, compared with 2014, the growth rate has reached 7.7% and 9.9% respectively^[1,2]. People's dependence on meat food has stimulated the prosperity of the global livestock and poultry industry, which has spawned farms of different sizes. However, livestock and poultry farms produce a large amount of sewage every day. This sewage mainly include livestock and poultry urine and feces, feed residues, washing water and sewage generated by workers' production^[3,4]. Moreover, the sewage contains lots of pathogens, such as Escherichia coli, Enterococcus, Ascaris eggs and so on. The indiscriminate discharge and treatment of aquaculture sewage have caused serious environmental pollution^[5,6]. The pollution caused by livestock and poultry farming has become the third largest source of pollution after industrial pollution and domestic pollution. Therefore, how to treat sewage from livestock and poultry farming effectively has become an important issue in sewage treatment.

Many environmental protection experts and scholars have conducted in-depth research and got many excellent results^[7,8,9,10]. Numerous studies have shown that microbial flocculants can flocculate bacteria such as Escherichia coli and yeast through various mechanisms of action such as adsorption bridging^[11], electrical neutralization^[12], and chemical reaction^[13], which makes it possible to remove microorganisms by adding microbial flocculation to sewage^[14]. The earliest flocculant-producing bacteria were screened from activated sludge by Butterfield ^[15]. In 1976, Nakamura J et al. screened microorganisms with flocculation ability from molds, bacteria, actinomycete, etc., among which the flocculation effect produced by Aspergillus sojae was the best, thus opening up the upsurge of microbial flocculants ^[16]. In 1985, Takagi H et al. studied the flocculant PF101 produced by the pseudomycin penicillin microorganism. PF101 has good flocculation effect on Bacillus subtilis, Escherichia coli, brewer's yeast, activated sludge, etc. ^[17]. In 1986, Kurane et al. developed a bioflocculant NOC-1 using Rhodococcus erythropolis, which has excellent flocculation and the decolorization effect for E. coli, yeast, muddy water, river water, fly ash water, expanded sludge, and pulp sewage which is the best microbial flocculating floc found at present ^[18]. With the development of microbial flocculants, new flocculants have emerged in recent years. In ^[19], Salehizadeh et al. studied the flocculant produced by Pasteurella and used the flocculant to flocculate dye wastewater and yeast wastewater well. Zhang et al. ^[20] studied the characteristics of high flocculating active microbial flocculant TJ-F1 produced by Proteus mirabilis and its flocculation mechanism. Recently, Song et al. proposed some models describing biodegradation of Microcystins^[21,22,23]. Guo et al. ^[24,25] considered two delayed microorganism flocculation models with different functional responses.

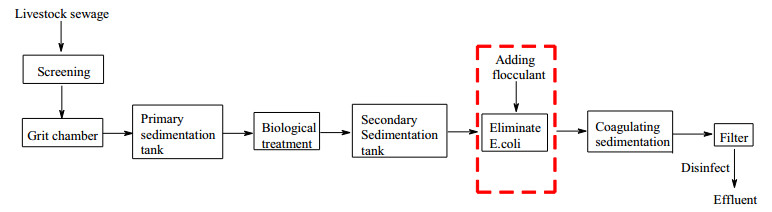
Previous work on general livestock and poultry sewage treatment process ^[24,25] motivated us in considering addition of a suitable microbial flocculant to eliminate microorganisms in the supernatant after the second precipitation (Figure 1). We try to establish some mathematical models to describe the kinetics of the treatment of microorganisms in sewage by using microbial flocculants.

First, we give the classical chemostat model, in which a population of microorganisms depend on a single growth limiting substrate, the two-dimensional system can be formulated as follows

where $ S(t), X(t) $ stand for the concentration of substrate, microorganisms in sewage at time $ t, $ respectively. $ D $ is the dilution rate, $ \mu_{m} $ and $ \delta $ represent the maximum growth rate and a growth yield constant, respectively. $ K_m $ represents the half-saturation constant. $ S_0 $ is the initial input concentration of substrate.

Second, we consider adding a certain flocculant to remove E. coli from sewage. Let $ P(t) $ stand for the concentration of flocculants in sewage at time $ t, $ considering the saturation effect of microbial flocculation, the consumption of flocculants can be expressed as $ \frac{h_3{X}(t)P(t)}{K_s+X(t)} $ and the amount of microorganisms flocculated by microbial flocculants can be expressed as $ \frac{h_2X(t)P(t)}{K_s+X(t)}. $ Then we get the equations of concentration change of flocculants and microorganisms during treatment respectively as follows,

and

where $ K_s $ represents the half-saturation constant, $ h_3 $ and $ h_2 $ are the maximum consumption rate of flocculants and the maximum flocculation rate, respectively. $ P_0 $ is the initial input concentration of flocculants. Then, a mathematical model by the ordinary differential equations (ODEs) is proposed to describe continuous eliminating microorganisms process using microbial flocculants as follows,

system (1.1) describes the process of treating microorganisms in sewage by continuously adding microbial flocculants based on a chemostat system.

However, sewage treatment is a discontinuous process^{[26,27,28,29]}. Generally, a certain amount of flocculant is released at intervals to treat microorganisms in sewage. Therefore, the concentration change of the flocculant in the system is not a continuous process, and the concentration of flocculant in the system will change sharply in the initial stage of flocculant delivery. This sharp change can be represented by Impulsive Differential Equations (IDEs), mathematically. In the past 20 years, IDEs have received extensive attention, and have been widely used in predator-prey model^{[32,33,34,35,36,37,38,39,40,41,42,43]}, chemostat model^{[44,45,46,47,48,49,50,51,52]}, epidemic model^{[53,54,55,56,57,58]} and general theoretical study^[59,60,61]. Then based on model (1.1), by introducing microbial flocculants periodically, a more realistic model for eliminating microorganisms is proposed as follows,

where $ T $ is the impulsive period, $ \gamma P_0 $ is the amount of flocculant added in each period $ T. $ $ \Delta{P}(t) = P(nT^+)-P(nT^-). $ $ P(nT^+) = \mathop{\lim}\limits_{t\to nT^+}P(t), $ $ P(t) $ is left continuous at $ t = nT, $ $ S(t) $ and $ X(t) $ are continuous for all $ t\geq0. $

This paper is organized as follows, some preliminary knowledge are given in Section 2. Then the global dynamics of system (1.2) are analyzed in Section 3, more precisely, the existence and globally asymptotic stability of the microorganisms-extinction periodic solution are discussed in the first part and the permanence of system (1.2) is discussed in the second part. The control strategy for microbial treatment is investigated in Section 4. In Section 5, an example with some computer simulations are given to illustrate the theoretical results. At last, a brief conclusion is given in Section 6.

2.   Preliminaries

In this section, we will give some preliminary knowledge. Let $ N $ be the set of all non-negative integers, and $ R_+ = [0, \infty), R^3_+ = \{x\in R^3: x \geq 0\}, \Omega = \rm{int}R^3_+. $ Denote the solution of system (1.2) by $ X = (S(t), X(t), P(t)):R_{+}\rightarrow R_{+}^{3}, $ then $ X $ is continuously differentiable on $ ((n-1)T, nT) (n \in N), $ moreover, the global existence and uniqueness of solutions of system (1.2) can be guaranteed by the smoothness properties of $ f, $ where the map $ f = (f_1, f_2, f_3)^T $ is defined by the system (1.2).

Definition 2.1. (^[62,63]) Suppose $ M : R_{+}\times R_{+}^{3}\rightarrow R_{+}, $ and $ M $ is continuous in $ ((n-1)T, nT]\times R_{+}^{3}, n\in N, $ for each $ x\in R_{+}^{3}, \lim_{(t, u)\to(nT^{+}, x)}M(t, z) $ exist, and $ M $ is locally Lipschitz continuous with respect to $ x $ in $ ((n-1)T, nT]\times R_{+}^{3}, n\in N. $ Then for $ (t, x)\in ((n-1)T, nT]\times R_{+}^{3}, n\in N, $ the upper right derivative of $ M(t, x) $ with respect to (1.2) can be defined as

Lemma 2.1. ^[54] Consider the impulsive differential system

then system (2.1) has a globally attractive $ T $ periodic solution $ h^*(t), $ where

Lemma 2.2. Let $ T(t) = (S(t), X(t), P(t)) $ be a solution of system (1.2) satisfying $ T(0^+)\geq0, $ then $ T(t)\geq0 $ for all $ t\geq0. $

Lemma 2.3. For the solution $ (S(t), X(t), P(t)) $ of system (1.2), we have

In fact, by standard analytical methods, it is easy to get that the solution of system (1.2) is ultimately bounded. This method is similar to ^[36], here, we omit it.

3.   Global dynamics analysis for model (1.2)

3.1.   Existence and globally asymptotic stability of the microorganisms-extinction periodic solution

At first, we discuss the case in which the microorganisms eventually become extinct. To this end, let $ X(t) = 0 $ in system (1.2), a low dimensional system is obtained as follows,

Then according to Lemma 2.1, the system (3.1) has a globally attractive positive $ T $ periodic solution $ (S^*(t), P^*(t)), $ where

thus, for system (1.2), we know that system (1.2) has a microorganisms-extinction periodic solution $ (S_0, 0, P^*(t)). $

Next, we further investigate the globally asymptotic stability of solution $ (S_0, 0, P^*(t)). $ Let

then the following theorem is obtained.

Theorem 3.1. If $ \mathscr{R'} < 1, $ then the microorganisms-extinction periodic solution $ (S_0, 0, P^*(t)) $ is globally asymptotical stable.

Proof. The proof is divided into two steps. Firstly, we prove that the microorganisms-extinction periodic solution is locally stable by using Floquet theory^[62] and a small amplitude perturbations methods. By transformation, let $ S(t) = v_{1}(t)+S_0, X(t) = v_{2}(t), P(t) = v_{3}(t)+P^*(t), $ for $ 0 \le t < T, $ there may be written

where $ \Psi $ satisfies

and $ \Psi(0) $ is the identity matrix. Thus we get

Linearizing the impulse expression of system (1.2) yeilds

Let

the eigenvalues are $ \lambda_1 = e^{-DT} < 1, \lambda_2 = {e^{\int_0^T \left(\frac{\mu_m{S}_0}{K_m+S_0}-D-\frac{h_2}{K_s}P^*(t)\right)dt}}, \lambda_3 = e^{-DT} < 1. $ If

then $ \lambda_2 < 1. $ By calculating the above integral, we can get

if let

obviously, if $ \mathscr{R} < 1, $ then $ \mid\lambda_2\mid < 1. $ While, we let

Obviously, $ \mathscr{R'} > \mathscr{R}. $ Thus if $ \mathscr{R'} < 1, $ we have $ \mid\lambda_2\mid < 1. $ Therefore by Floquet theory^[62], we obtain that $ (S_0, 0, P^*(t)) $ is locally stable.

Next step, we will discuss the globally attractive. Since $ \mathscr{R'} > 1, $ we can choose two arbitrary positive numbers $ \varepsilon_1, \varepsilon_2 $ such that

From system (1.2), one can get

Construct the auxiliary system

by Lemma 2.1, we have $ u_{1}(t)\rightarrow S_0 $ as $ t\rightarrow \infty. $ By the comparison theorem of ODEs^[64], we have $ S(t)\leq u_{1}(t), $ then for arbitrarily small positive number $ \varepsilon_1, $

holds for all $ t $ large enough.

On the other hand, we can also get from system (1.2),

then construct the auxiliary system

by Lemma 2.1, we have $ u_{2}(t)\rightarrow u_{2}^*(t) $ ($ t\rightarrow \infty $), where

is the periodic solution of system (3.5). According to the comparison theorem of IDEs, we obtain $ P(t)\geq u_{2}(t) $ and for sufficiently small $ \varepsilon_2 > 0, $

holds for all $ t $ large enough.

On the other hand, by the second equation, the fifth equation, (3.3) and (3.6), one can get that

for all $ t $ large enough.

Integrating both sides of (3.7) from $ nT $ to $ (n+1)T $ yields

Then we have $ X(nT)\leq X(0^+)e^{n\delta}. $ Since $ \delta < 0, $ hence $ X(nT)\rightarrow 0 $ and because $ 0\leq X(t)\leq X(nT)\exp\left(\frac{\mu_mS_0}{K_m+S_0}\right)T $ holds for $ nT < t\leq(n+1)T, $ therefore, we get $ X(t)\rightarrow 0 $ as $ t\rightarrow\infty. $

Since $ \lim_{t\rightarrow+\infty}x(t) = 0, $ thus for any $ \varepsilon > 0, $ there has a $ T_{1} > 0 $ such that $ X(t) < \varepsilon $ for $ t\geq T_{1}. $ Then by first equation of (1.2), we get

Consider the following auxiliary systems

and

Let $ z_1(t) $ and $ z_2(t) $ are solutions of (3.8) and (3.9), respectively. There have $ z_1(t)\rightarrow \frac{D}{D+\frac{\varepsilon\mu_m}{\delta K_m}}S_0, $ $ z_2(t)\rightarrow S_0 $($ t\rightarrow\infty $). Thus for any $ \varepsilon_1 > 0, $ there exist $ T_{2} > 0 $ and $ T_{3} > 0 $ such that

for $ t > T_{2} $ and $ t > T_{3}, $ respectively. By the comparison theorem of IDEs, for any $ \varepsilon_{1}, $ there must exist $ T_{4} = \max\{T_{2}, T_{3}\} $ such that

for $ t > T_{4}. $ Then we have

Let $ \varepsilon\rightarrow0, $ we get

i.e., $ S(t)\rightarrow S_0 $ as $ t\rightarrow \infty. $

Similarly, from system (1.2), one can get that

Consider the following auxiliary systems

and

where $ u_3(t) $ and $ u_4(t) $ are solutions of (3.10) and (3.11), respectively. Then have $ u_3(t)\rightarrow u_3^*(t), u_4(t)\rightarrow P^*(t) $($ t\rightarrow \infty $), where $ u_3^*(t) = \frac{\gamma P_0 e^{-(D+\frac{\varepsilon h_3}{K_s+\varepsilon})(t-nT)}}{1-e^{-(D+\frac{\varepsilon h_3}{K_s+\varepsilon})T}}, nT < t\leq(n+1)T. $ Thus for any $ \varepsilon_2 > 0, $ there exist $ T_{5} > 0 $ and $ T_{6} > 0 $ such that

for $ t > T_{5} $ and $ t > T_{6}, $ respectively. By the comparison theorem of IDEs, for any $ \varepsilon_{2}, $ there must exist $ T_{7} = \max\{T_{5}, T_{6}\} $ such that

then we obtain

let $ \varepsilon\rightarrow0, $ then we have $ P(t)\rightarrow P^*(t) $ ($ t\rightarrow \infty $). The proof is completed.

3.2.   Permanence

Theorem 3.2. System (1.2) is permanent if $ \mathscr{R} > 1 $ holds.

Proof. By Lemma 2.3, we obtain that the solution of system (1.2) is ultimately bounded. Then to prove the permanence of system (1.2), we only need find three positive constants $ l_{1}, $ $ l_{2} $ and $ l_{3} $ such that $ S(t)\geq l_1, X(t)\geq l_2, P(t)\geq l_3 $ for $ t $ large enough.

We assume $ S(t), X(t), P(t)\leq M $ for all $ t\geq 0. $ At first, from system (1.2), we obtain

By the comparison theorem of ODEs, we can derive that

for $ t $ large enough. And from (3.4), (3.5) and (3.6), we can obtain

for $ t $ large enough. Next, we aim to get $ l_2 > 0 $ such that $ X(t) > l_2 $ for $ t $ large enough. We will implement it in two steps.

Step one. Choose $ l_4 = \frac{D\delta(\mathscr{R}-1)(K_m+S_0)}{\mu_m(\mathscr{R}+1)}, $ because $ \mathscr{R} > 1, $ then $ l_4 > 0. $ Let

where $ \alpha = \frac{DS_0}{D+\frac{\mu_{m}l_4}{\delta K_m}}. $ Since $ \mathscr{R} = \frac{\frac{\mu_mS_0}{K_m+S_0}T}{DT+\frac{h_2\gamma P_0}{K_sD}}, $ then we have

thus we have

Therefore, there exist two positive constants $ \varepsilon_1, \varepsilon_2 $ small enough such that

In the following, we shall show that $ x(t) < l_4 $ cannot hold for all $ t\geq 0. $ If not, let us return to system (1.2), by simple inequality, we have

By the comparison theorem of ODEs, for $ t $ large enough we have

From (3.12), we obtain

Thus by (3.14) and (3.15), we obtain

Integrating (3.16) on $ (nT, (n+1)T], n\in N $ yields

Then we obatain $ X((N +n)T)\geq X(NT)e^{n\eta}\rightarrow \infty $ as $ n\rightarrow\infty, $ which is contradictory with the boundedness of system (1.2). Hence there has a $ t_1 > 0 $ such that $ X(t_1)\geq l_4. $

Step two. If $ X(t) > l_4 $ for all $ t\geq t_1, $ then our goal is achieved. Otherwise, denote $ t^\vartriangle = \inf_{t > t_1} \{X(t) < l_4\}, $ then we have $ X(t)\geq l_4 $ for $ t\in [t_1, t^\vartriangle] $ and $ X(t^\vartriangle) = l_4, $ assume there exists $ n_1\in Z_+ $ such that $ t^\vartriangle\in(n_1T, (n_1+1)T). $ According to the change of $ x(t) $ in the interval $ (n_1T, (n_1+1)T), $ there have two subcases to be discussed.

Case one, If $ X(t)\leq l_4 $ for all $ t\in(t^\vartriangle, (n_1+1)T). $ Let $ n_2, n_3\in N $ such that

where

We can confirm that there has a $ t_2\in[(n_1+1)T, (n_1+1+n_2+n_3)T] $ such that $ X(t_2) > l_4. $ If not, let us consider the following system,

and

we have

and

for $ t\in[(n_1+1)T, (n_1+1+n_2+n_3)T]. $ Then

and

this makes (3.16) hold for $ t\in[(n_1+1)T, (n_1+1+n_2+n_3)T]. $ Thus integrating both sides of (3.16) from $ (n_1+1+n_2)T $ to $ (n_1+1+n_2+n_3)T] $ yields

On the other hand, we can also obtain from system (1.2),

by integrating both sides of system (3.18) from $ t^\vartriangle $ to $ (n_1+1+n_2)T, $ we get

From the above system and (3.17), one can have

The result is contradictory.

Denote $ \tilde{t} = \mathop{\inf}\limits_{t > t^\vartriangle}\left\{{X(t) > l_4}\right\}, $ then for $ t\in(t^\vartriangle, \tilde{t}), X(t)\leq l_4 $ and $ X(\tilde{t}) = l_4. $ For $ t\in(t^\vartriangle, \tilde{t}), $ suppose $ t\in(n_1T+(k-1)T, n_1T+kT'], k\in Z_+, k\leq 1+n_2+n_3, $ one can get

Let $ l_2 = l_4e^{(1+n_2+n_3)\delta T} < l_4, $ then $ X(t)\geq l_2 $ for $ t\in(t^\vartriangle, \tilde{t}). $ For the case $ t > \tilde{t}, $ the same arguments can be continued since $ x(\tilde{t})\geq l_4. $ Thus $ X(t)\geq{l}_2 $ for all $ t > t_1. $

Case two, there has a $ t\in(t^\vartriangle, (n_1+1)T) $ such that $ X(t) > l_4. $ Choose $ t^{\vartriangle\vartriangle} = \mathop{\inf}\limits_{t > t^\vartriangle}\left\{{X(t) > l_4}\right\}, $ then for $ t\in(t^\vartriangle, t^{\vartriangle\vartriangle}), X(t)\leq l_4 $ and $ X(t^{\vartriangle\vartriangle}) = l_4. $ For $ t\in(t^\vartriangle, t^{\vartriangle\vartriangle}), $ (3.18) holds true, by integrating (3.18) from $ t^\vartriangle $ to $ t^{\vartriangle\vartriangle}), $ we can get

Because $ X(t^{\vartriangle\vartriangle})\geq l_2 $ for all $ t > t^{\vartriangle\vartriangle}, $ the same arguments can be continued. Thus $ X(t)\geq {l}_2 $ for all $ t\geq t_1. $ This completes the proof of Theorem 3.2.

4.   Control strategy for microorganisms treatment

In Section 3, we get the thresholds which can determine the extinction and existence of the microorganisms. In this section, we investigate the control strategy for microorganisms treatment based on the thresholds. By theorem 3.1, if the threshold $ \mathscr{R}' < 1, $ then the microorganisms-extinction periodic solution $ (S_0, 0, P^*(t)) $ is globally asymptotically stable. Biologically, the microorganisms will eventually become extinct. Then, in order to eliminate harmful microorganisms in sewage, we can take the following two control strategies.

Denote

Control strategy Ⅰ. Shorten the time interval $ T $ for entering the flocculants such that $ T < T^*. $ Biologically, shorter pulse action intervals increase the efficiency of using flocculants, which helps to eliminate the microorganisms in sewage.

Control strategy Ⅱ. Keep the time interval between the input of flocculant and increase the amount of flocculant such that $ P_0 > P_0^*. $ Biologically, higher concentrations of flocculants help to eliminate microorganisms.

Also, we can get the threshold value of $ \frac{P_0}{T}, $ the average amount of flocculants added in the long run. Whether it is shortening the time interval $ T $ such that $ T < T^* $ or increasing the amount of flocculant such that $ P_0 > P_0^*, $ it will eventually increase the usage of flocculant per unit of time $ \frac{P}{T} $ such that $ \frac{P}{T} > [\frac{P_0}{T}]^{\vartriangle}, $ while higher concentrations of flocculants is beneficial to the removal of harmful microorganisms.

Under above control strategies, we can achieve the treatment process of microorganisms by reducing the time interval for adding flocculants and increase the amount of flocculant used in each treatment cycle in the actual sewage treatment process.

5.   An example and computer simulations

In this section, we give an example with some computer simulations to illustrate the theoretical results and the the control strategy. Let basic parameters be $ S_0 = 4, D = 0.5, h_2 = 2.5, h_3 = 0.05, \mu_m = 1.5, \delta = 0.1, K_m = 0.5, K_s = 0.5, K_a = 0.5, \gamma = 1, $ then we can obtain

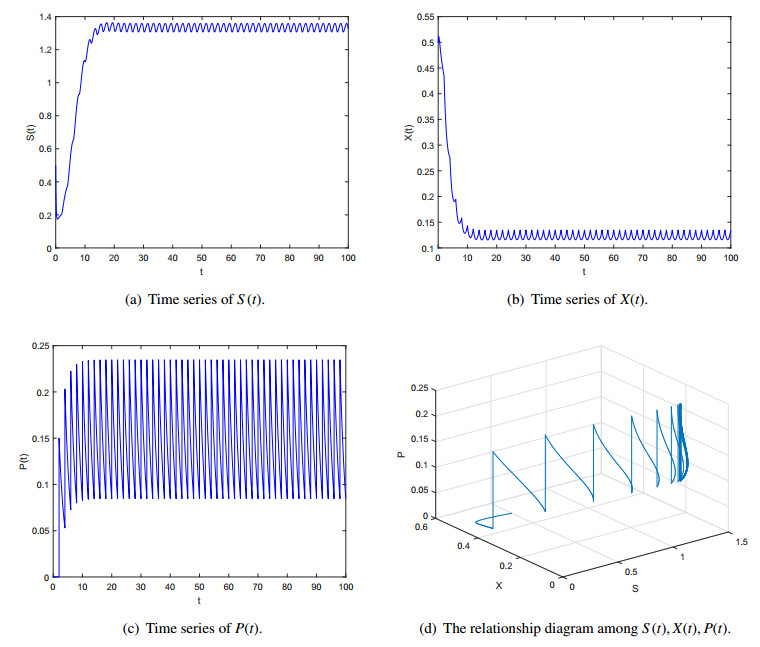
Let the initial value be $ (0.5, 0.5, 0). $ First, we let $ P_0 = 0.15, T = 2, $ by simple calculation, we obtain $ \mathscr{R} = 1.0667 > 1, $ by Theorem 3.2, we know that the system is permanent. Biologically, the microorganisms will exist in sewage for a long time. Numerical simulation shows, with the periodical input of flocculant (see Figure 2(c)), the microorganisms and substrate produce periodic oscillations (see Figure 2(a) and 2(b)). Mathematically, the system produces a global asymptotically stable periodic solution (see Figure 2(d)).

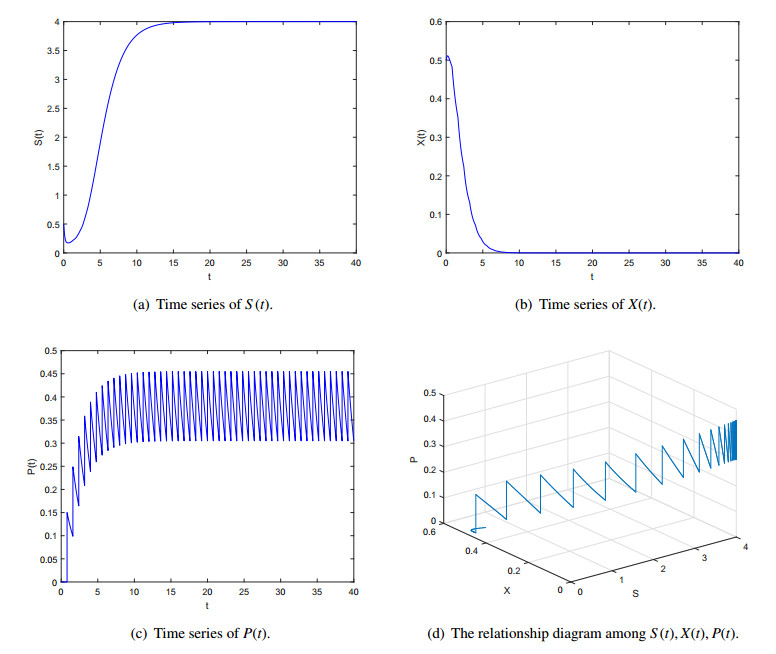
In order to verify the control strategy Ⅰ, we choose to reduce the period $ T $ of the pulse action (for example, from 2 to 0.8), by simple calculation, we obtain $ \mathscr{R}' = 0.8942 < 1. $ By Theorem 3.1, the system has a globally asymptotically stable microorganisms-extinction periodic solution $ (S_0, 0, P^*(t)) $ (see Figure 3(d)), this makes the microorganisms eventually extinct (see Figure 3(b)). At this time, the substrate and flocculant approach the periodic solution $ (S_0, P^*(t)) $ due to the action of the pulse (see Figure 3(a) and 3(c)).

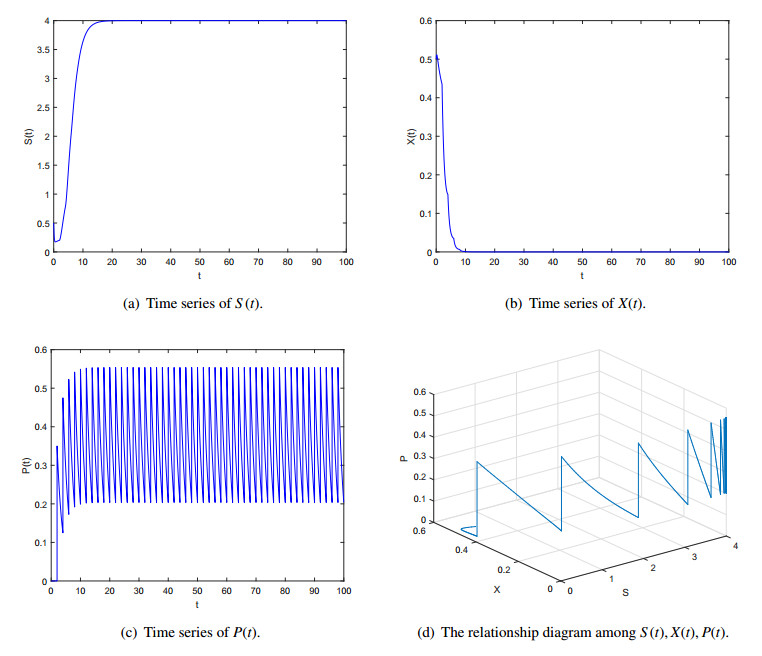
Next, in order to verify the control strategy Ⅱ, we increase the amount of flocculant used $ \gamma P_0 $ (for example, from $ 0.15 $ to $ 0.35 $, and other parameters are the same with those in Figure 2). By calculation, we obtain $ \mathscr{R}' = 0.9357 < 1. $ By Theorem 3.1, the system produces a global asymptotically stable periodic solution (see Figure 4(d)), that is, the microorganisms will eventually extinct.

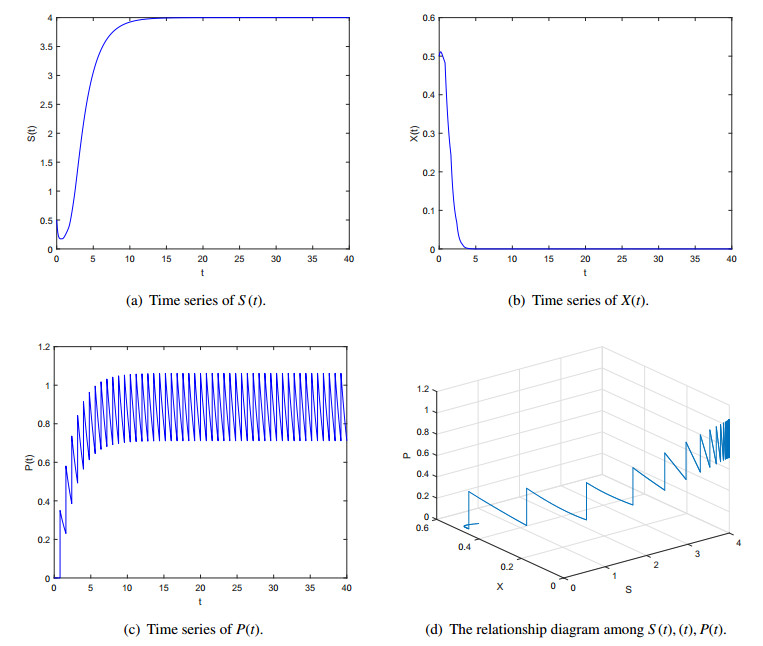
If we use strategies Ⅰ and Ⅱ at the same time, the harmful microorganisms can be removed more quickly (see Figure 5(b)).

In summary, computer simulations show that the control strategy is effective. The time of the pulse action $ T $ and the amount of flocculant used $ \gamma P_0 $ have an important influence on the removal of harmful microorganisms. From Figure 2(b), Figure 3(b) and Figure 4(b), we can see that the harmful microorganisms that have existed for a long time in the system will eventually be eliminated under the control strategy Ⅰ or Ⅱ.

6.   Conclusion

In this paper, a mathematical model by impulsive differential equations is proposed to describe the process of eliminating microorganisms from livestock and poultry sewage by adding microbial flocculants. In the model, we establish the law of variation among substrate, microorganisms and flocculants. Using standard mathematical theories and methods, we analyze the evolution of variables over time in the model system. We prove that the system has a microorganisms-extinction periodic solution which is globally asymptotically stable if $ \mathscr{R'} < 1. $ Biologically, this means that microorganisms will eventually be eliminated from the system. And if $ \mathscr{R} > 1, $ we prove that the system is permanent, that is, the substrate, microorganisms and flocculants will coexist for a long time. Based on theoretical analysis, we discuss control strategies for eliminating microorganisms. Our results show that microorganisms can be eliminated by adjusting the time interval for adding flocculants and the amount of flocculant used.

Acknowledgments

The first author was supported by Shandong Provincial Natural Science Foundation from China (No. ZR2019MA003), SDUST Research Fund (2014TDJH102) and Scientific Research Foundation of Shandong University of Science and Technology for Recruited Talents. The fifth author was supported by National Natural Science Foundation of China (Nos. 11801014), Natural Science Foundation of Hebei Province (No. A2018409004) and Hebei province university discipline top talent selection and training program (SLRC2019020) and Talent Training Project of Hebei Province.

Acknowledgments

The authors declare there is no conflicts of interest.