Photogravitational perturbations in the infinitesimal orbits around the libration points in the oblate RTBP

1.   Introduction

The meaning of the infinitesimal orbits are defined as follows: Those orbits that are very close to the equilibrium points. The radii of these orbits are very small. The scientific significance of these orbits come from the fact that the mission designers require to place missions at equilibrium points to have the advantage of these points. The infinitesimal orbits are already used for the mission near any point of the equilibrium points.

The restricted three bodies problem (in brief RTBP) can be investigated directly from the equations of motion given in any textbook of celestial mechanics. Authors can treat this problem even though with some considered perturbations: e.g., relativistic, photogravitational, dynamical shapes of the primaries, drag, ....etc. The history of the restricted problem is so long as the beginning of the reviviscence era began with Euler and Lagrange continues with Jacobi, Poincaré and Birkhoff. It continues intensively to the date, one cannot conclusive survey of these works but some relevant works are; Ahmed, et al. ^[1], Douskos and Perdios ^[2], Abd El-Salam and Abd El-Bar ^[3,4], Abd El-Salam and Katour ^[5], and Abd El-Salam ^[6]. The Infinitesimal orbits around the equilibrium points in the restricted three body problem (in brief RTBP) are very important for space community. NASA in 1978 launched ISEE3 into a halo orbit at the L₁ of the Earth-Sun system. It was designed to study the Earth-Sun connection through the interaction between the magnetic field of the Earth and the solar wind. In 1996 the SOHO mission was launched to investigate deepily the Sun's internal structure, the solar extensive outer atmosphere and the solar wind origin.

The dynamical behavior of the test particle near the libration points, namely the infinitesimal orbits has been collected in a work by Duncombe and Szebehely ^[7]. Richardson ^[8] used successive approximations technique in conjunction with a proceedure similar to Poincare-Lindstedt technique to obtain a 3^rd order analytical solution of halo type periodic orbits applied for the Earth-Moon system. Barden and Howell ^[9] used the centeral manifold theory to analyze the motions in the vicinity of the collinear equilibrium points. Howell ^[10] studied the families of orbits in the neighbourhood of the collinear libration points.

Gomez, et al. ^[11] gave some families of quasi-halo orbits in Sun- (Earth-Moon) and in Earth-Moon systems around L₁ and L₂. While Gomez, et al. ^[12] treated the transfer problem between infinitesimal orbits. Selaru and Dimitrescu ^[13] used asymptotic approximations based on Von Zeipel-type method to study the motions in the vicinity of a equilibrium point in the planar elliptic problem. The eccentricity and inclination effects on small amplitude librations around the triangular points L₄ and L₅ have been studied by Namouni and Murray ^[14]. The analytical continuation method have been used by Corbera and Llibre ^[15] to investigate the symmetric periodic orbits around a collinear point is the RTBP. Hamdy, et al. ^[16] used a perturbation proceedure based on Lie series to develope explicit analytical solutions for infinitesimal orbits about the equilibrium points in the elliptic RTBP.

Abd El-Salam ^[17] studied the periodic orbits around the libration points in the relativistic RTBP. He analysed the elliptic, hyperbolic and degenerate hyperbolic orbits in the vicinity of the L₁, L₂ and L₃. He found as well as elliptic orbits in the neighborhood of the L₄ and L₅.

Ibrahim, et al. ^[18] presented the special solutions of the RTBP specifying the locations of the equilibrium points. They obtained periodic orbits around these libration points analytically and numerically. Tiwary, et al. ^[19] described a third-order analytic approximation for computing the three-dimensional periodic halo orbits near the collinear L₁ and L₂ Lagrangian points for the photo gravitational circular RTBP in the Sun-Earth system. Tiwary and Kushvah ^[20] computed halo orbits using Lindstedt-Poincaré method up to fourth order approximation, then analyzed the effects of radiation pressure and oblateness on the orbits around Libration points L₁ and L₂.

In this work, we will use the Hamiltonian approach to compute the infinitesimal trajectories around the equilibrium points. We will construct first the Hamiltonian of the problem, then it will be followed by outlining of the perturbation proceedure used, namely the Delva-Hanselmeir technique, Delva ^[21] and Hanslmeier ^[22].

Mittal, et al. ^[23] have studied periodic orbits generated by Lagrangian solutions of the RTBP when both of the primaries is an oblate body. They have illustrated the periodic orbits for different values of the problem parameters.

Peng, et al. ^[24] proposed an optimal periodic controller based on continuous low-thrust for the stabilization missions of spacecraft station-keeping and formation-keeping along periodic Libration point orbits of the Sun-Earth system.

Peng, et al. ^[25] presented the nonlinear closed-loop feedback control strategy for the spacecraft rendezvous problem with finite low thrust between libration orbits in the Sun-Earth system.

Jiang ^[26] investigated the equilibrium points and orbits around asteroid 1333 Cevenola by considering the full gravitational potential caused by the 3D irregular shape. They calculated gravitational potential and effective potential of asteroid 1333 Cevenola. They also discussed the zero-velocity curves for a massless particle orbiting in the gravitational environment.

Wang ^[27] applied the developed symplectic moving horizon estimation method to the Earth-Moon L₂ libration point navigation. their numerical simulations demonstrated that though more time-consuming, the proposed method results in better estimation performance than the EKF and the UKF.

We aim to give the explicit formulas for coordinate and momenta of the infinitesimal orbits around one of the libration points in the photogravitational oblate RTBP. The article is organized as follows: In section 1, we gave a brief introduction. While in section 2, we formulated the Hamiltonian in rotating frame of reference. In section 3 we transformed the Hamiltonian near any one of the equilibrium point in the considered model. In section 4 we outline the perturbation approach used. In section 5, and its subsequent subsections we computed the coordinate and momentum vectors of an infinitesimal body revolving one of the equilibrium point in a halo orbit. At the end of the paper we summarize our obtained results.

2.   Hamiltonian in inertial frame of reference

The Lagrangian of the problem can be obtained from

where $\mathcal{L}$ is the Lagrangian of the problem, $T$ and $U$ are the kinetic and potential energies of the system respectively, written in terms of the generalised coordinates and velocities and the time $\left(\boldsymbol{q} \equiv q_{i}, \boldsymbol{\dot { q }} \equiv \dot{\boldsymbol{q}}_{i} ; t\right),$ $i = 1, 2, ...n.$

The Legendre transform allows us to switch from the Lagrangian to the Hamiltonian formulism,

where the Hamiltonian function $\mathcal{H} \equiv \mathcal{H}\left({\boldsymbol{q}, \boldsymbol{p}} \right)$ is a function in the generalized coordinates $q$ , and the conjugate generalized momenta $\boldsymbol{p}$ . The Lagrangian describing the motion of the infinitesimal mass in the inertial frame of reference is given by

where $\dot X$ and $\dot Y$ are the velocity components in the inertial frame of reference of the infinitesimal particle. We will assume the potential energy $U$ of the system is given, in the inertial frame of reference, by

where ${A_1}$ and ${A_2}$ denote to the oblateness coefficients of the more and less massive primaries respectively such that $0 < {A_i} \ll 1, \,$ ( $\, i = 1, 2$ ), the respective radiation factors for the massive and less massive primaries are ${q_i},$ ( $\, i = 1, 2$ ) such that $0 < 1 - {q_i} < 1$ , $\mu = {m_2}/({m_1} + {m_2}), \, \, \, \mu \in \left({0, 1/2} \right)$ is the mass ration of the less massive body to the total mass of the system, and $X, Y$ are the coordinate components in the inertial frame of reference of the infinitesimal particle.

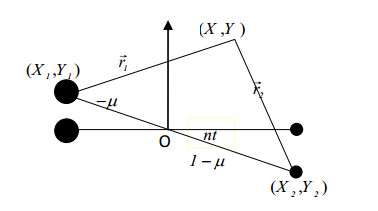
Since the trajectories of the primaries are given by

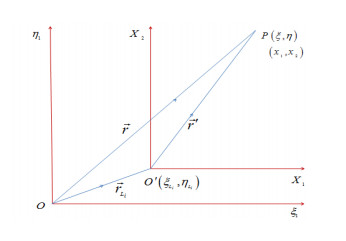
where $nt,$ the angle of rotation, is the product mean motion $n$ and time $t$ of the problem, and the location of the infinitesimal body with respect to the primaries in the inertial frame, see Figure 1, is

The ameneded potential $U$ and hence the inertial Lagrangian ${\text{L}_{{\rm{inertial}}}}$ is a time independent. To have a clearer insight into many behaviours of the RTBP epecifically the motion near the Lagrangian points, transform to a rotating system with coordinates $\xi$ and $\eta$ using

and the the corresponding velocities transform as

where $\dot \xi$ and $\dot \eta$ are the velocity components in the rotating system. The distances become

and the new Lagrangian is

Where the amended potential $U$ is given by

The distances of the infinitesimal mass from the barycenter is given by $\, r = \sqrt {{\xi ^2}\, + {\eta ^2}}$ , and $n$ is given by

Now, to formulate the Hamiltonian in terms of the generalized coordinates $\boldsymbol{q} \equiv \left({\xi, \eta } \right)$ and their canonical conjugate momenta $\boldsymbol{p} \equiv \left({{p_\xi }, {p_\eta }} \right)$ , the follwing relations are required

From Eqs. (9) and (10), we can obtain

solution for $\dot \xi$ and $\dot \eta$ yields

The Hamiltonian in the rotating frame is given as

or, using Eq. (15),

Using Eqs. (9), (10) and (15), the Hamiltonian of the problem in terms of the generalized coordinates $\boldsymbol{q} \equiv \left({\xi, \eta } \right)$ and the generalized momenta $\boldsymbol{p} \equiv \left({{p_\xi }, {p_\eta }} \right)$ can be written in the form

3.   Transformed Hamiltonian near any equilibrium point

At this point we are interested in the infintesimal orbits near any equilibrium point. Moving the origin to any point of equilibrium and denoting to the new coordinates and momenta be $\left({{x_1}, \, \, \, {x_2}, \, \, \, {P_{{x_1}}}, \, \, \, {P_{{x_2}}}} \right)$ , then from the gemetry illustrated in Figure 2, we have

from Eq. (19)

where $\left({{\xi _{{L_i}}}, {\eta _{{L_i}}}} \right), \, \, i = 1, 2, 3, 4, 5$ are the locations of the equilibruim points, given by Abd El-Salam, et al. ^[28], Abd El-Salam and Abd El-Bar ^[29,30], disregarding the relativistic effects in these works. The new momenta read

Now to obtain the Hamiltonian in the new coordinates, we substitute Eqs. (20) and (21) into Eq. (18) :

where

4.   Perturbation approach and solutions

We utilize an approach developed by Delva ^[21], and Hanslmeier ^[22]. They carried out the procedure with a differential operator $D$ , the Lie operator, which is a special linear operator that produces a Lie series. The convergence of this latter series is the same as Taylor series, It merely represents another form of the Taylor series whose terms are generated by the Lie operator. We will use the Lie series form for two reasons. The first reason is: The requirement to build up a perturbative scheme at different orders of the orbital elements. The second reason is: Its usefulness also in treating the non-autonomous system of differential equations and non-canonical systems. This enables a rapid successive calculation of the orbit. In addition we can arbitrarily choose the stepsize easily (if necessary). This is an important advantage for the treatment of the problems which has a variable stepsize, e.g., for the mass change of the primaries. The formulas has an easy analytical structure and may be programmed without difficulty and without imposing extra conditions on the convergence. The iteration can be used to generate any desired order of solution, the series can be continued up to any satisfactory convergence reached. The Lie operator is defined as

Leibnitz formula can be used for computing the ${n^{\underline t h}}$ derivative of a product, as

The ${n^{\underline t h}}$ application of the Lie operator denoted by ${D^{\; \left(n \right)}}$ takes the form

Now using the canonical equations of motion

we can evaluate the derivatives $\frac{{{d^{n - m}}{x_i}}}{{d{t^{n - m}}}}$ , $\frac{{{d^{n - m}}{P_{{x_i}}}}}{{d{t^{n - m}}}}$ then we can reach to the solutions (coordinate and momentum vectors, $\boldsymbol{x}$ and ${\bf{P}}$ respectively) as;

As is clear from Eq. (27), the applications of the Lie operator ${D^j}\Xi$ at different orders are evaluated for the initial conditions of the canonical elements.

5.   Solutions at different orders

In this section we are going to evaluate the solutions at different orders. From the definition of the operator ${D^{\left(n \right)}}$ , Eq. (26), we get the following explicit expressions at different orders as follows.

5.1.   The first order solution

Setting $n = 1$ in Eq. (26) we obtain the required coefficients in Eq. (27) to yield the first order. The required partial derivatives can be obtained using Eq. (22) as follows

Substituting Eqs. (28.1)–(28.4) into Eq. (26) and neglecting the very small magnitude terms yields

Where the nonvanishing included coefficients are given by

5.2.   The second order solution

Setting $n = 2$ in Eq. (26) we obtain the required coefficients in Eq. (27) to yield the second order solution as;

Substituting Eqs. (30.1)–(30.6) into Eq. (26) and neglecting the very small magnitude terms yields yields the second order solution as

Where the nonvanishing included coefficients are given by

6.   Conclusion

We can conclude our work in this research as follows: First we have outlined briefly the restricted three body problem, then we defined the infinitesimal orbits. We expressed the photogravitational oblate RTBP in both inertial and rotated coordinate systems. The Hamiltonian of the problem under investigation is constructed. Then it is transferred to any point of the equilibruim point as an origin. We have reviewed the Lie operator method, as a method of solution. Finally we have obtained the explicit first order as well as the second order solutions for the coordinates and their conjugate momenta of a test particle in an infinitesimal orbit around any equilibrium point.

Conflict of interests

The authors declare that there are no Conflict of interests associated with this work.