In this paper, we investigate the dynamics of a stochastic SIRS epidemic model with non-linear incidence rate and transfer from infectious to susceptible. Firstly, the existence and uniqueness of global positive solution of the model with any positive initial value are proved. Next, sufficient conditions for extinction and persistence of the disease are established. It is found that a large noise intensity has the effect of suppressing the epidemic. At last, some numerical simulations are introduced to demonstrate the theoretical results. Our results generalize and improve the existing results.
1.
Introduction
Mathematical models describing the population dynamics of infectious diseases have been playing an important role in better understanding of epidemiological patterns and disease control for a long time (see [1,2,3,4]). Some infectious diseases confer temporary or permanent immunity. For some diseases, such as cholera, pertussis and influenza, temporary immunity may disappear after some time. And recovered individuals will become susceptible after losing the temporary immunity. This kind of diseases can be modeled by SIRS models (see [5,6]). In addition, for some bacterial agent diseases such as meningitis and venereal diseases, recovery cannot generate immunity for a long time. Infected individuals may recover after some treatments and go back directly to the susceptible class on account of the presence of temporary antibodies. In [6], the authors investigated the following SIRS epidemic model with a nonlinear incidence βSf(I) and transfer from the infected class to the susceptible class
with the initial conditions
where S(t), I(t) and R(t) are respectively the number of susceptible, infectious and recovered individuals at time t. Λ is the recruitment rate of susceptible individuals, μ is the natural death rate, α is the mortality caused by the disease, γ1 denotes the transfer rate from the infected class to the susceptible class, γ2 is the recovery rate of the infective individuals, δ is the rate constant for loss of immunity. Suppose that Λ and μ are positive, while α, γ1, γ2 and δ are nonnegative. f is a real locally Lipschitz function on R+=[0,∞) satisfying f(0)=0, f(I)>0, f(I)/I is nonincreasing for I>0 and limI→0+f(I)I=f′(0)>0.
Denote R3+={(x,y,z)∈R3:x>0,y>0,z>0}. From [6], model (1.1) always has a disease-free equilibrium E0=(S0,I0,R0)=(Λμ,0,0). By using the next generation matrix method, [6] obtained the basic reproduction number R0=Λβf′(0)μ(μ+γ1+γ2+α). Further, E0 is globally asymptotically stable in D if R0<1, where D={(S,I,R)∈R3+:S+I+R≤Λμ}. If R0>1, there exists a globally asymptotically stable endemic equilibrium E∗=(S∗,I∗,R∗).
However, model (1.1) is just a deterministic model. In the real world, epidemic models are always affected by the environmental noise. Stochastic models may be a more appropriate way of modeling epidemics in many circumstances (see [7,8,9,10]). In [11,12,13], the authors investigated the stochastic SIS epidemic models. In [14,15,16,17,18,19,20], the authors investigated the stochastic SIRS epidemic models. In this paper, we investigate the following stochastic SIRS epidemic model
with initial values S0>0,I0>0,R0>0. Here we consider random perturbation in the environment, which is assumed to be affected by the contact rate, so that β→β+σ˙B(t), where B(t) is a standard Brownian motions defined on the compete probability space (Ω,F,P) with a filtration {Ft}t≥0 satisfying the usual conditions (i.e. it is right continuous and F0 contains all P-null sets). σ2 represents the intensity of B(t). Other parameters are defined as system (1.1). Model (1.3) covers many existing stochastic models in [6,9,21,22,23] as special cases. For example, an epidemic model with relapse and bilinear incidence was formulated in [9]. In [21], the authors investigated the dynamics of a stochastic SIR epidemic model with saturated incidence. In [22], extinction and persistence of a stochastic SIRS epidemic model with saturated incidence rate and transfer from infectious to susceptible were considered. In [23], the authors proposed a class of stochastic SIRS epidemic models with nonlinear incidence
where h(S) is continuously differentiable and monotonically increasing with respect to S, h(0)=0 and for any constant l>0, Ml:=sup0<S≤lh(S)S<∞; f(I) is nonnegative and twice continuously differentiable, f(I)/I is monotonically decreasing with respect to I, f(0)=0 and f′(0)>0. Other parameters are defined as those of system (1.3). In [23], the sufficient conditions for the extinction and persistence of the disease for model (1.4) were discussed. Compared with model (1.3), model (1.4) does not include the transfer from infectious to susceptible, although the incidence rate is more general.
Denote a∧b=min{a,b} for a,b∈R and E(X) represents the exception of random variable X. Throughout this paper, we give the following assumptions.
(H1) f(0)=0, f(I)>0 holds for I>0 and f is a real locally Lipschitz function on R+;
(H2) f(I)/I is nonincreasing on (0,+∞) and f′(0)>0;
(H3) There is a constant ϑ>0, such that
One can easily see from (H2) that
Our general results can be applied to some specific forms of the incidence rate, for instance:
(ⅰ) linear type: f(I)=I (see [9]);
(ⅱ) saturated incidence rate: f(I)=I1+aI (see [21,22]);
(ⅲ) non-monotonic incidence rate: f(I)=I1+aI2 (see [24]);
(ⅳ) incidence rate with "media coverage": f(I)=Ie−mI, where m is a positive constant (see [25]);
(ⅴ) incidence rate with "media coverage": f(I)=(1−˜βIβ(m+I))I, where m,β,˜β are all positive constants and β>˜β (see [26]).
Our aim is to investigate how white noise affects the spread of disease, as described by model (1.3). The remaining part of this paper is organized as follows. In Section 2, a unique global positive solution of stochastic model (1.3) with any positive initial value is proved. Sufficient conditions for disease extinction and persistence in the mean are given in Sections 3 and 4, respectively. In addition, we discuss the relationship between R0 and Rs for different values of white noise intensity σ2, and summarize a useful criterion for the extinction and persistence of the disease of model (1.3). In Section 5, some examples and numerical simulations are provided to illustrate the theoretical results. The paper ends with a conclusion that the intensity of noise plays an important role in epidemic dynamics.
2.
Existence and uniqueness of global positive solution
Since S(t), I(t) and R(t) in model (1.3) are respectively the numbers of the susceptible individuals, infected individuals and recovered individuals at time t, they should be non-negative. Therefore, we are only interested in its positive solutions. In this section, we show that model (1.3) has a unique global positive solution with positive initial value by using the Lyapunov analysis method.
Theorem 1. Suppose that (H1) and (H2) hold. Model (1.3) has a unique global positive solution (S(t),I(t),R(t)) on [0,∞) for any (S0,I0,R0)∈R3+, that is, the solution will remain in R3+ with probability one.
Proof. From (H1), it is easy to show that the coefficients of (1.3) are locally Lipschitz continuous. Thus, for any given initial value (S0,I0,R0)∈R3+, there is a unique maximal local solution (S(t),I(t),R(t)) on [0,τe), where τe is the explosion time. Now we show τe=∞ a.s. Let n0>0 be sufficiently large such that S0,I0 and R0 all lie within the interval (1n0,n0). For each integer n≥n0, define the stopping time
where throughout this paper, we set inf∅=∞. Clearly, τn is increasing as n→∞. Let τ∞=limn→∞τn. Thus τ∞≤τe a.s. If we can show that τ∞=∞ a.s., then τe=∞ and (S(t),I(t),R(t))∈R3+ a.s. for all t≥0.
Now, we show that τ∞=∞ a.s. Otherwise, there is a pair of constants T>0 and ε∈(0,1) such that P{τ∞≤T}>ε. Hence, for all n≥n0
where Ωn={ω∈Ω:τn(ω)≤T}. Moreover, for any n≥n0 and t≤τn,
which implies
Denote K=max{Λμ,S0+I0+R0}. Then
for any t≤τn. Define a C2-function V:R3+→R+ by
For any n≥n0, the Itˆo formula shows that
where LV:R3+→R is defined by
From (1.5) and (2.2), for any 0≤t≤τn∧T
Thus, from (2.3), it follows that
for any n≥n0. Note that for every ω∈Ωn, there exists S(τn,ω), I(τn,ω) or R(τn,ω) that equals either 1/n or n. Hence
It follows from (2.1) that
where IΩn is the indicator function of Ωn. Letting n→∞ leads to the contradiction
therefore we have τ∞=∞ a.s. The proof is therefore complete.
Remark 1. Theorem 1 shows that for any initial value (S0,I0,R0)∈R3+, model (1.3) has a unique global solution (S(t),I(t),R(t))∈R3+ a.s. In addition, it follows from model (1.3) that
Thus, S(t)+I(t)+R(t)≤(S0+I0+R0−Λμ)e−μt+Λμ, which implies
Moreover, if S0+I0+R0≤Λμ, then S(t)+I(t)+R(t)≤Λμ a.s.. Therefore, the region
is a positively invariant set of model (1.3).
For simplicity, we introduce the notations X(t)=S(t)+I(t)+R(t), ⟨x(t)⟩=1t∫t0x(s)ds.
3.
Extinction of disease
One of the main concerns in epidemiology is how we can regulate the disease dynamics so that the disease will be eradicated in a long term. In this section, we provide the sufficient conditions for extinction of the disease in model (1.3). Denote
For model (1.3), we give the following conditions.
(C1) Rs<1, σ2≤βμΛf′(0) and σ2≤βμΛf′(0)⋅R02;
(C2) Rs<1, βμΛf′(0)⋅R02<σ2<βμΛf′(0);
(C3) Rs<1, σ2≥βμΛf′(0) and σ2>βμΛf′(0)⋅R02;
(C4) Rs≥1, σ2>βμΛf′(0)⋅R02.
In addition, denote A1=(μ+γ1+γ2+α)(Rs−1), A2=−[(μ+γ1+γ2+α)−β22σ2].
Theorem 2. Suppose that (H1) and (H2) hold. Let (S(t),I(t),R(t)) be the solution of model (1.3) with any given initial condition (S0,I0,R0)∈R3+.
(i) If (C1) holds, then lim supt→∞lnI(t)t≤A1<0a.s.
(ii) If (C2) holds, then lim supt→∞lnI(t)t≤min{A1,A2}<0a.s.
(iii) If (C3) or (C4) holds, then lim supt→∞lnI(t)t≤A2<0a.s.
Furthermore,
Proof. Applying Itˆo formula to the second equation of model (1.3) leads to
Integrating both sides of the above equation from 0 to t, yields
where M1(t)=∫t0σSf(I)IdB(s).
Let us discuss three cases separately.
Case 1. Assume that Rs<1 and σ2<βμΛf′(0). Then Λf′(0)μ<βσ2 and Φ(Λf′(0)μ)=(μ+γ1+γ2+α)(Rs−1)<0. For any 0<ε<βσ2f′(0)−Λμ sufficiently small, we have (Λμ+ε)f′(0)<βσ2 and Φ((Λμ+ε)f′(0))<0. From Remark 1, for any ε>0, there exists a constant T=T(ε)>0 such that S(t)≤Λμ+ε for all t>T. Then it follows from (1.5) that
Substituting this into (3.1), we have
Clearly, M1(t) is a real-valued continuous local martingale vanishing at time 0 and
Note that
then, from the strong law of large numbers (see [27]), it follows that
From (3.2) and (3.3), we have
By the continuity of Φ(t) and the arbitrariness of ε, we obtain
Case 2. Assume that Rs<1 and σ2=βμΛf′(0). Then Λf′(0)μ=βσ2 and Φ(Sf(I)I)≤Φ(Λf′(0)μ)<0. The following proof is similar to that of Case 1.
Case 3. Assume that σ2>βμΛf′(0)⋅R02. Since
Substituting this into (3.1), we have
This, together with (3.3), yields
From the proofs of Cases 1-3, it is easy to see that conclusions (ⅰ)-(ⅲ) hold. Further, if one of conditions (C1)–(C4) holds, then
That is to say, the disease I(t) dies out with probability one.
Next, we show limt→∞R(t)=0 a.s. Let Ω1={ω∈Ω:limt→∞I(t,ω)=0}, then (3.5) implies P(Ω1)=1. Hence, for any ω∈Ω1 and any constant ε1>0, there exists a constant T1=T1(ω,ε1)>0 such that for any t≥T1
Substituting this into the third equation of model (1.3), we obtain
Then, from the comparison theorem, it follows that
Since R(t,ω)>0 for all ω∈Ω1 and t>0, by the arbitrariness of ε1, we get limt→∞R(t,ω)=0, ω∈Ω1. It follows from P(Ω1)=1 that
At last, we prove the assertion limt→∞S(t)=Λμ a.s. It follows from model (1.3) that
which implies
For any t>T, integrating both sides of (3.8) from T to t and using (3.6), we obtain that for any ω∈Ω1
Thus, for any ω∈Ω1, we have lim inft→∞X(t,ω)≥Λ−αε1μ, which, together with the arbitrariness of ε1, yields
From P(Ω1)=1, we get lim inft→∞X(t)≥Λμ a.s. On the other hand, from Remark 1, it follows that lim supt→∞X(t)≤Λμ. Thus, we have
which, together with (3.5) and (3.7), yields
The proof is therefore complete.
Remark 2. Theorem 2 shows that the disease of system (1.3) dies out with probability one if Rs<1 and σ2<βμΛf′(0). Moreover, we note Rs in Theorem 2 is smaller than the basic reproduction number R0 of system (1.1), and hence environmental noise may lead the disease to extinction. If σ=0, then system (1.3) can be reduced to system (1.1). Further, Theorem 2 is consistent with the result in [6].
Remark 3. If we let f(I)=I1+aI,γ1=0,γ2=γ,δ=0, system (1.3) can be transformed into system (1.3) in [21]. From Theorem 2, it follows that for any initial condition (S0,I0,R0)∈R3+, if one of conditions (C1)–(C4) holds then the disease of system (1.3) will extinct with probability one. However, Theorem 3.1 in [21] shows that for any initial condition (S0,I0,R0)∈Γ, if σ2>β22(μ+γ+α), then the disease of system (1.3) will tend to zero exponentially with probability one. Obviously, Theorem 2 generalizes and improves the corresponding result in [21].
Remark 4. Let f(I)=I1+aI, system (1.3) can be changed into (1.2) in [22]. Theorem 2 shows that the disease of system dies out with probability one. Particularly, if Rs≥1 and βμΛ⋅R02<σ2<βμΛ, Theorem 2 in this paper shows the extinction of the disease; however, Theorem 3.2 in [22] can not show the extinction of the disease. Furthermore, we obtain limt→∞S(t)=Λμ and limt→∞R(t)=0a.s. But, only the disease extinction is found in Theorem 3.2 in [22]. Therefore, Theorem 2 in this paper improves and generalizes Theorem 3.2 in [22].
Remark 5. Let h(S)=S,γ1=0,γ2=γ. From Theorem 2, extinction of model (1.3) requires that f(I) is a real locally Lipschitz function on R+. However, from Corollary 1 in [23], extinction of model (1.3) requires that f(I) is twice continuously differentiable on R+. Obviously, the conditions of Theorem 2 are weaker than those of Corollary 1 in [23].
4.
Persistence of disease
When considering epidemic models, we are interested in when the disease will prevail in the population. In the deterministic models, the problem can be solved by proving that the endemic equilibrium of the corresponding model is globally asymptotically stable. But for model (1.3), there is no endemic equilibrium. In this section, we will establish sufficient conditions to ensure that the disease in model (1.3) is permanent in the time mean. Now, we give the definition of persistence in the mean as follows.
Definition 1. Model (1.3) is said to be persistent in the mean if lim inft→∞⟨I(t)⟩>0 a.s.
For the convenience, we give the following useful lemma.
Lemma 1. (See [28]). Suppose x∈C(Ω×[0,+∞),R+) and F∈C(Ω×[0,+∞),(−∞,+∞)). If there are three positive constants λ, λ0 and T such that
where limt→∞F(t)t=0 a.s., then lim inft→∞1t∫t0x(s)ds≥λλ0 a.s.
Theorem 3. Suppose that (H1)−(H3) hold. Let (S(t),I(t),R(t)) be the solution of model (1.3) with any given initial condition (S0,I0,R0)∈R3+. If Rs>1, then
Proof. From Remark 1, it follows that, for any 0<ε<2βσ2f′(0)(1−Λσ2f′(0)2μβ), there exists a constant T=T(ε)>0 such that X(t)≤Λμ+ε for all t≥T. Note that 0<f(I)≤f′(0)I, then from the first equation of model (1.3), we can derive that
which implies
where M2(t)=∫t0σS(s)f(I(s))dB(s). Clearly, M2(t) is a real-valued continuous local martingale vanishing at time 0 and
Note that lim supt→∞⟨M2,M2⟩tt≤σ2(f′(0))2(Λμ+ε)4<∞. Then, from the strong law of large numbers (see [27]), it follows that
This, together with (4.1), yields
From the arbitrariness of ε, it follows that
In the following, we will prove that (ⅱ) holds. Note that S(t)≤Λμ+ε for all t≥T and 0<f(I)≤f′(0)I, then, for any t≥T, 0<Sf(I)I≤f′(0)S≤f′(0)(Λμ+ε), which implies 0<Sf(I)f′(0)(Λμ+ε)I≤1. Then, we have
That is,
Substituting this into (3.1) yields
In addition, from the first equation of model (1.3), it follows that
By the Assumption (H3), we know that there exists a constant ϑ>0, such that 1−f(I)I≤f′(0)ϑI. Note that f(I)≤f′(0)I and S(t)≤Λμ+ε for any t>T, we have
which implies
Substituting (4.5) into (4.3), we obtain
where
From (3.3) and (4.2), it follows that limt→∞F1(t)t=0 a.s. Note that Rs>1. For any ε>0 sufficiently small, we have
and 1−σ2f′(0)2β(Λμ+ε)>0. Using Lemma 1, it follows from the arbitrariness of ε that
The assertion (ⅱ) is proved.
Now, we will give the proof of (ⅲ). It follows from the third equation of model (1.3) that
which, together with (4.6), yields
The proof is therefore complete.
Remark 6. From Theorem 3, one can see that if Rs>1, the disease persists and prevails. Clearly, if we let σ=0, then Theorem 3 is coincident with the result in [6].
Remark 7. Theorems 2 and 3 imply that Rs is the threshold of system (1.3) if σ2<βμΛf′(0). Namely, the disease dies out with probability one if Rs<1, whereas if Rs>1, the infection may become almost surely persistent in the time mean.
Remark 8. By constructing V(I)=lnI and using Lemma 1, we obtain that, for any (S0,I0,R0)∈R3+, if Rs>1, then lim inft→∞⟨S(t)⟩>0, lim inft→∞⟨I(t)⟩>0, lim inft→∞⟨R(t)⟩>0a.s. However, Theorem 3.5 in [22] only obtained that, for any (S0,I0,R0)∈Γ, lim inft→∞⟨I(t)⟩>0 by constructing V(I)=lnI+aI, which can be seen in the proof.
Remark 9. Let h(S)=S, γ1=0 and γ2=γ, then system (1.3) is consistent with system (1.4). The methods in proofs of Theorem 3 in our paper and [23] are different. Further, the results in [23] and our paper are different. From Theorem 3 in our paper, we have
From Theorem 3 in [23], we have lim inft→∞⟨I(t)⟩≥(μ+γ+α)(Rs−1)D∗=:A2>0a.s., where D∗=f′(0)[β(μ+αμ+γμ+δ)+(μ+γ+α)max0≤ζ≤Λμf(ζ)−ζf′(ζ)f2(ζ)].
For general function f, it is difficult to compare A1 with A2. However, when f is a specific function, we can compare A1 with A2. For example, let f(I)=(1−˜βIm+I)I, where m>0 and 0<˜β<1. If 0<m<μ˜β(μ+δ)(μ+γ+α)βδγ(1(1−˜β)2−1), then A3:=2μβΛ[1−D∗μ2mβΛ(μ˜β+β)]<2μβΛ[1−μ(μ+γ+α)βΛ]=:A4. It is easy to show that A3<σ2<A4 yields A1=(μ+γ+α)(Rs−1)βΛμ2(1−Λσ22μβ)(μ˜βm+β)>(μ+γ+α)(Rs−1)D∗=A2. In addition, condition A3<σ2<A4 ensures that the conditions of Theorem 3 in our paper and [23] hold. In this case, our results are better than those in [23].
In the following, we will summarize a useful criterion for the extinction and persistence of the disease of model (1.3). First, we discuss the relationship between R0 and Rs for different values of white noise intensity σ2 in the following three cases.
Case of R0≤1. From Rs=R0−Λ2σ2(f′(0))22μ2(μ+γ1+γ2+α), it follows that Rs<1 for any σ>0. Clearly, βμΛf′(0)>βμΛf′(0)⋅R02. Now, we differ three cases
Hence, if R0≤1, then for any σ>0, the disease dies out with probability one.
Case of 1<R0≤2. It follows that
On the other hand, Rs<1 is equivalent to σ2>2μ2(μ+γ1+γ2+α)Λ2(f′(0))2(R0−1). Now, we differ four cases
Thus, for 1<R0≤2, if σ2>2μ2(μ+γ1+γ2+α)Λ2(f′(0))2(R0−1) (i.e. Rs<1), then the disease dies out with probability one, whereas if σ2<2μ2(μ+γ1+γ2+α)Λ2(f′(0))2(R0−1) (i.e. Rs>1), then the infection may become almost surely persistent in the time mean. Therefore, if 1<R0≤2, then Rs is the threshold parameter.
Case of R0>2. On the one hand, it follows that
On the other hand, Rs<1 is equivalent to σ2>2μ2(μ+γ1+γ2+α)Λ2(f′(0))2(R0−1). Now, we differ four cases
Thus, if σ2<2μ2(μ+γ1+γ2+α)Λ2(f′(0))2(R0−1) (i.e. Rs>1), then the infection may become almost surely persistent in the mean, whereas if σ2>βμΛf′(0)⋅R02 (i.e. (C3) in Theorem 2 holds), then the disease dies out.
We summarize the above analysis as follows.
Corollary 1. Let Assumptions (H1)−(H3) hold and let (S(t),I(t),R(t)) be the solution of model (1.3) with any given initial condition (S0,I0,R0)∈R3+. The solution (S(t),I(t),R(t)) has the property:
(ⅰ) if R0≤1, then the disease dies out with probability one for any σ>0;
(ⅱ) if 1<R0≤2, then Rs is the threshold parameter, i.e., the disease dies out with probability one if Rs<1, whereas if Rs>1, the infection may become almost surely persistent in the time mean;
(ⅲ) if R0>2, then the disease dies out with probability one if σ2>βμΛf′(0)⋅R02, while if σ2<2μ2(μ+γ1+γ2+α)Λ2(f′(0))2(R0−1), the infection may become almost surely persistent in the time mean.
5.
Numerical simulations
In this section, we analyze the stochastic behaviors of model (1.3) by means of the numerical simulations in order to make readers understand our results more better. Throughout the following numerical simulations, we choose f(I)=I, f(I)=I1+aI2 and f(I)=Ie−mI, respectively. Thus, f(I)≤I. The numerical simulations of epidemic dynamics are carried out for the academic tests with the arbitrary values of the parameters, which do not correspond to some epidemic and exhibit only the theoretical properties of numerical solutions of considered models.
Example 1. Let f(I)=I. Numerical experiments were made by using the following parameters:
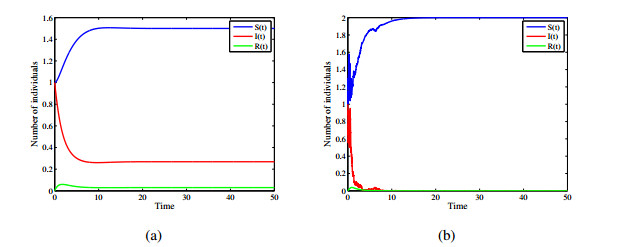
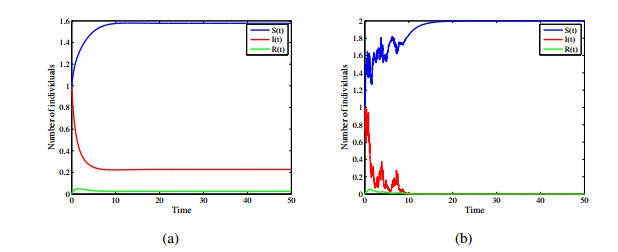
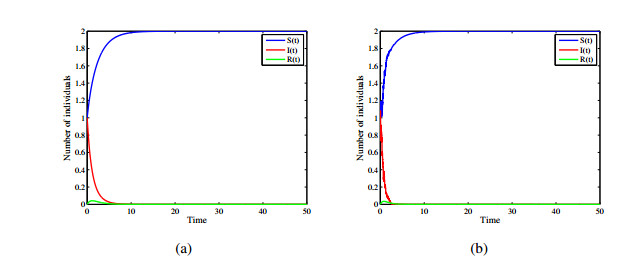
(i) In order to demonstrate the conclusion (i) in Corollary 1, we take β=0.3 and σ2=0.2. By a simple computation, R0=0.5<1. From the numerical simulations given in Figure 1, the disease I(t) in deterministic model (1.1) and stochastic model (1.3) will die out with probability one (Figure 1).
(ⅱ) In order to demonstrate the conclusion (ii) in Corollary 1, we take β=0.8. By a simple computation, we obtain 1<R0≈1.33<2. From the numerical simulations given in Figure 2(a), it is shown that disease I(t) of deterministic model (1.1) is permanent in the population.
1∘ Assume that σ2=0.4. By a simple computation, we obtain Rs≈0.66<1. It can be seen from Figure 2(b) that disease I(t) in model (1.3) will die out with probability one.
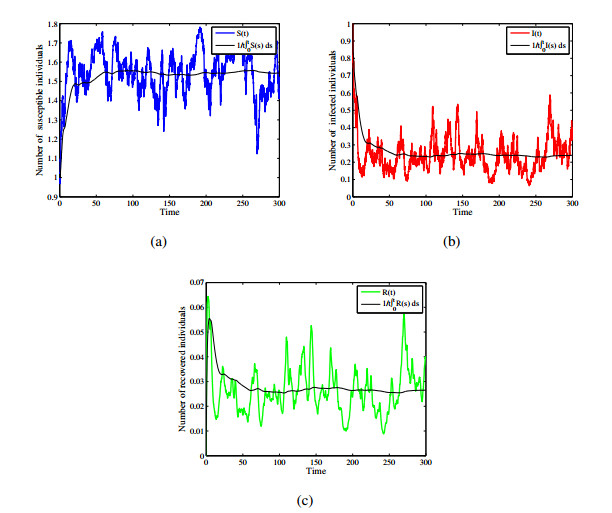
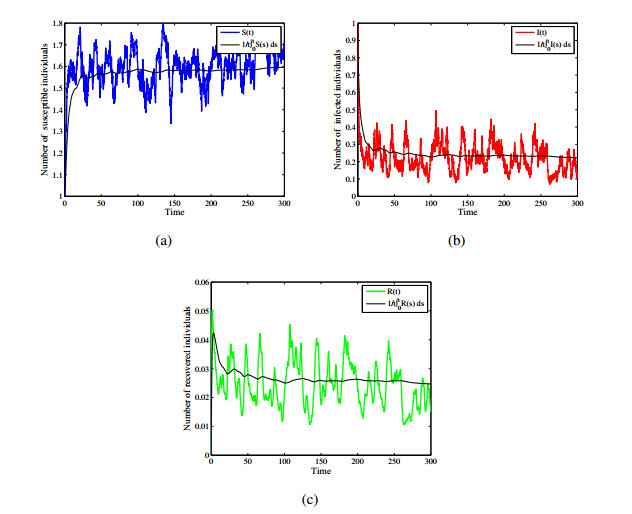
2∘ Assume that σ2=0.02. By computing, Rs≈1.297>1. From the numerical simulations given in Figure 3, it is shown that disease I(t) of model (1.3) is permanent in the mean with probability one.
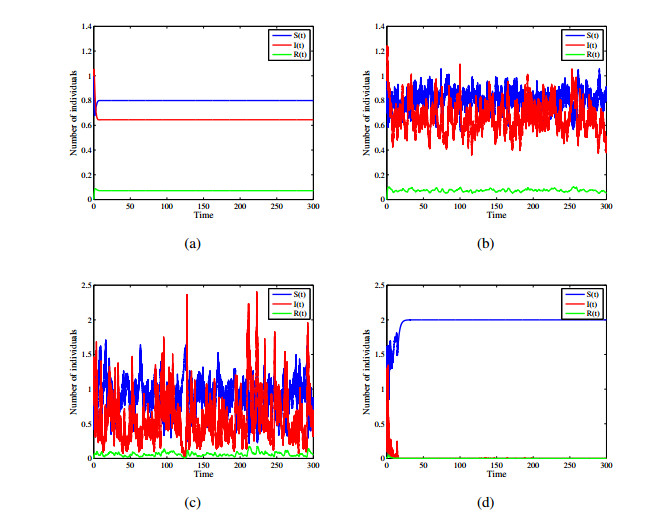
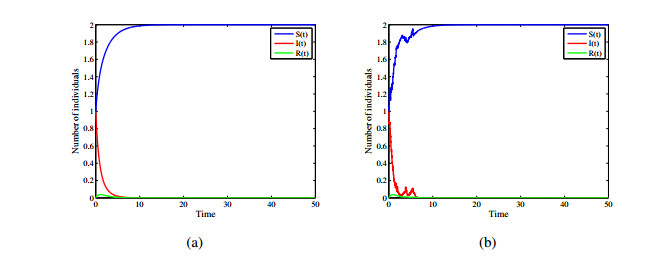
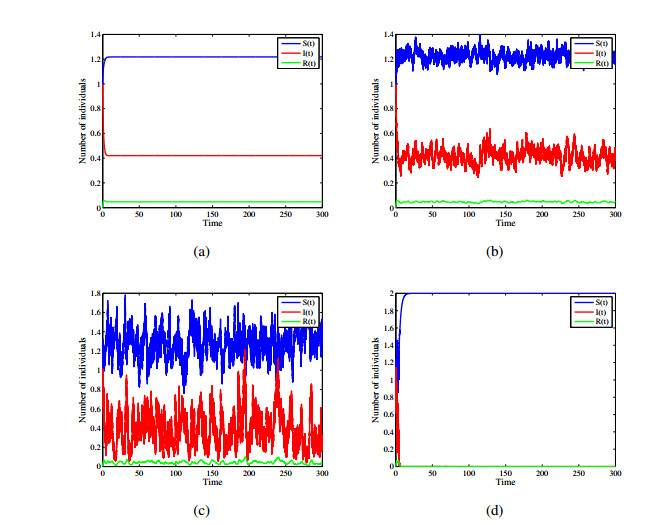
(ⅲ) In order to demonstrate the conclusion (ⅲ) in Corollary 1, we take β=1.5. By a simple computation, R0=2.5>2. From Figure 4(a), it follows that I(t) of (1.1) is permanent in the population.
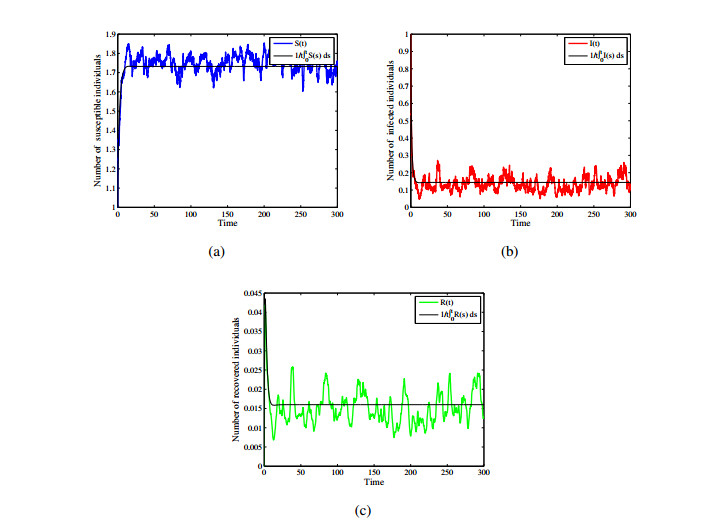
1∘ Assume that σ2=0.03 (σ2=0.3). By a simple computation, σ2<2μ2(μ+γ1+γ2+α)Λ2(R0−1)=0.9. It can be seen from Figure 4(b) (4(c)) that disease I(t) in model (1.3) is permanent in the time mean.
2∘ Assume that σ2=1. By computing, σ2>βμΛ⋅R02=0.9375. From the numerical simulations given in Figure 4(d), it is shown that disease I(t) of model (1.3) will die out with probability one.
Example 2. Let f(I)=I1+aI2 and a=1. Other parameters are defined as Example 1.
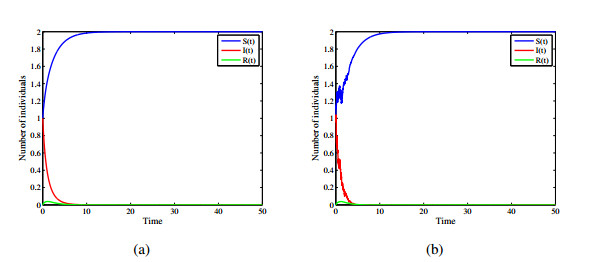
(ⅰ) From the numerical simulations given in Figure 5, the disease I(t) in deterministic model (1.1) and stochastic model (1.3) will die out with probability one.
(ⅱ) From Figure 6(a), we obtain that disease I(t) of (1.1) is permanent in the population.
1∘ If σ2=0.4. From Figure 6(b), disease I(t) in model (1.3) will be extinct with probability one.
2∘ If σ2=0.02. From Figure 7, disease I(t) of model (1.3) is permanent in the mean.
(ⅲ) From the numerical simulations given in Figure 8(a), it is shown that disease I(t) of deterministic model (1.1) is permanent in the population.
1∘ If σ2=0.03 (σ2=0.3). From Figure 8(b) (8(c)), disease I(t) in (1.3) is permanent in mean.
2∘ If σ2=1. From Figure 8(d), we obtain that disease I(t) of model (1.3) will be extinct.
Example 3. Let f(I)=Ie−mI and m=1. Other parameters are defined as Example 1.
(ⅰ) From Figure 9, the disease I(t) in model (1.1) and model (1.3) will die out with probability one.
(ⅱ) From Figure 10(a), we obtain that disease I(t) of (1.1) is permanent in the population.
1∘ If σ2=0.4. From Figure 10(b), disease I(t) in model (1.3) will be extinct with probability one.
2∘ If σ2=0.02. From Figure 11, disease I(t) of model (1.3) is permanent in the mean.
(ⅲ) From the numerical simulations given in Figure 12(a), it is shown that disease I(t) of deterministic model (1.1) is permanent in the population.
1∘ If σ2=0.03 (σ2=0.3) $. From Figure 12(b) (12(c)), we get that disease I(t) in model (1.3) is permanent in the mean.
2∘ If σ2=1. From Figure 12(d), we obtain that disease I(t) of model (1.3) will be extinct with probability one.
From Examples 1, 2 and 3, we can see that different incidence rates of diseases have different effects on the extinction and persistence in the mean of diseases. In addition, the intensity of noise plays an important role in epidemic dynamics. Comparing Figures 3 and 11 (or Figures 7 and 11), we can conclude that massive media coverages are needed to prevent the disease to spread widely in the population. This is consistent with the results in [26].
Comparing Figures 5(a) and 5(b), we conclude that if R0<1, then I(t) becomes extinct regardless of the intensity of noise. From Figures 6(a), 6(b) and 7, if 1<R0<2, then great intensity of the noise can make diseases extinction. From Figure 8(d), if R0>2, the disease becomes extinct as the intensity of the noise is large.
6.
Conclusions and discussions
This paper is concerned with the persistence and extinction of a stochastic SIRS epidemic model with nonlinear incidence rate and transfer from infectious to susceptible. To begin with, we consider the global existence and uniqueness of the positive solution to model (1.3) with any positive initial value. Next, sufficient criteria for the persistence and extinction of the disease are established. Then, we discuss the relationship between R0 and Rs for different values of white noise intensity σ2. In addition, we find that in case of neglecting the impact of environmental noises, the deterministic threshold R0 may exist and the threshold parameter will be overestimated. Furthermore, we discover that a large noise has the effect of suppressing the epidemic. So these results show that noises have important effects on the persistence and extinction of the disease. In addition, we can see that different incidence rates of diseases have different effects on epidemic dynamics.
Although there are important discoveries revealed by these studies, there are also limitations for the model. Theorem 2 shows that the particular expression for the nonlinear transmission makes no influence on the extinction of the disease. We leave these as our future work.
Acknowledgments
This work was supported by the National Natural Science Foundation of China (Nos. 11471197, 11571210, 11501339).
Conflict of interest
The authors declare there is no conflict of interest.









 DownLoad:
DownLoad: