A new alcoholism model with treatment and effect of Twitter is introduced. The stability of all equilibria which is determined by the basic reproductive number R0 is obtained. The occurrence of backward and forward bifurcation for a certain defined range of R0 are established by the center manifold theory. Numerical results and sensitivity analysis on several parameters are conducted. Our results show that Twitter may be a good indicator of alcoholism model and affect the emergence and spread of drinking behavior.
1.
Introduction
The harmful use of alcohol causes a large disease, social and economic burden in societies. In 2012, about 3.3 million deaths, or 5.9% of all global deaths, were attributable to alcohol consumption. Alcohol consumption can have an impact not only on the incidence of diseases, injuries and other health conditions, but also on the course of disorders and their outcomes in individuals [1]. According to a research report by the Shanghai Institute of Environmental Economics, the number of patients due to alcoholism has increased by 28.5 times, and the number of deaths has increased by 30.6 times in the past seven years [2]. Thus, it is very important to study drinking behavior.
Recently, many authors have studied mathematical models of drinking [3,4,5,6,7,8,9]. Bani et al. [3] studied the influence of environmental factors on college alcohol drinking patterns. Mulone et al. [4] developed a two-stage (four compartments) model for youths with serious drinking problems and their treatment, and the stability of all the equilibria was obtained. Mushayabasa et al. [5] formulated a deterministic model for evaluating the impact of heavy alcohol drinking on the reemerging gonorrhea epidemic. Lee et al. [6] studied the optimal control intervention strategies in low- and high-risk problem drinking populations. Mubayi et al. [7] studied the impact of relative residence times on the distribution of heavy drinkers in highly distinct environments and found that alcohol consumption is a function of social dynamics, environmental contexts, individuals' preferences and family history. Huo, Chen and Xiang [8] introduced a more realistic binge drinking model with time delay, in which time delay is used to represent the time lag of the immunity against drinking. Xiang, Liu and Huo [9] proposed a new SAIRS alcoholism model with birth and death on complex heterogeneous networks.
Media coverage is one of the effective ways to control alcoholism or infectious diseases, many authors have studied alcoholism or epidemic models with media coverage [10,11,12,13,14]. Cui et al. [10] developed a three dimensional compartmental model to investigate the impact of media coverage to the spread and control of infectious diseases. Pawelek et al. [11] studied the impact of twitter on influenza epidemics. Huo and Zhang [12] introduced a more realistic mathematical influenza model including dynamics of Twitter, which might reduce and increase the spread of influenza. Huo and Zhang [13] formulated a novel alcoholism model which involved impact of Twitter and investigated the occurrence of backward, forward bifurcation and Hopf bifurcation. Huo and Yang [14] introduced a novel SEIS epidemic model with the impact of media. Above results show that media coverage can regard as a good indicator in controlling the emergence and spread of the epidemic disease or alcoholism. Many scholars have done a lot of researches on drinking or infectious diseases with or without media coverage [15,16,17,18,19,20,21,22].
Alcoholism can be defined as a pattern of alcohol use that compromises the health and safety of oneself and others. There are a variety of treatment methods currently available, such as behavioral treatments, medications and mutual-support Groups [23]. The goal of a person pursuing treatment is to abstain from alcohol or to cut back on drinking. Many people have studied the epidemic or alcoholism models with treatment [24,25,26].
Motivated by the above [13,14], we set up a new alcoholism model with treatment and effect of Twitter in this paper. We derive the basic reproductive number of the model and study the stability of our model. Furthermore, we investigate the occurrence of backward and forward bifurcation.
The organization of this paper is as follows: In Section 2, we present a new alcoholism model with treatment and effect of Twitter. In Section 3, we derive the basic reproductive number and study the stability of all equilibria. We also study the occurrence of backward and forward bifurcation. In Section 4, we perform some numerical simulations to illustrate and extend our main results. Sensitivity analysis and some discussion are given in last section.
2.
The model formulation
2.1. System description
The total population in this model is divided into four compartments: S(t), L(t), H(t), R(t). S(t) represents the number of moderate drinkers, that is, the people who do not drink or drink within daily and weekly limits [13]. L(t) represents the number of light problem drinkers, that is, the drinkers who drink beyond daily or weekly ceiling [13]. H(t) represents the number of heavy problem drinkers, that is, the drinkers who drink far more than daily and weekly limits [13]. R(t) represents the number of quitting drinkers, that is, the people who quit problem drinking by treatment permanently. T(t) represents the number of messages that Twitter provide about alcoholism at time t. The total number of population at time t is given by
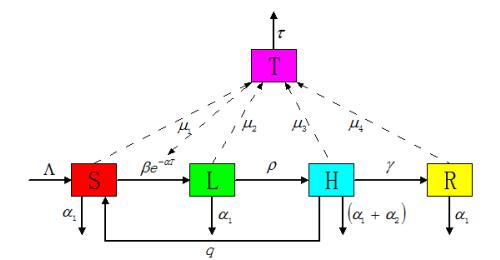
The population flowing among those compartments is shown in the following diagram (Figure 1).
The diagram leads to the following system of ordinary differential equations:
Where all the parameters are positive constants and Λ is the recruitment rate of the population. α1 is the natural death rate. α2 is the alcoholism-related death rate. β is the rate of transmission between moderate drinkers and heavy problem drinkers, and it is reduced by a factor e−αT due to the behavior change of the public after reading information about alcoholism. α is the coefficient that determines how effective the drinking information can reduce the transmission rate. τ is outdated-rate of tweets. ρ is the transmission rate from the light problem drinkers to the heavy problem drinkers. After treatment, the transfer rate of the heavy problem drinkers to the moderate drinkers is q, the transfer rate of the heavy problem drinkers to the quitting drinkers is γ. μi(i=1,2,3,4) are the rates that moderate drinkers, light problem drinkers, heavy problem drinkers and quitting drinkers may tweet about alcoholism during an alcoholism occasion, respectively.
Adding the first four equations of system (2.1), we have
Then it follows that limt→∞supN(t)≤Λα1.
According to the fifth equation of system (2.1), we obtain
then it follows that limt→∞supT(t)≤Λ(μ1+μ2+μ3+μ4)α1τ, so the set is
Therefore, we will consider the global stability of system (2.1) on the set Ω.
3.
Analysis of the model
3.1. Alcohol free equilibrium and the basic reproductive number
It is easy to see that system (2.1) always has a alcohol free equilibrium P0=(S0,L0,H0,R0,T0), where
By applying the method of the next generation matrix in [27], we obtain the basic reproduction number R0. System (2.1) can be written as
where x=(L,S,H,R,T)T,
The Jacobian matrices of F(x) and V(x) at the alcohol free equilibrium P0 are
and
Therefore, the basic reproduction number R0 is
3.2. Stability of alcohol free equilibrium
Theorem 1. When R0<1 and T(t)≥Λμ1α1τ, the alcohol free equilibrium P0 of system (2.1) is globally asymptotically stable; When R0<1 and T(t)<Λμ1α1τ, the alcohol free equilibrium P0 of system (2.1) is locally asymptotically stable; When R0>1, the alcohol free equilibrium P0 of system (2.1) is unstable.
Proof. The characteristic equation of the system (2.1) at the alcohol free equilibrium P0 is
Therefore, Eq.(3.2) can be written as
Therefore, the three eigenvalues of the Eq.(3.2) are λ1=−τ, λ2=−α1, λ3=−α1, and the other eigenvalues are determined by the equation
Therefore, the Eq.(3.4) can be written as
By Viete theorem, we have
and
Thus, when R0<1, the alcohol free equilibrium P0 is locally asymptotically stable; when R0>1, the alcohol free equilibrium P0 is unstable.
Define the Lyapunov function
It is clear that M(t)≥0 and the equality holds if and only if L(t)=H(t)=0. Differentiating M(S,L,H,R,T) and from the Eq.(2.2), we obtain S(t)≤Λα1. Therefore, when T(t)≥Λμ1α1τ, we have
It follows that M(S,L,H,R,T) is bounded and non-increasing. Thus, limt→∞M(S,L,H,R,T) exists. Note that R0<1 guarantees that dM(S,L,H,R,T)dt≤0 for all t≥0. Consequently, for system (2.1) there holds
Hence, by LaSalle's Invariance Principle [28], the alcohol free equilibrium is globally attractive. We show that the alcohol free equilibrium P0 is globally asymptotic stability when R0<1.
3.3. Existence of alcoholism equilibrium
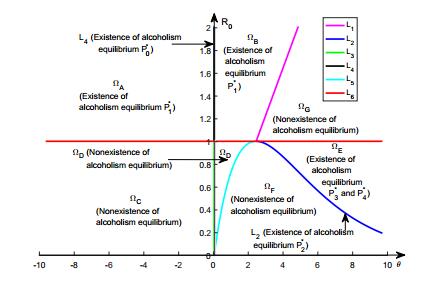
Theorem 2. (Ⅰ) When θ=0 and R0>1, the system (2.1) has a unique positive alcoholism equilibrium P∗0;
(Ⅱ)When θ≠0 and R0>max{R01,1}, the system (2.1) has a unique positive alcoholism equilibrium P∗1;
(Ⅲ)When R02=R0<min{R01,1}, the system (2.1) has a unique positive alcoholism equilibrium P∗2;
(Ⅳ)When R02<R0<min{R01,1}, the system (2.1) has two different positive alcoholism equilibria P∗3 and P∗4.
Proof. Assuming the right-hand sides of system (2.1) is 0, we have
Let (S,L,H,R,T)=(S∗,L∗,H∗,R∗,T∗) be the solution of Eq.(3.7), we have
By Eq.(3.8), we obtain
Combine the above Eqs.(3.9)-(3.12) and the first equation of Eq.(3.8), we have
where
and
For the sake of simplicity, we define
In what follows, we assume
Thus
The following work is to discuss the properties of Eq.(3.17).
(Ⅰ) When θ=0 and R0>1, the existence of the unique alcoholism equilibrium P∗0 of system (2.1) can be obtained by Eq.(3.13), as shown in line L4 of Figure 2, where
(Ⅱ) When θ≠0 and R0>1, we have F(0)=R0−1>0 and F(∞)=−∞<0. Assume that F′(H∗)<θ(1−R0R01). Thus, when θ>0 and R0>R01 or θ<0, we obtain F′(H∗)<0. Therefore, there is a unique positive solution for Eq.(3.17). Thus, the alcoholism equilibrium P∗1=(S∗1,L∗1,H∗1,R∗1,T∗1) can be obtained, as shown in regions ΩA and ΩB of Figure 2.
(Ⅲ) When θ>0 and R0<1, we have F(0)=R0−1<0, F(∞)=−∞<0 and F″(H∗)<0. Assume that F′(H∗)=θe−θH∗−R0R01θ=θ(e−θH∗−R0R01). If F′(H∗)=0, Eq.(3.17) has the unique positive solution H∗2=1θln(R01R0) when R0<R01. Meanwhile, we also have
Therefore, we obtain R0=R02=R01e(1−R01). Thus, the alcoholism equilibrium P∗2=(S∗2,L∗2,H∗2,R∗2,T∗2) can be obtained, as shown in line L2 of Figure 2.
(Ⅳ) When R02<R0<1, we have F(H∗2)>0. Eq.(3.17) has two different positive solutions H∗3 and H∗4, where H∗3 and H∗4 satisfy the following condition H∗3<H∗2<H∗4. Thus, the alcoholism equilibria P∗3=(S∗3,L∗3,H∗3,R∗3,T∗3) and P∗4=(S∗4,L∗4,H∗4,R∗4,T∗4) can be obtained, as shown in region ΩE of Figure 2.
Remark 1. For simplicity, the six curves (Li,i=1,2,3,4,5,6) divide the space in which R0 and θ are located into seven regions as shown in Figure 2.
3.4. Stability of the alcoholism equilibrium
In this section, we study the local stability of the alcoholism equilibria P∗i(i=0,1,2,3,4). First we obtain the characteristic matrix of system (2.1) at the alcoholism equilibria P∗i(i=0,1,2,3,4), as follows
In order to simplify Eq.(3.20), we have
Then the characteristic equation can be rewritten as:
where
Theorem 3. For system (2.1), we assume that μ1=μ2=μ3=μ4.
(Ⅰ) When θ=0,α2=0 and R0>1(i.e.,L4), the alcoholism equilibrium P∗0 is locally asymptotically stable;
(Ⅱ)When θ≠0, R0>max{1,R01}(i.e.,ΩA, and ΩB), Φ>α1θ and τ>ρ, the alcoholism equilibrium P∗1 is locally asymptotically stable;
(Ⅲ)When R02=R0<min{1,R01}(i.e.,L2), the alcoholism equilibrium P∗2 may be locally stable or not;
(Ⅳ)(ⅰ)When R02<R0<min{1,R01}(i.e.,ΩE), the alcoholism equilibrium P∗3 is unstable,
(ⅱ)When R02<R0<min{1,R01}(i.e.,ΩE) and τ>ρ, the alcoholism equilibrium P∗4 is locally asymptotically stable.
Proof. (Ⅰ)When θ=0, applying to the proof of (Ⅰ) of Theorem 2. We linearize the system (2.1) and evaluate the characteristic equation at the alcoholism equilibrium P∗0, and get
Thus, the characteristic equation can be rewritten as:
where
It is clear that b1>0, b2>0 and b3>0. Applying Routh−Hurwitz [13], by assuming that B=b1b2−b3. Then, we obtain
Hence, the alcoholism equilibrium P∗0 is locally asymptotically stable.
(Ⅱ)When μ1=μ2=μ3=μ4 and Φ>α1θ, by Eqs.(3.23)-(3.26), we have
Applying Routh−Hurwitz [13], let C=a1a2−a3. Then, we obtain
where
Then, let D=a3[a1a2−a3]−a21a4, and get
It is clear that D>0 and di>0(i=1,2,3), when τ>ρ. Because the expression of di(i=1,2,3) are too long, we list them in Appendix. Hence, the alcoholism equilibrium P∗1 is locally asymptotically stable.
(Ⅲ)Applying to the proof of (Ⅲ) of Theorem 2, when H∗2=1θln(R01R0), we obtain Φ=α1θ. Thus, by Eq.(3.26), we have
and by Eq.(3.23), we have
Thus, we know that Eq.(3.21) has negative and zero eigenvalues. Therefore, the alcoholism equilibrium P∗2 may be locally stable or not.
(Ⅳ)(ⅰ)Applying to the proof of (Ⅳ) of Theorem 2, when H∗3<H∗2=1θln(R01R0), we obtain Φ<α1θ. Thus, by Eq.(3.26), we have
and by Eq.(3.23), we have
Assuming g1(H∗3),g2(H∗3),g3(H∗3),g4(H∗3) is the root of Eq.(3.22), and we assume that the real parts satisfying Re(g1(H∗3))≤Re(g2(H∗3))≤Re(g3(H∗3))≤Re(g4(H∗3)), so we obtain
and
There are Re(g1(H∗3))<0 and Re(g4(H∗3))>0, thus, the alcoholism equilibrium P∗3 is unstable.
(ⅱ) When H∗4>H∗2=1θln(R01R0), we obtain Φ>α1θ. Thus, by Eq.(3.26), we have
By μ1=μ2=μ3=μ4, we have
Applying Routh−Hurwitz [13], by assuming that E=a1a2−a3. Then, we obtain
where
Then, by assuming that F=a3[a1a2−a3]−a21a4, and get
It is clear that D>0, when fi>0(i=1,2,3) and τ>ρ. Because the expression of fi(i=1,2,3) are too long, we do not list it here, and it is placed in Appendix. Hence, the alcoholism equilibrium P∗4 is locally asymptotically stable.
3.5. Forward and Backward Bifurcation
In this section, we study the change of the parameter β causing a forward or a backward bifurcation to occur.
Theorem 4. (Ⅰ) If R01>1, when R0=1, the system (2.1) appears a backward bifurcation.
(Ⅱ) If R01<1, when R0=1, the system (2.1) appears a forward bifurcation.
Proof. Using the central manifold theory described in [29]. Introducing x1=S, x2=L, x3=H, x4=R, x5=T, the system (2.1) becomes
Thus, the alcohol free equilibrium P0 is
In view of Theorem 4.1 [29], the Jacobian matrix J(P0) of the system (3.27) in the alcohol free equilibrium is
We establish the local stability of alcohol free equilibrium taking β as bifurcation parameter, when R0=1 corresponding to β=β∗=α1(α1+ρ)(α1+α2+q+γ)eΛαμ1α1τΛρ, thus, we obtain
It is clear that 0 is a simple eigenvalue of J(P0). Therefore, there is a right eigenvector associated with 0 eigenvalues that is R=(r1,r2,r3,r4,r5)T, there is a left eigenvector associated with 0 eigenvalues is L=(l1,l2,l3,l4,l5), and it is required to satisfy LR=1.
Therefore, the right eigenvector is
the left eigenvector is
In view of Theorem 4.1 [29], we know that
Therefore, we obtain
According to Theorem 4.1 of [29], we notice that the coefficient b is always positive. The coefficient a is positive when R01>1. In this case, the direction of the bifurcation of the system (2.1) at R0=1 is backward, as shown in the Figure 9(a). The coefficient a is negative when R01<1. Under this circumstance, the direction of the bifurcation of the system (2.1) at R0=1 is forward, as shown in the Figure 9(b).
4.
Numerical results
The goal of this section is to present some numerical simulations which complement the theoretical results in the previous sections. We choose some parameters based on the Table 1.
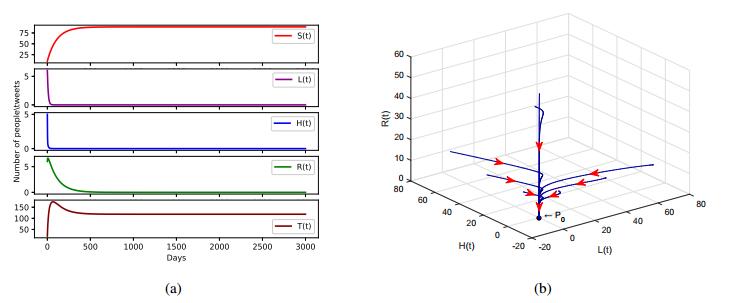
As an example, we choose a set of the following parameters, the parameter values are Λ=0.8,α=0.007,α1=0.009,α2=0.5,μ1=0.04,μ2=0.8,μ3=0.8,μ4=0.8,γ=0.1,q=0.07,ρ=0.09,τ=0.03 and β=0.001. It follows from Theorem 1 that the alcohol free equilibrium P0=(88.89,0,0,0,118.52) of system (2.1) is globally asymptotically stable for any value of time t when R0=0.0519<1 (see Figure 3 (a) and (b)). Furthermore, we can also observe that the value of the equilibrium P∗(t) changes as t increasing and eventually tends to P0=(88.89,0,0,0,118.52) from Figure 3 (a) and (b).
In order to verify the local stability of the alcoholism equilibrium P∗1, we choose a set of the following parameters, the parameter values are Λ=0.8,α=0.007,α1=0.009,α2=0.5,μ1=0.04,μ2=0.04,μ3=0.04,μ4=0.04,γ=0.1,q=0.07,ρ=0.09,τ=0.03 and β=0.004. It follows from Theorem 3 that the alcoholism equilibrium P∗1=(28.16,6.09,0.81,8.97,58.71) of system (2.1) is locally asymptotically stable for any value of time t when R0=2.0765>max{1,R01}, where R01=0.6128 (see Figure 4 (a) and (b)). Furthermore, we can also observe that the value of the equilibrium P∗(t) changes with t increasing and eventually tends to P∗1=(28.16,6.09,0.81,8.97,58.71) from Figure 4 (a) and (b).
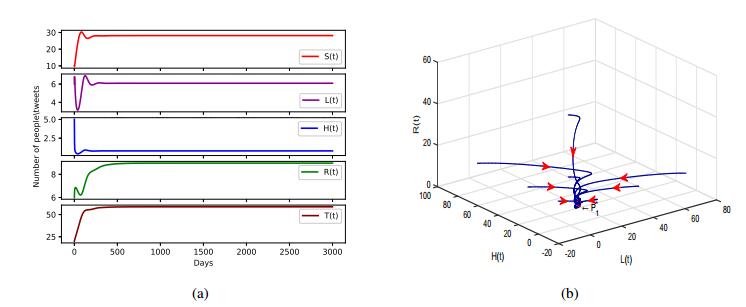
In order to verify the local stability of the alcoholism equilibrium P∗4, we choose a set of the following parameters, the parameter values are Λ=8,α=0.07,α1=0.003,α2=0.005,μ1=0.025,μ2=0.025,μ3=0.025,μ4=0.025,γ=0.01,q=0.07,ρ=0.4,τ=0.45 and β=0.9. It follows from Theorem 3 that the alcoholism equilibrium P∗4=(254.1677,85.2877,387.8429,1292.7081,112.2223) of system (2.1) is locally asymptotically stable for any value of time t when R02<R0<max{1,R01}, where R01=2.7788, R0=0.8486 and R02=0.4692 (see Figure 5 (a) and (b)). Furthermore, we can also observe that the value of the equilibrium P∗(t) changes with t increasing and eventually tends to P∗4=(254.1677,85.2877,387.8429,1292.7081,112.2223) from Figure 5 (a) and (b).
Then, we choose a set of the following parameters, the parameter values are Λ=0.8,α=0.007,α1=0.009,α2=0.5,μ1=0,μ2=0.008,μ3=0.8,μ4=0.8,γ=0.1,q=0.99,ρ=0.99,τ=0.03 and β=0.0204. It follows from Theorem 3 that the alcoholism equilibrium P∗1=(49.19,0.92,0.57,6.37,185.30) of system (2.1) is locally asymptotically stable for any value of time t when R0=1.1238>max{1,R01} and β<β∗, where R01=−2.9044 and β∗=0.021 (see Figure 6 (a) and (b)).
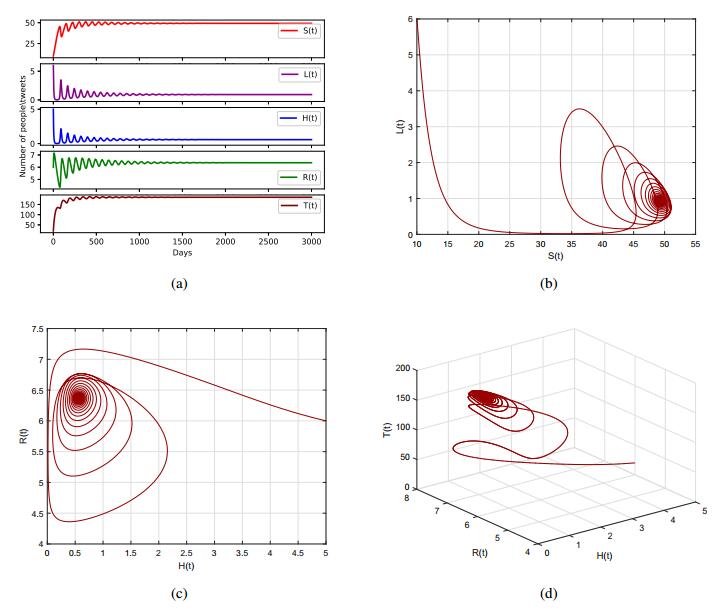
If we choose β as 0.076 (see Figure 7 (a) and (b)), we have more intricate dynamic behaviors on system (2.1). As an example, we choose a set of the following parameters, the parameter values are Λ=0.8,α=0.00626,α1=0.009,α2=0.4,μ1=0.009,μ2=0.004,μ3=0.8,μ4=0.8,γ=0.1,q=0.06,ρ=0.9,τ=0.03 and β=0.076. The alcoholism equilibrium P∗1 of system (2.1) occurs a Hopf bifurcation when R0=9.9478>1 and β>β∗, where β∗=0.011 (see Figure 7 (a-d)). In Figure 7 (a-d), we can readily see that the solution curves of system (2.1) perform a sustained periodic oscillation and phase trajectories approaches limit cycle.
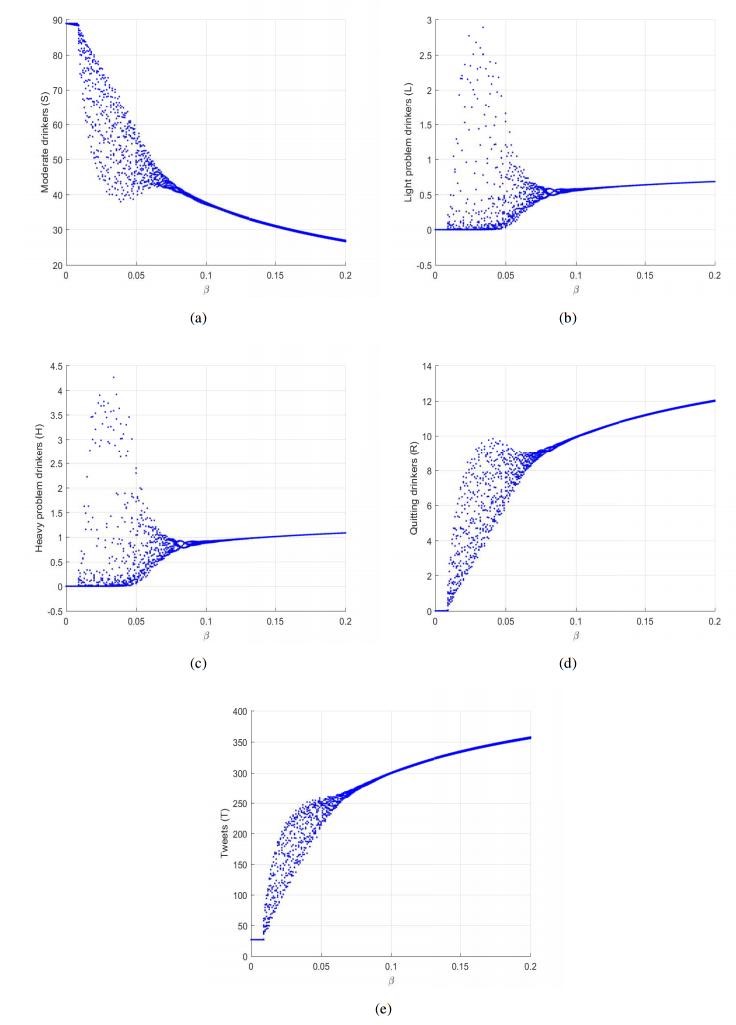
In order to demonstrate some results about Hopf bifurcation, we consider β as bifurcation parameter. We know that the alcoholism equilibrium P∗1 is feasible for β∈[0.0099,0.8]. Thus, system (2.1) is stable when 0.0099≤β<0.011, and Hopf bifurcation occurs at the alcoholism equilibrium P∗1 when 0.011≤β<0.08, and system (2.1) becomes stable again when 0.08≤β≤0.2, as depicted in Figure 8(a-e).
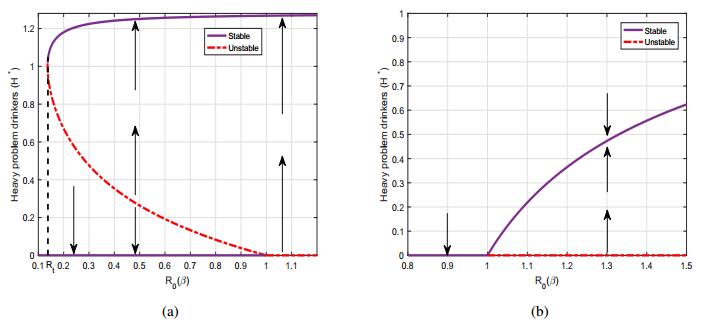
The backward and forward bifurcation diagram of system (2.1) is shown in Figure 9, and the direction of bifurcation depends upon the value of R01. As seen in the backward bifurcation diagram of Figure 9(a) when R01=4.4936>1, there is a threshold quantity Rt which is the value of R0. The alcohol free equilibrium is globally asymptotically stable when R0<Rt, where Rt=0.1350. There are two alcoholism equilibria and a alcohol free equilibrium when Rt<R0<1, the upper ones are stable, the middle ones are unstable and the lower ones is globally asymptotically stable. There are a stable alcoholism equilibria and an unstable alcohol free equilibrium when R0>1. As seen in the forward bifurcation diagram of Figure 9(b) when R01=0.5357<1, the alcohol free equilibrium is globally asymptotically stable when R0<1. There are a stable alcoholism equilibria and an unstable alcohol free equilibrium when R0>1.
5.
Sensitivity analysis
In this section, we examine the effects of changes in some parameters on the number of heavy problem drinkers. Therefore, we carry out the sensitivity analysis of heavy problem drinkers H.
Figure 10 shows a comparison between the parameters of system (2.1) versus the heavy problem drinkers, we main consider the effect of μ1,q,γ,τ on the dynamics of heavy problem drinkers. Firstly, we choose the effect of parameter μ1 on the dynamics of heavy problem drinkers, the other parameter values are Λ=0.8,α=0.07,α1=0.009,α2=0.5,μ2=0.8,μ3=0.8,μ4=0.8,γ=0.1,q=0.09,ρ=0.09,τ=0.03 and β=0.04, as depicted in Figure 10(a). We know that the number of heavy problem drinkers will decrease when μ1 increase from Figure 10(a). The simulation shows that more Twitter messages can result in the lower alcoholism cases, and changing the number of Twitter messages posted per day does affect the time when the alcoholism reaches the peak. Secondly, we choose the effect of parameter q on the dynamics of heavy problem drinkers, the other parameter values are Λ=0.8,α=0.007,α1=0.009,α2=0.5,μ1=0.04,μ2=0.8,μ3=0.8,μ4=0.8,γ=0.1,ρ=0.09,τ=0.03 and β=0.15, as depicted in Figure 10(b). We know that the number of heavy problem drinkers will decrease when q increase from Figure 10(b). Thirdly, we choose the effect of parameter γ on the dynamics of heavy problem drinkers, the other parameter values are Λ=0.8,α=0.007,α1=0.009,α2=0.5,μ1=0.04,μ2=0.8,μ3=0.8,μ4=0.8,q=0.07,τ=0.03,ρ=0.01 and β=0.15, as depicted in Figure 10(c). We know that the number of heavy problem drinkers will decrease when γ increase from Figure 10(c). The simulation results in Figure 10(b) and 10(c) show that treatment significantly reduces the number of alcoholism cases. Finally, we choose the effect of parameter τ on the dynamics of heavy problem drinkers, the other parameter values are Λ=0.8,α=0.007,α1=0.009,α2=0.5,μ1=0.04,μ2=0.8,μ3=0.8,μ4=0.8,γ=0.1,q=0.07,ρ=0.09 and β=0.15, as depicted in Figure 10(d). We know that the number of heavy problem drinkers will increase when τ increase from Figure 10(d). This indicates that the rate of upper outdated Twitter messages result in the upper alcoholism cases.
6.
Discussion and conclusion
We construct a new alcoholism model with treatment and effect of Twitter in this paper. We study the stability of all equilibria and derive the basic reproductive number R0. We also investigate the occurrence of backward and forward bifurcation for a certain defined range of R0 by the center manifold theory. Furthermore, we give some numerical results and sensitivity analysis to extend and illustrate our results. Our results show that Twitter may be a good indicator of alcoholism model and affect the emergence and spread of drinking behavior. How to prove existence of Hopf bifurcation analytically is interesting and still open. We will leave this work in future.
Acknowledgments
We are grateful to the anonymous referees and the editors for their valuable comments and suggestions which improved the quality of the paper. This work is supported by the National Natural Science Foundation of China (11861044 and 11661050), and the HongLiu first-class disciplines Development Program of Lanzhou University of Technology.
Conflict of interest
The authors declare there is no conflict of interest.
Appendix A
The formula of d1, d2, d3 in the proof of (Ⅱ) of Theorem 3.
The formula of f1, f2, f3.









 DownLoad:
DownLoad: