Dynamics of tuberculosis with fast and slow progression and media coverage

1.   Introduction

Tuberculosis (TB) is a common and fatal infectious disease. It has become a chronic infectious disease that threatens human health worldwide. Globally, in 2016 there were an estimated 10.4 million incident cases of TB, equivalent to 140 cases per 100000 population. Meanwhile, the proportion of people who develop TB and die from the disease (the case fatality ratio) was $16%$ ^[1]. Therefore, TB has become a global concern for social and public health issues.

Many scholars have carried out a lot of excellent researches on the transmission mechanism and prevention strategies of TB ^[2,3,4,5,6]. Silva et al. ^[4] introduced delays in a TB model, and studied optimal control of TB with state and control delays. Huo et al. ^[5] presented a two-strain TB model with general contact rate which allows TB patients with the drug sensitive of strain Mycobacterium tuberculosis to be treated and gave a detailed qualitative analysis about positivity, boundedness, existence, uniqueness and global stability of the equilibria of the model. Huo and Zou ^[6] studied a TB model with two kinds of treatment, that is, treatment at home and treatment in hospital and showed that the treatment at home has a negative influence on the spread of TB.

The susceptible individuals who carry the pathogen developing into infectious individuals are different from the progression of TB transmission. Some people may become symptomatic infectious individuals after a few days or months, and some people may occur after several years or even decades. For the former, it is considered that the susceptible individuals directly develop symptomatic infectious individuals without going through the latency period after infection, which is called the fast progression of TB transmission. For the latter, it is considered that the susceptible individuals become the latent individuals carrying the pathogen after infection with Mycobacterium tuberculosis, and they can become infected by exogenous reinfection or endogenous infection, which is called a slow progression of TB transmission. Huo and Feng ^[7] constructed an HIV/AIDS epidemic model with different latent stages and treatment. The model allowed for the latent individuals to have the fast and slow latent compartments. Mccluskey ^[8] introduced the spread of TB through two models which included fast and slow progression to the infected class. Berge et al. ^[9] considered a two patch cholera model with the aim of investigating the impact of human population movements between two cities(patches). Song et al. ^[10] studied TB models with fast and slow dynamics. Many scholars have studied infectious diseases related to the fast and slow progression (see e.g. ^[11] and references cited therein).

Media coverage is changing the way that we communicate with each other in our daily life, work and study. The media may be the most important source of public health information. At the same time, it also plays an important role in the spread and control of epidemics by providing some health information. Cui et al. ^[12] proposed a general contact rate $\beta(I) = c_1-c_2f(I)$ to reflect some intrinsic characters of media coverage. Huo and Zhang ^[13] introduced a novel alcoholism model which involves impact of Twitter, and showed that Twitter can serve as a good indicator of alcoholism model and affect the spread of the drinking. Huo et al. ^[14] presented a $SEIS$ epidemic model with the impact of media coverage. Their results manifested that media can be regarded as a good indicator in controlling the emergence and spread of the epidemic disease. Many scholars have done a lot of researches on infectious diseases with or without media coverage ^{[15,16,17,18,19,20,21]}.

Motivated by the above, we construct a new TB model which not only involves fast and slow progression but also incorporates the impact of media coverage in this paper. We study the stability of all the equilibria. Furthermore, we also investigate the occurrence of backward and forward bifurcation. Our results show that media coverage can encourage people to take countermeasures to avoid potential infections.

The rest of this paper is organized as follows. In Section 2, a new tuberculosis model with fast and slow progression and media coverage is constructed. In Section 3, we discuss the existence and stability of all the equilibria, then we analyze a forward and backward bifurcation. Some numerical simulations are presented in Section 4. Sensitivity analysis and some discussions are given in the last section.

2.   Model formulation

2.1.   System description

The total population $N(t)$ is divided into four compartments: $S(t)$, $E(t)$, $I(t)$ and $R(t)$. $S(t)$ denotes susceptible individuals. $E(t)$ is referred to as undetected non-symptomatic (latent) carriers. $I(t)$ is symptomatic infectious individuals. $R(t)$ represents recovered individuals. $M(t)$ represents the number of message that all of them provide about TB at time $t$. The total population $N(t)$ is given by

The transfer diagram of the model is shown in Figure 1. The transfer diagram leads to the following system of ordinary differential equations:

All the parameters are positive constants. $\delta$ is the constant recruitment rate of the population. $\beta$ is the transmission coefficient of TB. $\alpha$ is the coefficient that determines how effective the disease-related messages can influence the transmission rate and the transmission rate $\beta$ is reduced by a factor $e^{-\alpha M}$ (see ^[13,14]). $\mu$ is the natural death rate. $q$ is the proportion of disease by fast progression. $\varepsilon$ is the progression rate from the exposed individuals to the infected individuals. $d$ is the disease-related death rate of TB. $\gamma$ is the recovery rate of TB. $\tau$ is the rate that message become outdated. $\mu_1$, $\mu_2$, $\mu_3$ and $\mu_4$ are the rates that susceptible individuals, exposed individuals, infectious individuals, recovered individuals may send messages about TB, respectively.

2.2.   Basic properties

In this section, we will show positivity and boundedness for system (2.1).

2.2.1.   Positivity of solutions

Lemma 1. If $S(0)\ge 0, E(0)\ge 0, I(0)\ge 0, R(0)\ge 0, M(0)\ge 0$, the solutions $S(t)$, $E(t)$, $I(t)$, $R(t)$, $M(t)$ of system (2.1) are positive for all $t > 0$.

Proof. If $S(0)\ge 0$, according to the first equation of system (2.1), we have

It can be rewritten as:

Therefore,

Hence,

So,

Similarly, we can show that $E(t) > 0, I(t) > 0, R(t) > 0, M(t) > 0.$ So the solutions $S(t)$, $E(t)$, $I(t)$, $R(t)$, $M(t)$ of system (2.1) with initial conditions $S(0)\ge 0, E(0)\ge 0, I(0)\ge 0, R(0)\ge 0, M(0)\ge 0$ are positive for all $t > 0$. This completes the proof of Lemma 1.

2.2.2.   Invariant region

Lemma 2. The feasible region $\Omega$ defined by

with initial conditions $S(0)\ge 0, E(0)\ge 0, I(0)\ge 0, R(0)\ge 0, M(0)\ge 0$ is positively invariant for system (2.1).

Proof. Adding the former four equations of system (2.1), we obtain

It follows that

where $N(0)$ is the initial value of total number of people. Thus,

Then

Further, from the last equation of system (2.1), we have

It follows that

where $M(0)$ represents the initial value of cumulative density media coverage. Thus,

It implies that the region

is a positively invariant set for system (2.1). So we consider dynamics of system (2.1) on the set $\Omega$ in this paper. This completes the proof of Lemma 2.

3.   Analysis of the model

3.1.   The basic reproductive number

It is easy to see system (2.1) always has a disease-free equilibrium

We can obtain the basic reproductive number $R_0$ by using the next-generation method ^[22]. Let $x = (E, I, R, S, M)^T$, then system (2.1) can be written as

where,

The Jacobian matrices of $\mathcal{F}(x)$ and $\mathcal{V}(x)$ at the disease-free equilibrium $P_0$ are, respectively,

where

The basic reproductive number, denoted by $R_0$ is thus given by

3.2.   Stability of disease-free equilibrium

Theorem 1. The disease-free equilibrium $P_0 = \Big(\frac{\delta}{\mu}, 0, 0, 0, \frac{\mu_1\delta}{\mu\tau}\Big)$ of system (2.1) is locally asymptotically stable if $R_0 < 1$, and is unstable if $R_0 > 1$.

Proof. The Jacobian matrix corresponding to system (2.1) about $P_0 = \Big(\frac{\delta}{\mu}, 0, 0, 0, \frac{\mu_1\delta}{\mu\tau}\Big)$ is obtained as follows:

The characteristic equation corresponding to the Jacobian matrix $J(P_0)$ is given by $|\lambda E-J(P_0)| = 0$, where $\lambda$ is the eigenvalue and $E$ is the unit matrix. Thus, we get

Obviously, Eq. (3.3) has three negative roots $\lambda_1 = -\tau$, $\lambda_2 = \lambda_3 = -\mu$, and the other two roots $\lambda_4$ and $\lambda_5$ are determined by

According to the above calculation and analysis, we can obtain

If $R_0 < 1$, we have $\lambda_4\lambda_5 > 0$, $\lambda_4+\lambda_5 < 0$, hence $\lambda_4 < 0, \lambda_5 < 0$. Therefore, $P_0 = \Big(\frac{\delta}{\mu}, 0, 0, 0, \frac{\mu_1\delta}{\mu\tau}\Big)$ is locally asymptotically stable. If $R_0 > 1$, Eq. (3.4) has two real roots that one is positive and another is negative. In this case, $P_0 = \Big(\frac{\delta}{\mu}, 0, 0, 0, \frac{\mu_1\delta}{\mu\tau}\Big)$ is unstable. This completes the proof of Theorem 1.

Theorem 2. The disease-free equilibrium $P_0 = \Big(\frac{\delta}{\mu}, 0, 0, 0, \frac{\mu_1\delta}{\mu\tau}\Big)$ of the system (2.1) is globally asymptotically stable if $R_0 < 1$ and $M(t)\ge\frac{\mu_1\delta}{\mu\tau}$.

Proof. Motivated by Huo and Zhang ^[13], we define the Lyapunov function

It is clear that $V(t)\ge0$ and the equality holds if and only if $E(t) = I(t) = 0$.

From the first equation of the system (2.1), we have

and then we can obtain $S(t)\le\frac{\delta}{\mu}$.

Differentiating $V(t)$ with respect to time $t$ yields:

It follows that $V(t)$ is bounded and non-increasing. Therefore, $\lim\limits_{t\to \infty} V(t)$ exists. Note that $\frac{dV(t)}{dt} = 0$ if and only if $E = I = R = 0, S = S_0 = \frac{\delta}{\mu}, M = M_0 = \frac{\mu_1\delta}{\mu\tau}$. The maximum invariant set of the system (2.1) on the set $\{(S, E, I, R, M):\frac{dV(t)}{dt} = 0\}$ is the singleton $P_0 = \Big(\frac{\delta}{\mu}, 0, 0, 0, \frac{\mu_1\delta}{\mu\tau}\Big)$. And note that $R_0 < 1$ guarantees that $\frac{dV(t)}{dt}\le0$ for all $t\ge0$. By LaSalle's Invariance Principle ^[23], the disease-free equilibrium $P_0 = \Big(\frac{\delta}{\mu}, 0, 0, 0, \frac{\mu_1\delta}{\mu\tau}\Big)$ is globally asymptotically stable when $R_0 < 1$ and $M(t)\ge\frac{\mu_1\delta}{\mu\tau}$. This completes the proof of Theorem 2.

3.3.   Existence and Stability of the endemic equilibria

First, we introduce:

Remark 1. It is clear to check that: $R_{01} > 0$ if and only if $\Phi > 0$; $R_{01} = 0$ if and only if $\Phi = 0$; $R_{01} < 0$ if and only if $\Phi < 0$.

Theorem 3. For system (2.1),

(ⅰ) If $R_0 > \max\{1, R_{01}\}$, there is a unique endemic equilibrium $P_1^*$.

(ⅱ) If $R_c = R_0 < \min\{1, R_{01}\}$ and $R_{01} > 0$, there is a unique endemic equilibrium $P_2^*$.

(ⅲ) If $R_c < R_0 < \min\{1, R_{01}\}$ and $R_{01} > 0$, there are two distinct endemic equilibria $P_3^*$ and $P_4^*$.

Proof. The endemic equilibrium $P^*(S^*, E^*, I^*, R^*, M^*)$ of system (2.1) is determined by equations

Further, we obtain

where $\Phi$ is given by (3.5). Substituting $S, M$ into the first equation of (3.8) yields

According to (3.6) and (3.13), we have

We consider a function $F(I)$ defined by

Then, we have

Case 1. When $\Phi = 0$, according to (3.13), we have

Therefore, there is a unique endemic equilibrium if $\Phi = 0$ and $R_0 > 1$.

Case 2. When $\Phi\neq0$, we have

Thus, we get $F'(I) < F'(0)$, which means $\Phi e^{-\Phi I} < \Phi$.

(1) If $R_0 > 1$, we have $F(0) = R_0-1 > 0$, $F(+\infty) = -\infty < 0$, and

When $R_0 > R_{01} > 0$, we have $\Phi > 0$ and $1-\frac{R_0}{R_{01}} < 0$, which means $F'(I) < 0$.

When $R_0 > 0 > R_{01}$, we have $\Phi < 0$ and $1-\frac{R_0}{R_{01}} > 0$, which means $F'(I) < 0$.

Therefore, there is a unique endemic equilibrium if $\Phi\neq0$ and $R_0 > \max\{1, R_{01}\}$.

In conclusion, there is a unique endemic equilibrium $P_1^*$ if $R_0 > \max\{1, R_{01}\}$.

(2) If $R_0 < 1$, we have $F(0) = R_0-1 < 0$, $F(+\infty) = -\infty < 0$, Let's suppose

Then we obtain

When $R_0 < R_{01}$, we have $\Phi > 0$ and $\ln\frac{R_{01}}{R_0} > 0$, which means $I_c > 0$.

Substituting $I_c$ into (3.14), we get

(a) When $F(I_c) = 0$, we can obtain $I_2^* = I_c$ and $R_0 = R_c$, where $R_c$ is given by (3.7).

Therefore, there is a unique endemic equilibrium $P_2^*$ if $R_c = R_0 < \min\{1, R_{01}\}$ and $R_{01} > 0$.

(b) When $F(I_c) > 0$, we can obtain $R_0 > R_c$.

Since $F(0) = R_0-1 < 0$, $F(+\infty) = -\infty < 0$ and $F(I_c) > 0$, we know that $F(I) = 0$ has two different positive solutions $I_3^*$ and $I_4^*$. Let $I_3^*$ and $I_4^*$ satisfy $I_3^* < I_2^* < I_4^*$. Therefore, there are two distinct endemic equilibria $P_3^*$ and $P_4^*$ if $R_c < R_0 < \min\{1, R_{01}\}$ and $R_{01} > 0$. This completes the proof of Theorem 3.

Theorem 4. When $q = 0$, the endemic equilibria $P_i^*(i = 1, 2, 3, 4)$ of system (2.1) have the following qualities:

(ⅰ) If $R_0 > \max\{1, R_{01}\}$, $a_1(I_1^*)a_2(I_1^*)-a_3(I_1^*) > 0$, $a_3(I_1^*)\Big[a_1(I_1^*)a_2(I_1^*)-a_3(I_1^*)\Big]-\Big[a_1(I_1^*)\Big]^2a_4(I_1^*) > 0$ and $a_4(I_1^*) > 0$, the endemic equilibrium $P_1^*$ is locally asymptotically stable.

(ⅱ) If $R_c = R_0 < \min\{1, R_{01}\}$ and $R_{01} > 0$, the endemic equilibrium $P_2^*$ is unstable.

(ⅲ) If $R_c < R_0 < \min\{1, R_{01}\}$ and $R_{01} > 0$, the endemic equilibrium $P_3^*$ is unstable.

(ⅳ) If $R_c < R_0 < \min\{1, R_{01}\}$ and $R_{01} > 0$, the stability of the endemic equilibrium $P_4^*$ is uncertain.

Proof. When $q = 0$, the Jacobian matrix corresponding to system (2.1) about $P_i^*(i = 1, 2, 3, 4)$ are obtained as follows:

The characteristic equation corresponding to the Jacobian matrix $J(P_i^*)$ is given by $|\lambda E-J(P_i^*)| = 0$, where $\lambda$ is the eigenvalue and $E$ is the unit matrix. Thus, we get

We set $\Theta = \beta e^{-\alpha M_i^*}$, then

From the second equation of (3.8), we have

then

and

Therefore, the characteristic equation can be rewritten as:

where

where

(ⅰ) According to (3.17)-(3.20), we have

It is clear that $a_1(I_1^*) > 0$, according to Routh$-$Hurwitz criteria ^[24], the proof (ⅰ) of Theorem 4 is obtained.

(ⅱ) According to the proof of (ⅱ) of Theorem 3, we have $I_2^* = \frac{1}{\Phi}ln\frac{R_{01}}{R_0}$. Then, we can get $\Theta I_2^* = \mu\Phi I_2^*$. Therefore, based on (3.17)-(3.20), we can obtain

It is easy to know that $a_3(I_2^*)\neq0$, and $a_1(I_2^*)a_2(I_2^*)-a_3(I_2^*) < 0$. Therefore, we know that Eq. (3.15) has negative, positive and zero eigenvalues. So the endemic equilibrium $P_2^*$ of system (2.1) is unstable.

(ⅲ) Due to $I_3^* < I_2^* = \frac{1}{\Phi}ln\frac{R_{01}}{R_0}$, we can get $\Theta I_3^* < \mu\Phi I_3^*$. Therefore, based on (3.17)-(3.20), we can obtain

and

Let $g_j(I_3^*)(j = 1, 2, 3, 4)$ be the solutions of $F(\lambda) = 0$, and we assume that the real parts satisfy $Re(g_1(I_3^*))\le Re(g_2(I_3^*))\le Re(g_3(I_3^*))\le Re(g_4(I_3^*))$, where $Re$ means the real part of a complex number. Then we can obtain $g_j(I_3^*)(j = 1, 2, 3, 4)$ satisfying

and

So, we have $Re(g_1(I_3^*)) < 0$ and $Re(g_4(I_3^*)) > 0$. Then, we know that the endemic equilibrium $P_3^*$ of system (2.1) is unstable.

(ⅳ) Due to $I_4^* > I_2^* = \frac{1}{\Phi}ln\frac{R_{01}}{R_0}$, we have $\Theta I_4^* > \mu\Phi I_4^*$. Therefore, based on (3.17)-(3.20), we can obtain

and

Let $g_j(I_4^*)(j = 1, 2, 3, 4)$ be the solutions of $F(\lambda) = 0$, and we assume that the real parts satisfy $Re(g_1(I_4^*))\le Re(g_2(I_4^*))\le Re(g_3(I_4^*))\le Re(g_4(I_4^*))$. Then we can obtain $g_j(I_4^*)(j = 1, 2, 3, 4)$ satisfying

and

Therefore, if $Re(g_j(I_4^*)) < 0 (j = 1, 2, 3, 4)$, the endemic equilibrium $P_4^*$ of system (2.1) is stable. However, if $Re(g_1(I_4^*))\le Re(g_2(I_4^*)) < 0 < Re(g_3(I_4^*))\le Re(g_4(I_4^*))$ and $|Re(g_1(I_4^*))|+|Re(g_2(I_4^*))| > |Re(g_3(I_4^*))|+|Re(g_4(I_4^*))|$, the endemic equilibrium $P_4^*$ of system (2.1) is unstable. Thus, the stability of the endemic equilibrium $P_4^*$ is uncertain. This completes the proof of Theorem 4.

3.4.   Forward and backward bifurcation

Theorem 5. (ⅰ) If $R_{01} < 1$, system (2.1) exhibits a forward bifurcation at $R_0 = 1$.

(ⅱ)If $R_{01} > 1$, system (2.1) exhibits a backward bifurcation at $R_0 = 1$.

Proof. We suppose $x_1 = S, x_2 = E, x_3 = I, x_4 = R, x_5 = M$, system (2.1) becomes

When $R_0 = 1$, we obtain $\beta = \beta_c = \frac{\mu(\mu+\varepsilon)(d+\mu+\gamma)}{\delta(\varepsilon+\mu q)}e^{\frac{\alpha\mu_1\delta}{\mu\tau}}$. When $\beta = \beta_c$, the Jacobian matrix corresponding to system (2.1) about the disease-free equilibrium $P_0 = x_0 = \Big(\frac{\delta}{\mu}, 0, 0, 0, \frac{\mu_1\delta}{\mu\tau}\Big)$ is given by

It is clear that 0 is a simple eigenvalue of $J(x_0)$. A right eigenvector $\omega$ corresponding to the 0 eigenvalue is $\omega = (\omega_1, \omega_2, \omega_3, \omega_4, \omega_5)^T$, where

The left eigenvector $\upsilon$ corresponding to the 0 eigenvalue satisfying $\upsilon J = 0$ and $\upsilon\omega = 1$ is $\upsilon = (\upsilon_1, \upsilon_2, \upsilon_3, \upsilon_4, \upsilon_5)$, where

Furthermore, we have $a = \sum\limits_{k, i, j = 1}^5\upsilon_k\omega_i\omega_j\frac{\partial^2f_k(x_0)}{\partial x_i\partial x_j}$ and $b = \sum\limits_{k, i = 1}^5\upsilon_k\omega_i\frac{\partial^2f_k(x_0)}{\partial x_i\partial\beta}$. Substituting the values of all second order derivatives evaluated at the disease-free equilibrium $x_0 = \Big(\frac{\delta}{\mu}, 0, 0, 0, \frac{\mu_1\delta}{\mu\tau}\Big)$, we obtain

and

According to Theorem 4.1 of ^[25], note that the coefficient $b$ is always positive. If $R_{01} < 1$, the coefficient $a$ is negative. In this case, the direction of the bifurcation of system (2.1) at $R_0 = 1$ is forward. If $R_{01} > 1$, the coefficient $a$ is positive. Under this circumstance, the direction of the bifurcation of system (2.1) at $R—_0 = 1$ is backward. This completes the proof of Theorem 5.

4.   Numerical simulation

In this section, we will give some simulations using the parameter values which are given in Table 1.

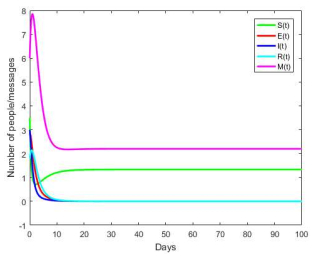
We choose a set of the following parameters: $\delta = 0.8$, $\beta = 0.8$, $\alpha = 0.08$, $\mu = 0.6$, $q = 0.5$, $\varepsilon = 0.09$, $d = 0.02$, $\gamma = 0.7$, $\mu_1 = 0.99$, $\mu_2 = 0.4$, $\mu_3 = 0.8$, $\mu_4 = 0.8$, $\tau = 0.6$. It is easy to check that the basic reproductive number $R_0 = 0.383 < 1$. Then the unique disease-free equilibrium $P_0 = (1.3333, 0, 0, 0, 2.2)$ of system (2.1) is globally asymptotically stable (see Figure 2).

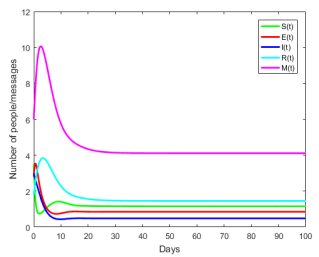
Next, we select a set of the following parameters: $\delta = 0.8$, $\beta = 0.8$, $\alpha = 0.08$, $\mu = 0.2$, $q = 0.1$, $\varepsilon = 0.4$, $d = 0.02$, $\gamma = 0.6$, $\mu_1 = 0.2$, $\mu_2 = 0.8$, $\mu_3 = 0.8$, $\mu_4 = 0.8$, $\tau = 0.6$. It is easy to check that the basic reproductive number $R_0 = 2.4553 > 1$. Then, from Theorem 4, the endemic equilibrium $P_1^*$ of system (2.1) is locally asymptotically stable when $R_0 > \max(1, R_{01})$, where $R_{01} = -0.0158$ (see Figure 3).

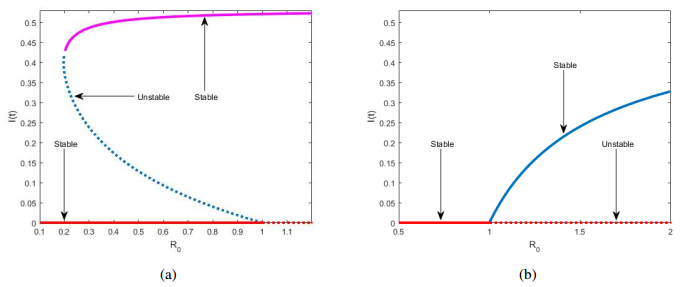
The backward and forward bifurcation diagram of system (2.1) is shown in Figure 4, and the direction of bifurcation depends upon the value of $R_{01}$. As seen in the backward bifurcation diagram of Figure 4(a) when $R_{01} = 4.4936 > 1$, there is a threshold quantity $R_c$ which is the value of $R_{0}$. The disease-free equilibrium is globally asymptotically stable when $R_0 < R_c$, where $R_c = 0.1350$. There are two endemic equilibria and a disease-free equilibrium when $R_c < R_0 < 1$, the upper ones are stable, the middle ones are unstable and the lower ones is globally asymptotically stable. There is a stable endemic equilibrium and an unstable disease-free equilibrium when $R_0 > 1$. As seen in the forward bifurcation diagram of Figure 4(b) when $R_{01} = 0.5357 < 1$, the disease-free equilibrium is globally asymptotically stable when $R_{0} < 1$. There are a stable endemic equilibrium and an unstable disease-free equilibrium when $R_0 > 1$.

5.   Sensitivity analysis and discussion

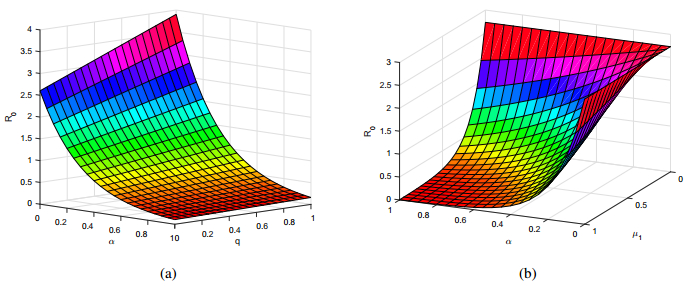
In this section, we discuss sensitivity analysis of the basic reproductive number $R_0$ and the infectious individuals $I$ at first. We study the influence of $\alpha$, $\mu_1$ and $\beta$ to $R_0$. It is straightforward from (3.2) that $R_0$ increases as $\beta$ increases. This agrees with the intuition that higher transmission coefficient increases the basic reproduction number. In order to see the relationship of these parameters and $R_0$, we regard $R_0$ as a function about those parameters. Note that

Therefore, we find that $\alpha$ and $\mu_1$ have a negative influence on the basic reproductive number $R_0$. However, $q$ has a positive influence on the basic reproductive number $R_0$. The parameter values are $\delta = 0.8$, $q = 0.1$, $\beta = 0.8$, $\mu = 0.2$, $\varepsilon = 0.4$, $\gamma = 0.6$, $d = 0.02$, $\mu_2 = 0.8$, $\mu_3 = 0.8$, $\mu_4 = 0.8$, $\tau = 0.6$. From Figure 5, we know that the basic reproductive number $R_0$ will decrease when $\alpha$ and $\mu_1$ increase. However, the basic reproductive number $R_0$ will increase when $q$ increases.

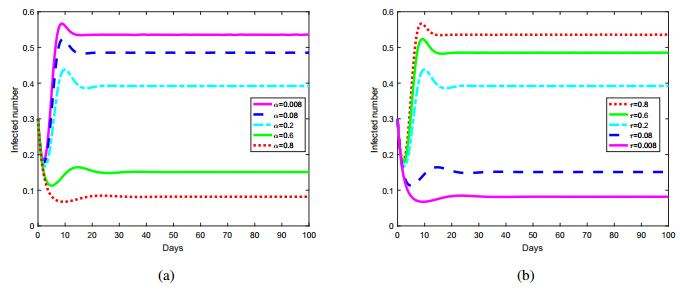
Next, in order to evaluate the effect of media coverage on the dynamics of tuberculosis, we choose different values of $\alpha$ and $\tau$ (see Figure 6). The parameters are $\delta = 0.8$, $q = 0.1$, $\beta = 0.8, \mu = 0.2$, $\varepsilon = 0.4$, $\gamma = 0.6$, $d = 0.02$, $\mu_1 = 0.2$, $\mu_2 = 0.8$, $\mu_3 = 0.8$, $\mu_4 = 0.8$.

From Figure 6, we know that infected number will decrease when $\alpha$ increase, and increase when $\tau$ increases. Therefore, we find that media coverage has a great impact on the transmission of tuberculosis.

Choosing $\beta$ as a parameter, it is also observed that with $\beta$ increasing, the positive equilibrium point $P_1^*$ loses its stability and a Hopf bifurcation occurs when $\beta$ passes a critical values $\beta^*$.

We select a set of the following parameters: $\delta = 0.8$, $\beta = 0.0099$, $\alpha = 0.007$, $\mu = 0.009$, $q = 0.1$, $\varepsilon = 0.99$, $d = 0.5$, $\gamma = 0.99$, $\mu_1 = 0.08$, $\mu_2 = 0.8$, $\mu_3 = 0.8$, $\mu_4 = 0.8$, $\tau = 0.6$. The endemic equilibrium $P_1^*$ of system (2.1) is locally asymptotically stable when $R_0 > \max\{1, R_{01}\}$ and $\beta < \beta^*$ (see Figure 7).

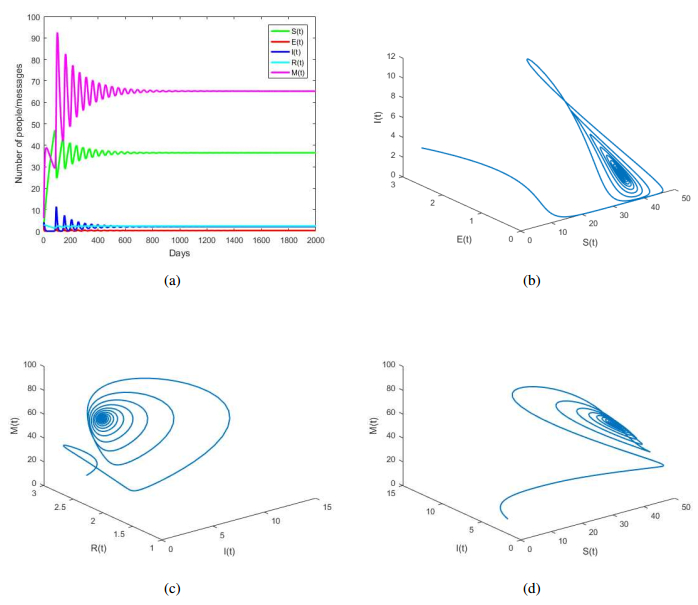
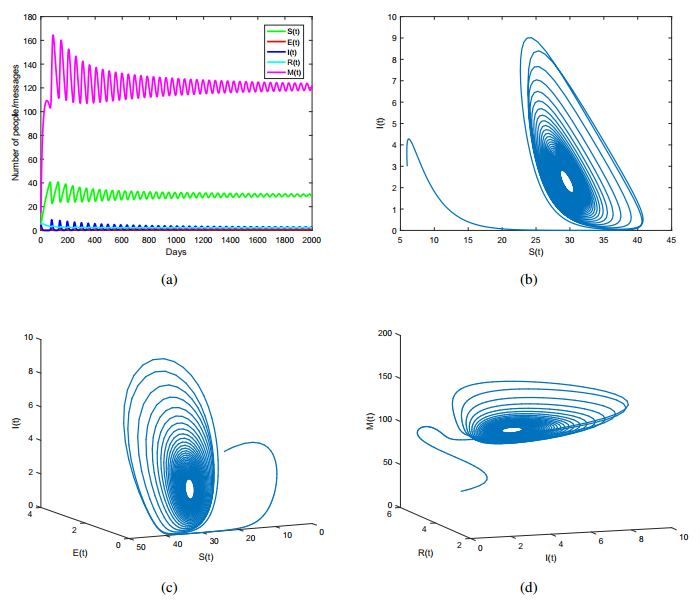
To illustrate the existence of Hopf bifurcation, we choose a set of the following parameters: $\delta = 0.8$, $\alpha = 0.007$, $\mu = 0.009$, $q = 0.5$, $\varepsilon = 0.99$, $d = 0.02$, $\gamma = 0.1$, $\mu_1 = 0.09$, $\mu_2 = 0.008$, $\mu_3 = 0.08$, $\mu_4 = 0.08$, $\tau = 0.03$. When $\beta$ passes through the critical value $\beta^*$, we find the positive endemic equilibrium $P_1^*$ loses its stability and a Hopf bifurcation occurs (see Figure 8).

In this paper, we propose and analyse a TB model with fast and slow progression and media coverage. By means of the next-generation matrix, we obtain the basic reproductive number $R_0$, which plays a crucial role in our model. By constructing Lyapunov function, we prove the global stability of the disease-free equilibrium. In addition, we obtain the existence and the local stability of the endemic equilibrium. By using the center manifold theory, we get a backward and forward bifurcation. Furthermore, we give a numerical result about a Hopf bifurcation occurs when $\beta$ passes through the critical value $\beta^*$. At last, we also use numerical method to simulate outcomes which we have been proved.

The initially exposed individuals have a higher risk of developing active TB. They still have the possibility of progressing to infectious TB with time passing. The likelihood of becoming an active infectious case decreases with the age of the infection. Taking these factors into consideration, we set up a new tuberculosis with fast and slow progression and media coverage. Through simulations, we know that $\beta$ plays an important role and induces Hopf bifucation in our model. Furthermore, we have done some simulations (not shown). We did not find other critical parameters (including $q$) for Hopf bifurcation. $q$ is the proportion of disease by fast progression. Since $R_0 = \rho(FV^{-1}) = \frac{(\varepsilon+\mu q)\beta\delta e^{-\frac{\alpha\mu_1\delta}{\mu\tau}}}{\mu(\mu+\varepsilon)(d+\mu+\gamma)}$, we can find the basic reproductive number $R_0$ will increase when $q$ increases. Tuberculosis may breakout due to the increase of $q$. The fast and slow progression can not induce Hopf bifurcation, but it still plays an important role in TB transmission and has a positive influence on the basic reproductive number $R_0$.

Our results show that media coverage has a substantial influence on the dynamics of tuberculosis and it can greatly influence the spread of the tuberculosis, thus, it is crucial to remind people to take countermeasures to avoid potential infections by media coverage.

In our model (2.1), we only consider the form of ordinary equation. Note that all of the people have a time delay in releasing and receiving information, it is more realistic to explore a time delay in the rate that media coverage become outdated. On the other hand, as suggested by Styblo et al. ^[27], recovered individuals may only have partial immunity. Indeed, TB is one kind of chronic infectious diseases that has a certain relapse rate due to the drug-resistant tuberculosis and lack of combination drug regimen. Thus, it is a very interesting and more realistic to study our model with reinfection, that is some individuals in the recovered class can relapse back into the active TB state. We leave these interesting works for the future.

Acknowledgments

The authors are very grateful to the Editor-in-Chief and the anonymous referees for their valuable comments and suggestions which helped us to improve the paper. This work is supported by the National Natural Science Foundation of China (11861044 and 11661050), and the HongLiu first-class disciplines Development Program of Lanzhou University of Technology.

Conflict of interest

The authors declare there is no conflict of interest.