Modeling COVID-19 transmission dynamics incorporating media coverage and vaccination

1.   Introduction

Coronavirus disease 2019 (COVID-19) has spread all over the world since December 2019, causing a dramatic impact on global public health. Up to now, COVID-19 is still producing a pandemic worldwide with the emergence of new SARS-CoV-2 variants ^[1]. In order to control the spread of the epidemic, many mathematical models were developed and studied. Some literature focuses on the impact of media coverage and vaccination on the prevalence of COVID-19. In 2020, Yan et al. proposed a novel model to analyze the impact of media coverage on the transmission of the COVID-19 epidemic ^[2]. The results implied that the management departments should guide people's behavior through media coverage and apply effective quarantine strategies. Collinson et al. employed a stochastic agent based model to study the effects of mass media coverage on the 2009 H1N1 pandemic. The study indicated that reporting rates and the speed of media fatigue greatly affected the variability of important public health measures. When mass media coverage data was considered, two peaks of infection occurred ^[3]. Koutou et al. proposed a mathematical model with a standard incidence rate to assess the role of media such as in the mitigation of the propagation of COVID-19 ^[4]. Liu et al. ^[5] evaluated how media coverage can alleviate the prevalence of COVID-19 by constructing a provincial dataset on COVID-19. It turned out that the impact of media reports on the spread of COVID-19 in China was an inverted U-shaped curve, and it was mediated by intra-provincial and inter-provincial population mobility. In 2022, based on the transportation network among 31 provinces in China, Zou et al. established a multi-patch coupling model under the strategy of combining vaccination and quarantine, and then derived critical quarantine rate to control the spread of the pandemic and vaccination rate to achieve herd immunity ^[11]. In ^[12], Avila-Ponce de León et al. developed a mathematical model to explain the effects of two strains and vaccination on the transmission of COVID-19. The mathematical model yielded the minimum percentage of fully vaccinated individuals to reduce the spread of the variants. In ^[13] Song et al. investigated a mathematical model of COVID-19 transmission with vaccination and isolation delay, and analyzed the optimal control strategy. They found that the optimal isolation rate could minimize the cumulative number of infected people and the cost of disease control, and it could effectively control the propagation of disease with limited resources. In ^[14], Krueger et al. evaluated the impact of vaccines, other public health measures and the decline of immunity on the control of COVID-19. The results showed that the high re-vaccination rates and the reduction of the proportion of unvaccinated population increased the benefits of vaccination pass and need to remain vigilant. In order to restrain the rapid spread of the omicron variant in Europe, health professionals and researchers from across Europe clarified three measures in ^[15] as follows: reducing the number of infected people, protecting children from infection and buying time for more people to be vaccinated. Markovic et al. proposed and studied a social network epidemiological model considering population heterogeneity and different vaccination strategies. They explained how COVID-19 evolved and how different vaccination schemes controlled the epidemic ^[16]. ^[17,18,19] proposed a series of COVID-19 transmission dynamics models with vaccination, and proved that COVID-19 vaccination can decrease the size of infection. Additionally, there are also literatures modeling and demonstrating the positive role of media reporting in controlling the spread of the epidemic ^[20,21,22].

Motivated by the above literature, we focus on revealing the impact of media coverage and vaccination on the transmission of COVID-19 by different behavior change constant $k$ and vaccine efficiency. Furthermore, some people will be infected after vaccination. However, as far as we know, there is no research on combining the two measures. So, we formulate an SVEAIQR model based on the data from Shanghai in April 2022, and we estimate the parameters of the force of infection, media coverage, vaccine efficiency and other related parameters using the least squares method. Through numerical simulations, we clarify the impact of media coverage and vaccination on the final size of infected people, and we verify the significance of isolating asymptomatic infected persons for the epidemic in Shanghai in April 2022.

The rest of the paper is organized as follows. In Section 2, we present the SVEAIQR model, and the final size without vaccination is derived. In Section 3, first, we fit the parameters according to the data of Shanghai and analyze the sensitivity of the parameters. Second, we simulate the changes of the number of infected people under different media coverage, vaccine efficiency and isolation rates. Then, we summarize our findings in Section 4.

2.   Mathematical model

2.1.   COVID-19 Epidemic Model

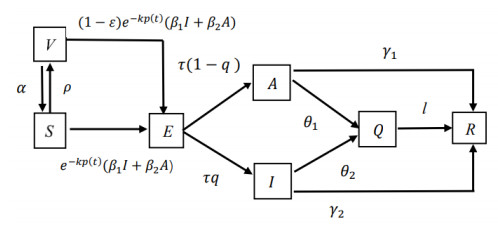
Due to the transmission mechanism of COVID-19 and control measures, we divide the population into seven subclasses including susceptible ($S$), vaccinated ($V$), exposed ($E$), asymptomatic ($A$), symptomatic ($I$), quarantined ($Q$), and recovered ($R$). Let $N$ denote the total number of the population. We provide the transfer flow chart in Figure 1:

Then, we construct an epidemic model as follows:

For model (2.1), we propose the following assumptions:

1. Vaccinated individuals in compartment $V$ lose vaccine protection and become susceptible at per-capita rates $\alpha$ and $\rho$, respectively. However, the vaccine fails to protect a portion $(1-\varepsilon)$ of individuals.

2. The force of infection is reduced by the function of mask wearing rate $e^{-kp(t)}$, which describes the impact of individual behavioral changes (media) on the COVID-19 epidemic.

3. Individuals in compartment $E$ become symptomatic infected in the proportion of $q$, and the transmission rate is $\tau$.

4. Asymptomatic infected persons and symptomatic infected persons are isolated at rates $\theta _{1}$ and $\theta _{2}$, respectively.

5. The average duration of infectiousness is $1/l$.

The variable parameters and biological significance in model (2.1) are shown in Table 1.

Let $I$ = 0 and all the right sides equal zero in model (2.1), and we can obtain the disease-free equilibrium $E^{0} = (S^{0}, V^{0}, 0, 0, 0, 0, 0)$.

The control reproduction number $R_{c}$ is a threshold that reflects the transmission ability, indicating the average number of secondary infections produced by an infected person during the infection period under the implementation of certain prevention and control measures. When $R_{c} < 1$, the disease can be effectively controlled, and when $R_{c} > 1$, the disease will persist. The control reproduction number can be calculated by the method of the next generation matrix ^[9].

We write as

Then, we can calculate $R_{c} = \rho (FV^{-1} \mid _{E^{0} }) = \frac{(1-q)\beta _{2}M}{\gamma _{1}+\theta _{1} }+\frac{q\beta _{1}M}{\gamma _{2}+\theta _{2} }$, where $M = [S^{0} +(1-\varepsilon)V^{0}]e^{-kp(t)}$.

The biological interpretation of $R_{c}$ is as follows: The component 1 represents the numbers of secondary cases produced by an asymptomatic infected case with $(1-q)$ ratio in the $\frac{1}{\gamma _{1}+\theta _{1} }$ infection period. The component 2 indicates the number of new cases of symptomatic infected persons with $q$ ratio during the infection period $\frac{1}{\gamma _{2}+\theta _{2} }$. Based on the fitted data in Table 1, we calculate the control reproduction number $R_{c} = 0.5266$.

2.2.   Final size

Let $V = 0$ in model (2.1), then model (2.1) reduces to the following model (2.2):

with initial conditions $S(0) = N-E_{0}-A_{0}-I_{0}-Q_{0}-R_{0}$, $E(0) = E_{0}$, $A(0) = A_{0}$, $I(0) = I_{0}$, $Q(0) = Q_{0}$, $R(0) = R_{0}$. Similar to the method used in model (2.1), we can get the control reproduction number of model (2.2):

Adding the first two equations of model (2.2), we obtain

We call $\dot{S}(t) +\dot{E}(t)$ uniformly continuous, and then $\lim\limits_{t\to \infty}(\dot{S(t)}+\dot{E(t)}) = 0$, so for any $\varepsilon > 0$, there exists a $\delta = \delta (\varepsilon) > 0$ satisfying

Hence, the limit of $S(t)+E(t)$ exists. By convergence principle, there is a $T = T(\varepsilon) > 0$, such that when $t > T$ we have

So, the following formula holds:

where $\alpha \in (t, t+\delta)$. Thus,

According to the third and fourth equations of model (2.2), we can gain $\lim\limits_{t\to \infty}$ sup $I(t)\le 0$ and $\lim\limits_{t\to \infty}$ sup $A(t)\le 0$. Since $A(t) > 0, I(t) > 0$, $\lim\limits_{t\to \infty} I(t) = \lim\limits_{t\to \infty} A(t) = 0$ holds. For the sixth equation of model (2.2), similarly, we have $\lim\limits_{t\to \infty} Q(t) = 0$. Therefore, $S(\infty)$ exists.

In model (2.2), it follows that

Furthermore, we have

Adding the first three equations of model (2.2), we have

and then integrating both sides yields

Substituting (2.4) into the above equation gives

Similarly, adding the first four equations of model (2.2), and we have

Combining (2.5) gives

From (2.5) and (2.6), we can conclude that

is convergent. Therefore,

Divide the first equation of model (2.2) by $S$ and then integrate, we have

That is,

Furthermore, we have

Based on ^[10], we take $E(0) = A(0) = I(0) = 0$, and $S(0) = N$. Then,

By the above formula, we can combine $R_{c0}$ to infer the influence of parameters on the final size of the epidemic, the number of the population who are infected over the course of the epidemic, is $N-S(\infty) = S(0)-S(\infty)$ ^[23].

3.   Model simulations

In this study, the COVID-19 data comes from the Shanghai Municipal Health Commission ^[6] and National Health Commission of the People's Republic of China ^[24]. The total population of Shanghai is around $N$ = 24894300, and thus we choose the initial values as $S(0) = 20000000, V(0) = 5000000, E(0) = 7000, A(0) = 8000, I(0) = 2000, Q(0) = 3600, R(0) = 10000$.

In Figure 2, we draw the actual number of COVID-19 cases from Shanghai and the fitted curve by model (2.1), respectively. Simultaneously, the fitted parameter values are presented in Table 1.

The LHS / PRCC method, namely, latin hypercube sampling / partial rank correlation coefficient method, is a global parameter sensitivity analysis method, which can quickly and effectively identify the sensitive parameters to $R_{c}$ in the model, and is applicable to the correlation analysis among multiple variables. The greater the absolute value of partial rank correlation coefficient (PRCC), the stronger the correlation is. A positive (negative) value represents a positive (negative) correlation.

As shown in Figure 3 (a), the isolation rate of asymptomatic infection $\theta _{1}$ and recovery rate of asymptomatic infection $\gamma _{1}$ are negatively correlated with the control reproduction number $R _{c}$. In addition, the force of infection for asymptomatic $\beta_{2}$, the proportion of symptomatic infection $q$ and the force of infection for symptomatic $\beta_{1}$ are positively correlated with $R _{c}$, where $\beta_{2}$ is the strongest negative partial rank correlation coefficient, which is because the asymptomatic infections accounted for a large proportion of infections in Shanghai. Therefore, the isolation rate of infected people should be strengthened, and people should be called for nucleic acid testing, so as to effectively prevent and control the disease transmission during the epidemic in Shanghai in April 2022.

To study the impact of media coverage and vaccination, we conducted a sensitivity analysis on $k$ and $\varepsilon$. From Figure 3 (b), we know that with the increase of behavior change constant $k$ and vaccine efficiency $\varepsilon$, the control reproduction number $R _{c}$ decreases. Therefore, it is necessary to reinforce the impact of media coverage and vaccine efficiency for COVID-19.

Figure 4 describes how different measures affect the final size of COVID-19 epidemic in Shanghai in April 2022. First of all, without any measures, the final size of infectious diseases is the largest. Also, the final size with only vaccination is 1.08 times that with only media coverage, and media coverage can reduce the final size by about 0.26 times. So, the outcome suggests that media coverage is more effective than vaccination.

In case of only considering the influence of media coverage, Figure 5(a) shows that the peak value of the epidemic decreases by 5000 when the behavior change constant $k$ from 0.01 to 0.5. Figure 5 (b) indicates that under the combined effect of vaccination and media coverage, the peak value of the epidemic cuts down by about 4000 with the increase of the behavior change constant $k$ changes from 0.01 to 0.5. Comparing Figure 5(a) and 5(b), we can see that, when $k = 0.01$, vaccination can reduce the peak of infection by more than 2000 during the outbreak of COVID-19 epidemic in 2022 in Shanghai. Therefore, public health departments should encourage people to take vaccine actively, and timely provide the information about the dynamic situation of the epidemic and the effective prevention strategy by media coverage.

In Figure 6, we examine the effect of vaccine efficiency $\varepsilon$ on the number of infected people. One of the purposes is to observe whether increasing vaccination efficiency will always be beneficial in terms of reducing the infection level. Compared with 50% vaccine efficiency, when the vaccine efficiency reaches 90%, the peak value of infected people decreases by about 0.07 times. Furthermore, with the improvement of vaccine efficiency $\varepsilon$, the peak time for COVID-19 can be advanced.

Since there are many asymptomatic infections during the outbreak of COVID-19 in Shanghai in April 2022, isolation of asymptomatic infected persons is very significant to control COVID-19. Figure 7 indicates that the number of infected people always increases when the isolation rate of asymptomatic infection $\theta _{1}$ is 0.3, and when the isolation rate is not less than 50%, the number of infected people eventually decreases. Hence, the number of infected people declines only if the isolation rate reaches a certain threshold. This is consistent with the previous sensitivity analysis (in section 3). Therefore, during the COVID-19 outbreak in Shanghai, it is necessary to enhance the isolation of asymptomatic infections.

4.   Conclusion

On the basis of the available, we investigate the impact of media coverage and vaccination on the spread of COVID-19. Concurrently, the efficacy of the vaccine will continue to decline over time, and then some people will be infected again. Thus, we put forward a class of SVEAIQR infectious disease model. The main intention of this study is to examine the joint impact of vaccination and media coverage on the prevalence of COVID-19. The control reproduction number is calculated, and there is formula derivation for the final size of the outbreak in case of non-vaccine.

According to the COVID-19 data of Shanghai in April 2022, we fitted the relevant parameters through the least squares method and then performed control measures related numerical experiments. Sensitivity analysis makes clear that the behavior change constant $k$ and vaccine efficiency $\varepsilon$ are negatively correlated with $R_{c}$. Under the combined effect of vaccination and media coverage, the peak value of the epidemic cuts down by about 4000 with the increase of the behavior change constant $k$. When the vaccine efficiency $\varepsilon$ increases from 0.5 to 0.9, the peak value of infected people decreases by about 2000. This indicates that increasing vaccine efficiency and media coverage intensity will reduce the control reproduction number and consequently will alleviate the risk of disease transmission. At the same time, our simulations have revealed the number of infected people always increases when the isolation rate of asymptomatic infection $\theta _{1}$ is 0.3. It declines only if the isolation rate reaches a certain threshold.

Our model contains seven essential compartments, but it still has some deficiencies. First of all, this paper simulates the epidemic data only from Shanghai in April 2022, and the COVID-19 data of other countries and regions were not used for fitting. If you want to apply this model to fit the COVID-19 transmission of other countries and regions, you should consider different strategies. Second, although media coverage can change people's behaviors, our research depicts this aspect in a very simple form, that is, the effect of media education on the propagation of COVID-19 is reflected in the rate function of wearing masks. In addition, the media broadcast will also have saturation or retroaction, and we feel impelled to investigate further. Finally, we only consider the fixed vaccination rate. In fact, vaccination strategy is a very complex process. Generally, our study reveals that vaccination and media coverage have significant effects on alleviating the spread of COVID-19. So, the authorities should stress precise and differentiated strategies in the prevention and control of the novel coronavirus epidemic.

Acknowledgments

This research is supported by the National Nature Science Foundation of China (Grant No. 12071445), the BUCEA Post Graduate Education Teaching Quality Improvement Project (J2023021) and the BUCEA Post Graduate Innovation Project (No. PG2022142, PG2023145). We thank all the individuals who provided support and guidance to this study.

Conflict of interest

The authors declare there is no conflict of interest.