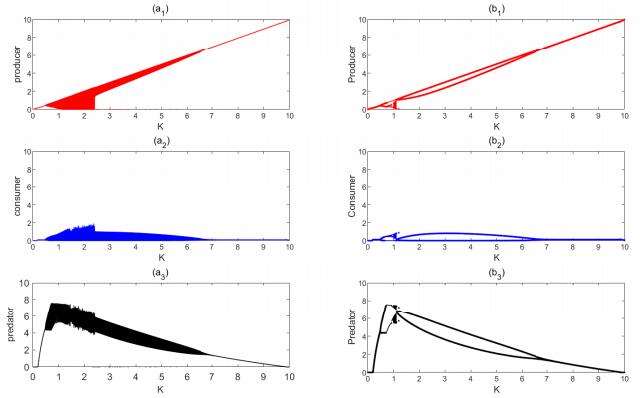
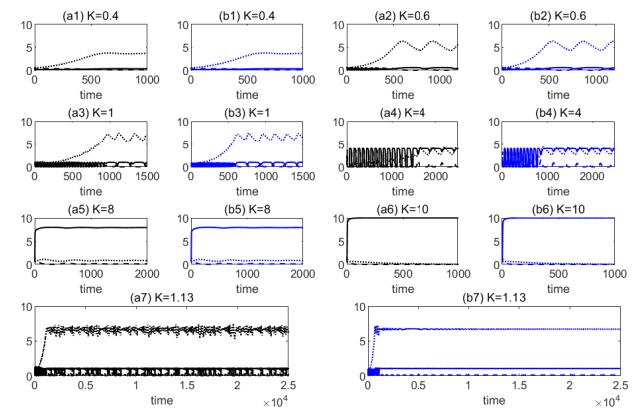
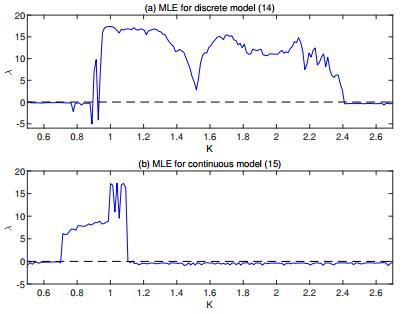
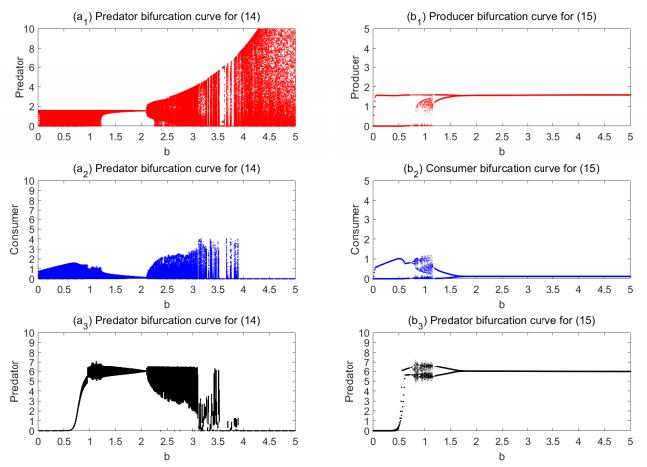
Stoichiometry-based models can yield many new insights into producer - grazer systems. Many interesting results can be obtained from models continuous in time. There raises the question of how robust the model predictions are to time discretization. A discrete stoichiometric food-chain model is analyzed and compared with a corresponding continuous model. Theoretical and numerical results show that the discrete and continuous models have many properties in common but differences also exist. Stoichiometric impacts of producer nutritional quality also persist in the discrete system. Both types of models can exhibit qualitatively different behaviors with the same parameter sets. Discretization enlarges the parameter ranges for the existence of chaotic dynamics. Our results suggest that the stoichiometric mechanisms are robust to time discretization and the nutritional quality of the producer can have dramatic and counterintuitive impacts on population dynamics, which agrees with the existing analysis of pelagic systems.
1.
Introduction
Recently, the promising attention of statisticians towards the construction of new generalized distributions has been increased to explore and develop more flexible models from the baseline models. These generalized distributions open new horizons to study real-world problems and to provide more flexibility to model the asymmetric and complex random phenomena. Therefore, several models have been developed in the literature. One of the most handy and simplest lifetime distributions induced in the statistical literature is the exponential (Ex) distribution. It has been extensively adopted for analyzing lifetime data due to its simplicity and its lack of memory property.
On the other hand, the Ex distribution with only a constant hazard rate (HR) shape is not suitable for fitting data with different HR shapes as decreasing, unimodal (upside down bathtub), increasing or bathtub shaped hazard rates which are common in reliability and engineering, among other areas. This limitation has motivated the researchers to develop more flexible extensions of the Ex model.
For more details about other methods, the interested reader can see [1,2,3,4,5,6,7,8,9,10,11,12,13,14,15].
In the present article, we are motivated to develop a new flexible version of the Ex model called the weighted-exponential (WDEx) distribution, which can provide greater flexibility in fitting insurance data. Additionally, the aim of the article is three-fold: the first is devoted to studying a new form of the Ex distribution based on the weighted-G (WD-G) family [16], called the WDEx distribution. Basic distributional properties of the WDEx model are explored. The WDEx model has some desirable characteristics. It can accommodate upside-down bathtub, decreasing, decreasing-constant, increasing, and increasing-constant HR shapes. Its probability density function (PDF) possesses unimodal, right-skewed, reversed-J shape, left-skewed. The tail of the WDEx distribution is heavier than the EEx and Ex distributions as shown in Section 4 based on computational values of risk measures. The WDEx distribution can be used to model insurance data. It provides an adequate fit than other fourteen competing Ex distributions as illustrated in Section 7 for this insurance data set.
The second objective of this paper is devoted to exploring five important actuarial or risk measures, called the value at risk (VaR), tail value at risk (TVaR), tail-variance (TV), tail-variance premium (TVP), and expected shortfall (ES), for the WDEx distribution. These measures play an important role in portfolio optimization under uncertainty.
The third objective is to study the estimation of the WDEx parameters by classical and Bayesian methods. Hence, nine estimation approaches are adopted for this purpose. We explore and rank the performance of these estimators based on empirical simulation results to develop a guideline for choosing the best estimation approach for estimating the WDEx parameters.
The paper is organized in eight Sections. In Section 2, we define the WDEx model. Its basic properties are explored in Section 3. Five actuarial indicators are studies in Section 4. The model parameters are estimated using classical and Bayesian estimation in Section 5. Simulation results are provided in Section 6. Section 7 is devoted to analyzing a real-life data. Finally, some remarks are given to conclude the paper in Section 8.
2.
The WDEx distribution
In this section, we define the two-parameter WDEx distribution based on the WD-G family of [16]. The cumulative distribution function (CDF) and PDF of the WDEx distribution has the forms
and
The survival function (SF), HR function (HRF) and the reversed-HRF (RHRF) are, respectively, given by
and
The limiting behaviors of the PDF (2.2) and HRF (2.4) are given by
and
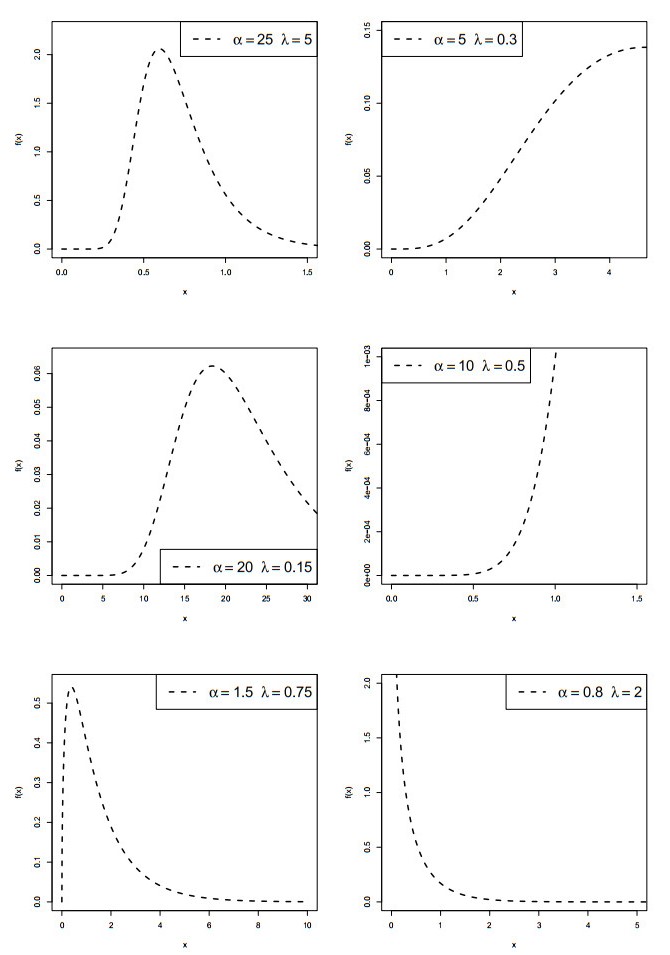
Plots of the PDF and HRF of the WDEx distribution are illustrated in Figures 1 and 2, respectively. These graphs show that the WDEx model possesses decreasing-constant, upside-down bathtub, increasing, decreasing, and increasing-constant HR functions along with sudden spikes at the end of upside-down bathtub shape, which manages the model to analyze a non-stationary real-life data.
The quantile function (QF) of the WDEx distribution is given by
we can determine first, second and third quartile of the WDEx distribution by setting u=0.25,0.5,0.75, respectively. Also, by using Eq (2.6), we can determine Bowley's skewness and Moor's kurtosis for the WDEx distribution. The WDEx skewness and kurtosis graphs for the different parametric values of α and λ are shown in Figure 3.
3.
Probabilistic and statistical properties
In this section, we provide some mathematical properties of the WDEx distribution.
3.1. Linear representation
A useful linear representations of the CDF and PDF of the WDEx model are introduced in this subsection. For −1<x≤1, we have
By applying the power series (3.1) to (2.1), the CDF and PDF of the WDEx distribution can be expressed as
and
where Φk=(−1)k+1klog(2), Gk(x)=(1−e−λx)αk and gk(x)=αkλe−λx(1−e−λx)αk−1 are the CDF and PDF of the exponentiated-exponential (EEx) distribution with parameters λ and αk.
3.2. Moments and generating function
The rth moments of the WDEx distribution takes the form
where D(a−1,b)=(a−1)…(a−b)b!. Replacing r=1,2,3, and 4 in the last formula, we obtain the first four moments for the WDEx distribution.
The nth moment residual life of the WDEx distribution, E(Xn|X>x), has the form
where z=exp(−λy) and the mean residual life function of the proposed model is E(X|X>x)−1.
the moment generating function (MGF) of the WDEx distribution is
where β(a,b) is beta function. The characteristic function of the WDEx model is
3.3. Mean deviation, bonferroni and Lorenz curves
The mean deviation about the mean of the WDEx model reduces to
The mean deviation about the median of the WDEx distribution takes the form
The Bonferroni and Lorenz curves for a positive random variable X are defined the following formulae
where the quantity E(X|X≤x) has the form
Hence,
and
where xp=−log[1−(2p−1)1/α]λ is the QF of the WDEx model.
3.4. Order statistics
The PDF and CDF of the ith order statistic for the WDEx distribution are given by
and
where 2F1(1,i−n;i+1;−log((1−e−xλ)α+1)log(2(1−e−xλ)α+1)) is a hyper geometric function.
4.
Actuarial measures
Five risk measures are explored for the WDEx distribution in this section. Some actuarial measures such as VaR, TVaR, TV, TVP, and ES are calculated. A brief simulation study for these measures is provided. Finally, an application to the insurance loss data set is analyzed. For more information we refer to refs [17,18,19,20,21].
4.1. VaR measure
The VaR is often referred to as the quantile risk measure or the concept of quantile premium, and it is defined with a certain amount of confidence, say q (typically 90, 95, or 99%). In comparison, VaR is a quantitative sum of accumulated loss distribution. Risk management are also interested in the "chance of an unfavorable outcome" and can be represented at a specific likelihood level using the VaR. The VaR of a random variable X is the qth quantile of its CDF, denoted by VaRq, and it is defined by VaRq=Q(q) (see Artzner [22]).
The VaR measure of the WDEx distribution is expressed by
4.2. TVaR measure
The TVaR, which is now being assigned many names, including conditional tail-expectation and conditional VaR, is another significant indicator. The TVaR is used to measure the potential value of the loss provided that there has been an occurrence outside of a provided probability level. The TVaR of a random variable X is represented by
The TVaR of the WDEx distribution is
4.3. TV measure
Landsman [23] presented the TV risk that is described by the variance of the loss distribution beyond some critical value. The TV of the WDEx distribution is described as
where
by using Eqs (4.1)–(4.3), we get the TV of the WDEx distribution.
4.4. TVP measure
The TVP is also another effective criterion in the insurance fields. The TVP of the WDEx distribution has the form
where 0<q<1. The TVP of the WDEx distribution follows by replacing Eqs (4.1) and (4.2) in (4.4).
4.5. ES measure
Artzner [22] developed the expected shortfall (ES) as a common indicator in financial risk. In some definitions, if the underlying distribution of X is continuous then the ES coincides with the Conditional tail expectation and the TVaR. The ES of the WDEx distribution takes the form
4.6. Numerical simulations for risk measures
In this sub-section, we discuss some empirical results for the five aforemention risk measures for the WDEx, EEx, and Ex distributions for some parametric values. The findings are obtained through to the followings steps:
(1) Random sample of size n=100 is produced from the WDEx, EEx, and Ex distributions, and their parameters are calculated via the maximum likelihood (ML) approach.
(2) 1000 Replications are designed to determine the mean of the VaR, TVaR, TV, TVP as well as ES for the three distributions.
Simulation results of the average VaR, TVaR, TV, TVP and ES for the WDEx, EEx, and Ex distributions are provided in Tables 1 and 2 and displayed graphically in Figures 4 and 5.
The model with highest value of VaR, TVaR, TV, TVP and ES metrics does also have a heavier tail than other competitor models. The results illustrate that the WDEx model has improved quantities of the five risk factors than the EEx, and Ex distributions. Thus, the WDEx model with these parameter values has a heavier-tail than other distributions and could be used efficiently to model heavy-tailed insurance data.
5.
Classical and Bayesian estimation
This section is devoted to address the estimation of the WDEx parameters by various estimation methods including eight classical estimation approaches, such as the MLE, Anderson–Darling (ADE) and right-tail Anderson–Darling (RTADE), Cramér–von Mises (CVME), least-squares (LSE), weighted least-squares (WLSE), maximum product of spacings (MPS) and percentile estimation (PCE), and Bayesian approach under three loss functions namely, square error (SE), linear exponential (LINEX), and the general entropy (GE).
Consider the random sample of size n, say x1,x2,…,xn, from the PDF (2.2), and let x1:n,x2:n,…,x2:n be their associated order statistics.
5.1. Maximum likelihood
The log-likelihood function of the WDEx distribution has the form
Hence, the MLEs of α and λ are determined by maximizing Eq (5.1) with respect to α and λ, as the solutions of the following equations
and
Furthermore, solving the above two equations mathematically is complicated, hence the equations can be solved by the numerical methods to obtain the MLE of α and λ.
5.2. Ordinary and weighted least-squares
The LSE of the WDEx parameters come from minimizing
The LSE of the WDEx parameters are also obtained by solving the following equations
where
The WLSE of the WDEx parameters can be calculated by minimizing
Furthermore, the WLSE of the WDEx parameters are obtained by solving the following equations
where Δs(xi:n),s=1,2,3 are given by Eqs (5.2) and (5.3).
5.3. Anderson-Darling and right-tail Anderson-Darling
The ADE of the WDEx parameters are obtained by minimizing
The ADE are also calculated by solving the following equations
where Δs(xi:n),s=1,2,3 were defined in (5.2) and (5.3), respectively.
The RTADE of the WDEx parameters can be calculated by minimizing
The RADE can also be follow by solving the non-linear equations
where Δs(xi:n),s=1,2,3 are defined in (5.2) and (5.3).
5.4. Maximum product of spacings
As an alternative to the ML method, the maximum product spacing (MPS) approach is adopted to estimate the parameters of continuous univariate distributions. The uniform spacings, say Di, of a random sample of size n from the WDEx distribution are defined by
where F(x0)=0, F(xn+1=1) and ∑n+1i=1Di=1. Then, the MPSE of the WDEx parameters can be obtained by maximizing
with respect to α and λ. Further, the MPSE of the WDEx parameters are also obtained by solving
where Δs(xi:n),s=1,2,3 are presented in (5.2) and (5.3).
5.5. Percentile estimation
Let pi=in+1 be an estimate of F(xi:n). Hence, the PCE of the WDEx parameters are obtained by minimizing
The PCE are calculated by solving the following equations
where
5.6. Cramér–von mises
The CVME of the WDEx parameters are obtained by minimizing
They also are obtained by solving the following equations
where Δs(xi:n),s=1,2,3 are given in (5.2) and (5.3).
5.7. Bayesian estimation
The Bayesian estimation (BE) for the distribution parameters under different loss functions such as the SE, LINEX, and GE loss functions is studied. The parameters are assumed to have gamma priors. Thus,
and
Suppose that the parameters are independent, therefore the joint PDF of the prior distribution has the form
The posterior density function of the parameters α and λ can be obtained from (5.1) and (5.4), as follows
The BE of the function of parameters U=U(Θ), Θ=(γ,α,θ1,b) under the SE loss function (LF) is given by
Under the LINEX LF, the BE of U=U(Θ) reduces to
where c≠0 is the shape parameter of the LINEX LF.
Based on the GE LF, the BE of U takes the form
where q≠0.
It is obvious that the integrals in Eqs (5.6)–(5.8) are complicated. Consequently, the important sampling method and the MCMC method are applied to obtain an approximation for these integrals. The Metropolis Hasting (MH) algorithm produces a series of draws from the WDEx distribution as follows:
5.8. TK-approximation for Bayesian estimates
One of the most computationally efficient methods, for estimating the distribution parameter is the Tierney and Kadane [24] (TK) method. They developed an easily calculable integration approximation approach for evaluating the distribution parameters. The advantage of the TK approach is that it provides an easy and fast approximation method, however some methods are very hard to find the values of integration and takes a lot of time and effort.
Throughout this segment, we use the TK approximation method for calculating the Bayesian estimates (BEs) of the parameters that are focused on the SE, Linex, and GE LFs. TK method [24] is among the techniques to obtain the estimated value of the ratio of the two integrals as given in the following equation
where U(Θ) is any function of the parameters (α,λ), l(Θ|t) is defined in (5.1), and ρ(Θ|t) is the logarithm joint prior distribution which is defined by
To obtain an explicit expression for ˆUSE(Θ) using TK approximation, we consider the following functions
and
Now, assume that the following groups of values (ˆαδ,ˆλδ) and (ˆαδ∗,ˆλδ∗) maximize the functions δ(α,λ) and δ∗(α,λ), respectively.
Hence, we can evaluate the approximation of ˆU(Θ) by
where |Δ| and |Δ∗| refers to the determinants of negative inverse hessian of δ(α,λ) and δ∗(α,λ), respectively.
6.
Simulation results
Now, we asses the performance of the above estimation techniques in estimating the WDEx parameters using simulation results. Different sample sizes, n={15,30,70,100,200,350}, and some initial values are assigned. We generated 5000 random samples from the WDEx distribution to calculate the average absolute biases (ABBs), mean square errors (MSEs), and mean relative errors of estimates (MREs) using the R program©.
The following respective mathematical formulae are used to evaluate the ABBs, MSEs, and MREs (for θθ=(α,λ)′)
Table 3 reports the simulation results including the ABBs, MSEs, and MREs of the WDEx parameters using the nine estimation approaches. One can note that all methods provide small ABBs, MSEs and MREs in all parametric values. The nine estimators are consistent, i.e., the MSEs decrease as the sample size increases, for all studied cases.
7.
Modeling an insurance data
Here, we analyze a data set from the insurance sector to illustrate the utility of the WDEx distribution. This data set reflects the monthly unemployment insurance metrics from July 2008 to April 2013, and it includes 58 observations as recorded by the department of labor, state of Maryland, USA. The data frame consists of 21 variables and the variable number 9 is fitted using the WDEx model. Data is listed below and it can be found at: https://catalog.data.gov/dataset/unemployment-insurance-data-from-July-2008-to-April-2013.
We compare the WDEx distribution with some well-known competing distributions including the beta exponential (BEx) [25], gamma (G), exponentiated Weibull (EW) [26], Harris extend exponential (HEEx) [27], alpha power exponentiated-exponential (APEEx) [28], Frechet–Weibull mixture exponential (FWMEx) [29], Marshall–Olkin alpha-power exponential (MOAPEx) [30], Marshall Olkin exponential (MOEx) [31], Frechet–Weibull (FW) [32], Weibull (W), alpha-power exponential (APEx) [33], transmuted exponential (TEx) [34], EEx, and Ex distributions. The competing models are compared by using some discrimination measures such as Anderson–Darling (AD), Cramér–von Mises (CM), and Kolmogorov–Smirnov (KS) with its p-value (KS p-value).
The MLEs and the statistical metrics are calculated using the R software©. Table 6 provides analytical metrics along with the MLEs and their standard errors (SEs). The findings in Table 6 suggest that the WDEx offers better fits than other competitive models and it can be selected as an appropriate model to evaluate the analyzed insurance data.
The fitted PDF, CDF, SF, and probability-probability (P-P) graphs of the WDEx model are displayed in Figure 6, whereas the total time on test (TTT) and WDEx HRF plots for the analyzed data are depicted in Figure 7. The TTT plots confirms that the data has an increasing HRF. We conclude that the WDEx distribution is suitable for modeling the insurance data.
Moreover, the nine estimation approaches mentioned before in Section 5 are adopted to estimate the WDEx parameters. Table 7 records the calculations of the WDEx parameters using such estimation techniques and the empirical values for insurance data.
The P-P graphs and histogram of insurance data with the fitted WDEx density for different estimation techniques are shown in Figure 8. Figure 9 shows the histogram of the data with the fitted WDEx PDF for different estimators.
8.
Conclusions
In this paper, we studied a new extension of the exponential model that exhibits upside-down bathtub, decreasing, decreasing-constant, increasing, and increasing-constant hazard rates. The studied model is called the weighted-exponential (WDEx) distribution. Some basic distributional characteristics are derived. Five risk measures are calculated for the WDEx distribution. Eight classical estimation methods along with the Bayesian estimation are utilized to estimate the WDEx parameters. The simulation results show that the proposed estimators provide good performance. Moreover, the practical applicability of the WDEx distribution is addressed using a real-life insurance data set, showing its superiority fit over fourteen competing exponential models.
Acknowledgments
The authors thanks the respected editors for supporting us in the review process.
Conflict of interest
The authors declare there is no conflict of interest.









 DownLoad:
DownLoad: