We consider cancer chemotherapy as an optimal control problem with the aim to minimize a combination of the tumor volume and side effects over an a priori specified therapy horizon when the tumor consists of a heterogeneous agglomeration of many subpopulations. The mathematical model, which accounts for different growth and apoptosis rates in the presence of cell densities, is a finite-dimensional approximation of a model originally formulated by Lorz et al. [18,19] and Greene et al. [10,11] with a continuum of possible traits. In spite of an arbitrarily high dimension, for this problem singular controls (which correspond to time-varying administration schedules at less than maximum doses) can be computed explicitly in feedback form. Interestingly, these controls have the property to keep the entire tumor population constant. Numerical computations and simulations that explore the optimality of bang-bang and singular controls are given. These point to the optimality of protocols that combine a full dose therapy segment with a period of lower dose drug administration.
1.
Introduction
In 1973, the classic Black-Scholes (BS) model [1] provided an effective method for pricing financial derivatives and greatly simplifying the pricing process. Since then, many scholars have conducted research and promoted the model [2,3]. Asian options, a special type of financial derivative, are appealing due to their unique valuation method, which relies on the mean value of the underlying asset over a set contract period.
However, the BS model may not align with actual financial markets and could fail to provide accurate pricing for complex financial derivatives. In 1968, Mandelbrot and Van Ness [4] proposed a stochastic process: fractional Brownian motion (fBm). If the Hurst parameter H≠12, the fractional Brownian motion is not a semi-martingale, which implies that there must be arbitrage opportunities [5,6]. Bojdecki et al. [7] advanced that sub-fractional Brownian motion (sfBm), which not only possesses similar properties to fractional Brownian motion but also features non-stationary increments with weaker correlations on non-overlapping intervals and a faster decay of covariance, aligns better with financial market dynamics. Based on this, Tubor [8] investigated new properties of sfBm. Xu and Li [9] tackled the valuation conundrum linked to compound options. For further applications of sfBm in financial models, please refer to [10,11,12]. Despite these advancements, the use of sfBm as a stochastic driver may still result in arbitrage opportunities akin to those associated with fBm. Zhang and Xiao [13] showed that the Black-Scholes model driven by fractional Gaussian processes allows for arbitrage opportunities. Since sub-fractional Brownian motion is a more general Gaussian process and is also not a semi-martingale, the application of this model to financial markets necessitates the study of arbitrage possibilities. EI-Nouty and Zili [14] proposed the concept of mixed sub-fractional Brownian motion (msfBm), which lies between Brownian motion and sfBm. The mixed sub-fractional Brownian motion incorporates the semi-martingale condition when H∈(34,1), making this stochastic process more suitable for inclusion in option pricing models [15]. Furthermore, constructing an appropriate portfolio can enable models with the Hurst parameter H∈(0,1) to avoid arbitrage in financial markets [16]. For example, Guo et al. [17] combined the fractal option pricing model with a new intelligent algorithm to predict the implied volatility in financial assets. Cai et al. [18] found the LSE of the drift parameter of mixed sub-fractional O-U process.
The aforementioned studies collectively presupposed fixed short-term interest rates, which do not reflect the dynamic nature of real interest rates that exhibit mean reversion. The Vasicek model [19], a fundamental interest rate model in finance, describes the evolution of interest rates and has become a cornerstone in the analysis and management of interest rate risks. It provides a theoretical framework that aids in making informed financial decisions and developing sophisticated risk management strategies. Ewald et al. [20] priced options for Asian commodity futures contracts by incorporating stochastic convenience yields, stochastic interest rates, and commodity spot prices, and considered the scenario with jumps. More related studies can be found in [21,22,23,24,25] to further understand the importance of stochastic interest rate models in option pricing. Building on these studies, in this paper, a model for pricing geometric average Asian options is formulated under the msfBm regime, while the short rate follows the Vasicek process.
Then, in Section 2, we state the necessary foundational knowledge. In Section 3, we provide the formula for a zero-coupon bond under the msfBm, based on certain assumptions. In Section 4, we give the solution for valuing geometric average Asian options with fixed strike price. In Section 5, we present relevant numerical calculations and an empirical study to further explore the effects of varying parameters on the model.
2.
Preliminaries
The Vasicek model is recognized as a significant model for the short rate that can be combined with other pricing models. The incorporation of mixed sub-fractional Brownian motion can offer more comprehensive risk management solutions. Now, we introduce the relevant knowledge of msfBm, which is covered in [7,14,26].
Definition 2.1. The Gaussian process ξH={ξH(t),t⩾0} defined on a probability space (Ω,F,P) that satisfies the following conditions
(1) ξH(0)=0,
(2) E(ξH(t)⋅ξH(s))=s2H+t2H−12[(s+t)2H+|t−s|2H],
is called a sub-fractional Brownian motion, where H is the Hurst parameter with a value range of (0,1), and BH(t) is a standard Brownian motion when H=12.
Definition 2.2. Let (Ω,F,P) be a probability space. The mixed sub-fractional Brownian motion Mβ,γ,H={Mβ,γ,Ht,t⩾0}is a stochastic process with H∈(0,1), defined by
where B(t) is a Brownian motion and ξH(t) is a sub-fractional Brownian motion. We have
where M_t^{\beta, \gamma, H} is a sfBm when \beta = 0 and \gamma = 1 , M_t^{\beta, \gamma, H} is a standard Brownian motion when \beta = 1 and \gamma = 0 or when \beta = 0 , \gamma = 1 and H = \frac{1}{2} .
Definition 2.3. Asian options are divided into geometric average Asian options and arithmetic average Asian options. Taking fixed strike Asian call options as an example, the payoff is {\left({{J_T} - K} \right)^ + } , where K is the strike price, T is the expiration date, and {J_t} is the average price of the underlying asset over the predetermined interval. In continuous time, the arithmetic average is represented by
the geometric average is represented by
Then, we present some basic assumptions of this paper.
(ⅰ) Short selling is allowed without penalty; there are no taxes and friction; stocks do not pay dividends; investors borrow and lend at the risk-free rate.
(ⅱ) The stock price S\left(t \right) satisfies the mixed sub-fractional Brownian motion of risk neutrality, given by
where r\left(t \right) is a short rate and satisfies the following msfBm-Vasicek model
where {\sigma _{{S_1}}} , {\sigma _{{S_2}}} , {\sigma _{{r_1}}} , and {\sigma _{{r_2}}} are constants, {B^S}\left(t \right) , \xi _H^S\left(t \right) , {B^r}\left(t \right) , and \xi _H^r\left(t \right) are independent of each other.
3.
Pricing formula for zero-coupon bond
A zero-coupon bond is issued at a price below its face value and does not pay any interest during the period. Upon maturity at time T , investors are entitled to receive a cash return equivalent to \$ 1. The price of such a bond is influenced by the passage of time and the variability in interest rates. We denote the price of a zero-coupon bond at time t , maturing at time T , as P\left({r, t; T} \right) .
Theorem 3.1. In the mixed sub-fractional Vasicek process, the price of a zero-coupon bond with maturity T at time t \in \left[{0, T} \right] is given by
where
Proof. By using the risk hedging formula and Itô formula [19], select two zero-coupon bonds with different maturities, denoted as {P_1} = {P_1}\left({r, t; {T_1}} \right) and {P_2} = {P_2}\left({r, t; {T_2}} \right) , to hedge the risks. Now consider a portfolio \Pi consisting of one unit of {P_1} and \Delta units of {P_2} , we can obtain
The change in the portfolio over the time interval \left({t, t + {\text{d}}t} \right) is given by
where {\left({{\text{d}}r} \right)^2} = \left({\sigma _{{r_1}}^2 + 2H{t^{2H - 1}}\left({2 - {2^{2H - 1}}} \right)\sigma _{{r_2}}^2} \right){\text{d}}t + o\left({{\text{d}}t} \right).
Let \Delta = \frac{{\partial {P_1}/\partial r}}{{\partial {P_2}/\partial r}} , Eq (3.2) becomes
Furthermore, since the investment portfolio is risk-free, that is, E\left({{\text{d}}\Pi } \right) = r\left(t \right)\Pi {\text{d}}t , we have
Then, we can obtain
Thus, the zero-coupon bond P\left({r, t; T} \right) satisfies the following partial differential equation given by
Given that A\left({T, T} \right) = 0 and B\left({T, T} \right) = 0 , it is not difficult to find a solution for the price of a zero-coupon bond at time t of the following form
Substituting Eq (3.6) into Eq (3.5), we can derive
After simplification, we have
Then, we can obtain
Proof is completed.
4.
Valuation equation for geometric average Asian options
In this section, examine a mixed sub-fractional version of the BS model, i.e., a simple financial market consisting of zero-coupon bonds, underlying assets, and options on underlying assets.
Theorem 4.1. The value of geometric average Asian call options with fixed strike price is denoted as V = V\left({S, J, r, t} \right) . Based on assumptions (2.1) and (2.2), the partial differential equation and boundary conditions are given by
Proof. Considering that the portfolio \Pi consists of one unit option V\left({S, J, r, t} \right) , {\Delta _{1t}} units of underlying assets and {\Delta _{2t}} units of zero-coupon bonds P\left({r, t; T} \right) , the value of the portfolio at time t is given by
Choosing the appropriate {\Delta _{1t}} and {\Delta _{2t}} makes the portfolio risk-free in \left({t, t + {\text{d}}t} \right) , we can obtain
Let {\Delta _{1t}} = \frac{{\partial V}}{{\partial S}} and {\Delta _{2t}} = \frac{{\partial V/\partial r}}{{\partial P/\partial r}} , using the principle of no arbitrage, we have
we can calculate that
Proof is completed.
Theorem 4.2. Assuming that the stock price satisfies Eq (2.1), and the interest rate satisfies Eq (2.2), the price of the geometric average Asian call option at time t \in \left[{0, T} \right] with strike price K and maturity date T is given by
where
Proof. To simplify the variable-coefficient equation with three variables down to one with two variables, we perform a variable substitution. Thus, let
Sometimes, we denote P\left({r, t; T} \right) as P to simplify notation. Then, through calculation, we have
Substituting Eq (4.5) into Eq (4.1) and organizing it, we can obtain
By integrating Eqs (4.4) and (3.5), we can derive
where
By substituting the deformation of Eq (4.4) into Eq (4.6), the equation can be transformed into
where {\beta _2}\left(t \right) = - A\left({t, T} \right) - rB\left({t, T} \right).
To simplify Eq (4.7), we can make the following variable substitution
It can be determined that
Substituting the above results into Eq (4.7), we find that
where {\beta _3}\left(t \right) = {\left({\frac{{T - t}}{T}} \right)^2}\beta _1^2\left(t \right) and {\beta _4}\left(t \right) = \frac{1}{T}{\beta _2}\left(t \right) - \frac{{T - t}}{T}\beta _1^2\left(t \right) .
The last time using variable substitution to simplify Eq (4.10) into a heat conduction equation, let
It can be deduced that
Then, Eq (4.10) can be converted to
According to the heat conduction theory, the solution of this equation can be expressed as
where
Substituting Eq (4.8) into Eq (4.14), we can obtain
where
Combining Eqs (4.4) and (4.15), it follows that
where
Proof is completed.
Theorem 4.3. The call-put parity relationship for geometric average Asian options with fixed strike price is given by
where p\left({S, J, r, t} \right) is the price of geometric average Asian put options and
Proof. Let
According to Theorem 4.1, W\left({S, J, r, t} \right) satisfies the following definite solution problem
Making the following variable substitutions
Substituting Eq (4.17) into Eq (4.16), we have
where
Let
By inserting Eq (4.19) into Eq (4.18), this yields
where
By letting
then, we can obtain
and
To solve Eq (4.22), we select appropriate a\left(t \right) and b\left(t \right) so that
Thus, we can derive
where
Substituting Eqs (4.21) and (4.23) into Eq (4.17), we have
Proof is completed.
Corollary 4.1. Let the price of the arithmetic average Asian call options with fixed strike price be denoted as \hat V\left({S, J, r, t} \right) , and let the price of the put options be \hat p\left({S, J, r, t} \right) . The put-call parity formula for arithmetic average Asian options is
where
Proof. Denoting
According to Theorem 4.1 and Theorem 4.3, we can similarly derive
By employing a change of variables, we define
Substituting Eq (4.25) into Eq (4.24), we can obtain
where
Let
By substituting Eq (4.27) into Eq (4.26), we can deduce
Let
then, we have
and
By combining Eqs (4.28) and (4.30), we can compare the coefficients to obtain
Solving the aforementioned system of equations yields
Finally, we can derive
5.
Numerical analysis
In this section, we provide specific values for each parameter to conduct the following numerical calculations.
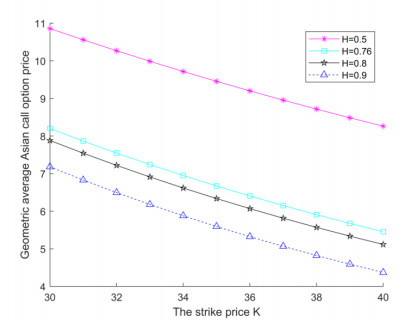
From Figure 1, it can be observed that when H = 0.5 , the msfBm simplifies to a geometric Brownian motion. At this point, the price of geometric average Asian call options peaks, whereas it reaches its minimum at H = 0.9 . An increment in the Hurst index is indicative of the manifestation of long-range correlations in the asset price, suggesting a persistent price trend. In specific situations, the expected small fluctuations in the asset price in the market will lead to a decrease in the option value. Figure 2 indicates that as the short rate rises, the price of the options gradually increases. This is because an increase in the interest rate could potentially raise the payoff of the options at maturity, thereby enhancing the holding value of the options. Figure 3 shows that the valuation of the options is positively influenced by a rise in the initial stock price. From Figure 4, the price of the options increases with the extension of the expiration date. As the remaining time for the option contract increases, it gives the holders more time to wait for a potential rise in the stock price. Therefore, the possibility of the investors making a profit is greater, and the price of the options will also increase accordingly. Conversely, it diminishes with an upsurge in the strike price. As the strike price increases, buyers have to pay a higher price to exercise the options, which compresses the profit margin and thus reduces the value of the options. Figure 5 illustrates that the pricing of options under the current model closely mirrors that of the sub-fractional Vasicek model. Notably, the pricing discrepancy for options between our model and Vasicek model widens initially and then narrows, and the pricing gap between our model and BS model exhibits a consistent upward trend.
According to Tables 1 and 2, it can be observed that the lowest pricing for geometric average Asian call options is associated with the BS model. In the case of a brief expiration period, the price of the options under the sfBm-Vasicek model marginally surpasses that of our model, especially with an increasing stock price, and there is minimal variation between the two models. For longer expiration dates, the option price under our model exceeds that under the sfBm-Vasicek model, with the price difference becoming more pronounced. Moreover, the price of options under our model and the Vasicek model are getting closer. Overall, our model demonstrates a degree of rationality and is deemed suitable for developing option pricing models in financial markets.
We select copper options for three-month futures as the sample, with a cutoff time of October 2006, and the data are sourced from the London Metal Exchange. Since all trading products on the LME are priced in US dollars, we adopt the interest rate of one-year US Treasury bonds.
We calculate the value of H using the R/S method. Define a return sequence \left\{ {{R_t}, {R_t} = \frac{{\ln {P_{t + 1}}}}{{\ln {P_t}}}} \right\} of length N and divide it into A consecutive sub-intervals of length n . Label each sub-interval as {I_a}, a = 1, \cdots, A. Thus, each point in {I_a} can be represented as
For each sub-interval {I_a} of length n , calculate its mean as
The cumulative mean deviation {X_{k, a}} for a single sub-interval is calculated as
The sum of the cumulative mean deviation sequence \left\{ {{X_{1, a}}, {X_{2, a}}, \cdots {X_{n, a}}} \right\} for a single sub-interval is zero. The range of an individual sub-interval is defined as
Subsequently, the standard deviation {S_{{I_a}}} for each sub-interval is given by
Therefore, for the partition length n , we can compute the average rescaled range for A sub-intervals as
Repeat the above calculation process for different partition lengths (i.e., different time scales) n to obtain multiple average rescaled range values. There is a linear relationship between \log \left({\frac{R}{S}} \right) and \log \left(n \right) [27]
Finally, a double logarithmic regression is performed on n and R/S, and the slope is the parameter of long-range correlations, that is, the Hurst index. Therefore, we can obtain H = 0.6 .
From Table 3, it is evident that the RMSE of our model is the smallest, indicating that the option price derived from our model is the closest to the market price. Furthermore, the price from our model is also quite close to that of the sfBm-Vasicek model. This suggests that it is essential to include factors such as the long-range correlations of the underlying asset and stochastic interest rate in the option pricing model, as they significantly influence the option price.
6.
Conclusions
Geometric average Asian options are important exotic options. The mixed sub-fractional Brownian motion, chosen as a stochastic process, is capable of more accurately depicting the characteristics of long-range correlations. Since the Vasicek model is a very classical model, it is combined with the msfBm to simultaneously address the pricing issue. By using partial differential equations and multiple variable substitution methods, the valuation equations for the model are deduced. Finally, numerical computations are utilized to assess the effects of diverse parameters. The efficacy of our model is demonstrated through a comparative analysis of the pricing discrepancies for options between different models, which provides a reference for option pricing theory and practice.
Author contributions
Xinyi Wang: Conceptualization, Methodology, Writing—original draft, Writing—review & editing, Funding acquisition; Chunyu Wang: Writing—review & editing. All authors have read and approved the final version of the manuscript for publication.
Acknowledgments
This work was supported by the General Project of Philosophy and Social Science Research in Colleges and Universities of Jiangsu Province (2023SJYB1714).
Conflict of interest
The authors declare no conflicts of interest.









 DownLoad:
DownLoad:






