1.
Introduction
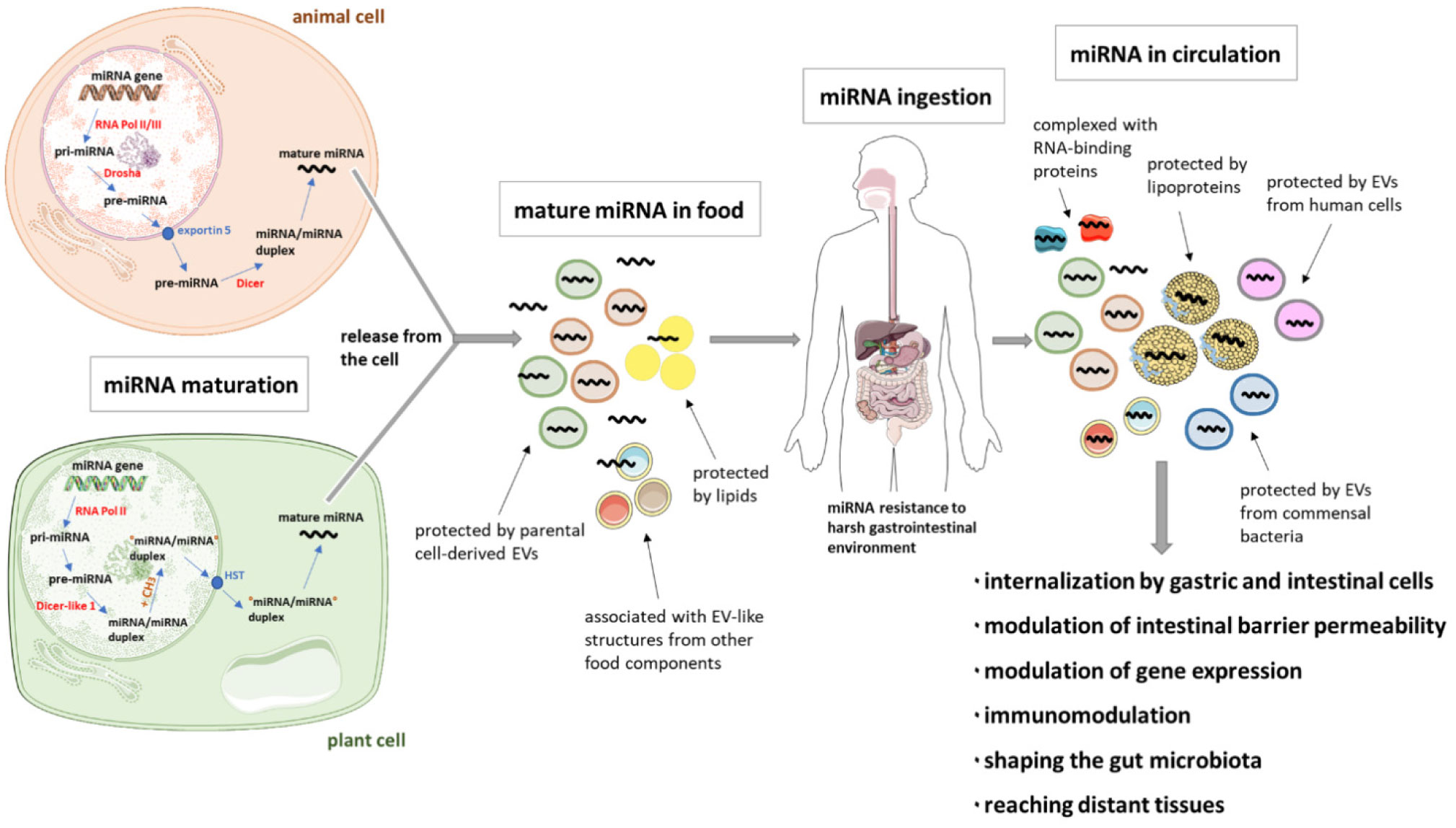
MicroRNAs (miRNAs) are short single-stranded, non-coding RNA molecules, 19–25 nucleotides in length, involved in gene regulation by mediating post-transcriptional gene silencing in eukaryotes [1],[2]. The first report of this phenomenon concerns a short RNA molecule complementary to the 3′ untranslated region (UTR) of mRNA for the lin-4 gene in Caenorhabditis elegans [3]. Depending on the organism, the base complementarity in the miRNA-mRNA can be precise or nearly precise (in plants), as well as imprecise (most animal miRNAs) [4],[5]. The canonical pathway of miRNA biogenesis in mammalian cells begins in the nucleus by the RNA polymerase II or III-mediated transcription of DNA sequences into a primary miRNA (pri-miRNA) with a hairpin-like structure. Pre-miRNA formed in the next step under the activity of the Drosha enzyme is exported to the cytoplasm by exportin 5, where it is cut by the Dicer enzyme to the miRNA duplex [6] (Figure 1). Finally, one of the strands of mature miRNA duplex can be loaded into the Argonaute (Ago) proteins to form a miRNA-induced silencing complex (miRISC or RISC) [7]. The general function of miRNAs is to inhibit translation and destabilize target mRNAs, e.g., through their deadenylation [8]. However, recent findings of a high-throughput analysis strongly suggest that miRNAs could also upregulate mRNA translation [9]. On the other hand, extracellularly secreted miRNAs mediate cell-to-cell communication [7],[10]. Interestingly, these molecules are the most abundant nucleic acids in extracellular fluid as well as in cerebrospinal fluid in humans [11]. miRNA present in various biological fluids may function as diagnostic markers of various pathological conditions due to the possibility of reflecting molecular changes in cells and are also proposed as potential therapeutics in RNA-based therapy [12]. However, the crossing of kingdom boundaries by miRNA is still under discussion.
2.
How miRNA is protected in an extracellular environment
It is well established that many miRNAs circulate in the extracellular environment and, moreover, their export from the cell may be an active, ATP-dependent transport process [13]. Extracellular miRNAs found in biological fluids can be associated with RNA-binding proteins, like Argonaute proteins, especially Ago2 [14], or loaded into extracellular vesicles (EVs), such as microvesicles or exosomes [15],[16]. Notably, Ago with mature single-stranded miRNA participates in forming the RISC, a crucial mediator of RNA interference inside the cell [17],[18]. The importance of Ago proteins is enormous; besides miRNAs, they also interact with other non-coding RNAs, like small interfering RNAs (siRNAs) and are also involved in the maturation of both molecules [19],[20]. Other RNA-binding proteins, especially nucleophosmin 1 (NPM1), are also described as factors that protect mammalian miRNA from extracellular degradation [13] (Figure 1).
Endogenous circulating miRNAs may also be transported in extracellular fluids by high-density lipoproteins (HDL) [21], which mediate intercellular communication by delivering specific miRNAs to recipient cells [22]. Moreover, some regulatory functions of HDL arise from its delivery of miRNAs to specific cells, as shown in the example of the suppression of intercellular adhesion molecule 1 (ICAM-1) expression through the transfer of miR-223 to endothelial cells after incubation with HDL [23]. It has also been suggested that low-density lipoprotein (LDL) molecules may be involved in the transport of extracellular miRNAs [24] (Figure 1).
As mentioned above, EVs can be intercellular communication vectors, transferring a variety of molecules to targeted cells, including miRNAs [25]. Increasingly, miRNAs encapsulated in EVs are described as a novel drug delivery system in miRNA-based therapy [26], especially due to their immunomodulatory properties [27] and/or ability to cross biological barriers [28].
3.
Food-derived miRNAs—animal versus plant
So far, the immunomodulatory effects of food have been well documented [29]. Despite the fact that foods contain many different minerals, nutrients and trace elements, it is also believed that they provide a wide variety of miRNAs [30]. This leads to the suggestion that, in addition to the traditional endogenous regulation of genes, food-borne miRNAs can influence the expression of genes of other species [31]. Ingested miRNAs, similar to those synthesized endogenously, may have an impact on various physiological and pathological conditions, which is also reflected in the different miRNA profiles in sera depending on the nutritional status [32]. The necessity to develop research on the relations between nutrition and the human epigenome has increasingly been emphasized, which concerns, inter alia, modifications in non-coding RNAs [33]. Given the ambiguous evidence regarding the bioavailability of short non-coding RNAs derived from food and their role in the cross-kingdom information transfer, the question arises: “Can we eat gene regulators?” [34].
3.1. Differences in structure and biogenesis
Combinations of different features at the molecular level indicate significant differences between animal and plant miRNAs [35]. One of the fundamental differences between these two types of miRNAs is that the conversion of animal pre-miRNA into a mature miRNA takes place in the cytoplasm, while the maturation of plant miRNA occurs within the nucleus [36]. Moreover, in the process of plant miRNA biogenesis, its methylation plays a key role in protecting it from degradation, while it is not present in animal miRNAs [37] (Figure 1). The impact of 2′-O-methylation at the 3′ terminal ribose of plant miRNAs on increasing stability in unfavorable environments has also been highlighted [38],[39], as it confers, inter alia, protection against degrading uridylation and truncation processes [40]. Animal miRNAs predominantly bind motifs located in the 3′UTR of target mRNAs, inhibiting protein synthesis, while plant miRNAs mainly cleave the coding region of the target mRNA, promoting its degradation [41],[42].
There are also indications that some of the miRNA families (like the miR-854 family) are expressed in both animal and plant kingdoms, which suggests a common origin of these basal transcription regulators and mediators of RNA interference [43].
3.2. The presence of miRNA in the intake food
Nowadays, miRNAs have already been identified in many foods consumed daily, and research into them could open up new avenues for nutritional implications and other perspectives [44].
3.2.1. Human breast milk
It is well known that human breast milk provides many immunologically active compounds [45]. Especially in the first six months of lactation, it contains many different miRNAs, principally immune-related miRNAs [46], associated with both innate immune responses (like miR-125b, a negative regulator of tumor necrosis factor-α (TNF-α) or miR-146b) and T- and B-cell-mediated immune responses (like miR-181a and miR-181b, regulators of CD4+ T-cell selection and B-cell differentiation or miR-155, a regulator of, among others, T- and B-cell maturation) [47]. Interestingly, granulocyte-regulating miR-223 was present in breast milk, but at a lower level than in sera [47]. Moreover, immune-related pre-miRNAs are also abundant in human milk [48]. While some studies described similar levels of miRNAs in colostrum and mature milk [49], some show lower miRNA levels in mature milk compared to transition milk (collected 4-5 days after delivery) [50]. However, it has been unequivocally indicated that infant formulas, compared to breast milk, have a much lower content of the most common immune-related dairy miRNAs (miR-125b-5p, miR-146b-5p, miR-148a-3p, miR-155-5p, miR-181a-5p, miR-200a-3p) [49],[50].
The lipid fraction of human breast milk (milk fat globules) is described as rich in miRNAs (like miR-148a, miR-200c, or miR-146b) [51],[52] and allows the maintenance of miRNA stability [51],[53]. The content of individual miRNAs and other non-coding RNAs in milk depends on the mother's diet [54]. Further, an abundance of miRNAs in fat globules depends on a high-fat diet [51]. Moreover, the mother's health condition, level of exercise, or nutrition can affect the miRNA profile in milk [55]. In addition to fat globules, it has been recognized that EVs, such as exosomes or exosome-like vesicles, are essential to maintain the stability of miRNAs in milk [47],[48],[56]–[59], especially due to their resilient lipid bilayer membrane [60]. Interestingly, prenatal exposure to marine pollutants has no influence on the abundance of individual milk EV-miRNAs [61]. It is worth noting that human milk miRNAs may originate from the mammary gland epithelial cells [62] and their levels are higher in the milk cellular fraction than in the lipid fraction [52].
3.2.2. Milk and dairy products
In 2010, a large number of miRNAs in cow's milk (245 miRNAs in raw milk) and their fluctuation depending on the lactation period were described for the first time [63]. Development-related (miR-27b, miR-34a, miR-130a) and immune-related (miR-15b, miR-27b, miR-106b, miR-155, miR-223) miRNAs are especially abundant in bovine milk, and almost two times as many miRNA types were detected in colostrum, compared to mature milk [64]. Also in this case, raw cow's milk contains significantly more miRNAs than infant formulas [63]. The role of EVs in carrying and transmitting miRNAs in bovine milk has been clearly emphasized [65],[66]. Additionally, a comparative analysis showed that miRNA enriched in milk fat could serve as an indicator of the miRNA profile of mammary gland tissue [67]. However, many miRNAs (like miR-27b or miR-106b) present in milk can also be found in mammary gland samples [68],[69]. Interestingly, numerous miRNAs have also been found in commercially processed dairy products, like homogenized heat-treated skimmed milk, heavy whipping cream, or sweet cream buttermilk [70]. Moreover, it has been found that ultra-high-temperature treatment (UHT), but not the pasteurization process, dramatically reduces the content of milk miRNA [71].
Some miRNAs (e.g., miR-22-3p, miR-26a, miR-30a-5p or miR-148a) present in bovine milk and colostrum, are also abundant in caprine milk and colostrum, and in both cases the fact that they are carried in EVs is emphasized [72],[73]. While mRNA in bovine milk whey is present in EVs, miRNA derived from whey has been detected in both EVs and their supernatants and may differ in content [74]. In several studies on milk miRNA, recently summarized by Benmoussa and Provost [75], miR-148a-3p and members of the let-7 family (let-7a, let-7b and let-7f especially) were consistently reported among 10 of the most highly enriched milk miRNA molecules. Moreover, the pattern of some of these immune-related miRNAs is highly conserved, because in addition to the mentioned animals, they are present in human milk [72]. Likewise, donkey milk contains various EV-associated immune-related miRNAs [73].
It has also been suggested that cow's milk EVs-derived miRNAs may be potentially harmful to humans due to the association between miRNAs and numerous diseases [76].
Several miRNAs (miR-30c-5p, miR-92-3p, miR-215-5p and miR-2188) are also present in both chicken egg albumin and yolk, and the level of each miRNA is higher in the yolk [77]. Additionally, the presence of miR-16, miR-21, miR-155 and miR-168 was shown in boiled eggs [78].
3.2.3. Meat and meat products
The presence of various miRNAs in meats, meat products and products of animal origin is also undeniable. For example, miR-16, miR-21 (highest levels) and miR-155 are abundant in samples derived from, e.g., raw and roasted beef and pork, salami, poultry mortadella, liverwurst, as well as smoked salmon. Interestingly, they also contain plant-specific miR-168, which may potentially be related with an animal diet. Moreover, food processing, like roasting or cooking, did not significantly affect the stability of miRNAs [78]. The content of specific miRNAs in beef and other bovine tissue-derived products depends on tissue origin rather than food preparation method. For example, the most prominent miRNAs in cooked sirloin are miR-10b-5p, and also muscle-specific miR-1 and miR-206; miR-1, miR-99a-5p and miR-100-5p were dominant in dried heart extracts, whereas especially miR-10b-5p, but also miR-143-3p and miR-146b-5p were present in dried adrenal extracts [79].
3.2.4. Honey
In 2017, the detection of plant RNAs, including miRNAs (miR-156a, miR-159c, miR-162a, miR-171a, miR-395a, miR-396c, miR-482b, miR-858 and miR-2118a), was described in honey for the first time [80]. In another study, next-generation sequencing revealed the presence of highly conserved plant miR-156a, miR-162a and miR-396a in both monofloral and polyfloral honeys [81]. Among various biologically active particles, miR-4057 is considered responsible for the anti-inflammatory properties of honey [82]. Many flower miRNAs are also detected in honeybee midguts [83], confirming the transmission of these particles between kingdoms.
3.2.5. Plants
The expression of various non-coding RNA molecules, including miRNAs, has been studied in a wide variety of edible plants and plant products due to their wide availability. For this reason, Table 1 shows specific examples of commonly occurring miRNAs in selected comestible plants. Similar to animal miRNA, plant miRNA may be protected by exosome-like nanoparticles [84],[85]. Interestingly, the presence of some plant miRNAs in human breast milk exosomes was suggested following an in silico study [86].
3.3. Stability and bioavailability of miRNAs after oral administration
Orally delivered miRNA must overcome many unfavorable conditions throughout the gastrointestinal tract, including salivary RNases, low pH and digestive enzymes in the stomach, pancreatic RNases and degrading enzymes in the intestines, as well as peristaltic activity and microbial enzymes in the ileum and colon [92],[93] (Figure 1). The possibility of a transfer of miRNAs from ingested food to the blood, tissues and organs remains inconclusive [94]. Despite the examples of animal and plant miRNA described below, miRNA mimics, experimentally loaded into plant-derived nanovesicles or milk-derived EVs, have also been detected in various organs after per os administration to mice [95],[96]. Interestingly, the biodistribution of EVs with their cargo in a living organism seems to depend on the donor cell source, route of administration and dose of administered EVs [97]. However, the sequence homology of miRNAs between miRNA-source species and the species of the consumer is the leading limitation in studies on the horizontal inter-species or cross-kingdom transfer of miRNAs.
3.3.1. Animal miRNA
In healthy humans enrolled in a proof-of-principle study evaluating the impact of diet type on miRNA detectability, a short-term (5–7 days), meat-rich diet failed to significantly influence the miRNA levels, both in the feces and blood, despite the relatively high content of various miRNAs in consumed food [78]. However, some meat-borne miRNAs, like miR-154c derived from edible parts of beef (bovine adipose and muscle tissues), can survive after cooking and the in vitro digestion process, albeit at a lower concentration than before [98]. In contrast, milk-derived miR-29b and miR-200c are largely absorbed by humans into the bloodstream—a dose-dependent increase of miRNA levels was found in plasma, and, moreover, these studies showed an increase in the concentration of miRNAs in human peripheral blood mononuclear cells (PBMCs) after milk feeding [99]. Additionally, some preliminary observations suggest that miRNAs from boiled eggs can also reach the circulating plasma in humans several hours after egg consumption [100].
Studies with mice revealed unique tissue distribution patterns of milk miRNAs administered by different routes [95]. Some cow milk miRNAs can reach distant mouse organs after oral administration. Interestingly, miR-26a-5p, miR-146b-5p and miR-148a-3p levels decreased in the gastrointestinal tract during transit, and after 1 h or 3 h of exposure significantly increased in the liver and brain, respectively. However, the changes in plasma and muscle miRNA enrichment were not statistically significant [101]. After per os delivery, milk miR-320-3p complexed with lipidic aminoglycoside derivatives as a vehicle was enriched in the highest levels in rats' gastric cells, and was also present in the blood and liver but to a lesser extent [102]. Both bovine and porcine milk EVs containing specific miRNAs may alter serum miRNA profiles in piglets after milk feeding [103]. Transfer of milk EVs with their cargo via the blood-brain-barrier (BBB) was also noted in mice [104].
Moreover, EV-encapsulated, bovine milk-derived miR-148a-3p can survive artificial digestive processes (oral and gastric phases) [101]. Other studies revealed the resistance of immune-related miR-21, miR-25, miR-148a and miR-182-5p present in bovine milk EVs to artificial digestion juices, such as saliva as well as gastric, pancreatic and bile juices [105]. Also, human milk-derived EVs containing miRNAs, obtained during various lactation periods and from mothers who delivered preterm and term infants, can survive gastric and pancreatic digestion processes, mimicking the infant digestive environment in vitro [106],[107].
3.3.2. Plant miRNA
Based on data from the human plasma sequence database, the presence of numerous miRNAs derived from comestible plants was found, and miR-2910 seems to be particularly conserved in vegetables and fruits [108]. A positive correlation has been reported between a diet rich in rice and high levels of miR-168a in sera [109], as well as between drinking watermelon juice and the effective detection of several plant miRNAs in sera [91]. For example, the kinetics of miR-167a plasma level changes were time-dependent, while levels of miR-172a or miR-528, after an initial increase, decreased in the blood several hours after drinking the juice [91]. On the other hand, contrary to a short-term meat diet, a week-long vegetarian diet is associated with a pronounced increase in miR-168 levels in the feces, as well as in intestinal mucosa, but not in the sera [78]. Similar results were obtained for plant-specific miR-167a and miR-824—no changes in the presence of these molecules in plasma were observed after feeding with broccoli sprouts [99].
In mice gavaged with total RNA extract from cabbage (Brassica oleracea), the presence of different plant miRNAs, especially miR-172—the most abundant in this plant, was detected in the stomach, intestine and feces between 2 and 72 h after feeding. Moreover, miR-172 can reach other parts of the mouse body through the gastrointestinal tract—its presence has been detected up to 72 hours after oral administration in serum and the spleen [87]. Similarly, maize-derived miRNAs (like miR-164a-5p, miR-167e-5p, miR-168a-5p or miR-319a-3p) have been detected in the blood and different organs (especially the heart, brain, lungs and kidneys) of pigs after 7 days of eating a fresh corn diet. These miRNAs may be packed into EVs in the digestive tract to allow them to survive in the bloodstream and in the presence of nucleases [90]. In turn, a honeysuckle diet and herbal diets promote miR-2911 detection in the body fluids of mice, including sera and urine, suggesting kidney action in filtering the dietary miRNAs [110],[111].
Despite the above-described evidence for the possibility of plant miRNA absorption after ingestion, some evidence is still inconclusive, such as studies on pigtailed macaques that consumed food rich in plant miR-168 [112]. Interestingly, horizontal cross-kingdom transfer of dietary plant miRNA has also been described as ineffective in studies involving humans, mice and bees consuming plant miRNA-rich foods, for which changes in plasma and tissue miRNA levels were either negligible or not observed [113]. In other studies, after ingestion of miRNA-rich pollen (with a particularly high level of miR-156a), the robust increase of plant miRNAs was found in the honeybee midgut tissues, but not in other distant tissues, indicating that the intestinal epithelium is a barrier to further miRNA biodistribution [114]. In addition, in mice gavaged with miRNAs isolated from corn kernels (miR-156a, miR-164a and miR-167a) or fed a diet supplemented with 3% corn powder for two weeks, no significant changes in miRNA levels in the blood, fecal, cecal and liver samples were observed [115].
On the other hand, some in vitro studies examined plant miRNA resistance to various stages of digestion, allowing for further miRNA translocation and reaching other organs beyond the gastrointestinal tract. It has recently been reported that the digestion of synthesized typical plant miRNAs, such as miR-157a (peanut), miR-159 (cabbage), miR-160 (soybean), miR-168a (rice), miR-172a (tomato) and miR-894 (sorghum), begins in the mouth—extensive miRNA degradation has been observed as a result of incubation with human saliva [116]. Conversely, both food component-derived EV-like nanoparticles and microcapsules, as well as 3′ end modifications, like the addition of complementary DNA and 2′-O-methylation, protect miRNAs from salivary RNases [116]. Similarly, these synthesized plant miRNAs can survive in unfavorable stomach and intestine environments, through a mixture of food ingredients that form protective EV-like structures or 2′-O-methyl modification at the 3′ end, respectively [39]. Also, rice- and soybean-derived miRNAs have been shown to survive during a simulated early digestion process, which, according to the authors, suggests their possible bioavailability in further parts of the gastrointestinal tract [117]. Conversely, low bioavailability of the above-described maize-derived miRNAs may result from their extensive degradation after digestion [115]. Similarly, most of the artichoke-derived miRNAs were degraded in an in vitro artificial digestion process, and, additionally, the remaining, non-degraded miRNAs were not uptaken by Caco-2 intestinal cells [85].
Some studies revealed that food processing, cooking and storage do not abolish the presence of plant miRNA in products [117], while others indicate reduced miRNA abundance after cooking [85]. Moreover, processes such as the steaming, drying and puffing of corn caused a decrease in miRNA concentrations to a certain extent [90].
Interestingly, the stability of miRNAs may be increased by forming a secondary structure [39]. Furthermore, the observations of Qin et al. [116] suggest that the binding of complementary DNA oligomer at the 3′ end of plant miRNA enhanced its resistance to salivary digestion. Accordingly, one can speculate that DNA oligomers resulting from food-derived DNA digestion may play a role in increasing miRNA stability. The role of DNA in miRNA protection was also suggested in other studies on the immune suppressive effects of miR-150 [118]. Obviously, miRNA's stability greatly increases after encapsulation into membranous vesicles or vesicle-like nanoparticles as mentioned above. Supposedly, EVs derived from the acceptor cells may also play a role in miRNA protection in target tissues [118]. However, these aspects require further detailed investigation.
4.
The fate of consumed miRNAs in the digestive tract and beyond. An immunomodulatory effect
Various miRNAs present in fruits, vegetables, meat, and milk, as well as obtained from different cells and administered orally, can perform many biological functions and seem to interact, for example, with the intestinal barrier, gut bacteria, cancer and immune cells [119],[120]. The possible fates of these short non-coding RNAs that managed to survive the unfavorable environment of the gastrointestinal tract are discussed below and summarized in Figure 1.
4.1. Internalization of miRNA by gastric and intestinal cells (Figure 2)
The intestinal epithelium consists of many types of cells, simultaneously forming a protective barrier as well as a place of communication between the inside of the body and the molecules entering it from the outside [121]. A scenario is suggested, in which exogenous miRNAs, e.g., from the diet, may enter the circulation due to the “leaky gut” [122]. However, extracellular miRNA absorption by cells in the gastrointestinal tract seems to be crucial for their possible further effects after oral delivery. Recent studies revealed that food-derived miRNAs can be absorbed by the SID-1 transmembrane family member 1 (SIDT1) protein located in the plasma membrane of pit cells in the stomach epithelium. In turn, pit cells package the engulfed miRNA into the EVs and secrete it into the circulatory system [123]. The potential of miRNA protection by EVs and their special role in future oral miRNA administration has been clearly emphasized [124]. Different intestinal (FHs-74), colonic (Caco-2 and HT-29) and hepatic (Hep-G2) cells can incorporate bovine milk-derived EVs carrying miRNAs [101],[105]. Recently, it has been shown that undifferentiated Caco-2 cells uptake approximately 5% of applied milk EVs, while differentiated Caco-2 cells incorporate over 13% of the initial EVs [125]. Furthermore, Caco-2 cells can also uptake plant miRNAs (miR-159, miR-160 and miR-168a) [39]. However, human breast milk-derived miRNAs encapsulated into EVs may be time-dependently uptaken by human intestinal epithelial crypt-like cells (HIEC) and 10% of these EVs were shown to localize to the nucleus [106]. Similarly, after oral gavage, milk-derived miR-320-3p accumulated within the nucleoli in rats' gastric cells [102]. Bovine-milk derived EVs containing miRNAs can also be incorporated by human macrophages (differentiated THP-1 cells) [74]. Intestinal stem cells and macrophages in the small and large intestines can also incorporate edible plant-derived nanovesicles containing plant miRNAs after oral administration to mice [84]. Trans-epithelial transport of bovine milk-derived EVs carrying miRNAs via the Caco-2 monolayer was also confirmed, suggesting the possibility of miRNA crossing the intestinal barrier [105].
Possible mechanisms for the internalization of EVs containing miRNAs by recipient cells are phagocytosis or membrane fusion [126]. Other mentioned endocytic EV uptake pathways include macropinocytosis, clathrin-dependent endocytosis or caveolae-dependent endocytosis [127]. In the direct fusion pathway, EVs' cargo (including nucleic acids) is directly released to the cytoplasm. However, in endocytic pathways, which also include micropinocytosis or receptor-mediated endocytosis, EVs with their cargo may be transported to endosomes or lysosomes and then follow an “endosomal escape” into the cytoplasm [128]. Further, the EV's resistance to acid/enzymatic environment inside phagolysosomes has also been described, suggesting new possible mechanisms of low-dose-over-time intracellular miRNA release that require further elucidation [129]. In addition, transcytosis of exogenous short RNAs packaged in EVs through the intestinal cells into the circulation has also been suggested [122], and transcytosis of probiotic Bacillus subtilis-derived EVs by the Caco-2 cell monolayer was recently described in vitro [130]. This may indicate a possible transcytosis-mediated migration of miRNAs packed in EVs from the intestinal lumen to the circulation.
Studies on bovine milk-derived EVs indicate involvement of the “neonatal” Fc receptor (FcRn) present in the human gastrointestinal tract in the EVs' absorption by the gut epithelium, and bovine IgG presence in or on milk EVs may allow them to be transported into the blood [131]. The presence of specific surface glycoproteins on both EVs and cells is crucial in the process of vesicle endocytosis [132],[133]. The role of EV surface proteins, such as integrins, in their pharmacokinetics after administration to mice has also been highlighted [134]. However, the exact mechanisms of the capture of extracellular miRNAs, including those associated with proteins and lipids, by the acceptor cell in the gastrointestinal tract and their entry into the cell have yet to be elucidated.
In the next step, it is important to confirm if miRNAs transmitted from the gastrointestinal tract to the circulation could be taken up by other types of human cells to induce biological effects. Along these lines, it has been suggested that the presence of scavenger receptor class B type 1 (SR-B1) in endothelial cells may be engaged in the uptake of orally delivered miRNA associated with HDL and their delivery from plasma to recipient cells [22],[23],[122].
4.2. Modulation of gene expression
Studies involving healthy individuals with different dietary habits (vegans, vegetarians and omnivores) revealed that total miRNA levels in both stool and serum samples are diet-dependent [135]. This suggests that diet-derived miRNAs may indirectly influence the process of RNA interference mediated by human miRNAs. On the other hand, other studies suggested that some miRNAs, e.g., from milk, may directly affect human gene expression [136]. After milk consumption by healthy humans, PBMC's analysis showed an increase in gene expression for runt-related transcription factor 2 (RUNX2), which is positively regulated by miR-29b and is abundant in milk [99]. Incubation of human colon epithelial cells with human milk-derived miRNAs led to upregulation of miR-148a that correlated with decreased expression of the targeted DNA methyltransferase 1 gene [137]. However, other studies revealed that the effect of milk-derived miRNAs on gene expression was slight and insignificant [101].
The role of orally-administered, plant-derived miRNA in the regulation of gene expression has also been considered [138]. Accordingly, plant miR-168a is present in the sera and tissues of many animals, including humans. Furthermore, this miRNA was demonstrated to target the mammalian mRNA for human/mouse low-density lipoprotein receptor adapter protein 1 (LDLRAP1), resulting in both inhibited LDLRAP1 gene expression and a decrease in the level of this protein in the liver. As a consequence, an increased concentration of LDL in the plasma was observed [109]. In addition, many beneficial effects of plant miRNA intake in food have been indicated, such as anti-tumor, anti-viral, anti-apoptotic and anti-inflammatory activities [139]. Moreover, plant miRNAs have been proposed as a link between plant and animal kingdoms, because of the targeting genes involved in DNA damage response (DDR) in both of them, which may have an impact on mammalian DNA repair, replication, chromatin remodeling, cell cycle and cell death [140].
Bioinformatic approaches also provide interesting conclusions. A notable example is the recent description of 15 miRNAs in bovine milk that are completely complementary to the 13 mRNAs of human target genes and 12 miRNAs with 98% complementarity to 19 human genes mRNA [141]. In silico analysis of the edible plant non-coding RNA database reveals that several common plant miRNAs were identified as perfectly matching 22 human transcripts, associated with, e.g., cell cycle, angiogenesis or sarcomere proteins (actin and myosin) [142]. Interestingly, some of the miRNAs derived from neem (Azadirachta indica), a traditional medicinal plant, are suggested to target human genes involved in various diseases, including tuberculosis and viral infections [143]. However, it has been highlighted that the positive prediction of dietary miRNAs and human gene complementarity may be the result of a false-positive effect resulting from contamination with other nucleic acids [144],[145].
4.3. Impact on gut microbiota
Recent reports suggest a possible role of gut microbiota in the adsorption of exogenous miRNAs by modulation of intestinal barrier permeability and integrity, as well as in releasing plant miRNAs from exosome-like vesicles [146]. Studies with ginger-derived nanoparticles demonstrated uptake of plant miRNAs by gut bacteria depending on nanoparticles' lipids. Moreover, these miRNAs have been shown to preferentially target Lactobacillus rhamnosus (LGG) genes. For example, miR-7267-3p targets the LGG monooxygenase ycnE gene, which increases indole-3-carboxaldehyde (I3A) levels and consequently induces IL-22 production [147]. Moreover, miR-167a-5p derived from these particles may protect intestinal epithelial cells from the entry of LGG due to the downregulation of the LGG pilus-specific protein SpaC [147]. In a rat model, oral administration of corn kernel powder or purified corn miRNAs (miR-156a, miR-164a, miR-166a, miR-167a, miR-168a and miR-169p) has been shown to reduce total cecal bacteria, especially Firmicutes, which provides evidence on the shaping of gut microbiota by exogenous miRNA [89]. Strong cross-talk between food miRNA, gut microbiota and dysregulating processes in aging has also been discussed. The role of food-derived miR-21, miR-146a and miR-155 has been particularly highlighted in these pathways [148]. Interestingly, miRNAs generated by intestinal cells can also influence the composition of gut microbiota [93]. Obviously, the impact of dietary miRNAs on intestinal microbiota is of particular importance in miRNA-mediated immunomodulation and is an interesting topic of current research.
4.4. Immunomodulatory effects
Milk is considered a scalable source of EVs serving as vehicles for therapeutic miRNAs that alone seem to be deprived of adverse inflammatory effects after oral delivery [149]. Accordingly, recent studies have shown that bovine milk EVs alone may not activate immune cells [150]. However, vesicles from commercial milk have been demonstrated to carry transforming growth factor β (TGF-β) [151]. On the other hand, incubation of human PBMCs with milk EVs in the presence of IL-2 and IL-12 (mimicking inflammatory conditions) influenced the activation of natural killer (NK) cells and γδ T cells and enhanced interferon-γ (IFN-γ) production [150], whilst it is well established that NK-cell receptors and their ligands are regulated, among others, by different miRNAs [152]. Furthermore, cow colostrum EVs increased IL-1 and IL-6 secretion by RAW macrophages stimulated with lipopolysaccharide (LPS), and miRNAs could partly mediate this effect [153]. While the results of the Probiotics in the Prevention of Allergy among Children trial negated the contribution of miRNAs from human breast milk to a probiotic-induced reduction in the risk of atopic dermatitis development in infancy [154], milk EVs carrying the immunoregulatory miR-155 have been suggested to be crucial for the development of the immune system. Furthermore, their presence in human breast milk could regulate the thymic FoxP3+ regulatory T cell (Treg) maturation, preventing the development of atopy [155]. Moreover, milk ingredients, including miRNAs, have an impact on the increased demethylation of the FOXP3 locus, which increases the number of Tregs, and also regulates their differentiation [156]. As recently described, one of the anti-inflammatory properties of honey is the inhibition of NLRP3 inflammasome activity, and miR-4057 located in the honey-derived vesicle-like nanoparticles seems to mediate this effect. Among others, suppressing inflammasome activation in macrophages caused the inhibition of IL-1β and IL-18 secretion, Casp1 action and pyroptotic cell death [82].
EVs derived from CD8+ suppressor T lymphocytes of mice and carrying immunoregulatory miR-150 play a pivotal role in the regulation of allergies and autoimmunity [157], as well as in inducing T cell tolerance in contact sensitivity [158]. Furthermore, these miRNA-carrying EVs administered orally inhibit delayed-type hypersensitivity (DTH) to food allergens, namely casein and ovalbumin, more effectively than other routes of administration [159],[160]. In this mechanism, B1a cell-derived, antigen-specific antibody free light chains coating EVs [161] enhance their suppressive activity against antigen-presenting cells [160],[162].
5.
Perspectives for therapeutic applications of orally delivered miRNAs
miRNA-based therapeutics have a potential role as a future new avenue for the treatment of many diseases [163]. The different limitations of such a therapy present a multitude of challenges for researchers [164]–[166]. To date, there is little research on the oral delivery of therapeutic miRNAs [167]. However, proven bioactivity and possible therapeutic effects of orally delivered animal and plant miRNAs [119], due to their protection by EVs and nanoparticles [124] or complexing with lipidic derivatives [102], offer hope for the future application of such therapies.
The importance of miRNAs as biomarkers, and also for the pathogenesis and protection against inflammatory bowel disease (IBD), as well as miRNA-microbiota cross-talk in IBD, has been emphasized [168],[169]. Ginger-derived nanoparticles carrying plant miRNAs can ameliorate colitis in mice by enhancing IL-22 production associated with the improvement of intestinal barrier function, and reduction of TNF-α and IL-1β secretion [147]. Orally administered human breast milk-derived EVs carrying various miRNAs, including miR-320 and miR-375, which are reduced in inflamed colon tissues, enhanced the levels of these molecules in the colon and alleviated symptoms of dextran sulfate sodium (DSS)-induced colitis in mice [170]. Additionally, the protective effect of vesicle-carried miR-148a-3p derived from human milk against necrotizing enterocolitis following intraperitoneal administration was recently described [171]. However, studies with small intestinal epithelial IEC6 cells revealing the role of miR-148a-3p in the prevention of LPS-induced cell injury and reduction of p53 expression [171] suggest the possibility of using these molecules in oral treatment. Similarly, porcine milk EVs containing miR-219 and miR-4334 reduced LPS-induced damage in intestinal epithelial cells through inhibition of Toll-like receptor 4/nuclear factor kappa B (TLR4/NF-κB) and p53 pathways [172]. Interestingly, these vesicles attenuate mycotoxin-induced damage in murine intestines through the contained miRNAs, promoting intestinal cell proliferation and improving tight junctions [173].
It is well established that many metabolic disorders are associated with disturbances in gut microbiota [174],[175]. The regulatory role of various miRNAs in metabolic diseases has also been emphasized [176],[177]. One example of using orally delivered plant miRNA from this perspective is a study with corn-derived miRNAs, which may prevent excessive growth of Firmicutes in the intestines, offering protection from obesity and metabolic diseases, in which the abundance of these bacteria is observed [89].
Orally administered plant-derived miR-2911, acting via the SIDT-1-mediated pathway, has an antifibrotic effect by downregulating TGF-β1 in mice suffering from carbon tetrachloride (CCl4)-induced liver fibrosis characterized by upregulation of TGF-β1 [123].
It is well established that oral treatment with milk-derived EVs improves bone condition and reduces osteoclastogenesis [178], and, moreover, many miRNAs are involved in these processes [179]. Milk EVs containing immunoregulatory miR-30a, miR-92a and miR-223, when delivered orally, ameliorated collagen-induced arthritis in DBA/1 mice and spontaneous polyarthritis in IL-1Ra−/− mice. Disease alleviation was associated with the downregulation of pro-inflammatory cytokine production (IL-6 and monocyte chemoattractant protein-1 (MCP-1)), reduced cartilage depletion and bone marrow cellularity, reduced anti-collagen type II antibodies, as well as macroscopic relief of joint inflammation [180]. In turn, healthy mice treated with bovine milk EVs containing miRNAs showed enhancement of the osteogenesis process [181].
Orally administered bovine milk-derived small EVs, after BBB crossing, may affect gene expression in the brain, promote neuronal growth and improve brain function, which may be related to the activity of miR-30d and let-7b abundant in this milk [104].
As mentioned above, miRNAs derived from immune cells and protected by EVs may be administered orally to mice. Accordingly, suppressor T cell-derived EVs carrying miR-150 alleviated ear swelling caused by DTH reaction to casein in mice after administration by oral gavage [159]. In addition, strong suppression of cutaneous DTH reaction to ovalbumin was observed following oral administration of suppressor T cell- and B1a cell-derived EVs carrying miR-150 [160]. Therefore, orally delivered, EV-protected miR-150 appears to be a promising candidate for the therapy of allergies by inducing antigen-specific tolerance [157].
Interestingly, a cocktail of three tumor suppressor miRNAs (miR-34a, miR-143, and miR-145) synthesized on the base of mouse miRNAs, but with plant-specific methylation, reduced tumor burden when orally administered to mice. This opens up a future perspective for a non-toxic, non-invasive and effective oral route of administration for chemopreventive miRNAs [182].
Altogether, similarly to EVs from various sources [183], orally-delivered miRNAs constitute promising candidates for immunomodulatory therapies.
6.
Conclusions
Although there are still many challenges to be solved, the above-discussed observations provide important evidence for the possibility to utilize food-borne miRNAs and orally-administered miRNAs from other sources for personalized therapies in various immune-related diseases. This is a significant part of the current research on the possibility of specific regulation of genes via mediators of RNA interference for therapeutic purposes and implies that they may be effectively administered orally. This route is generally accepted by patients, so it may facilitate the application of these novel research findings to routine clinical practice. Thus, miRNAs should be taken into consideration as important functional food components, as well as food-derived nanoparticles (e.g., milk EVs), are interesting vehicles for the delivery of therapeutic miRNAs and other non-coding RNAs, including small interfering RNA (siRNA).









 DownLoad:
DownLoad: