Double fuzzy $ \alpha $-$ \eth $-continuous multifunctions

M. N. Abu_Shugair; A. A. Abdallah; S. E. Abbas; Ismail Ibedou; M. N. Abu_Shugair; A. A. Abdallah; S. E. Abbas; Ismail Ibedou

doi:10.3934/math.2024806

AIMS Mathematics

2024, Volume 9, Issue 6: 16623-16642. doi: 10.3934/math.2024806

Previous Article Next Article

Research article Special Issues

Double fuzzy $\alpha$ - $\eth$ -continuous multifunctions

1.
Department of Mathematics, College of Science, Jazan University, P.O. Box 114, Jazan 45142, Kingdom of Saudi Arabia
2.
Mathematics Department, Faculty of Science, Sohag University, Sohag 82524, Egypt
3.
Department of Mathematics, Faculty of Science, Benha University, Benha 13518, Egypt

Received: 29 March 2024 Revised: 27 April 2024 Accepted: 07 May 2024 Published: 14 May 2024
MSC : 54C10, 54C60, 54A40, 32A12

Various types of double fuzzy continuity of fuzzy multifunctions are introduced in this paper. These types are double fuzzy upper and lower $\alpha$ - $\eth$ -continuous, almost $\alpha$ - $\eth$ -continuous, weakly $\alpha$ - $\eth$ -continuous and almost weakly $\alpha$ - $\eth$ -continuous multifunctions. Double fuzzy ideals are playing the main role in defining these types of continuous multifunctions. All implications associated with these types are ensured; also, many examples are introduced to illustrate these implications, and to explain the advantages of these new types of continuity for some previous definitions.

Keywords:

multifunctions,
double fuzzy ideal topological space,
double fuzzy $\alpha$ - $\eth$ -continuous multifunction

Citation: M. N. Abu_Shugair, A. A. Abdallah, S. E. Abbas, Ismail Ibedou. Double fuzzy $\alpha$ - $\eth$ -continuous multifunctions[J]. AIMS Mathematics, 2024, 9(6): 16623-16642. doi: 10.3934/math.2024806

Related Papers:

[1]	Ahmet Sinan Cevik, Ismail Naci Cangul, Yilun Shang . Matching some graph dimensions with special generating functions. AIMS Mathematics, 2025, 10(4): 8446-8467. doi: 10.3934/math.2025389
[2]	Dalal Awadh Alrowaili, Uzma Ahmad, Saira Hameeed, Muhammad Javaid . Graphs with mixed metric dimension three and related algorithms. AIMS Mathematics, 2023, 8(7): 16708-16723. doi: 10.3934/math.2023854
[3]	Yuni Listiana, Liliek Susilowati, Slamin Slamin, Fadekemi Janet Osaye . A central local metric dimension on acyclic and grid graph. AIMS Mathematics, 2023, 8(9): 21298-21311. doi: 10.3934/math.20231085
[4]	Ahmed Alamer, Hassan Zafar, Muhammad Javaid . Study of modified prism networks via fractional metric dimension. AIMS Mathematics, 2023, 8(5): 10864-10886. doi: 10.3934/math.2023551
[5]	Supachoke Isariyapalakul, Witsarut Pho-on, Varanoot Khemmani . The true twin classes-based investigation for connected local dimensions of connected graphs. AIMS Mathematics, 2024, 9(4): 9435-9446. doi: 10.3934/math.2024460
[6]	Abdessatar Souissi, El Gheteb Soueidy, Mohamed Rhaima . Clustering property for quantum Markov chains on the comb graph. AIMS Mathematics, 2023, 8(4): 7865-7880. doi: 10.3934/math.2023396
[7]	Hashem Bordbar, Sanja Jančič-Rašovič, Irina Cristea . Regular local hyperrings and hyperdomains. AIMS Mathematics, 2022, 7(12): 20767-20780. doi: 10.3934/math.20221138
[8]	S. Gajavalli, A. Berin Greeni . On strong geodeticity in the lexicographic product of graphs. AIMS Mathematics, 2024, 9(8): 20367-20389. doi: 10.3934/math.2024991
[9]	Xia Hong, Wei Feng . Completely independent spanning trees in some Cartesian product graphs. AIMS Mathematics, 2023, 8(7): 16127-16136. doi: 10.3934/math.2023823
[10]	Chenggang Huo, Humera Bashir, Zohaib Zahid, Yu Ming Chu . On the 2-metric resolvability of graphs. AIMS Mathematics, 2020, 5(6): 6609-6619. doi: 10.3934/math.2020425

Abstract

1. Introduction

Fractional calculus is a modification of the notion of differentiation and integration of arbitrary orders ^{[1,2,3,4,5,6,7]}. As there exist various prototypes in engineering and sciences, fractional calculus has attained the researcher's interest. Multiple models in numerous aspects such as; physics, biology, and engineering are tackled as per fractional differential and integral calculus. Some examples are electrochemistry, signal processing, diffusion, finance, acoustic, plasma physics, image processing, and others ^{[8,9,10,11,12,13]}.

Integral transforms are notified as one of the most suitable approaches to tackle models regarding applied mathematics, mathematical physics, engineering, and some other branches as well. The primary motivation is to deal with the provided mathematical model via any suitable integral transform and to retrieve the associated outcome in the best possible approach.

Via an appropriate selection of integral transform, the differential and integral equations can be transformed into an algebraic equations system, which can be easily tackled.

Various integral transforms and integral transform-based approaches are generated and incorporated for this purpose, such as; Laplace transform ^[14], Sumudu transform ^[15], Elzaki transform ^[16], and Natural transform ^[17], and many others.

Definition 1: Shehu transform of the function $\theta \left(\tau \right)$ is defined over the following functions ^[18,19,20].

$A = \left\{\epsilon \left(\tau \right):there\;exists\;N, {k}_{1}, {k}_{2} > 0\;s.t.\;\left|\theta \left(\tau \right)\right| < Nexp\left(\frac{\left|\tau \right|}{{k}_{i}}\right),\; where\;\tau \in {\left(-1\right)}^{j}\times [0, \infty )\right\}.$

(1)

Definition 2: Shehu transform of function $\epsilon \left(\tau \right)$ is defined as follows ^[18,19,20]:

$S\left[\epsilon \left(\tau \right)\right] = F\left[s, u\right] = {\int }_{0}^{\infty }exp\left[-\frac{s\tau }{u}\right]\epsilon \left(\tau \right)d\tau .$

(2)

Definition 3: Inverse Shehu transform is defined as follows ^[18,19,20]:

${S}^{-1}\left[F\left(s, u\right)\right] = \epsilon \left(\tau \right).$

(3)

Where $s$ , $u$ are the Shehu transform variables. $\alpha \in R$ .

Definition 4: ^[18,19,20]

$S\left[{\epsilon }^{{'}}\left(\tau \right)\right] = \frac{s}{u}F\left[s, u\right]-\epsilon \left(0\right) ,$

(4)

$S\left[{\epsilon }^{{'}{'}}\left(\tau \right)\right] = \frac{{s}^{2}}{{u}^{2}}F\left(s, u\right)-\frac{s}{u}\epsilon \left(0\right)-\epsilon {'}\left(0\right) ,$

(5)

$S\left[{\epsilon }^{{'}{'}{'}}\left(\tau \right)\right] = \frac{{s}^{3}}{{u}^{3}}F\left[s, u\right]-\frac{{s}^{2}}{{u}^{2}}\epsilon \left(0\right)-\frac{s}{u}{\epsilon }^{{'}}\left(0\right)-\epsilon {'}{'}\left(0\right) .$

(6)

Definition 5: Linearity property of Shehu transform ^[18,19,20]:

$S\left[{c}_{1}{\epsilon }_{1}\left(\tau \right)+{c}_{2}{\epsilon }_{2}\left(\tau \right)\right] = {c}_{1}S\left[{\epsilon }_{1}\left(\tau \right)\right]+{c}_{2}S\left[{\epsilon }_{2}\left(\tau \right)\right] .$

(7)

Definition 6: Linearity property of inverse Shehu transform ^[18,19,20]:

${\epsilon }_{1}\left(\tau \right) = {S}^{-1}\left[{F}_{1}\right(s, u\left)\right]\; {\rm{and}} \; {\epsilon }_{2}\left(\tau \right) = {S}^{-1}\left[{F}_{2}\right(s, u\left)\right] ,$

then

${S}^{-1}\left[{c}_{1}{F}_{1}(s, u)+{c}_{2}{F}_{2}(s, u)\right] = {c}_{1}{S}^{-1}\left[{F}_{1}(s, u)\right]+{c}_{2}{S}^{-1}\left[{F}_{2}(s, u)\right],$

${S}^{-1}\left[{c}_{1}{F}_{1}(s, u)+{c}_{2}{F}_{2}(s, u)\right] = {c}_{1}{\epsilon }_{1}\left(\tau \right)+{c}_{2}{\epsilon }_{2}\left(\tau \right) .$

Definition 7: Shehu transform of Caputo fractional derivative [C.F.D.] ^[20,21]:

$S\left[{D}_{t}^{\alpha }\epsilon \left(\mu , \tau \right)\right] = \frac{{s}^{\alpha }}{{\nu }^{\alpha }}S\left[\epsilon \left(\mu , \tau \right)\right]-{\sum }_{r = 0}^{\theta -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\epsilon }^{r}\left(\mu , 0\right).$

(8)

Definition 8: Mittag-Leffler function considered for two parameters was given in ^[22,23,24].

${E}_{\mu , \nu }\left(n\right) = {\sum }_{k = 0}^{\infty }\frac{{n}^{k}}{\mathrm{\Gamma }(k\mu +\nu )} .$

(9)

Where ${E}_{\mathrm{1, 1}}\left(n\right) = \mathrm{e}\mathrm{x}\mathrm{p}\left(n\right)$ and ${E}_{\mathrm{2, 1}}\left({n}^{2}\right) = \mathrm{c}\mathrm{o}\mathrm{s}\left(n\right)$ .

In Tables 1 and 2 basic formulae regarding Shehu transform and inverse Shehu transform are provided.

Table 1. Chart regarding to the Shehu transform ^[20].

	$\mathit{\boldsymbol{\epsilon }}\left(\mathit{\boldsymbol{\tau }}\right)$	$\mathit{\boldsymbol{S}}\left[\mathit{\boldsymbol{\epsilon }}\right(\mathit{\boldsymbol{\tau }}\left)\right]=\mathit{\boldsymbol{F}}(\mathit{\boldsymbol{s}}, \mathit{\boldsymbol{\nu }})$
1.	$1$	$\frac{\nu }{s}$
2.	$\tau$	$\frac{{\nu }^{2}}{{s}^{2}}$
3.	${\tau }^{m}, m\in N$	$\angle m{\left(\frac{\nu }{s}\right)}^{m+1}$
4.	${\tau }^{m}, m > -1$	$\mathrm{\Gamma }(m+1){\left(\frac{\nu }{s}\right)}^{m+1}$
5.	${e}^{a\tau }$	$\frac{\nu }{s-a\nu }$
6.	$\mathrm{s}\mathrm{i}\mathrm{n}\left(m\tau \right)$	$\frac{m{\nu }^{2}}{{s}^{2}+{m}^{2}{\nu }^{2}}$
7.	$cos\left(m\tau \right)$	$\frac{s{\nu }^{2}}{{s}^{2}+{m}^{2}{\nu }^{2}}$
8.	$sinh\left(m\tau \right)$	$\frac{m{\nu }^{2}}{{s}^{2}-{m}^{2}{\nu }^{2}}$
9.	$cosh\left(m\tau \right)$	$\frac{s{\nu }^{2}}{{s}^{2}-{m}^{2}{\nu }^{2}}$

| Show Table

DownLoad: CSV

Table 2. Chart regarding to the inverse Shehu transform ^[20].

	$\mathit{\boldsymbol{F}}(\mathit{\boldsymbol{s}}, \mathit{\boldsymbol{\nu }})$	$\mathit{\boldsymbol{\epsilon }}\left(\mathit{\boldsymbol{\tau }}\right)={\mathit{\boldsymbol{S}}}^{{\bf{-1}}}\left[\mathit{\boldsymbol{F}}\right(\mathit{\boldsymbol{s}}, \mathit{\boldsymbol{\nu }}\left)\right]$
1.	$\frac{\nu }{s}$	$1$
2.	$\frac{{\nu }^{2}}{{s}^{2}}$	$\tau$
3.	${\left(\frac{\nu }{s}\right)}^{m+1}$	$\frac{{\tau }^{m}}{\angle m}$
4.	$\mathrm{\Gamma }(m+1){\left(\frac{\nu }{s}\right)}^{m+1}$	$\frac{{\tau }^{m}}{\mathrm{\Gamma }(m+1)}$
5.	$\frac{\nu }{s-a\nu }$	${e}^{a\tau }$
6.	$\frac{m{\nu }^{2}}{{s}^{2}+{m}^{2}{\nu }^{2}}$	$\mathrm{s}\mathrm{i}\mathrm{n}\left(m\tau \right)$
7.	$\frac{s{\nu }^{2}}{{s}^{2}+{m}^{2}{\nu }^{2}}$	$cos\left(m\tau \right)$
8.	$\frac{m{\nu }^{2}}{{s}^{2}-{m}^{2}{\nu }^{2}}$	$sinh\left(m\tau \right)$
9.	$\frac{s{\nu }^{2}}{{s}^{2}-{m}^{2}{\nu }^{2}}$	$cosh\left(m\tau \right)$

| Show Table

DownLoad: CSV

${\bf{1}}\mathit{\boldsymbol{D}}$ Non-linear time-fractional Schrödinger equation.

$i{D}_{t}^{\alpha }\theta \left(\mu , \tau \right) = R\left[\theta \left(\mu , \tau \right)\right]+N\left[\theta \left(\mu , \tau \right)\right]+\phi \left(\mu , \tau \right).$

(10)

${\bf{2}}\mathit{\boldsymbol{D}}$ Non-linear time-fractional Schrödinger equation.

$i{D}_{t}^{\alpha }\theta ({\mu }_{1}, {\mu }_{2}, \tau ) = R\left[\theta \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]+N\left[\theta \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]+\phi ({\mu }_{1}, {\mu }_{2}, \tau ) .$

(11)

${\bf{3}}\mathit{\boldsymbol{D}}$ Non-linear time-fractional Schrödinger equation.

$i{D}_{t}^{\alpha }\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right) = R\left[\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right]+N\left[\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right]+\phi \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right).$

(12)

One of the well-known models in mathematical physics is Schr $\ddot{o}$ dinger equation model. There exist various implementations in numerous branches, such as; non-linear optics ^[25], mean-field theory of Bose-Einstein condensates ^[26,27], and plasma physics ^[28]. One emerging aspect of quantum physics is considered as fractional Schr $\ddot{o}$ dinger equation; which is associated with the notion of non-local quantum phenomena.

Naber ^[29] notified time-fractional Schr $\ddot{o}$ dinger equation regarding Caputo derivative. Wang and Xu ^[30] elaborated on the linear Schr $\ddot{o}$ dinger equation regarding the space-fractional and time-fractional aspects as well as tackled the models via integral transform approach. Due to the existence of numerous implementations of the time-fractional Schr $\ddot{o}$ dinger equation; various researchers have worked in this field. Regarding this, novel analytical and numerical regimes have been generated for the time-fractional Schr $\ddot{o}$ dinger equation ^{[31,32,33,34]}. Hemida et al. ^[35] implemented HAM to provide the approximated results for the space-time fractional Schr $\ddot{o}$ dinger equation. More work related to fractional Schr $\ddot{o}$ dinger equation is provided in ^[36,37,38]. Other noteworthy work in this regard is notified as ^{[39,40,41,42]}.

The main advantage of ADM is that it does not rely upon perturbation or linearization or any discretization. Therefore, the actual outcome of the model remains unchanged. Discretization of variables is not demanded, which is a difficult and challenging approach. It means that the obtained results are error-free, which occurred because of discretization. Furthermore, it is accurate in finding the approximated and exact solutions of the non-linear prototypes. Such methods can be implemented to the diversified differential equations such as; integro-differential equations, differential-algebraic equations, differential-difference equations, as well as some functional equations, eigenvalue problems, and Stochastic system problems.

The main idea of the present study is to concentrate on the implementation of Shehu ADM for attaining the exact solution to the Schr $\ddot{o}$ dinger equations in various dimensions. Some latest research regarding this field is provided on ^{[43,44,45,46,47]}.

Novelty and significance of the paper

There are several schemes observed in the literature that deal with fractional Schr $\ddot{o}$ dinger equation in one, two, or three dimensions, but rare methods are provided that tackles fractional Schr $\ddot{o}$ dinger equation in all one, two, and three dimensions. Therefore, the authors have focused on developing a technique that proves the validity of the approximated-analytical solution of the mentioned equations in one, two, and three dimensions.

An iterative scheme is developed in the present research regarding the solution of fractional Schr $\ddot{o}$ dinger equation in one, two, and three dimensions. The present scheme is easy to implement and needs no complex programming regarding numerical discretization. Developing the numerical programs for the fractional PDEs is not an easy task; therefore, developing such iterative schemes is the need of time to find the approximated-analytical solutions. There exist several transforms provided in the literature, but from the calculation aspect, some transforms are easy to implement, and some are not. Shehu ADM is noticed as one of the easiest methods to implement integral transform among all existing integral transforms; as in the case of Shehu ADM, no perturbation parameter is required. Via literature, it is observed that fractional Schrödinger equations have never been solved in one, two, and three dimensions with the aid of a single integral transform. Therefore, due to the importance of such equations, in this research, concentration is focused upon the solution for the same, which retains the novelty of the study. Furthermore, convergence analyses are also incorporated in the article.

Motivation of the study

In the present research, an iterative regime is developed and incorporated named Shehu ADM regarding the solution of fractional Schr $\ddot{o}$ dinger equation in one, two, and three dimensions. The present regime is easy to implement and needs no complex calculation in the process of numerical discretization. Generating the numerical programs to deal with the fractional PDEs is cumbersome; therefore, generating such novel iterative regimes is demanded to fetch the approximated-analytical solutions.

There exist numerous transforms in the literature, but as per the calculation aspect, some transforms are easy to incorporate, but some are not. Shehu transform ADM is notified as one of the easiest methods. From an exploration of the literature, it is noticed that fractional Schrödinger equations are rarely solved in one, two, and three dimensions with the aid of a single integral transform-based method. Therefore, in this research, the focus is on finding the solution for the same, which contains the novelty of the research. Moreover, error analysis and convergence analysis are also elaborated in this article.

In the present paper, the convergence of the method is checked numerically. Convergence is affirmed via Tables 3–. As per –, it is observed that on increasing the number of grid points the ${L}_{\infty }$ error norm got reduced rapidly, which is robust proof of the convergence of the generated semi-analytical techniques.

Table 3. Comparison of

${L}_{\infty }$ errors at different time levels regarding Example 1.

$\mathit{\boldsymbol{N}}$	${\mathit{\boldsymbol{L}}}_{{\infty }}$ at $\mathit{\boldsymbol{t}}$ =1	${\mathit{\boldsymbol{L}}}_{{\infty }}$ at $\mathit{\boldsymbol{t}}$ =2	${\mathit{\boldsymbol{L}}}_{{\infty }}$ at $\mathit{\boldsymbol{t}}$ =3
11	2.4979e-08	5.0711e-05	4.3236e-03
21	4.4755e-16	4.1023e-14	2.0302e-10
31	4.4755e-16	5.6610e-16	1.3911e-15
	Convergence up to ${{\bf{10}}}^{{\bf{-16}}}$	Convergence up to ${{\bf{10}}}^{{\bf{-16}}}$	Convergence up to ${{\bf{10}}}^{{\bf{-16}}}$

| Show Table

DownLoad: CSV

Table 4. Comparison of

${L}_{\infty }$ errors at different time levels regarding Example 2.

$\mathit{\boldsymbol{N}}$	${\mathit{\boldsymbol{L}}}_{\mathit{\boldsymbol{\infty }}}$ at $\mathit{\boldsymbol{t}}$ =0.1	${\mathit{\boldsymbol{L}}}_{\mathit{\boldsymbol{\infty }}}$ at $\mathit{\boldsymbol{t}}$ =0.2	${\mathit{\boldsymbol{L}}}_{\mathit{\boldsymbol{\infty }}}$ at $\mathit{\boldsymbol{t}}$ =0.3
11	7.8430e-09	1.5949e-05	1.3635e-03
21	2.4825e-16	4.8963e-15	2.2250e-11
31	3.7238e-16	5.5788e-16	1.1802e-15
	Convergence up to ${{\bf{10}}}^{{\bf{-16}}}$	Convergence up to ${{\bf{10}}}^{{\bf{-16}}}$	Convergence up to ${{\bf{10}}}^{{\bf{-15}}}$

| Show Table

DownLoad: CSV

Table 5. Comparison of

${L}_{\infty }$ errors at different time levels regarding Example 3.

$\mathit{\boldsymbol{N}}$	${\mathit{\boldsymbol{L}}}_{\mathit{\boldsymbol{\infty }}}$ at $\mathit{\boldsymbol{t}}$ =1.0	${\mathit{\boldsymbol{L}}}_{\mathit{\boldsymbol{\infty }}}$ at $\mathit{\boldsymbol{t}}$ =1.3	${\mathit{\boldsymbol{L}}}_{\mathit{\boldsymbol{\infty }}}$ at $\mathit{\boldsymbol{t}}$ =1.5
21	3.1906e-07	7.8034e-05	1.5627e-03
31	8.4843e-15	7.0869e-12	5.9702e-10
41	6.4393e-15	2.9407e-14	5.6576e-14
	Convergence up to ${{\bf{10}}}^{{\bf{-15}}}$	Convergence up to ${{\bf{10}}}^{{\bf{-14}}}$	Convergence up to ${{\bf{10}}}^{{\bf{-14}}}$

| Show Table

DownLoad: CSV

Table 6. Comparison of

${L}_{\infty }$ errors at different time levels regarding Example 4.

$\mathit{\boldsymbol{N}}$	${\mathit{\boldsymbol{L}}}_{\mathit{\boldsymbol{\infty }}}$ at $\mathit{\boldsymbol{t}}$ =1.0	${\mathit{\boldsymbol{L}}}_{\mathit{\boldsymbol{\infty }}}$ at $\mathit{\boldsymbol{t}}$ =1.3	${\mathit{\boldsymbol{L}}}_{\mathit{\boldsymbol{\infty }}}$ at $\mathit{\boldsymbol{t}}$ =1.5
11	1.8114e-06	3.2318e-05	1.5541e-04
21	1.3092e-16	2.0510e-14	4.0767e-13
31	2.2377e-16	2.4825e-16	2.4825e-16
	Convergence up to ${{\bf{10}}}^{{\bf{-16}}}$	Convergence up to ${{\bf{10}}}^{{\bf{-16}}}$	Convergence up to ${{\bf{10}}}^{{\bf{-16}}}$

| Show Table

DownLoad: CSV

Table 7. Comparison of

${L}_{\infty }$ errors at different time levels regarding Example 5.

$\mathit{\boldsymbol{N}}$	${\mathit{\boldsymbol{L}}}_{\mathit{\boldsymbol{\infty }}}$ at $\mathit{\boldsymbol{t}}$ =1	${\mathit{\boldsymbol{L}}}_{\mathit{\boldsymbol{\infty }}}$ at $\mathit{\boldsymbol{t}}$ =2	${\mathit{\boldsymbol{L}}}_{\mathit{\boldsymbol{\infty }}}$ at $\mathit{\boldsymbol{t}}$ =3
21	4.0910e-14	8.4807e-08	4.1536e-04
31	3.3766e-16	1.9860e-15	1.5697e-10
41	3.3766e-16	1.7342e-15	1.6577e-14
	Convergence up to ${{\bf{10}}}^{{\bf{-16}}}$	Convergence up to ${{\bf{10}}}^{{\bf{-15}}}$	Convergence up to ${{\bf{10}}}^{{\bf{-14}}}$

| Show Table

DownLoad: CSV

Table 8. Comparison of

${L}_{\infty }$ errors at different time levels regarding Example 6.

$\mathit{\boldsymbol{N}}$	${\mathit{\boldsymbol{L}}}_{\mathit{\boldsymbol{\infty }}}$ at $\mathit{\boldsymbol{t}}$ =1	${\mathit{\boldsymbol{L}}}_{\mathit{\boldsymbol{\infty }}}$ at $\mathit{\boldsymbol{t}}$ =2	${\mathit{\boldsymbol{L}}}_{\mathit{\boldsymbol{\infty }}}$ at $\mathit{\boldsymbol{t}}$ =3
21	4.4168e-13	9.1039e-07	4.4156e-03
31	5.7220e-17	5.5268e-14	1.5682e-08
41	4.6548e-17	6.9573e-16	8.8374e-15
	Convergence up to ${{\bf{10}}}^{{\bf{-17}}}$	Convergence up to ${{\bf{10}}}^{{\bf{-16}}}$	Convergence up to ${{\bf{10}}}^{{\bf{-15}}}$

| Show Table

DownLoad: CSV

2. Outline of the paper

● The present paper is divided into different sections and subsections.

● In Section 3, Implementation of the Shehu ADM is developed for various kinds of fractional Schr $\ddot{o}$ dinger equations.

● In Sub-section 3.1, the general formula is generated for $1D$ time-fractional Schrödinger equation.

● In Sub-section 3.2, the general formula is generated for $2D$ time-fractional Schrödinger equation.

● In Sub-section 3.3, the general formula is generated for $3D$ time-fractional Schrödinger equation.

● In Section 4, six examples are elaborated to validate the efficiency and efficacy of the developed regime.

● In Section 5, graphical analysis, error analysis, and convergence analysis is notified.

● Section 6 is provided as the concluding remarks.

3. Implementation of the proposed regime

3.1. Implementation of the scheme on $1{D}$ time-fractional Schr $\ddot{{o}}$ dinger equation

Applying Shehu transform upon Eq (1):

$\begin{array}{l} iS\left[{D}_{\tau }^{\alpha }\theta \left(\mu , \tau \right)\right] = S\left[R\left[\theta \left(\mu , \tau \right)\right]+N\left[\theta \left(\mu , \tau \right)\right]+\phi \left(\mu , \tau \right)\right] \\ \Rightarrow S\left[{D}_{\tau }^{\alpha }\theta \left(\mu , \tau \right)\right] = -iS\left[R\left[\theta \left(\mu , \tau \right)\right]+N\left[\theta \left(\mu , \tau \right)\right]+\phi \left(\mu , \tau \right)\right]\\ {\Rightarrow \left(\frac{s}{\nu }\right)}^{\alpha }S\left[\theta \left(\mu , \tau \right)\right]-\sum\limits_{r = 0}^{\theta -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right) = -iS\left[R\left[\theta \left(\mu , \tau \right)\right]+N\left[\theta \left(\mu , \tau \right)\right]+\phi \left(\mu , \tau \right)\right] \\ {\Rightarrow \left(\frac{s}{\nu }\right)}^{\alpha }S\left[\theta \left(\mu , \tau \right)\right] = \sum\limits_{r = 0}^{\theta -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right)-iS\left[R\left[\theta \left(\mu , \tau \right)\right]+N\left[\theta \left(\mu , \tau \right)\right]+\phi \left(\mu , \tau \right)\right] \\ \Rightarrow S\left[\theta \left(\mu , \tau \right)\right] = {\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\theta -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right)-i{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[R\left[\theta \left(\mu , \tau \right)\right]+N\left[\theta \left(\mu , \tau \right)\right]+\phi \left(\mu , \tau \right)\right] \\ \Rightarrow \theta \left(\mu , \tau \right) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\theta -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right)\right]-i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[R\left[\theta \left(\mu , \tau \right)\right]+N\left[\theta \left(\mu , \tau \right)\right]+\phi \left(\mu , \tau \right)\right]\right] \\ \Rightarrow \sum\limits_{n = 0}^{\infty }{\theta }_{n}(\mu , \tau ) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\theta -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right)+\phi \left(\mu , \tau \right)\right] \\ \;\;\;\;\;\;\;\;\;\; -i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[R\left[{\sum }_{n = 0}^{\infty }{\theta }_{n}(\mu , \tau )\right]+N\left[{\sum }_{n = 0}^{\infty }{\theta }_{n}(\mu , \tau )\right]\right]\right] . \end{array}$

(13)

Where,

${\theta }_{0}\left(\mu , \tau \right) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }{\sum }_{r = 0}^{\theta -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right)+\phi \left(\mu , \tau \right)\right].$

(14)

${\theta }_{n+1}(\mu , \tau ) = -i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[R\left[{\sum }_{n = 0}^{\infty }{\theta }_{n}\left(\mu , \tau \right)\right]+N\left[{\sum }_{n = 0}^{\infty }{\theta }_{n}\left(\mu , \tau \right)\right]\right]\right], n = 0, 1, 2, 3, \dots$

(15)

3.2. Implementation of the scheme on $2{D}$ time-fractional Schr $\ddot{{o}}$ dinger equation

Applying Shehu transform upon Eq (2):

$\begin{array}{l} iS\left[{D}_{t}^{\alpha }\theta \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right] = S\left[R\left[\theta \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]+N\left[\theta \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]+\phi \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]\\ \Rightarrow S\left[{D}_{t}^{\alpha }\theta \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right] = -iS\left[R\left[\theta \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]+N\left[\theta \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]+\phi \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right] \\ {\Rightarrow \left(\frac{s}{\nu }\right)}^{\alpha }S\left[\theta \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]-\sum\limits_{r = 0}^{\theta -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, 0\right) \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;= -iS\left[R\left[\theta \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]+N\left[\theta \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]+\phi \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right] \\ \Rightarrow {\left(\frac{s}{\nu }\right)}^{\alpha }S\left[\theta \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right] \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;= \sum\limits_{r = 0}^{\theta -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, 0\right)-iS\left[R\left[\theta \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]+N\left[\theta \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]+\phi \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right] \\ \Rightarrow S\left[\theta \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right] \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;= {\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\theta -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, 0\right)\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;-i{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[R\left[\theta \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]+N\left[\theta \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]+\phi \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right] \\ \Rightarrow \theta \left({\mu }_{1}, {\mu }_{2}, \tau \right) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\theta -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, 0\right)\right]\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;-i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[R\left[\theta \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]+N\left[\theta \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]+\phi \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]\right] \\ \Rightarrow \sum\limits_{n = 0}^{\infty }{\theta }_{n}\left({\mu }_{1}, {\mu }_{2}, \tau \right) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\theta -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, 0\right)+\phi \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right] \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;-i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[R\left[{\sum }_{n = 0}^{\infty }{\theta }_{n}\left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]+N\left[{\sum }_{n = 0}^{\infty }{\theta }_{n}\left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]\right]\right] .\end{array}$

(16)

Where,

$\begin{array}{l} {\theta }_{0}\left({\mu }_{1}, {\mu }_{2}, \tau \right) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }{\sum }_{r = 0}^{\theta -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, 0\right)+\phi \left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]. \\ {\theta }_{n+1}\left({\mu }_{1}, {\mu }_{2}, \tau \right) \end{array}$

(17)

$= -i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[R\left[{\sum }_{n = 0}^{\infty }{\theta }_{n}\left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]+N\left[{\sum }_{n = 0}^{\infty }{\theta }_{n}\left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]\right]\right], n = 0, 1, 2, 3, \dots$

(18)

3.3. Implementation of the scheme on $3{D}$ time-fractional Schr $\ddot{{o}}$ dinger equation

Applying Shehu transform upon Eq (3):

$\begin{array}{l} iS\left[{D}_{t}^{\alpha }\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right] = S\left[R\left[\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right]+N\left[\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right]+\phi \left(x, y, z, t\right)\right] \\ \Rightarrow S\left[{D}_{t}^{\alpha }\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right] = -iS\left[R\left[\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right]+N\left[\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right]+\phi \left(x, y, z, t\right)\right] \\ {\Rightarrow \left(\frac{s}{\nu }\right)}^{\alpha }S\left[\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right]-\sum\limits_{r = 0}^{\theta -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, 0\right) \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;= -iS\left[R\left[\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right]+N\left[\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right]+\phi \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right] \\ {\Rightarrow \left(\frac{s}{\nu }\right)}^{\alpha }S\left[\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right] \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;= \sum\limits_{r = 0}^{\theta -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, 0\right)\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;-iS\left[R\left[\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right]+N\left[\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right]+\phi \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right] \\ \Rightarrow S\left[\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right] \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;= {\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\theta -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, 0\right)\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;-i{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[R\left[\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right]+N\left[\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right]+\phi \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right] \\ \Rightarrow \theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right) \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;= {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\theta -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, 0\right)\right]\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;-i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[R\left[\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right]+N\left[\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right]+\phi \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right]\right] \\ \Rightarrow \sum\limits_{n = 0}^{\infty }{\theta }_{n}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\theta -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, 0\right)+\phi \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right] \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;-i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[R\left[{\sum }_{n = 0}^{\infty }{\theta }_{n}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right]+N\left[{\sum }_{n = 0}^{\infty }{\theta }_{n}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right]\right]\right] . \end{array}$

(19)

Where,

$\begin{array}{l} {\theta }_{0}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }{\sum }_{r = 0}^{\theta -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, 0\right)+\phi \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right].\\ {\theta }_{n+1}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right) \end{array}$

(20)

$= -i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[R\left[{\sum }_{n = 0}^{\infty }{\theta }_{n}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right]+N\left[{\sum }_{n = 0}^{\infty }{\theta }_{n}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right]\right]\right], n = 0, 1, 2, 3, \dots$

(21)

4. Examples and derivation

In the present section, six examples are tested to ensure the validity of the proposed regime, Examples 1–4 are associated with $1D$ time-fractional Schr $\ddot{o}$ dinger. Example 5 is provided regarding $2D$ time-fractional Schrödinger. Example 6 is associated with $3D$ time-fractional Schrödinger equation. In all the provided cases, approximated and exact profiles are generated.

Example 1: Considered $1D$ non-linear time-fractional Schr $\ddot{o}$ dinger as follows ^[36]:

${iD}_{t}^{\alpha }\theta +{\theta }_{\mu \mu }+2{\left|\theta \right|}^{2}\theta = 0 .$

(22)

I.C.: $\theta \left(\mu , 0\right) = {e}^{i\mu }.$

Applying Shehu transform upon Eq (22):

$\begin{array}{l} iS\left[{D}_{\tau }^{\alpha }\theta (\mu , \tau )\right] = -S\left[{\theta }_{\mu \mu }\right(\mu , \tau )+2{\left|\theta (\mu , \tau )\right|}^{2}\theta (\mu , \tau \left)\right] \\ \Rightarrow S\left[{D}_{\tau }^{\alpha }\theta (\mu , \tau )\right] = iS\left[{\theta }_{\mu \mu }\right(\mu , \tau )+2{\left|\theta (\mu , \tau )\right|}^{2}\theta (\mu , \tau \left)\right] \\ \Rightarrow {\left(\frac{s}{\nu }\right)}^{\alpha }S\left[\theta (\mu , \tau )\right]-\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right) = iS\left[{\theta }_{\mu \mu }(\mu , \tau )+2{\left|\theta (\mu , \tau )\right|}^{2}\theta (\mu , \tau )\right] \\ \Rightarrow {\left(\frac{s}{\nu }\right)}^{\alpha }S\left[\theta (\mu , \tau )\right] = \sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right)+iS\left[{\theta }_{\mu \mu }(\mu , \tau )+2{\left|\theta (\mu , \tau )\right|}^{2}\theta (\mu , \tau )\right] \\ \Rightarrow S\left[\theta (\mu , \tau )\right] = {\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right)+i{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[{\theta }_{\mu \mu }\right(\mu , \tau )+2{\left|\theta (\mu , \tau )\right|}^{2}\theta (\mu , \tau \left)\right] \\ \Rightarrow \theta (\mu , \tau ) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right)\right]+i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[{\theta }_{\mu \mu }(\mu , \tau )+2{\left|\theta (\mu , \tau )\right|}^{2}\theta (\mu , \tau )\right]\right] \\ \Rightarrow \sum\nolimits_{n = 0}^{\infty }{\theta }_{n}(\mu , \tau ) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }\sum\nolimits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right)\right]+i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[{\left({\sum }_{n = 0}^{\infty }{\theta }_{n}(\mu , \tau )\right)}_{\mu \mu }+2{\sum }_{n = 0}^{\infty }{A}_{n}\right]\right]. \end{array}$

(23)

Where

$F\left(\theta \right) = {\left|\theta (\mu , \tau )\right|}^{2}\theta (\mu , \tau ) = \sum\limits_{n = 0}^{\infty }{A}_{n}.$

${\theta }_{0}\left(\mu , \tau \right) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right)\right].$

${\theta }_{n+1}(\mu , \tau ) = i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[{\left({\theta }_{n}(\mu , \tau )\right)}_{\mu \mu }+2{A}_{n}\right]\right], n = 0, 1, 2, 3, \dots$

Considered, $\xi = 1$ :

${\theta }_{0}(\mu , \tau ) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right)\right]$

$\Rightarrow {\theta }_{0}(\mu , \tau ) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }{\left(\frac{s}{\nu }\right)}^{\alpha -1}\theta \left(\mu , 0\right)\right]$

$\Rightarrow {\theta }_{0}\left(\mu , \tau \right) = \theta \left(\mu , 0\right) = {e}^{i\mu }.$

Considered $n = 0$ :

${\theta }_{1}(\mu , \tau ) = i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[{\left({\theta }_{0}(\mu , \tau )\right)}_{\mu \mu }+2{A}_{0}\right]\right] .$

Where,

${\left({\theta }_{0}\right)}_{\mu \mu }\left(\mu , \tau \right) = -{e}^{ix}, {A}_{0} = F\left({\theta }_{0}\right) = {\theta }_{0}^{2}\stackrel{-}{{\theta }_{0}} = {e}^{i\mu }.$

${\theta }_{1}\left(\mu , \tau \right) = i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[-{e}^{i\mu }+2{e}^{i\mu }\right]\right].$

$\Rightarrow {\theta }_{1}(\mu , \tau ) = i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[{e}^{i\mu }\right]\right]$

$\Rightarrow {\theta }_{1}(\mu , \tau ) = i{e}^{i\mu }{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[1\right]\right]$

$\Rightarrow {\theta }_{1}\left(\mu , \tau \right) = i{e}^{i\mu }{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{2\alpha }\right]\Rightarrow {\theta }_{1}\left(\mu , \tau \right) = i{e}^{i\mu }\frac{{t}^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}.$

Considered $n = 1:$

${\theta }_{2}\left(\mu , \tau \right) = i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[{\left({\theta }_{1}\right)}_{\mu \mu }+2{A}_{1}\right]\right].$

Where,

${\left({\theta }_{1}\right)}_{\mu \mu }(\mu , \tau ) = -i{e}^{i\mu }\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)} ,$

${A}_{1} = 2{\theta }_{0}{\theta }_{1}\stackrel{-}{{\theta }_{0}}+{\theta }_{0}^{2}\stackrel{-}{{\theta }_{1}} = i{e}^{i\mu }\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)} ,$

${\theta }_{2}(\mu , \tau ) = i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[-i{e}^{i\mu }\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}+2i{e}^{i\mu }\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}\right]\right]$

$\Rightarrow {\theta }_{2}(\mu , \tau ) = i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[i{e}^{i\mu }\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}\right]\right]$

$\Rightarrow {\theta }_{2}(\mu , \tau ) = \left({i}^{2}{e}^{i\mu }\right){S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }{\left(\frac{\nu }{s}\right)}^{2\alpha }\right]$

$\Rightarrow {\theta }_{2}(\mu , \tau ) = \left({i}^{2}{e}^{i\mu }\right){S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{3\alpha }\right]$

${\Rightarrow \theta }_{2}(\mu , \tau ) = {i}^{2}{e}^{i\mu }\frac{{\tau }^{3\alpha -1}}{\mathrm{\Gamma }\left(3\alpha \right)} ,$

$\theta (\mu , \tau ) = {\theta }_{0}(\mu , \tau )+{\theta }_{1}(\mu , \tau )+{\theta }_{2}(\mu , \tau )+{\theta }_{3}(\mu , \tau )+\dots$

$\Rightarrow \theta (\mu , \tau ) = {e}^{i\mu }+i{e}^{i\mu }\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}+{i}^{2}{e}^{i\mu }\frac{{\tau }^{3\alpha -1}}{\mathrm{\Gamma }\left(3\alpha \right)}+\dots$

$\Rightarrow \theta (\mu , \tau ) = {e}^{i\mu }\left[1+i\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}+{i}^{2}\frac{{\tau }^{3\alpha -1}}{\mathrm{\Gamma }\left(3\alpha \right)}+\dots \right] .$

Considered $\alpha = 1$ :

$\Rightarrow \theta (\mu , \tau ) = {e}^{i\mu }\left[1+\frac{i\tau }{1!}+\frac{{\left(i\tau \right)}^{2}}{2!}+\dots \right]$

$\Rightarrow \theta (\mu , \tau ) = {e}^{i[\mu +t]} .$

Example 2: Considered $1D$ linear time-fractional Schr $\ddot{o}$ dinger equation as follows ^[37]:

${D}_{\tau }^{\alpha }\theta (\mu , \tau )+i{\theta }_{\mu \mu }(\mu , \tau ) = 0 .$

(23)

I.C.: $\theta \left(\mu , 0\right) = {e}^{3i\mu }$

Applying Shehu transform in Eq (23):

${\left(\frac{s}{\nu }\right)}^{\alpha }S\left[\theta (\mu , \tau )\right]-\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right) = -iS\left[{\theta }_{\mu \mu }(\mu , \tau )\right]$

$\Rightarrow {\left(\frac{s}{\nu }\right)}^{\alpha }S\left[\theta (\mu , \tau )\right] = \sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right)-iS\left[{\theta }_{\mu \mu }(\mu , \tau )\right]$

$\Rightarrow S\left[\theta (\mu , \tau )\right] = {\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right)-i{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[{\theta }_{\mu \mu }(\mu , \tau )\right]$

$\Rightarrow \theta (\mu , \tau ) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right)\right]-i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[{\theta }_{\mu \mu }(\mu , \tau )\right]\right]$

$\Rightarrow \sum\limits_{n = 0}^{\infty }{\theta }_{n}\left(\mu , \tau \right) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right)\right]-i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[{\left(\sum\limits_{n = 0}^{\infty }{\theta }_{n}\left(\mu , \tau \right)\right)}_{\mu \mu }\right]\right],$

${\theta }_{n+1}(\mu , \tau ) = -i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[{\left({\theta }_{n}(\mu , \tau )\right)}_{\mu \mu }\right]\right], n = 0, 1, 2, 3, \dots$

Considered $\xi = 1$ :

${\theta }_{0}(\mu , \tau ) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }{\left(\frac{s}{\nu }\right)}^{\alpha -1}\theta \left(\mu , 0\right)\right]$

${\Rightarrow \theta }_{0}(\mu , \tau ) = {S}^{-1}\left[\left(\frac{\nu }{s}\right)\theta \left(\mu , 0\right)\right]$

$\Rightarrow {\theta }_{0}(\mu , \tau ) = \theta \left(\mu , 0\right){S}^{-1}\left[\frac{\nu }{s}\right]$

$\Rightarrow {\theta }_{0}\left(\mu , \tau \right) = \theta \left(\mu , 0\right) = {e}^{3i\mu }.$

Considered $n = 0$ :

${\theta }_{1}(\mu , \tau ) = -i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[{\left({\theta }_{0}(\mu , \tau )\right)}_{\mu \mu }\right]\right] , {\left({\theta }_{0}\right)}_{\mu \mu } = {\left(3i\right)}^{2}{e}^{3i\mu }$

$\Rightarrow {\theta }_{1}(\mu , \tau ) = -i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[{\left(3i\right)}^{2}{e}^{3i\mu }\right]\right]$

$\Rightarrow {\theta }_{1}(\mu , \tau ) = -i{\left(3i\right)}^{2}{e}^{3i\mu }{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[1\right]\right]$

${\Rightarrow \theta }_{1}(\mu , \tau ) = -i{\left(3i\right)}^{2}{e}^{3i\mu }{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{2\alpha }\right]$

$\Rightarrow {\theta }_{1}\left(\mu , \tau \right) = 9i{e}^{3i\mu }\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}.$

Considered $n = 1$ :

${\theta }_{2}(\mu , \tau ) = -i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[{\left({\theta }_{1}\right)}_{\mu \mu }\right]\right] , {\left({\theta }_{1}(\mu , \tau )\right)}_{\mu \mu } = 81{i}^{3}{e}^{3i\mu }\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}$

$\Rightarrow {\theta }_{2}(\mu , \tau ) = -i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[81{i}^{3}{e}^{3i\mu }\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}\right]\right]$

$\Rightarrow {\theta }_{2}(\mu , \tau ) = -i\left(81{i}^{3}{e}^{3i\mu }\right){S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}\right]\right]$

${\Rightarrow \theta }_{2}(\mu , \tau ) = -i\left(81{i}^{3}{e}^{3i\mu }\right){S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{3\alpha }\right]$

$\Rightarrow {\theta }_{2}(\mu , \tau ) = 81{i}^{2}{e}^{3i\mu }\frac{{\tau }^{3\alpha -1}}{\mathrm{\Gamma }\left(3\alpha \right)}$

$\Rightarrow \theta (\mu , \tau ) = {\theta }_{0}(\mu , \tau )+{\theta }_{1}(\mu , \tau )+{\theta }_{2}(\mu , \tau )+{\theta }_{3}(\mu , \tau )+\dots$

$\Rightarrow \theta (\mu , \tau ) = {e}^{3i\mu }+9i{e}^{3i\mu }\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}+81{i}^{2}{e}^{3i\mu }\frac{{\tau }^{3\alpha -1}}{\mathrm{\Gamma }\left(3\alpha \right)}+\dots$

Considered $\alpha = 1$ :

$\Rightarrow \theta (\mu , \tau ) = {e}^{3i\mu }\left[1+\frac{9i\tau }{1!}+\frac{{\left(9i\tau \right)}^{2}}{2!}+\dots \right]$

$\Rightarrow \theta \left(\mu , \tau \right) = {e}^{3i\left[\mu +3\tau \right]}.$

Example 3: Considered $1D$ linear time-fractional Schr $\ddot{o}$ dinger equation as follows ^[37]:

${D}_{\tau }^{\alpha }\theta (\mu , \tau )+i{\theta }_{\mu \mu }(\mu , \tau ) = 0 .$

(24)

I.C.: $\theta \left(\mu , 0\right) = 1+cosh\left(2\mu \right)$

Applying Shehu transform upon Eq (24):

$S\left[{D}_{\tau }^{\alpha }\theta \right] = -iS\left[{\theta }_{\mu \mu }\right]$

${\Rightarrow \left(\frac{s}{\nu }\right)}^{\alpha }S\left[\theta (\mu , \tau )\right]-\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right) = -iS\left[{\theta }_{\mu \mu }(\mu , \tau )\right]$

${\Rightarrow \left(\frac{s}{\nu }\right)}^{\alpha }S\left[\theta (\mu , \tau )\right] = \sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right)-iS\left[{\theta }_{\mu \mu }(\mu , \tau )\right]$

Where

${\theta }_{n+1}(\mu , \tau ) = -i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[{\left({\theta }_{n}(\mu , \tau )\right)}_{\mu \mu }\right]\right], n = 0, 1, 2, 3, \dots$

Considered $\xi = 1$ :

$\Rightarrow {\theta }_{0}(\mu , \tau ) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }{\left(\frac{s}{\nu }\right)}^{\alpha -1}\theta \left(\mu , 0\right)\right]$

$\Rightarrow {\theta }_{0}(\mu , \tau ) = \theta \left(x, 0\right){S}^{-1}\left[\frac{\nu }{s}\right]$

$\Rightarrow {\theta }_{0}\left(\mu , \tau \right) = \theta \left(\mu , 0\right) = 1+\mathrm{cosh}\left(2\mu \right).$

Considered $n = 0$ :

${\theta }_{1}(\mu , \tau ) = -i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[{\left({\theta }_{0}(\mu , \tau )\right)}_{\mu \mu }\right]\right], {\left({\theta }_{0}\right)}_{\mu \mu } = 4\mathrm{c}\mathrm{o}\mathrm{s}\mathrm{h}\left(2\mu \right)$

${\Rightarrow \theta }_{1}(\mu , \tau ) = -i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[4\mathrm{c}\mathrm{o}\mathrm{s}\mathrm{h}\left(2\mu \right)\right]\right]$

$\Rightarrow {\theta }_{1}(\mu , \tau ) = -4i\mathrm{c}\mathrm{o}\mathrm{s}\mathrm{h}\left(2\mu \right){S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{2\alpha }\right]$

$\Rightarrow {\theta }_{1}\left(\mu , \tau \right) = -4i\mathrm{cosh}\left(2\mu \right)\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}.$

Considered $n = 1$ :

${\theta }_{2}(\mu , \tau ) = -i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[{\left({\theta }_{1}\right)}_{\mu \mu }\right]\right], {\left({\theta }_{1}(\mu , \tau )\right)}_{\mu \mu } = -16i\mathrm{cosh}\left(2\mu \right)\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}$

$\Rightarrow {\theta }_{2}(\mu , \tau ) = -i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[-16i\mathrm{cosh}\left(2\mu \right)\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}\right]\right]$

$\Rightarrow {\theta }_{2}(\mu , \tau ) = 16{i}^{2}\mathrm{cosh}\left(2\mu \right){S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{3\alpha }\right]$

${\Rightarrow \theta }_{2}(\mu , \tau ) = 16{i}^{2}\mathrm{cosh}\left(2\mu \right)\frac{{\tau }^{3\alpha -1}}{\mathrm{\Gamma }\left(3\alpha \right)}$

$\Rightarrow \theta (\mu , \tau ) = {\theta }_{0}(\mu , \tau )+{\theta }_{1}(\mu , \tau )+{\theta }_{2}(\mu , \tau )+{\theta }_{3}(\mu , \tau )+\dots$

$\Rightarrow \theta (\mu , \tau ) = 1+\mathrm{cosh}\left(2\mu \right)-4i\mathrm{cosh}\left(2\mu \right)\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}+16{i}^{2}\mathrm{cosh}\left(2\mu \right)\frac{{\tau }^{3\alpha -1}}{\mathrm{\Gamma }\left(3\alpha \right)}-\dots$

Considered $\alpha = 1$ :

$\Rightarrow \theta (\mu , \tau ) = 1+\mathrm{cosh}\left(2\mu \right)\left[1-\frac{4i\tau }{1!}+\frac{{\left(4i\tau \right)}^{2}}{2!}-\dots \right]$

$\Rightarrow \theta \left(\mu , \tau \right) = 1+\mathrm{cosh}\left(2\mu \right)\;\mathrm{exp}\left[-4\mathrm{i}\tau \right].$

Example 4: Considered $1D$ non-linear time-fractional Schr $\ddot{o}$ dinger equation as follows ^[38]:

$i{D}_{t}^{\alpha }\theta (\mu , \tau )+\frac{1}{2}{\theta }_{\mu \mu }(\mu , \tau )-\theta \left(\mu , \tau \right)co{s}^{2}\mu -\theta (\mu , \tau ){\left|\theta (\mu , \tau )\right|}^{2} = 0 , \text{μ}\in [0, 1] .$

(25)

I.C.: $u\left(\mu , 0\right) = sin\mu$

Applying Shehu transform upon Eq (25):

$iS\left[{D}_{\tau }^{\alpha }\theta (\mu , \tau )\right] = S\left[-\frac{1}{2}{u}_{\mu \mu }(\mu , \tau )+\theta \left(\mu , \tau \right)co{s}^{2}\mu +\theta (\mu , \tau ){\left|\theta (\mu , \tau )\right|}^{2}\right]$

$\Rightarrow S\left[{D}_{\tau }^{\alpha }\theta (\mu , \tau )\right] = -iS\left[-\frac{1}{2}{u}_{\mu \mu }(\mu , \tau )+\theta \left(\mu , \tau \right)co{s}^{2}\mu +\theta (\mu , \tau ){\left|\theta (\mu , \tau )\right|}^{2}\right]$

$\begin{array}{l} \Rightarrow {\left(\frac{s}{\nu }\right)}^{\alpha }S\left[\theta (\mu , \tau )\right]-\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right) \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;= -iS\left[-\frac{1}{2}{u}_{\mu \mu }(\mu , \tau )+\theta \left(\mu , \tau \right)co{s}^{2}\mu +\theta (\mu , \tau ){\left|\theta (\mu , \tau )\right|}^{2}\right] \end{array}$

$\begin{array}{l} {\Rightarrow \left(\frac{s}{\nu }\right)}^{\alpha }S\left[\theta (\mu , \tau )\right] \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;= \sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right)-iS\left[-\frac{1}{2}{u}_{\mu \mu }(\mu , \tau )+\theta \left(\mu , \tau \right)co{s}^{2}\mu +\theta (\mu , \tau ){\left|\theta (\mu , \tau )\right|}^{2}\right] \end{array}$

$\begin{array}{l} \Rightarrow S\left[\theta (\mu , \tau )\right] = {\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right)\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;-i{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[-\frac{1}{2}{u}_{\mu \mu }(\mu , \tau )+\theta \left(\mu , \tau \right)co{s}^{2}\mu +\theta (\mu , \tau ){\left|\theta (\mu , \tau )\right|}^{2}\right] \end{array}$

$\begin{array}{l} \Rightarrow \theta (\mu , \tau ) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right)\right]\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;-i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[-\frac{1}{2}{u}_{\mu \mu }(\mu , \tau )+\theta \left(\mu , \tau \right)co{s}^{2}\mu +\theta (\mu , \tau ){\left|\theta (\mu , \tau )\right|}^{2}\right]\right] \end{array}$

$\begin{array}{l} \Rightarrow \sum\limits_{n = 0}^{\infty }{\theta }_{n}(\mu , \tau ) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(\mu , 0\right)\right]\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;+i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[\frac{1}{2}{\left(\sum\limits_{n = 0}^{\infty }{u}_{n}(\mu , \tau )\right)}_{\mu \mu }-co{s}^{2}\mu \left(\sum\limits_{n = 0}^{\infty }{u}_{n}(\mu , \tau )\right)-\sum\limits_{n = 0}^{\infty }{A}_{n}\right]\right] \end{array}$

${\theta }_{n+1}(\mu , \tau ) = i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[\frac{1}{2}{\left({\theta }_{n}(\mu , \tau )\right)}_{\mu \mu }-co{s}^{2}\mu \left({\theta }_{n}(\mu , \tau )\right)-{A}_{n}\right]\right], n = 0, 1, 2, 3, \dots$

Considered $\xi = 1$ :

$\Rightarrow {\theta }_{0}(\mu , \tau ) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }{\left(\frac{s}{\nu }\right)}^{\alpha -1}\theta \left(\mu , 0\right)\right]$

$\Rightarrow {\theta }_{0}(\mu , \tau ) = \theta \left(\mu , 0\right){S}^{-1}\left[\frac{\nu }{s}\right]$

${\Rightarrow \theta }_{0} = u\left(\mu , 0\right) = sin\mu .$

Considered $n = 0$ :

${\theta }_{1}\left(\mu , \tau \right) = i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[\frac{1}{2}{\left({\theta }_{0}\right)}_{\mu \mu }-co{s}^{2}\mu \left({\theta }_{0}\right)-{A}_{0}\right]\right].$

Where,

${\left({\theta }_{0}\right)}_{\mu \mu } = -sin\mu , {A}_{0} = F\left({\theta }_{0}\right) = {\theta }_{0}^{2}\stackrel{-}{{\theta }_{0}} = {\mathrm{sin}}^{3}\mu$

$\Rightarrow {\theta }_{1}(\mu , \tau ) = i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[\frac{1}{2}(-sin\mu )-co{s}^{2}x\left(sin\mu \right)-{\mathrm{sin}}^{3}\mu \right]\right]$

$\Rightarrow {\theta }_{1}(\mu , \tau ) = i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[-\frac{3}{2}sin\mu \right]\right]$

$\Rightarrow {\theta }_{1}(\mu , \tau ) = -\frac{3i}{2}sin\mu {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{2\alpha }\right]$

$\Rightarrow {\theta }_{1}\left(\mu , \tau \right) = -\frac{3i}{2}sin\mu \frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}.$

Considered $n = 1$ :

${\theta }_{2}\left(\mu , \tau \right) = i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[\frac{1}{2}{\left({\theta }_{1}\left(\mu , \tau \right)\right)}_{\mu \mu }-co{s}^{2}\mu \left({\theta }_{1}\left(\mu , \tau \right)\right)-{A}_{1}\right]\right].$

Where

${\left({\theta }_{1}\right)}_{\mu \mu }(\mu , \tau ) = \left(\frac{3i}{2}\right)sin\mu \frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)} , {A}_{1} = 2{\theta }_{0}{\theta }_{1}\stackrel{-}{{\theta }_{0}}+{\theta }_{0}^{2}\stackrel{-}{{\theta }_{1}} = \left(-\frac{3i}{2}\right){\mathrm{sin}}^{3}\mu \frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}$

$\begin{array}{l} \Rightarrow {\theta }_{2}(\mu , \tau ) = i{S}^{-1}[{\left(\frac{\nu }{s}\right)}^{\alpha }S[\frac{1}{2}\left(\left(\frac{3i}{2}\right)sin\mu \frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}\right)-co{s}^{2}\mu \left(-\frac{3i}{2}sin\mu \frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}\right)\\ \;\;\;\;\;\;\;\;\;-\left(\left(-\frac{3i}{2}\right){\mathrm{sin}}^{3}\mu \frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}\right)]] \end{array}$

${\Rightarrow \theta }_{2}(\mu , \tau ) = \left(\frac{9{i}^{2}}{4}\right)sin\mu {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}\right]\right]$

${\Rightarrow \theta }_{2}(\mu , \tau ) = \left(\frac{9{i}^{2}}{4}\right)sin\mu {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{3\alpha }\right]$

${\Rightarrow \theta }_{2}(\mu , \tau ) = \left(\frac{9{i}^{2}}{4}\right)sin\mu \frac{{\tau }^{3\alpha -1}}{\mathrm{\Gamma }\left(3\alpha \right)}$

$\Rightarrow \theta (\mu , \tau ) = {\theta }_{0}(\mu , \tau )+{\theta }_{1}(\mu , \tau )+{\theta }_{2}(\mu , \tau )+{\theta }_{3}(\mu , \tau )+\dots$

$\Rightarrow \theta (\mu , \tau ) = sin\mu -\frac{3i}{2}sin\mu \frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}+\left(\frac{9{i}^{2}}{4}\right)sin\mu \frac{{\tau }^{3\alpha -1}}{\mathrm{\Gamma }\left(3\alpha \right)}-\dots$

Considered $\alpha = 1$ :

$\Rightarrow \theta (\mu , \tau ) = sin\mu \left[1-\frac{\left(\frac{3i\tau }{2}\right)}{1!}+\frac{{\left(\frac{3i\tau }{2}\right)}^{2}}{2!}-\dots \right]$

$\Rightarrow \theta (\mu , \tau ) = sin\mu \;\mathrm{exp}\left[-\frac{3i\tau }{2}\right] .$

Example 5: Considered $2D$ non-linear time-fractional Schr $\ddot{o}$ dinger equation as follows ^[38]:

$i{D}_{\tau }^{\alpha }\theta = -\frac{1}{2}\left[{\theta }_{{\mu }_{1}{\mu }_{1}}+{\theta }_{{\mu }_{2}{\mu }_{2}}\right]+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}\right)\theta +\theta {\left|\theta \right|}^{2} ,$

(26)

where ${\mu }_{1}, \mu {\text{}}_{2}\in \left[0, 2\pi \right]\times [0, 2\pi ]$ .

I.C.: $\theta \left({\mu }_{1}, {\mu }_{2}, 0\right) = sin{\mu }_{1}sin{\mu }_{2}$

Applying Shehu transform in Eq (26):

$\begin{array}{l} iS\left[{D}_{\tau }^{\alpha }\theta ({\mu }_{1}, {\mu }_{2}, \tau )\right] \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;= S [-\frac{1}{2}\left[{\theta }_{{\mu }_{1}{\mu }_{1}}({\mu }_{1}, {\mu }_{2}, \tau )+{\theta }_{{\mu }_{2}{\mu }_{2}}({\mu }_{1}, {\mu }_{2}, \tau )\right]+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}\right)\theta ({\mu }_{1}, {\mu }_{2}, \tau )\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;+\theta ({\mu }_{1}, {\mu }_{2}, \tau ){\left|\theta ({\mu }_{1}, {\mu }_{2}, \tau )\right|}^{2}] \end{array}$

$\begin{array}{l} \Rightarrow S\left[{D}_{\tau }^{\alpha }\theta ({\mu }_{1}, {\mu }_{2}, \tau )\right] \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;= -iS [-\frac{1}{2}\left[{\theta }_{{\mu }_{1}{\mu }_{1}}({\mu }_{1}, {\mu }_{2}, \tau )+{\theta }_{{\mu }_{2}{\mu }_{2}}({\mu }_{1}, {\mu }_{2}, \tau )\right]+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}\right)\theta ({\mu }_{1}, {\mu }_{2}, \tau )\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;+\theta ({\mu }_{1}, {\mu }_{2}, \tau ){\left|\theta ({\mu }_{1}, {\mu }_{2}, \tau )\right|}^{2}] \end{array}$

$\begin{array}{l} \Rightarrow {\left(\frac{s}{\nu }\right)}^{\alpha }S\left[\theta ({\mu }_{1}, {\mu }_{2}, \tau )\right]-\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, 0\right) \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;= -iS [-\frac{1}{2}\left[{\theta }_{{\mu }_{1}{\mu }_{1}}({\mu }_{1}, {\mu }_{2}, \tau )+{\theta }_{{\mu }_{2}{\mu }_{2}}({\mu }_{1}, {\mu }_{2}, \tau )\right]+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}\right)\theta ({\mu }_{1}, {\mu }_{2}, \tau )\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;+\theta ({\mu }_{1}, {\mu }_{2}, \tau ){\left|\theta ({\mu }_{1}, {\mu }_{2}, \tau )\right|}^{2}] \end{array}$

$\begin{array}{l} \Rightarrow {\left(\frac{s}{\nu }\right)}^{\alpha }S [\theta ({\mu }_{1}, {\mu }_{2}, \tau )] \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;= \sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, 0\right)\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;-iS [-\frac{1}{2}\left[{\theta }_{{\mu }_{1}{\mu }_{1}}({\mu }_{1}, {\mu }_{2}, \tau )+{\theta }_{{\mu }_{2}{\mu }_{2}}({\mu }_{1}, {\mu }_{2}, \tau )\right]+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}\right)\theta ({\mu }_{1}, {\mu }_{2}, \tau )\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;+\theta ({\mu }_{1}, {\mu }_{2}, \tau ){\left|\theta ({\mu }_{1}, {\mu }_{2}, \tau )\right|}^{2}] \end{array}$

$\begin{array}{l} \Rightarrow S\left[\theta ({\mu }_{1}, {\mu }_{2}, \tau )\right] \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;= {\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, 0\right)\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;-i{\left(\frac{\nu }{s}\right)}^{\alpha }S [-\frac{1}{2}\left[{\theta }_{{\mu }_{1}{\mu }_{1}}({\mu }_{1}, {\mu }_{2}, \tau )+{\theta }_{{\mu }_{2}{\mu }_{2}}({\mu }_{1}, {\mu }_{2}, \tau )\right]+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}\right)\theta ({\mu }_{1}, {\mu }_{2}, \tau )\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;+\theta ({\mu }_{1}, {\mu }_{2}, \tau ){\left|\theta ({\mu }_{1}, {\mu }_{2}, \tau )\right|}^{2}] \end{array}$

$\begin{array}{l} \Rightarrow \theta ({\mu }_{1}, {\mu }_{2}, \tau ) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, 0\right)\right]\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;-i{S}^{-1} [{\left(\frac{\nu }{s}\right)}^{\alpha }S [-\frac{1}{2}\left[{\theta }_{{\mu }_{1}{\mu }_{1}}({\mu }_{1}, {\mu }_{2}, \tau )+{\theta }_{{\mu }_{2}{\mu }_{2}}({\mu }_{1}, {\mu }_{2}, \tau )\right]\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}\right)\theta ({\mu }_{1}, {\mu }_{2}, \tau )+\theta ({\mu }_{1}, {\mu }_{2}, \tau ){\left|\theta ({\mu }_{1}, {\mu }_{2}, \tau )\right|}^{2}]] \end{array}$

$\begin{array}{l} \Rightarrow \sum\limits_{n = 0}^{\infty }{\theta }_{n}({\mu }_{1}, {\mu }_{2}, \tau ) \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;= {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, 0\right)\right]\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;-i{S}^{-1}[{\left(\frac{\nu }{s}\right)}^{\alpha }S[-\frac{1}{2}\left[{\left(\sum\limits_{n = 0}^{\infty }{\theta }_{n}({\mu }_{1}, {\mu }_{2}, \tau )\right)}_{{\mu }_{1}{\mu }_{1}}+{\left(\sum\limits_{n = 0}^{\infty }{\theta }_{n}({\mu }_{1}, {\mu }_{2}, \tau )\right)}_{{\mu }_{2}{\mu }_{2}}\right]\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}\right)\sum\limits_{n = 0}^{\infty }{u}_{n}({\mu }_{1}, {\mu }_{2}, \tau )+\sum\limits_{n = 0}^{\infty }{A}_{n}]] \end{array}$

${u}_{0}\left({\mu }_{1}, {\mu }_{2}, \tau \right) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, 0\right)\right],$

$\begin{array}{l} {u}_{n+1}({\mu }_{1}, {\mu }_{2}, \tau ) \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;= -i{S}^{-1}[{\left(\frac{\nu }{s}\right)}^{\alpha }S[-\frac{1}{2}\left[{\left({\theta }_{n}\right)}_{{\mu }_{1}{\mu }_{1}}({\mu }_{1}, {\mu }_{2}, \tau )+{\left({\theta }_{n}\right)}_{{\mu }_{2}{\mu }_{2}}({\mu }_{1}, {\mu }_{2}, \tau )\right]\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}\right){\theta }_{n}({\mu }_{1}, {\mu }_{2}, \tau )+{A}_{n}]], n = 0, 1, 2, 3, \dots \end{array}$

Considered $\xi = 1$ :

$\Rightarrow {\theta }_{0}({\mu }_{1}, {\mu }_{2}, \tau ) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }{\left(\frac{s}{\nu }\right)}^{\alpha -1}\theta \left({\mu }_{1}, {\mu }_{2}, 0\right)\right]$

$\Rightarrow {\theta }_{0}\left({\mu }_{1}, {\mu }_{2}, \tau \right) = \theta \left({\mu }_{1}, {\mu }_{2}, 0\right),$

${\theta }_{0}\left({\mu }_{1}, {\mu }_{2}, \tau \right) = sin{\mu }_{1}sin{\mu }_{2}.$

Considered $n = 0:$

$\begin{array}{l} {u}_{1}\left({\mu }_{1}, {\mu }_{2}, \tau \right) = -i{S}^{-1}[{\left(\frac{\nu }{s}\right)}^{\alpha }S[-\frac{1}{2}\left[{\left({\theta }_{0}\right)}_{{\mu }_{1}{\mu }_{1}}\left({\mu }_{1}, {\mu }_{2}, \tau \right)+{\left({\theta }_{0}\right)}_{{\mu }_{2}{\mu }_{2}}\left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]\\ \;\;\; +\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}\right){\theta }_{0}\left({\mu }_{1}, {\mu }_{2}, \tau \right)+{A}_{0}]]. \end{array}$

Where

${\left({\theta }_{0}\right)}_{{\mu }_{1}{\mu }_{1}}\left({\mu }_{1}, {\mu }_{2}, \tau \right) = {\left({\theta }_{0}\right)}_{{\mu }_{2}{\mu }_{2}}\left({\mu }_{1}, {\mu }_{2}, \tau \right) = -sin{\mu }_{1}sin{\mu }_{2},$

and

${A}_{0} = {\theta }_{0}^{2}\stackrel{-}{{\theta }_{0}} = {\mathrm{sin}}^{3}{\mu }_{1}{\mathrm{sin}}^{3}{\mu }_{2}$

$\Rightarrow {\theta }_{1}\left({\mu }_{1}, {\mu }_{2}, \tau \right)$

$= -i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[-\frac{1}{2}\left[-2sin{\mu }_{1}sin{\mu }_{2}\right]+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}\right)\left(sin{\mu }_{1}sin{\mu }_{2}\right)+{\mathrm{sin}}^{3}{\mu }_{1}{\mathrm{sin}}^{3}{\mu }_{2}\right]\right]$

$\Rightarrow {\theta }_{1}({\mu }_{1}, {\mu }_{2}, \tau ) = -i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[2sin{\mu }_{1}sin{\mu }_{2}\right]\right]$

${\Rightarrow \theta }_{1}({\mu }_{1}, {\mu }_{2}, \tau ) = -i\left(2sin{\mu }_{1}sin{\mu }_{2}\right){S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[1\right]\right]$

$\Rightarrow {\theta }_{1}({\mu }_{1}, {\mu }_{2}, \tau ) = -2isin{\mu }_{1}sin{\mu }_{2}{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{2\alpha }\right]$

${\Rightarrow \theta }_{1}\left({\mu }_{1}, {\mu }_{2}, \tau \right) = -2isin{\mu }_{1}sin{\mu }_{2}\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}.$

Considered $n = 1:$

$\begin{array}{c} {\theta }_{2}\left({\mu }_{1}, {\mu }_{2}, \tau \right) = -i{S}^{-1}[{\left(\frac{\nu }{s}\right)}^{\alpha }S[-\frac{1}{2}\left[{\left({u}_{1}\right)}_{{\mu }_{1}{\mu }_{1}}\left({\mu }_{1}, {\mu }_{2}, \tau \right) +{\left({u}_{1}\right)}_{{\mu }_{2}{\mu }_{2}}\left({\mu }_{1}, {\mu }_{2}, \tau \right)\right]\\+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}\right){u}_{1}\left({\mu }_{1}, {\mu }_{2}, \tau \right)+{A}_{1}]]. \end{array}$

Where,

${\left({\theta }_{1}\right)}_{{\mu }_{1}{\mu }_{1}} = {\left({\theta }_{1}\right)}_{{\mu }_{2}{\mu }_{2}} = 2isin{\mu }_{1}sin{\mu }_{2}\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)},$

${\left({\theta }_{1}\right)}_{{\mu }_{1}{\mu }_{1}}+{\left({\theta }_{1}\right)}_{{\mu }_{2}{\mu }_{2}} = 4isin{\mu }_{1}sin{\mu }_{2}\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)},$

${A}_{1} = 2{\theta }_{0}{\theta }_{1}\stackrel{-}{{\theta }_{0}}+{\theta }_{0}^{2}\stackrel{-}{{\theta }_{1}} = -2i{\mathrm{sin}}^{3}{\mu }_{1}{\mathrm{sin}}^{3}{\mu }_{2}\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}$

$\Rightarrow {\theta }_{2}({\mu }_{1}, {\mu }_{2}, \tau )$

$\begin{array}{l} = -i{S}^{-1}[{\left(\frac{\nu }{s}\right)}^{\alpha }S[-\frac{1}{2}\left[4isin{\mu }_{1}sin{\mu }_{2}\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}\right]+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}\right)\left(-2isin{\mu }_{1}sin{\mu }_{2}\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}\right)\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;+\left(-2i{\mathrm{sin}}^{3}x{\mathrm{sin}}^{3}y\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}\right)]] \end{array}$

$\Rightarrow {\theta }_{2}({\mu }_{1}, {\mu }_{2}, \tau ) = -i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left\{-4isin{\mu }_{1}sin{\mu }_{2}\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}\right\}\right]$

$\Rightarrow {\theta }_{2}({\mu }_{1}, {\mu }_{2}, \tau ) = 4{i}^{2}sin{\mu }_{1}sin{\mu }_{2}{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{3\alpha }\right]$

${\Rightarrow \theta }_{2}({\mu }_{1}, {\mu }_{2}, \tau ) = 4{i}^{2}sin{\mu }_{1}sin{\mu }_{2}\frac{{\tau }^{3\alpha -1}}{\mathrm{\Gamma }\left(3\alpha \right)}$

$\Rightarrow \theta \left({\mu }_{1}, {\mu }_{2}, \tau \right) = {u}_{0}\left({\mu }_{1}, {\mu }_{2}, \tau \right)+{u}_{1}\left({\mu }_{1}, {\mu }_{2}, \tau \right)+{u}_{2}\left({\mu }_{1}, {\mu }_{2}, \tau \right)+{u}_{3}\left({\mu }_{1}, {\mu }_{2}, \tau \right)+\dots$

$\Rightarrow \theta ({\mu }_{1}, {\mu }_{2}, \tau ) = sin{\mu }_{1}sin{\mu }_{2}-2isin{\mu }_{1}sin{\mu }_{2}\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}+4{i}^{2}sin{\mu }_{1}sin{\mu }_{2}\frac{{\tau }^{3\alpha -1}}{\mathrm{\Gamma }\left(3\alpha \right)}-\dots$

Considered $\alpha = 1:$

$\Rightarrow \theta ({\mu }_{1}, {\mu }_{2}, \tau ) = sin{\mu }_{1}sin{\mu }_{2}\left[1-\frac{2i\tau }{1!}+\frac{{\left(2i\tau \right)}^{2}}{2!}-\dots \right]$

$\Rightarrow \theta \left({\mu }_{1}, {\mu }_{2}, \tau \right) = sin{\mu }_{1}sin{\mu }_{2}\;\mathrm{exp}\left(-2i\tau \right).$

Example 6: Considered $2D$ non-linear time-fractional Schr $\ddot{o}$ dinger equation as follows ^[34]:

$\begin{array}{l} i{D}_{\tau }^{\alpha }\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right) = -\frac{1}{2}\left[{\theta }_{{\mu }_{1}{\mu }_{1}}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)+{\theta }_{{\mu }_{2}{\mu }_{2}}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)+{\theta }_{{\mu }_{3}{\mu }_{3}}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right] \\ \;\;\;\;\;\; +\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}si{n}^{2}{\mu }_{3}\right)\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau ){\left|\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )\right|}^{2} . \end{array}$

(27)

Where ${\mu }_{1}, {\mu }_{2}, {\mu }_{3}\in \left[0, 2\pi \right]\times \left[0, 2\pi \right]\times \left[0, 2\pi \right].$

I.C.: $\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, 0\right) = sin{\mu }_{1}sin{\mu }_{2}sin{\mu }_{3}$

Applying Shehu transform in Eq (27):

$\begin{array}{l} iS\left[{D}_{t}^{\alpha }\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )\right] \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;= S [-\frac{1}{2}\left[{\theta }_{{\mu }_{1}{\mu }_{1}}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+{\theta }_{{\mu }_{2}{\mu }_{2}}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+{\theta }_{{\mu }_{3}{\mu }_{3}}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )\right]\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}si{n}^{2}{\mu }_{3}\right)\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau ){\left|\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )\right|}^{2}] \end{array}$

$\begin{array}{l} \Rightarrow S\left[{D}_{t}^{\alpha }\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )\right] \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;= -iS [-\frac{1}{2}\left[{\theta }_{{\mu }_{1}{\mu }_{1}}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+{\theta }_{{\mu }_{2}{\mu }_{2}}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+{\theta }_{{\mu }_{3}{\mu }_{3}}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )\right]\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}si{n}^{2}{\mu }_{3}\right)\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau ){\left|\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )\right|}^{2}] \end{array}$

$\begin{array}{l} {\Rightarrow \left(\frac{s}{\nu }\right)}^{\alpha }S\left[\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )\right]-\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{u}^{r}\left(x, y, z, 0\right)\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\; = -iS [-\frac{1}{2}\left[{\theta }_{{\mu }_{1}{\mu }_{1}}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+{\theta }_{{\mu }_{2}{\mu }_{2}}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+{\theta }_{{\mu }_{3}{\mu }_{3}}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )\right]\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}si{n}^{2}{\mu }_{3}\right)\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau ){\left|\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )\right|}^{2}] \end{array}$

$\begin{array}{l} \Rightarrow {\left(\frac{s}{\nu }\right)}^{\alpha }S\left[\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )\right] \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;= \sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left(x, y, z, 0\right)\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;-iS [-\frac{1}{2}\left[{\theta }_{{\mu }_{1}{\mu }_{1}}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+{\theta }_{{\mu }_{2}{\mu }_{2}}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+{\theta }_{{\mu }_{3}{\mu }_{3}}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )\right]\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}si{n}^{2}{\mu }_{3}\right)\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau ){\left|\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )\right|}^{2}] \end{array}$

$\begin{array}{l} \Rightarrow S\left[\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )\right] \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;= {\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, 0\right)\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;-i{\left(\frac{\nu }{s}\right)}^{\alpha }S [-\frac{1}{2}\left[{\theta }_{{\mu }_{1}{\mu }_{1}}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+{\theta }_{{\mu }_{2}{\mu }_{2}}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+{\theta }_{{\mu }_{3}{\mu }_{3}}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )\right]\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}si{n}^{2}{\mu }_{3}\right)\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau ){\left|\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )\right|}^{2}] \end{array}$

$\begin{array}{l} \Rightarrow \theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau ) \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;= {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, 0\right)\right]\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;-i{S}^{-1}[{\left(\frac{\nu }{s}\right)}^{\alpha }S[-\frac{1}{2}\left[{\theta }_{{\mu }_{1}{\mu }_{1}}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+{\theta }_{{\mu }_{2}{\mu }_{2}}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+{\theta }_{{\mu }_{3}{\mu }_{3}}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )\right]\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}si{n}^{2}{\mu }_{3}\right)\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau ){\left|\theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )\right|}^{2}]] \end{array}$

$\begin{array}{l} \Rightarrow \sum\limits_{n = 0}^{\infty }{\theta }_{n}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right) \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;= {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, 0\right)\right]\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;-i{S}^{-1}[{\left(\frac{\nu }{s}\right)}^{\alpha }S[-\frac{1}{2}[{\left(\sum\limits_{n = 0}^{\infty }{\theta }_{n}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right)}_{{\mu }_{1}{\mu }_{1}}+{\left(\sum\limits_{n = 0}^{\infty }{\theta }_{n}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right)}_{{\mu }_{2}{\mu }_{2}}\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;+{\left(\sum\limits_{n = 0}^{\infty }{\theta }_{n}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\right)}_{{\mu }_{3}{\mu }_{3}}]+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}si{n}^{2}{\mu }_{3}\right)\sum\limits_{n = 0}^{\infty }{\theta }_{n}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)+\sum\limits_{n = 0}^{\infty }{A}_{n}]], \end{array}$

${\theta }_{0}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }\sum\limits_{r = 0}^{\xi -1}{\left(\frac{s}{\nu }\right)}^{\alpha -r-1}{\theta }^{r}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, 0\right)\right],$

$\begin{array}{l} {\theta }_{n+1}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau ) \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;= -i{S}^{-1}[{\left(\frac{\nu }{s}\right)}^{\alpha }S[-\frac{1}{2}\left[{\left({\theta }_{n}\right)}_{{\mu }_{1}{\mu }_{1}}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+{\left({\theta }_{n}\right)}_{{\mu }_{2}{\mu }_{2}}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+{\left({\theta }_{n}\right)}_{{\mu }_{3}{\mu }_{3}}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )\right]\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}si{n}^{2}{\mu }_{3}\right){\theta }_{n}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+{A}_{n}]], n = 0, 1, 2, 3, \dots \end{array}$

Considered $\xi = 1$ :

$\Rightarrow {\theta }_{0}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau ) = {S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }{\left(\frac{s}{\nu }\right)}^{\alpha -1}\theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, 0\right)\right]$

$\Rightarrow {\theta }_{0}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau ) = \theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, 0\right)$

$\Rightarrow {\theta }_{0}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right) = sin{\mu }_{1}sin{\mu }_{2}sin{\mu }_{3}.$

Considered $n = 0:$

$\begin{array}{l} \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\; {\theta }_{1}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right) \\ = -i{S}^{-1}[{\left(\frac{\nu }{s}\right)}^{\alpha }S [-\frac{1}{2}[{\left({\theta }_{0}\right)}_{{\mu }_{1}{\mu }_{1}}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)+{\left({\theta }_{0}\right)}_{{\mu }_{2}{\mu }_{2}}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\\+{\left({\theta }_{0}\right)}_{{\mu }_{3}{\mu }_{3}}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)] +\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}si{n}^{2}{\mu }_{3}\right){\theta }_{0}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)+{A}_{0}]]. \end{array}$

Where

$\begin{array}{l} {\left({\theta }_{0}\right)}_{{\mu }_{1}{\mu }_{1}}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right) = {\left({\theta }_{0}\right)}_{{\mu }_{2}{\mu }_{2}}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right) = {\left({\theta }_{0}\right)}_{{\mu }_{3}{\mu }_{3}}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right) \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;= -sin{\mu }_{1}sin{\mu }_{2}sin{\mu }_{3}{\left({\theta }_{0}\right)}_{{\mu }_{1}{\mu }_{1}}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)+{\left({\theta }_{0}\right)}_{{\mu }_{2}{\mu }_{2}}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;{+\left({\theta }_{0}\right)}_{{\mu }_{3}{\mu }_{3}}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right) = -3sin{\mu }_{1}sin{\mu }_{2}sin{\mu }_{3}, \end{array}$

and

${A}_{0} = {\theta }_{0}^{2}\stackrel{-}{{\theta }_{0}} = {\mathrm{sin}}^{3}{\mu }_{1}{\mathrm{sin}}^{3}{\mu }_{2}{\mathrm{s}\mathrm{i}\mathrm{n}}^{3}{\mu }_{3}$

$\Rightarrow {\theta }_{1}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )$

$\begin{array}{l} = -i{S}^{-1}[{\left(\frac{\nu }{s}\right)}^{\alpha }S[-\frac{1}{2}\left[-3sin{\mu }_{1}sin{\mu }_{2}sin{\mu }_{3}\right]+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}si{n}^{2}{\mu }_{3}\right)\left(sin{\mu }_{1}sin{\mu }_{2}sin{\mu }_{3}\right)\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;+{\mathrm{sin}}^{3}{\mu }_{1}{\mathrm{sin}}^{3}{\mu }_{2}{\mathrm{sin}}^{3}{\mu }_{3}]] \end{array}$

$\Rightarrow {\theta }_{1}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau ) = -i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[\frac{5}{2}sin{\mu }_{1}sin{\mu }_{2}sin{\mu }_{3}\right]\right]$

$\Rightarrow {\theta }_{1}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau ) = -\frac{5i}{2}sin{\mu }_{1}sin{\mu }_{2}sin{\mu }_{3}{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left[1\right]\right]$

${\Rightarrow \theta }_{1}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau ) = -\frac{5i}{2}sin{\mu }_{1}sin{\mu }_{2}sin{\mu }_{3}{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{2\alpha }\right]$

${\Rightarrow \theta }_{1}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right) = -\frac{5i}{2}sin{\mu }_{1}sin{\mu }_{2}sin{\mu }_{3}\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}.$

Considered $n = 1:$

$\begin{array}{l} {\theta }_{2}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right) \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;= -i{S}^{-1}[{\left(\frac{\nu }{s}\right)}^{\alpha }S[-\frac{1}{2}[{\left({\theta }_{1}\right)}_{{\mu }_{1}{\mu }_{1}}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)+{\left({\theta }_{1}\right)}_{{\mu }_{2}{\mu }_{2}}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;+{\left({\theta }_{1}\right)}_{{\mu }_{3}{\mu }_{3}}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)]+\left(1-si{n}^{2}{\mu }_{1}si{n}^{2}{\mu }_{2}si{n}^{2}{\mu }_{3}\right){\theta }_{1}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)+{A}_{1}]]. \end{array}$

Where,

${\left({\theta }_{1}\right)}_{{\mu }_{1}{\mu }_{1}}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right) = {\left({\theta }_{1}\right)}_{{\mu }_{2}{\mu }_{2}}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right) = {\left({\theta }_{1}\right)}_{{\mu }_{3}{\mu }_{3}}\left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right)$

$= \left(\frac{5i}{2}\right)sin{\mu }_{1}sin{\mu }_{2}sin{\mu }_{3}\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}.$

${A}_{1} = 2{\theta }_{0}{\theta }_{1}\stackrel{-}{{\theta }_{0}}+{\theta }_{0}^{2}\stackrel{-}{{\theta }_{1}} = -\frac{5i}{2}{\mu }_{1}{\mathrm{sin}}^{3}{\mu }_{2}{\mathrm{sin}}^{3}{\mu }_{3}\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}$

$\Rightarrow {\theta }_{2}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau ) = -i{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{\alpha }S\left\{-\frac{25i}{4}sin{\mu }_{1}sin{\mu }_{2}sin{\mu }_{3}\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}\right\}\right]$

$\Rightarrow {\theta }_{2}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau ) = \frac{25{i}^{2}}{4}sin{\mu }_{1}sin{\mu }_{2}sin{\mu }_{3}{S}^{-1}\left[{\left(\frac{\nu }{s}\right)}^{3\alpha }\right]$

$\Rightarrow {\theta }_{2}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau ) = \frac{25{i}^{2}}{4}sin{\mu }_{1}sin{\mu }_{2}sin{\mu }_{3}\frac{{\tau }^{3\alpha -1}}{\mathrm{\Gamma }\left(3\alpha \right)}$

$\Rightarrow \theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau ) = {\theta }_{0}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+{\theta }_{1}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+{\theta }_{2}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+{\theta }_{3}({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau )+\dots$

$\Rightarrow \theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau ) = sin{\mu }_{1}sin{\mu }_{2}sin{\mu }_{3}-\frac{5i}{2}sin{\mu }_{1}sin{\mu }_{2}sin{\mu }_{3}\frac{{\tau }^{2\alpha -1}}{\mathrm{\Gamma }\left(2\alpha \right)}+\frac{25{i}^{2}}{4}sin{\mu }_{1}sin{\mu }_{2}sin{\mu }_{3}\frac{{\tau }^{3\alpha -1}}{\mathrm{\Gamma }\left(3\alpha \right)}-\dots$

Considered $\alpha = 1:$

$\Rightarrow \theta ({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau ) = sin{\mu }_{1}sin{\mu }_{2}sin{\mu }_{3}\left[1-\frac{\left(\frac{5i\tau }{2}\right)}{1!}+\frac{{\left(\frac{5i\tau }{2}\right)}^{2}}{2!}-\dots \right]$

$\Rightarrow \theta \left({\mu }_{1}, {\mu }_{2}, {\mu }_{3}, \tau \right) = sin{\mu }_{1}sin{\mu }_{2}sin{\mu }_{3}\;\mathrm{exp}\left(-\frac{5i\tau }{2}\right).$

5. Graphical and tabular discussion

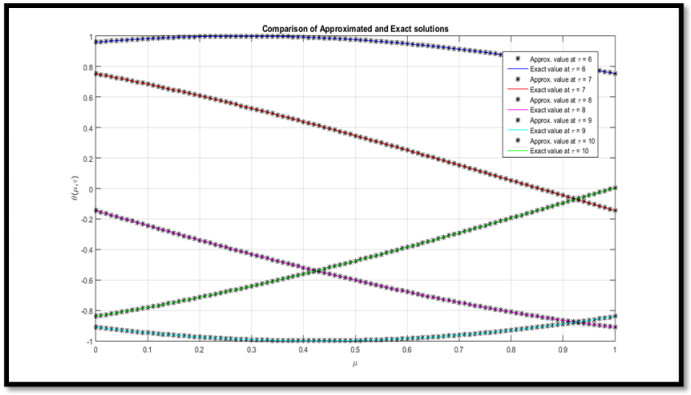
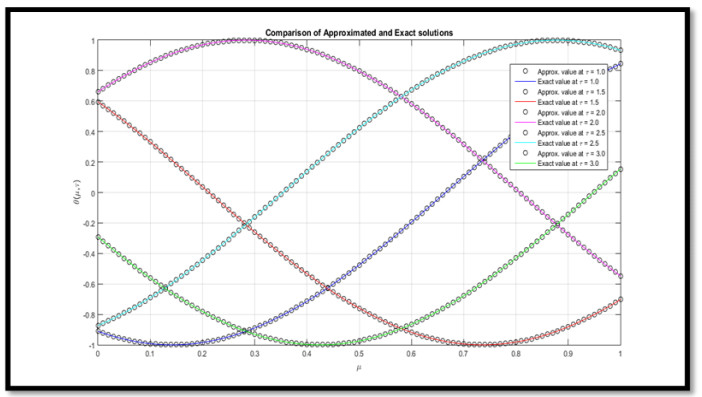
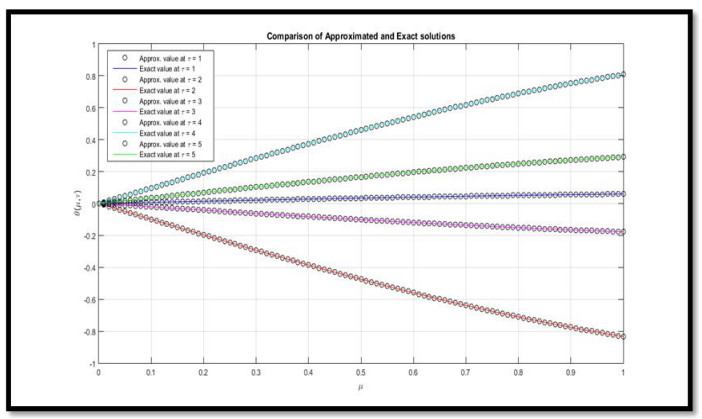
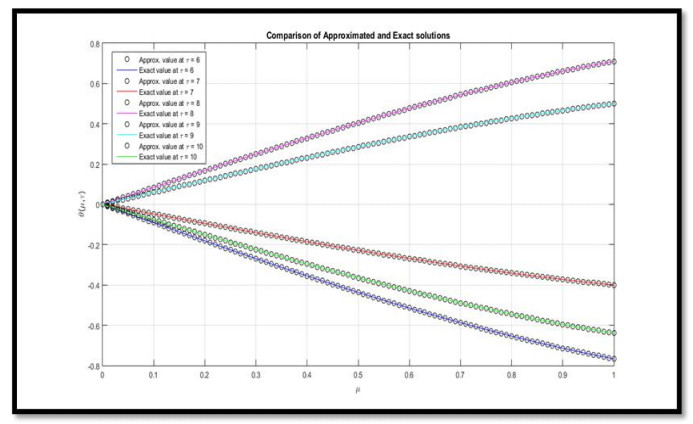
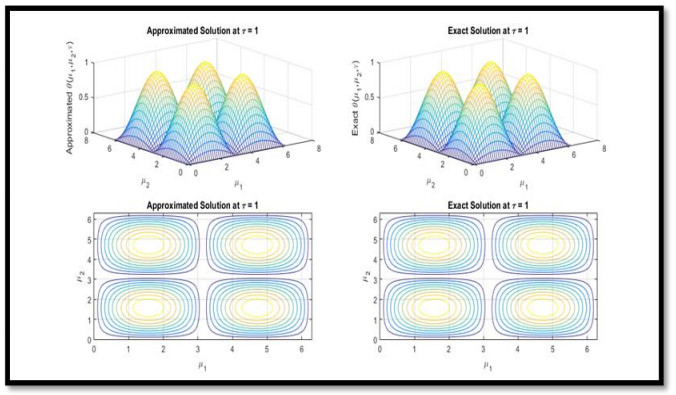
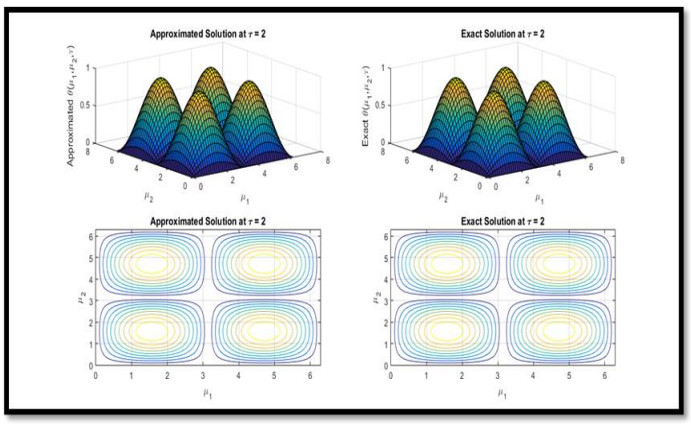
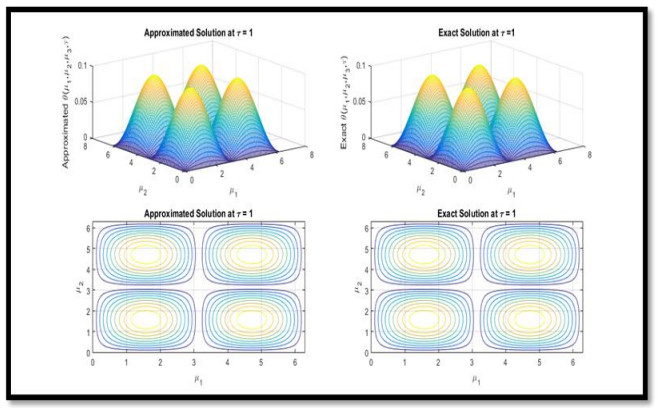
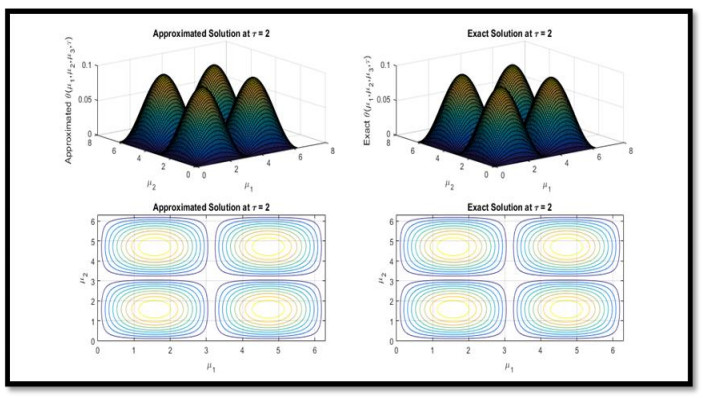
In the present section, the validity of the proposed regime is affirmed vias graphical and tabular analysis of the results. In , approx. and exact profiles are matched at $\tau$ = 1, 2, 3, 4, and 5 for Example 1. In , matching of approx. and exact profiles are provided at $\tau$ = 6, 7, 8, 9, and 10 for Example 1. A wide range of time levels is checked for compatibility. In , a match of approx. and exact profiles is provided at $\tau$ = 1.0, 1.5, 2.0, 2.5 and 3.0 for Example 2. is related to the approx. and exact profile compatibility at $\tau$ = 1, 2, 3, 4, and 5 for Example 3. In , compatibility of approx. and exact profiles are matched at $\tau$ = 1, 2, 3, 4, and 5 for Example 4. In , approx.-exact compatibility is mentioned at $\tau$ = 6, 7, 8, 9, and 10 for Example 4. and are related to the mesh-contour and surface-contour presentations at $\tau$ = 1 and $\tau$ = 2 respectively for Example 5. and are related to the mesh-contour and surface-contour presentations at $\tau$ = 1 and $\tau$ = 2, respectively for Example 6.

Figure 1. Approx. and exact profiles at

$t$ = 1, 2, 3, 4 and 5 regarding Example 1.

DownLoad: Full-Size Img PowerPoint

Figure 2. Approx. and exact profiles at

$t$ = 6, 7, 8, 9, and 10 where

$N$ = 101 regarding Example 1.

DownLoad: Full-Size Img PowerPoint

Figure 3. Approx. and exact profiles at

$t$ = 1.0, 1.5, 2.0, 2.5, and 3.0 where

$N$ = 101 regarding Example 2.

DownLoad: Full-Size Img PowerPoint

Figure 4. Approx. and exact profiles at

$t$ = 1, 2, 3, 4, and 5 where

$N$ = 101 regarding Example 3.

DownLoad: Full-Size Img PowerPoint

Figure 5. Approx. and exact profiles at

$t$ = 1, 2, 3, 4, and 5 where

$N$ = 101 regarding Example 4.

DownLoad: Full-Size Img PowerPoint

Figure 6. Approx. and exact profiles at

$t$ = 6, 7, 8, 9, and 10 where

$N$ = 101 regarding Example 4.

DownLoad: Full-Size Img PowerPoint

Figure 7. Approx. and exact profiles at

$t$ = 1 where

$N$ = 51 regarding Example 5.

DownLoad: Full-Size Img PowerPoint

Figure 8. Approx. and exact profiles at

$t$ = 2 where

$N$ = 51 regarding Example 5.

DownLoad: Full-Size Img PowerPoint

Figure 9. Approx. and exact profiles at

$t$ = 1, where

$N$ = 101,

$z$ = 0.1 regarding Example 6.

DownLoad: Full-Size Img PowerPoint

Figure 10. Approx. and exact profiles at

$t$ = 2 where

$N$ = 101,

$z$ = 0.1 regarding Example 6.

DownLoad: Full-Size Img PowerPoint

Via Tables 3–8, it can be affirmed that the approx. and exact profiles are matched for a wide range of time levels regarding the proposed scheme. Error analysis and convergence property are done by means of Tables 3–. In –, ${L}_{\infty }$ errors are provided at various grid points and time levels. Via –, it is reported that on increasing the number of grid points at different time levels, ${L}_{\infty }$ error got reduced, and in most of the cases, convergence is affirmed up to higher order.

6. Conclusions

In the present study, the motive is to deal with $1D$ , $2D$ , and $3D$ time-fractional Schr $\ddot{o}$ dinger equations regarding the approx.-analytical outcome. Shehu transform ADM is incorporated for this purpose. The prime key of a developed regime is it's easy-to-implement approach and accurate results. Furthermore, with the aid of the six mentioned examples, it is ensured that the retrieved approximated profiles are compatible with exact profiles. The graphical matching aspect between the approximated and exact results is also notified, which ensures that the developed technique is a good alternative to solve complex natured time-fractional PDEs. ${L}_{\infty }$ error is mentioned in–. Via mentioned tables, it is ensured that the proposed methodology is convergent, as on increasing the number of grid points, ${L}_{\infty }$ error got reduced. With the aid of the developed regime, various complex natured fractional PDEs can be easily solved.

Acknowledgments

This research received funding support from the NSRF via the Program Management Unit for Human Resources & Institutional Development, Research and Innovation (grant number B05F640092).

Conflict of interest

The authors declare no conflict of interest.

References

[1]	S. E. Abbas, A. H. Zakaria, Lattice valued double fuzzy ideal structure, J. Fuzzy Math., 20 (2012), 899–910.
[2]	S. E. Abbas, M. A. Hebeshi, I. M. Taha, On fuzzy upper and lower semi-continuous multifunctions, J. Fuzzy Math., 22 (2014), 951–962.
[3]	S. E. Abbas, M. A. Hebeshi, I. M. Taha, On upper and lower almost weakly continuous fuzzy multifunctions, Iran. J. Fuzzy Syst., 12 (2015), 101–114.
[4]	S. E. Abbas, M. A. Hebeshi, I. M. Taha, On upper and lower contra-continuous fuzzy multifunctions, Punjab Uni. J. Math., 47 (2015), 105–117.
[5]	S. E. Abbas, I. Ibedou, Fuzzy topological concepts via ideals and grills, Ann. Fuzzy Math. Inform., 15 (2018), 137–148.
[6]	M. N. A. Shugair, A. A. Abdallah1, S. E. Abbas, E. El-Sanowsy, I. Ibedou, Double fuzzy ideal multifunctions, Mathematics, 2024 (2024), 1128.
[7]	J. Albrycht, M. Maltoka, On fuzzy multivalued functions, Fuzzy Set. Syst., 12 (1984), 61–69.
[8]	K. T. Atanassov, New operations defined over the intuitionistic fuzzy sets, Fuzzy Set. Syst., 61 (1994), 137–142.
[9]	C. L. Chang, Fuzzy topological spaces, J. Math. Anal. Appl., 24 (1968), 182–190.
[10]	D. Coker, An introduction to intuitionistic fuzzy topological spaces, Fuzzy Set. Syst., 88 (1997), 81–89.
[11]	J. G. Garcia, S. E. Rodabaugh, Order-theoretic, topological, categorical redundancies of interval-valued sets, grey sets, vague sets, interval-valued intuitionistic sets, intuitionistic fuzzy sets and topologies, Fuzzy Set. Syst., 156 (2005), 445–484.
[12]	A. Ghareeb, Normality of double fuzzy topological spaces, Appl. Math. Lett., 24 (2011), 533–540.
[13]	M. A. Hebeshi, I. M. Taha, On upper and lower $\alpha$ -continuous fuzzy multifunctions, J. Intell. Fuzzy Syst., 25 (2015), 2537–2546.
[14]	T. Kubiak, On fuzzy topologies, Ph.D. dissertation, Adam Mickiewicz University, Poznan, Poland, 1985.
[15]	F. M. Mohammed, M. S. M. Noorani, A. Ghareeb, Somewhat slightly generalized double fuzzy semi continuous functions, Int. J. Math. Math. Sci., 2014 (2014), 756376.
[16]	T. K. Mondal, S. K. Samanta, On intuitionistic gradation of openness, Fuzzy Set. Syst., 131 (2002), 323–336.
[17]	N. Rajesh, M. Vanishree, B. Brundha, Double fuzzy contra-continuous multifunctions, Malaya J. Mat., 7 (2019), 862–866.
[18]	A. P. Sostak, On a fuzzy topological structure, In: Proceedings of the 13th Winter School on Abstract Analysis, Palermo, Italy: Circolo Matematico di Palermo, 1985, 89–103.
[19]	A. P. Sostak, Basic structures of fuzzy topology, J. Math. Sci., 78 (1996), 662–701.
[20]	I. M. Taha, On fuzzy upper and lower $\alpha$ - $\ell$ -continuity and their decomposition, J. Math. Comput. Sci., 11 (2021), 427–441.
[21]	E. Tsiporkova, B. De Baets, E. Kerre, A fuzzy inclusion based approach to upper inverse images under fuzzy multivalued mappings, Fuzzy Set. Syst., 85 (1997), 93–108.
[22]	E. Tsiporkova, B. De Baets, E. Kerre, Continuity of fuzzy multivalued mappings, Fuzzy Set. Syst., 94 (1998), 335–348.
[23]	L. A. Zadeh, Fuzzy sets, Inform. Control, 8 (1965), 338–353.

This article has been cited by:

1.	Nourhane Attia, Ali Akgül, Djamila Seba, Abdelkader Nour, Manuel De la Sen, Mustafa Bayram, An Efficient Approach for Solving Differential Equations in the Frame of a New Fractional Derivative Operator, 2023, 15, 2073-8994, 144, 10.3390/sym15010144
2.	Mamta Kapoor, Samanyu Khosla, Series Solution to Fractional Telegraph Equations Using an Iterative Scheme Based on Yang Transform, 2024, 0971-3514, 10.1007/s12591-024-00679-w
3.	Mamta Kapoor, Varun Joshi, A comparative study of Sumudu HPM and Elzaki HPM for coupled Burgers’ equation, 2023, 9, 24058440, e15726, 10.1016/j.heliyon.2023.e15726
4.	M. L. Rupa, K. Aruna, Optical solitons of time fractional Kundu–Eckhaus equation and massive Thirring system arises in quantum field theory, 2024, 56, 0306-8919, 10.1007/s11082-023-05914-2

Reader Comments

Your name:*

Email:*
© 2024 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

AIMS Mathematics

1.8 3.4

Metrics

Article views(743) PDF downloads(29) Cited by(1)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

AIMS Mathematics

Double fuzzy $\alpha$ - $\eth$ -continuous multifunctions

Related Papers:

Abstract

1. Introduction

2. Outline of the paper

3. Implementation of the proposed regime

3.1. Implementation of the scheme on $1{D}$ time-fractional Schr $\ddot{{o}}$ dinger equation

3.2. Implementation of the scheme on $2{D}$ time-fractional Schr $\ddot{{o}}$ dinger equation

3.3. Implementation of the scheme on $3{D}$ time-fractional Schr $\ddot{{o}}$ dinger equation

4. Examples and derivation

5. Graphical and tabular discussion

6. Conclusions

Acknowledgments

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Other Articles By Authors

Catalog

AIMS Mathematics

Double fuzzy α \alpha -ð \eth -continuous multifunctions

Related Papers:

Abstract

1. Introduction

2. Outline of the paper

3. Implementation of the proposed regime

3.1. Implementation of the scheme on 1D 1{D} time-fractional Schr¨o \ddot{{o}} dinger equation

3.2. Implementation of the scheme on 2D 2{D} time-fractional Schr¨o \ddot{{o}} dinger equation

3.3. Implementation of the scheme on 3D 3{D} time-fractional Schr¨o \ddot{{o}} dinger equation

4. Examples and derivation

5. Graphical and tabular discussion

6. Conclusions

Acknowledgments

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog

Double fuzzy $\alpha$ - $\eth$ -continuous multifunctions

3.1. Implementation of the scheme on $1{D}$ time-fractional Schr $\ddot{{o}}$ dinger equation

3.2. Implementation of the scheme on $2{D}$ time-fractional Schr $\ddot{{o}}$ dinger equation

3.3. Implementation of the scheme on $3{D}$ time-fractional Schr $\ddot{{o}}$ dinger equation