Detecting affine equivalences between certain types of parametric curves, in any dimension

1.   Introduction

We say that two curves are affinely equivalent if one of them is the image of the other curve by means of an affine mapping. If the affine mapping preserves angles, then the two curves are similar, i.e., both correspond to the same shape and differ only in position and/or scaling. If the affine mapping preserves distances, the curves are congruent or isometric, so they differ only in position. Finally, if the two curves coincide, finding the self-congruences of the curve is equivalent to computing its (Euclidean) symmetries.

Because of the nature of the problem, it has received much attention in applied fields like computer aided geometric design, pattern recognition and computer vision. In the last years, however, the problem has been also addressed in the computer algebra field, and here we follow this trend. Examples of computer algebra papers where this question has been studied are ^[2,4,7,10]; for other related papers, the interested reader can check the bibliographies of ^[2,4,7,10]. These papers address the problem for rational curves, i.e., parametric curves whose components are quotients of polynomial functions; while ^[2] only considers symmetries, and ^[4,7,10] aim for the more general question of checking projective, and not just affine, equivalence. In ^[4,10], we can find solutions for this problem with different strategies for curves in any dimension and use it as a fundamental tool for polynomial system solving, whereas the paper ^[7] addresses the question only for space rational curves, but employing bivariate factoring as an alternative to solving polynomial systems; this leads to better timings and performance. To do this, in ^[7], two rational invariants, i.e., two functions rationally depending on the parametrizations and their derivatives to be studied, which stay invariant under projective transformations, are found and used.

In this paper, we generalize the ideas of ^[7] in three different ways. First, while the development of the invariants in ^[7] was more of an "art" than a "craft", here we provide a complete algorithm to generate such invariants. Second, the technique is valid for curves in any dimension, and not just space curves, which was the case addressed in ^[7]: We provide an algorithm that can be downloaded from ^[8] to generate the corresponding invariants for any dimension, that needs to be executed just once for each dimension. Third, our strategy is valid not only for rational curves, but also for non-algebraic, parametric curves with meromorphic components under certain conditions, so we can apply the algorithm for catenary curves or 3D spirals, for instance, whenever some hypotheses are fulfilled. In order to also include this type of non-algebraic curves, we stick to affine equivalences, and not projective equivalences, although our ideas could be developed in a projective setting for rational curves. Notice that strategies like ^[4,10], based on polynomial system solving, cannot handle non-algebraic curves because we would need to solve systems involving analytic functions.

Although the implementation of our algorithm is relatively simple, and can be downloaded from ^[8] jointly with the examples worked out in this paper, justifying how the invariants are generated is involved, and extremely technical; because of this, in this paper we focus on the ideas and the algorithm itself, and refer the interested readers to the ArXiv version of the paper ^[3] for the detailed deduction of the invariants.

The structure of this paper is the following. In Section 2, we review some previous work related to the problem in order to provide some intuition on our solution. Also in that section, we make precise the kind of curves we can work with and present some necessary tools to understand the algorithm. In Section 3, we develop the method and give the results leading to the algorithm; here, we focus on the ideas and theorems and skip the (very technical) details, for which the interested reader is referred to ^[3]. Finally, the algorithm itself, together with several examples and an account of the experimentation carried out in ${\mathtt{Maple}}$, is given in Section 4. We close the paper with our conclusion and some open questions in Section 5.

2.   Background, statement of the problem and required tools

2.1.   Review of previous work

The fundamental theorem of space curves ^[5] states that the curvature $\kappa_{{{\boldsymbol{p}}}}$ and the torsion $\tau_{{{\boldsymbol{p}}}}$ of a space curve ${\mathcal C}$ defined by a parametrization ${{\boldsymbol{p}}}$,

where $|\bullet|$ denotes the norm and $\Vert \bullet\Vert$ represents the determinant, define the curve up to congruences. As a consequence, if we are given two curves ${\mathcal C}_1, {\mathcal C}_2$ defined by parametrizations ${{\boldsymbol{p}}}, {{\boldsymbol{q}}}$, we can check whether the corresponding curves are congruent by checking whether or not their curvature and torsion coincide. Thus, we say that $\kappa_{{{\boldsymbol{p}}}}, \tau_{{{\boldsymbol{p}}}}$ are invariants for congruences, in the sense that $\kappa_{{{\boldsymbol{p}}}}, \tau_{{{\boldsymbol{p}}}}$ stay invariant when a congruence is applied. Additionally, $\tau_{{{\boldsymbol{p}}}}$ and also $\kappa^2_{{{\boldsymbol{p}}}}$ (instead of $\kappa_{{{\boldsymbol{p}}}}$) are said to be rational invariants because they correspond to invariant (under congruences), rational expressions in ${{\boldsymbol{p}}}$ and its derivatives.

Curvature and torsion are used in ^[2] to compute the Euclidean symmetries of a rational space curve, i.e., a curve defined by a rational parametrization (so that their components are quotients of polynomials). The reason is the following theorem, used in ^[2] and other papers treating similar questions, like ^[4,7,10]. Two ingredients are important in this theorem: the first one is that it is required that the parametrizations of the curves are proper, i.e., birational, meaning that they are invertible and have rational inverses. This hypothesis is easy to check ^[17]; also, if a parametrization is not proper, it can be properly reparametrized ^[17]. The second ingredient is the fact (see also ^[17]) that the only birational mappings of the real or complex line are the Möbius transformations,

Theorem 2.1. Let ${{\mathit{\boldsymbol{C}}}}_1, {{\mathit{\boldsymbol{C}}}}_2\subset {\Bbb C}^n$ be two parametric curves defined by rational proper parametrizations ${{\boldsymbol{p}}}(z), {{\boldsymbol{q}}}(z)$. If $f$ is a birational mapping such that $f({{\mathit{\boldsymbol{C}}}}_1) = {{\mathit{\boldsymbol{C}}}}_2$, then there exists a Möbius transformation $\varphi(z)$ satisfying that

i.e., making commutative the following diagram:

The reason why curvature and torsion are useful when applying the above theorem for $f$ a Euclidean symmetry, i.e., an isometry, is not only that they are rational invariants, but the fact that they satisfy the following relationships: If ${{\boldsymbol{p}}}$ is a parametization and $\varphi$ is a Möbius transformation, then ^[2]

We refer to this property by saying that the curvature and torsion commute with Möbius transformations. This allows us to recover the symmetries of a rational curve by using bivariate factoring ^[2], where the factors we are looking for are the Möbius-like factors $H(z, \omega) = \omega (cz+d)-(az+b)$. This immediately provides us with the Möbius transformations $\varphi$ in Theorem 2.1; the transformations $f$ themselves are then computed from Eq (2.2).

This strategy is generalized, also for space curves, in ^[7] in order to find the projective equivalences, if any, between two space rational curves defined by proper, rational parametrizations ${{\boldsymbol{p}}}(z), {{\boldsymbol{q}}}(z)$. We say that two curves ${{\mathit{\boldsymbol{C}}}}_1, {{\mathit{\boldsymbol{C}}}}_2$ are projectively equivalent if there exists a projective mapping $f$ such that $f({{\mathit{\boldsymbol{C}}}}_1) = {{\mathit{\boldsymbol{C}}}}_2$, in which case we call $f$ a projective equivalence between the curves. If we are interested in computing projective equivalences, the curvature and torsion are no longer useful, and we need to produce other rational invariants, namely invariants under the group of projective transformations. Furthermore, since projective mappings are birational, Theorem 2.1 can be applied, but to generalize the strategy of ^[2] and use also bivariate factoring to solve the problem, we need to produce rational invariants which commute with Möbius transformations. This is exactly the task carried out in ^[7], for rational space curves.

2.2.   Making the input and the problem precise

In this paper we want to generalize the approach of ^[7] in two different directions, namely the dimension, so that now we aim at curves living in any dimension $n$ and not only space curves, and the class of curves, which will be not only the class of rational curves but also a more general class of non-algebraic curves parametrized by meromorphic functions, satisfying certain hypotheses. The key idea is that we want to work in a more general setup, but where Theorem 2.1 is still valid.

Because we want to include nonalgebraic curves, we will limit ourselves to affine equivalences, and not projective equivalences. Given two curves ${{\mathit{\boldsymbol{C}}}}_1, {{\mathit{\boldsymbol{C}}}}_2\subset{\Bbb C}^n$ we say that ${{\mathit{\boldsymbol{C}}}}_1, {{\mathit{\boldsymbol{C}}}}_2$ are affinely equivalent if there exists a mapping $f:{\Bbb C}^n\to {\Bbb C}^n$, $f({{\boldsymbol{x}}}) = A{{\boldsymbol{x}}}+{{\boldsymbol{b}}}$ with $A\in {\mathcal M}_{n\times n}({\Bbb C})$, i.e., $A$ is an $n\times n$ matrix (in general, over the complex), $A$ nonsingular, ${{\boldsymbol{b}}}\in {\Bbb C}^n$, such that $f({{\mathit{\boldsymbol{C}}}}_1) = {{\mathit{\boldsymbol{C}}}}_2$; furthermore, we say that $f$ is an affine equivalence between ${{\mathit{\boldsymbol{C}}}}_1, {{\mathit{\boldsymbol{C}}}}_2$. Although for technical reasons we will consider ${{\mathit{\boldsymbol{C}}}}_1, {{\mathit{\boldsymbol{C}}}}_2\subset{\Bbb C}^n$, we will mostly work with real curves, i.e., curves with infinitely many real points, and we will be interested in real affine equivalences; thus, we will be mostly looking at the case when $A\in {\mathcal M}_{n\times n}({\Bbb R})$, ${{\boldsymbol{b}}}\in {\Bbb R}^n$. If ${{\mathit{\boldsymbol{C}}}}_1 = {{\mathit{\boldsymbol{C}}}}_2 = {{\mathit{\boldsymbol{C}}}}$ is a real curve and $A, {{\boldsymbol{b}}}$ are real with $A$ an orthogonal matrix, so $A^TA = I$ where $I$ is the identity matrix, then we say that $f$ is a (Euclidean) symmetry of ${{\mathit{\boldsymbol{C}}}}$.

Remark 2.1. Notice that in order to work with projective equivalences we need to work with homogeneous parametrizations. However, while the homogenization of a rational parametrization is a well-defined notion, the same notion is not well-defined for nonrational, meromorphic parametrizations, since we lack a notion of degree.

In order to enlarge the class of curves to deal with, which will include as a subset the class of rational curves, we need to take a closer look to the hypotheses in Theorem 2.1. Essentially, we need two things: (1) to guarantee that the parametrizations ${{\boldsymbol{p}}}(z), {{\boldsymbol{q}}}(z)$ of the curves ${{\mathit{\boldsymbol{C}}}}_1, {{\mathit{\boldsymbol{C}}}}_2$ have global inverses, so the diagram in Eq (2.3) is commutative; (2) to guarantee that the mapping $\varphi$ at the bottom of Eq (2.3) is a Möbius transformation. An essential observation is that Möbius transformations are not only the birational transformations of the complex line, but the bimeromorphic transformations of the complex line (see Remark 2 in ^[1]); recall that a transformation $g:{\Bbb C}\to {\Bbb C}$ is bimeromorphic iff $g$ is meromorphic, and has an inverse $g^{-1}$ which is also meromorphic. Since the commutativity of the diagram Eq (2.3) implies that $\varphi = {{\boldsymbol{q}}}^{-1}\circ f \circ {{\boldsymbol{p}}}$, $\varphi^{-1} = {{\boldsymbol{p}}}^{-1}\circ f^{-1}\circ {{\boldsymbol{q}}}$, if ${{\boldsymbol{p}}}, {{\boldsymbol{q}}}$ are bimeromorphic parametrizations, Theorem 2.1 works perfectly replacing the hypothesis that ${{\boldsymbol{p}}}, {{\boldsymbol{q}}}$ are proper rational parametrizations, by the hypothesis that ${{\boldsymbol{p}}}, {{\boldsymbol{q}}}$ are bimeromorphic parametrizations. We state this as a theorem.

Theorem 2.2. Let ${{\mathit{\boldsymbol{C}}}}_1, {{\mathit{\boldsymbol{C}}}}_2\subset {\Bbb C}^n$ be two parametric curves defined by bimeromorphic parametrizations ${{\boldsymbol{p}}}(z), {{\boldsymbol{q}}}(z)$. If $f$ is an affine mapping such that $f({{\mathit{\boldsymbol{C}}}}_1) = {{\mathit{\boldsymbol{C}}}}_2$, then there exists a Möbius transformation $\varphi(z)$ satisfying Eq (2.2), i.e., making commutative the diagram in Eq (2.3).

Notice that proper rational parametrizations are bimeromorphic, so we are definitely enlarging the class of curves we work with. However, while checking whether or not a rational parametrization is proper is easy and fast, checking whether a non-rational parametrization is bimeromorphic is extremely difficult. This is understandable, since it requires to verify whether a non-rational mapping admits a global inverse, which is a very hard problem. For this reason, we will present now a scheme, which includes rational parametrizations and a wider class of non-rational, meromorphic parametrizations, where the requirement of being bimeromorphic is guaranteed, and can be algorithmically checked. In order to do that, we start with a meromorphic function $\xi:{\Bbb C}\to {\Bbb C}$. Defining

we observe that $\Pi$ is an invertible function over its image, which is the graph ${\mathcal G}_\xi$ of the function $\xi$,

Indeed, for $(z, \omega)\in {\Bbb C}^2$, $\omega = \xi(z)$, we have $\Pi^{-1}(z, \omega) = z$. Next, consider a rational mapping $\Phi:{\Bbb C}^2\to {\Bbb C}^n$. If we compose these two mappings, we get a new mapping

which provides a parametrization ${{\boldsymbol{p}}}(z) = \Phi(z, \xi(z))$ of a curve ${{\mathit{\boldsymbol{C}}}}\subset {\Bbb C}^n$, which is the image of ${\mathcal G}_\xi$ under $\Phi$. Notice that ${{\boldsymbol{p}}}$ is a vector function with meromorphic components. Of course if $\xi$ is a rational function, ${{\boldsymbol{p}}}$ is just a rational parametrization. We will also assume that the curve defined by ${{\boldsymbol{p}}}$ is not contained in a hyperplane.

Now we want to impose sufficient conditions on $\Phi$ to ensure that ${{\boldsymbol{p}}}^{-1}$ exists and is meromorphic, in which case ${{\boldsymbol{p}}}$ is bimeromorphic. Of course, this holds when $\xi$ is rational and ${{\boldsymbol{p}}} = \Phi\circ \Pi$ is proper, since ${{\boldsymbol{p}}}$ is a proper rational parametrization. However let us see that this is also the case whenever $\Phi$ is a birational mapping, so that $\Phi^{-1}$ exists and is rational, and $\xi$ is a non-algebraic meromorphic function; we will see that this is also a condition that we can algorithmically verify. Thus, let us assume that $\Phi$ is a birational mapping, and let ${{\boldsymbol{p}}} = \Phi\circ \Pi$. Recall that the cardinality of the fiber of $\Phi$, which we denote by $\#(\Phi)$, is the number of points in the pre-image of $\Phi({\bf q})$ with ${\bf q}\in {\Bbb C}^2$ a generic point. The birationality of $\Phi$ is equivalent to $\#(\Phi) = 1$ (see for instance Proposition 7.16 in ^[9]), so we can check this condition by just picking a random point ${\bf q}$, and computing the number of points in the pre-image of $\Phi({\bf q})$. Furthermore, we have the following lemma, inspired by ^[15,16].

Lemma 2.1. Let $\Phi:{\Bbb C}^2\to {\Bbb C}^n$ be a birational mapping, then the set of points ${\bf q}\in {\Bbb C}^2$ such that $\#(\Phi({\bf q})) > 1$ is included in an algebraic variety ${\mathcal V}\subset{\Bbb C}^2$ of dimension at most 1.

Proof. Let

If $\Phi$ is birational, then $\Phi^{-1}$ exists and is rational, i.e.,

where for $i = 1, 2$,

with $A_i, B_i$ polynomials. The set of points ${\bf q}\in {\Bbb C}^2$ such that $\#(\Phi({\bf q})) > 1$ is included in the set ${\mathcal V}\subset {\Bbb C}^2$ where $\Phi^{-1}$ is not defined, ${\mathcal V}$ being the union of the sets defined by $(B_i\circ \Phi)(x_1, x_2) = 0$ with $i = 1, 2$, and $N_i(x_1, x_2) = 0$, $i = 1, 2$, where $N_i$ is the denominator of $(A_i\circ \Phi)(x_1, x_2)$. Notice that ${\mathcal V}$ is an algebraic planar curve.

Proposition 2.1. Assume that $\Phi$ is a birational mapping, $\xi$ is a non-algebraic, meromorphic function, ${\mathcal G}_\xi$ corresponds to Eq (2.4), and ${{\boldsymbol{p}}}(z) = (\Phi\circ \Pi)(z)$ as in Eq (2.5), then ${{\boldsymbol{p}}}(z)$ is invertible over ${{\mathit{\boldsymbol{C}}}} = \Phi({\mathcal G}_\xi)$, and the inverse ${{\boldsymbol{p}}}^{-1}$ has rational components. As a consequence, ${{\boldsymbol{p}}}(z)$ is bimeromorphic.

Proof. Since by assumption $\Phi$ is a birational mapping, i.e., $\#(\Phi) = 1$, $\Phi^{-1}$ exists and is rational. Next from Lemma 2.1 we have that $\#(\Phi)$ is constant except perhaps for the points of an algebraic variety ${\mathcal V}\subset {\Bbb C}^2$ of dimension at most one. Since $\xi$ is not an algebraic function, ${\mathcal G}_\xi$ is not an algebraic curve. Therefore, by the identity theorem (see Theorem 3.1.9 in ^[11]) ${\mathcal G}_\xi\cap {\mathcal V}$ is either finite, or infinite but without any accumulation point. Thus, for a generic point ${\bf q}\in {\mathcal G}_\xi$ we get that the cardinality of $\Phi({\bf q})$ is $1$, so $\Phi^{-1}$ is well-defined for almost all points in $\Phi({\mathcal G}_\xi)$, and ${{\boldsymbol{p}}}^{-1} = \Pi^{-1}\circ\Phi^{-1}$. Since $\Phi^{-1}, \Pi^{-1}$ are rational, ${{\boldsymbol{p}}}^{-1}$ is rational as well. Since every rational function is meromorphic, ${{\boldsymbol{p}}}^{-1}$ is meromorphic; and since ${{\boldsymbol{p}}}$ is meromorphic by construction, ${{\boldsymbol{p}}}$ is bimeromorphic.

Therefore, we have found a new class of parametric, non-rational curves, for which Theorem 2.2 holds. We formulate this as a corollary.

Corollary 2.1. Let ${{\mathit{\boldsymbol{C}}}}_1, {{\mathit{\boldsymbol{C}}}}_2\subset {\Bbb C}^n$ be two parametric curves defined by ${{\boldsymbol{p}}}(z), {{\boldsymbol{q}}}(z)$, where ${{\boldsymbol{p}}}(z), {{\boldsymbol{q}}}(z)$ are either proper rational parametrizations, or can be written as

where for $i = 1, 2$, $\Pi_i(z) = (z, \xi_i(z))$, $\xi_i(z)$ is a non-algebraic, meromorphic function, and $\Phi_i:{\Bbb C}^2\to {\Bbb C}^n$ is a birational mapping. If $f$ is an affine mapping such that $f({{\mathit{\boldsymbol{C}}}}_1) = {{\mathit{\boldsymbol{C}}}}_2$, then there exists a Möbius transformation $\varphi(z)$ satisfying Eq (2.2), i.e., making commutative the diagram in Eq (2.3).

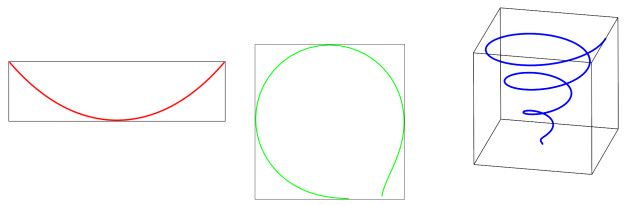
Thus, the curves meeting the hypotheses in Corollary 2.1 will be the curves that we will consider as the input to our problem. The next example provides some non-algebraic, planar and space, parametric curves satisfying the hypotheses in Corollary 2.1; these curves are plotted in Figure 1.

Example 2.1. The following curves satisfy the requirements in Corollary 2.1.

(1) Catenary. Consider the planar curve ${{\mathit{\boldsymbol{C}}}}$ parametrized by

where $\mbox{cosh}$ denotes the hyperbolic cosine (see Figure 1, left). Here ${{\boldsymbol{p}}}(z) = (\Phi\circ \Pi)(z)$, with $\Pi(z) = (z, \xi(z))$, where $\xi(z) = \mbox{cosh}(z)$, which is meromorphic, and $\Phi(x, y) = (x, y)$, which is clearly birational. In fact, any other planar curve ${{\boldsymbol{p}}}(z) = (z, \xi(z))$ with $\xi(z)$ a non-algebraic, meromorphic function also satisfies the hypotheses in Corollary 2.1.

(2) Image of the graph of the exponential curve under an inversion. Let the planar curve ${{\mathit{\boldsymbol{C}}}}$ (see Figure 1, middle) be parametrized by

Here ${{\boldsymbol{p}}}(z) = (\Phi\circ \Pi)(z)$, with $\Pi(z) = (z, \xi(z))$, where $\xi(z) = e^z$, which is clearly meromorphic, and

which is an inversion from the origin, and therefore a birational mapping. In fact, substituting the above $\Phi$ by any other birational planar mapping (e.g., a projective or affine mapping), and the function $e^z$ by any other meromorphic function, we also get a curve satisfying our hypotheses.

(3) 3D spiral. Consider the space curve ${{\mathit{\boldsymbol{C}}}}$ parametrized by $(z\cos(z), z\sin(z), z)$, which is a 3D spiral (see Figure 1, right). Writing

the parametrization of ${{\mathit{\boldsymbol{C}}}}$ can be expressed as

Thus, ${{\boldsymbol{p}}}(z) = (\Phi\circ \Pi)(z)$, with $\Pi(z) = (z, \xi(z))$, where $\xi(z) = e^{{\bf i}z}$, which is meromorphic, and

which is a birational mapping.

Certainly, not all parametric curves meet the hypotheses of Corollary 2.1. In order to clarify our input curves, we present two examples of such curves.

Example 2.2. The following two curves do not satisfy the requirements in Corollary 2.1.

(1) Cycloid. Consider the planar curve ${{\mathit{\boldsymbol{C}}}}$ parametrized by $(z-\mbox{sin}(z), 1-\mbox{cos}(z))$. Using Eq (2.8), we can rewrite this parametrization as

We can see ${{\boldsymbol{p}}}(z)$ as ${{\boldsymbol{p}}}(z) = (\Phi\circ \Pi)(z)$, with $\Pi(z) = (z, e^{{\bf i}z})$, where $e^{{\bf i}z}$ is a meromorphic function, and

However, $\Phi(x, y)$ is a rational mapping, but it is not birational, since a generic point $(u, v) = (\Phi_1(x, y), \Phi_2(x, y))$ has two pre-images $(x, y)$; indeed, notice that imposing $v = \Phi_2(x, y)$ we get two different values for $y$.

(2) Spherical spiral. Consider the space curve ${{\mathit{\boldsymbol{C}}}}$ parametrized by

Using again Eq (2.8), we can write ${{\boldsymbol{p}}}(z) = (\Phi\circ \Pi)(z)$ with $\Pi(z) = (z, e^{{\bf i}z})$. However, $\Phi$ is no longer a rational function in this case, because of the presence of a square-root in the denominators.

Therefore, we are finally ready to state the problem that we want to solve.

Problem: Given two curves ${{\mathit{\boldsymbol{C}}}}_1, {{\mathit{\boldsymbol{C}}}}_2\subset {\Bbb C}^n$, not contained in hyperplanes, parametrized by mappings ${{\boldsymbol{p}}}(z), {{\boldsymbol{q}}}(z)$ satisfying the hypotheses in Corollary 2.1, compute the affine equivalences, if any, between ${{\mathit{\boldsymbol{C}}}}_1, {{\mathit{\boldsymbol{C}}}}_2$.

Notice that for these curves the diagram Eq (2.3) is commutative, and the function $\varphi(z)$ at the bottom of Eq (2.3) is a Möbius function. So in order to solve the problem generalizing the approach in ^[7], we need to find rational invariants, in any dimension. This will be addressed in Section 3.

2.3.   Additional tools

In this subsection we recall two notions that we will be using later in the paper. The first one is the Schwartzian derivative: Given a holomorphic function $f:{\Bbb C}\to {\Bbb C}$, the Schwartzian derivative ^[14] $S(f)$ of $f$ is

The Schwartzian derivative of any Möbius transformation is identically zero. The following lemma is a consequence of this.

Lemma 2.2. Let $\omega: = \varphi(z)$ a Möbius transformation, and let $\omega^{(k)}$ denote the $k$-th derivative of $\omega$ with respect to $k$. For $k\geq 3$,

Proof. Since the Schwartzian derivative of a Möbius transformation is identically zero, we get that

which corresponds to Eq (2.10) for $k = 3$, then the result follows by induction on $k$.

The second tool is the Lah number $L(k, m)$ (see for instance ^[12]),

which allows us to define the following function, which we spell here for future reference:

3.   Development of the method

3.1.   Overall strategy and first step

We want to exploit Eq (2.2) to first find the Möbius transformation $\varphi$, if any, and then derive $f$ from $\varphi$. If we expand Eq (2.2), we get

Our overall strategy will consist of three steps that we will refer to as steps (ⅰ)–(ⅲ), which somehow mimic the strategy in ^[7], although for a completely general dimension:

(ⅰ) Find initial invariants: We start by constructing certain functions $I_1, \ldots, I_n$ satisfying that $I_i({{\boldsymbol{p}}}) = I_i({{\boldsymbol{q}}}\circ \varphi)$, which are rational in the sense that they are rational functions of ${{\boldsymbol{p}}}$ and its derivatives. Since by Eq (3.1) we observe that ${{\boldsymbol{q}}}\circ \varphi$ is the image of ${{\boldsymbol{p}}}$ under an affine mapping $f({{\boldsymbol{x}}}) = A{{\boldsymbol{x}}}+{{\boldsymbol{b}}}$, we say that $I_1, \ldots, I_n$ are affine invariants, i.e., functions depending on a parametrization (and its derivatives) that stay the same when an affine transformation is applied.

(ⅱ) Find Möbius-commuting invariants: Recall, from Subsection 2.1 that we say that a function $F$ depending on a parametrization ${{\boldsymbol{u}}} = {{\boldsymbol{u}}}(z)$ and its derivatives is Möbius-commuting if for any Möbius function we have

The functions $I_i$ found in step (ⅰ) are not, in general, Möbius-commuting. Thus, in a second step we will compute Möbius-commuting functions $F_1, \ldots, F_{n-1}$ from the $I_i$. The $F_j$ not only satisfies that $F_j({{\boldsymbol{p}}}) = F_j({{\boldsymbol{q}}} \circ \varphi)$ for $j = 1, \ldots, n-1$, but they also satisfy that $F_j({{\boldsymbol{q}}}\circ \varphi) = F_j({{\boldsymbol{q}}})\circ \varphi$. In turn, for $j = 1, \ldots, n-1$ we have

Notice that while we have $n$ initial invariants $I_i$, we have $n-1$ Möbius-commuting invariants. Furthermore, the $F_j$ will also be rational invariants.

(ⅲ) Compute $\varphi$ using bivariate factoring, and derive $f$ from $\varphi$: Setting $\omega: = \varphi(z)$, the equalities $F_j({{\boldsymbol{p}}}) = F_j({{\boldsymbol{q}}})\circ \varphi$, after clearing denominators, are translated into $n-1$ conditions $M_j(z, \omega) = 0$, with $j = 1, \ldots, n-1$. The Möbius function $\varphi$ corresponds to a common factor of all the $M_j$, and the affine equivalence itself, $f({{\boldsymbol{x}}}) = A{{\boldsymbol{x}}}+{{\boldsymbol{b}}}$, follows from Eq (3.1).

In this subsection we will present step (ⅰ); the remaining steps will be described in the next subsections. Also, in the rest of the paper we will use the notation $[{{\boldsymbol{w}}}_1, \cdots, {{\boldsymbol{w}}}_n]$ for an $n\times n$ matrix whose columns are ${{\boldsymbol{w}}}_1, \ldots, {{\boldsymbol{w}}}_n\in {\Bbb C}^n$, and $\Vert {{\boldsymbol{w}}}_1, \cdots, {{\boldsymbol{w}}}_n\Vert$ for the determinant of the matrix $[{{\boldsymbol{w}}}_1, \cdots, {{\boldsymbol{w}}}_n]$.

The description of step (ⅰ) is analogous to Section 3.2 in ^[7]. Thus, here we focus on the main ideas, and refer the interested reader to ^[7] for details and proofs. Going back to Eq (2.2), let us write ${{\boldsymbol{u}}}: = {{\boldsymbol{p}}}(z)$, ${{\boldsymbol{v}}}: = ({{\boldsymbol{q}}}\circ \varphi)(z)$, so that Eq (3.1) becomes simply $A{{\boldsymbol{u}}}+{{\boldsymbol{b}}} = {{\boldsymbol{v}}}$. Repeatedly differentiating this equation with respect to $z$ yields $AD({{\boldsymbol{u}}}) = D({{\boldsymbol{v}}})$ where

i.e., $D({{\boldsymbol{u}}}), D({{\boldsymbol{v}}})$ are matrices whose columns consist of the first $n$ derivatives of ${{\boldsymbol{u}}}, {{\boldsymbol{v}}}$ with respect to $z$. Whenever ${{\boldsymbol{p}}}, {{\boldsymbol{q}}}$ and ${{\boldsymbol{u}}}, {{\boldsymbol{v}}}$ are not contained in hyperplanes, $D({{\boldsymbol{u}}}), D({{\boldsymbol{v}}})$ are invertible ^[18]. Thus, we can write $A = D({{\boldsymbol{v}}})(D({{\boldsymbol{u}}}))^{-1}$. Differentiating this equality with respect to $z$ and taking into account that $A$ is a constant matrix, we get that

Expanding the derivative in the left-hand side of the above equation, we arrive at

Denoting

one can check that

Next, let us define

Thus, $A_i({{\boldsymbol{u}}})$ is the result of replacing ${{\boldsymbol{u}}}^{(i)}$ in $\Vert {{\boldsymbol{u}}}' {{\boldsymbol{u}}}'' \cdots {{\boldsymbol{u}}}^{(n)}\Vert$ by ${{\boldsymbol{u}}}^{(n+1)}$. Finally, for $i = 1, \ldots, n$, let

which corresponds to the entries of the last column of $U$; notice that whenever ${{\boldsymbol{u}}}, {{\boldsymbol{v}}}$ are not contained in hyperplanes $\Delta({{\boldsymbol{u}}})$ is not identically zero ^[18], so the $I_i$ are well-defined.

By Eq (3.2), $U, V$ are equal and therefore their last columns coincide. Thus, $I_i({{\boldsymbol{u}}}) = I_i({{\boldsymbol{v}}})$ for $i = 1, \ldots, n$, i.e., $I_i({{\boldsymbol{p}}}) = I_i({{\boldsymbol{q}}}\circ \varphi)$, which, by Theorem 2.1, is a necessary condition for affine equivalence. The following result, analogous to Theorem 7 in ^[7] and which can be proved in a similar way using Corollary 2.1 shows that this condition is also sufficient.

Theorem 3.1. Let $\mathit{\boldsymbol{C}}_1, \mathit{\boldsymbol{C}}_2\subset {\Bbb C}^n$ be two curves, not contained in a hyperplane, parametrized by mappings $\mathit{\boldsymbol{p}}, \mathit{\boldsymbol{q}}$ satisfying the hypotheses in Corollary 2.1. If $\mathit{\boldsymbol{C}}_1, \mathit{\boldsymbol{C}}_2$ are affinely equivalent, then there exists a Möbius transformation $\varphi$ such that

for $i = 1, \ldots, n$.

In order to carry out step (ⅱ), which will be addressed in the next subsection, we need an auxiliary invariant, $I_0$, defined as

The following lemma proves that $I_{0}$ lies in the differential field spanned by $I_1, \ldots, I_n$.

Lemma 3.1. $I_{0} = \dfrac{dI_n}{dz}+I_{n-1}+I_n^2$.

Proof. Differentiating $I_n$ we get

Isolating $I_{0}$ from the above equality, we get $I_{0} = \dfrac{dI_n}{dz}+I_{n-1}+I_n^2$.

Since, according to Lemma 3.1, $I_0$ is generated by $I_1, \ldots, I_n$, the result in Theorem 3.1 also holds when we add $I_0$ to the list of the $I_i$s.

Corollary 3.1. Let $\mathit{\boldsymbol{C}}_1, \mathit{\boldsymbol{C}}_2\subset {\Bbb C}^n$ be two curves, not contained in a hyperplane, parametrized by mappings $\mathit{\boldsymbol{p}}, \mathit{\boldsymbol{q}}$ satisfying the hypotheses in Corollary 2.1. If $\mathit{\boldsymbol{C}}_1, \mathit{\boldsymbol{C}}_2$ are affinely equivalent then there exists a Möbius transformation $\varphi$ such that

for $i\in\{0, 1\ldots, n\}$.

3.2.   Second step (overview)

The $I_i$ developed in the previous section are not Möbius-commuting, i.e., $I_i({{\boldsymbol{q}}}\circ\varphi)\neq I_i({{\boldsymbol{q}}})\circ \varphi$; in other words, calling $\omega: = \varphi(z)$, $I_i({{\boldsymbol{q}}}(\omega))\neq I_i({{\boldsymbol{q}}})(\omega)$. For instance, in the case $n = 3$, expanding $I_i({{\boldsymbol{q}}}(\omega))$ for $i = 1, 2, 3$ we get that

where $\omega', \omega''$ are the first and second derivatives of $\omega = \varphi(z)$ with respect to $z$; to produce these equalities, we have taken into account the definition of $I_1, I_2, I_3$ as quotients of determinants, the chain rule, and the fact that, because of Eq (2.10) in Lemma 2.2, the derivatives of $\omega$ of order higher than 2 can be written in terms of $\omega', \omega''$. However, by eliminating $\omega', \omega''$ in Eq (3.10), one can show that

so that

is Möbius-commuting, i.e., $F({{\boldsymbol{q}}}\circ \varphi) = F({{\boldsymbol{q}}})\circ \varphi$.

One can certainly manipulate Eq (3.10) by hand to get rid of $\omega', \omega''$, reach Eq (3.11), and therefore find the invariant in Eq (3.12). However, we want to produce invariants like the one in Eq (3.12) in an algorithmic fashion, and for any dimension: that is the task in step (ⅱ). The rough idea, as in Eq (3.10), is to get rid of the derivatives $\omega^{(k)}$, $k = 1, 2, \ldots, n+2$, in the system consisting of the expressions

where $\xi_i$ is the result of expanding $I_i({{\boldsymbol{q}}}(\omega))$, with $i = 0, 1, \ldots, n$. In fact, because of Eq (2.10) in Lemma 2.2, the left-hand side only depends on $\omega', \omega''$.

The most difficult part is to provide an explicit expression for the righthand side of Eq (3.13). This is a long, technical process involving far from trivial combinatorial questions, so we will skip the details here, and refer the interested reader to the ArXiv version of this paper ^[3] for a complete deduction. We will just point out that one can write $\omega''$ at the righthand side of Eq (3.13) in terms of the powers of $\omega'$, $I_n({{\boldsymbol{q}}}(\omega))$ and $I_n({{\boldsymbol{q}}})(\omega)$ (see Lemma 11 of ^[3]), and that it is the final elimination of the powers of $\omega'$ in the resulting equations that yield the Möbius-commuting invariants (see Section 4.3 of ^[3]). In order to introduce these invariants, we recall the function $\tilde{B}_{k, m}$ defined in Eq (2.11) (see Subsection 2.3), and denote by $M_{i}^{n+1, \ell}$ the $(n-\ell+2)\times (n-\ell+2)$ determinant satisfying that:

● If $j < i$, the $j$-th column of $M_{i}^{n+1, \ell}$ is $\tilde{B}_{n+1, n+1-j}, \tilde{B}_{n, n+1-j}, \ldots, \tilde{B}_{\ell, n+1-j}$.

● If $j\geq i$, the $j$-th column of $M_{i}^{n+1, \ell}$ is $\tilde{B}_{n+1, n-j}, \tilde{B}_{n, n-j}, \ldots, \tilde{B}_{\ell, n-j}$.

See Section 4.3 of ^[3] for the motivation for introducing this determinant. Additionally, let

We have the following theorem (see Section 4.3 of ^[3] for a proof).

Theorem 3.2. Let $F_1({{\boldsymbol{q}}})$ be the expression in Eq (3.14), and for $k = 3, \ldots, n$, let

where $e_k$ is, for $3\leq k\leq n$, the least common multiple of $2, k$, i.e., $e_k =$lcm$(2, k)$. The $F_\ell$, for $\ell = 1, \ldots, n-1$, are Möbius-commuting.

The generation of the Möbius-commuting invariants, for any dimension $n$, is implemented in ^[8], which can be freely downloaded, and can be done just once for each dimension $n$. In Table 1, we spell the invariants for low dimension, $2\leq n\leq 4$.

3.3.   Third step

Next, let us address step (ⅲ). Let $F_j$ be a Möbius-commuting invariant, $j\in \{1, \ldots, n-1\}$. Since $F_j$ is a rational function of the $I_i$, $F_j$ is also an affine invariant, i.e., from Theorem 3.1 we get that $F_j({{\boldsymbol{p}}}) = F_j({{\boldsymbol{q}}}\circ \varphi)$. Therefore, in terms of the variables $z$ and $\omega: = \varphi(z)$, and taking into account that $F_j({{\boldsymbol{q}}}\circ \varphi) = F_j({{\boldsymbol{q}}})\circ \varphi$, we deduce that $F_j({{\boldsymbol{p}}})(z) = F_j({{\boldsymbol{q}}})(\omega)$, thus we have the following result.

Proposition 3.1. Let $\mathit{\boldsymbol{C}}_1, \mathit{\boldsymbol{C}}_2\subset {\Bbb C}^n$ be two curves, not contained in a hyperplane, parametrized by mappings $\mathit{\boldsymbol{p}}, \mathit{\boldsymbol{q}}$ satisfying the hypotheses in Corollary 2.1. $\mathit{\boldsymbol{C}}_1, \mathit{\boldsymbol{C}}_2$ are affinely equivalent if and only if there exists a Möbius transformation $\varphi$ such that

for $j\in\{1, 2, \ldots, n-1\}$ with $\omega = \varphi(z)$, such that $D({{\boldsymbol{q}}} \circ \varphi)(D({{\boldsymbol{p}}}))^{-1}(z)$ is a constant matrix $A$ and ${{\boldsymbol{b}}} = ({{\boldsymbol{q}}}\circ\varphi-A{{\boldsymbol{p}}})(z)$ is a constant vector. Furthermore, $f({{\boldsymbol{x}}}) = A{{\boldsymbol{x}}}+{{\boldsymbol{b}}}$ is an affine equivalence between ${{\mathit{\boldsymbol{C}}}}_1, {{\mathit{\boldsymbol{C}}}}_2$.

Proof. $(\Rightarrow)$ Let $f$ be an affine equivalence between $\mathit{\boldsymbol{C}}_1, \mathit{\boldsymbol{C}}_2$. By Theorem 2.1, there exists a Möbius function $\varphi$ such that $f\circ {{\boldsymbol{p}}} = {{\boldsymbol{q}}} \circ \varphi$. By Corollary 3.1 we have that $I_i({{\boldsymbol{p}}})(z) = I_i({{\boldsymbol{q}}}(\omega))$ for all $i\in\{0, \ldots, n\}$. Since the $F_j$ are rational functions of the $I_i$, $I_i({{\boldsymbol{p}}})(z) = I_i({{\boldsymbol{q}}}(\omega))$ yields $F_j({{\boldsymbol{p}}})(z) = F_i({{\boldsymbol{q}}})(\omega)$ for $j\in\{1, 2, \ldots, n-1\}$. Finally, writing $f({{\boldsymbol{x}}}) = A{{\boldsymbol{x}}}+{{\boldsymbol{b}}}$, the condition $f\circ {{\boldsymbol{p}}} = {{\boldsymbol{q}}} \circ \varphi$ implies that $A{{\boldsymbol{p}}}(z)+{{\boldsymbol{b}}} = {{\boldsymbol{q}}}(\varphi(z))$, so ${{\boldsymbol{b}}} = ({{\boldsymbol{q}}}\circ\varphi-A{{\boldsymbol{p}}})(z)$, which is a constant vector. Furthermore, by differentiating the condition $A{{\boldsymbol{p}}}(z)+{{\boldsymbol{b}}} = {{\boldsymbol{q}}}(\varphi(z))$ (see Subsection 3.1) we deduce that $A = D({{\boldsymbol{q}}} \circ \varphi)(D({{\boldsymbol{p}}}))^{-1}(z)$. $(\Leftarrow)$ Let $\varphi$ be a Möbius transformation satisfying $F_i({{\boldsymbol{p}}}(z))-F_i({{\boldsymbol{q}}})(\omega) = 0$ for $\omega = \varphi(z)$. If $A = D({{\boldsymbol{q}}} \circ \varphi)(D({{\boldsymbol{p}}}))^{-1}(z)$ is a constant matrix, then $D(A{{\boldsymbol{p}}})(z) = D({{\boldsymbol{q}}}\circ \varphi)(z)$, so $A{{\boldsymbol{p}}}(z)-({{\boldsymbol{q}}}\circ \varphi)(z)$ is a constant equal to $-{{\boldsymbol{b}}}$. Therefore, $A{{\boldsymbol{p}}}(z)+{{\boldsymbol{b}}} = {{\boldsymbol{q}}}(\varphi(z))$. However this equality implies that $A{{\boldsymbol{p}}}(z)+{{\boldsymbol{b}}}$, which is the image of $\mathit{\boldsymbol{C}}_1$ under the affine mapping $f({{\boldsymbol{x}}}) = A{{\boldsymbol{x}}}+{{\boldsymbol{b}}}$, and ${{\boldsymbol{q}}}(z)$ parametrize the same curve, namely, $\mathit{\boldsymbol{C}}_2$. Thus, $f({{\boldsymbol{x}}}) = A{{\boldsymbol{x}}}+{{\boldsymbol{b}}}$ is an affine equivalence between ${{\mathit{\boldsymbol{C}}}}_1$ and ${{\mathit{\boldsymbol{C}}}}_2$.

4.   Algorithm and examples

To finally turn Proposition 3.1 into an algorithm, let $M_j(z, \omega)$ be obtained by clearing denominators in $F_j({{\boldsymbol{p}}})(z)-F_j({{\boldsymbol{q}}})(\omega)$. We need to request that $M_j(z, \omega)$ is not identically zero, which amounts to requiring that not all the $F_j$ are constant; although this is rare, it can happen: two examples are conic planar curves, and helices, i.e., space curves where the quotient between curvature and torsion is constant (including circular helices). If $M_j(z, \omega)$ is not zero, then $M_j(z, \omega) = 0$ defines an analytic curve in the plane $z, \omega$. Now if $\varphi(z)$, as in Eq (2.1), is a Möbius function satisfying Proposition 3.1, calling $\omega = \varphi(z)$ we get that all the points $(z, \omega)$ of the curve

which is an irreducible analytic curve, are also points of the curve $M_j(z, \omega)$. As a consequence of Study's Lemma (see Section 6.13 of^[6]), $H(z, \omega) = \omega(cz+d)-(az+d)$ must be a factor of $M_j(z, \omega)$; we say that $H(z, \omega) = \omega(cz+d)-(az+d)$ is a Möbius-like factor of $M_j(z, \omega)$, and that the Möbius function $\varphi$ in Eq (2.1) is associated with $H(z, \omega)$. So we have the following theorem, which follows from Proposition 3.1.

Theorem 4.1. Let $\mathit{\boldsymbol{C}}_1, \mathit{\boldsymbol{C}}_2\subset {\Bbb C}^n$ be two curves, not contained in a hyperplane, parametrized by mappings $\mathit{\boldsymbol{p}}, \mathit{\boldsymbol{q}}$ satisfying the hypotheses in Corollary 2.1 and where not all the $F_j$ are constant. $\mathit{\boldsymbol{C}}_1, \mathit{\boldsymbol{C}}_2$ are affinely equivalent if and only if there exists a Möbius-like factor $H(z, \omega)$ common to $M_j(z, \omega)$, $j = 1, \ldots, n-1$ such that the corresponding associated Möbius function $\varphi$ satisfies that: (1) $D({{\boldsymbol{q}}} \circ \varphi)(D({{\boldsymbol{p}}}))^{-1}(z)$ is a constant matrix $A$, (2) ${{\boldsymbol{b}}} = ({{\boldsymbol{q}}}\circ\varphi-A{{\boldsymbol{p}}})(z)$ is a constant vector. Furthermore, in that case, $f({{\boldsymbol{x}}}) = A{{\boldsymbol{x}}}+{{\boldsymbol{b}}}$ is an affine equivalence between ${{\mathit{\boldsymbol{C}}}}_1, {{\mathit{\boldsymbol{C}}}}_2$.

Thus, we get the following procedure ${\mathtt{ AffineEquivalences}}$ to find the affine equivalences between the curves $\mathit{\boldsymbol{C}}_1, \mathit{\boldsymbol{C}}_2$ defined by ${{\boldsymbol{p}}}, {{\boldsymbol{q}}}$.

If ${{\boldsymbol{p}}}, {{\boldsymbol{q}}}$ are rational, the $M_j(x, z)$ are rational and $H(z, \omega)$ is a factor of $\mbox{gcd}(M_1(z, \omega), \ldots, M_{n-1}(z, \omega))$. However, the computer algebra system ${\mathtt{Maple}}$ ^[13] where we implemented the procedure (see ^[8]) can compute $H(z, \omega)$ also in the case when ${{\boldsymbol{p}}}, {{\boldsymbol{q}}}$ are not rational, but satisfies the hypotheses of the procedure. In this last case, we ask ${\mathtt{Maple}}$ to solve $H(z, \omega)$ for $\omega$ to find the Möbius functions.

Remark 4.1. Although ${\mathtt{Maple\ Help System}}$ is not too specific about this, in the case when the $M_j(x, z)$ are not rational the idea seems to be that ${\mathtt{Maple}}$ renames repeated non-rational expressions found in the $M_j(x, z)$ (e.g., $\mbox{cos}(z), e^z$, etc.) to form rational functions, and then proceeds by applying the algorithm for the rational case. Furthermore, in the case of non-rational parametrizations we have considered examples where the adjoined function $\xi(z)$ (see Section 2.2) is the same for both ${{\boldsymbol{p}}}, {{\boldsymbol{q}}}$, since it is not guaranteed that ${\mathtt{Maple}}$ can solve $H(z, \omega)$ for $\omega$ otherwise.

In order to illustrate the performance of the procedure ${\mathtt{AffineEquivalences}}$, we consider now two examples where we compute the affine equivalences between curves taken from Example 2.1, and the images of these curves under an affine mapping. These examples were computed with ${\mathtt{Maple}}$ and executed in a PC with a 3.60 GHz Intel Core i7 processor and 32 GB RAM, and are accessible in ^[8] as well.

Example 4.1. (2D catenary curves) Consider the curves ${{\mathit{\boldsymbol{C}}}}_1$ and ${{\mathit{\boldsymbol{C}}}}_2$ parametrized by

The curve ${{\boldsymbol{q}}}(z)$ corresponds to the first curve in Example 2.1, which is a catenary curve. After appying our algorithm, we find two factors $\widehat{H}_i, (z, \omega)$, $i = 1, 2$, common to the $M_j$, namely

When solving for $\omega$, we get infinitely many (complex) Möbius functions leading to infinitely many (complex) affine equivalences, which reveals that the $\widehat{H}_i(\omega, z)$ contain Möbius-like factors. The affine equivalences can be classified in three classes $f_j({{\boldsymbol{x}}}) = A_j{{\boldsymbol{x}}}+{{\boldsymbol{b}}}_j$, $j\in\{1, 2, 3\}$, with associated Möbius functions $\varphi_j(z)$:

and

where ${\bf i}^2 = -1$. If we just consider real affine equivalences, we have three of them, which correspond to fixing $k_1 = 0$ for $f_1({{\boldsymbol{x}}})$, $k_2 = 0$ for $f_2({{\boldsymbol{x}}})$, and $k_2 = -1/2$ for $f_3({{\boldsymbol{x}}})$. The whole computation took 0.172 seconds.

Example 4.2. (3D spirals) Consider the curves ${{\mathit{\boldsymbol{C}}}}_1$ and ${{\mathit{\boldsymbol{C}}}}_2$ parametrized by

The curve ${{\boldsymbol{q}}}(z)$ corresponds to the third curve in Example 2.1, which is a 3D spiral. After applying our algorithm, we find two Möbius-like factors $H_i(z, \omega)$, $i = 1, 2$, common to the $M_j(z, \omega)$, namely

When solving for $\omega$, we get two Möbius transformations $\varphi_1(z) = -2z$ and $\varphi_2(z) = 2z$ corresponding to the affine equivalences $f_1({{\boldsymbol{x}}}) = A_1{{\boldsymbol{x}}}+{{\boldsymbol{b}}}_1$ and $f_2({{\boldsymbol{x}}}) = A_2{{\boldsymbol{x}}}+{{\boldsymbol{b}}}_2$ with

and

The whole computation took 0.032 seconds.

Example 4.3. (Rational curves in $n$-th dimension) Finally, in Table 2, we present the results of performance tests to compute affine equivalences between rational curves of various degrees in different dimensions. The rational curves in the experiments were randomly generated ^[8] with coefficients between $-10$ and $10$. After generating the first curve, the second curve was obtained by applying an affine mapping $f({{\boldsymbol{x}}}) = A{{\boldsymbol{x}}}+{{\boldsymbol{b}}}$ to the first curve, where the matrix and the translation vector, for each dimension, are shown in Table 3; additionally, the resulting curve was reparametrized using a Möbius transformation $\varphi(z) = 2z-1$. The timings to recover the affine equivalences are shown in Table 3: the rows of Table 3 correspond to dimensions from $n = 2$ to $n = 6$, and the columns, to degrees from $d = 6$ to $d = 12$. For degrees up to 10, we can compute the affine equivalences between the curves in less than a minute, for all the dimensions tested.

5.   Conclusions

We have presented an algorithm, generalizing the algorithm in ^[7], to compute the affine equivalences, if any, between two parametric curves in any dimension. Our strategy relies on bivariate factoring, and avoids polynomial system solving. The algorithm works for rational curves and also certain types of non-algebraic parametric curves with bimeromorphic parametrizations, where we are adjoining a non-algebraic, meromorphic function $\xi(z)$. We have implemented the algorithm in ${\mathtt{Maple}}$, and evidence of its performance has been presented.

The algorithm works whenever not all the Möbius-commuting invariants are constant. This happens generically, but identifying the curves where this does not occur, as well as providing a solution to the problem for this special case, are questions that we pose here as open problems.

Additionally, in the case of non-algebraic curves, right now we need some hypotheses that are not always satisfied: for instance, planar curves like the cycloid, or the tractrix, or classical planar spirals, do not satisfy our hypotheses. However, we have observed that the algorithm seems to work also for many of those curves, which makes us think that our hypotheses could be relaxed. This requires more theoretical work regarding analytic curves.

It would be desirable to extend our ideas to the case of rational surfaces/hypersurfaces. This probably requires some extra hypotheses, e.g., nonexistence of base points or special types of surfaces/hypersurfaces, that allow us to guess the type of transformation that we have in the parameter space: such transformation would play a role similar to the role played by Möbius transformations here. These are questions that we would like to address in the future.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgements

Juan Gerardo Alcázar is supported by the grant PID2020-113192GB-I00 (Mathematical Visualization: Foundations, Algorithms and Applications) from the Spanish MICINN. Juan G. Alcázar is also a member of the Research Group asynacs (Ref. ccee2011/r34). Uğur Gözütok is supported by the grant $121C421$, in the scope of $2218$-National Postdoctoral Research Fellowship Program, from TUBITAK (The Scientific and Technological Research Council of Türkiye). We thank the reviewer of the paper for his/her comments, which helped to improve the first version of the paper.

Conflict of interest

Juan Gerardo Alcázar is the Guest Editor of special issue "Computer Algebra, Geometry and Applications" for AIMS Mathematics. Juan Gerardo Alcázar was not involved in the editorial review and the decision to publish this article.

The authors declare no conflict of interest in this paper.