LS (3)-equivalence conditions of control points and application to spatial Bézier curves and surfaces

1.   Introduction

In mechanics the concept of similarity is mostly used in development of dimensional analysis. Dimensional analysis arose from an attempt to extend to physics some concepts like similarity, ratio, and proportion ^[1,2]. It was first applied by Galileo in 1638 to predict the strength of beams of given material as a function of linear dimensions ^[1]. Other applications were given by Mariotte in 1679 and Newton in 1686 ^[1]. However, Fourier stated for the first time in 1822 that all physical quantities are expressed in a number of fundamental units and that the conversion of physical systems to other physical systems is possible by the conversion of these fundamental units to each other ^[1,2].

Developments in invariant theory at the end of the 19th century have affected different areas of mathematics. The basis of invariant theory studies is the problem of examining whether the invariant polynomial ring $R[x]^{G}$ has finite generator or not ^[3]. After David Hilbert M. Nagata showed that the ring $R[x]^{G}$ has finite generators if $G$ is linear reductive ^[4]. In case $G$ is not a linear reductive group, how conditions of that the ring $R[x]^{G}$ has finite generators are given in ^[5,6].

Until F. Klein, only certain geometries were known. In 1872, Klein showed that groups are important building blocks of geometry in his Erlangen Programme. According to Klein two elements $A$ and $B$ are equivalent if and only if there exist a transformation $f$ such that $B = f(A)$ is satisfied ^[7]. In the 20th century Bridgman ^[8], Sedov ^[9], and Langhaar ^[10] are some contributors in this area. In 1946, Herman Weyl gave the complete invariant system of points for real n dimensional orthogonal group $O(n)$ in ^[11].

Some applications of invariant theory for various groups to points systems and the differential curves are given in ^{[12,13,14,15,16,17,18,19,20]} recently.

Computer aided design (CAD) is based on parametric curves and parametric surfaces. The most important tools in computer aided geometric design (CAGD) are Bézier Curves and surfaces, Coons patches and then B-spline methods ^[21]. The CAGD has gained discipline in itself under the leadership of Barnhill and Riesenfeld, following a conference at the University of Utah in 1974 in the United States ^[22].

The best examples of points systems are Bézier curves and Bézier surfaces. The invariants of these curves and surfaces under an affine transformation have the same meaning as the invariants of the control points of these curves and surfaces. Therefore it is necessary that the invariant properties of modelling of mechanism allow durable and precision, and reliable results under transformation groups.In this sense, knowing of complete of invariants of Bézier and B-spline curves and surfaces which gives most stable solutions within CAD systems ^[23] is important to apply it to different areas.

Bézier curves and surfaces have been used in a wide range of applications from automotive industry to aircraft industry. Many example i.e. on offset surfaces, G. Farin ^[24], R. Farouki ^[25], J. Hoschek ^[26], W. Tiller ^[27], H. Potmann ^[28]; on different surfaces and conics, R. Farouki and Carla Manni ^[29], F. Chen ^[30], H. Pottmann ^[31], R. Krasauskas ^[32]; on the properties of trigonometric functions, Reyes ^[33]; on kinematics, B. Jüttler ^[34], Q.J. Ge ^[35], B. Roth ^[36] and on the application of heat transfer by the limited element method R. Cholewa et al. ^[37] can be given as examples of using Bézier curves and surfaces.

Recently, many of studies have been studied on Bézier curves and surfaces and their CAD CAM applications. There are some examples in ^{[38,39,40,41,42,43,44,45,46,47,48,49,50,51,52]}.

Let $G$ be a transformation group and act on $X$. Any elements $x, y \in X$ are $G-$equivalent elements if there exist a transformation $g \in G$ such that $y = gx$ is satisfied. Similarly Let $A = \left\{x_{1}, x_{2}, ..., x_{n}\right\}$ and $B = \left\{ y_{1}, y_{2}, ..., y_{n} \right\}$ be any two subspaces of $X$ with $n-$elements. In this case the subspaces $A$ and $B$ are $G-$equivalent if there exist a transformation $g \in G$ such that $y_{i} = gx_{i}$ is satisfied for every $i = 1, 2, ..., n$.

When planar points systems be given, for the group $G = LS(2)$, the generators of $G-$invariant rational functions and the $G-$equivalence conditions of these points systems were given in terms of the generators invartiants of these systems in $\mathbb{R}^{2}$ in ^[13].

This paper presents the $G-$equivalence conditions of the subspaces $A$ and $B$ with $m-$elements in $3-$dimensional Euclidean space $E^{3}$ under the transformation group $G = LS(3)$ which is the linear similarity transformation group in $E^{3}$ in section 3. So while in ^[13] only coplanar points are discussed, but in this paper, the $G = LS(3)$-equivalence conditions are given in terms of their rational $G = LS(3)$ invariants for non-coplanar points.

In section 4 and section 5, the $G = LS(3)-$equivalence conditions of spatial Bézier curves and Bézier surfaces in terms of their rational $G = LS(3)$ invariants of the control points of these curves and surfaces as the application of the $G = LS(3)-$equivalence conditions of points sets to curves and surfaces which are geometric structures are given.

In addition in section 6, the font design, which is an important application of Bézier curves and surfaces in CAD systems, is discussed. By using quadratic Bézier curves, a simple letter s is designed and two different shadow curves of this letter (composite curves) are obtained. As well It is emphasized that the only requirement that the obtained curves are shadow or other type of the designed letter should be $G = LS(3)-$equivalent.

2.   The rational $LS(3)-$invariants of points

As well known in linear algebra, a linear transformation between two vector spaces $V$ and $W$ is a map $T:V \rightarrow W$ such that the following hold:

$T(v_{1}+v_{2}) = T(v_{1})+T(v_{2})$ and $T(\alpha v) = \alpha T(v)$

for any vectors $v_{1}$ and $v_{2}$ in $V$ and for any scalar $\alpha \in F$.

Let E be a three dimensional Euclidean space then the transformation $F:E\mapsto E$ is called a similarity transformation if there exist a positive real number $\lambda$ such that $\left\|F(x)-F(y)\right\| = \lambda \left\|x - y \right\|$ is satisfied for every $x, y \in E$.

The homotethy transformation in three dimensional Euclidean space $E$ is defined by $F:E\mapsto E$ such that $F(x) = a + \lambda \left(x - a\right)$ for every $x \in E$ and for $\lambda \in \mathbb{R}$, where $a$ is called the center of the homotethy $F$.

The group of all the orthogonal transformations defined in 3-dimensional Euclidean space $E$ is denoted by $O(3, E)$. For shortness this group is denoted by $O(3)$. The group of all the rotations is denoted by $SO(3)$. Accordingly the linear homotethy transformation $F$ in three dimensional Euclidean space $E$ is defined by $F(x) = \lambda x$ for every $x \in E$.

Since a linear similarity transformation is composed of multiplication of two transformations: A linear homothety and a linear isometry or an orthogonal transformation, any linear similarity transformation $F:E\mapsto E$ in three dimensional Euclidean space $E$ can be stated as

where $g \in O\left(3\right)$ ^[13].

Let $G$ be any transformation group. Then, the function $f$ is called $G-$ invariant function, if

for every $x \in E$ and every $g\in G$.

Let $R\left[x^{(1)}, x^{(2)}, ..., x^{(m)}\right]$ be a ring of polynomials for m vector variables $x^{(1)}, x^{(2)}, ..., x^{(m)}$ in 3- dimensional Euclidean space $\mathbb{R}^{3}$ over the field $\mathbb{R}$ and a transformation group G be given. Then the algebra of $G-$invariant polynomials for m vector variables $x^{(1)}, x^{(2)}, ..., x^{(m)}$ in 3-dimensional Euclidean space $\mathbb{R}^{3}$ over the field $\mathbb{R}$ is denoted by $R\left[x^{(1)}, x^{(2)}, ..., x^{(m)}\right]^{G}$.

Theorem 2.1. Let $x^{(1)}, x^{(2)}, ..., x^{(m)}$ be m vector variables in 3- dimensional Euclidean space $\mathbb{R}^{3}$. Then, the system of functions

is the generator system of the algebra $R\left[x^{(1)}, x^{(2)}, ..., x^{(m)}\right]^{O\left(3\right)}$.

Proof: See, ^[11].

Theorem 2.2. Let $x^{(1)}, x^{(2)}, ..., x^{(m)}$ be m vector variables in 3- dimensional Euclidean space $\mathbb{R}^{3}$. If $m > 3$ then, for $i, j = 1, 2, 3$ and $p = 4, ..., m$ the generator system of the field of the $O(3)$-invariant rational functions $R\left(x^{(1)}, x^{(2)}, ..., x^{(m)}\right) ^{O\left(3\right) }$ is as follows

If $m \leq 3$ then, the generator system of the field $R\left(x^{(1)}, x^{(2)}, ..., x^{(m)}\right)^{O\left(3\right)}$ is the same as the system (2.3).

Proof: See, ^[11].

The generators of any $G$-invariant polynomial ring or the generators of any field of $G$-invariant rational functions are also the generator invariants. So any $G$-invariant function is stated as a function of these generators.

Any $LS(3)-$invariant polynomial function is constant ^[3]. So it is stated here only $LS(3)-$invariant rational functions.

Theorem 2.3. Let $x^{(1)}, x^{(2)}, ..., x^{(m)}$ be m vector variables different from zero in 3-dimensional Euclidean space $\mathbb{R}^{3}$. If $m > 3$ then, for $i, j = 1, 2, 3$ and $p = 4, ..., m$ the generator system of the field $R\left(x^{(1)}, x^{(2)}, ..., x^{(m)}\right) ^{LS\left(3\right) }$ is as follows

If $m \leq 3$ then, the generator system of the field $R\left(x^{(1)}, x^{(2)}, ..., x^{(m)}\right)^{LS\left(3\right)}$ is the system

Proof: See, ^[53].

3.   $LS(3)-$equivalence conditions of control points

Let $G$ be a transformation group and $E$ be a three dimensional Euclidean space. Then, the points $x, y \in E$ are called $G$-equivalent points if there exist a transformation $g \in G$ such that $y = gx$ satisfies. If $x$ and $y$ are $G$-equivalent points then the notation $x \overset{G}{ \cong } y$ is used.

Let $G$ be a transformation group and $E$ be a three dimensional Euclidean space and two points systems $\left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\}$ and $\left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ in $E$ be given. Then, these systems are called $G$-equivalent if there exist a transformation $g \in G$ such that $y^{(i)} = gx^{(i)}$ satisfies for every $i \in \left\{1, 2, ..., m\right\}$. If these points systems $\left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\}$ and $\left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ are $G$-equivalent systems then the notation $\left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{G}{ \cong} \left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ is used.

Theorem 3.1. Let two vectors $x = (x_{1}, x_{2}, x_{3})$ and $y = (y_{1}, y_{2}, y_{3})$ be given in $\mathbb{R}^{3}$. Then,

1. if $x = 0$ and $y\neq 0$ or $x\neq 0$ and $y = 0$ then $x$ and $y$ are not $LS(3)$-equivalent. ie. $x \overset{LS(3)}{ \ncong } y$

2. if $x = 0$ and $y = 0$ or $x\neq 0$ and $y\neq 0$ then $x \overset{LS(3)}{\cong } y$ is always satisfied.

Proof.

1. Let $x\neq 0$ and $y = 0$ be given and $x \overset{LS(3)}{ \cong } y$ be supposed. In this case there exist a transformation $h \in LS(3)$ such that $y = hx$ is satisfied. It means there exist $g \in O(3)$ and $\lambda > 0$ such that $y = \lambda gx$ is satisfied. Since the orthogonal transformations save the inner product, $\left\langle y, y\right\rangle = \lambda^{2}\left\langle x, x\right\rangle$ is obtained. So because of $x\neq 0$ and $\lambda > 0$, the inner product $\left\langle y, y\right\rangle$ must be different zero and $y$ can not be $0$ vector. This is a contradiction. So $x$ and $y$ are not $LS(3)$-equivalent vectors. similarly in case $x = 0$ and $y\neq 0$ the statement can be reduced first case since the relationship $\overset{G}{\cong }$ is an equivalence relation and has symmetry property.

2. Let $x = (0, 0, 0)$ and $y = (0, 0, 0)$ be given. In case $gx = 0$ for every $g \in O(3)$ and $y = \lambda gx$ can be stated since $\lambda gx = 0$ Thus from (2.2) $x \overset{LS(3)}{ \cong } y$ is proved.

Let $x\neq 0$ and $y\neq 0$ be given. Then $\left\langle x, x\right\rangle$ and $\left\langle y, y\right\rangle$ are different from zero. Thus, the positive real number $\lambda$ can be chosen as $\lambda = \frac{\left\langle y, y\right\rangle}{\left\langle x, x\right\rangle}$. So $\left\|y\right\| = \left\| \lambda x \right\|$ is obtained. In this case the vectors $y$ and $\lambda x$ are on the same $O(3)$-orbit. It means there exist an orthogonal transformation $g \in O(3)$ such that $y = g (\lambda x) = \lambda gx$ satisfies. From this, $x \overset{LS(3)}{ \cong } y$ is proved.

Theorem 3.2. Let two points systems $\left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\}$ and $\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ in $E$ be given. So,

1. if $x^{(i)} = 0$ and $y^{(i)}\neq 0$ or $x^{(i)}\neq 0$ and $y^{(i)} = 0$ for any $i = 1, 2, ..., m$, then these systems are not $LS(3)$- equivalent. ie. $\left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{ \ncong } \left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$

2. if $x^{(i)} = 0$ and $y^{(i)} = 0$ for any $i = 1, 2, ..., m$, then the equivalence conditions of the systems with $m$ vectors is reduced to the equivalence conditions with $m-1$ vectors.

3. if $x^{(i)}\neq 0$ and $y^{(i)}\neq 0$ for every $i = 1, 2, ..., m$, then

(a) if the ranks of the matrices $\left[x^{(1)} x^{(2)}... x^{(m)} \right]$ and $\left[y^{(1)} y^{(2)}... y^{(m)} \right]$ are equal to 3 then $\left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{ \cong } \left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ if and only if $\frac{ \left\langle x^{(i)}, x^{(j)} \right\rangle}{\left\langle x^{(1)}, x^{(1)}\right\rangle} = \frac{\left\langle y^{(i)}, y^{(j)} \right\rangle}{ \left\langle y^{(1)}, y^{(1)} \right\rangle}$ and $\frac{\left\langle x^{(i)}, x^{(p)} \right\rangle}{\left\langle x^{(1)}, x^{(1)} \right\rangle} = \frac{\left\langle y^{(i)}, y^{(p)} \right\rangle}{\left\langle y^{(1)}, y^{(1)} \right\rangle}$where $i, j = 1, 2, 3$ and $i\leq j$ and $p = 4, ..., m$.

(b) if the ranks of the matrices $\left[x^{(1)} x^{(2)}... x^{(m)} \right]$ and $\left[y^{(1)} y^{(2)}... y^{(m)} \right]$ is equal to 2 then $\left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{ \cong } \left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ if and only if $\frac{ \left\langle x^{(i)}, x^{(j)} \right\rangle}{\left\langle x^{(1)}, x^{(1)} \right\rangle} = \frac{\left\langle y^{(i)}, y^{(j)} \right\rangle}{ \left\langle y^{(1)}, y^{(1)} \right\rangle}$ where $i = 1, 2$ and $j = 2, 3, ..., m$

(c) if the ranks of the matrices $\left[x^{(1)} x^{(2)}... x^{(m)} \right]$ and $\left[y^{(1)} y^{(2)}... y^{(m)} \right]$ is equal to 1 then $\left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{ \cong }\left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ if and only if $\frac{\left\langle x^{(1)}, x^{(j)} \right\rangle}{\left\langle x^{(1)}, x^{(1)} \right\rangle} = \frac{\left\langle y^{(1)}, y^{(j)} \right\rangle}{ \left\langle y^{(1)}, y^{(1)} \right\rangle}$ where $j = 2, 3, ..., m$ is satisfied.

(d) if the rank of the matrix $\left[x^{(1)} x^{(2)}... x^{(m)} \right]$ is different from the rank of the matrix $\left[y^{(1)} y^{(2)}... y^{(m)} \right]$ then these system can not be $LS(3)$-equivalent.

Proof.

1. Let $x^{(i)} = 0$ and $y^{(i)}\neq 0$ be given for any $i = 1, 2, ..., m$ and $\left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\}\overset{LS(3)}{ \cong } \left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ be supposed. Then there exist a scalar $\lambda > 0$ and an orthogonal transformation $g \in O(3)$ such that $y^{(j)} = \lambda gx^{(j)}$ for every $j = 1, 2, ..., m$ is satisfied. So $y^{(i)}$ must be $0$ since $x^{(i)} = 0$. This is a contradiction. Thus these systems $\left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\}$ and $\left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ are not $LS(3)$- equivalent. Similarly in case $x^{(i)}\neq 0$ and $y^{(i)} = 0$ for any $i = 1, 2, ..., m$, then we will prove that these systems $\left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\}$ and $\left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ are not $LS(3)$- equivalent. Suppose that these systems are $LS(3)$- equivalent. Then, there exist a $\lambda > 0$ and $g \in O(3)$ such that $y^{(j)} = \lambda gx^{(j)}$ for every $j = 1, 2, ..., m$ is satisfied. From this equation for the integer $i$, $x^{(i)} = \frac{1}{\lambda } g^{T}y^{(i)}$ can be written. Then $x^{(i)}$ must be $0$ Since $y^{(i)} = 0$. This is a contradiction. So these systems $\left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\}$ and $\left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ are not $LS(3)$-equivalent.

2. Let $x^{(i)} = 0$ and $y^{(i)} = 0$ for any $i = 1, 2, ..., m$ be given and $\left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{ \cong } \left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ be supposed. Then, $y^{(j)} = \lambda gx^{(j)}$ for every $j = 1, 2, ..., m$ is satisfied. Excluding the i th elements of these systems $y^{(j)} = \lambda gx^{(j)}$ for every $j = 1, 2, ..., i-1, i+1, ..., m$ is satisfied. So $\left\{x^{(1)}, ..., x^{(i-1)}, x^{(i+1)}, ..., x^{(m)}\right\}\overset{LS(3)}{\cong }\left\{y^{(1)}, ..., y^{(i-1)}, y^{(i+1)}, ..., y^{(m)}\right\}$ can be written. Conversely let $\left\{x^{(1)}, x^{(2)}, ..., x^{(i-1)}, x^{(i+1)}, ..., x^{(m)}\right\} \overset{LS(3)}{ \cong }\left\{y^{(1)}, y^{(2)}, ..., y^{(i-1)}, y^{(i+1)}, ..., y^{(m)}\right\}$ be supposed. Then, there exist a scalar $\lambda > 0$ and $g \in O(3)$ such that $y^{(j)} = \lambda gx^{(j)}$ for every $j = 1, 2, ..., i-1, i+1, ..., m$. The i th elements of these systems can be added here since $x^{(i)} = 0$ and $y^{(i)} = 0$ So $y^{(j)} = \lambda gx^{(j)}$ for every $j = 1, 2, ..., m$ is satisfied then $\left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{ \cong } \left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ is obtained.

3. (a) Let $x^{(i)}\neq 0$ and $y^{(i)}\neq 0$ for every $i = 1, 2, ..., m$ and the ranks of the matrices $\left[x^{(1)}x^{(2)}...x^{(m)}\right]$ and $\left[y^{(1)}y^{(2)}...y^{(m)}\right]$ are equal to 3 be given and $\left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\cong }\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ be supposed. In this case there exist a scalar $\lambda > 0$ and $g\in O(3)$ such that $y^{(j)} = \lambda gx^{(j)}$ satisfies for every $j = 1, 2, ..., m$. Because the orthogonal transformation $g\in O(3)$ save inner product, this equality

is obtained.

Conversely for every $i, j = 1, 2, 3$, $p = 4, ..., m$. and $i\leq j$ let this equalities $\frac{\left\langle x^{(i)}, x^{(j)}\right\rangle }{\left\langle x^{(1)}, x^{(1)}\right\rangle } = \frac{\left\langle y^{(i)}, y^{(j)}\right\rangle }{\left\langle y^{(1)}, y^{(1)}\right\rangle }$ and $\frac{\left\langle x^{(i)}, x^{(p)}\right\rangle }{\left\langle x^{(1)}, x^{(1)}\right\rangle } = \frac{\left\langle y^{(i)}, y^{(p)}\right\rangle }{\left\langle y^{(1)}, y^{(1)}\right\rangle }$ be given. It will be proved that $\left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\cong }\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$. Now from hyphothesis for every $i, j = 1, 2, 3$, $p = 4, ..., m$

can be written. So if the multiple $\frac{\left\langle y^{(1)}, y^{(1)}\right\rangle }{\left\langle x^{(1)}, x^{(1)}\right\rangle } > 0$ is denoted by $\lambda ^{2}$, then

is obtained for every $i, j = 1, 2, 3$, $p = 4, ..., m$ and $i\leq j$. Since the the ranks of the matrices $\left[x^{(1)}x^{(2)}...x^{(m)}\right]$ and $\left[y^{(1)}y^{(2)}...y^{(m)}\right]$ are equal to 3, $m\geq 3$ must be chosen. if $m = 3$ then since the vector systems $\left\{ x^{(1)}, x^{(2)}, x^{(3)}\right\}$ and $\left\{ y^{(1)}, y^{(2)}, y^{(3)}\right\}$ in three dimensional Euclidean space are linearly independent, the Gram determinant of the vectors $\lambda x^{(1)}$, $\lambda x^{(2)}$and $\lambda x^{(3)}$

is different from zero. Since

From (3.1) the equality of the matrices

is obtained. Since the matrices $\left[\lambda x^{(1)}\lambda x^{(2)}\lambda x^{(3)}\right]$ and $\left[y^{(1)}y^{(2)}y^{(3)}\right]$ are regular and have inverses, there exist a regular matrix $g$ such that

satisfies. If the equality (3.5) Substitutes to (3.4) and multiply both sides of (3.4) by $\left(\left[\lambda x^{(1)}\lambda x^{(2)}\lambda x^{(3)}\right] ^{T}\right) ^{-1}$ at left firstly and by $\left[\lambda x^{(1)}\lambda x^{(2)}\lambda x^{(3)}\right] ^{-1}$ at right secondly, then $I = g^{T}g$ is obtained. This means that $g$ is orthogonal i.e. there exist $\widetilde{g}\in O\left(3\right)$. From (3.5),

can be written for $i = 1, 2, 3$. So $\left\{ x^{(1)}, x^{(2)}, x^{(3)}\right\} \overset{LS(3)}{\cong }\left\{ y^{(1)}, y^{(2)}, y^{(3)}\right\}$ are obtained.

If $m > 3$ then, Since the rank of the matrix $\left[x^{(1)}x^{(2)}...x^{(m)} \right]$ is equal to 3, the vector systems $\left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\}$ and $\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ in three dimensional Euclidean space are linearly dependent. Suppose that $\exists k, l, z\in \left\{ 1, ..., m\right\}$ such that the vectors $x^{(k)}$ $x^{(l)}$ and $x^{(z)}$ are linearly independent. In this case the vectors $y^{(k)}$ $y^{(l)}$ and $y^{(z)}$ are also linearly independent since the Gram determinant of the vectors $\lambda x^{(k)}$, $\lambda x^{(l)}$ and $\lambda x^{(z)}$ is different from zero. So the determinants

is different from zero. Now any vector in the systems of $\left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\}$ and $\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ can be stated as in terms of linearly independent vectors as follow

for every integer $i = 1, 2, ...m$ are obtained. Since

From (3.6) the equality of the matrices

is obtained. Because the matrices $\left[\lambda x^{(k)} \lambda x^{(l)} \lambda x^{(z)}\right]$ and $\left[y^{(k)} y^{(l)} y^{(z)} \right]$ are regular and have inverses, there exist a regular matrix $g$ such that

satisfies. If the equality (3.9) Substitutes to (3.8) and multiply both sides of (3.8) by $\left(\left[\lambda x^{(k)}\lambda x^{(l)}\lambda x^{(z)}\right] ^{T}\right) ^{-1}$ at left firstly and by $\left[\lambda x^{(k)}\lambda x^{(l)}\lambda x^{(z)}\right] ^{-1}$ at right secondly, then

is obtained. This means that $g$ is orthogonal i.e. there exist $\widetilde{g}\in O\left(3\right)$. From (3.9),

are obtained. From this and (3.6)

for every $i = 1, 2, ..., m$ are obtained.Now we must prove the cofficients of the vectors $y^{(k)}, y^{(l)}, y^{(z)}$ in equations (3.7) and (3.10) are the same. i.e.

$\beta _{ik} = \alpha _{ik}$, $\beta _{il} = \alpha _{il}$ and $\beta _{iz} = \alpha _{iz}$. From (3.6) and (3.7)

and

can be wriiten. Solving these linear equations system

and

is obtained for every $i = 1, 2, ...m$. Using (3.1) and (3.2) $\beta _{ik} = \alpha _{ik}$, $\beta _{il} = \alpha _{il}$ and $\beta _{iz} = \alpha _{iz}$ are obtained. So from (3.10) $y^{(i)} = \lambda \widetilde{g}x^{(i)}$ for every $i = 1, 2, ...m$ are obtained and $\left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\cong }\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ is proved.

(b) Let $x^{(i)}\neq 0$ and $y^{(i)}\neq 0$ for every $i = 1, 2, ..., m$ and the rank of the matrices $\left[x^{(1)}x^{(2)}...x^{(m)}\right]$ and $\left[y^{(1)}y^{(2)}...y^{(m)}\right]$ are equal to 2 be given and $\left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\cong }\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ be supposed. In this case there exist a scalar $\lambda > 0$ and $g\in O(3)$ such that $y^{(j)} = \lambda gx^{(j)}$ satisfies for every $j = 1, 2, ..., m$. Then because the orthogonal transformation $g\in O(3)$ save inner product.

is obtained for $i = 1, 2$ and $j = 3, ..., m$.

Conversely for every $i = 1, 2$ and $j = 2, 3, ..., m$ let this equality $\frac{ \left\langle x^{(i)}, x^{(j)}\right\rangle }{\left\langle x^{(1)}, x^{(1)}\right\rangle } = \frac{\left\langle y^{(i)}, y^{(j)}\right\rangle }{\left\langle y^{(1)}, y^{(1)}\right\rangle }$ be given. It will be proved that

Now from hyphothesis for every $i = 1, 2$ and $j = 2, 3, ..., m$,

can be written. So if the multiple $\frac{\left\langle y^{(1)}, y^{(1)}\right\rangle }{\left\langle x^{(1)}, x^{(1)}\right\rangle } > 0$ is denoted by $\lambda ^{2}$, then

is obtained for every $i = 1, 2$ and $j = 2, 3, ..., m$. Since the ranks of thematrices $\left[x^{(1)}x^{(2)}...x^{(m)}\right]$ and $\left[y^{(1)}y^{(2)}...y^{(m)}\right]$ are equal to 2, $m\geq 2$ must be chosen. The vector systems $\left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\}$ and $\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ in three dimensional Euclidean space are linearly dependent. Suppose that the vectors $x^{(1)}$ and $x^{(2)}$ are linearly independent. In this case the vectors $y^{(1)}$ and $y^{(2)}$ are also linearly independent since the Gram determinant of the vectors $\lambda x^{(1)}$ and $\lambda x^{(2)}$

is different from zero. Now any vector in the systems of $\left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\}$ and $\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ can be stated as in terms of linearly independent vectors as follow

for every integer $i = 1, 2, ...m$ are obtained. Since

From (3.12) the equality of the matrices

is obtained. Because the matrices $\left[\lambda x^{(1)} \lambda x^{(2)} \right]$ and $\left[y^{(1)} y^{(2)} \right]$ are regular and have inverses, there exist a regular matrix $g$ such that

satisfies. If the equality (3.15) substitutes to (3.14) and multiply both sides of (3.14) by $\left(\left[\lambda x^{(1)}\lambda x^{(2)} \right]^{T} \right)^{-1}$ at left firstly and by $\left[\lambda x^{(1)} \lambda x^{(2)} \right]^{-1}$ at right secondly, then

is obtained. this means that $g$ is orthogonal i.e. there exist $\widetilde{g} \in O\left(3\right)$. From (3.15),

are obtained. From this and (3.13)

for every $i = 1, 2, ..., m$ are obtained.Now it must proved that the cofficients of the vectors $y^{(k)}, y^{(l)}$ in equations (3.13) and (3.16) are the same. i.e.

$\beta_{ik} = \alpha_{ik}$ and $\beta_{il} = \alpha_{il}$. From (3.12) and (3.13)

and

can be wriiten. Solving these linear equations system

and

is obtained for every $i = 1, 2, ...m$. Using (3.11) $\beta_{ik} = \alpha_{ik}$ and $\beta_{il} = \alpha_{il}$ are obtained. So $\left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{ \cong } \left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ is proved.

(c) Let $x^{(i)}\neq 0$ and $y^{(i)}\neq 0$ for every $i = 1, 2, ..., m$ and the rank of the matrices $\left[x^{(1)} x^{(2)}... x^{(m)} \right]$ and $\left[y^{(1)} y^{(2)}... y^{(m)} \right]$ are equal to 1 be given and $\left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\cong }\left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ be supposed. In this case there exist a scalar $\lambda > 0$ and $g\in O(3)$ such that $y^{(i)} = \lambda gx^{(i)}$ is satisfied for every $i = 1, 2, ..., m$. Since the rank of above matrices are 1, there exist one linear independent vector in each system $\left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\}$ and $\left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$. Let these linear independent vectors be denoted $x^{(s)}$ and $y^{(s)}$ for any $s \in \left\{1, ...m\right\}$. For every $i = 1, ..., m$

can be written.

So from (3.17)

is obtained for every $i = 2, ..., m$.

Conversely let this equality $\frac{\left\langle y^{(1)}, y^{(i)} \right\rangle}{\left\langle y^{(1)}, y^{(1)} \right\rangle} = \frac{\left\langle x^{(1)}, x^{(i)} \right\rangle}{\left\langle x^{(1)}, x^{(1)}\right\rangle}$ be given for every $j = 2, ..., m$.It will be proved that

From Theorem 3.1 $x^{(s)}\overset{LS(3)}{\cong }y^{(s)}$ always satisfies and then there exist a scalar $\lambda > 0$ and $g\in O(3)$ such that $y^{(s)} = \lambda gx^{(s)}$ satisfies. Then from (3.17)

is obtained and since $y^{(s)} = \lambda gx^{(s)}$ then, $\frac{1}{m_{i}} y^{(i)} = \lambda g\frac{1}{l_{i}}x^{(i)}$ can be written. So for every $i$, $y^{(i)} = \lambda \frac{m_{i}}{l_{i}}gx^{(i)}$ is obtained. From hypotthesis $\frac{m_{i}}{l_{i}} = \frac{m_{1}}{1_{1}} = \sqrt{ \frac{\left\langle y^{(1)}, y^{(1)} \right\rangle}{\left\langle x^{(1)}, x^{(1)}\right\rangle}}$ is always positive. If the real number $\frac{m_{1}}{l_{1}}\lambda$ is denoted by $\overline{\lambda }$, then it is seen that $\overline{\lambda } > 0$.So for every $j = 1, ..., m$;

can be written. So

is proved.

(d) Let $x^{(i)}\neq 0$ and $y^{(i)}\neq 0$ for every $i = 1, 2, ..., m$ and the ranks of the matrices $\left[x^{(1)}x^{(2)}...x^{(m)}\right]$ and $\left[y^{(1)}y^{(2)}...y^{(m)}\right]$ be different from each other. Firstly each rank of given matrices must be different from zero. Otherwise it means all of the vectors in these systems are zero. It is mentioned above. Just let $rank\left[x^{(1)}x^{(2)}...x^{(m)}\right] = 1$ and $rank\left[y^{(1)}y^{(2)}...y^{(m)}\right] = 2$ be given. In this case there exist the integers $i, j, s\in \left\{ 1, 2, ..., m\right\}$ such that the vector $x^{(i)}$ is linearly independent in the system $\left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\}$ and these vectors $y^{(j)}, y^{(s)}$ are linearly independent in the system $\left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$. So

can be written for every $k = 1, 2, ..., m$. It is clear that $a_{i} = b_{jj} = b_{ss} = 1$ and $b_{js} = b_{sj} = 0$. Let $\left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\cong }\left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ be supposed. In this case there exist a $\lambda > 0$ and $g\in O(3)$ such that $y^{(k)} = \lambda gx^{(k)}$ for every $k = 1, ...m$. Then it follows

for every $k = 1, ...m$. It means each vectors ${y^{(1)}, y^{(2)}, ..., y^{(m)}}$ can be expressed by the vector $x^{(i)}$. This is a contradiction and so $\left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\ncong }\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$. Similarly in case $rank\left[x^{(1)}x^{(2)}...x^{(m)}\right] = 1$ and $rank \left[y^{(1)}y^{(2)}...y^{(m)}\right] = 3$ these systems are not equivalent. i.e. $\left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\ncong } \left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$.

Let $rank\left[x^{(1)}x^{(2)}...x^{(m)}\right] = 2$ and $rank$ $\left[x^{(1)}x^{(2)}...x^{(m)}\right] = 1$ be given. In this case there exist the integers $i, j, s\in \left\{ 1, 2, ..., m\right\}$ such that these vectors $x^{(i)}, x^{(j)}$ are linearly independent in the system $\left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\}$ and the vector $y^{(s)}$ is linearly independent in the system $\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ begiven. So,

can be written for every $k = 1, ...m$. Let $\left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\cong }\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$ be supposed. In this case there exist a $\lambda > 0$ and $g\in O(3)$ such that $y^{(k)} = \lambda gx^{(k)}$ for every $k = 1, ...m$. This statement can be written as matrix form as

It follows

It means for every $k = 1, ..., m$

can be written. And every vectors $\left\{ \lambda x^{(1)}, \lambda x^{(2)}, ..., \lambda x^{(m)}\right\}$ can be stated by the vector $\lambda x^{(s)}$ and it is a contradiction. So the assumption is wrong. As a result of this

Similarly in case $rank\left[x^{(1)}x^{(2)}...x^{(m)}\right] = 3$ and $rank\left[y^{(1)}y^{(2)}...y^{(m)}\right] = 1$ these systems are not equivalent. i.e. $\left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\ncong } \left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}$.

4.   Application to spatial Bézier curves

The best examples of points system are Bézier curves and Bézier surfaces. The invariants of these curves and surfaces under an affine transformation have the same meaning as the invariants of the control points of these curves and surfaces. Therefore it is necessary that the invariant properties of modelling of mechanism allow durable and precision, and reliable results under transformation groups. In this sense, knowing of complete of invariants of Bézier and B-spline curves and surfaces which gives most stable solutions within CAD systems ^[23] is important.

A general Bézier curve $X(t)$ of degree $n$ with control points $b_{0}, b_{1}, b_{2}, ..., b_{n}$ is defined by

where $t\in \lbrack 0, 1]$ and $B_{i}^{n}(t)$ are Bernstein basis polynomials of degree n defined by ^[21].

Theorem 4.1. (Invariance under Affine Transformations property)Let $X(t)$ be given a general Bézier curves of degree $n$ with control points ${b_{0}, b_{1}, b_{2}, ..., b_{n}}$ in $R^{3}$. So, $X$ satisfies as follow:

Let $T$ be an (affine) transformation (for example, a rotation, reflection, translation, or scaling or similarity transformation). Then

is satisfied. ^[54]

Definition: Let $X(t)$ and $Y(t)$ be given two general Bézier curves of degree $n$ with control points ${b_{0}, b_{1}, b_{2}, ..., b_{n}}$ and ${q_{0}, q_{1}, q_{2}, ..., q_{n}}$ in $R^{3}$ respectively. Then these Bézier curves are called $LS(3)-$equivalent if there exist a ransformation $g \in LS(3)-$ such that $q_{i} = gb_{i}$ for every $i = 1, ..., n$.

4.1.   Linear Bézier curves

A linear Bézier curve $X(t)$ with control points $b_{0}, b_{1}$ is defined by

where $t\in \lbrack 0, 1]$ ^[54].

Theorem 4.2. Let $X, Y$ be given two linear Bézier curves with control points ${b_{0}, b_{1}}$ and ${p_{0}, p_{1}}$ in $R^{3}$ respectively. So,

1. if any $b_{i} = (0, 0, 0)$ and any $p_{i} = (0, 0, 0)$ for $i = 0, 1$ then these curves are always $LS(3)-$equivalent.

2. if any $b_{i} = (0, 0, 0)$ and each $p_{j}\neq (0, 0, 0)$ or each $b_{i}\neq (0, 0, 0)$ and any $p_{j} = (0, 0, 0)$ for $i, j = 0, 1$ then these curves are not $LS(3)-$equivalent.

3. if every $b_{i}\neq (0, 0, 0)$ and $p_{j}\neq (0, 0, 0)$ for $i, j = 0, 1$ then

(a) if $rank\left[b_{0}\text{ }b_{1}\right] = rank\left[p_{0}\text{ }p_{1}\right] = 2$ then these Bézier curves are $LS(3)-$equivalent if and only if

$\frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{0}, p_{1}\right\rangle }{\left\langle b_{0}, b_{1}\right\rangle } = \frac{\left\langle p_{1}, p_{1}\right\rangle }{\left\langle b_{1}, b_{1}\right\rangle }$ is satisfied.

(b) if $rank\left[b_{0}\text{ }b_{1}\right] = rank\left[p_{0}\text{ }p_{1} \right] = 1$ then these Bézier curves are $LS(3)-$equivalent if and only if

$\frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{0}, p_{1}\right\rangle }{\left\langle b_{0}, b_{1}\right\rangle }$ is satisfied.

Proof. This theorem is a conclusion of Theorem 3.2. In third case the ranks of matrice of control points of each Bézier curves $X$ and $Y$ are equal to 2 or 1. If the rank of matrices is 2, then according to theorem 3.2, these equality $\frac{\left\langle b_{0}, b_{1}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{ \left\langle p_{0}, p_{1}\right\rangle }{\left\langle p_{0}, p_{0}\right\rangle }$ and $\frac{\left\langle b_{1}, b_{1}\right\rangle}{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{1}, p_{1}\right\rangle }{\left\langle p_{0}, p_{0}\right\rangle }$ must be satisfied. So $\frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{0}, p_{1}\right\rangle }{\left\langle b_{0}, b_{1}\right\rangle } = \frac{\left\langle p_{1}, p_{1}\right\rangle }{\left\langle b_{1}, b_{1}\right\rangle }$ is true.

If the rank of matrices is 1 then from Theorem 3.2. $\frac{\left\langle b_{0}, b_{1}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{ \left\langle p_{0}, p_{1}\right\rangle }{\left\langle p_{0}, p_{0}\right\rangle }$ must be satisfied. So $\frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{0}, p_{1}\right\rangle }{\left\langle b_{0}, b_{1}\right\rangle }$ is satisfied.

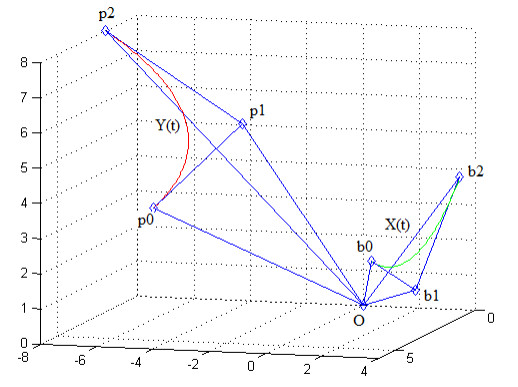
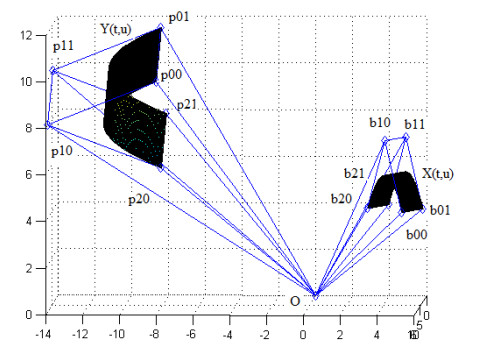
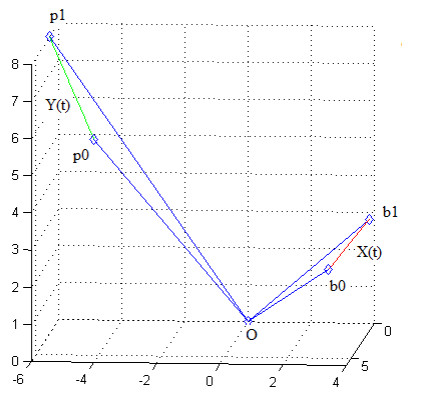
In Figure 1 $LS(3)-$equivalent linear Bézier curves $X(t)$ and $Y(t)$ are illustrated.

4.2.   Quadratic Bézier curves

A quadratic Bézier curve $X(t)$ with control points $b_{0}, b_{1}, b_{2}$ is defined by

where $t\in \lbrack 0, 1]$ ^[54].

Theorem 4.3. Let $X, Y$ be given two quadratic Bézier curves with control points ${b_{0}, b_{1}, b_{2}}$ and ${p_{0}, p_{1}, p_{2}}$ in $R^{3}$ respectively. So,

1. if any $b_{i} = (0, 0, 0)$ and $p_{i} = (0, 0, 0)$ for $i = 0, 1, 2$ then these curves are $LS(3)-$equivalent if and only if the condition of third case of Theorem 3.2 is satisfied excluding $b_{i}$ and $p_{i}$.

2. if any $b_{i} = (0, 0, 0)$ and each $p_{j}\neq (0, 0, 0)$ or each $b_{i}\neq (0, 0, 0)$ and any $p_{j} = (0, 0, 0)$ for $i, j = 0, 1, 2$ then these curves are not $LS(3)-$equivalent.

3. if every $b_{i}\neq (0, 0, 0)$ and $p_{j}\neq (0, 0, 0)$ for $i, j = 0, 1, 2$ and

(a) the ranks of matrices of control points of each Bézier curves $X$ and $Y$ are equal to 3 then these Bézier curves are $LS(3)-$equivalent if and only if all of these ratio must be same for $i, j = 1, 2$ as follows:

$\frac{\left\langle p_{i}, p_{0}\right\rangle }{\left\langle b_{i}, b_{0}\right\rangle } = \frac{\left\langle p_{i}, p_{1}\right\rangle }{\left\langle b_{i}, b_{1}\right\rangle } = \frac{\left\langle p_{2}, p_{2}\right\rangle }{\left\langle b_{2}, b_{2}\right\rangle } = \frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle }$

are satisfied.

(b) the ranks of matrices of control points of each Bézier curves $X$ and $Y$ are equal to 2 then, these Bézier curves are $LS(3)-$equivalent if and only if for $i = 1, 2$

$\frac{\left\langle p_{i}, p_{0}\right\rangle }{\left\langle b_{i}, b_{0}\right\rangle } = \frac{\left\langle p_{i}, p_{1}\right\rangle }{\left\langle b_{i}, b_{1}\right\rangle } = \frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle }$

are satisfied.

(c) the ranks of matrice of control points of each Bézier curves $X$ and $Y$ are not equal to each other then, these Bézier curves are not $LS(3)-$equivalent.

Proof. This theorem is also a conclusion of Theorem 3.2. Since the Bézier Curves are quadratic in this theorem, the ranks of matrices of control points of each Bézier curves $X$ and $Y$ can be equal to 2 (in case the control points of these Bézier Curves lie the plane consisted of the origin point and two of control points) or 3 (other case).

1. If the ranks of matrices of control points of each Bézier curves $X$ and $Y$ can be equal to 3 then in this case from Theorem 3.2. for $i = 0, 1, 2$ and $i\leq j$, $\frac{\left\langle b_{i}, b_{j}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{i}, p_{j}\right\rangle }{\left\langle p_{0}, p_{0}\right\rangle }$; must be satisfied to be $X$ equivalent to $Y$. Then from this equalities

are obtained for every $i = 1, 2$.

2. If ranks of matrices of control points of each Bézier curves $X$ and $Y$ can be equal to 2 then

are satisfied. From these equalities, the conclusion that all of these ratios for $i = 1, 2$ must be equal are obtained as follows:

In Figure 2 $LS(3)-$equivalent quadratic Bézier curves $X(t)$ and $Y(t)$ are plotted.

4.3.   Cubic Bézier curves

A Cubic Bézier curve $X(t)$ with control points $b_{0}, b_{1}, b_{2}, b_{3}$ is defined by

where $t\in \lbrack 0, 1]$ ^[54].

Theorem 4.4. Let $X, Y$ be given two Cubic Bézier curves with control points ${b_{0}, b_{1}, b_{2}, b_{3}}$ and ${p_{0}, p_{1}, p_{2}, p_{3}}$ in $R^{3}$ respectively. So,

1. if any $b_{i} = (0, 0, 0)$ and $p_{i} = (0, 0, 0)$ for $i, j = 0, 1, 2, 3$ then these curves are $LS(3)-$equivalent if and only if the condition of third case of theorem 3.2 is satisfied excluding $b_{i}$ and $p_{i}$.

2. if any $b_{i} = (0, 0, 0)$ and each $p_{j}\neq (0, 0, 0)$ or each $b_{i}\neq (0, 0, 0)$ and any $p_{j} = (0, 0, 0)$ for $i, j = 0, 1, 2, 3$ then these curves are not $LS(3)-$equivalent.

3. if every $b_{i}\neq (0, 0, 0)$ and $p_{j}\neq (0, 0, 0)$ for $i, j = 0, 1, 2, 3$ and

(a) If the ranks of matrices of control points of each Bézier curves $X$ and $Y$ are equal to 3 then these Bézier curves are $LS(3)-$equivalent if and only if for $i = 1, 2, 3$ for $j = 2, 3$

$\frac{\left\langle p_{i}, p_{0}\right\rangle }{\left\langle b_{i}, b_{0}\right\rangle } = \frac{\left\langle p_{i}, p_{1}\right\rangle }{\left\langle b_{i}, b_{1}\right\rangle } = \frac{\left\langle p_{j}, p_{2}\right\rangle }{\left\langle b_{j}, b_{2}\right\rangle } = \frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle }$

are satisfied.

(b) If the ranks of matrices of control points of each Bézier curves $X$ and $Y$ are equal to 2 then, these Bézier curves are $LS(3)-$equivalent if and only if for $j = 1, 2, 3$

$\frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{0}, p_{j}\right\rangle }{\left\langle b_{0}, b_{j} \right\rangle} = \frac{\left\langle p_{1}, p_{j}\right\rangle }{\left\langle b_{1}, b_{j}\right\rangle}$

must be same.

(c) If the ranks of matrices of control points of each Bézier curves $X$ and $Y$ are not equal to each other then, these Bézier curves are not $LS(3)-$equivalent.

Proof. This theorem is also a conclusion of Theorem 3.2. Since the Bézier Curves are Cubic in this theorem, the ranks of matrices of control points of each Bézier curves $X$ and $Y$ can be equal to 2 or 3 like Theorem 4.3. If these ranks are equal to 3 then from Theorem 3.2. for $i = 1, 2, 3$ and $j = 2, 3$

can be written. Then from this,

are obtained.

If these ranks are equal to 2 then from Theorem 3.2. for $i = 0, 1$ and $j = 1, 2, 3$

is satisfied. So from this for $j = 1, 2, 3$

can be written. And so for $j = 1, 2, 3$ these equalities of ratios

are obtained.

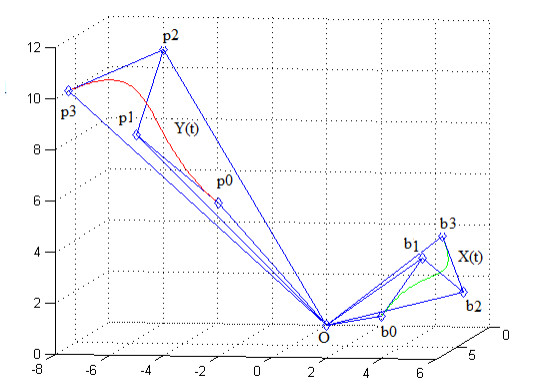
In Figure 3 $LS(3)-$equivalent Cubic Bézier curves $X(t)$ and $Y(t)$ are graphed.

4.4.   General Bézier curves

Theorem 4.5. Let $X, Y$ be given two general Bézier curves of degree $n$ with control points ${b_{0}, b_{1}, b_{2}, ..., b_{n}}$ and ${p_{0}, p_{1}, p_{2}, ..., p_{n}}$ in $R^{3}$ respectively. So,

1. if any $b_{i} = (0, 0, 0)$ and any $p_{i} = (0, 0, 0)$ for $i = 0, 1, 2, ..., n$ then these curves are $LS(3)-$equivalent if and only if the condition of third case of theorem 3.2 is satisfied excluding $b_{i}$ and $p_{i}$.

2. if any $b_{i} = (0, 0, 0)$ and each $p_{j}\neq (0, 0, 0)$ or each $b_{i}\neq(0, 0, 0)$ and any $p_{j} = (0, 0, 0)$ for $i, j = 0, 1, 2, ..., n$ then these curves are not $LS(3)-$equivalent.

3. if every $b_{i}\neq (0, 0, 0)$ and $p_{j}\neq (0, 0, 0)$ for $i, j = 0, 1, 2, ..., n$ then these Bézier curves are $LS(3)-$equivalent if and only if

(a) for $i, j = 0, 1, 2$; $k = 3, ..., n$ and $i\leq j$.

must be same where $i, j = 0, 1, 2$; $k = 3, ..., n$ and $i\leq j$ if the ranks of matrices of control points of each Bézier curves $X$ and $Y$ are equal to 3.

(b)

it is satisfied if the ranks of matrices of control points of each Bézier curves $X$ and $Y$ are equal to 2 where $i, j = 0, 1$; $k = 2, ..., n$ and $i\leq j$.

Proof. This theorem is also a conclusion of Theorem 3.2. The ranks of matrices of control points of each Bézier curves $X$ and $Y$ can be equal to 2 or 3 similarly.

If these raks are equal to 3 then from Theorem 3.2. for $i, j = 0, 1, 2$; $k = 3, ..., n$ and $i\leq j$.

can be written. So from this for $i, j = 0, 1, 2$; $k = 3, ..., n$ and $i\leq j$.

must be same.

If these raks are equal to 2 then from Theorem 3.2. for $i, j = 0, 1$; $k = 2, ..., n$ and $i\leq j$.

can be written. So from this for $i, j = 0, 1$; $k = 2, ..., n$ and $i\leq j$ it is obtained that

must be same.

5.   Application to Bézier surfaces

A general (n, m)- Typed Bézier Surface $X(t, u)$ with control points $b_{ij}$ in $R^{3}$ for $i = 0, 1, ..., n$ and $j = 0, 1, ..., m$ is defined by

where $B_{i}^{n}\left(u\right), B_{j}^{m}\left(v\right)$ are the Bernstein basis functions and $t, u\in \lbrack 0, 1]$ ^[54].

The parameter curves of a Bézier surface are spatial Bézier curves. In particular, the parameter curves X(t, 0), X(t, 1), X(0, u), X(1, u), are Bézier curves which form the four edges of the Bézier surface. A number of the properties of Bézier and B-spline surfaces can be deduced in a similar manner to the corresponding properties for curves. The details are omitted.

Theorem 5.1. (Invariance under Affine Transformations property) Let $X(t, u)$ be given a general (n, m) Typed Bézier Surfaces with control points $b_{ij}$ for $i = 0, 1, ..., n$ and $j = 0, 1, ..., m$ in $R^{3}$. So The Bézier surface $X(t, u)$ satisfies as follow:

Let $T$ be a affine transformation. Then ^[54].

Definition: Let $X(t, u)$ and $Y(t, u)$ be given two general (n, m) Typed Bézier Surfaces with control points $b_{ij}$ and $q_{ij}$ for $i = 0, 1, ..., n$ and $j = 0, 1, ..., m$ in $R^{3}$. Then these Bézier Surfaces are called $LS(3)-$equivalent if there exist a transformation $g \in LS(3)$ such that $q_{ij} = g b_{ij}$ for every $i = 0, 1, ..., n$ and $j = 0, 1, ..., m$ are satisfied.

5.1.   (1, 1) Typed Bézier surfaces

A (1, 1) Typed Bézier Surface $X(u, v)$ with control points $b_{00}, b_{01}, b_{10}, b_{11}$ is defined by

where $t, u\in \lbrack 0, 1]$ ^[54].

Theorem 5.1. Let $X(t, u)$ and $Y(t, u)$ be given two (1, 1) Typed Bézier Surfaces with control points ${b_{00}, b_{01}}, {b_{10}, b_{11}}$ and ${p_{00}, p_{01}, {p_{10}, p_{11}}}$ in $R^{3}$respectively. So,

1. if any $b_{ij} = (0, 0, 0)$ and each $p_{ij}\neq (0, 0, 0)$ or each $b_{ij}\neq (0, 0, 0)$ and any $p_{ij} = (0, 0, 0)$ for $i, j = 0, 1$ then these surfaces are not $LS(3)-$equivalent.

2. if every $b_{ij}\neq (0, 0, 0)$ and $p_{ij}\neq (0, 0, 0)$ for $i, j = 0, 1$ then these Bézier surfaces are $LS(3)-$equivalent if and only if the proportions $\frac{\left\langle p_{_{_{ij}}}, p_{_{_{sk}}}\right\rangle }{ \left\langle b_{_{ij}}, b_{_{sk}}\right\rangle }$ are the same for $i, j = 0, 1$ and for $s, k = 0, 1$

Proof. The coordinat curves $X(0, u), X(1, u), X(t, 0), X(t, 1)$ and $Y(0, u), Y(1, u), Y(t, 0), Y(t, 1)$ of every Bézier surfaces are Bézier curves. So to be $LS(3)-$equivalent of two Bézier surfaces $X(t, u)$ and $Y(t, u)$, the Bézier curves with control points $\left\{ {b_{00}, b_{01}}\right\}$, $\left\{ {b_{00}, b_{10}}\right\}, \left\{ {b_{01}, b_{11}}\right\}, \left\{ {b_{10}, b_{11}}\right\}$ and $\left\{ {p_{00}, p_{01}}\right\}$, $\left\{ { p_{00}, p_{10}}\right\}, \left\{ {p_{01}, p_{11}}\right\}, \left\{ {p_{10}, p_{11}}\right\}$ must be mutually $LS(3)-$equivalent. This means that $\left\{ {b_{00}, b_{01}}\right\} \overset{LS(3)}{\cong }\ \left\{ {p_{00}, p_{01}}\right\}$, $\left\{ {b_{00}, b_{10}}\right\} \overset{LS(3)}{\cong }\ \left\{ {p_{00}, p_{10}}\right\}$, $\left\{ {b_{01}, b_{11}}\right\} \overset{LS(3)}{\cong }\left\{ {p_{01}, p_{11}}\right\}$, $\left\{ {b_{10}, b_{11}}\right\} \overset{LS(3)}{\cong }$ $\left\{ {p_{10}, p_{11}}\right\}$ must be separately satisfied. So $\frac{\left\langle p_{00}, p_{00}\right\rangle }{\left\langle b_{00}, b_{00}\right\rangle } = \frac{\left\langle p_{00}, p_{01}\right\rangle }{\left\langle b_{00}, b_{01}\right\rangle } = \frac{\left\langle p_{01}, p_{01}\right\rangle }{\left\langle b_{01}, b_{01}\right\rangle } = \frac{\left\langle p_{00}, p_{10}\right\rangle }{\left\langle b_{00}, b_{10}\right\rangle } = \frac{\left\langle p_{10}, p_{10}\right\rangle }{\left\langle b_{10}, b_{10}\right\rangle } =$ $\frac{\left\langle p_{10}, p_{11}\right\rangle }{\left\langle b_{10}, b_{11}\right\rangle } = \frac{\left\langle p_{01}, p_{11}\right\rangle }{\left\langle b_{01}, b_{11}\right\rangle } = \frac{\left\langle p_{11}, p_{11}\right\rangle }{\left\langle b_{11}, b_{11}\right\rangle }$ is obtained.

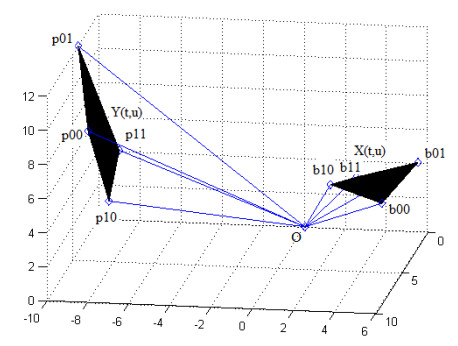
In Figure 4 $LS(3)-$equivalent (1, 1) Typed Bézier surfaces $X(t, u)$ and $Y(t, u)$ are plotted.

5.2.   (1, 2) or (2, 1)-Typed Bézier surfaces

A (1, 2)-Typed Bézier surface $X(u, v)$ with control points $b_{00}, b_{01}, b_{02}, b_{10}, b_{11}, b_{12}$ is defined by

where $u, v\in \lbrack 0, 1]$ ^[54].

A (2, 1)-Typed Bézier Surface $X(u, v)$ with control points $b_{00}, b_{01}, b_{10}, b_{11}, b_{20}, b_{21}$ is defined by

where $u, v\in \lbrack 0, 1]$ ^[54].

Theorem 5.2. Let $X(t, u)$ and $Y(t, u)$ be given two (2, 1) Typed Bézier Surfaces with control points $b_{00}, b_{01}, b_{10}, b_{11}, b_{20}, b_{21}$ and $p_{00}, p_{01}, p_{10}, p_{11}, p_{20}, p_{21}$ in $R^{3}$respectively. So,

1. if any $b_{ij} = (0, 0, 0)$ and each $p_{ij}\neq (0, 0, 0)$ or each $b_{ij}\neq (0, 0, 0)$ and any $p_{ij} = (0, 0, 0)$ for $i = 0, 1, 2$ and $j = 0, 1$ then these surfaces are not $LS(3)-$equivalent.

2. if every $b_{ij}\neq (0, 0, 0)$ and $p_{ij}\neq (0, 0, 0)$ for $i = 0, 1, 2$ and $j = 0, 1$ then these Bézier surfaces are $LS(3)-$equivalent if and only if these ratios or proportions $\frac{\left\langle p_{i0}, p_{j0}\right\rangle }{\left\langle b_{i0}, b_{j0}\right\rangle }, \frac{\left\langle p_{i1}, p_{j1}\right\rangle }{\left\langle b_{i1}, b_{j1}\right\rangle }$ are the same for $i, j = 0, 1, 2$.

Proof. Let $X(t, u)$ and $Y(t, u)$ be given two (2, 1) Typed Bézier Surfaces with control points $b_{00}, b_{01}, b_{10}, b_{11}, b_{20}, b_{21}$ and $p_{00}, p_{01}, p_{10}, p_{11}, p_{20}, p_{21}$ in $R^{3}$respectively. So the coordinat curves $X(0, u), X(1, u), X(t, 0), X(t, 1)$ and $Y(0, u), Y(1, u), Y(t, 0), Y(t, 1)$ of every Bézier surfaces must be mutually $LS(3)-$equivalent. i.e. $\left\{ {b_{00}, b_{01}}\right\} \overset{LS(3)}{\cong }\ \left\{ {p_{00}, p_{01}}\right\}$, $\left\{ {b_{00}, b_{10}, b_{20}}\right\} \overset{LS(3)}{\cong }\ \left\{ {p_{00}, p_{10}, p_{20}}\right\}$, $\left\{ {b_{01}, b_{11}, b_{21}}\right\} \overset{LS(3)}{\cong }\ \left\{ {p_{01}, p_{11}, p_{21}}\right\}$, $\left\{ {b_{20}, b_{21}}\right\} \overset{LS(3)}{\cong }$ $\left\{ {p_{20}, p_{21}}\right\}$ must be satisfied. From Theorem 4.2 and Theorem 4.3, these equalities $\frac{\left\langle p_{00}, p_{00}\right\rangle }{\left\langle b_{00}, b_{00}\right\rangle } = \frac{\left\langle p_{00}, p_{01}\right\rangle }{\left\langle b_{00}, b_{01}\right\rangle } = \frac{\left\langle p_{01}, p_{01}\right\rangle }{\left\langle b_{01}, b_{01}\right\rangle }$; $\frac{\left\langle p_{00}, p_{00}\right\rangle }{\left\langle b_{00}, b_{00}\right\rangle } = \frac{\left\langle p_{00}, p_{10}\right\rangle }{\left\langle b_{00}, b_{10}\right\rangle } = \frac{\left\langle p_{00}, p_{20}\right\rangle }{\left\langle b_{00}, b_{20}\right\rangle } = \frac{\left\langle p_{10}, p_{10}\right\rangle }{\left\langle b_{10}, b_{10}\right\rangle } = \frac{\left\langle p_{10}, p_{20}\right\rangle }{\left\langle b_{10}, b_{20}\right\rangle } = \frac{\left\langle p_{20}, p_{20}\right\rangle }{ \left\langle b_{20}, b_{20}\right\rangle }$; $\frac{\left\langle p_{01}, p_{01}\right\rangle }{\left\langle b_{01}, b_{01}\right\rangle } = \frac{\left\langle p_{01}, p_{11}\right\rangle }{\left\langle b_{01}, b_{11}\right\rangle } = \frac{\left\langle p_{01}, p_{21}\right\rangle }{\left\langle b_{01}, b_{21}\right\rangle } = \frac{\left\langle p_{11}, p_{11}\right\rangle }{\left\langle b_{11}, b_{11}\right\rangle } = \frac{\left\langle p_{11}, p_{21}\right\rangle }{\left\langle b_{11}, b_{21}\right\rangle } = \frac{\left\langle p_{21}, p_{21}\right\rangle }{\left\langle b_{21}, b_{21}\right\rangle }$; $\frac{\left\langle p_{20}, p_{20}\right\rangle }{\left\langle b_{20}, b_{20}\right\rangle } = \frac{\left\langle p_{20}, p_{21}\right\rangle }{\left\langle b_{20}, b_{21}\right\rangle } = \frac{\left\langle p_{21}, p_{21}\right\rangle }{\left\langle b_{21}, b_{21}\right\rangle }$ can be obtained. So the minimal equivalent conditions of these surfaces is these ratios or proportions $\frac{\left\langle p_{i0}, p_{j0}\right\rangle }{\left\langle b_{i0}, b_{j0}\right\rangle }, \frac{\left\langle p_{i1}, p_{j1}\right\rangle }{\left\langle b_{i1}, b_{j1}\right\rangle }$ are the same for $i, j = 0, 1, 2$.

Theorem 5.3. Let $X(t, u)$ and $Y(t, u)$ be given two (1, 2) Typed Bézier Surfaces with control points $b_{00}, b_{01}, b_{02}, b_{10}, b_{11}, b_{12}$ and $p_{00}, p_{01}, p_{02}, p_{10}, p_{11}, p_{12}$ in $R^{3}$respectively. So,

1. if any $b_{ij} = (0, 0, 0)$ and each $p_{ij}\neq (0, 0, 0)$ or each $b_{ij}\neq (0, 0, 0)$ and any $p_{ij} = (0, 0, 0)$ for $i = 0, 1$ and $j = 0, 1, 2$ then these surfaces are not $LS(3)-$equivalent.

2. if every $b_{ij}\neq (0, 0, 0)$ and $p_{ij}\neq (0, 0, 0)$ for $i = 0, 1$ and $j = 0, 1, 2$ then these Bézier surfaces are $LS(3)-$equivalent if and only if these ratios or proportions $\frac{\left\langle p_{0i}, p_{0j}\right\rangle }{\left\langle b_{0i}, b_{0j}\right\rangle }, \frac{\left\langle p_{1i}, p_{1j}\right\rangle }{\left\langle b_{1i}, b_{1j}\right\rangle }$ are the same for $i, j = 0, 1, 2$.

Proof. As Theorem 5.2., let $X(t, u)$ and $Y(t, u)$ be given two (1, 2) Typed Bézier Surfaces with control points $b_{00}, b_{01}, b_{02}, b_{10}, b_{11}, b_{12}$ and $p_{00}, p_{01}, p_{02}, p_{10}, p_{11}, p_{12}$ in $R^{3}$respectively. So the coordinat curves $X(0, u), X(1, u), X(t, 0), X(t, 1)$ and $Y(0, u), Y(1, u), Y(t, 0), Y(t, 1)$ of every Bézier surfaces must be mutually $LS(3)-$equivalent. i.e. $\left\{ {b_{00}, b_{10}}\right\} \overset{LS(3)}{ \cong }\ \left\{ {p_{00}, p_{10}}\right\}$, $\left\{ {b_{00}, b_{01}, b_{02}}\right\} \overset{LS(3)}{\cong }\ \left\{ {p_{00}, p_{01}, p_{02}}\right\}$, $\left\{ {b_{10}, b_{11}, b_{12}}\right\} \overset{LS(3)}{\cong }\ \left\{ { p_{10}, p_{11}, p_{12}}\right\}$, $\left\{ {b_{02}, b_{12}}\right\} \overset{LS(3)}{\cong }$ $\left\{ {p_{02}, p_{12}}\right\}$ must be satisfied. From Theorem 4.2 and Theorem 4.3, these ratios or proportions $\frac{\left\langle p_{i0}, p_{j0}\right\rangle }{\left\langle b_{i0}, b_{j0}\right\rangle }, \frac{ \left\langle p_{i1}, p_{j1}\right\rangle }{\left\langle b_{i1}, b_{j1}\right\rangle }$ are the same for $i, j = 0, 1, 2$. can be obtained similarly with Theorem 5.2.

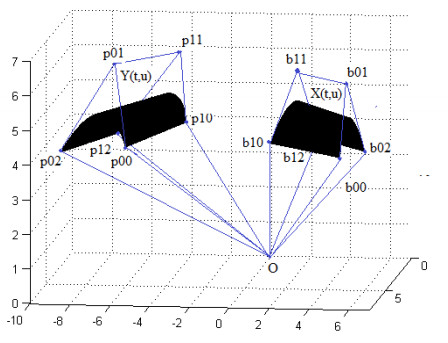
In Figures 5 and 6 $LS(3)-$equivalent (1, 2) Typed and (2, 1) Typed Bézier surfaces $X(t, u)$ and $Y(t, u)$ are plotted respectively.

5.3.   General (n, m)-Typed Bézier surfaces

A general (n, m)-Typed Bézier Surface $X(u, v)$ with control points $b_{ij}$ for $i = 0, 1, ..., n$ and $j = 0, 1, ..., m$ in $R^{3}$ is defined by

where $B_{i}^{n}\left(u\right), B_{j}^{m}\left(v\right)$ are the Bernstein basis functions and $t, u\in \lbrack 0, 1]$ ^[54].

Theorem 5.4. Let $X(t, u)$ and $Y(t, u)$ be given two general $(n, m)$ Typed Bézier Surfaces with control points $b_{ij}$ and $p_{ij}$ for $i = 0, 1, ..., n$ and $j = 0, 1, ..., m$ in $R^{3}$respectively. So,

1. if any $b_{ij} = (0, 0, 0)$ and each $p_{ij}\neq (0, 0, 0)$ or each $b_{ij}\neq (0, 0, 0)$ and any $p_{ij} = (0, 0, 0)$ then these surfaces are not $LS(3)-$equivalent.

2. if every $b_{ij}\neq (0, 0, 0)$ and $p_{ij}\neq (0, 0, 0)$ then these Bézier surfaces are $LS(3)-$equivalent if and only if

$\frac{\left\langle p_{00}, p_{u0}\right\rangle }{\left\langle b_{00}, b_{u0}\right\rangle }$, $\frac{\left\langle p_{10}, p_{v0}\right\rangle }{\left\langle b_{10}, b_{v0}\right\rangle }$, $\frac{\left\langle p_{20}, p_{w0}\right\rangle }{\left\langle b_{20}, b_{w0}\right\rangle }$; $\frac{\left\langle p_{00}, p_{0i}\right\rangle }{\left\langle b_{00}, b_{0i}\right\rangle }$, $\frac{\left\langle p_{01}, p_{0j}\right\rangle }{\left\langle b_{01}, b_{0j}\right\rangle }$, $\frac{\left\langle p_{02}, p_{0k}\right\rangle }{\left\langle b_{02}, b_{0k}\right\rangle }$; $\frac{\left\langle p_{0m}, p_{im}\right\rangle }{\left\langle b_{0m}, b_{im}\right\rangle }$, $\frac{\left\langle p_{1m}, p_{jm}\right\rangle }{\left\langle b_{1m}, b_{jm}\right\rangle }$, $\frac{\left\langle p_{2m}, p_{km}\right\rangle }{\left\langle b_{2m}, b_{km}\right\rangle }$; $\frac{\left\langle p_{n0}, p_{ni}\right\rangle }{\left\langle b_{n0}, b_{ni}\right\rangle }$, $\frac{\left\langle p_{n1}, p_{nj}\right\rangle }{\left\langle b_{n1}, b_{nj}\right\rangle }$, $\frac{\left\langle p_{n2}, p_{nk}\right\rangle }{\left\langle b_{n2}, b_{nk}\right\rangle }$ for $u = 0, 1, ..., n$, $v = 1, ..., n$; $w = 2, ..., n$, $i = 0, 1, ..., m$, $j = 1, ..., m$; $k = 2, ..., m$ must be same.

Proof. As Theorem 5.2. and Theorem 5.3., let $X(t, u)$ and $Y(t, u)$ be given two $(n, m)$ Typed Bézier Surfaces with control points $b_{ij}$ and $p_{ij}$ for $i = 0, 1, ..., n$ and $j = 0, 1, ..., m$ in $R^{3}$ respectively. So the coordinat curves $X(0, u), X(1, u), X(t, 0), X(t, 1)$ and $Y(0, u), Y(1, u), Y(t, 0), Y(t, 1)$ of each Bézier surfaces must be mutually $LS(3)-$equivalent. i.e. $\left\{ {b_{00}, b_{10}, ..., b}_{n0}\right\} \overset{ LS(3)}{\cong }\ \left\{ {p_{00}, p_{10}, ..., p}_{n0}\right\}$, $\left\{ { b_{00}, b_{01}, ..., b_{0m}}\right\} \overset{LS(3)}{\cong }\ \left\{ { p_{00}, p_{01}, ..., p_{0m}}\right\}$, $\left\{ {b_{n0}, b_{n1}, ..., b_{nm}} \right\} \overset{LS(3)}{\cong }\ \left\{ {p_{n0}, p_{n1}, ..., p_{nm}}\right\}$, $\left\{ {b_{0m}, b_{1m}, ..., b}_{nm}\right\} \overset{LS(3)}{\cong }$ $\left\{ {p_{0m}, p_{1m}, ..., p}_{nm}\right\}$ must be satisfied. Then from Theorem 4.5. these proportions $\frac{\left\langle p_{00}, p_{u0}\right\rangle }{\left\langle b_{00}, b_{u0}\right\rangle }$, $\frac{\left\langle p_{10}, p_{v0}\right\rangle }{\left\langle b_{10}, b_{v0}\right\rangle }$, $\frac{\left\langle p_{20}, p_{w0}\right\rangle }{\left\langle b_{20}, b_{w0}\right\rangle }$; $\frac{\left\langle p_{00}, p_{0i}\right\rangle }{\left\langle b_{00}, b_{0i}\right\rangle }$, $\frac{\left\langle p_{01}, p_{0j}\right\rangle }{\left\langle b_{01}, b_{0j}\right\rangle }$, $\frac{\left\langle p_{02}, p_{0k}\right\rangle }{\left\langle b_{02}, b_{0k}\right\rangle }$; $\frac{\left\langle p_{0m}, p_{im}\right\rangle }{\left\langle b_{0m}, b_{im}\right\rangle }$, $\frac{\left\langle p_{1m}, p_{jm}\right\rangle }{\left\langle b_{1m}, b_{jm}\right\rangle }$, $\frac{\left\langle p_{2m}, p_{km}\right\rangle }{\left\langle b_{2m}, b_{km}\right\rangle }$; $\frac{\left\langle p_{n0}, p_{ni}\right\rangle }{\left\langle b_{n0}, b_{ni}\right\rangle }$, $\frac{\left\langle p_{n1}, p_{nj}\right\rangle }{\left\langle b_{n1}, b_{nj}\right\rangle }$, $\frac{\left\langle p_{n2}, p_{nk}\right\rangle }{\left\langle b_{n2}, b_{nk}\right\rangle }$ for $u = 0, 1, ..., n$, $v = 1, ..., n$; $w = 2, ..., n$, $i = 0, 1, ..., m$, $j = 1, ..., m$; $k = 2, ..., m$ must be same.

6.   An example of the application of Bézier curves to CAD: The font design

One of the most important applicaitons of Bézier curves in CAD systems is font design. Mostly quadratic and Cubic Bézier curves are used in this area. Higher degree curves are more computationally expensive to evaluate. When more complex shapes are needed, low order Bézier curves are patched together, producing a composite Bézier curve. A composite Bézier curve is commonly referred to as a "path" in vector graphics languages (like PostScript), vector graphics standards (like SVG) and vector graphics programs (like Artline, Timeworks Publisher, Adobe Illustrator, CorelDraw and Inkscape) ^[55].

TrueType fonts use composite Bézier curves composed of quadratic Bézier curves. Other languages and imaging tools (such as PostScript, Asymptote, Metafont, and SVG) use composite Béziers composed of Cubic Bézier curves for drawing curved shapes. OpenType fonts can use either kind, depending on the flavor of the font. ^[55,56]. Some other studies can be seen in ^[38,57,58]. An example to design a typeface of letter 's' can be given as follows:

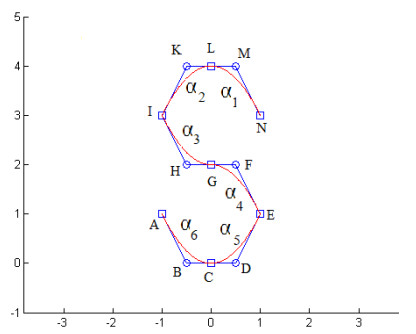
To design a typeface of letter S the anchor and other control points are determined firstly. The anchor points lie on the curve "s", and other control points are outside of this curve. Let's use composite Bézier curves composed of quadratic Bézier curves even though it has disadvantages such as the need to add more anchor nodes and big file sizes. This typeface have 7 anchor points and 6 other control points (see in Figure 7). These points can be choose as follows:

The anchor points: $A = (-1, 1)$, $C = (0, 0)$, $E = (1, 1)$, $G = (0, 2)$, $I = (-1, 3)$, $L = (0, 4)$, and $N = (1, 3)$. The other control points $B = (-0.5, 0)$, $D = (0.5, 0)$, $F = (0.5, 2)$, $H = (-0.5, 2)$, $K = (-0.5, 4)$, $M = (0.5, 4)$.

If these curve segments on the letter "S" are denoted as follows:

From the point $N$ to the point $L$ as $\alpha_{1}$, from the point $L$ to the point $I$ as $\alpha_{2}$, from the point $I$ to the point $G$ as $\alpha_{3}$, from the point $G$ to the point $E$ as $\alpha_{4}$, from the point $E$ to the point $C$ as $\alpha_{5}$ and from the point $C$ to the point $A$ as $\alpha_{6}$ then these quadratic Bézier curves $\alpha_{1}$, $\alpha_{2}$, $\alpha_{3}$, $\alpha_{4}$, $\alpha_{5}$, and $\alpha_{6}$ form the composite Bézier curve $\alpha$.

So these points $N, M, L; L, K, I; I, H, G; G, F, E; E, D, C; C, B, A$ are the control points of these quadratic Bézier curves $\alpha_{i}$ respectively for $i = 1, ..., 6$.

Considering the first coordinates as 0, these points A to N can be recognized as in 3 dimensional points. Now let's apply any $g \in LS(3)$ transform to this created letter S. It can be used to obtain different font shadows.Let the transform $g$ be choosen as $g = \left(\begin{array}{ccc} 0 & 0 & -1\\ 0 & 1 & 0 \\ 1 & 0 & 0 \\ \end{array} \right)$

which rotates all points with 90 degree in the $xz-$plane.

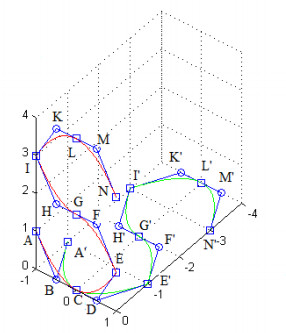
The points A to N transform the points A' to N' with this transformation $g$. Thus, the anchor points transform to these points: $A' = (-1, -1, 0)$, $C' = (0, 0, 0)$, $E' = (-1, 1, 0)$, $G' = (-2, 0, 0)$, $I' = (-3, -1, 0)$, $L' = (-4, 0, 0)$, and $N' = (-3, 1, 0)$. The other control points transform to points $B' = (0, -0.5, 0)$, $D' = (0, 0.5, 0)$, $F' = (-2, 0.5, 0)$, $H' = (-2, -0.5, 0)$, $K' = (-4, -0.5, 0)$, $M' = (-4, 0.5, 0)$. From Theorem 4.1 the composite Bézier curve $\alpha$ transform the composite Bézier curve $\beta$ consisted of $\beta_{i}$ for $i = 1, ..., 6$ with this transformation $g$. From Theorem 4.1 all of these curves $\beta_{i}$ are also quadratic Bézier curves which control points $N', M', L'; L', K', I'; I', H', G'; G', F', E'; E', D', C'; C', B', A'$ respectively for $i = 1, ..., 6$. According to this the control points A to N are $LS(3)$-equivalent to the points A' to N'. So the composite Bézier curve $\alpha$ is $LS(3)$-equivalent to the composite Bézier curve $\beta$. In Figure 8 the composite Bézier curves $\alpha$ and $\beta$ is given together.

Let the transform $g$ be choosen as $g = \left(\begin{array}{ccc} 0 & -\sqrt{2} & -\sqrt{2} \\ 0 & \sqrt{2} & -\sqrt{2} \\ 2 & 0 & 0 \\ \end{array} \right)$

which rotates all points with 90 degree in the $xz-$ plane and rotates all points with 45 degree in the $xy-$ plane with scale $\lambda = 2$.

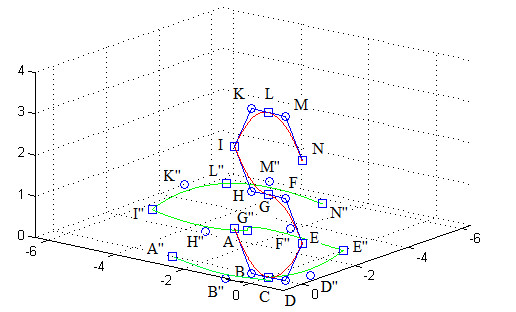
The points A to N transform the points A" to N" with this transformation $g$. Thus, the anchor points transform to these points: $A'' = (0, -2\sqrt{2}, 0)$, $C'' = (0, 0, 0)$, $E'' = (-2\sqrt{2}, 0, 0)$, $G'' = (-2\sqrt{2}, -2\sqrt{2}, 0)$, $I'' = (-2\sqrt{2}, -4\sqrt{2}, 0)$, $L'' = (-4\sqrt{2}, -4\sqrt{2}, 0)$, and $N'' = (-4\sqrt{2}, -2\sqrt{2}, 0)$. The other control points transform to points $B'' = (\frac{\sqrt{2}}{2}, -\frac{\sqrt{2}}{2}, 0))$, $D'' = (-\frac{\sqrt{2}}{2}, \frac{\sqrt{2}}{2}, 0)$, $F'' = (-5\frac{\sqrt{2}}{2}, -3\frac{\sqrt{2}}{2}, 0)$, $H'' = (-3\frac{\sqrt{2}}{2}, -5\frac{\sqrt{2}}{2}, 0)$, $K'' = (-7\frac{\sqrt{2}}{2}, -9\frac{\sqrt{2}}{2}, 0)$, $M'' = (-9\frac{\sqrt{2}}{2}, -7\frac{\sqrt{2}}{2}, 0)$. Also From Theorem 4.1 the composite Bézier curve $\alpha$ transform the composite Bézier curve $\gamma$ consisted of $\gamma_{i}$ for $i = 1, ..., 6$ with this transformation $g$. From Theorem 4.1 all of these curves $\gamma_{i}$ are also quadratic Bézier curves which control points $N'', M'', L''$, $L'', K'', I''$, $I'', H'', G''$, $G'', F'', E''$, $E'', D'', C''$, $C'', B'', A''$ respectively for $i = 1, ..., 6$. According to this the control points A to N are $LS(3)$-equivalent to the points $A''$ to $N''$. So the composite Bézier curve $\alpha$ is $LS(3)$-equivalent to the composite Bézier curve $\gamma$. In Figure 9 the composite Bézier curves $\alpha$ and $\gamma$ is given together.

In the other view if a shadow of a typeface could not read clearly then the operation process mentioned above can be apply by reversed to obtain more readable typefaces.

The authors are grateful to the editors and referees for giving every useful piece of information they have checked and developed.

Conflict of interest

The authors declare no conflict of interest.