A novel technique on flexibility and adjustability of generalized fractional Bézier surface patch

1.   Introduction

In Computer Aided Geometric Design (CAGD) and Computer-Aided Manufacturing, the development of curves and surfaces is crucial. Researchers studied the representation of free-form curves and surfaces, applying them in numerous fields, such as engineering, manufacturing and designing. In real-world problems, most curves and surfaces are complex to model. Therefore, having a tool to create flexible and adjustable curves and surfaces becomes necessary to model any complex shapes. However, finding a representation of desired complex curves and surfaces is difficult and impractical ^[2,3]. To tackle the problem of modeling the complex shapes, the curves and surfaces are broken down into simpler curves and patches, joining them to form the complex curves or surfaces. To join such curves or surfaces, a continuity concept is applied to the adjacent curves or surfaces.

Generally, the higher the order of continuity, the smoother the curves or surfaces are connected. Therefore, connecting the curves or surfaces is important with a high degree of continuity. Two standard metrics have been used in connecting these curves and surfaces: parametric and geometric continuity. Parametric continuity of order $r$, or $C^r$ continuity, is the simplest form of the continuity concept. Nonetheless, parametric continuity has its restrictions. For example, when the surfaces are connected by $C^1$ continuity, they still need to possess a common tangent at their boundary points. Hence, parametric continuity cannot be the only exact standard method in constructing smooth curves or surfaces ^[4]. As a result, researchers have developed an upgraded version of parametric continuity called geometric continuity, or $G^r$ continuity. $G^r$ continuity is the less restrictive form where scale factors are embedded in the continuity. These scale factors overcome a common tangent for the curves or surfaces.

For shape designing and geometric representation in CAGD, parametric curves and surfaces are useful tools. Classical Bézier curves and surfaces are one of many parametric curves or surfaces. The Bézier method has become one of the famous methods in modeling curves and surfaces due to the simple formulation and excellent geometric properties ^[5]. However, there is a constraint in the classical Bézier method; the shape of curves or surfaces cannot be changed without changing the control points. To overcome this constraint, researchers have developed aesthetic Bézier curves and surfaces that still preserve the excellent features of classical Bézier curves and surfaces but with added flexibility and adjustability. Rational Bézier curve is one of the aesthetic curves that was introduced earlier. The weight factors in rational Bézier curves and surfaces allow shape modification without altering the control points ^[6]. However, compared to the classical Bézier curves and surfaces, the rational Bézier curves and surfaces have considerably more difficult computation, convoluted integrals and repeated differentiation, since rational functions define them ^[7].

To overcome the limitations of the rational Bézier, scholars have created several Bézier curves and surfaces with shape parameters to preserve the benefits of the Bézier model and increase the shape adjustability of curves and surfaces. With three shape parameters, ^[7] created enhanced classical Bézier curves and surfaces dubbed the shape-adjustable generalized Bézier (or SG-Bézier for short). For example, ^[8] presented a class of quasi-quintic trigonometric Bézier curves with two shape parameters. Moreover, ^[9] produced quintic trigonometric Bézier basis functions with two shape parameters. The generalized Hybrid-Trigonometric Bézier (GHT-Bézier) basis functions with embedding exponential and trigonometric functions along with shape parameters have been formulated by ^[10]. On the other hand, ^[11] proposed new cubic hyperbolic Bézier basis functions derived from hyperbolic functions.

Researchers have used these aesthetic Bézier curves to develop complex curves and surfaces using continuity. $GC^1$ continuity conditions between adjacent rectangular and triangular Bézier surface patches have been developed by ^[12]. On the other hand, $G^1$ continuity of piecewise Bézier surfaces has been discussed in ^[13]. Meanwhile, ^[14] discussed $G^2$ continuity for conditions for generalized Bézier-like surfaces with multiple shape parameters. Surface construction using $C^2$ continuity for quintic trigonometric Bézier curves is proposed in ^[15]. Furthermore, ^[16] proposed $G^2$ continuity for the biquintic trigonometric surface and modeled a few engineering surfaces. In addition, SG-Bézier surfaces have been modeled up until $G^2$ continuity in ^[17], while ^[18] constructed the GHT-Bézier surfaces up until $G^2$ continuity. Continuity also has been used in the modeling of the developable surface, while developable $\lambda$-Bézier surfaces have been discussed with their geometric design and continuity conditions up until $G^2$ in ^[19]. Apart from that, ^[20] introduced the generalized developable cubic trigonometric surface up to $G^2$ continuity. Moreover, ^[21] developed a generalized developable hybrid trigonometric surface up until $G^3$ continuity.

$C^r$ and $G^r$ continuity enable modeling complicated curves and surfaces by joining several simple curves and surfaces to form the desired curves and surfaces. Nevertheless, the existing continuity has significant limitations, in which the curves or surfaces can only be connected at the endpoints or boundary points. For example, if the designers want to connect the midpoint of the first curve to the second curve, it can only be done by splicing the first curve using the subdivision method first. Then, after the curve splicing, the new curve will join the second curve using continuity. The subdivision method is good for splicing the curve or surfaces, but it has a high computational time when involving a higher degree curve.

Recently, ^[22] has developed an improved version of geometric continuity dubbed fractional continuity or $F^r$ continuity and connects the curves up until $F^2$. Here, the fractional continuity enables the curves or surfaces to be connected at the endpoints or boundary points and any arbitrary point on the first curve and arbitrary line (according to $u$ or $v$ direction) of the first surface as well. The fractional parameter embedded in fractional continuity is the key to the development of fractional continuity. This paper will discuss the $F^2$ continuity for the generalized fractional Bézier surfaces. The fractional parameter will become a key point in the fractional continuity. By varying the fractional parameter, the common boundary between two surfaces can be adjusted along the first surface while preserving the degree of continuity. Shape parameters are also included in the generalized fractional Bézier surface. Hence, shape parameters will be added as an additional tool for the designer to change the shape of the surface.

The work is organized as follows. The generalized fractional Bézier basis functions are defined in Section 2. Section 3 discussed $F^2$ continuity conditions between three consecutive curves. In Section 4, the definition and the properties of the generalized fractional Bézier tensor product surface are explained. Next, in Section 5, the $F^2$ continuity conditions for the generalized fractional Bézier surfaces are discussed. The procedures and some examples for the $F^2$ continuity will be shown in Section 6. Last but not least, in Section 7, some conclusions and recommendations for future work will be addressed.

2.   Generalized fractional Bézier basis functions

Generalized fractional Bézier basis functions

Definition 2.1 (Riemann-Liouville fractional integral for $f(t) = t$). Let $Re(w) > 0$ and $f(t) = t$. Then, for $t > 0$, the Riemann-Liouville fractional integral of $f(t) = t$ of order $w$ is as follows:

The general form and derivation of the definition can be seen in ^[1].

Definition 2.2 (Generalized fractional Bézier basis functions). For $t\in [0, 1]$, $a_i\in \mathbb{R}$ and $w\geq 0$, the following function is defined as the generalized fractional Bézier curve basis function of degree $n$ with $n$ shape parameters:

where ${f}_{i, n}(t) = \binom{n}{i}(1-D_t^{-w}(t))^{n-i}D_t^{-w}(t)^{i}$ and $D_t^{-w} (t) = \frac{1}{\Gamma(w+2)} t^{w+1}$.

For $n\geq2$, the generalized fractional Bézier basis functions can be defined recursively as follows:

Theorem 2.1 (Generalized fractional Bézier basis function). The generalized fractional basis functions with shape parameters have the following properties, such as degeneracy, non-negativity, a partition of unity, symmetry and linear independence.

Proof. The proof for degeneracy, non-negativity, a partition of unity, and symmetry can be seen in ^[22]. The linear independence property will be proven as follows:

Linear independence: $\sum_{i = 0}^{n}\bar{f}_{i, n}(t)c_i = 0 \iff c_i = 0, i = 0, 1, \dots, n; n\geq1.$ The sufficient condition is clear, and the principle of mathematical induction will be used for the necessary condition.

Base step: $n = 1$.

Substituting Eq (2.2) into the above equation yields:

By comparing coefficients, the following is obtained:

By simplifying the above equations, $c_0 = c_1 = 0$ is obtained. Hence, the base case is true. Now, the inductive step will be proven:

Inductive step: $n = k$ is true; show for $n = k+1$.

Assume that $\sum_{i = 0}^{k}\bar{f}_{i, k}(t)c_i = 0 \iff c_i = 0, i = 0, 1, \dots, k; k\geq1$ is true. Consider

Substituting Eq (2.3) in Eq (2.10) and rearranging it, the following is obtained:

Since $D_t^{-w}(t)$ is an arbitrary value on interval $t\in[0, 1]$,

Note that $\bar{f}_{i, k}(t) = 0$ for $i = -1$ or $i > k$. Hence, from hypothesis, we obtain $c_i = 0, i = 0, 1, \dots, k$ for Eq (2.12) and $c_i = 0, i = 1, 2, \dots, k+1$ for Eq (2.13). By the principle of mathematical induction, linear independence is satisfied. It is clear that the generalized fractional Bézier basis functions span any polynomial equation with at most degree $n(w+1)+1$. Therefore, the generalized fractional Bézier basis functions are a basis for polynomial space with at most degree $n(w+1)+1$.

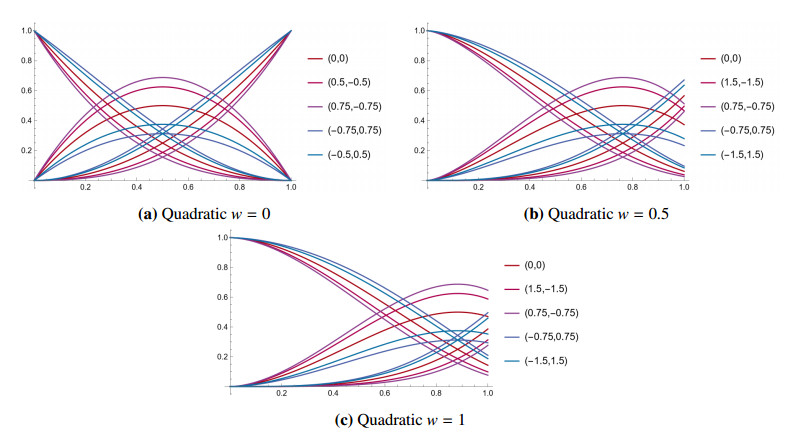
Figure 1 illustrates the quadratic fractional Bézier basis functions with multiple spectrum values of shape and fractional parameters. Figure 1 also indicates the same as the classical Bernstein basis functions, especially when the values of the shape and fractional parameters are zero.

3.   $F^2$ continuity conditions for generalized fractional Bézier curve

3.1.   Definition and properties of the generalized fractional Bézier curve

Definition 3.1 (Generalized fractional Bézier curve). The generalized fractional Bézier curve of $n$ degree with $n$ shape parameters is defined as follows:

where $P_{i}$ for $i = 0, 1, ..., n$ is the set of control points in $\mathbb{R}^{2}$.

Theorem 3.1 (Properties of generalized fractional Bézier curve). The generalized fractional Bézier curve has the following properties:

(1) Endpoint terminal. The generalized fractional Bézier curves are always interpolated their first control points $(t = 0)$ regardless the values of $w$. For the last control point, the curve will interpolated the last control point $(t = 1)$ when $w = 0$. However, for $w > 0$, the endpoint for $t = 1$ can still be calculated using the given equation below:

(2) Endpoint tangent. The endpoint tangent for the generalized fractional Bézier curve at $t = 0$ is independent of the $w$. However for $t = 1$, the endpoint tangent depends on $w$. The equations for endpoint tangent are given as follows:

(3) Shape adjustable property. The numbers of shape parameters of the generalized fractional Bézier curve depend on the curve's degree. These shape parameters allow local control of the curve's shape.

(4) Fractional curve adjustable property. One fractional parameter is associated with the generalized fractional Bézier curve. The fractional parameter allows the control of the curve's length adjustability along the $u$ direction. Please refer Section 3.2 for more details.

Proof. The rest of the properties and their proofs can be seen in ^[22].

3.2.   The curve adjustability property via the fractional parameters

The fractional parameter provides the curve adjustable property, as in Theorem 3.1. In Eqs (3.2) and (3.3), it is clear that for $t = 0$, the fractional parameter does not affect both equations. However, for $t = 1$, both equations depend on the fractional parameter. This implies that at $t = 1$ and $w > 0$, the curve does not interpolate at the last control point, $P_n$. However, the new endpoint (position/coordinate) can be calculated using the equation below:

Example 3.1. Figure 2 shows the curve adjustability of the fractional parameter, $w_1$ for the cubic fractional Bézier curve, $C_1(t; w_1)$. In this figure, the shape parameters used are $(a_1, a_2, a_3) = (-1, 0, 1)$, and the control points are represented by the red points. Each sub-figure has an arrow that is pointing to the endpoints of the curve with their respective value. The arrow is pointing towards endpoint at $t = 1$ with arbitrary value of $w_1$. Note that the interval of $t$ remains on the interval $[0, 1]$ regardless of the value of $w_1$.

3.3.   $F^2$ fractional continuity conditions

Let three consecutive generalized fractional Bézier curves be as follows:

where

$(1)$ $\bar{f}_{i, n}$, $\bar{f}_{j, m}$ and $\bar{f}_{k, l}$ are the generalized fractional Bézier basis functions of degree $n$, $m$ and $l$, respectively.

$(2)$ $P_{i}(i = 0, 1, ..., n)$, $Q_{j}(j = 0, 1, ..., m)$ and $R_{k}(k = 0, 1, ..., l)$ are the control points.

$(3)$ $a_{1}, a_{2}, \dots, a_{n}$, $b_{1}, b_{2}, \dots, b_{m}$ and $c_{1}, c_{2}, \dots, c_{l}$ are the shape parameters.

$(4)$ $w_{1}$, $w_{2}$ and $w_3$ are the fractional parameters.

Definition 3.2 (Fractional continuity for three consecutive generalized fractional Bézier curves, $F^r$). Suppose three curves $C_1(t; w_1)$, $C_2(t; w_2)$ and $C_3(t; w_3)$ are $F^r$ continuous and will connect to each other consecutively, i.e., $C_1$ connects to $C_2$, and $C_2$ connects to $C_3$. $F^r$ continuity between three consecutive curves can be achieved if the following conditions are satisfied:

The range of the scale factor, $\phi_i, \chi_i$ for $i = 0, 1, ..., r$ depends on the degree of continuity, $r$. Generally, for $F^r$ continuity, the range for the scale factors are $\phi_1, \chi_1 > 0$ and $\phi_i, \chi_i\in\mathbb{R}$ for $i = 2, 3, ..., r$.

Theorem 3.2 (Fractional continuity for generalized fractional Bézier curve, $F^r$). Consider three generalized fractional Bézier curves as in Eq (3.5), and the necessary and sufficient conditions for fractional continuity at the joint points are given as follows.

(1) $F^{0}$ continuity:

(2) $F^{1}$ continuity:

(3) $F^{2}$ continuity:

Proof. The theorem can be proven as follows:

By using the endpoint terminal and tangent properties in Theorem 3.1, Theorem 3.2 can be derived. From Eqs (3.2) and (3.3), the endpoint terminal and tangent for $t = 0$ are independent of $w$. This implies that for the right-hand side of the equation of Definition 3.2, the value of the fractional parameter can be set to zero to simplify the equations. Generally, if the curves $C_1, C_2\dots, C_n$ are connected consecutively, the fractional parameters $w_1, w_2, \dots, w_{n-1}$ (fractional parameters of the first curve, $C_1$ until $(n-1)$th curve, $C_{n-1}$) are needed to satisfy the continuity conditions, while the last fractional parameter, $w_n$, can be used to control the curve adjustability of the last curve, $C_n$.

$(1)$ $F^0$ continuity condition is obtained by solving $Q_0$ and $R_0$ in $C_1(1;w_1) = C_2(0;w_2 = 0)$ and $C_2(1;w_2) = C_3(0;w_3 = 0)$, respectively.

$(2)$ $F^0$ continuity must be achieved first. $F^1$ continuity condition is obtained by solving for $Q_1$ and $R_1$ in $C_1'(1;w_1) = \phi_1 C_2'(0;w_2 = 0)$ and $C_2'(1;w_2) = \chi_1 C_3'(0;w_3 = 0)$, respectively.

$(3)$ $F^1$ continuity must be satisfied first. $F^2$ continuity condition is achieved by solving for $Q_2$ and $R_2$ in $C_1''(1;w_1) = \phi_1^2 C_2''(0;w_2 = 0)+\phi_2 C_2'(0;w_2 = 0)$ and $C_2''(1;w_2) = \chi_1^2 C_3''(0;w_3 = 0)+\chi_2 C_3'(0;w_3 = 0)$, respectively.

Example 3.2. Figures 3 and 4 depict the adjustment of the common point via $w_1$ and the curve adjustability of second curve via $w_2$, respectively. Blue and orange curves with degree three are $C_1$ and $C_2$, respectively. The control points and shape parameters are the same as in Example 3.1. $Q_0$, $Q_1$ and $Q_2$ can be calculated by Theorem 3.2. Other control point, shape parameters and scale factors are $Q_3 = (3, -5)$, $(b_1, b_2, b_3) = (0.5, 0, 0.5)$, $\phi_1 = 0.75$ and $\phi_2 = -0.5$, respectively. The values of $Q_0$, $Q_1$ and $Q_2$ for each sub-figure in Figure 3 are

(1) Figure 3a:

(2) Figure 3b:

(3) Figure 3c:

(4) Figure 3d:

From Figure 4, it is obvious that $w_2$ did not affect the control points of $C_2$. This means that the common point or joined point between $C_1$ and $C_2$ is independent of $w_2$.

Example 3.3. Figure 5 shows three consecutive cubic generalized fractional Bézier curves with $F^2$ continuity. Blue, orange and green curves are $C_1$, $C_2$ and $C_3$, respectively. The control points and shape parameters are the same as in Example 3.2. $Q_0$, $Q_1$, $Q_2$, $R_0$, $R_1$ and $R_2$ can be obtained by Theorem 3.2. Other control point, shape parameters and scale factors are $R_3 = (0, -2)$, $(c_1, c_2, c_3) = (-1.5, 0, 1.5)$, $\chi_1 = 1.25$ and $\chi_2 = -0$, respectively. The values of $Q_0$, $Q_1$, $Q_2$, $R_0$, $R_1$ and $R_2$ for each sub-figure in Figure 5 are

(1) Figure 5a:

(2) Figure 5b:

(3) Figure 5c:

(4) Figure 5d:

(5) Figure 5e:

(6) Figure 5f:

Figure 6 illustrates the curve adjustability for the third curve via $w_3$. Based on Figure 6, clearly $w_3$ did not affect the control points of $C_3$. This means that the common point or joined point between $C_2$ and $C_3$ is independent of $w_3$.

In Figure 5b, when the value of the fractional parameter $w_1$ varies, it will cause the control points $Q_0, Q_1, Q_2, R_1,$ and $R_2$ to change their positions to maintain $F^2$ continuity between the curves $C_1$ and $C_2$ without affecting the convex hull of the curve, $C_1$, and the control points $Q_3$ and $R_0$.

In Figure 5c, when the fractional parameter $w_2$ differs, the coordinates of the control points $R_0, R_1,$ and $R_2$ are moved to maintain the $F^2$ continuity between the curves $C_2$ and $C_3$ without changing the convex hull of the curves $C_1$ and $C_2$ and the control point $R_3$.

Figure 5d–5f illustrates that the convex hulls of the curves $C_2$ and $C_3$ are shifted when the values of the fractional parameters $w_1$ and $w_2$ are altered. Figure 6 depicts that changing the value of the fractional parameter $w_3$ does not affect the convex hull of all curves, but the fractional parameter $w_3$ can be used to adjust the curve adjustability of the curve $C_3$.

4.   Generalized fractional Bézier tensor product surface

Definition 4.1 (Generalized fractional Bézier tensor product surface). Suppose $P_{i, j}$, where $i = 0, 1, \dots, m$ and $j = 0, 1, \dots, n$ are the control points. The generalized fractional Bézier tensor product surface is defined as follows:

where $a_i$ and $b_j$ are the shape parameters for $i = 1, \dots, m$ and $j = 1, \dots, n$, while $w_1$ and $w_2$ are the fractional parameters. Moreover, $\bar{f}_{i, m}(u; w_1, a_1, a_2, \dots, a_m)$ and $\bar{f}_{i, n}(v; w_2, b_1, b_2, \dots, b_n)$ are the generalized fractional Bézier basis functions. Also, note that $a_0 = a_{m+1} = b_0 = b_{n+1} = 0$. Hence, the generalized fractional Bézier tensor product surface has $m+n$ shape parameters with an additional two fractional parameters.

Remark 1. The tensor product generalized fractional Bézier surfaces (see Eq (4.1)) inherited almost all the properties of the classical tensor product Bézier surfaces, such as geometric and affine invariance, convex hull, endpoint interpolation (when the fractional parameters are zero), degeneracy and symmetry properties.

Remark 2. The generalized fractional Bézier tensor product surface has two additional properties: local shape flexibility and surface patch adjustability. Shape parameters give flexibility in changing the shape of the surface locally without changing the control points. The fractional parameters enable the adjustability of the surface patch. By varying the values of the fractional parameters, the fraction of the surface can be generated.

4.1.   Geometric effect of the shape parameters on the surface

A classical Bézier tensor product surface has a lack of flexibility in changing shape. This is because the surface can be changed only by altering its control points. Moving the control points can cause the whole surface to change. Some of the modeling surfaces preferred only the local part of the surface to change. Hence, the implementation of shape parameters enables the shape to change locally or globally by simply changing the spectrum value of the shape parameters.

The generalized fractional Bézier tensor product surface has excellent local dynamic shape parameters. This shape parameter can be used to change the shape of the surface. This section will demonstrate the geometric effect of the shape parameters on the surface.

Proposition 4.1. On the premise that the generalized fractional Bézier surface's control points and fractional parameters remain unchanged, the following holds:

(1) Based on Eq (4.1), the number of shape parameters is $m+n$. The corner points, $P_{0, 0}$, $P_{m, 0}$, $P_{0, n}$ and $P_{m, n}$ will be associated with two shape parameters. Control points $P_{k, 0}$, $P_{0, l}$, $P_{k, n}$, and $P_{m, l}$ where $k = 1, 2, \dots, m-1$ and $l = 1, 2, \dots, n-1$ corresponded to three shape parameters. The rest of the control points will have four shape parameters. For a clear visual, Figure 7 shows each control point in the control mesh corresponding to their respective shape parameters.

(2) Increasing the value of $a_i$ causes the surface to move closer to the control point $P_{i, j}$ but further from $P_{i-1, j}$. Conversely, if the value of $a_i$ decreases, the surface will move further from the control point $P_{i, j}$. For $b_j$, increasing the value will cause the surface to move closer to the control point $P_{i, j}$ but further from $P_{i, j-1}$. Meanwhile, decreasing the value of $b_i$ causes the surface to move further from the control point $P_{i, j}$.

(3) Hence, to generate the surface that is as close as possible to the control point $P_{i, j}$, the shape parameters need to be closer to the following values:

(a) $\sup(a_i), \sup(a_{i-1}), \dots, \sup(a_1)$.

(b) $\sup(b_j), \sup(b_{j-1}), \dots, \sup(b_1)$.

(c) $\inf(a_{i+1}), \inf(a_{i+2}), \dots, \inf(a_m)$.

(d) $\inf(b_{j+1}), \min(b_{j+2}), \dots, \inf(b_n)$.

Conversely, to generate the shape of a surface that is as far as possible from the control point of $P_{i, j}$, the shape parameters need to be closer to the following values:

(a) $\inf(a_i), \inf(a_{i-1}), \dots, \inf(a_1)$.

(b) $\inf(b_j), \inf(b_{j-1}), \dots, \inf(b_1)$.

(c) $\sup(a_{i+1}), \sup(a_{i+2}), \dots, \sup(a_m)$.

(d) $\sup(b_{j+1}), \sup(b_{j+2}), \dots, \sup(b_n)$.

Remark 3. The terms $\sup$ and $\inf$ used in Proposition 4.1 are based on Definition 2.2. Since the range of the shape parameters is open range, the value of shape parameters can only be close to the extreme values. Therefore, $\sup$ and $\inf$ are used to indicate that the shape parameter values can only approach the extreme values.

Example 4.1. Figure 8 indicates the biquartic fractional Bézier surface with zero shape parameters. This example shows how to control the shape of the surface to get as close as possible to the control point $P_{2, 2}$. Here, the shape parameters are $(a_1, a_2, a_3, a_4, b_1, b_2, b_3, b_4)$.

Example 4.2. The shape-changing process of the biquartic fractional Bézier surface to get as far as possible from point $P_{2, 2}$ is illustrated in Figure 8. The control points are the same as in Example 4.1. At the same time, the fractional parameters are zero.

From Figures 8 and 9, designers can manipulate these shape parameters to adjust the shape of the surface to their liking. To change the shape locally, the designer can manipulate one of the shape parameters. To change the shape globally, the designers need to change $a_i$ $(i = 1, \dots, m)$ or change $b_j$ $(j = 1, \dots, n)$.

4.2.   Geometric effect of fractional parameter on the surface

The generalized fractional Bézier surface has another kind of parameter called the fractional parameter. This fractional parameter gives a surface patch adjustability property to the surface. The surface patch adjustability property means the surface can adjust its length in $u$ and $v$ directions according to the respective fractional parameter.

Any degree of the surfaces will have two fractional parameters, $w_1$ and $w_2$. Here, $w_1$ is used to adjust the surface patch in $u$ direction while $w_2$ controls the length of surface generated in the $v$ direction. By observing the geometric behavior of each fractional parameter, it is possible to derive the fractional continuity either in $u$, $v$ or both directions (will be discussed further in Section 5).

Example 4.3. Figures 10 and 11 illustrate the surface patch adjustability using the fractional parameters. The control points and the value of the shape parameters for Figures 10 and 11 are the same as in Figures 8d and 9d, respectively.

Remark 4. There will be several figures of surfaces that have been created with reduced opacity color from this part till the end of the section. These surfaces with a lower opacity color depict the constructed surfaces when fractional parameters are set to zero. This type of modeling makes a comparison between surfaces with zero fractional value parameters and surfaces with non-zero fractional value parameters easier.

Figures 10 and 11 depict that $w_1$ is used to control the surface length in the $u$ direction while $w_2$ is for controlling the surface length in the $v$ direction.

5.   $F^2$ continuity conditions for generalized fractional Bézier surface

Previously, if the designers wanted to connect the second surface to the first surface's mid-line, the first surface must be spliced using the subdivision approach and then combined to the second surface. This method is time-consuming and expensive to compute, especially with high surface degrees. A new sort of continuity needs to be developed to address these restrictions.

Fractional continuity is an upgraded version of geometric continuity, where its definitions and theorems for curves up to degree two are discussed meticulously in ^[22]. By referring to Figures 10–12 in ^[23], the behavior of the fractional continuity can be seen clearly with the help of visualization of the curvature plot and comb. The same paper also shows that the degree of continuity is still maintained regardless of the position of the common point and the values of fractional parameters. Any part of the first curve can be connected to the second curve as the method of cut and combine is introduced in ^[24]. Using the cut and combine technique in ^[24], it is possible to construct outline of the real-life objects and animations with low curvature profile.

Although parametric and geometric continuities only allow two surfaces to be joined at the boundary ends, fractional continuity, on the other hand, allows two surfaces to be connected at any arbitrary line along the first surface in either $u$ or $v$ directions. The fractional parameter plays an essential role in making the fractional continuity feasible. This is due to the surface patch adjustability from the fractional parameter that makes the surfaces can be joined along any line of the first surface either in $u$ or $v$ direction. Hence, the fractional continuity is a good substitution for the subdivision method since the same result can be obtained by simply varying the fractional parameter.

Remark 5. Note that it is recommended for the readers to go through the definitions, theorems and analysis of the fractional continuity in ^[22,23,24].

Before deriving the $F^2$ continuity conditions, two adjacent generalized fractional Bézier surfaces must be defined first to facilitate the proving and derivation of the following theorems. Suppose there are two adjacent generalized fractional Bézier surfaces as follows:

The first and second surfaces will be denoted as $S_1(u, v; w_{1, 1}, w_{1, 2})$ and $S_2(u, v; w_{2, 1}, w_{2, 2})$, respectively, to make the notation simpler for derivation and proving since the original notation is too long.

Definition 5.1 (Fractional continuity for generalized fractional Bézier surface, $F^r$). Consider two generalized fractional Bézier surfaces $S_1(u, v; w_{1, 1}, w_{1, 2})$ and $S_2(u, v; w_{2, 1}, w_{2, 2})$ on $(u, v)\in[0, 1]\times[0, 1]$. The two surfaces are $F^r$ continuous in the $u$ direction if the following condition is satisfied:

For the $v$ direction, the following condition must be satisfied:

For $u$ and $v$ directions, the following condition must be satisfied:

Generally, for $F^r$ continuity, $\phi > 0$ and $\phi\in\mathbb{R}$.

5.1.   $F^2$ continuity in the $u$ direction

Theorem 5.1. If the two adjacent generalized fractional Bézier surfaces $S_1(u, v; w_{1, 1}, w_{1, 2})$ and $S_2(u, v; w_{2, 1}, w_{2, 2})$ satisfy all the following conditions:

then $F^2$ continuity will be achieved for the $u$ direction with $\phi > 0$.

Proof. To obtain $F^2$ continuity, $F^1$ continuity at the joints must be accomplished first where for every point on the same boundary, the two surfaces must have a shared tangent plane. Similarly, to obtain $F^1$ continuity, the $F^0$ continuity condition must be satisfy first by having the same common boundary of the two surfaces. Note that $w_{1, 1}$, $w_{2, 1}$ and $w_{2, 2}$ are set to zero to simplify the equation. This is due to the fractional surface adjustability property since $w_{1, 2}$ is the only fractional parameter responsible for changing the boundary point for $S_1(u, 1)$ in the $u$ direction. Hence, it can be written in the equation form as

Using the boundary properties of the surfaces in Theorem 2.1, the previous equation can be written as

Since the basis functions in (5.3) are linearly independent, it can be simplified by comparing the coefficients as follows:

By $F^1$ continuity definition, the two surfaces must have a common tangent at any point at their common boundary. Thus, the following equation needs to be satisfied:

where $\phi(u)$ is the scaling factor between the normal vectors at the joint and $\phi(u) > 0$. To simplify the calculation, the Faux method will be used, in which Eq (5.5) can be simplified as follows:

where $\phi$ is a positive real constant. Here, Eq (5.6) shows that the tangent vector of the cross-border at their common boundary should be continuous.

Using the boundary tangent property in the $v$ direction and substituting in the previous equation, it can be expressed as follows:

Since the fractional parameters $w_{1, 1}$, $w_{2, 1}$ and $w_{2, 2}$ are independent in Eq (5.7), it can be further simplified to

Combining the results from Eqs (5.4) and (5.8) can be simplified by taking $Q_{i, 1}$ as a subject, which yields

In pursuance of $F^2$ smooth continuity, the two surfaces need to hold the same normal curvature at any point on the common boundary; hence, they need to satisfy

where $\chi(u)$ and $\omega(u)$ are linear functions of $u$, while $\psi$ and $\phi$ are the arbitrary constants. In practical applications, $\chi(u) = \omega(u) = \psi = 0$ are set to simplify the previous equation, which can be written as follows:

The second-order derivatives of the generalized fractional Bézier basis functions $(i = 0, 1, \dots, n; n\leq2)$ at terminal point $u = 0$ are given by

Equation (5.12) shows that the fractional parameter, $w$, is independent for any derivative at terminal points $u = 0$. Thus, $w = 0$ is set to simplify the calculation. However, for terminal point $u = 1$, the second derivative becomes the linear combination of function $u$ ^[22].

Thus, from Eqs (5.12) and (5.13), we have

Substituting Eq (5.14) in Eq (5.11) yields

Finally, combining the $F^0$ and $F^1$ continuity conditions of Eqs (5.4) and (5.9) with Eq (5.15), $F^2$ continuity conditions can be obtained as follows:

To conclude, if the $S_1(u, v; w_{1, 1}, w_{1, 2})$ and $S_2(u, v; w_{2, 1}, w_{2, 2})$ satisfy Eqs (5.4), (5.9) and (5.15), the surface will be connected with $F^2$ continuity in the $u$ direction at the joint. Hence, Theorem 5.1 is proven. To achieve $F^1$ continuity in the $u$ direction at the joint, the two surfaces need to satisfy only Eqs (5.4) and (5.9).

5.2.   $F^2$ continuity in $u$ and $v$ directions

Theorem 5.2. If the two adjacent generalized fractional Bézier surfaces $S_1(u, v; w_{1, 1}, w_{1, 2})$ and $S_2(u, v; w_{2, 1}, w_{2, 2})$ satisfy all the following conditions:

then $F^2$ continuity will be achieved for $u$ and $v$ directions.

Proof. Suppose that $S_1(u, v; w_{1, 1}, w_{1, 2})$ in the $u$ direction wants to be connected at $F^2$ continuity with $S_2(u, v; w_{2, 1}, w_{2, 2})$ in the $v$ direction. The derivation is similar to Theorem 5.1, where the two surfaces must achieve $F^1$ continuity conditions in the $u$ and $v$ directions. Hence,

When $F^1$ continuity conditions have been fulfilled, the two surfaces need to get the same normal curvature at any point on their common boundary. This can be written in the equation as follows:

where $\phi$ is similar in Eq (5.18).

Using the same method in Theorem 5.1, i.e., using the second-order cross-border tangent vector in Eq (5.19), the following equation can be obtained:

Since $a_{1, k} = b_{2, l}$ for $k = 0, 1, \dots, m_1$ and $l = 0, 1, \dots, n_2$, it can be simplified further as follows:

To sum up, when the surfaces $S_1(u, v; w_{1, 1}, w_{1, 2})$ and $S_2(u, v; w_{2, 1}, w_{2, 2})$ satisfy the conditions in Eqs (5.19) and (5.21), then the two surfaces achieve the $F^2$ continuity in the direction of $u$ and $v$ at the joint. Thus, Theorem 5.2 is proven.

5.3.   $F^2$ continuity in the $v$ direction

Theorem 5.3. If the two adjacent generalized fractional Bézier surfaces $S_1(u, v; w_{1, 1}, w_{1, 2})$ and $S_2(u, v; w_{2, 1}, w_{2, 2})$ satisfy all the following conditions:

then $F^2$ continuity will be achieved for $v$ direction with $\phi > 0$.

Proof. The proof is similar to Theorem 5.1, implying that it will not be covered. Note that the fractional parameter $w_{1, 1}$ will be the only fractional parameter contributing to $F^2$ continuity in the $v$ direction. Hence, the other fractional parameters can be set to zero to simplify the calculations in the proof.

The $F^2$ continuity can be reverted to $G^2$ continuity by simply setting all the fractional parameters to zero.

6.   Procedures and examples of $F^2$ continuity between generalized fractional Bézier surfaces

6.1.   The procedure of $F^2$ continuity between generalized fractional Bézier surface

Fractional continuity for generalized fractional Bézier surfaces is a key in constructing numerous complex surfaces. In addition, shape flexibility and length adjustability are the main features of versatile surface designing. This section will show procedures for $F^2$ continuity of two generalized fractional Bézier surfaces. On account of Theorem 5.1, the procedures are as follows:

$(1)$ The following requirement must be fulfilled first: the order $m_1$, $n_1$ of $S_1(u, v; w_{1, 1}, w_{1, 2})$ and its control points, $P_{i, j}$ as well as shape parameters $a_{1, i}$ and $b_{1, j}$ $(i = 0, 1, \dots, m_1;j = 0, 1, \dots, n_1)$ and fractional parameters $w_{1, 1}$ and $w_{1, 2}$ are set to any arbitrary values within their respective ranges.

$(2)$ Suppose $w_{1, 1} = w_{2, 1}$, $m_1 = m_2$, $a_{1, k} = a_{2, l}$ $(k = 0, 1, \dots, m_1;l = 0, 1, \dots, m_2)$ and $Q_{i, 0}$ $(i = 0, 1, \dots, m_1)$; this enables $S_1(u, v; w_{1, 1}, w_{1, 2})$ and $S_2(u, v; w_{2, 1}, w_{2, 2})$ to achieve $F^0$ continuity.

$(3)$ Set the values of shape parameters $b_{2, j}$ $(j = 0, 1, \dots, n_2)$, the constant $\phi > 0$, the fractional parameter $w_{2, 2}$ and the order of $n_2$ for the $S_2(u, v; w_{2, 1}, w_{2, 2})$. Then, calculate for $Q_{i, 1}$ $(i = 0, 1, \dots, m_1)$ of $S_2(u, v; w_{2, 1}, w_{2, 2})$.

$(4)$ After calculating $Q_{i, 1}$, then proceed for $Q_{i, 2}$ $(i = 0, 1, \dots, m_1)$ calculation.

$(5)$ The remaining $n_2-2$ control points of $S_2(u, v; w_{2, 1}, w_{2, 2})$ are up to the designers' discretion. Hence, $F^2$ continuity between two generalized fractional Bézier surfaces in the $u$ direction is achieved.

The procedures above can be repeated to connect multiples of generalized fractional Bézier surface with $F^2$ continuity.

6.2.   Examples of $F^2$ continuity between generalized fractional Bézier surface

To demonstrate the $F^2$ continuity, some examples will be shown. In this section, $F^0$ and $F^1$ continuity will be shown first to compare different values of fractional parameters. Then, for $F^2$ continuity, some examples are given with different values of fractional parameters, shape parameters and scale factors.

Remark 6. The remaining control points for Examples 6.1 – 6.6 are up to the designer's discretion to choose, due to the fact that the remaining control points do not involve as a constraint in continuity conditions.

6.2.1.   Influence of fractional parameters on the $F^2$ continuity

The fractional parameter plays an essential role in fractional continuity. By varying the fractional parameter, the designer can control the boundary line between two surfaces while maintaining continuity.

Example 6.1. Figure 12 shows the $F^0$ continuity in the $u$ direction between two biquartic fractional Bézier surfaces with different values of fractional parameters. $w_{1, 2}$ is for controlling the connection line between the two surfaces in the $u$ direction. Meanwhile, $w_{2, 2}$ is for controlling the length of the second surface in the $u$ direction. Moreover, $w_{1, 1} = w_{2, 1}$ are for controlling the length of both surfaces in $v$ direction.

Example 6.2. Figures 13 and 14 depict the $F^1$ continuity and $F^2$ continuity in the $u$ direction between two biquartic fractional Bézier surfaces with different values of fractional parameters, respectively. The scale factor, $\phi = 1.25$, is used in this example, and the control points are the same as in Example 6.1.

6.2.2.   Influence of shape parameters on the $F^2$ continuity

The shape parameter gives an advantage to the aesthetic curve in terms of flexibility. This is due to the shape parameter enabling the shape of the surface to change without changing the control points. The shape parameter also allows the shape of the surface to change locally. To change shape locally, the designers need to change one of the shape parameters. To change shape globally, the designers need to change $a_i$ $(i = 0, 1, \dots, m)$ or change $b_j$ $(j = 0, 1, \dots, n)$.

Proposition 4.1 gives some insight into controlling the shape using shape parameters. The control points and convex hull will guide how to control the surface shape at a specific point.

Example 6.3. The $F^2$ continuity between two biquartic fractional Bézier surfaces in $u$ direction with the variation of shape parameters is depicted in Figure 15. Here, $w_{1, 1} = w_{2, 1} = w_{2, 2} = 0$, $w_{1, 2} = 0.75$ and $\phi = 1.25$ are chosen. The shape parameters used for each sub-figure are

$a.$ $(a_{1, 1}, a_{1, 2}, a_{1, 3}, a_{1, 4}, b_{1, 1}, b_{1, 2}, b_{1, 3}, b_{1, 4}, b_{1, 1}, b_{1, 2}, b_{1, 3}, b_{1, 4}) = (0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0)$.

$b.$

$c.$

$d.$

6.2.3.   Influence of scale factor on the $F^2$ continuity

Example 6.4. Figure 16 illustrates the $F^2$ continuity in the $u$ direction between two biquartic fractional Bézier surfaces with different scale factor values, $\phi$. The control points, shape and fractional parameters are similar to Figure 15a.

6.2.4.   Example of $F^2$ continuity for three consecutive surface patches.

The $F^2$ continuity conditions can also be applied to generate three consecutive surfaces either in $u$ or $v$ directions. The third generalized fractional Bézier tensor surface will be defined as follows:

Example 6.5. Figure 17 depicts the Möbius strip generated by three consecutive surfaces with $F^2$ continuity in $u$ direction with different fractional parameters. The green surface is the third surface defined by Eq (6.1). The $F^2$ continuity is between magenta and orange surfaces with orange and green surfaces. Green and magenta surfaces are connected by simple $C^0/G^0$ continuity; hence the value of $w_{3, 2} = 0$ is set and cannot be changed, or else they will be disconnected. $w_{1, 1} = w_{2, 1} = w_{3, 1} = w_{3, 2} = 0$ are set.

6.2.5.   Application of $F^2$ continuity in the modeling of the ruled surface.

In this section, the application of $F^2$ continuity will be shown in modeling of one of the engineering surfaces known as the ruled surface. Assume four generalized fractional Bézier space curves given as follows:

By using Eq (6.2), two ruled surfaces will be defined as follows:

Definition 6.1 (Two generalized fractional Bézier ruled surface). Suppose $x_{1, 1}(u; w_{1, 1}, a_{1, 1}, a_{1, 2}, ..., a_{1, {m_1}})$, $x_{1, 2}(u; w_{1, 2}, b_{1, 1}, b_{1, 2}, ..., b_{1, {n_1}})$, $x_{2, 1}(u; w_{2, 1}, a_{2, 1}, a_{2, 2}, ..., a_{2, {m_2}})$ and $x_{2, 2}(u; w_{2, 2}, b_{2, 1}, b_{2, 2}, ..., b_{2, {n_2}})$ with $m_1$, $n_1$, $m_2$ and $n_2$ degree are the generalized fractional Bézier space curves, respectively. Two ruled surfaces are defined as follows:

Example 6.6. Figure 18 shows the $F^2$ continuity between two bicubic fractional Bézier ruled surfaces with a variation of fractional parameters. The control points, shape parameters and scale factors are as follows:

The control points $Q_{i, j}$ for $i = 1, 2$ and $j = 0, 1, 2$ are obtained by $F^2$ continuity conditions as in ^[23]. The red, blue, green and pink curves are $x_{1, 1}(u)$, $x_{1, 2}(u)$, $x_{2, 1}(u)$ and $x_{2, 2}(u)$, respectively. In order to connect two ruled surfaces with $F^2$ continuity, $x_{1, 1}(u)$ and $x_{1, 2}(u)$ must be connected with $F^2$ continuity with $x_{2, 1}(u)$ and $x_{2, 2}(u)$, respectively.

7.   Conclusions

This paper discusses the $F^2$ smooth continuity conditions for generalized fractional Bézier surfaces. The benefit of this work is that fractional continuity overcomes the conventional parametric and geometric continuity in the aspect of connecting surfaces at any arbitrary boundary along the first surface. Hence, fractional continuity simplifies two different processes (subdivision method and continuity conditions) into one process (continuity conditions) by simply varying the fractional parameters. The generalized fractional Bézier basis functions have complex formulation compared to the classical Bernstein basis functions, which becomes our limitation of study. Future research recommends expanding the basis functions in constructing developable surfaces and optimizing the shape and fractional parameters in modeling smart surface manufacturing for the industry.

Acknowledgments

This research is supported by Universiti Sains Malaysia under Short Term Grant (Khas) (304/PMATHS/6315587) and the School of Mathematical Sciences, Universiti Sains Malaysia. In addition, the authors are very grateful to the anonymous referees for their valuable suggestions.

Conflict of interest

The authors declare that they have no competing interests in this paper.