Melnikov functions and limit cycle bifurcations for a class of piecewise Hamiltonian systems

1.   Introduction

In recent years, there has been a growing focus on the study of non-smooth systems, particularly piecewise near-Hamiltonian systems, see ^[4,18,19] and the references therein. Within the realm of bifurcation problems associated with piecewise smooth systems, a significant area of investigation involves determining the number of limit cycles or periodic solutions. This exploration can be considered an extension of the Hilbert's $16$th problem. Researchers commonly employ two principal methods to analyze limit cycle bifurcations: the Melnikov function method ^{[1,2,6,7,12,15,19,23]} and the averaging method ^{[3,4,9,13,14,16,17]}. It was proved in ^[5,14] that the above two methods are equivalent in studying the number of limit cycles of planar $C^\infty$ near-Hamiltonian systems or piecewise $C^\infty$ near-integrable systems in two or higher dimensional spaces.

In 2010, Liu and Han ^[12] considered a piecewise near-Hamiltonian system of the form

where $H_x^{\pm}, \; H_y^{\pm}, \; f^{\pm}, \; g^{\pm}\in C^{\infty}$, and $\epsilon\geq 0$ is a small real parameter, and established a formula of the first order Melnikov function which was widely used in studying the number of limit cycles bifurcated from periodic orbits, see ^[8,10,21] for example. Recently, more general results have appeared for piecewise smooth systems with multiple zones ^{[11,18,20,24]}. For instance, Tian and Han ^[18] studied the number of limit cycles bifurcated from a period annulus of a class of planar piecewise near-Hamiltonian systems with three different switching curves. The authors ^[11] investigated limit cycle bifurcations in piecewise near-Hamiltonian systems with multiple switching curves and obtained a formula of the first order Melnikov function. Yang, Yang and Yu ^[23] studied a planar piecewise Hamiltonian system with two zones separated by the two semi-straight lines and presented expressions of the first and second order Melnikov functions. In ^[22], Yang, Han and Huang considered a piecewise Hamiltonian system of the form

where

and $H^{\pm}(x, y)\in C^{\omega}$ with $H^{\pm}(0, 0) = 0$. They studied the number of limit cycles appearing in Hopf bifurcations of piecewise planar Hamiltonian system.

Motivated by the works mentioned above, in this paper, we consider a piecewise Hamiltonian system of the form

where

with $H_{i}^{\pm}(x, y)\in C^{\infty}, \; i = 0, 1, 2, \cdots$, $\epsilon\geq 0$ is a small real parameter, $\Sigma_{1}$ and $\Sigma_{2}$ are the regions with a common boundary consisting of two semi-straight lines. Let $\Sigma_{1}\cup\Sigma_{2}\subset\mathbb{R}^{2}$ with $l_{j}\subset\Sigma_{j}$, $\overline{l}_{1}\cup\overline{l}_{2} = \overline{\Sigma}_{1}\cap\overline{\Sigma}_{2}$, and $\Sigma_{1}\cap\Sigma_{2} = \varnothing$. Suppose that the two lines satisfy

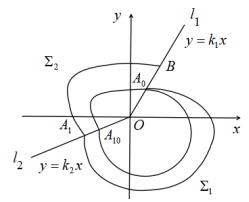
where $\mu_{1}, \; \mu_{2} = \pm1$ with $(k_{1}, \mu_{1})\neq(k_{2}, \mu_{2})$, see Figure 1. It is easy to see that the condition $(k_1, \mu_1)\neq(k_2, \mu_2)$ means $l_1\neq l_2$. When $k_1 = k_2$ and $\mu_1 = -\mu_2$, the two semi-straight lines degenerate into a regular single line. The authors in ^[23] pointed that when the separation line is nonregular, there will be more limit cycles in general.

The rest of this paper is organized as follows. In Section 2, we establish a bifurcation function of system (1.1) and present expressions of any order Melnikov functions. In Section 3, we give an application to illustrate our results and estimate the number of limit cycles bifurcated from a piecewise polynomial Hamiltonian system.

2.   Expressions of any order Melnikov functions

Consider system (1.1). We make the following basic assumptions for the unperturbed system $(1.1)|_{\epsilon = 0}$ as in ^[11]:

$(\mathit{\pmb{A1}})$ There exists an interval $J = (\alpha, \beta)$ and two points $A_{0}(h) = (a_{0}(h), k_{1}a_{0}(h))\in l_1$ and $A_{10}(h) = (a_{10}(h), k_{2}a_{10}(h))\in l_2$ such that for $h\in J$

$(\mathit{\pmb{A2}})$ There is a family of closed orbits denoted by $L_{h} = L_{h}^{1}\cup L_{h}^{2}, h\in J$ with clockwise orientation, where $L_{h}^{1}$ is defined by $H_{0}^{+}(x, y) = h$, $(x, y)\in\Sigma_1$, starting from $A_{0}(h)$ and ending at $A_{10}(h)$, and $L_{h}^{2}$ is defined by $H_{0}^{-}(x, y) = H^{-}(A_{0}(h))$, $(x, y)\in\Sigma_2$, starting from $A_{10}(h)$ and ending at $A_{0}(h)$.

$(\mathit{\pmb{A3}})$ The arcs $L_{h}^{1}$ and $L_{h}^{2}\;$ are not tangent to the switching lines $l_{1}$ and $l_{2}$ at points $A_{0}(h)$ and $A_{10}(h)$ for $h\in J$. In other words, for each $h\in J$,

Our main goal is to study the number of limit cycles bifurcated from the period annulus $\{L_{h}\}_{h\in J}$. First of all, we introduce a bifurcation function of system (1.1). Consider the orbit of system (1.1) starting from $A_{0}(h)\in l_{1}$. For sufficiently small $|\epsilon| > 0$, it has a first intersection point with the line $l_{2}$, denoted by

such that $\widehat{A_{0}A_{1}}\subset \overline{\Sigma}_{1}$.

The orbit of system (1.1) starting from $A_{1}(\epsilon, h)\in l_2$ has its first intersection point with the line $l_{1}$, denoted by

Then, $\widehat{A_{1}B}\subset \overline{\Sigma}_{2}$. See Figure 1 for illustration. From ^[11] we know that if assumptions $(\mathit{\pmb{A1}})$–$(\mathit{\pmb{A3}})$ hold, then the functions $A_0(h), A_{10}(h)$, $A_{1}(\epsilon, h)$, and $B(\epsilon, h)$ are all $C^\infty$ smooth with respect to $(h, \epsilon)$ on their domain.

Following ^[11,12], for any integer $k\geq 1$, we can write for $h\in J$ and $|\epsilon| > 0$ sufficiently small

Here, the functions $F(h, \epsilon)$ and $M_{j}(h)$ are called a bifurcation function and the $j$th order Melnikov function of system (1.1), respectively. The orbit from $A_{0}(h)$ to $B(\epsilon, h)$ defines a Poincaré map or return map of system (1.1). From ^[7], we have the following bifurcation theorem.

Lemma 1. ^[7] Let the assumptions $(\mathit{\pmb{A1}})$–$(\mathit{\pmb{A3}})$ be satisfied. Suppose that for all $1\leq j\leq k-1$, $M_{j}(h)\equiv0$ in (2.5) and that $M_{k}(h)$ has at most $l$ zeros in $h\in J$, multiplicity taken into account. Then, for small $|\epsilon| > 0$, system (1.1) has at most $l$ limit cycles bifurcating from the period annulus $\{L_{h}\}_{h\in J}$, multiplicity taken into account.

The aim of this paper is to develop formulas of the Melnikov functions up to higher order. From (2.5), it is obvious that

where $V^{+}_{0}(\epsilon, h) = H_{0}^{+}(B(\epsilon, h))$.

Before presenting our main results, we first give four preliminary lemmas which will be used in deducing expressions of $M_{j}(h)$ in (2.6). For convenience, we introduce the following notations and functions.

Define the following functions of $(\epsilon, h)$

Define the following functions of $h$

For integers $r$ and $l$ satisfying $1\leq l\leq r$, we define

where $\mathbb{N}$ represents the set of non-negative integers. Taking $r = 4$ as an example, we have

Lemma 2. Under the notations in (2.8), we have

Proof. Taking the partial derivative with respect to $\epsilon$ on both sides of $\mathbb{K}_{ij}^{\pm}(\epsilon, h)$ in (2.7) gives

where

By direct calculation, we have

Adding the above two equalities together, we have

Note that $C_{j}^{p+1}+C_{j}^{p} = C_{j+1}^{p+1}$ and

Substituting the above equality into (2.12) yields

Hence, by (2.11), we have

$\mathbb{W}_{ij}^{\pm}(\epsilon, h)$ in (2.10) can be obtained in a manner similar to the previous steps. This ends the proof. □

Lemma 3. Under the notations in (2.8), we have

Proof. We continue our proof of the formula of $f_{ir}^{\pm}(\epsilon, h)$ in (2.13) by induction on $r$. For $r = 1$, from the definition of $f_{ir}^{\pm}(\epsilon, h)$ in (2.7), we can obtain

Thus, the first formula in (2.13) is true for $r = 1$.

Suppose that it is true for $r = n$. That is,

We want to prove that the conclusion on $f_{ir}^{\pm}(\epsilon, h)$ in (2.13) is true for $r = n+1$. By the definition of $T_{nl}$ and $N(b_{1}, \cdots, b_{n})$ in (2.9), the above equality can be rewritten as

where

By the definition in (2.7), we have

By (2.14),

where

By applying Lemma 2 to above equalities, we have

which means that

Therefore, the first formula in (2.13) is true for $r = n+1$. The second formula on $g_{i, n+1}^{\pm}(\epsilon, h)$ in (2.13) can also be proved in a similar manner. This ends the proof. □

Lemma 4. Under the notations in (2.8), for the function $a(\epsilon, h)$ in (2.3), we have

where $\phi_{s}(h) = \left(\frac{\partial^{s} a}{\partial\epsilon^{s}}(0, h)\right)^{b_{s}}$.

Proof. From (1.2),

For $|\epsilon| > 0$ sufficiently small, $S_{i}^{\pm}(\epsilon, h)$ has the following Taylor expansion in $\epsilon$

By using Lemma 3 and letting $\epsilon = 0$ in (2.13), we get

where $1\leq l\leq r$, $T_{rl}$, and $N(b_{1}, \cdots, b_{r})$ are defined in (2.9).

Substituting (2.16) into the above equation yields

Again, from (1.2),

Then note that system (1.1) is Hamiltonian and

By inserting (2.19) and the expansion of $H^{+}(A_{1}(\epsilon, h), \epsilon)$ in (2.18) into the above equality, and comparing the like powers of $\epsilon$, we can obtain

For $j = 1$, (2.20) gives us

Substituting (2.17) into the above equation, we have $\frac{\partial a}{\partial\epsilon}(0, h) = \frac{v_{1}^{+}(h)}{K_{01}^{+}(h)}$.

In order to get $\frac{\partial^{j} a}{\partial\epsilon^{j}}(0, h)$ for $j\geq2$, substituting (2.17) into (2.20) and multiplying both sides of (2.20) by $j!$ lead to

The left side of (2.21) can be rewritten as

From (2.9), we know that if $l = 1$

and if $l\geq2$, then

By direct calculation from (2.23) and (2.24), if $l = 1$, then

and if $l\geq2$, then

Substituting (2.23)–(2.26) into (2.22) and (2.21), we have

which implies that

For example,

This ends the proof. □

Lemma 5. Under the notations in (2.8), for the function $b(\epsilon, h)$ in (2.4), we have

where

Proof. We obtain from (1.2) that

For $|\epsilon| > 0$ sufficiently small, $V_{i}^{\pm}(\epsilon, h)$ has the following Taylor expansion

By Lemma 3, we have

where $1\leq l\leq r$. $T_{rl}$ and $N(b_{1}, \cdots, b_{r})$ are defined in (2.9). Substituting (2.27) into the above equation, similar to (2.18), we have

Noting that system (1.1) is Hamiltonian and

substituting $H^{-}(A_{1}(\epsilon, h), \epsilon)$ in (2.18) and (2.29) into the equality above gives us

According to the second equation of (2.1) and comparing the like powers of $\epsilon$ on the left and right sides of (2.30), we have

Taking $j = 1$ in (2.31) gives us

Substituting (2.17) and (2.28) into the above equation yields

which gives us

For $j\geq2$, in order to solve $\frac{\partial^{j} b}{\partial\epsilon^{j}}(0, h)$ from (2.31), substituting (2.17) and (2.28) into (2.31) and multiplying both sides of (2.31) by $j!$ yields

Similar to (2.22), we have

Similar to (2.23) and (2.24), we can see that the above equation can be simplified to

Substituting (2.33) into (2.32) yields

which implies that

For example,

This ends the proof. □

Based on the previous discussion, we now present explicit formulas of any order Melnikov functions of piecewise Hamiltonian system (1.1).

Theorem 1. Consider system (1.1) with the assumptions $(\mathit{\pmb{A1}})$-$(\mathit{\pmb{A3}})$. The Melnikov functions $M_{j}(h)$ have the following formula for $j\geq2$

where

Proof. In order to get the explicit expressions of $M_{j} (h)$ in (2.6), we need to obtain the Taylor expansion of the function $H_{0}^{+}(B(\epsilon, h))$ with respect to $\epsilon$, where $B(\epsilon, h) = (b(\epsilon, h), k_{1}b(\epsilon, h))$. To that end, we should first find $\frac{\partial^{j} b}{\partial\epsilon^{j}}(0, h), j\geq1$, which are related to $\frac{\partial^{j} a}{\partial\epsilon^{j}}(0, h), j\geq1$.

To simplify the proof, we have presented formulas of $\frac{\partial^{j} a}{\partial\epsilon^{j}}(0, h), j\geq1$ in Lemma 4, and formulas of $\frac{\partial^{j} b}{\partial\epsilon^{j}}(0, h), j\geq1$ in Lemma 5. Next, we want to find $M_{j}(h)$. According to (2.28) and (2.6), if $j\geq2$, then

Thus, when $M_{1}(h) = M_{2}(h) = \cdots = M_{j-1}\equiv0$, we have

Substituting (2.35) into (2.34) yields

By substituting the expression of $\frac{\partial^{j} b}{\partial\epsilon^{j}}(0, h)$ in Lemma 5 into the above equation, we have

According to the derivation of (2.22), (2.23), and (2.24), the above equation can be written as

From Lemma 4, it can be calculated directly that

Substituting the above equality into (2.36) gives us

According to Theorem 2.2 in ^[25], we know

Hence, (2.37) becomes

where

For example, when $j = 2, 3$, we have

The proof is completed. □

For $j = 1$, the expression of $M_{1}(h)$ is given in ^[25], which is expressed by

where $Q(h) = \frac{\frac{\partial H_{0}^{+}}{\partial x}(A_{0}(h))+k_{1}\frac{\partial H_{0}^{+}}{\partial y}(A_{0}(h))}{\frac{\partial H_{0}^{-}}{\partial x}(A_{0}(h))+k_{1}\frac{\partial H_{0}^{-}}{\partial y}(A_{0}(h))}$.

To illustrate the impact of one straight line and two semi-lines on the number of limit cycles in a system, we consider the following piecewise polynomial Hamiltonian perturbation of the linear center

where $0 < \epsilon\ll1$,

Case 1: $l = l_{1}\cup l_{2} = \{(x, x)|x\in \mathbb{R}\}$. See Figure 2(a).

Case 2: $l_{1} = \{(x, x)|x > 0\}$ and $l_{2} = \{(x, -x)|x > 0\}$. See Figure 2(b).

By direct calculation, we have

for Case 1 and

for Case 2.

From (2.41) and (2.42), one can see that system (2.39) has no limit cycle in Case 1 and one limit cycle in Case 2 if $a_{01}^{+} < 0$ and $a_{11}^{+} > a_{11}^{-}$.

3.   The number of limit cycles of a piecewise polynomial system

In this section, as an application of the main results, we consider a piecewise Hamiltonian polynomial system of the form

where $0 < \epsilon\ll1$, ${H_{i}}_{x}^{\pm}(x, y)$ and ${H_{i}}_{y}^{\pm}(x, y)$ represent the partial derivatives of the function $H_{i}^{\pm}(x, y)$ with respect to $x$ and $y$, respectively,

and $\Sigma_{1}$ and $\Sigma_{2}$ are the regions bounded by the two semi-straight lines $l_{1}:y = x, \; x > 0$ and $l_{2}:y = -x, \; x > 0$. See Figure 3 for illustration.

Obviously, for (3.1) we have

Let

In this case, we have $A_{0}(h) = (-\frac{2h-1}{2}, -\frac{2h-1}{2})$ and $A_{10}(h) = (-\frac{2h-1}{2}, \frac{2h-1}{2})$. For any integer $m$, we further investigate the upper bound of the number of limit cycles by calculating $M_{1}(h), \; M_{2}(h), \; M_{3}(h)$, and $M_{4}(h)$ for piecewise polynomial Hamiltonian system (3.1).

Theorem 2. If the first order Melnikov function $M_{1}(h)$ of (3.1) is not zero identically, then for sufficiently small $|\epsilon| > 0$, it has at most $m+1$ limit cycles bifurcated from the period annulus defined by $\{L_{h}\}_{h\in J}$, multiplicity taken into account.

Proof. Below, we give an expression of $M_{1}(h)$ to estimate the maximum number of limit cycles of system (3.1). According to (2.38), the first order Melnikov function of system (3.1) is

According to (2.8), we have

According to (2.8) and (3.2), we have

Substituting (3.5) and (3.6) into (3.4) yields

From (3.7) it is easy to observe that $M_{1}(h)$ has at most $m+1$ zeros in $h$ on $(0, +\infty)$, multiplicity taken into account. Hence, by Lemma 1, system (3.1) has at most $n$ limit cycles bifurcated from the period annulus $\{L_{h}\}_{h\in J}$, multiplicity taken into account. □

Theorem 3. Suppose that $M_{1}(h)\equiv0$ and $M_{2}(h)\not\equiv0$. Then, for sufficiently small $|\epsilon| > 0$, system (3.1) has at most $2m$ limit cycles bifurcated from the period annulus defined by $\{L_{h}\}_{h\in J}$, multiplicity taken into account.

Proof. When $M_{1}(h)\equiv0$, we can obtain from (3.7) that

Below, we give an expression of $M_{2}(h)$ in the case of $M_{1}(h)\equiv0$ to estimate the maximum number of limit cycles of system (3.1). According to Theorem 1, the second order Melnikov function of system (3.1) is

where

In the following, we calculate the expressions of $K_{01}^{+}(h)$, $K_{02}^{\pm}(h)$, $K_{11}^{\pm}(h)$, and $v_{2}^{\pm}(h)$. According to (2.8), (3.2), and (3.3), we have

According to (2.8) and (3.2), we have

Similarly, according to (2.8) and (3.2), we have

Substituting (3.10)–(3.12) into (3.8) yields

where

Inserting (3.11) into $G_{3}(h)$ yields

Substituting (3.12) and (3.6) into $G_{4}(h)$ yields

where

and

Substituting (3.16) and (3.17) into (3.15) yields

Inserting (3.14) and (3.18) into (3.13) yields

where

Thus, according to Lemma 1 and (3.19), system (3.1) has at most $2m$ limit cycles bifurcated from the period annulus defined by $\{L_{h}\}_{h\in J}$ by the second order Melnikov function, multiplicity taken into account. □

Theorem 4. Suppose that $M_{1}(h) = M_{2}(h)\equiv0$ and $M_{3}(h)\not\equiv0$. Then, for sufficiently small $|\epsilon| > 0$, system (3.1) has at most $3m-1$ limit cycles bifurcated from the period annulus defined by $\{L_{h}\}_{h\in J}$, multiplicity taken into account.

Proof. When $M_{2}(h)\equiv0$, we can obtain from (3.19) that

Below, we give an expression of $M_{3}(h)$ in the case of $M_{1}(h) = M_{2}(h)\equiv0$ to estimate the maximum number of limit cycles of system (3.1). According to Theorem 1, the third order Melnikov function of system (3.1) is

where

In the following, we calculate the expressions of $K_{12}^{+}(h)$, $K_{21}^{\pm}(h)$, $v_{3}^{\pm}(h)$, and $M_{2}^{+}(h)$ based on (2.8) and (3.3).

According to (2.8) and (3.2), we have

We can directly obtain from (2.8) and (3.2) that

According to (2.8) and (3.2), we have

According to (3.3), we have

Substituting $K_{01}^{+}(h)$ and $K_{02}^{+}(h)$ in (3.10) into $M_{2}^{+}(h)$ in (3.9) yields

Substituting (3.10) and (3.24) into (3.20) yields

where

In the following, we will give an expression of $M_{3}(h)$ by evaluating $\widetilde{H}_{1}(h), \; \widetilde{H}_{2}(h), \; \widetilde{H}_{3}(h)$, and $\widetilde{H}_{4}(h)$ one by one.

Inserting (3.21) and (3.51) into the first equation in (3.27) yields

We can obtain from (3.28) that

Substituting (3.5) into the second equation in (3.27) gives

For $\widetilde{H}_{21}(h)$, substituting (3.22) into (3.30) yields

We can obtain from (3.31) that

For $\widetilde{H}_{22}(h)$, similar to $G_{42}(h)$, we have

We can obtain from (3.33) that

Substituting (3.31) and (3.33) into (3.30) gives

For $\widetilde{H}_{3}(h)$, inserting (3.10) into $\widetilde{H}_{3}(h)$ in (3.27) yields

In the following, we give $\widetilde{H}_{31}(h)$, $\widetilde{H}_{32}(h)$, and $\widetilde{H}_{33}(h)$ one by one. Substituting (3.23) into $\widetilde{H}_{31}(h)$ yields

We can obtain from (3.37) that

For $\widetilde{H}_{32}(h)$, similar to $G_{42}(h)$, we have

From the above equality, we can obtain

Inserting (3.23) into $\widetilde{H}_{33}(h)$ gives us

From (3.40), we can obtain

Substituting (3.37) and (3.40) into (3.36) yields

For $\widetilde{H}_{4}(h)$, inserting (3.10) into $H_{4}(h)$ in (3.27) yields

Plugging (3.12) into $\widetilde{H}_{41}(h)$ yields

From (3.44), we can obtain

For $\widetilde{H}_{42}(h)$, we have

From (3.46), we can obtain

Due to $\xi_{5}(m+1)\xi_{3}(m-1) = 0$, substituting (3.44) and (3.46) into (3.43) gives us

Substituting (3.28), (3.35), (3.42), and (3.48) into (3.26) yields

Thus, according to Lemma 1 and (3.49), system (3.1) has at most $3m-1$ limit cycles bifurcated from the period annulus defined by $\{L_{h}\}_{h\in J}$ by the third order Melnikov function, multiplicity taken into account. □

Theorem 5. Suppose that $M_{1}(h) = M_{2}(h) = M_{3}(h)\equiv0$ and $M_{4}(h)\not\equiv0$. Then, for sufficiently small $|\epsilon| > 0$, system (3.1) has at most $4m$ limit cycles bifurcated from the period annulus defined by $\{L_{h}\}_{h\in J}$, multiplicity taken into account.

Proof. Below, we give an expression of $M_{4}(h)$ in the case of $M_{1}(h) = M_{2}(h) = M_{3}(h)\equiv0$ to estimate the maximum number of limit cycles of system (3.1). According to Theorem 1, the fourth order Melnikov function of system (3.1) is

where

In the following, we calculate $v_{4}^{\pm}(h)$, $K_{13}^{\pm}(h)$, $K_{22}^{\pm}(h)$, $K_{31}^{\pm}(h)$, and $M_{3}^{+}(h)$ based on (2.8) and (3.3). According to (2.8) and (3.2), we have

According to (2.8) and (3.2), we have

According to (2.8) and (3.2), similar to the derivation of (3.23), we have

Similar to (3.12) and (3.22), from (2.8) and (3.2), we can directly obtain

For $M_{3}^{+}(h)$, according to (3.20),

Returning our attention to (3.50), substituting $K_{01}^{+}(h)$, $K_{02}^{\pm}(h)$, $K_{03}^{\pm}(h)$, and $K_{04}^{\pm}(h)$ into (3.50) yields

where

In the following, we will give an expression for $M_{4}(h)$ by evaluating $Y_{i}(h), \; (i = 1, \cdots, 8)$.

For $\widetilde{Y}_{1}(h)$, substituting (3.5) and (3.51) into $\widetilde{Y}_{1}(h)$ yields

From (3.57), we can obtain

For $\widetilde{Y}_{2}(h)$, substituting (3.54) and (3.6) into $\widetilde{Y}_{2}(h)$ yields

Inserting (3.54) into $\widetilde{Y}_{21}(h)$ yields

From (3.60), we can obtain

Similarly, we have

Plugging (3.60) and (3.62) into (3.59) yields

Substituting (3.53) and (3.6) into $\widetilde{Y}_{3}(h)$ yields

Inserting (3.53) into $\widetilde{Y}_{31}(h)$ yields

From (3.65), we can obtain

Similarly, we have

Substituting (3.65) and (3.67) into (3.64) yields

For $\widetilde{Y}_{4}(h)$, substituting (3.52) and (3.6)into $\widetilde{Y}_{4}(h)$ yields

Inserting (3.52) into $\widetilde{Y}_{41}(h)$ yields

From (3.70), we can obtain

Similarly, we have

Substituting (3.70) and (3.72) into (3.69) yields

Inserting (3.22) and (3.25) into $\widetilde{Y}_{5}(h)$ yields

From (3.32), (3.47), and (3.74), we can obtain

Plugging (3.23) and (3.25) into $\widetilde{Y}_{6}(h)$ yileds

For $\widetilde{Y}_{61}(h)$, we have

From (3.77), we can obtain

Similarly, we have

Substituting (3.77) and (3.79) into (3.76) yields

Inserting (3.25) into $\widetilde{Y}_{7}(h)$ yields

By direct calculation, we have

From (3.82), we can obtain

Hence, we have

For $Y_{8}(h)$, substituting (3.12) and (3.6) into $\widetilde{Y}_{8}(h)$ yields

Plugging (3.12) and (3.6) into $\widetilde{Y}_{81}(h)$ yields

From (3.86), we can obtain

Substituting (3.55) into $\widetilde{Y}_{82}(h)$ yields

By direct calculation, we have

Thus,

Similarly, we have

Thus,

Similarly, we have

Thus,

Similarly, we have

Thus,

Substituting (3.89)–(2.37) and (3.93) into (3.88) yields

Inserting (3.87) and (3.93) into (3.85) yields

Plugging (3.58), (3.63), (3.68), (3.73), (3.75), (3.80), (3.84), and (3.29) into (3.56) yields

Thus, according to Lemma 1 together with (3.95), system (3.1) has at most $4m$ limit cycles bifurcated from the period annulus defined by $\{L_{h}\}_{h\in J}$ by the fourth order Melnikov function, multiplicity taken into account. □

In this part, we consider the piecewise polynomial Hamiltonian system (3.1) with $m = 1, 2$. Before giving the main results, we first introduce the following two lemmas, which are calculated directly from Theorems 2-5.

Lemma 6. When $m = 1$, we have

where

where

and

Lemma 7. When $m = 2$, we have

where

where

$M_{3}(h)$ and $M_{4}(h)$ are expressed in the following three cases.

Case 1: When $a_{11}^{+} = 0, \; a_{30}^{+}+a_{12}^{+}\neq0$, we have

where

and

Case 2: When $a_{30}^{+}+a_{12}^{+} = 0, \; a_{11}^{+}\neq0$, we have

where

and

Case 3: When $a_{30}^{+}+a_{12}^{+} = 0$ and $a_{11}^{+} = 0$, we have

where

and

Case 1: When $a_{11}^{+} = 0, \; a_{30}^{+}+a_{12}^{+}\neq0$, we have

where

and

Case 2: When $a_{30}^{+}+a_{12}^{+} = 0, \; a_{11}^{+}\neq0$, we have

where

and

Case 3: When $a_{30}^{+}+a_{12}^{+} = 0$ and $a_{11}^{+} = 0$, we have

where

and

Theorem 6. Consider the piecewise polynomial Hamiltonian system (3.1) with m = 1. By using the up to the fourth order Melnikov function, the following statements hold.

(i) If the first order Melnikov function $M_{1}(h)$ is not zero identically, then for sufficiently small $|\epsilon| > 0$, system (3.1) has at most $2$ limit cycles bifurcated from the period annulus $\{L_{h}\}_{h\in J}$, multiplicity taken into account. Moreover, this maximum is achievable.

(ii) If $M_{1}(h)\equiv0$ and $M_{2}(h)\not\equiv0$, for sufficiently small $|\epsilon| > 0$, system (3.1) has at most $2$ limit cycles bifurcated from the period annulus $\{L_{h}\}_{h\in J}$, multiplicity taken into account. Moreover, this maximum is achievable.

(iii) If $M_{1}(h) = M_{2}(h)\equiv0$ and $M_{3}(h)\not\equiv0$, for sufficiently small $|\epsilon| > 0$, system (3.1) has at most $2$ limit cycles bifurcated from the period annulus $\{L_{h}\}_{h\in J}$, multiplicity taken into account. Moreover, this maximum is achievable.

(iv) If $M_{1}(h) = M_{2}(h) = M_{3}(h)\equiv0$ and $M_{4}(h)\not\equiv0$, for sufficiently small $|\epsilon| > 0$, system (3.1) has at most $4$ limit cycles bifurcated from the period annulus $\{L_{h}\}_{h\in J}$, multiplicity taken into account. Moreover, this maximum is achievable.

Proof. According to the expression for $M_{1}(h)$ in Lemma 6, we know that

where

Let $a_{01}^{-} = 0, \; a_{11}^{+} = 2, \; a_{11}^{-} = 2$. Denote

Then, through direct calculation, we have

Further more,

It follows that $A_{0}, A_{1}$ can be taken as free parameters. We can obtain that system $(3.1)$ has $2$ limit cycles for $m = 1$. The proof of (ⅰ) is completed.

According to the expression for $M_{2}(h)$ in Lemma 6, we know that

where

Let $a_{01}^{+} = 0, \; b_{01}^{+} = 1, \; b_{11}^{+} = 2$. Denote

Then, through direct calculation, we have

Further more,

It follows that $A_{0}, A_{1}$ can be taken as free parameters. We can obtain that system $(3.1)$ has $2$ limit cycles for $m = 1$. The proof of (ⅱ) is completed.

According to the expression for $M_{3}(h)$ in Lemma 6, let $a_{01}^{+} = 0, \; b_{01}^{+} = 0, \; c_{11}^{-} = 2, \; c_{11}^{+} = 1$. Denote

Then, through direct calculation, we have

Further more,

It follows that $A_{0}, A_{1}, A_{2}$ can be taken as free parameters. We can obtain that system $(3.1)$ has $2$ limit cycles for $m = 1$. The proof of (iii) is completed.

In the expression of $M_{4}(h)$ in Lemma 6, let $a_{01}^{+} = 0, \; b_{01}^{+} = 0, \; a_{02}^{+} = \frac{1}{2}, \; a_{20}^{+} = \frac{1}{2}, \; c_{01}^{+} = -2, \; a_{10}^{+} = 1, \; a_{11}^{-} = 0, \; a_{02}^{-} = 0, \; a_{20}^{-} = 0, \; a_{10}^{-} = -2, \; d_{11}^{-} = 2$. Denote

Then, through direct calculation, we have

Further more,

It follows that $A_{0}, A_{1}, A_{2}, A_{3}$ can be taken as free parameters. We can obtain that system $(3.1)$ has $4$ limit cycles for $m = 1$. The proof of (ⅳ) is completed. □

Theorem 7. Consider the piecewise polynomial Hamiltonian system (3.1) with m = 2. By using the up to the fourth order Melnikov function, the following statements hold.

(i) If the first order Melnikov function $M_{1}(h)$ is not zero identically, then for sufficiently small $|\epsilon| > 0$, system (3.1) has at most $3$ limit cycles bifurcated from the period annulus $\{L_{h}\}_{h\in J}$, multiplicity taken into account.

(ii) If $M_{1}(h)\equiv0$ and $M_{2}(h)\not\equiv0$, for sufficiently small $|\epsilon| > 0$, system (3.1) has at most $4$ limit cycles bifurcated from the period annulus $\{L_{h}\}_{h\in J}$, multiplicity taken into account.

(iii) If $M_{1}(h) = M_{2}(h)\equiv0$ and $M_{3}(h)\not\equiv0$, for sufficiently small $|\epsilon| > 0$, system (3.1) has at most $4$ limit cycles bifurcated from the period annulus $\{L_{h}\}_{h\in J}$, multiplicity taken into account.

(iv) If $M_{1}(h) = M_{2}(h) = M_{3}(h)\equiv0$ and $M_{4}(h)\not\equiv0$, for sufficiently small $|\epsilon| > 0$, system (3.1) has at most $6$ limit cycles bifurcated from the period annulus $\{L_{h}\}_{h\in J}$, multiplicity taken into account.

Proof. According to $M_{1}(h)$ in Lemma 7, let $a_{01}^{+} = 1, \; a_{11}^{-} = 2, \; a_{21}^{-} = 4, \; a_{03}^{-} = 4, \; a_{03}^{+} = 4, \; a_{21}^{+} = 4$. Denote

Then, through direct calculation, we have

Further more,

It follows that $A_{0}, A_{1}, A_{2}$ can be taken as free parameters. We can obtain that system $(3.1)$ has $3$ limit cycles for $m = 2$. The proof of (ⅰ) is completed.

According to $M_{2}(h)$ in the Lemma 7, let $a_{01}^{+} = 0,$ $a_{11}^{+} = 1,$ $a_{30}^{+} = \frac{1}{2},$ $a_{12}^{+} = \frac{1}{2},$ $b_{11}^{-} = 1,$ $a_{10}^{-} = 1,$ $a_{10}^{+} = 0,$ $a_{20}^{-} = 0,$ $a_{02}^{-} = 0,$ $b_{21}^{-} = 2,$ $b_{03}^{-} = 2,$ $b_{03}^{+} = 2,$ $a_{30}^{-} = 2,$ $a_{12}^{-} = 2,$ $a_{20}^{+} = \frac{1}{2},$ $a_{02}^{+} = \frac{1}{2}$. Denote

Then, through direct calculation, we have

Further more,

It follows that $A_{0}, A_{1}, A_{2}, A_{3}$ can be taken as free parameters. We can obtain that system $(3.1)$ has $4$ limit cycles for $m = 2$. The proof of (ⅱ) is completed.

According to the $M_{3}(h)$ in the Case 1, let $a_{01}^{+} = 0,$ $b_{01}^{+} = 0,$ $b_{11}^{+} = 2,$ $a_{10}^{-} = 1,$ $c_{11}^{-} = 2,$ $c_{21}^{-} = 2,$ $c_{03}^{-} = 2,$ $a_{10}^{+} = 1,$ $c_{03}^{+} = 14,$ $a_{11}^{-} = 2,$ $a_{30}^{+} = 2,$ $a_{12}^{+} = 2,$ $a_{20}^{-} = 1,$ $a_{02}^{-} = -1,$ $a_{02}^{+} = 0,$ $a_{20}^{+} = 0,$ $a_{21}^{-} = 2,$ $a_{03}^{-} = 2,$ $a_{30}^{-} = 2,$ $a_{12}^{-} = 2$. Denote

Then, through direct calculation, we have

Further more,

It follows that $A_{0}, A_{1}, A_{2}, A_{3}$ can be taken as free parameters. We can obtain that system $(3.1)$ has $4$ limit cycles for $m = 2$ in Case 1.

According to $M_{3}(h)$ in Case 2, Let $a_{01}^{+} = 0, \; b_{01}^{+} = 0, \; a_{10}^{-} = 0,$ $c_{11}^{-} = 2, \; a_{11}^{+} = 2, \; b_{01}^{-} = 1,$ $b_{10}^{-} = 1, \; a_{02}^{+} = 0, \; a_{20}^{+} = 0,$ $a_{21}^{-} = 0, \; a_{03}^{-} = 0, \; a_{30}^{-} = 0,$ $a_{12}^{-} = 0, \; b_{03}^{+} = \frac{1}{2}, \; b_{12}^{+} = 1, \; b_{21}^{+} = \frac{1}{2},$ $b_{30}^{+} = 1, \; a_{10}^{+} = 0, \; a_{11}^{-} = 0,$ $a_{20}^{-} = 0, \; a_{02}^{-} = 0, \; b_{21}^{-} = 1,$ $b_{30}^{-} = \frac{1}{2}, \; c_{03}^{+} = 2,$ $c_{21}^{-} = 2, \; c_{03}^{-} = 2, \; b_{10}^{+} = 0,$ $b_{02}^{-} = 0, \; b_{11}^{-} = 0, \; b_{20}^{-} = 0,$ $b_{02}^{+} = 0, \; b_{11}^{+} = \frac{3}{4}, \; b_{20}^{+} = 0$. Denote

Then, through direct calculation, we have

Further more,

It follows that $A_{0}, A_{1}, A_{2}, A_{3}$ can be taken as free parameters. We can obtain that system $(3.1)$ has $4$ limit cycles for $m = 2$ in Case 2.

According to $M_{3}(h)$ in Case 3, Let $a_{01}^{+} = 0, \; b_{01}^{+} = 0, \; a_{10}^{-} = 0, \; c_{11}^{-} = 2, \; b_{11}^{+} = 0, \; c_{21}^{-} = 2, \; c_{03}^{-} = 2, \; c_{21}^{+} = 2, \; c_{03}^{+} = 2$. Denote

Then, through direct calculation, we have

Further more,

It follows that $A_{0}, A_{1}, A_{2}$ can be taken as free parameters. We can obtain that system $(3.1)$ has $3$ limit cycles for $m = 2$ in Case 3. The proof of (ⅲ) is completed.

According to $M_{4}(h)$ in Case 1, let $a_{01}^{+} = 0, \; b_{01}^{+} = 0, \; a_{10}^{-} = 0,$ $d_{11}^{-} = 2, \; c_{01}^{+} = 0, \; d_{03}^{-} = 4,$ $d_{21}^{-} = 4, \; d_{21}^{+} = 4, \; a_{20}^{-} = 1,$ $a_{02}^{-} = 1, \; a_{11}^{-} = 2, \; b_{03}^{+} = 0,$ $b_{12}^{+} = 0, \; b_{21}^{+} = 0, \; b_{30}^{+} = 0,$ $a_{20}^{+} = 0, \; a_{02}^{+} = 0, \; a_{21}^{-} = 0,$ $a_{03}^{-} = 0, \; a_{30}^{-} = 0, \; a_{12}^{-} = 0,$ $a_{30}^{+} = 2, \; a_{12}^{+} = 2,$ $c_{21}^{+} = 4,$ $c_{03}^{+} = 4$. Denote

Then, through direct calculation, we have

Further more,

It follows that $A_{0}, A_{1}, A_{2}, A_{3}, A_{4}, A_{5}$ can be taken as free parameters. We can obtain that system $(3.1)$ has $6$ limit cycles for $m = 2$ in Case 1.

According to $M_{4}(h)$ in Case 2, Let $a_{01}^{+} = 0,$ $b_{01}^{+} = 0,$ $a_{10}^{-} = 0,$ $a_{11}^{+} = 1,$ $b_{11}^{+} = 0,$ $c_{01}^{-} = 0,$ $c_{10}^{-} = 0,$ $b_{01}^{-} = 1,$ $b_{10}^{-} = 1,$ $d_{11}^{-} = 2,$ $c_{01}^{+} = 1,$ $c_{10}^{+} = 1,$ $c_{02}^{-} = 1,$ $c_{11}^{-} = 1,$ $c_{20}^{-} = 1,$ $b_{10}^{+} = 1,$ $b_{02}^{-} = 1,$ $b_{11}^{-} = 1,$ $b_{20}^{-} = 1,$ $a_{10}^{+} = 1,$ $a_{11}^{-} = 2,$ $a_{20}^{-} = 1,$ $a_{02}^{-} = -1,$ $d_{03}^{-} = -4,$ $d_{21}^{-} = 4,$ $c_{03}^{-} = 1,$ $c_{12}^{-} = 1,$ $c_{21}^{-} = 1,$ $c_{30}^{-} = 1,$ $c_{02}^{+} = 1,$ $c_{11}^{+} = 0,$ $c_{20}^{+} = 1,$ $d_{21}^{+} = -3,$ $a_{30}^{-} = 2,$ $a_{12}^{-} = 2,$ $c_{21}^{+} = -1,$ $a_{20}^{+} = 0,$ $a_{02}^{+} = 0,$ $a_{21}^{-} = 0,$ $a_{03}^{-} = 0,$ $b_{03}^{+} = 0,$ $b_{12}^{+} = 0,$ $b_{21}^{+} = 0,$ $b_{30}^{+} = 0,$ $b_{02}^{+} = 0,$ $b_{20}^{+} = 0,$ $b_{03}^{-} = 0,$ $b_{12}^{-} = 0,$ $b_{21}^{-} = 0,$ $b_{30}^{-} = 0,$ $c_{12}^{+} = 0,$ $c_{30}^{+} = 0$. Denote

Then, through direct calculation, we have

Further more,

It follows that $A_{0}, A_{1}, A_{2}, A_{3}, A_{4}$ can be taken as free parameters. We can obtain that system $(3.1)$ has $5$ limit cycles for $m = 2$ in Case 2.

According to $M_{4}(h)$ in Case 3, let $a_{01}^{+} = 0,$ $b_{01}^{+} = 0,$ $a_{10}^{-} = 0,$ $b_{11}^{+} = 0,$ $d_{11}^{-} = 2,$ $c_{01}^{+} = 0,$ $a_{02}^{-} = 0,$ $a_{10}^{+} = -1,$ $d_{03}^{-} = 0,$ $d_{21}^{-} = 0,$ $d_{21}^{+} = 4,$ $a_{11}^{-} = 0,$ $a_{20}^{-} = 0,$ $c_{21}^{+} = 4,$ $c_{03}^{+} = 4,$ $c_{11}^{+} = 0$. Denote

Then, through direct calculation, we have

Further more,

It follows that $A_{0}, A_{1}, A_{2}, A_{3}$ can be taken as free parameters. We can obtain that system $(3.1)$ has $4$ limit cycles for $m = 2$ in Case 3. The proof of (ⅳ) is completed. □

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

The corresponding author is supported by the National Natural Science Foundation of China (11931016).

Conflict of interest

The authors declare that they have no conflict of interest.