1.
Introduction
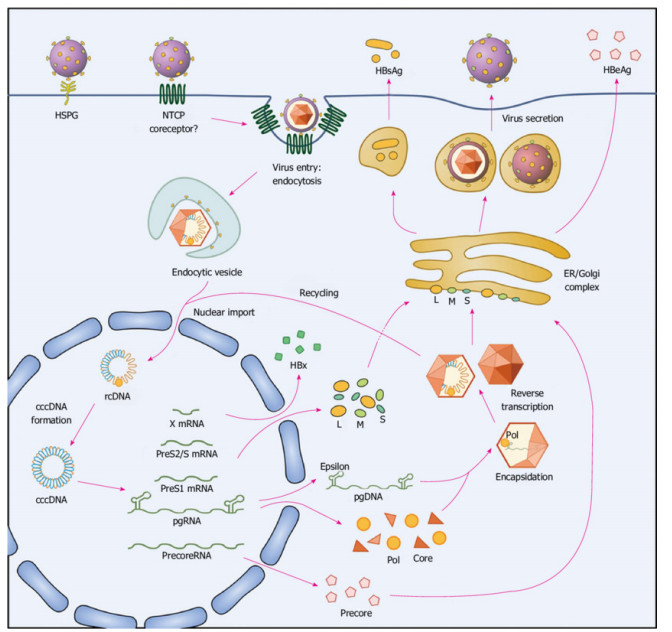
Hepatitis B virus causes hepatitis B (HB), an extremely fatal liver disease, and is responsible for more than 2 billion chronic infections that have been discovered globally [1,2]. It is a significant issue for wellbeing promotion. It can lead to prolonged liver damage, persistent inflammation, and a significant chance of fatality through hepatocellular carcinoma and encephalopathy [3]. Hepatitis B infestations can only happen if the pathogen can get into the circulatory system and affect the liver (see Figure 1). Once inside the liver, the infection multiplies and emits a significant quantity of fresh pathogens into the interstitial fluid [4,5].
However, there are two potential stages of this infectious disease: acute and chronic. HB virus that is severe seems to last less than 6 months, typically. If the illness is severe, the defensive mechanism is expected to eradicate the organism of the pathogen, and the patient should fully recover in the next few weeks. The majority of individuals who develop HB have an active virus. The incubation period for persistent HB is 12 weeks or more. Most newborns who contract HBV during infancy, as well as several children between the ages of one and six, develop a degenerative disease. Individuals having persistent HBV disease constitute a sizable 2/3 of chronically transmitted individuals. Despite carrying and spreading germs, these individuals do not exhibit any indications of illness [6]. The surviving one-third experienced acute hepatitis, a liver condition that could potentially be incredibly dangerous (see Figure 2). Over 2.4 billion individuals worldwide suffer from recurrent liver problems. HB's severe or long-term effects cause over 600,000 deaths a year [6]. Cytotoxic T lymphocytes (CTLs) can deliberately threaten contaminated hepatocellular through severe HBV infections and contribute to the pathophysiology of liver failure by coordinating various immunological mechanisms; see [6].
It is crucial to take into account the impact of temporal constraints on the HBV pathway because intercellular propagation and disease penetration are processes that require to be accomplished within a certain environment [7,8]. Additionally, the criteria for development and contact are based on the disease's kind and phase, the defensive system's health, and the milieu of the organism wherein the association works [9]. The participant's general lack of well-being affects the environment of the body. One strategy for investigating the effects of internal environmental parameters on the evolution of HBV transmission could be to modify the determinism account of the bacteriophage association to incorporate the randomized pressure in both an incremental and random manner. Numerous studies, including [10,11,12], have investigated numerical simulations to look into the mechanisms of HBV spreading with sound intensity.
When trying to evaluate the analogous prediction systems, stochastic modelling of highly contagious infection agents has a vital influence and adds a sense of authenticity [13]. In general, various organisms respond in the equivalent habitat to virus-induced designs and highly infectious pathogens, and the consequences can vary. Because the environment is constantly changing, the system's attributes actually oscillate around certain optimum levels [14]. Wang et al. [15] created an insidiously contagious and randomized stochastic HIV infectious framework and studied the results of stationary transmission durability. The researchers [16] addressed how a randomized HBV candida transmission concept with a significant delay in the propagation factor causes regular eruptions and the stagnant distribution and elimination consequences. In addition, the pathogens will be wiped out if the stochastic procreative quantity is below one, and if the stochastic procreative index is significantly higher than one, the infectious disease will be stochastically persistent with a perturbation theory model generated. According to Sun et al. [17], the presence of a solution for the stochastic highly contagious pathogenic framework of CTL feedback and decentralized delay was investigated. Rihan and Alsakaji [18] presented an investigation of an HBV infection framework, including a delayed immune reaction. For further details on epidemic systems; see [19,20,21] and the references cited therein.
Due to their involvement in assisting representatives in exploring the concealed characteristics of the complexities of interactive structures in rheological, permeation, machine design, electromagnetic fields, control, remote sensing, and thermodynamics, implementations of deterministic fractional differential equations (DEs) and fractional stochastic DEs motivated by Brownain motion (BM) have historically attracted the majority of attention in potential implementation. The basic differential and integral operators are unable to capture imperfections, but both fractional derivative/integral operators have been recognized as powerful computing tools [22,23,24]. Furthermore, when simulating physical and experimental events, F-F formulations exhibit diverse and distinct types of variability [25,26,27]. I think it seems that there are undoubtedly many real-world problems that neither fractal nor fractional interpretations are able to accurately reproduce on an individualized dimension. Researchers realized that novel mathematical procedures were required to replicate extremely complicated formations. Despite the assertion that there is hardly anything novel or transformative, it is difficult to believe that the combination of two existing concepts may produce a groundbreaking procedure. For added complexity, a unique differential formula was first implemented in [28]. This logical statement might be understood as the result of the accumulation of the fractal differentiation of a fractional derivative of a specific model. Evidently, there are three possible readings; it all depends on the kernels [29,30,31,32]. The concept was contested and applied to other challenges such as chaotic outgrowths, outbreaks, and diffusion, among others ([33,34,35,36]), and the overwhelming majority of publications provided extremely impressive predicted findings.
In fact, random events are prevalent worldwide. Systems frequently experience random disturbances. Various studies have been conducted on stochastic dynamics; for example, a wide range of scientific theories, including meteorology, accounting, biology, and telecommunication systems, frequently exhibit randomized fluctuations with long-term dependency. In order to analyze fractional stochastic processes, fractional BM employing the Hurst index H(1/2,1) has been proposed as an alternative to classical BM [37,38]. Kerboua et al. [39] investigated the SFDEs with perturbed regulatory frameworks involving fractional BM. Pei and Xu [40] investigated the non-Lipschitz SDEs driven by fractional BM. In 2021, authors [41] presented a novel notion for analyzing and predicting the transmission of COVID-19 throughout Africa and Europe using stochastic and deterministic methods. Alkahtani and Koca [42] contemplated the fractional stochastic SIR system within the fractional calculus technique. Rashid et al. [43] expounded the stochastic F-F tuberculosis model via a nonsingular kernel with random densities.
In the research analysis, we examine the behaviour of fractional stochastic delay DEs of the HBV system involving cell-to-cell propagation and CTLs immunological responsiveness via the fractal-fractional operator based on the exponential decay kernel with random densities, which is inspired by the aforementioned clinical and mathematical concerns. This biological model governed by fractional BM has not yet been demonstrated in the mainstream. As a result of this reality and in an effort to fill this discrepancy, we commence scientific work on one of these formulae in this article. We include the impact of several time delays (TD) and randomness inside a recipient to furnish a highly authentic scenario for the virus's design phase. In the meantime, existence-uniqueness, stochastic basic reproductive number, and the local stability of disease steady states are investigated in the stochastic context. Numerical results are presented by employing the revolutionary technique proposed by Atangana and Araz [41] in the F-F derivative sense. Graphical illustrations are presented with low random densities, incorporating the fractal-dimension and fractional-order. In a nutshell, we presented the simulation findings with and without control.
2.
Preliminaries
Before advancing on to the formal description, it is imperative to study certain fundamental F-F operator concepts. Take into account the parameters provided in [28] as well as the functional w(t1), which is continuous and fractal differentiable on [c,d] with fractal-dimension ϖ and fractional-order δ.
Definition 2.1. ([28]) The F-F operator of w(t1) involving the index kernel in terms of Riemann–Liouville (RL) can be presented as follows for δ∈[0,1]:
where dw(u)duϖ=limt1↦uw(t1)−w(u)tϖ1−uϖ and s−1<δ,ϖ≤s∈N.
Definition 2.2. ([28]) The F-F operator of w(t1) involving the exponential function kernel in terms of RL can be described as follows for δ∈[0,1]:
such that M(0)=M(1)=1 having δ>0,ϖ≤s∈N.
Definition 2.3. ([28]) The corresponding F-F integral operator of (2.1) is stated as:
Definition 2.4. ([28]) The corresponding F-F integral operator of (2.2) is stated as:
3.
Model configuration
In this study, we reveal a revolutionary system of stochastic delay DEs for Hepatitis B virus replication in a single recipient, despite the fact that the intracellular stage of over expression is not entirely appreciated. We surmise that throughout an HBV infestation, balanced (unexposed) metabolic enzymes can become infectious both by interaction with contaminated hepatocytes and by freshly generated complimentary pathogens. We furthermore suppose that the challenge-contaminated lymphocytes can be especially attacked by the cytotoxic T lymphocytes (CTLs). With the infiltration of a membrane and the ejection of retroviruses and the creation of viruses, there is still an unavoidably intracellular TD (significant-delay). Additionally, TD is necessary to reflect the gestation, which is the duration needed for the creation of fresh pathogens. Furthermore, we offer a delay differential framework to integrate the CTL community alongside infections predicated on the underlying framework of Nowak et al. [44]. A model is used to evaluate the structure
where ˜H(t1),˜I(t1),˜V(t1),˜D(t1), respectively, indicate the hepatocytes that are pure and productive of pathogens, infectious hepatocellular, HB infection pathogens as well as the CTLs. From the first component of the following formula, TD containing ζ1 is applied to estimate the length of period it takes from the first infections of a tissue and the generation of additional vesicles. The other component also incorporates the response time vital for proper hepatocytes to be invaded by malignant hepatocytes interactions before becoming contaminated hepatocellular, whilst ζ2 denotes the time that is required for recently generated particulates to develop before becoming contagious components. Either uncontrolled pathogens attack normal tissue at a pace of γ1˜H˜V (disease transmission mechanism), or viral proteins communicate directly normal tissues at a speed of γ2˜H˜I (cell-to-cell spread mechanism). As a result, the expression "γ1˜H˜V+γ2˜H˜I" denotes the overall disease incidence of susceptible organisms. CTLs generate at a consistent rate of η2 from the hypothalamus and at a pace of γ4˜I˜D as a consequence of stimulating invading pathogens, and they remove invading pathogens at a rate of γ3˜I˜D, where ω is the rate at which viral proteins producing free radicals infections (see Figure 3).
In addition, the complexities of HBV transmission may be impacted by unpredictable perturbations in the mechanism of transmission within the recipient, including fluctuations in climate, emotion, and other endogenous rhythms. Because of this, many researchers have included randomization to determinism in studies of biologic processes to illustrate the influence of environmental heterogeneity, as seen in [44,45].
By incorporating nonlinear disturbance on the spontaneous mortality rate using white noise into every other component of the scheme, we capture the influence of randomness in the host for a more reasonable position of the virus's progression (3.2). Although the HBV infection model's characteristics are not known in advance, the region to which they correspond can indeed be easily identified. So, we suggest a delayed probabilistic approach of the following:
depending on the ICs ˜H(δ)=μ1(δ),˜I(δ)=μ2(δ),˜V(δ)=μ3(δ),˜D(δ)=μ4(δ).
Also, δ∈[−ζ,0],ζ=max{ζ1,ζ2},μκ(δ)∈C,κ=1,...,4. C([−ζ,0],R4+) is the collection of Lebesgue integrable functions in this case including Bκ,κ=1,...,4 is a real-valued standard BM specified on a complete probability space (ω,A,P) meeting the basic requirements [46] and σκ,κ=1,...,4 denote the concentrations of the white noise.
4.
Qualitative analysis
In previous decades, the idea of reproduction has been extensively used in epidemiological modelling since it has been recognized as a valuable mathematical tool for evaluating reproduction in a specific illness. According to the concept proposed by Atangana [48], one will identify two components F and ˜V, then
will be analyzed to generate reproductive number [49]. The component F is particularly intriguing because it is derived from the nonlinear part of the infected classes.
and
At disease free equilibrium E0=(λϕ1,0,0,ϑϕ4), we have
Therefore, we have
Then,
gives
Also, A indicates that the expansion will not repeat and will consequently have a single magnitude and wipe out. A>0 indicates that there is sufficient intensity to initiate the regeneration phase, implying that the dispersion will have more than one cycle. Consequently, researchers will supply a strong insight of the aforesaid number.
4.1. Existence-uniqueness of the HBV model
In this part, we outline a few prerequisites that will ensure a non-negative solution of the stochastic delay DEs scheme presented in (3.2). This is feasible because a favourable result will exist if the system's coefficients satisfy the growth and Lipschitz assumptions.
Theorem 4.1. Suppose there is a system (3.2) (˜H(t1),˜I(t1),˜V(t1),˜D(t1)) with ICs having t1≥−ζ and the solution will stay in R4+, almost probably.
Proof. By means of the system (3.2), satisfies the Lipschitz continuous, there exists a peculiar solution (˜H(t1),˜I(t1),˜V(t1),˜D(t1)) defined on [−ζ,ζe), where ζe signifies the explosion time. In order to demonstrate the solution, we need to to illustrate that ζe=∞. Assume that Λ0>0 be a large enough, thus, we have (˜H(t1),˜I(t1),˜V(t1),˜D(t1))={(μ1(t1),μ2(t1),μ3(t1),μ4(t1)):t1∈(−ζ,0)}∈C([−ζ,0];R4+) contained in [1Λ0,Λ0]. Introducing the stopping time, so for every Λ≥Λ0, we have
or equivalently
Now letting infψ=∞ and increasing function ζΛ on Λ. Also, suppose ζ∞=limΛ↦∞ζΛ, then ζ∞≤ζe and need to prove ζ∞=∞ almost surely. We intend to find that ζe=∞ almost surely. If this claim is factually inaccurate, then ∃ some constants T>0 and ϵ∈(0,1) such that P{ζ∞≤T}>ϵ. Thus, for an integer Λ1≥Λ0 such that P{ζΛ≤T}>ϵ,∀Λ≥Λ1. Introducing a mapping U1:R4+↦R+ as:
where Λ2 refer to be a non-negative constant to be determined. In view of the Itô's formula, we have
Simple computations yield
where HU1=(λ−ϕ1˜H−λ˜H+ϕ1+γ1˜V+γ2˜I+12σ21)+(ϕ2+γ3˜D−γ1˜H(t1−ζ1)˜V(t1−ζ1)˜I−γ2˜H(t1−ζ1)˜I(t1−ζ1)˜I+12σ22)+Λ2(ω˜I−ϕ3˜V)+Λ2(ϑ−ϕ4˜D+γ4˜I˜D−ϑ˜D+ϕ4−γ4˜I+12σ24)=C.
Thus, we have
Performing integration from 0toζΛ∧Ψ, it can be deduced that
using the fact that ζΛ∧T=min{ζn,t1}. Implementing the expectation on the aforesaid variants gives
This implies that
As U1(χ(ζΛ∧T))>0, then
Further, for ζΛ, since certain factors of χ(ζΛ),say(˜H(ζΛ)) including 0<˜H(ζΛ)≤1Λ<1.
Thus, U1(χ(ζΛ))≥−ln(1Λ), this allow us to write U1(χ(ζΛ))=ln(˜H(ζΛ))≤ln(1Λ).
As a result, from (4.2) and the previous expression, we have
Combining (4.1)-(4.3), we have
It follows that
Applying limit sup Λ↦∞ on (4.4), ∀T>0, we find
This is the desired result.
The average number of subsequent viral infections that a contagious individual causes while they are still dangerous is the primary reproductive component in this scenario. We also want to show that stochastic reproduction (Rs0) is a special kind of basic reproduction number.
4.2. Basic reproduction number (Rs0)
Initially, considering the system's (3.2) second cohort, that is
Considering the Itô's technique for twice differentiation mapping f1(˜I)=ln(I), the Taylor series representation is
This implies that
It follows that
where A1=γ1˜H(t1−ζ1)˜V(t1−ζ1)+γ2˜H(t1−ζ1)˜I(t1−ζ1)−γ3˜I(t1)˜D(t1)−ϕ3˜I(t1) and A2=σ2˜I, then (4.6) can be written as
As dt1↦0,(dt1)2,dt1dB2(t1)↦0 and (dB2(t1))2 can be converted to dt1 (By the variance of Wiener technique), we have
Taking into consideration the next generation matrices [47] are as follows
Therefore, F and ˜V at disease-free equilibrium E0=(λϕ1,0,0,ϑϕ4), we find
Thus, the basic reproduction number is Rs0=ρ(F˜V−1) is
which is the required stochastic fundamental reproduction number.
4.3. Local stability of diseases-free equilibrium point (DFEP) in stochastic sense
Theorem 4.2. For a community's infection to be eradicated, then Rs0<1.
If Rs0<1, then for any provided ICs (˜H(0),˜V(0),˜I(0),˜D(0))=(˜H0,˜V0,˜I0,R0)∈R4+. Therefore, ˜I(t1) admits limt1↦∞supln(˜I(t1))t1≤ϕ3(Rs0−1) almost surely.
Proof. Taking into consideration (4.5), we have
It follows that
After performing integration, we have
where Υ(t1)=σ3t1∫0dB3(t1) is the martingale. therefore, by the strong principal of large values for Υ(t1), see [50], we get limt1↦∞supΥ(t1)t1=0 almost probably.
After dividing by t1 and applying limit t1↦∞, then (4.8) reduces to
This indicates that Rs0<1.
Finally, Rs0 should be smaller than 1 for virus elimination in a population.
Remark 4.1. Under some settings, the solution of framework (3.2) oscillates all around endemic equilibrium of the undisturbed system (3.1) if Rs0>1. This indicates that as long as the concentrations of white noise are low enough, the sickness will endure.
5.
Numerical illustration of the fractal-fractional stochastic HBV model
In what follows, the framework is further expanded to F-F derivative operators.
Firstly, we present the Caputo-Fabrizio F-F derivative for the classical derivative formulation. As a result, the stochastic F-F framework will be quantitatively determined employing the numerical technique described previously. This type of system is presented by
For tm+1=(m+1)Δt1, then we the aforementioned system can be integrated as follows
Utilizing the fact of polynomials in the aforesaid system, we find
Furthermore, we can write
where
5.1. Results and discussion
To illustrate the aforementioned mathematical findings, we will provide a few simulation studies in this part. We find the system's stochastic F-F derivative in the Caputo-Fabrizio context using a revolutionary numerical approach introduced in [41]. An evolutionary algorithm was developed to numerically predict outcomes since state formulas possess ICs. Table 2 is an overview of the primary attribute settings. The outcomes with no controls, just vaccine restrictions, just therapy regulation, and both vaccination and medication regulations are compared.
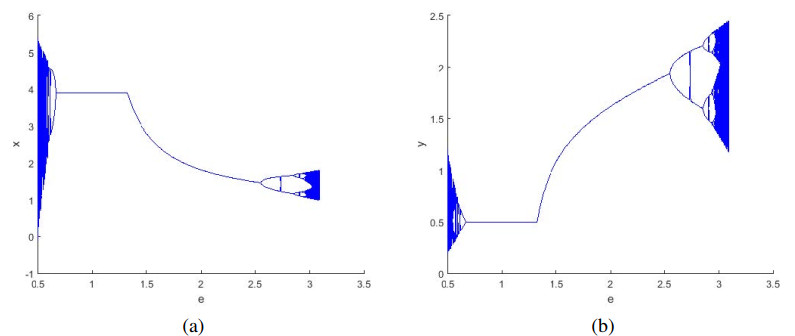
Figures 4–5 presents the dynamics of the F-F HBV model (5.1) with attributed values of Table 2 involving TDs \zeta_{1} = 1, \; \zeta_{2} = 2, assuming \sigma_{1} = 0.003, \sigma_{2} = 0.004, \; \sigma_{3} = 0.006 and \sigma_{4} = 0.004. Straightforward computations result in \mathbb{R}_{0}^{{\bf s}} < 1 , satisfying the requirements of Theorem 4.2. Figures 4-5 demonstrate that there are fewer individuals who have been treated by untreated adults and that the proportion of acutely and chronically contaminated youngsters is declining, respectively. Therefore, we observe that controlling the preponderance of individuals who are HBV-positive may significantly lower or reduce the amount of contaminated neonates who are released during the distribution process when fractional-order \delta decreasing and fractal-dimension \varpi remains fixed. It is concluded that the F-F HBV model (5.1) generated in this research is perfectly accurate. It is thought that it can highlight several important characteristics that are also true in more simulation designs of HBV infection.
Figures 6–7 define the complexities of the F-F HBV model (5.1) with aforementioned parametric descriptions considering TDs \zeta_{1} = 1, \; \zeta_{2} = 2, assuming \sigma_{1} = 0.003, \sigma_{2} = 0.004, \; \sigma_{3} = 0.006 and \sigma_{4} = 0.004. This also predicts that, through cytolytic and non-cytolytic processes, CTL cells are essential for the prevention and treatment of HBV infection. Infectious colonies are killed by cytolytic control by manipulating, while noncytolytic regulatory processes "treat" the intracellular pathogens [51]. According to F-F investigations, the infectious equilibrium state is robust and the pace at which pathogens produce free viral infection is low. If a medicine with a profound impact is discovered, the value of the rate of the viral bacteria's item will decrease, and other immunization collaborators should step up their efforts to reduce interaction rates to a meaningful scale when \delta is fixed and \varpi declines. The HBV infection can be treated.
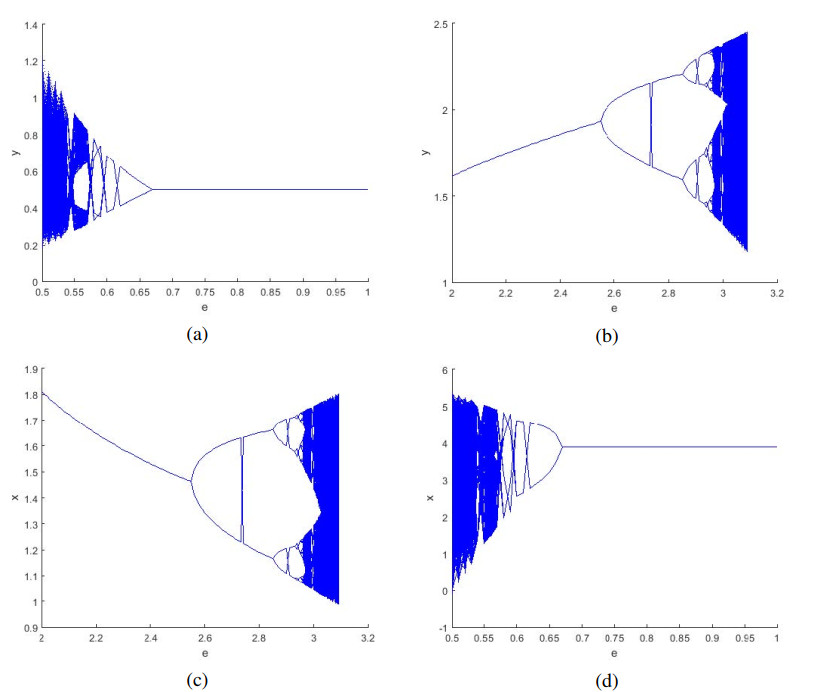
Figures 8–9 illustrate the complexities of the F-F HBV model, (5.1) with aforementioned parametric descriptions, considering TDs \zeta_{1} = 1, \; \zeta_{2} = 2, assuming \sigma_{1} = 0.1, \sigma_{2} = 0.2, \; \sigma_{3} = 0.3 and \sigma_{4} = 0.4. The likelihood that the sickness will disappear is, therefore, one. It has been demonstrated that a white noise setting with a higher intensity may aid in curing the illness more quickly than a simulation sans noise when \delta decreases and \varpi increased. Our analysis's clear and fundamental goal is to minimize the harm inflicted by HBV by reducing the population's infection rate while increasing the population's rate of recovery. The authorities have a mission to ensure that people are notified of the proliferation of the HBV.
Figures 10–11 show the intricacies of the F-F HBV model, (5.1) utilizing the parameterized representations stated above and TDs \zeta_{1} = 1, \; \zeta_{2} = 2, assuming \sigma_{1} = 0.1, \sigma_{2} = 0.2, \; \sigma_{3} = 0.3 and \sigma_{4} = 0.4. The aforementioned configurations 10-11 makes it evident that the basic reproduction ratio \mathbb{R}_{0}^{{\bf s}} in framework (3.1) does not incorporate the index of CTL cells, which means that \mathbb{R}_{0}^{{\bf s}} is unable to accurately represent the function of immunogenicity. However, formula (5.1) includes the \delta and \varpi , which helps illustrate the function of the CTL cells. As a result, the model (5.1) ought to be more feasible.
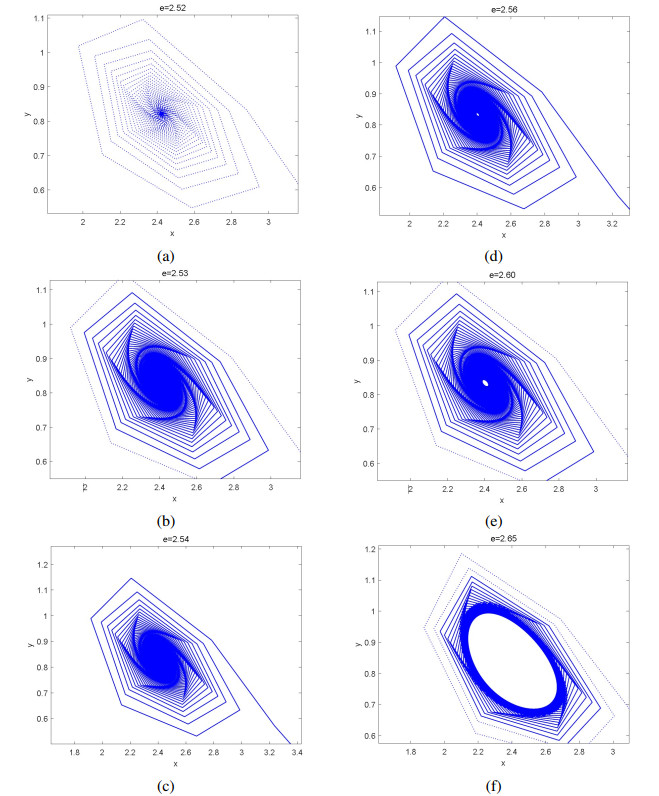
Figures 12–13 presents the solution for low densities of \sigma_{\kappa}, \; \kappa = 1, 2, 3, 4 using the Matlab package. Therefore, if a treatment with a huge influence can be discovered, the HBV infection can be treated provided that the value of \vartheta is sufficiently diminished. According to the above mechanism, since many people are uneducated and unaware of viral diseases associated with them, particularly HBV, it indicates that the current regime should encourage good hygiene precautions in the general populace. Both the recycling of syringes on minors in rural areas and inadequate sanitary standards in public health sectors, which are major causes of HBV, should be prohibited. Blood donations must follow a specified protocol and must first be tested and approved by an officially recognized lab.
In a nutshell, the presence of an F-F derivative operator with stochastic disturbance (noise) and intracellular TDs in the framework (3.1) is hypothesized to deliver a better awareness in the presentation of the presented data, which has serious repercussions for treating alternatives and targeted therapies.
6.
Conclusion
In the current study, we examined the effects of high-order stochastic disturbances on the complexities of the delay differential framework of HBV disease, which includes internalized latency, immunotherapy, disease-to-organ and their transmissions via the F-F derivative within the exponential decay kernel. We also presented the non-negative solution pertaining to the unit probability, stochastic reproduction number, and local stability, which is calculated for the steady states. Under certain assumptions, the sickness may eventually go away under certain circumstances, with probability 1. A revolutionary approach is used in a handful of simulated findings to demonstrate the viability of the outcomes. In the therapy of HBV and other viral disorders, the volume of Gaussian white noise is crucial. The presence of random disturbances (noise) and biochemical TDs in the framework is predicated to provide a greater understanding of the quantitative results, which has significant consequences for antibiotic compounds and regenerative medicine. Several additional intriguing subjects need to be looked into more thoroughly. Other types of environmental noise, including Lévy noise, may be taken into consideration [52]. Additionally, the deterministic system can be expanded to incorporate fractional derivatives in the framework to be able to take into account long-run memory of the bacteria's behavior, which is suggested by the research in [53,54].
Acknowledgments
All sources of funding of the study must be disclosed
Conflict of interest
The authors declare there is no conflict of interest.









 DownLoad:
DownLoad: