A novel analysis of the time-fractional nonlinear dispersive K(m, n, 1) equations using the homotopy perturbation transform method and Yang transform decomposition method

Abdul Hamid Ganie; Fatemah Mofarreh; Adnan Khan; Abdul Hamid Ganie; Fatemah Mofarreh; Adnan Khan

doi:10.3934/math.2024092

AIMS Mathematics

2024, Volume 9, Issue 1: 1877-1898. doi: 10.3934/math.2024092

Previous Article Next Article

Research article Special Issues

A novel analysis of the time-fractional nonlinear dispersive K(m, n, 1) equations using the homotopy perturbation transform method and Yang transform decomposition method

1.
Basic Science Department, College of Science and Theoretical Studies, Saudi Electronic University, Riyadh 11673, Saudi Arabia
2.
Department of Mathematical Science, College of Sciences, Princess Nourah Bint Abdulrahman University, Riyadh 11546, Saudi Arabia
3.
Department of Mathematics, Abdul Wali Khan University Mardan, Mardan 23200, Pakistan

Received: 28 August 2023 Revised: 23 November 2023 Accepted: 07 December 2023 Published: 18 December 2023
MSC : 26A33, 35A20, 35L05

The main features of scientific effort in physics and engineering are the development of models for various physical issues and the development of solutions. In this paper, we investigate the numerical solution of time-fractional non-linear dispersive K(m, n, 1) type equations using two innovative approaches: the homotopy perturbation transform method and Yang transform decomposition method. Our suggested approaches elegantly combine Yang transform, homotopy perturbation method (HPM) and adomian decomposition method (ADM). With the help of the Yang transform, we first convert the problem into its differential partner before using HPM to get the He's polynomials and ADM to get the Adomian polynomials, both of which are extremely effective supports for non-linear issues. In this case, Caputo sense is used for defining the fractional derivative. The derived solutions are shown in series form and converge quickly. To ensure the effectiveness and applicability of the proposed approaches, the examined problems were analyzed using various fractional orders. We analyze and demonstrate the validity and applicability of the solution approaches under consideration with given initial conditions. Two and three dimensional graphs reflect the outcomes that were attained. To verify the effectiveness of the strategies, numerical simulations are presented. The numerical outcomes demonstrate that only a small number of terms are required to arrive at an approximation that is exact, efficient, and trustworthy. The results of this study demonstrate that the studied methods are effective and strong in solving nonlinear differential equations that appear in science and technology.

Keywords:

analytical methods,
time-fractional nonlinear dispersive K(m, n, 1) equation,
Caputo operator

Citation: Abdul Hamid Ganie, Fatemah Mofarreh, Adnan Khan. A novel analysis of the time-fractional nonlinear dispersive K(m, n, 1) equations using the homotopy perturbation transform method and Yang transform decomposition method[J]. AIMS Mathematics, 2024, 9(1): 1877-1898. doi: 10.3934/math.2024092

Related Papers:

[1]	Aslı Alkan, Halil Anaç . The novel numerical solutions for time-fractional Fornberg-Whitham equation by using fractional natural transform decomposition method. AIMS Mathematics, 2024, 9(9): 25333-25359. doi: 10.3934/math.20241237
[2]	Azzh Saad Alshehry, Naila Amir, Naveed Iqbal, Rasool Shah, Kamsing Nonlaopon . On the solution of nonlinear fractional-order shock wave equation via analytical method. AIMS Mathematics, 2022, 7(10): 19325-19343. doi: 10.3934/math.20221061
[3]	Jagdev Singh, Arpita Gupta . Computational analysis of fractional modified Degasperis-Procesi equation with Caputo-Katugampola derivative. AIMS Mathematics, 2023, 8(1): 194-212. doi: 10.3934/math.2023009
[4]	Naveed Iqbal, Saleh Alshammari, Thongchai Botmart . Evaluation of regularized long-wave equation via Caputo and Caputo-Fabrizio fractional derivatives. AIMS Mathematics, 2022, 7(11): 20401-20419. doi: 10.3934/math.20221118
[5]	Muath Awadalla, Abdul Hamid Ganie, Dowlath Fathima, Adnan Khan, Jihan Alahmadi . A mathematical fractional model of waves on Shallow water surfaces: The Korteweg-de Vries equation. AIMS Mathematics, 2024, 9(5): 10561-10579. doi: 10.3934/math.2024516
[6]	Shabir Ahmad, Aman Ullah, Ali Akgül, Fahd Jarad . A hybrid analytical technique for solving nonlinear fractional order PDEs of power law kernel: Application to KdV and Fornberg-Witham equations. AIMS Mathematics, 2022, 7(5): 9389-9404. doi: 10.3934/math.2022521
[7]	Manal Alqhtani, Khaled M. Saad, Rasool Shah, Thongchai Botmart, Waleed M. Hamanah . Evaluation of fractional-order equal width equations with the exponential-decay kernel. AIMS Mathematics, 2022, 7(9): 17236-17251. doi: 10.3934/math.2022949
[8]	Yousef Jawarneh, Humaira Yasmin, M. Mossa Al-Sawalha, Rasool Shah, Asfandyar Khan . Fractional comparative analysis of Camassa-Holm and Degasperis-Procesi equations. AIMS Mathematics, 2023, 8(11): 25845-25862. doi: 10.3934/math.20231318
[9]	M. Mossa Al-Sawalha, Azzh Saad Alshehry, Kamsing Nonlaopon, Rasool Shah, Osama Y. Ababneh . Approximate analytical solution of time-fractional vibration equation via reliable numerical algorithm. AIMS Mathematics, 2022, 7(11): 19739-19757. doi: 10.3934/math.20221082
[10]	Khalid Khan, Amir Ali, Manuel De la Sen, Muhammad Irfan . Localized modes in time-fractional modified coupled Korteweg-de Vries equation with singular and non-singular kernels. AIMS Mathematics, 2022, 7(2): 1580-1602. doi: 10.3934/math.2022092

Abstract

Nomenclature

The following abbreviations are used in this article:

FC	Fractional calculus
PDEs	Partial differential equations
FPDEs	Fractional-order partial differential equations
YT	Yang transform
YTDM	Yang transform decomposition method
HPTM	Homotopy perturbation transform method
$\Psi$	Independent variable
$\mathcal{T}$	Time
$\Theta(\Psi, \mathcal{T})$	Dependent function representing the physical quantity
$\wp$	Fractional order
$Y$	Yang transform
$Y^{-1}$	Inverse Yang transform
$\epsilon$	Perturbation parameter

1. Introduction

Fractional calculus (FC) offers a more simple representation of reality. FC offers a better explanation of the fundamental nature of reality that makes this subject as a common interest in the world of science and engineering. Since FC is the language that nature knows, communicating with it in this way is effective. The FC problem has been with mathematicians for the past three centuries, and only in the last few years has it been brought into more practical sectors like engineering, science and economics. Integrals and derivatives are addressed by FC to an arbitrary real or complex order. Recently, a number of fractional operators, including Caputo, Caputo Fabrizio, Atangana-Baleanu, Katugampola, Hilfer, etc, have been proposed and implemented for dealing with real-world applications ^{[1,2,3,4,5,6]}. Fractional calculus has demonstrated that it is the best tool for studying problems in the actual world. This area of applied analysis has been utilised in a number of technological, engineering, and scientific fields. In order to optimise the wave equation, Bulut et al. ^[7] examined the time-fractional generalised Burger equation and trial equations. In 1998, He ^[8] examined the compact solution for the seepage flow equation in porous media. In 2020, Dubey et al. investigated the computer virus propagation model with fractional order ^[9]. The mathematical model for the chemical system was presented by Kumar et al. ^[10]. The fractional order multi-dimensional diffusion issues were studied by Singh et al. ^[11]. The Caputo fractional derivative is often used in practical applications, as it enables one to include the traditional initial and boundary conditions in formulating mathematical models. Moreover, as in the integer-order derivative, the Caputo fractional derivative of a constant is zero ^[12].

Partial differential equations (PDEs) can be used to express a specific relationship between an unknown function and its partial derivatives. PDEs are widely used in all branches of engineering and science. PDE usage has grown significantly in recent years in fields like biology, economics, image processing and graphics, and social sciences. As a result, suitable functions in these fields can be recognized when some independent variables interact with one another in each of the aforementioned fields, allowing for the modeling of a number of processes by generating equations for the corresponding functions. The study of PDEs has many aspects. Numerous applications can be made from PDE theoretical analysis. It should be mentioned that there are extremely hard equations that even supercomputers cannot solve. All that can be done in such instances is try to collect accurate data on the solution. Additionally, a significantly key issue is the formulation of the equation and its associated side conditions. Usually, a model of a physical or engineering problem serves as the foundation for the equation and it is not immediately obvious that the model is consistent with regard to the results in a solved PDE. Furthermore, it is preferable in the majority of circumstances for the solution to be distinct and stable against minor data disruptions. To determine whether these requirements are met, it is helpful to have a theoretical understanding of the equation. There have been many different approaches for solving classical PDEs proposed, as well as several solutions revealed ^{[13,14,15,16,17,18,19,20]}.

Due to their numerous applications in diverse scientific domains, the idea of fractional partial differential equations (FPDEs) has been the subject of several studies and is an important topic in computational mathematics ^{[21,22,23,24,25,26,27]}. The diffusion process can be described more precisely using the fractional derivative by taking into account the long-range interactions, memory effects, and other physics-related phenomena as well as the majority of biological systems. Researchers have recently shown that many phenomena can be accurately modeled by non-integer order mathematical models using mathematical tools, such as the diffusion wave equations, Keller-Segel model for chemotaxis ^[28], fractional Radhakrishnan-Kundu-Lakshmanan equation ^[29] and fractional Riccati differential equations ^[30]. Despite their extensive use and applications, there is a significant problem with the numerical approaches that are presently available for finding solutions to FPDEs. The present study was encouraged by the need for a comprehensive approach that could be applied to problems such as homogeneous, nonhomogeneous, linear, nonlinear and multivariable FPDEs, without requiring important adjustments. The growing academic interest in the numerical solutions of fractional PDEs has led to significant advancements in the study of nonlinear PDEs. However, some of the main drawbacks of numerical approaches are the restrictions in accuracy, mesh construction, transformations, stability, convergence, and the difficulty of applying them to complex geometries.

Mathematicians have created a variety of numerical and analytical strategies to solve FPDEs in light of the aforementioned applications. For example, in order to find series form solutions to various partial differential equations (PDEs) and FPDEs with initial and boundary conditions, Duan et al. ^[31] implemented the Adomian decomposition method (ADM). Natural transform decomposition method has been applied by Botmart et al. for solving fractional approximate long wave and the modified Boussinesq equations ^[32] and fractional-order kaup-kupershmidt equation ^[33]. Similarly, in ^[34], Fathima et al. have applied the natural transform decomposition method for solving fractional Caudrey-Dodd-Gibbon equations. In ^[35], Jafari et al. have used the fractional sub-equation method for the solution of the fractional generalized reaction Duffing model and the nonlinear fractional Sharma-Tasso-Olver equation. The analytical solution of the seventh-order Lax's Korteweg-de Vries equation has been found by Mishra et al. in ^[36]. With the help of the tanh method, Wazwaz in ^[37] found the exact solutions of the sine-Gordon and the sinh-Gordon equations. The numerical solution of fractional phi-four equation has been found in ^[38], using the Yang transform decomposition method. Deng implemented the finite element method for solving the space and time fractional Fokker-Planck equation ^[39]. Mohammed Kbiri Alaoui et al. in ^[40] investigated the time-fractional Belousov-Zhabotinsky reaction analytically.

This work examines the nonlinear dispersive K(m, n, 1) equation with fractional time derivatives:

$\begin{align} \begin{split} &D_\mathcal{T}^\wp{\Theta(\Psi,\mathcal{T})}+(\Theta^{2}(\Psi,\mathcal{T}))_{\Psi}-(\Theta^{2}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi}+(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi} = 0,\ \ \ \ \ 0 < \wp\leq1.\\ \end{split} \end{align}$

(1.1)

For specific values of m and n, the classic nonlinear dispersive K(m, n, 1) equation exhibits solitary waves that are compactly supported. This equation was first proposed by Rosenau and Hymann in 1993. Many other approaches have recently been proposed for studying the nonlinear dispersive K(m, n) equations, including the Adomian method ^[41], the Exp-function method ^[42], the variational iteration method ^[43], the variational method ^[44] and the HPM ^[45]. These types of equations can be solved using a variety of efficient and practical techniques as shown in ^[46]. Equation (1.1) with fractional order of time derivation occurs in discontinuous time on huge time scales in weather forecasting or the extremely short time scale in high energy physics. The fractional model is most suitable to describe such issues because time is discontinuous (but hierarchical) according to the E-infinity hypothesis. Time-fractional equations always exhibit remarkable behaviour as shown in ^[47].

The aim of this study is to extend the use of the Yang transform decomposition technique (YTDM) and homotopy perturbation transform method (HPTM) to get analytical and approximative solutions to the nonlinear dispersive K(m, n, 1) equations with fractional time derivatives. In order to decompose the nonlinear terms, the Adomian and homotopy polynomials are used, and the Yang transform is used to convert differential equations into algebraic equations. Our methods produced infinite series as the results in the numerical examples. When we write the series in closed form, it gives precise solutions to the relevant equations. Researchers can use this study as a fundamental reference to examine these strategies and employ it in many applications to get accurate and approximative results in a few easy steps. The unique aspect of this work is the description of two novel techniques for fractional nonlinear dispersive K(m, n, 1) equations with minimal and consecutive steps.

The current article follows the following format: We begin with the basic concept of FC in Section 2. The main ideas of the recommended approaches are covered in Sections 3 and 4. These techniques are applied to solve the time-fractional nonlinear dispersive K(m, n, 1) problem in Section 5 with the provided initial condition. In Section 6, the conclusion is offered.

2. Preleminaries

In order to give more analysis and define the solution methods, we will present some illustrative definitions about the fractional calculus.

Definition 2.1. The fractional operator in Caputo mean is as ^[48]

$\begin{align} \ \begin{split} &D_{\mathcal{T}}^{\wp}\Theta(\Psi,\mathcal{T}) = \frac{1}{\Gamma(k-\wp)}\int_{0}^{\mathcal{T}}(\mathcal{T}-\gamma)^{k-\wp-1}\Theta^{(k)}(\Psi,\gamma)d\gamma,\quad k-1 < \wp\leq k, \quad k\in N.\\ \end{split} \end{align}$

(2.1)

Definition 2.2. The Yang transform (YT) of the given function is as ^[49]

$\begin{align} Y\{\Theta(\mathcal{T})\} = M(u) = \int_0^{\infty}e^{\frac{-\mathcal{T}}{u}}\Theta(\mathcal{T})d\mathcal{T},\ \ \mathcal{T} > 0, \ \ u\in(-\mathcal{T}_1,\mathcal{T}_2), \end{align}$

(2.2)

illustrating inverse YT as

$\begin{align} Y^{-1}\{M(u)\} = \Theta(\mathcal{T}). \end{align}$

(2.3)

Definition 2.3. The YT associated with nth order derivative is as^[49]

$\begin{align} Y\{\Theta^{n}(\mathcal{T})\} = \frac{M(u)}{u^{n}}-\sum\limits_{k = 0}^{n-1}\frac{\Theta^{k}(0)}{u^{n-k-1}}, \ \forall n = 1,2,3,\cdots. \end{align}$

(2.4)

Definition 2.4. The YT associated with the fractional order derivative is as ^[49]

$\begin{align} Y\{\Theta^{\wp}(\mathcal{T})\} = \frac{M(u)}{u^{\wp}}-\sum\limits_{k = 0}^{n-1}\frac{\Theta^{k}(0)}{u^{\wp-(k+1)}}, \ \ 0 < \wp \leq n. \end{align}$

(2.5)

3. Basic procedure of HPTM

To illustrate the basic idea of this approach, we discuss a nonlinear FPDE.

$\begin{align} \ D_{\mathcal{T}}^{\wp}\Theta(\Psi,\mathcal{T}) = \mathcal{J}_1[\Psi]\Theta(\Psi,\mathcal{T})+\mathcal{K}_1[\Psi]\Theta(\Psi,\mathcal{T}), \ \ 0 < \wp\leq1, \end{align}$

(3.1)

associated with initial guess

$\Theta(\Psi,0) = \xi(\Psi).$

By plugging in the YT, we obtain

$\begin{align} \ Y[D_{\mathcal{T}}^{\wp}\Theta(\Psi,\mathcal{T})] = Y[\mathcal{J}_1[\Psi]\Theta(\Psi,\mathcal{T})+\mathcal{K}_1[\Psi]\Theta(\Psi,\mathcal{T})], \end{align}$

(3.2)

$\begin{align} \frac{1}{u^{\wp}}\{M(u)- u \Theta(0)\} = Y[\mathcal{J}_1[\Psi]\Theta(\Psi,\mathcal{T})+\mathcal{K}_1[\Psi]\Theta(\Psi,\mathcal{T})]. \end{align}$

(3.3)

We have by simplifying

$\begin{align} M(\Theta) = u \Theta(0)+u^{\wp}Y[\mathcal{J}_1[\Psi]\Theta(\Psi,\mathcal{T})+\mathcal{K}_1[\Psi]\Theta(\Psi,\mathcal{T})]. \end{align}$

(3.4)

By employing inverse YT, we get

$\begin{align} \Theta(\Psi,\mathcal{T}) = \Theta(0)+Y^{-1}[u^{\wp}Y[\mathcal{J}_1[\Psi]\Theta(\Psi,\mathcal{T})+\mathcal{K}_1[\Psi]\Theta(\Psi,\mathcal{T})]]. \end{align}$

(3.5)

Now in terms of HPM, we obtain

$\begin{align} \Theta(\Psi,\mathcal{T}) = \sum\limits_{k = 0}^{\infty}\epsilon^{k}\Theta_{k}(\Psi,\mathcal{T}), \end{align}$

(3.6)

with $\epsilon\in[0, 1]$ .

Let

$\begin{align} \mathcal{K}_1[\Psi]\Theta(\Psi,\mathcal{T}) = \sum\limits_{k = 0}^{\infty}\epsilon^{k}H_{n}(\Theta), \end{align}$

(3.7)

with

$\begin{align} \ H_{n}(\Theta_{0},\Theta_{1},...,\Theta_{n}) = \frac{1}{\Gamma(n+1)}D_{\epsilon}^{k}\left[\mathcal{K}_1\left(\sum\limits_{k = 0}^{\infty}\epsilon^{i}\Theta_{i}\right)\right]_{\epsilon = 0}, \end{align}$

(3.8)

where $D_{\epsilon}^{k} = \frac{\partial^{k}}{\partial \epsilon^{k}}$ .

Now, we substitute Eqs (3.6) and (3.7) in Eq (3.5) to get

$\begin{align} \ \sum\limits_{k = 0}^{\infty}\epsilon^{k}\Theta_{k}(\Psi,\mathcal{T}) = \Theta(0)+\epsilon\times\left(Y^{-1}\left[u^{\wp}Y\{\mathcal{J}_1\sum\limits_{k = 0}^{\infty}\epsilon^{k}\Theta_{k}(\Psi,\mathcal{T})+ \sum\limits_{k = 0}^{\infty}\epsilon^{k}H_{k}(\Theta)\}\right]\right). \end{align}$

(3.9)

On comparing the $\epsilon$ coefficients, we conclude

$\begin{align} \begin{split} &\epsilon^{0}:\Theta_{0}(\Psi,\mathcal{T}) = \Theta(0),\\ &\epsilon^{1}:\Theta_{1}(\Psi,\mathcal{T}) = Y^{-1}\left[u^{\wp}Y(\mathcal{J}_1[\Psi]\Theta_{0}(\Psi,\mathcal{T})+H_{0}(\Theta))\right],\\ &\epsilon^{2}:\Theta_{2}(\Psi,\mathcal{T}) = Y^{-1}\left[u^{\wp}Y(\mathcal{J}_1[\Psi]\Theta_{1}(\Psi,\mathcal{T})+H_{1}(\Theta))\right],\\ &.\\ &.\\ &.\\ &\epsilon^{k}:\Theta_{k}(\Psi,\mathcal{T}) = Y^{-1}\left[u^{\wp}Y(\mathcal{J}_1[\Psi]\Theta_{k-1}(\Psi,\mathcal{T})+H_{k-1}(\Theta))\right],\; k > 0,\; k\in N. \end{split} \end{align}$

(3.10)

Finally, the analytical solution is given by

$\begin{align} \Theta(\Psi,\mathcal{T}) = \lim\limits_{M\rightarrow \infty}\sum\limits_{k = 1}^{M}\Theta_{k}(\Psi,\mathcal{T}). \end{align}$

(3.11)

4. Basic procedure of YTDM

To illustrate the basic idea of this approach, we discuss a nonlinear YTDM.

$\begin{align} D_\mathcal{T}^\wp{\Theta(\Psi,\mathcal{T})} = \mathcal{J}_1(\Psi,\mathcal{T})+\mathcal{K}_1(\Psi,\mathcal{T}), \ \ 0 < \wp\leq1, \end{align}$

(4.1)

associated with initial guess

$\Theta(\Psi,0) = \xi(\Psi).$

By plugging in the YT, we obtain

$\begin{align} \begin{split} &Y[D_\mathcal{T}^\wp{\Theta(\Psi,\mathcal{T})}] = Y[\mathcal{J}_1(\Psi,\mathcal{T})+\mathcal{K}_1(\Psi,\mathcal{T})],\\ &\frac{1}{u^{\wp}}\{M(u)- u \Theta(0)\} = Y[\mathcal{J}_1(\Psi,\mathcal{T})+\mathcal{K}_1(\Psi,\mathcal{T})]. \end{split} \end{align}$

(4.2)

We have by simplifying

$\begin{align} M(\Theta) = u \Theta(0)+u^{\wp}Y[\mathcal{J}_1(\Psi,\mathcal{T})+\mathcal{K}_1(\Psi,\mathcal{T})]. \end{align}$

(4.3)

By employing inverse YT, we obtain

$\begin{align} \Theta(\Psi,\mathcal{T}) = \Theta(0)+Y^{-1}[u^{\wp}Y[\mathcal{J}_1(\Psi,\mathcal{T})+\mathcal{K}_1(\Psi,\mathcal{T})]. \end{align}$

(4.4)

Now, the solution in terms of infinite series is

$\begin{align} \Theta(\Psi,\mathcal{T}) = \sum\limits_{m = 0}^{\infty}\Theta_m(\Psi,\mathcal{T}). \end{align}$

(4.5)

Also, the nonlinear term is illustrated as

$\begin{align} \mathcal{K}_1(\Psi,\mathcal{T}) = \sum\limits_{m = 0}^{\infty}\mathcal{A}_m, \end{align}$

(4.6)

with

$\begin{align} \mathcal{A}_m = \frac{1}{m!}\left[\frac{\partial^m}{\partial\ell^m}\left\{\mathcal{K}_1\left(\sum\limits_{k = 0}^{\infty}\ell^k\Psi_k,\sum\limits_{k = 0}^{\infty}\ell^k\mathcal{T}_k\right)\right\}\right]_{\ell = 0}. \end{align}$

(4.7)

Now, we substitute Eqs (4.5) and (4.6) in Eq (4.4) to get

$\begin{align} \sum\limits_{m = 0}^{\infty}\Theta_m(\Psi,\mathcal{T}) = \Theta(0)+Y^{-1} u^\wp \left[Y\left\{\mathcal{J}_1(\sum\limits_{m = 0}^{\infty}\Psi_m,\sum\limits_{m = 0}^{\infty}\mathcal{T}_m)+\sum\limits_{m = 0}^{\infty}\mathcal{A}_m\right\}\right]. \end{align}$

(4.8)

On comparison of both sides, we conclude

$\begin{align} \Theta_0(\Psi,\mathcal{T}) = \Theta(0), \end{align}$

(4.9)

$\begin{equation*} \Theta_1(\Psi,\mathcal{T}) = Y^{-1}\left[u^\wp Y\{\mathcal{J}_1(\Psi_0,\mathcal{T}_0)+\mathcal{A}_0\}\right]. \end{equation*}$

Finally, the analytical solution is given by

$\begin{equation*} \Theta_{m+1}(\Psi,\mathcal{T}) = Y^{-1}\left[u^\wp Y\{\mathcal{J}_1(\Psi_m,\mathcal{T}_m)+\mathcal{A}_m\}\right]. \end{equation*}$

5. Applications

Example 1. Assume the fractional K(2, 2, 1) equation is

(5.1)

associated with initial guess

$\begin{equation*} \Theta(\Psi,0) = \frac{16\rho-1}{12}\cosh^{2}\Bigg(\frac{\Psi}{4}\Bigg). \end{equation*}$

By plugging in the YT, we obtain

$\begin{align} \ Y\left(\frac{\partial^{\wp}\Theta}{\partial \mathcal{T}^{\wp}}\right) = Y\Bigg((\Theta^{2}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi}-(\Theta^{2}(\Psi,\mathcal{T}))_{\Psi}-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg). \end{align}$

(5.2)

We have by simplifying

$\begin{align} \ \frac{1}{u^{\wp}}\{M(u)- u \Theta(0)\} = Y\Bigg((\Theta^{2}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi}-(\Theta^{2}(\Psi,\mathcal{T}))_{\Psi}-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg), \end{align}$

(5.3)

$\begin{align} \ M(u) = u \Theta(0)+u^{\wp} \Bigg((\Theta^{2}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi}-(\Theta^{2}(\Psi,\mathcal{T}))_{\Psi}-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg). \end{align}$

(5.4)

By employing inverse YT, we obtain

$\begin{align} \begin{split} &\Theta(\Psi,\mathcal{T}) = \Theta(0)+Y^{-1}\Bigg[u^\wp\Bigg\{Y\Bigg((\Theta^{2}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi}-(\Theta^{2}(\Psi,\mathcal{T}))_{\Psi}-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg)\Bigg\}\Bigg],\\ &\Theta(\Psi,\mathcal{T}) = \frac{16\rho-1}{12}\cosh^{2}\Bigg(\frac{\Psi}{4}\Bigg)+Y^{-1}\Bigg[u^\wp\Bigg\{Y\Bigg((\Theta^{2}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi}-(\Theta^{2}(\Psi,\mathcal{T}))_{\Psi}-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg)\Bigg\}\Bigg]. \end{split} \end{align}$

(5.5)

Now in terms of HPM, we get

$\begin{align} \begin{split} \sum\limits_{k = 0}^{\infty}\epsilon^{k}\Theta_{k}(\Psi,\mathcal{T}) = &\frac{16\rho-1}{12}\cosh^{2}\Bigg(\frac{\Psi}{4}\Bigg)+\epsilon\Bigg(Y^{-1}\Bigg[u^{\wp}Y\Bigg[\Bigg(\sum\limits_{k = 0}^{\infty}\epsilon^{k}H_{k}^{1}(\Theta)\Bigg)-\Bigg(\sum\limits_{k = 0}^{\infty}\epsilon^{k}H_{k}^{2}(\Theta)\Bigg)\\ &-\Bigg(\sum\limits_{k = 0}^{\infty}\epsilon^{k}\Theta_{k}(\Psi,\mathcal{T})\Bigg)_{\Psi\Psi\Psi\Psi\Psi}\Bigg]\Bigg]\Bigg). \end{split} \end{align}$

(5.6)

Assume the He's polynomial $H_{k}(\mathbb{U})$ as

$\begin{align} \begin{split} &\sum\limits_{k = 0}^{\infty}\epsilon^{k}H^{1}_{k}(\Theta) = (\Theta^{2}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi},\\ &\sum\limits_{k = 0}^{\infty}\epsilon^{k}H^{2}_{k}(\Theta) = (\Theta^{2}(\Psi,\mathcal{T}))_{\Psi}. \end{split} \end{align}$

(5.7)

The first components are illustrated as

$\begin{equation*} \ \begin{split} &H^{1}_{0}(\Theta) = (\Theta_{0}^{2})_{\Psi\Psi\Psi},\\ &H^{1}_{1}(\Theta) = (2\Theta_{0}\Theta_{1})_{\Psi\Psi\Psi},\\ &H^{1}_{2}(\Theta) = (\Theta_{1}^{2}+2\Theta_{0}\Theta_{2})_{\Psi\Psi\Psi},\\ &\vdots\\ &H^{2}_{0}(\Theta) = (\Theta_{0}^{2})_{\Psi},\\ &H^{2}_{1}(\Theta) = (2\Theta_{0}\Theta_{1})_{\Psi},\\ &H^{2}_{2}(\Theta) = (\Theta_{1}^{2}+2\Theta_{0}\Theta_{2})_{\Psi}. \end{split} \end{equation*}$

On comparing the $\epsilon$ coefficients, we conclude

$\begin{equation*} \ \begin{split} &\epsilon^{0}:\Theta_{0}(\Psi,\mathcal{T}) = \frac{16\rho-1}{12}\cosh^{2}\Bigg(\frac{\Psi}{4}\Bigg),\\ &\epsilon^{1}:\Theta_{1}(\Psi,\mathcal{T}) = -\frac{(16\rho-1)\rho}{24\cdot2}\sinh\Bigg(\frac{\Psi}{2}\Bigg)\frac{\mathcal{T}^{\wp}}{\Gamma(\wp+1)},\\ &\epsilon^{2}:\Theta_{2}(\Psi,\mathcal{T}) = \frac{(16\rho-1)\rho^{2}}{24\cdot2^{2}}\cosh\Bigg(\frac{\Psi}{2}\Bigg)\frac{\mathcal{T}^{2\wp}}{\Gamma(2\wp+1)},\\ &\epsilon^{3}:\Theta_{3}(\Psi,\mathcal{T}) = -\frac{(16\rho-1)\rho^{3}}{24\cdot2^{3}}\sinh\Bigg(\frac{\Psi}{2}\Bigg)\frac{\mathcal{T}^{3\wp}}{\Gamma(3\wp+1)},\\ &\vdots \end{split} \end{equation*}$

Finally, the analytical solution is given by

$\begin{equation*} \ \begin{split} \Theta({\Psi},{\mathcal{T}}) = &{\Theta}_{0}({\Psi},{\mathcal{T}})+{\Theta}_{1}({\Psi},{\mathcal{T}})+{\Theta}_{2}({\Psi},{\mathcal{T}})+{\Theta}_{3}({\Psi},{\mathcal{T}})+\cdots,\\ \Theta({\Psi},{\mathcal{T}}) = &\frac{16\rho-1}{12}\cosh^{2}\Bigg(\frac{\Psi}{4}\Bigg)-\frac{(16\rho-1)\rho}{24\cdot2}\sinh\Bigg(\frac{\Psi}{2}\Bigg)\frac{\mathcal{T}^{\wp}}{\Gamma(\wp+1)}+\frac{(16\rho-1)\rho^{2}}{24\cdot2^{2}}\cosh\Bigg(\frac{\Psi}{2}\Bigg)\frac{\mathcal{T}^{2\wp}}{\Gamma(2\wp+1)}\\ &-\frac{(16\rho-1)\rho^{3}}{24\cdot2^{3}}\sinh\Bigg(\frac{\Psi}{2}\Bigg)\frac{\mathcal{T}^{3\wp}}{\Gamma(3\wp+1)}+\cdots . \end{split} \end{equation*}$

Solution by implementing YTDM

By plugging in the YT, we obtain

$\begin{align} Y\left\{\frac{\partial^{\wp}\Theta}{\partial \mathcal{T}^{\wp}}\right\} = Y\Bigg((\Theta^{2}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi}-(\Theta^{2}(\Psi,\mathcal{T}))_{\Psi}-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg). \end{align}$

(5.8)

We have by simplifying

$\begin{align} \frac{1}{u^{\wp}}\{M(u)- u \Theta(0)\} = Y\Bigg((\Theta^{2}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi}-(\Theta^{2}(\Psi,\mathcal{T}))_{\Psi}-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg), \end{align}$

(5.9)

$\begin{align} M(u) = u \Theta(0)+u^{\wp}Y\Bigg((\Theta^{2}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi}-(\Theta^{2}(\Psi,\mathcal{T}))_{\Psi}-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg). \end{align}$

(5.10)

By employing inverse YT, we obtain

$\begin{align} \ \begin{split} &\Theta(\Psi,\mathcal{T}) = \Theta(0)+Y^{-1}\Bigg[u^\wp\Bigg\{Y\Bigg((\Theta^{2}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi}-(\Theta^{2}(\Psi,\mathcal{T}))_{\Psi}-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg)\Bigg\}\Bigg],\\ &\Theta(\Psi,\mathcal{T}) = \frac{16\rho-1}{12}\cosh^{2}\Bigg(\frac{\Psi}{4}\Bigg)+Y^{-1}\Bigg[u^\wp\Bigg\{Y\Bigg((\Theta^{2}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi}-(\Theta^{2}(\Psi,\mathcal{T}))_{\Psi}-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg)\Bigg\}\Bigg]. \end{split} \end{align}$

(5.11)

Now the solution in terms of infinite series is

$\begin{align} \Theta(\Psi,\mathcal{T}) = \sum\limits_{m = 0}^{\infty}\Theta_m(\Psi,\mathcal{T}). \end{align}$

(5.12)

Also, the nonlinear term is illustrated as $(\Theta^{2}(\Psi, \mathcal{T}))_{\Psi} = \sum_{m = 0}^{\infty}\mathcal{A}_m, (\Theta^{2}(\Psi, \mathcal{T}))_{\Psi} = \sum_{m = 0}^{\infty}\mathcal{B}_m.$ So, we get

$\begin{align} \begin{split} &\sum\limits_{m = 0}^{\infty}\Theta_m(\Psi,\mathcal{T}) = \Theta(\Psi,0) +Y^{-1}\Bigg[u^\wp Y\Bigg[\Bigg(\sum\limits_{m = 0}^{\infty}\mathcal{A}_m\Bigg)-\Bigg(\sum\limits_{m = 0}^{\infty}\mathcal{B}_m\Bigg)-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg]\Bigg],\\ &\sum\limits_{m = 0}^{\infty}\Theta_m(\Psi,\mathcal{T}) = \frac{16\rho-1}{12}\cosh^{2}\Bigg(\frac{\Psi}{4}\Bigg) +Y^{-1}\Bigg[u^\wp Y\Bigg[\Bigg(\sum\limits_{m = 0}^{\infty}\mathcal{A}_m\Bigg)-\Bigg(\sum\limits_{m = 0}^{\infty}\mathcal{B}_m\Bigg)-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg]\Bigg]. \end{split} \end{align}$

(5.13)

The first components are illustrated as

$\begin{equation*} \ \begin{split} &\mathcal{A}_0 = (\Theta_{0}^{2})_{\Psi\Psi\Psi},\\ &\mathcal{A}_1 = (2\Theta_{0}\Theta_{1})_{\Psi\Psi\Psi},\\ &\mathcal{A}_2 = (\Theta_{1}^{2}+2\Theta_{0}\Theta_{2})_{\Psi\Psi\Psi},\\ &\vdots\\ &\mathcal{B}_0 = (\Theta_{0}^{2})_{\Psi},\\ &\mathcal{B}_1 = (2\Theta_{0}\Theta_{1})_{\Psi},\\ &\mathcal{B}_2 = (\Theta_{1}^{2}+2\Theta_{0}\Theta_{2})_{\Psi}. \end{split} \end{equation*}$

On comparing both sides, we conclude

$\begin{equation*} \Theta_0(\Psi,\mathcal{T}) = \frac{16\rho-1}{12}\cosh^{2}\Bigg(\frac{\Psi}{4}\Bigg). \end{equation*}$

On $m = 0$ ,

$\begin{equation*} \Theta_1(\Psi,\mathcal{T}) = -\frac{(16\rho-1)\rho}{24\cdot2}\sinh\Bigg(\frac{\Psi}{2}\Bigg)\frac{\mathcal{T}^{\wp}}{\Gamma(\wp+1)}. \end{equation*}$

On $m = 1$ ,

$\begin{equation*} \Theta_2(\Psi,\mathcal{T}) = \frac{(16\rho-1)\rho^{2}}{24\cdot2^{2}}\cosh\Bigg(\frac{\Psi}{2}\Bigg)\frac{\mathcal{T}^{2\wp}}{\Gamma(2\wp+1)}. \end{equation*}$

On $m = 2$ ,

$\begin{equation*} \Theta_3(\Psi,\mathcal{T}) = -\frac{(16\rho-1)\rho^{3}}{24\cdot2^{3}}\sinh\Bigg(\frac{\Psi}{2}\Bigg)\frac{\mathcal{T}^{3\wp}}{\Gamma(3\wp+1)}. \end{equation*}$

Finally, the analytical solution is given by

$\begin{equation*} \Theta(\Psi,\mathcal{T}) = \sum\limits_{m = 0}^{\infty}\Theta_m(\Psi,\mathcal{T}) = \Theta_0(\Psi,\mathcal{T})+\Theta_1(\Psi,\mathcal{T})+\Theta_2(\Psi,\mathcal{T})+\Theta_2(\Psi,\mathcal{T})+\cdots, \end{equation*}$

$\begin{equation*} \begin{split} \Theta(\Psi,\mathcal{T}) = &\frac{16\rho-1}{12}\cosh^{2}\Bigg(\frac{\Psi}{4}\Bigg)-\frac{(16\rho-1)\rho}{24\cdot2}\sinh\Bigg(\frac{\Psi}{2}\Bigg)\frac{\mathcal{T}^{\wp}}{\Gamma(\wp+1)}+\frac{(16\rho-1)\rho^{2}}{24\cdot2^{2}}\cosh\Bigg(\frac{\Psi}{2}\Bigg)\frac{\mathcal{T}^{2\wp}}{\Gamma(2\wp+1)}\\&- \frac{(16\rho-1)\rho^{3}}{24\cdot2^{3}}\sinh\Bigg(\frac{\Psi}{2}\Bigg)\frac{\mathcal{T}^{3\wp}}{\Gamma(3\wp+1)}+\cdots . \end{split} \end{equation*}$

Taking $\wp = 1$ , we get

$\begin{align} \ \Theta({\Psi},{\mathcal{T}}) = \frac{16\rho-1}{12}\cosh^{2}\Bigg(\frac{\rho\mathcal{T}-\Psi}{4}\Bigg). \end{align}$

(5.14)

Example 2. Assume the fractional K(3, 3, 1) equation is

$\begin{align} \begin{split} &D_\mathcal{T}^\wp{\Theta(\Psi,\mathcal{T})}+(\Theta^{3}(\Psi,\mathcal{T}))_{\Psi}-(\Theta^{3}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi}+(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi} = 0,\ \ \ \ \ 0 < \wp\leq1, \end{split} \end{align}$

(5.15)

associated with initial guess

$\begin{equation*} \Theta(\Psi,0) = \sqrt{\frac{81\rho-1}{54}}\cosh\Bigg(\frac{\Psi}{3}\Bigg). \end{equation*}$

By plugging in the YT, we have

$\begin{align} \ Y\left(\frac{\partial^{\wp}\Theta}{\partial \mathcal{T}^{\wp}}\right) = Y\Bigg((\Theta^{3}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi}-(\Theta^{3}(\Psi,\mathcal{T}))_{\Psi}-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg). \end{align}$

(5.16)

We have by simplifying

$\begin{align} \ \frac{1}{u^{\wp}}\{M(u)- u \Theta(0)\} = Y\Bigg((\Theta^{3}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi}-(\Theta^{3}(\Psi,\mathcal{T}))_{\Psi}-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg), \end{align}$

(5.17)

$\begin{align} \ M(u) = u \Theta(0)+u^{\wp} \Bigg((\Theta^{3}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi}-(\Theta^{3}(\Psi,\mathcal{T}))_{\Psi}-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg). \end{align}$

(5.18)

By employing inverse YT, we get

$\begin{align} \ \begin{split} &\Theta(\Psi,\mathcal{T}) = \Theta(0)+Y^{-1}\Bigg[u^\wp\Bigg\{Y\Bigg((\Theta^{3}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi}-(\Theta^{3}(\Psi,\mathcal{T}))_{\Psi}-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg)\Bigg\}\Bigg],\\ &\Theta(\Psi,\mathcal{T}) = \sqrt{\frac{81\rho-1}{54}}\cosh\Bigg(\frac{\Psi}{3}\Bigg)+Y^{-1}\Bigg[u^\wp\Bigg\{Y\Bigg((\Theta^{3}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi}-(\Theta^{3}(\Psi,\mathcal{T}))_{\Psi}-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg)\Bigg\}\Bigg]. \end{split} \end{align}$

(5.19)

Now in terms of HPM, we get

$\begin{align} \begin{split} \sum\limits_{k = 0}^{\infty}\epsilon^{k}\Theta_{k}(\Psi,\mathcal{T}) = &\sqrt{\frac{81\rho-1}{54}}\cosh\Bigg(\frac{\Psi}{3}\Bigg)+\epsilon\Bigg(Y^{-1}\Bigg[u^{\wp}Y\Bigg[\Bigg(\sum\limits_{k = 0}^{\infty}\epsilon^{k}H_{k}^{1}(\Theta)\Bigg)-\Bigg(\sum\limits_{k = 0}^{\infty}\epsilon^{k}H_{k}^{2}(\Theta)\Bigg)\\ &-\Bigg(\sum\limits_{k = 0}^{\infty}\epsilon^{k}\Theta_{k}(\Psi,\mathcal{T})\Bigg)_{\Psi\Psi\Psi\Psi\Psi}\Bigg]\Bigg]\Bigg). \end{split} \end{align}$

(5.20)

Assume the He's polynomial $H_{k}(\mathbb{U})$ as

$\begin{align} \begin{split} &\sum\limits_{k = 0}^{\infty}\epsilon^{k}H^{1}_{k}(\Theta) = (\Theta^{3}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi},\\ &\sum\limits_{k = 0}^{\infty}\epsilon^{k}H^{2}_{k}(\Theta) = (\Theta^{3}(\Psi,\mathcal{T}))_{\Psi}.\\ \end{split} \end{align}$

(5.21)

The first components are illustrated as

$\begin{equation*} \ \begin{split} &H^{1}_{0}(\Theta) = (\Theta_{0}^{3})_{\Psi\Psi\Psi},\\ &H^{1}_{1}(\Theta) = (3\Theta_{0}^{2}\Theta_{1})_{\Psi\Psi\Psi},\\ &H^{1}_{2}(\Theta) = (3\Theta_{2}\Theta_{0}^{2}+3\Theta_{0}\Theta_{1}^{2})_{\Psi\Psi\Psi},\\ &\vdots\\ &H^{2}_{0}(\Theta) = (\Theta_{0}^{3})_{\Psi},\\ &H^{2}_{1}(\Theta) = (3\Theta_{0}^{2}\Theta_{1})_{\Psi},\\ &H^{2}_{2}(\Theta) = (3\Theta_{2}\Theta_{0}^{2}+3\Theta_{0}\Theta_{1}^{2})_{\Psi}. \end{split} \end{equation*}$

On comparing the $\epsilon$ coefficients, we conclude

$\begin{equation*} \ \begin{split} &\epsilon^{0}:\Theta_{0}(\Psi,\mathcal{T}) = \sqrt{\frac{81\rho-1}{54}}\cosh\Bigg(\frac{\Psi}{3}\Bigg),\\ &\epsilon^{1}:\Theta_{1}(\Psi,\mathcal{T}) = -\sqrt{\frac{81\rho-1}{54}}\frac{\rho}{3}\sinh\Bigg(\frac{\Psi}{3}\Bigg)\frac{\mathcal{T}^{\wp}}{\Gamma(\wp+1)},\\ &\epsilon^{2}:\Theta_{2}(\Psi,\mathcal{T}) = \sqrt{\frac{81\rho-1}{54}}\frac{\rho^{2}}{3^{2}}\cosh\Bigg(\frac{\Psi}{3}\Bigg)\frac{\mathcal{T}^{2\wp}}{\Gamma(2\wp+1)},\\ &\epsilon^{3}:\Theta_{3}(\Psi,\mathcal{T}) = -\sqrt{\frac{81\rho-1}{54}}\frac{\rho^{3}}{3^{3}}\sinh\Bigg(\frac{\Psi}{3}\Bigg)\frac{\mathcal{T}^{3\wp}}{\Gamma(3\wp+1)},\\ &\vdots \end{split} \end{equation*}$

Finally, the analytical solution is given by

$\begin{equation*} \begin{split} \Theta({\Psi},{\mathcal{T}}) = &{\Theta}_{0}({\Psi},{\mathcal{T}})+{\Theta}_{1}({\Psi},{\mathcal{T}})+{\Theta}_{2}({\Psi},{\mathcal{T}})+{\Theta}_{3}({\Psi},{\mathcal{T}})+\cdots,\\ \Theta({\Psi},{\mathcal{T}}) = &\sqrt{\frac{81\rho-1}{54}}\cosh\Bigg(\frac{\Psi}{3}\Bigg)-\sqrt{\frac{81\rho-1}{54}}\frac{\rho}{3}\sinh\Bigg(\frac{\Psi}{3}\Bigg)\frac{\mathcal{T}^{\wp}}{\Gamma(\wp+1)}+\sqrt{\frac{81\rho-1}{54}}\frac{\rho^{2}}{3^{2}}\cosh\Bigg(\frac{\Psi}{3}\Bigg)\frac{\mathcal{T}^{2\wp}}{\Gamma(2\wp+1)}\\ &-\sqrt{\frac{81\rho-1}{54}}\frac{\rho^{3}}{3^{3}}\sinh\Bigg(\frac{\Psi}{3}\Bigg)\frac{\mathcal{T}^{3\wp}}{\Gamma(3\wp+1)}+\cdots . \end{split} \end{equation*}$

Solution by implementing YTDM

By plugging in the YT, we obtain

$\begin{align} Y\left\{\frac{\partial^{\wp}\Theta}{\partial \mathcal{T}^{\wp}}\right\} = Y\Bigg((\Theta^{3}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi}-(\Theta^{3}(\Psi,\mathcal{T}))_{\Psi}-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg).\\ \end{align}$

(5.22)

We have by simplifying

$\begin{align} \frac{1}{u^{\wp}}\{M(u)- u \Theta(0)\} = Y\Bigg((\Theta^{3}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi}-(\Theta^{3}(\Psi,\mathcal{T}))_{\Psi}-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg), \end{align}$

(5.23)

$\begin{align} M(u) = u \Theta(0)+u^{\wp}Y\Bigg((\Theta^{3}(\Psi,\mathcal{T}))_{\Psi\Psi\Psi}-(\Theta^{3}(\Psi,\mathcal{T}))_{\Psi}-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg). \end{align}$

(5.24)

By employing inverse YT, we get

(5.25)

Now the solution in terms of infinite series is

$\begin{align} \Theta(\Psi,\mathcal{T}) = \sum\limits_{m = 0}^{\infty}\Theta_m(\Psi,\mathcal{T}). \end{align}$

(5.26)

Also, the nonlinear term is illustrated as $(\Theta^{3}(\Psi, \mathcal{T}))_{\Psi} = \sum_{m = 0}^{\infty}\mathcal{A}_m, (\Theta^{3}(\Psi, \mathcal{T}))_{\Psi} = \sum_{m = 0}^{\infty}\mathcal{B}_m.$ So, we get

$\begin{align} \begin{split} &\sum\limits_{m = 0}^{\infty}\Theta_m(\Psi,\mathcal{T}) = \Theta(\Psi,0) +Y^{-1}\Bigg[u^\wp Y\Bigg[\Bigg(\sum\limits_{m = 0}^{\infty}\mathcal{A}_m\Bigg)-\Bigg(\sum\limits_{m = 0}^{\infty}\mathcal{B}_m\Bigg)-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg]\Bigg],\\ &\sum\limits_{m = 0}^{\infty}\Theta_m(\Psi,\mathcal{T}) = \sqrt{\frac{81\rho-1}{54}}\cosh\Bigg(\frac{\Psi}{3}\Bigg) +Y^{-1}\Bigg[u^\wp Y\Bigg[\Bigg(\sum\limits_{m = 0}^{\infty}\mathcal{A}_m\Bigg)-\Bigg(\sum\limits_{m = 0}^{\infty}\mathcal{B}_m\Bigg)-(\Theta(\Psi,\mathcal{T}))_{\Psi\Psi\Psi\Psi\Psi}\Bigg]\Bigg]. \end{split} \end{align}$

(5.27)

The first components are illustrated as

$\begin{equation*} \begin{split} &\mathcal{A}_0 = (\Theta_{0}^{3})_{\Psi\Psi\Psi},\\ &\mathcal{A}_1 = (3\Theta_{0}^{2}\Theta_{1})_{\Psi\Psi\Psi},\\ &\mathcal{A}_2 = (3\Theta_{2}\Theta_{0}^{2}+3\Theta_{0}\Theta_{1}^{2})_{\Psi\Psi\Psi},\\ &\vdots\\ &\mathcal{B}_0 = (\Theta_{0}^{3})_{\Psi},\\ &\mathcal{B}_1 = (3\Theta_{0}^{2}\Theta_{1})_{\Psi},\\ &\mathcal{B}_2 = (3\Theta_{2}\Theta_{0}^{2}+3\Theta_{0}\Theta_{1}^{2})_{\Psi}.\\ \end{split} \end{equation*}$

On comparing both sides, we conclude

$\begin{equation*} \Theta_0(\Psi,\mathcal{T}) = \sqrt{\frac{81\rho-1}{54}}\cosh\Bigg(\frac{\Psi}{3}\Bigg). \end{equation*}$

On $m = 0$ ,

$\begin{equation*} \Theta_1(\Psi,\mathcal{T}) = -\sqrt{\frac{81\rho-1}{54}}\frac{\rho}{3}\sinh\Bigg(\frac{\Psi}{3}\Bigg)\frac{\mathcal{T}^{\wp}}{\Gamma(\wp+1)}. \end{equation*}$

On $m = 1$ ,

$\begin{equation*} \Theta_2(\Psi,\mathcal{T}) = \sqrt{\frac{81\rho-1}{54}}\frac{\rho^{2}}{3^{2}}\cosh\Bigg(\frac{\Psi}{3}\Bigg)\frac{\mathcal{T}^{2\wp}}{\Gamma(2\wp+1)}. \end{equation*}$

On $m = 2$ ,

$\begin{equation*} \Theta_3(\Psi,\mathcal{T}) = -\sqrt{\frac{81\rho-1}{54}}\frac{\rho^{3}}{3^{3}}\sinh\Bigg(\frac{\Psi}{3}\Bigg)\frac{\mathcal{T}^{3\wp}}{\Gamma(3\wp+1)}. \end{equation*}$

Finally, the analytical solution is given by

$\begin{equation*} \begin{split} \Theta(\Psi,\mathcal{T}) = &\sqrt{\frac{81\rho-1}{54}}\cosh\Bigg(\frac{\Psi}{3}\Bigg)-\sqrt{\frac{81\rho-1}{54}}\frac{\rho}{3}\sinh\Bigg(\frac{\Psi}{3}\Bigg)\frac{\mathcal{T}^{\wp}}{\Gamma(\wp+1)}\\ &+\sqrt{\frac{81\rho-1}{54}}\frac{\rho^{2}}{3^{2}}\cosh\Bigg(\frac{\Psi}{3}\Bigg)\frac{\mathcal{T}^{2\wp}}{\Gamma(2\wp+1)}-\sqrt{\frac{81\rho-1}{54}}\frac{\rho^{3}}{3^{3}}\sinh\Bigg(\frac{\Psi}{3}\Bigg)\frac{\mathcal{T}^{3\wp}}{\Gamma(3\wp+1)}+\cdots. \end{split} \end{equation*}$

Taking $\wp = 1$ , we get

$\begin{align} \ \Theta({\Psi},{\mathcal{T}}) = \sqrt{\frac{81\rho-1}{54}}\cosh\Bigg(\frac{\rho\mathcal{T}-\Psi}{3}\Bigg). \end{align}$

(5.28)

6. Physical interpretation of results

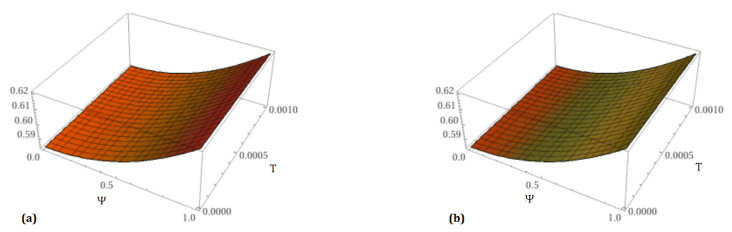
This section's graphical and numerical analysis provides important insights into the behaviour and precision of our suggested non-singular kernel operators and natural transform solution approach for the time-fractional K(m, n, 1) equations for a range of fractional parameter $\wp$ values. We examined two nonlinear problems to demonstrate that the suggested technique can handle challenging nonlinear problems. These remarkably positive results demonstrate the efficacy of the strategy being discussed. The surface in shows the exact solution and shows the approximated solution of the proposed techniques at $\wp = 1$ . The surface in shows the approximated solution of the proposed techniques at $\wp = 0.8$ and shows the approximated solution of the proposed techniques at $\wp = 0.9$ . illustrates the nature of the proposed techniques solution in terms of absolute error and illustrates the 2D nature of the proposed techniques solution at distinct values of fractional order $\wp = 0.7, 0.8, 0.9, 1$ . presents the accurate solution and computed approximate solution generated from the 4th-order series solution obtained by using the proposed techniques at different orders of $\wp$ .

Figure 1. The plot (a) showing the precise solution and (b) showing behavior of the offered techniques at

$\wp = 1$ .

DownLoad: Full-Size Img PowerPoint

Figure 2. The plot (a) showing behavior of the offered techniques solution at

$\wp = 0.8$ and (b) showing behavior of the offered techniques solution at

$\wp = 0.9$ .

DownLoad: Full-Size Img PowerPoint

Figure 3. The plot (a) showing the absolute error and (b) showing the behavior of offered techniques solution at

$\wp = 0.7, 0.8, 0.9, 1$ .

DownLoad: Full-Size Img PowerPoint

Table 1. Analysis of the accurate solution and our approach's solution at numerous

$\wp$ orders.

${\Psi}$	${\mathcal{T}}$	$\wp=0.85$	$\wp=0.90$	$\wp=0.95$	$\wp=1(appro)$	$\wp=1(exact)$
	0.2	0.58477120	0.58477777	0.58478237	0.58478558	0.58478558
	0.4	0.58914247	0.58915572	0.58916499	0.58917146	0.58917146
0.001	0.6	0.59649098	0.59651104	0.59652507	0.59653487	0.59653487
	0.8	0.60689027	0.60691735	0.60693628	0.60694950	0.60694950
	1	0.62044443	0.62047879	0.62050282	0.62051958	0.62051958
	0.2	0.58475394	0.58476475	0.58477261	0.58477831	0.58477831
	0.4	0.58910757	0.58912945	0.58914532	0.58915681	0.58915681
0.002	0.6	0.59643809	0.59647126	0.59649530	0.59651269	0.59651269
	0.8	0.60681886	0.60686365	0.60689611	0.60691957	0.60691957
	1	0.62035378	0.62041064	0.62045184	0.62048162	0.62048162
	0.2	0.58473810	0.58475246	0.58476314	0.58477105	0.58477105
	0.4	0.58907543	0.58910459	0.58912620	0.58914217	0.58914217
0.003	0.6	0.59638932	0.59643357	0.59646634	0.59649053	0.59649053
	0.8	0.60675298	0.60681276	0.60685701	0.60688967	0.60688967
	1	0.62027013	0.62034604	0.62040221	0.62044367	0.62044367
	0.2	0.58472314	0.58474064	0.58475386	0.58476381	0.58476381
	0.4	0.58904498	0.58908061	0.58910743	0.58912756	0.58912756
0.004	0.6	0.59634308	0.59639720	0.59643789	0.59646840	0.59646840
	0.8	0.60669048	0.60676364	0.60681860	0.60685979	0.60685979
	1	0.62019074	0.62028367	0.62035346	0.62040575	0.62040575
	0.2	0.58470883	0.58472917	0.58474473	0.58475659	0.58475659
	0.4	0.58901575	0.58905729	0.58908893	0.58911296	0.58911296
0.005	0.6	0.59629864	0.59636180	0.59640984	0.59644628	0.59644628
	0.8	0.60663040	0.60671579	0.60678071	0.60682993	0.60682993
	1	0.62011441	0.62022291	0.62030535	0.62036784	0.62036784

| Show Table

DownLoad: CSV

The surface in shows the exact and shows the approximated solution of the proposed techniques at $\wp = 1$ . The surface in shows the approximated solution of the proposed techniques at $\wp = 0.8$ and shows the approximated solution of the proposed techniques at $\wp = 0.9$ . illustrates the nature of the proposed techniques solution in terms of absolute error and illustrates the 2D nature of the proposed techniques solution at distinct values of fractional order $\wp = 0.7, 0.8, 0.9, 1$ . presents the accurate solution and computed approximate solution generated from the 4th-order series solution obtained by using the proposed techniques at different orders of $\wp$ . The accuracy of the findings is ensured by the numerical simulations that are provided. In comparison to the exact solution, tables yield much better outcomes. Finally, we believe that the methods we propose are very dependable and applicable to multidisciplinary study classifications including fractional-order nonlinear scientific techniques, which enhances our comprehension of nonlinear compound phenomena in related disciplines of science and innovation.

Figure 4. The plot (a) showing the precise solution and (b) showing behavior of the offered techniques at

$\wp = 1$ .

DownLoad: Full-Size Img PowerPoint

Figure 5. The plot (a) showing behavior of the offered techniques solution at

$\wp = 0.8$ and (b) showing behavior of the offered techniques solution at

$\wp = 0.9$ .

DownLoad: Full-Size Img PowerPoint

Figure 6. The plot (a) showing the absolute error and (b) showing the behavior of offered techniques solution at

$\wp = 0.7, 0.8, 0.9, 1$ .

DownLoad: Full-Size Img PowerPoint

Table 2. Analysis of the accurate solution and our approach's solution at numerous

$\wp$ orders.

${\Psi}$	${\mathcal{T}}$	$\wp=0.85$	$\wp=0.90$	$\wp=0.95$	$\wp=1(appro)$	$\wp=1(exact)$
	0.2	0.85571760	0.85619944	0.85667967	0.85715869	0.85715870
	0.4	0.85997291	0.86093872	0.86190132	0.86286148	0.86286149
0.001	0.6	0.86805173	0.86950582	0.87095506	0.87240063	0.87240065
	0.8	0.87998998	0.88193881	0.88388115	0.88581856	0.88581857
	1	0.89584075	0.89829298	0.90073705	0.90317492	0.90317493
	0.2	0.85569827	0.85618406	0.85666754	0.85714918	0.85714923
	0.4	0.85993415	0.86090790	0.86187700	0.86284242	0.86284247
0.002	0.6	0.86799338	0.86945941	0.87091844	0.87237194	0.87237198
	0.8	0.87991178	0.88187661	0.88383206	0.88578010	0.88578015
	1	0.89574235	0.89821472	0.90067529	0.90312652	0.90312657
	0.2	0.85568040	0.85616947	0.85665572	0.85713967	0.85713978
	0.4	0.85989833	0.86087865	0.86185330	0.86282336	0.86282346
0.003	0.6	0.86793945	0.86941537	0.87088276	0.87234324	0.87234334
	0.8	0.87983951	0.88181759	0.88378425	0.88574163	0.88574174
	1	0.89565141	0.89814045	0.90061512	0.90307812	0.90307823
	0.2	0.85566342	0.85615537	0.85664409	0.85713016	0.85713035
	0.4	0.85986430	0.86085038	0.86183000	0.86280429	0.86280449
0.004	0.6	0.86788821	0.86937281	0.87084768	0.87231454	0.87231473
	0.8	0.87977083	0.88176054	0.88373723	0.88570317	0.88570336
	1	0.89556499	0.89806867	0.90055596	0.90302972	0.90302992
	0.2	0.85564707	0.85614161	0.85663261	0.85712065	0.85712095
	0.4	0.85983153	0.86082281	0.86180700	0.86278523	0.86278553
0.005	0.6	0.86783887	0.86933130	0.87081305	0.87228584	0.87228614
	0.8	0.87970470	0.88170492	0.88369081	0.88566470	0.88566501
	1	0.89548178	0.89799868	0.90049755	0.90298132	0.90298163

| Show Table

DownLoad: CSV

7. Conclusions

Many systems and equations in several branches of research, including mathematics, physics, engineering statics, etc., require solutions. Numerous numerical and analytical techniques were developed by mathematicians in order to determine the solution exactly or approximately. In this paper, we have successfully applied two unique methods to solve time fractional non-linear dispersive K(m, n, 1)-type equations analytically. The method uses the recurrence relations of the suggested methods to provide a number of successive approximations. We have solved two cases and examined our findings with the precise solution of the problem in order to demonstrate the precision and effectiveness of our methodology. The generated results and the actual solution to the issue are very similar, as shown by the solution graphs and tables. The solutions obtained at each fractional order are found to converge to the problems integer orders. We consequently come to the conclusion that the presented approaches are significant non-sophisticated effective tools that generate good quality approximations for nonlinear partial differential equations using straightforward computations and that achieve convergence with a minimal number of terms. Thus, the expansion will be significantly valued to add other operators and approaches in the future, especially in light of the advantages of the current operator. The offered strategies were determined to be suitable to address any physical problem that arises in engineering and the sciences because of their straightforward operation.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

The author Fatemah Mofarreh expresses her gratitude to the Princess Nourah bint Abdulrahman University Researcher Support Project (number PNURSP2023R27), Princess Nourah bint Abdulrahman University, Riyadh, Saudi Arabia.

Conflict of interest

The authors declare that there are no conflicts of interest regarding the publication of this article.

References

[1]	M. Yavuz, T. A. Sulaiman, F. Usta, H. Bulut, Analysis and numerical computations of the fractional regularized long-wave equation with damping term, Math. Methods Appl. Sci., 44 (2021), 7538–7555. https://doi.org/10.1002/mma.6343 doi: 10.1002/mma.6343
[2]	S. Kumar, S. Ghosh, B. Samet, E. F. Doungmo Goufo, An analysis for heat equations arises in diffusion process using new Yang-Abdel-Aty-Cattani fractional operator, Math. Methods Appl. Sci., 43 (2020), 6062–6080. https://doi.org/10.1002/mma.6347 doi: 10.1002/mma.6347
[3]	E. F. Doungmo Goufo, S. Kumar, Shallow water wave models with and without singular kernel: existence, uniqueness, and similarities, Math. Probl. Eng., 2017 (2017), 1–9. https://doi.org/10.1155/2017/4609834 doi: 10.1155/2017/4609834
[4]	M. Yavuz, Characterizations of two different fractional operators without singular kernel, Math. Model. Nat. Phenom., 14 (2019), 302. https://doi.org/10.1051/mmnp/2018070 doi: 10.1051/mmnp/2018070
[5]	E. F. Doungmo Goufo, S. Kumar, S. B. Mugisha, Similarities in a fifth-order evolution equation with and with no singular kernel, Chaos Solitons Fract., 130 (2020), 109467. https://doi.org/10.1016/j.chaos.2019.109467 doi: 10.1016/j.chaos.2019.109467
[6]	R. Subashini, K. Jothimani, K. S. Nisar, C. Ravichandran, New results on nonlocal functional integro-differential equations via Hilfer fractional derivative, Alex. Eng. J., 59 (2020), 2891–2899. https://doi.org/10.1016/j.aej.2020.01.055 doi: 10.1016/j.aej.2020.01.055
[7]	H. Bulut, H. M. Baskonus, Y. Pandir, The modified trial equation method for fractional wave equation and time fractional generalized Burgers equation, Abstr. Appl. Anal., 2013 (2013), 1–8. https://doi.org/10.1155/2013/636802 doi: 10.1155/2013/636802
[8]	J. H. He, Approximate analytical solution for seepage flow with fractional derivatives in porous media, Comput. Methods Appl. Mech. Eng., 167 (1998), 57–68. https://doi.org/10.1016/s0045-7825(98)00108-x doi: 10.1016/s0045-7825(98)00108-x
[9]	V. P. Dubey, R. Kumar, D. Kumar, A hybrid analytical scheme for the numerical computation of time fractional computer virus propagation model and its stability analysis, Chaos Solitons Fract., 133 (2020), 109626. https://doi.org/10.1016/j.chaos.2020.109626 doi: 10.1016/j.chaos.2020.109626
[10]	S. Kumar, Y. Khan, A. Yildirim, A mathematical modeling arising in the chemical systems and its approximate numerical solution, Asia Pac. J. Chem. Eng., 7 (2012), 835–840. https://doi.org/10.1002/apj.647 doi: 10.1002/apj.647
[11]	J. Singh, D. Kumar, S. D. Purohit, A. M. Mishra, M. Bohra, An efficient numerical approach for fractional multidimensional diffusion equations with exponential memory, Numer. Methods Partial Differ. Equ., 37 (2021), 1631–1651. https://doi.org/10.1002/num.22601 doi: 10.1002/num.22601
[12]	M. Caputo, Linear models of dissipation whose Q is almost frequency independent–Ⅱ, Geophys. J. Int., 13 (1967), 529–539. https://doi.org/10.1111/j.1365-246x.1967.tb02303.x doi: 10.1111/j.1365-246x.1967.tb02303.x
[13]	Z. Li, C. Huang, B. J. Wang, Phase portrait, bifurcation, chaotic pattern and optical soliton solutions of the Fokas-Lenells equation with cubic-quartic dispersion in optical fibers, Phys. Lett. A, 465 (2023), 128714. https://doi.org/10.1016/j.physleta.2023.128714 doi: 10.1016/j.physleta.2023.128714
[14]	M. S. Ullah, Interaction solution to the (3+1)-D negative-order KdV first structure, Partial Differ. Equ. Appl. Math., 8 (2023), 100566. https://doi.org/10.1016/j.padiff.2023.100566 doi: 10.1016/j.padiff.2023.100566
[15]	A. L. Hodgkin, A. F. Huxley, A quantitative description of membrane current and its application to conduction and excitation in nerve, J. Physiol., 117 (1952), 500–544. https://doi.org/10.1113/jphysiol.1952.sp004764 doi: 10.1113/jphysiol.1952.sp004764
[16]	M. S. Ullah, D. Baleanu, M. Z. Ali, H. O. Roshid, Novel dynamics of the Zoomeron model via different analytical methods, Chaos Solitons Fract., 174 (2023), 113856. https://doi.org/10.1016/j.chaos.2023.113856 doi: 10.1016/j.chaos.2023.113856
[17]	M. H. Protter, H. F. Weinberger, Maximum principles in differential equations, New York: Springer, 1984. https://doi.org/10.1007/978-1-4612-5282-5
[18]	M. Schatzman, J. Taylor, Numerical analysis: a mathematical introduction, Oxford University Press, 2002. https://doi.org/10.1093/oso/9780198502791.001.0001
[19]	J. W. Thomas, Numerical partial differential equations: finite difference methods, New York: Springer, 1995. https://doi.org/10.1007/978-1-4899-7278-1
[20]	K. George, E. H. Twizell, Stable second-order finite-difference methods for linear initial-boundary-value problems, Appl. Math. Lett., 19 (2006), 146–154. https://doi.org/10.1016/j.aml.2005.04.003 doi: 10.1016/j.aml.2005.04.003
[21]	K. Nonlaopon, A. M. Alsharif, A. M. Zidan, A. Khan, Y. S. Hamed, R. Shah, Numerical investigation of fractional-order Swift-Hohenberg equations via a novel transform, Symmetry, 13 (2021), 1–21. https://doi.org/10.3390/sym13071263 doi: 10.3390/sym13071263
[22]	J. G. Liu, X. J. Yang, Symmetry group analysis of several coupled fractional partial differential equations, Chaos Solitons Fract., 173 (2023), 113603. https://doi.org/10.1016/j.chaos.2023.113603 doi: 10.1016/j.chaos.2023.113603
[23]	A. H. Ganie, M. M. AlBaidani, A. Khan, A comparative study of the fractional partial differential equations via novel transform, Symmetry, 15 (2023), 1–21. https://doi.org/10.3390/sym15051101 doi: 10.3390/sym15051101
[24]	J. G. Liu, Y. F. Zhang, J. J. Wang, Investigation of the time fractional generalized (2 + 1)-dimensional Zakharov-Kuznetsov equation with single-power law nonlinearity, Fractals, 31 (2023), 2350033. https://doi.org/10.1142/s0218348x23500330 doi: 10.1142/s0218348x23500330
[25]	A. H. Ganie, F. Mofarreh, A. Khan, A fractional analysis of Zakharov-Kuznetsov equations with the Liouville-Caputo operator, Axioms, 12 (2023), 1–18. https://doi.org/10.3390/axioms12060609 doi: 10.3390/axioms12060609
[26]	M. M. AlBaidani, A. H. Ganie, F. Aljuaydi, A. Khan, Application of analytical techniques for solving fractional physical models arising in applied sciences, Fractal Fract., 7 (2023), 1–19. https://doi.org/10.3390/fractalfract7080584 doi: 10.3390/fractalfract7080584
[27]	J. G. Liu, X. J. Yang, L. L. Geng, X. J. Yu, On fractional symmetry group scheme to the higher-dimensional space and time fractional dissipative Burgers equation, Int. J. Geom. Methods Modern Phys., 19 (2022), 2250173. https://doi.org/10.1142/s0219887822501730 doi: 10.1142/s0219887822501730
[28]	J. Azevedo, C. Cuevas, E. Henriquez, Existence and asymptotic behaviour for the time-fractional Keller-Segel model for chemotaxis, Math. Nachr., 292 (2019), 462–480. https://doi.org/10.1002/mana.201700237 doi: 10.1002/mana.201700237
[29]	C. Peng, Z. Li, Soliton solutions and dynamics analysis of fractional Radhakrishnan-Kundu-Lakshmanan equation with multiplicative noise in the Stratonovich sense, Results Phys., 53 (2023), 106985. https://doi.org/10.1016/j.rinp.2023.106985 doi: 10.1016/j.rinp.2023.106985
[30]	N. A. Zabidi, Z. A. Majid, A. Kilicman, F. Rabiei, Numerical solutions of fractional differential equations by using fractional explicit Adams method, Mathematics, 8 (2020), 1–23. https://doi.org/10.3390/math8101675 doi: 10.3390/math8101675
[31]	J. S. Duan, T. Chaolu, R. Rach, Solutions of the initial value problem for nonlinear fractional ordinary differential equations by the Rach-Adomian-Meyers modified decomposition method, Appl. Math. Comput., 218 (2012), 8370–8392. https://doi.org/10.1016/j.amc.2012.01.063 doi: 10.1016/j.amc.2012.01.063
[32]	T. Botmart, R. P. Agarwal, M. Naeem, A. Khan, R. Shah, On the solution of fractional modified Boussinesq and approximate long wave equations with non-singular kernel operators, AIMS Math., 7 (2022), 12483–12513. https://doi.org/10.3934/math.2022693 doi: 10.3934/math.2022693
[33]	N. A. Shah, Y. S. Hamed, K. M. Abualnaja, J. D. Chung, R. Shah, A. Khan, A comparative analysis of fractional-order Kaup-Kupershmidt equation within different operators, Symmetry, 14 (2022), 1–23. https://doi.org/10.3390/sym14050986 doi: 10.3390/sym14050986
[34]	D. Fathima, R. A. Alahmadi, A. Khan, A. Akhter, A. H. Ganie, An efficient analytical approach to investigate fractional Caudrey-Dodd-gibbon equations with non-singular kernel derivatives, Symmetry, 15 (2023), 1–18. https://doi.org/10.3390/sym15040850 doi: 10.3390/sym15040850
[35]	H. Jafari, H. Tajadodi, D. Baleanu, A. Al-Zahrani, Y. Alhamed, A. Zahid, Fractional sub-equation method for the fractional generalized reaction Duffing model and nonlinear fractional Sharma-Tasso-Olver equation, Open Phys., 11 (2013), 1482–1486. https://doi.org/10.2478/s11534-013-0203-7 doi: 10.2478/s11534-013-0203-7
[36]	N. K. Mishra, M. M. AlBaidani, A. Khan, A. H. Ganie, Two novel computational techniques for solving nonlinear time-fractional Lax's Korteweg-de Vries equation, Axioms, 12 (2023), 1–13. https://doi.org/10.3390/axioms12040400 doi: 10.3390/axioms12040400
[37]	A. M. Wazwaz, The tanh method: exact solutions of the sine-Gordon and the sinh-Gordon equations, Appl. Math. Comput., 167 (2005), 1196–1210. https://doi.org/10.1016/j.amc.2004.08.005 doi: 10.1016/j.amc.2004.08.005
[38]	N. K. Mishra, M. M. AlBaidani, A. Khan, A. H. Ganie, Numerical investigation of time-fractional Phi-four equation via novel transform, Symmetry, 15 (2023), 1–18. https://doi.org/10.3390/sym15030687 doi: 10.3390/sym15030687
[39]	W. H. Deng, Finite element method for the space and time fractional Fokker-Planck equation, SIAM J. Numer. Anal., 47 (2009), 204–226. https://doi.org/10.1137/080714130 doi: 10.1137/080714130
[40]	M. K. Alaoui, R. Fayyaz, A. Khan, R. Shah, M. S. Abdo, Analytical investigation of Noyes-Field model for time-fractional Belousov-Zhabotinsky reaction, Complexity, 2021 (2021), 1–21. https://doi.org/10.1155/2021/3248376 doi: 10.1155/2021/3248376
[41]	A. M. Wazwaz, New solitary-wave special solutions with compact support for the nonlinear dispersive K(m, n) equations, Chaos Solitons Fract., 13 (2002), 321–330. https://doi.org/10.1016/s0960-0779(00)00249-6 doi: 10.1016/s0960-0779(00)00249-6
[42]	J. H. He, X. H. Wu, Exp-function method for nonlinear wave equations, Chaos Solitons Fract., 30 (2006), 700–708. https://doi.org/10.1016/j.chaos.2006.03.020 doi: 10.1016/j.chaos.2006.03.020
[43]	J. H. He, X. H. Wu, Construction of solitary solution and compacton-like solution by variational iteration method, Chaos Solitons Fract., 29 (2006), 108–113. https://doi.org/10.1016/j.chaos.2005.10.100 doi: 10.1016/j.chaos.2005.10.100
[44]	J. H. He, Some asymptotic methods for strongly nonlinear equations, Int. J. Modern Phys. B, 20 (2006), 1141–1199. https://doi.org/10.1142/s0217979206033796 doi: 10.1142/s0217979206033796
[45]	J. H. He, Application of homotopy perturbation method to nonlinear wave equations, Chaos Solitons Fract., 26 (2005), 695–700. https://doi.org/10.1016/j.chaos.2005.03.006 doi: 10.1016/j.chaos.2005.03.006
[46]	M. Inc, New exact solitary pattern solutions of the nonlinearly dispersive R(m, n) equations, Chaos Solitons Fract., 29 (2006), 499–505. https://doi.org/10.1016/j.chaos.2005.08.051 doi: 10.1016/j.chaos.2005.08.051
[47]	J. H. He, String theory in a scale dependent discontinuous space-time, Chaos Solitons Fract., 36 (2008), 542–545. https://doi.org/10.1016/j.chaos.2007.07.093 doi: 10.1016/j.chaos.2007.07.093
[48]	I. Podlubny, M. Kacenak, Isoclinal matrices and numerical solution of fractional differential equations, In: 2001 European Control Conference (ECC), Portugal: Porto, 2001, 1467–1470. https://doi.org/10.23919/ecc.2001.7076125
[49]	X. J. Yang, D. Baleanu, H. M. Srivastava, Local fractional Laplace transform and applications, In: Local fractional integral transforms and their applications, Cambridge, MA, USA: Academic Press, 2016,147–178. https://doi.org/10.1016/b978-0-12-804002-7.00004-8

This article has been cited by:

1.	Abdul Hamid Ganie, Adnan Khan, Ghaliah Alhamzi, Abdulkafi Mohammed Saeed, Mdi begum Jeelani, A new solution of the nonlinear fractional logistic differential equations utilizing efficient techniques, 2024, 14, 2158-3226, 10.1063/5.0197704
2.	Abdul Hamid Ganie, Fatemah Mofarreh, Adnan Khan, On new computations of the time-fractional nonlinear KdV-Burgers equation with exponential memory, 2024, 99, 0031-8949, 045217, 10.1088/1402-4896/ad2e60
3.	Muath Awadalla, Abdul Hamid Ganie, Dowlath Fathima, Adnan Khan, Jihan Alahmadi, A mathematical fractional model of waves on Shallow water surfaces: The Korteweg-de Vries equation, 2024, 9, 2473-6988, 10561, 10.3934/math.2024516
4.	Mashael M. AlBaidani, Fahad Aljuaydi, Shahad Abdullah F. Alsubaie, Abdul Hamid Ganie, Adnan Khan, Computational and Numerical Analysis of the Caputo-Type Fractional Nonlinear Dynamical Systems via Novel Transform, 2024, 8, 2504-3110, 708, 10.3390/fractalfract8120708
5.	Abdul Hamid Ganie, N. S. Alharthi, Adnan Khan, Abdulkafi Mohammed Saeed, Mohd Asif Shah, Saurav Mallik, The series solutions of fractional foam drainage and fractional modified regularized long wave problems, 2024, 2024, 1029-242X, 10.1186/s13660-024-03227-w
6.	Abdul Hamid Ganie, Adnan Khan, N. S. Alharthi, Fikadu Tesgera Tolasa, Mdi Begum Jeelani, Nan-Jing Huang, Comparative Analysis of Time‐Fractional Coupled System of Shallow‐Water Equations Including Caputo’s Fractional Derivative, 2024, 2024, 2314-4629, 10.1155/jom/2440359
7.	Abdulrahman B M Alzahrani, Solving the fractional nonlinear dispersive K(m, n, 1) partial differential equation: techniques and applications, 2025, 100, 0031-8949, 035215, 10.1088/1402-4896/ad9ee3
8.	N. S. Alharthi, Behzad Ghanbari, Efficient simulation of plasma physics’ time fractional modified Korteweg-de Vries equations, 2025, 20, 1932-6203, e0316218, 10.1371/journal.pone.0316218
9.	Samir A El-Tantawy, Amnah S Al-Johani, Aljawhara H Almuqrin, Adnan Khan, Lamiaa S El-Sherif, Novel approximations to the fourth-order fractional Cahn–Hillard equations: Application to the Tantawy Technique and other two techniques with Yang transform, 2025, 1461-3484, 10.1177/14613484251322240

Reader Comments

Your name:*

Email:*
© 2024 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

AIMS Mathematics

1.8 3.4

Metrics

Article views(1326) PDF downloads(50) Cited by(9)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

Figures and Tables

Figures(6) / Tables(2)

AIMS Mathematics

A novel analysis of the time-fractional nonlinear dispersive K(m, n, 1) equations using the homotopy perturbation transform method and Yang transform decomposition method

Related Papers:

Abstract

Nomenclature

1. Introduction

2. Preleminaries

3. Basic procedure of HPTM

4. Basic procedure of YTDM

5. Applications

6. Physical interpretation of results

7. Conclusions

Use of AI tools declaration

Acknowledgments

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Catalog

AIMS Mathematics

A novel analysis of the time-fractional nonlinear dispersive K(m, n, 1) equations using the homotopy perturbation transform method and Yang transform decomposition method

Related Papers:

Abstract

Nomenclature

1. Introduction

2. Preleminaries

3. Basic procedure of HPTM

4. Basic procedure of YTDM

5. Applications

6. Physical interpretation of results

7. Conclusions

Use of AI tools declaration

Acknowledgments

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog