Evaluation of fractional-order equal width equations with the exponential-decay kernel

Manal Alqhtani; Khaled M. Saad; Rasool Shah; Thongchai Botmart; Waleed M. Hamanah; Manal Alqhtani; Khaled M. Saad; Rasool Shah; Thongchai Botmart; Waleed M. Hamanah

doi:10.3934/math.2022949

AIMS Mathematics

2022, Volume 7, Issue 9: 17236-17251. doi: 10.3934/math.2022949

Previous Article Next Article

Research article Special Issues

Evaluation of fractional-order equal width equations with the exponential-decay kernel

1.
Department of Mathematics, College of Sciences and Arts, Najran University, Najran, Kingdom of Saudi Arabia
2.
Department of Mathematics, Abdul Wali Khan University Mardan Pakistan
3.
Department of Mathematics, Faculty of Science, Khon Kaen University, Khon Kaen, 40002, Thailand
4.
Interdisciplinary Research Center in Renewable Energy and Power Systems, King Fahd University for Petroleum and Minerals, P.O. Box 5028, Dhahran 31261, Saudi Arabia

Received: 09 June 2022 Revised: 06 July 2022 Accepted: 15 July 2022 Published: 22 July 2022
MSC : 34A34, 35A20, 35A22, 44A10, 33B15

In this article we consider the homotopy perturbation transform method to investigate the fractional-order equal-width equations. The homotopy perturbation transform method is a mixture of the homotopy perturbation method and the Yang transform. The fractional-order derivative are defined in the sense of Caputo-Fabrizio operator. Several fractions of solutions are calculated which define some valuable evolution of the given problems. The homotopy perturbation transform method results are compared with actual results and good agreement is found. The suggested method can be used to investigate the fractional perspective analysis of problems in a variety of applied sciences.

Keywords:

Citation: Manal Alqhtani, Khaled M. Saad, Rasool Shah, Thongchai Botmart, Waleed M. Hamanah. Evaluation of fractional-order equal width equations with the exponential-decay kernel[J]. AIMS Mathematics, 2022, 7(9): 17236-17251. doi: 10.3934/math.2022949

Related Papers:

[1]	Rehana Ashraf, Saima Rashid, Fahd Jarad, Ali Althobaiti . Numerical solutions of fuzzy equal width models via generalized fuzzy fractional derivative operators. AIMS Mathematics, 2022, 7(2): 2695-2728. doi: 10.3934/math.2022152
[2]	Naveed Iqbal, Saleh Alshammari, Thongchai Botmart . Evaluation of regularized long-wave equation via Caputo and Caputo-Fabrizio fractional derivatives. AIMS Mathematics, 2022, 7(11): 20401-20419. doi: 10.3934/math.20221118
[3]	Azzh Saad Alshehry, Naila Amir, Naveed Iqbal, Rasool Shah, Kamsing Nonlaopon . On the solution of nonlinear fractional-order shock wave equation via analytical method. AIMS Mathematics, 2022, 7(10): 19325-19343. doi: 10.3934/math.20221061
[4]	Muath Awadalla, Abdul Hamid Ganie, Dowlath Fathima, Adnan Khan, Jihan Alahmadi . A mathematical fractional model of waves on Shallow water surfaces: The Korteweg-de Vries equation. AIMS Mathematics, 2024, 9(5): 10561-10579. doi: 10.3934/math.2024516
[5]	Shabir Ahmad, Aman Ullah, Ali Akgül, Fahd Jarad . A hybrid analytical technique for solving nonlinear fractional order PDEs of power law kernel: Application to KdV and Fornberg-Witham equations. AIMS Mathematics, 2022, 7(5): 9389-9404. doi: 10.3934/math.2022521
[6]	Aslı Alkan, Halil Anaç . A new study on the Newell-Whitehead-Segel equation with Caputo-Fabrizio fractional derivative. AIMS Mathematics, 2024, 9(10): 27979-27997. doi: 10.3934/math.20241358
[7]	Manoj Singh, Ahmed Hussein, Msmali, Mohammad Tamsir, Abdullah Ali H. Ahmadini . An analytical approach of multi-dimensional Navier-Stokes equation in the framework of natural transform. AIMS Mathematics, 2024, 9(4): 8776-8802. doi: 10.3934/math.2024426
[8]	Yousef Jawarneh, Humaira Yasmin, M. Mossa Al-Sawalha, Rasool Shah, Asfandyar Khan . Fractional comparative analysis of Camassa-Holm and Degasperis-Procesi equations. AIMS Mathematics, 2023, 8(11): 25845-25862. doi: 10.3934/math.20231318
[9]	Zeliha Korpinar, Mustafa Inc, Dumitru Baleanu . On the fractional model of Fokker-Planck equations with two different operator. AIMS Mathematics, 2020, 5(1): 236-248. doi: 10.3934/math.2020015
[10]	Asif Khan, Tayyaba Akram, Arshad Khan, Shabir Ahmad, Kamsing Nonlaopon . Investigation of time fractional nonlinear KdV-Burgers equation under fractional operators with nonsingular kernels. AIMS Mathematics, 2023, 8(1): 1251-1268. doi: 10.3934/math.2023063

Abstract

1. Introduction

Many academics have explored fractional evaluation equations over the last century due to their vast relevance in various fields of science and technology. It has been observed that fractional order equations can be used to describe numerous physical systems and to address a variety of problems. Consequently, achieving more productive results in fractional calculus ^{[1,2,3,4,5,6]} is essential to achieving the whole objective. Caputo deemed the fractional Caputo derivative ^[7] to be the many suitable technique for discovering fractional systems because it accurately incorporates beginning conditions that are absent from numerous specific designs ^[8]. Oldham et al. conclude that fractional-order derivative and integral can be utilized to demonstrate significantly more useful synthetic issues than current approaches ^[9]. In addition, the literature contains further agreements on fractional theories and applications, such as fractal mathematics ^{[10,11,12,13]}.

Numerous scholars have worked on partial evaluation equations in recent years due to its extensive applications in numerous scientific and technological fields. These fractional equations are suitable for describing a variety of remarkable phenomena in classical dynamic, acoustics, electrodynamics, material science, plasma physics, electrostatics, viscoelasticity, optoelectronic frameworks, and so on ^[14,15]. The fractional non-linear equal width (EW) equations are extremely important partial differential equations that show various complicated non-linear phenomena in the area of science, solid state physics, particularly plasma waves, astrophysics, materials science, chemical physics, etc. The EW equations characterized the behaviour of nonlinear waves in a variety of nonlinear schemes, including magnetohydrodynamic waves in nanoparticle waves in plasma, surface waves in fluid flow, cold plasma, shallow water waves, acoustic waves in enharmonic crystal, etc ^{[16,17,18,19]}.

He formulated the homotopy perturbation method (HPM) ^[20] in 1999, which is a mixture of the homotopy technique and the classical perturbation method and has been broadly utilized both on linear and non-linear equations ^[21,22]. The significance of the homotopy perturbation method lies in the fact that it does not require a small parameter in the equation, thereby mitigating the disadvantages of conventional perturbation methods. The main aim of this paper is to implement integral transform named "Yang transformation" discovered by Yang ^[23] with homotopy perturbation method to solve nonlinear fractional order partial differential equations. We solve nonlinear EW equations through the homotopy perturbation transform method (HPTM). We obtain a power series solution within the context of a rapidly convergent series, and only a few iterations are required to obtain extremely efficient results. There is no need for discretization or linearization of the nonlinear problem, and only a few iterations are required to arrive at a solution that can be quickly estimated using these methods.

Due to the aforementioned tendency, the fractional-order nonlinear equal width equations are solved utilizing the HPTM. For renewability analytic technique, the Yang transform integrates the HPTM in an efficient manner. Several transforms are combined to form the Yang transform. Both of these technique produce interpretive results in the form of a convergent series. The fractional derivative operator of Caputo-Fabrizio is used to explain quantitative categorizations of nonlinear equal width equations. In modeling and enumeration investigations, the method provided have been proven effective. The exact-analytical findings are a valuable method for analyzing the dynamics of computationally challenging systems, particularly fractional PDEs. Using this approximate expression, financial and monetary phenomena can be examined.

2. Preliminaries concepts

In this part, we address several key ideas, conceptions, and terminologies related to fractional derivative operators involving index and exponential decay as a kernel, as well as the Yang transform specific repercussions.

Definition 2.1. If $\mathbb{P}(\eta) \in \mathbf{H}^{1}[0, T], T > 0$ , then the Caputo-Fabrizio (CF) derivative is defined as follows ^[24]:

$\begin{equation} ^{CF}D_{\eta}^{{\alpha}}[\mathbb{P}({\eta})] = \frac{N({\alpha})}{1-{\alpha}}\int_{0}^{{\eta}}\mathbb{P}^{'}(\varrho)K({\eta}, \varrho)d\varrho, \ \ 0 < \alpha \leq 1. \end{equation}$

(2.1)

$N({\alpha})$ is the normalization term with $N(0) = N(1) = 1$ . However, if $\mathbb{P}(\eta) \notin \mathbf{H}^{1}[0, T]$ , then the above derivative is defined as follows:

$\begin{equation} ^{CF}D_{\eta}^{{\alpha}}[\mathbb{P}({\eta})] = \frac{N({\alpha})}{1-{\alpha}}\int_{0}^{{\eta}}[\mathbb{P}({\eta})-\mathbb{P}(\varrho)]K({\eta}, \varrho)d\varrho. \end{equation}$

(2.2)

Definition 2.2. The fractional CF integral is given as ^[24]

$\begin{equation} ^{CF}I_{\eta}^{{\alpha}}[\mathbb{P}({\eta})] = \frac{1-{\alpha}}{N({\alpha})}\mathbb{P}({\eta})+\frac{{\alpha}}{N({\alpha})}\int_{0}^{{\eta}}\mathbb{P}(\varrho)d\varrho, \ \ {\eta}\geq0, {\alpha}\in(0, 1]. \end{equation}$

(2.3)

Definition 2.3. For $N({\alpha}) = 1$ , shows the Laplace transform of CF derivative is defined as ^[24]:

$\begin{equation} L\left[^{CF}D_{\eta}^{{\alpha}}[\mathbb{P}({\eta})]\right] = \frac{vL[\mathbb{P}({\eta})-\mathbb{P}(0)]}{v+{\alpha}(1-v)}. \end{equation}$

(2.4)

Definition 2.4. The Yang transform of $\mathbb{P}({\eta})$ is given as ^[26]

$\begin{equation} {\mathbb{Y}}\left[\mathbb{P}({\eta})\right] = \chi(v) = \int_{0}^{\infty}\mathbb{P}({\eta})e^{-\frac{\eta}{v}}d{\eta}. \ \ {\eta} > 0, \end{equation}$

(2.5)

Remark. Yang transform of some useful function is define as below.

$\begin{equation} \begin{split} \mathbb{Y}[1] = &v, \\ \mathbb{Y}[{\eta}] = &v^{2}, \\ \mathbb{Y}[\eta^{i}] = &\Gamma(i+1)v^{i+1}. \end{split} \end{equation}$

(2.6)

Lemma 2.5. (Laplace-Yang duality).

Let the Laplace transformation of $\mathbb{P}({\eta})$ is $F(v)$ , then $\chi(v) = F(1/v)$ .

Proof. For proof, see reference ^[25].

Lemma 2.6. Let $\mathbb{P}({\eta})$ is function of continuous; then, the CF derivative of Yang transform of $\mathbb{P}({\eta})$ is defined by ^[25]

$\begin{equation} \mathbb{Y}\left[\mathbb{P}({\eta})\right] = \frac{\mathbb{Y}[\mathbb{P}({\eta})-v\mathbb{P}(0)]}{1+{\alpha}(v-1)}. \end{equation}$

(2.7)

Proof. The fractional CF Laplace transform is expressed as

$\begin{equation} L\left[\mathbb{P}({\eta})\right] = \frac{L[v\mathbb{P}({\eta})-\mathbb{P}(0)]}{v+{\alpha}(1-v)}. \end{equation}$

(2.8)

Also, we have that the connection among Laplace and Yang property, i.e., $\chi(v) = F(1/v)$ . To obtain the desired conclusion, we substitute $v$ with $1/v$ in Eq (2.8), obtaining

$\begin{equation} \begin{split} \mathbb{Y}\left[\mathbb{P}({\eta})\right] = &\frac{\frac{1}{v}\mathbb{Y}[\mathbb{P}({\eta})-\mathbb{P}(0)]}{\frac{1}{v}+{\alpha}(1-\frac{1}{v})}, \\ \mathbb{Y}\left[\mathbb{P}({\eta})\right] = &\frac{\mathbb{Y}[\mathbb{P}({\eta})-v\mathbb{P}(0)]}{1+{\alpha}(v-1)}. \end{split} \end{equation}$

(2.9)

The proof is completed.

3. Methodology of the HPTM

The HPTM method for solving generic nonlinear fractional CF partial differential equations. Take the generic non-linear CF partial differential equations with nonlinear function as an example. $N(\mathbb{V}({\psi}, {\eta}))$ with linear fractional notation $L(\mathbb{V}({\psi}, {\eta}))$ is the same as ^[25]

$\begin{equation} \begin{cases} &^{CF}D_{\eta}^{{\alpha}}{\mathbb{V}}({\varsigma}, {\eta})+L({\mathbb{V}}({{\varsigma}}, {\eta}))+N({\mathbb{V}}({{\varsigma}}, {\eta})) = g({{\varsigma}}, {\eta}), \\ &{\mathbb{V}}({{\varsigma}}, 0) = h({{\varsigma}}), \end{cases} \end{equation}$

(3.1)

the source term is $g({{\varsigma}}, {\eta})$ . Applying Yang transform to Eq (3.1), we get

$\frac{\mathbb{Y}[{\mathbb{V}}({{\varsigma}}, {\eta})-v{\mathbb{V}}({{\varsigma}}, 0)]}{1+{\alpha}(v-1)} = -\mathbb{Y}[L({\mathbb{V}}({{\varsigma}}, {\eta}))+N({\mathbb{V}}({{\varsigma}}, {\eta}))]+\mathbb{Y}[g({{\varsigma}}, {\eta})],$

$\begin{equation} \mathbb{Y}[{\mathbb{V}}({{\varsigma}}, {\eta})] = vh({{\varsigma}})-(1+{\alpha}(v-1))[\mathbb{Y}[L({\mathbb{V}}({{\varsigma}}, {\eta}))+N({\mathbb{V}}({{\varsigma}}, {\eta}))]+\mathbb{Y}[g({{\varsigma}}, {\eta})]. \end{equation}$

(3.2)

Implement inverse Yang transformation, we obtain

$\begin{equation} {\mathbb{V}}({{\varsigma}}, {\eta}) = \mathcal{{\mathbb{V}}}({{\varsigma}}, {0})-\mathbb{Y}^{-1}[(1+{\alpha}(v-1))[\mathbb{Y}[L({\mathbb{V}}({{\varsigma}}, {\eta}))+N({\mathbb{V}}({{\varsigma}}, {\eta}))]+\mathbb{Y}[g({{\varsigma}}, {\eta})]], \end{equation}$

(3.3)

where the source term is ${\mathbb{V}}({{\varsigma}}, {\eta})$ . Now, we use HPTM

$\begin{equation} {\mathbb{V}}({{\varsigma}}, {\eta}) = \sum\limits_{{i} = 0}^{\infty}\rho^{{i}}{\mathbb{V}}_{{i}}({{\varsigma}}, {\eta}). \end{equation}$

(3.4)

We decompose the nonlinear term $N({\mathbb{V}}({{\varsigma}}, {\eta}))$ as

$\begin{equation} N({\mathbb{V}}({{\varsigma}}, {\eta})) = \sum\limits_{{i} = 0}^{\infty}\rho^{{i}}H_{{i}}({\mathbb{V}}), \end{equation}$

(3.5)

where He's polynomial is $H_{{i}}({\mathbb{V}})$ :

$\begin{equation} H_{{i}}({\mathbb{V}}_{1}, {\mathbb{V}}_{2}, {\mathbb{V}}_{3}, \cdots, {\mathbb{V}}_{{i}}) = \frac{1}{\Gamma({i}+1)}\frac{\partial^{{i}}}{\partial \rho^{{i}}}\left[N\left(\sum\limits_{i = 0}^{\infty}\rho^{i}{\mathbb{V}}_{i}\right)\right]_{\rho = 0}, \ \ {i} = 1, 2, 3, \cdots. \end{equation}$

(3.6)

Substituting Eqs (3.4) and (3.5) in Eq (3.3), we obtain

$\begin{equation} \sum\limits_{{i} = 0}^{\infty}\rho^{{i}}{\mathbb{V}}_{{i}}({{\varsigma}}, {\eta}) = \mathcal{{\mathbb{V}}}({{\varsigma}}, {\eta})-\rho\left(\mathbb{Y}^{-1}\left[(1+{\alpha}(v-1))\mathbb{Y}\left[L\sum\limits_{{i} = 0}^{\infty}\rho^{{i}}{\mathbb{V}}_{{i}}({{\varsigma}}, {\eta})+N\sum\limits_{{i} = 0}^{\infty}\rho^{{i}}H_{{i}}({\mathbb{V}})\right]\right]\right). \end{equation}$

(3.7)

We acquire the following terms by comparing coefficients: of $\rho$ in (3.7):

$\begin{equation} \begin{split} \rho^{0}:{\mathbb{V}}_{0}({{\varsigma}}, {\eta}) = &\mathcal{{\mathbb{V}}}({{\varsigma}}, {\eta}), \\ \rho^{1}:{\mathbb{V}}_{1}({{\varsigma}}, {\eta}) = &\mathbb{Y}^{-1}\left[(1+{\alpha}(v-1))\mathbb{Y}\left[L({\mathbb{V}}_{0}({{\varsigma}}, {\eta}))+H_{0}({\mathbb{V}})\right]\right], \\ \rho^{2}:{\mathbb{V}}_{2}({{\varsigma}}, {\eta}) = &\mathbb{Y}^{-1}\left[(1+{\alpha}(v-1))\mathbb{Y}\left[L({\mathbb{V}}_{1}({{\varsigma}}, {\eta}))+H_{1}({\mathbb{V}})\right]\right], \\ \rho^{3}:{\mathbb{V}}_{3}({{\varsigma}}, {\eta}) = &\mathbb{Y}^{-1}\left[(1+{\alpha}(v-1))\mathbb{Y}\left[L({\mathbb{V}}_{2}({{\varsigma}}, {\eta}))+H_{2}({\mathbb{V}})\right]\right], \\ &\vdots\\ \rho^{{i}}:{\mathbb{V}}_{{i}}({{\varsigma}}, {\eta}) = &\mathbb{Y}^{-1}\left[(1+{\alpha}(v-1))\mathbb{Y}\left[L({\mathbb{V}}_{{i}}({{\varsigma}}, {\eta}))+H_{{i}}({\mathbb{V}})\right]\right]. \end{split} \end{equation}$

(3.8)

As a solution, the achieved result of Eq (3.1) is given as:

$\begin{equation} {\mathbb{V}}({{\varsigma}}, {\eta}) = {\mathbb{V}}_{0}({{\varsigma}}, {\eta})+{\mathbb{V}}_{1}({{\varsigma}}, {\eta})+\cdots. \end{equation}$

(3.9)

Convergence and error analysis

The following theorems are crucial to convergence and error analysis method that handle the fundamental frameworks (3.1).

Theorem 3.1. Let ${\mathbb{V}}({{\varsigma}}, {\eta})$ be the exact solution of (3.1) and let ${\mathbb{V}}_{i}({{\varsigma}}, {\eta})\in H$ and $\sigma\in(0, 1)$ , where the Hilbert space show by H. Then, the obtained solution $\sum_{{i} = 0}^{\infty}{\mathbb{V}}_{{i}}({{\varsigma}}, {\eta})$ will convergence ${\mathbb{V}}({{\varsigma}}, {\eta})$ if ${\mathbb{V}}_{{i}}({{\varsigma}}, {\eta})\leq {\mathbb{V}}_{{i}-1}({{\varsigma}}, {\eta}) \ \ \forall {i} > A$ , i.e., for any $\omega > 0\exists A > 0$ , such that $||{\mathbb{V}}_{{i}+n}({{\varsigma}}, {\eta})||\leq\beta, \forall i, n\in N$ ^[25].

Proof. We make a sequence of $\sum_{{i} = 0}^{\infty}{\mathbb{V}}_{{i}}({{\varsigma}}, {\eta}).$

$\begin{equation} \begin{split} C_{0}({{\varsigma}}, {\eta}) = &{\mathbb{V}}_{0}({{\varsigma}}, {\eta}), \\ C_{1}({{\varsigma}}, {\eta}) = &{\mathbb{V}}_{0}({{\varsigma}}, {\eta})+{\mathbb{V}}_{1}({{\varsigma}}, {\eta}), \\ C_{2}({{\varsigma}}, {\eta}) = &{\mathbb{V}}_{0}({{\varsigma}}, {\eta})+{\mathbb{V}}_{1}({{\varsigma}}, {\eta})+{\mathbb{V}}_{2}({{\varsigma}}, {\eta}), \\ C_{3}({{\varsigma}}, {\eta}) = &{\mathbb{V}}_{0}({{\varsigma}}, {\eta})+{\mathbb{V}}_{1}({{\varsigma}}, {\eta})+{\mathbb{V}}_{2}({{\varsigma}}, {\eta})+{\mathbb{V}}_{3}({{\varsigma}}, {\eta}), \\ &\vdots\\ C_{{i}}({{\varsigma}}, {\eta}) = &{\mathbb{V}}_{0}({{\varsigma}}, {\eta})+{\mathbb{V}}_{1}({{\varsigma}}, {\eta})+{\mathbb{V}}_{2}({{\varsigma}}, {\eta})+\cdots+{\mathbb{V}}_{{i}}({{\varsigma}}, {\eta}), \\ \end{split} \end{equation}$

(3.10)

To produce the proper result, we must establish that $C_{{i}}({{\varsigma}}, {\eta})$ constitutes a "Cauchy sequences". Consider, for instance,

$\begin{equation} \begin{split} ||C_{{{i}}+1}({{\varsigma}}, {\eta})-C_{{i}}({{\varsigma}}, {\eta})||& = ||{\mathbb{V}}_{{{i}}+1}({{\varsigma}}, {\eta})||\leq\sigma||{\mathbb{V}}_{{i}}({{\varsigma}}, {\eta})||\leq \sigma^{2}||{\mathbb{V}}_{{i}-1}({{\varsigma}}, {\eta})||\leq\sigma^{3}||{\mathbb{V}}_{{i}-2}({{\varsigma}}, {\eta})||\cdots\\ &\leq\sigma_{{i}+1}||{\mathbb{V}}_{0}({{\varsigma}}, {\eta})||. \end{split} \end{equation}$

(3.11)

For ${i}, n\in N$ , we acquire

$\begin{equation} \begin{split} ||C_{{i}}({{\varsigma}}, {\eta})-C_{n}({{\varsigma}}, {\eta})|| = &||{\mathbb{V}}_{{{i}}+n}({{\varsigma}}, {\eta})|| = ||C_{{i}}({{\varsigma}}, {\eta})-C_{{i}-1}({{\varsigma}}, {\eta})+(C_{{i}-1}({{\varsigma}}, {\eta})-C_{{i}-2}({{\varsigma}}, {\eta}))\\ &+(C_{{i}-2}({{\varsigma}}, {\eta})-C_{{i}-3}({{\varsigma}}, {\eta}))+\cdots+(C_{n+1}({{\varsigma}}, {\eta})-C_{n}({{\varsigma}}, {\eta}))||\\ \leq&||C_{{i}}({{\varsigma}}, {\eta})-C_{{i}-1}({{\varsigma}}, {\eta})||+||(C_{{i}-1}({{\varsigma}}, {\eta})-C_{{i}-2}({{\varsigma}}, {\eta}))||\\ &+||(C_{{i}-2}({{\varsigma}}, {\eta})-C_{{i}-3}({{\varsigma}}, {\eta}))||+\cdots+||(C_{n+1}({{\varsigma}}, {\eta})-C_{n}({{\varsigma}}, {\eta}))||\\ \leq&\sigma^{{i}}||{\mathbb{V}}_{0}({{\varsigma}}, {\eta})||+\sigma^{{i}-1}||{\mathbb{V}}_{0}({{\varsigma}}, {\eta})||+\cdots+\sigma^{{i}+1}||{\mathbb{V}}_{0}({{\varsigma}}, {\eta})||\\ = &||{\mathbb{V}}_{0}({{\varsigma}}, {\eta})||(\sigma^{{i}}+\sigma^{{i}-1}+\sigma^{{i}+1})\\ = &||{\mathbb{V}}_{0}({{\varsigma}}, {\eta})||\frac{1-\sigma^{{i}-n}}{1-\sigma^{{i}+1}}\sigma^{n+1}. \end{split} \end{equation}$

(3.12)

Since $0 < \sigma < 1$ , and ${\mathbb{V}}_{0}({{\varsigma}}, {\eta})$ is bounded, let us take $\beta = 1-\sigma/(1-\sigma_{{i}-n})\sigma^{n+1}||{\mathbb{V}}_{0}({{\varsigma}}, {\eta})||$ , and we obtain Thus, $\{{\mathbb{V}}_{{i}}({{\varsigma}}, {\eta})\}_{{i} = 0}^{\infty}$ forms a "Cauchy sequence" in H. It follows that the sequence $\{{\mathbb{V}}_{{i}}({{\varsigma}}, {\eta})\}_{{i} = 0}^{\infty}$ is a convergent sequence with the limit $\lim_{{i}\rightarrow \infty}{\mathbb{V}}_{{i}}({{\varsigma}}, {\eta}) = {\mathbb{V}}({{\varsigma}}, {\eta})$ for $\exists {\mathbb{V}}({{\varsigma}}, {\eta})\in \mathcal{H}$ . Hence, this ends the proof.

Theorem 3.2. Let $\sum_{h = 0}^{k}{\mathbb{V}}_{h}({{{\varsigma}}}, {\eta})$ is finite and ${\mathbb{V}}({{\varsigma}}, {\eta})$ represents the obtained series solution. Let $\sigma > 0$ such that $||{\mathbb{V}}_{h+1}({{\varsigma}}, {\eta})||\leq||{\mathbb{V}}_{h}({{\varsigma}}, {\eta})||$ , then the following relation gives the maximum absolute error ^[25].

$\begin{equation} ||{\mathbb{V}}({{\varsigma}}, {\eta})-\sum\limits_{h = 0}^{k}{\mathbb{V}}_{h}({{\varsigma}}, {\eta})|| < \frac{\sigma^{k+1}}{1-\sigma}||{\mathbb{V}}_{0}({{\varsigma}}, {\eta})||. \end{equation}$

(3.13)

Proof. Since $\sum_{h = 0}^{k}{\mathbb{V}}_{h}({{\varsigma}}, {\eta})$ is finite, this implies that $\sum_{h = 0}^{k}{\mathbb{V}}_{h}({{\varsigma}}, {\eta}) < \infty$ .

Consider

$\begin{equation} \begin{split} ||{\mathbb{V}}({{\varsigma}}, {\eta})-\sum\limits_{h = 0}^{k}{\mathbb{V}}_{h}({{\varsigma}}, {\eta})|| = &||\sum\limits_{h = k+1}^{\infty}{\mathbb{V}}_{h}({{\varsigma}}, {\eta})||\\ \leq&\sum\limits_{h = k+1}^{\infty}||{\mathbb{V}}_{h}({{\varsigma}}, {\eta})||\\ \leq&\sum\limits_{h = k+1}^{\infty}\sigma^{h}||{\mathbb{V}}_{0}({{\varsigma}}, {\eta})||\\ \leq&\sigma^{k+1}(1+\sigma+\sigma^{2}+\cdots)||{\mathbb{V}}_{0}({{\varsigma}}, {\eta})||\\ \leq&\frac{\sigma^{k+1}}{1-\sigma}||{\mathbb{V}}_{0}({{\varsigma}}, {\eta})||. \end{split} \end{equation}$

(3.14)

This ends the theorem's proof.

4. Implementation of the technique

4.1. Example

Consider the fractional nonlinear EW equation

$\begin{equation} D_{\eta}^{{\alpha}}\mathbb{V}+\mathbb{V}\mathbb{V}_{\varsigma}-\mathbb{V}_{\varsigma\varsigma\eta} = 0, \ \ \eta > 0, \ \ \varsigma \in R, \ \ 0 < {\alpha}\leq 1, \end{equation}$

(4.1)

with the initial condition

$\begin{equation} \mathbb{V}(\varsigma, 0) = 3sech^2\Bigg(\frac{\varsigma-15}{2}\Bigg). \end{equation}$

(4.2)

Employing the Yang transform on (4.1) with initial condition (4.2), we have

$\begin{equation} \begin{split} \frac{1}{(1+{\alpha}(v-1))}\mathbb{Y}\Bigg(\mathbb{V}(\varsigma, \eta)\Bigg)-\frac{v}{(1+{\alpha}(v-1))}\mathbb{V}(\varsigma, 0) = {\mathbb{Y}}\Bigg[\mathbb{V}_{\varsigma \varsigma \eta}-\mathbb{V} \mathbb{V}_{\varsigma}\Bigg], \\ \mathbb{Y}\Bigg[\mathbb{V}(\varsigma, \eta)\Bigg] = {v} 3sech^2\Bigg(\frac{\varsigma-15}{2}\Bigg)+(1+{\alpha}(v-1)){\mathbb{Y}}\Bigg[\mathbb{V}_{\varsigma \varsigma \eta}-\mathbb{V} \mathbb{V}_{\varsigma}\Bigg]. \end{split} \end{equation}$

(4.3)

Now using the inverse Yang transform we have

$\begin{equation} \mathbb{V}(\varsigma, \eta) = 3sech^2\Bigg(\frac{\varsigma-15}{2}\Bigg)+{\mathbb{Y}}^{-1}\Bigg[(1+{\alpha}(v-1)){\mathbb{Y}}\Bigg\{\mathbb{V}_{\varsigma \varsigma \eta}-\mathbb{V} \mathbb{V}_{\varsigma}\Bigg\}\Bigg]. \end{equation}$

(4.4)

Now we implemented HPM, we get

$\begin{equation} \sum\limits_{{\jmath} = 0}^{\infty}p^{\jmath} \mathbb{V}_{\jmath}(\varsigma, \eta) = 3sech^2\Bigg(\frac{\varsigma-15}{2}\Bigg)+\\ p\Bigg[{\mathbb{Y}}^{-1}\Bigg\{(1+{\alpha}(v-1)){\mathbb{Y}}\Bigg(\Bigg(\sum\limits_{{\jmath} = 0}^{\infty}p^{\jmath} \mathbb{V}_{\jmath}(\varsigma, \eta)_{\varsigma \varsigma \eta}\Bigg)-\Bigg(\sum\limits_{{\jmath} = 0}^{\infty}p^{\jmath} H_{\jmath}(\mathbb{V})\Bigg)\Bigg)\Bigg\}\Bigg]. \end{equation}$

(4.5)

The nonlinear term can be find with the help of He's polynomials

$\begin{equation} \Sigma_{{\jmath} = 0}^{\infty}p^{\jmath} H_{\jmath}(\mathbb{V}) = \mathbb{V} \mathbb{V}_{\varsigma}. \end{equation}$

(4.6)

The He's polynomials can be written as

$\begin{equation*} \begin{split} H_0(\mathbb{V})& = \mathbb{V}_{0}(\mathbb{V}_0)_{\varsigma}, \\ H_1(\mathbb{V})& = \mathbb{V}_{0}(\mathbb{V}_1)_{\varsigma}+\mathbb{V}_{1}(\mathbb{V}_0)_{\varsigma}, \\ \vdots \end{split} \end{equation*}$

Coefficients $p$ comparing, we obtain as

$p^0:\mathbb{V}_0(\varsigma, \eta) = 3sech^2\Bigg(\frac{\varsigma-15}{2}\Bigg), \\ p^1:\mathbb{V}_1(\varsigma, \eta) = {\mathbb{Y}}^{-1}\Bigg[(1+{\alpha}(v-1)){\mathbb{Y}}\Bigg\{(\mathbb{V}_0)_{\varsigma \varsigma \eta}-H_0\Bigg\}\Bigg], \\ p^1:\mathbb{V}_1(\varsigma, \eta) = 9sech^4\Bigg(\frac{\varsigma-15}{2}\Bigg)\tanh\Bigg(\frac{\varsigma-15}{2}\Bigg)\Big(1+{\alpha}{{\eta}}-{\alpha}\Big), \\ p^2:\mathbb{V}_2(\varsigma, \eta) = {\mathbb{Y}}^{-1}\Bigg[(1+{\alpha}(v-1)){\mathbb{Y}}\Bigg\{(\mathbb{V}_1)_{\varsigma \varsigma \eta}-H_1\Bigg\}\Bigg],$

$p^2:\mathbb{V}_2(\varsigma, \eta) = \frac{9}{4}\frac{1}{\cosh^12\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)}\\ \Bigg[\sinh\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)\Bigg\{-24\Big((1-{\alpha})2{\alpha}{{\eta}}+(1-{\alpha})^{2}+\frac{{\alpha}^{2}{{\eta}}^{2}}{2}\Big)\cosh^3\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)\\ +30\Big((1-{\alpha})2{\alpha}{{\eta}}+(1-{\alpha})^{2}+\frac{{\alpha}^{2}{{\eta}}^{2}}{2}\Big)\cosh\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)-\\ 72\Big(1+{\alpha}{{\eta}}-{\alpha}\Big)\sinh\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)\cosh\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)\\ +135\Big(1+{\alpha}{{\eta}}-{\alpha}\Big)\sinh\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)+4\cosh^7\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)\Bigg\}\Big(1+{\alpha}{{\eta}}-{\alpha}\Big)\Bigg], \\ \vdots$

Provides the series form solution is $\mathbb{V}(\varsigma, \eta) = \Sigma_{k = 0}^{\infty}\mathbb{V}_k(\varsigma, \eta)$

$\mathbb{V}(\varsigma, \eta) = 3sech^2\Bigg(\frac{\varsigma-15}{2}\Bigg)+9sech^4\Bigg(\frac{\varsigma-15}{2}\Bigg)\tanh\Bigg(\frac{\varsigma-15}{2}\Bigg)\Big(1+{\alpha}{{\eta}}-{\alpha}\Big)+\\ \frac{9}{4}\frac{1}{\cosh^{12}\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)}\Bigg[\sinh\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)\Bigg\{-24\Big((1-{\alpha})2{\alpha}{{\eta}}+(1-{\alpha})^{2}+\frac{{\alpha}^{2}{{\eta}}^{2}}{2}\Big)\\ \cosh^3\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)\\ +30\Big((1-{\alpha})2{\alpha}{{\eta}}+(1-{\alpha})^{2}+\frac{{\alpha}^{2}{{\eta}}^{2}}{2}\Big)\cosh\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)-72\Big(1+{\alpha}{{\eta}}-{\alpha}\Big)\\ \sinh\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)\cosh\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)\\ +135\Big(1+{\alpha}{{\eta}}-{\alpha}\Big)\sinh\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)+4\cosh^7\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)\Bigg\}\Big(1+{\alpha}{{\eta}}-{\alpha}\Big)\Bigg]+\cdots.$

(4.7)

The Eq (4.7) put ${\alpha} = 1$ , we obtain the solution of the suggested problem as:

$\begin{equation} \begin{split} \mathbb{V}(\varsigma, \eta)& = 3sech^2\Bigg(\frac{\varsigma-15}{2}\Bigg)+9sech^4\Bigg(\frac{\varsigma-15}{2}\Bigg)\tanh\Bigg(\frac{\varsigma-15}{2}\Bigg)\eta+\\ &\frac{9}{4}\frac{1}{\cosh^{12}\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)}\Bigg[\sinh\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)\Bigg\{-24\eta^{2}\cosh^3\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)\\ &+30\eta^{2}\cosh\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)-72\eta\sinh\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)\cosh\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)\\ &+135\eta\sinh\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)+4\cosh^7\Bigg(\frac{1}{2}\varsigma-\frac{15}{2}\Bigg)\Bigg\}\eta\Bigg]+\cdots. \end{split} \end{equation}$

(4.8)

The exact result is:

$\begin{equation} \mathbb{V}(\varsigma, \eta) = 3sech^2\Bigg(\frac{\varsigma-15-\eta}{2}\Bigg). \end{equation}$

(4.9)

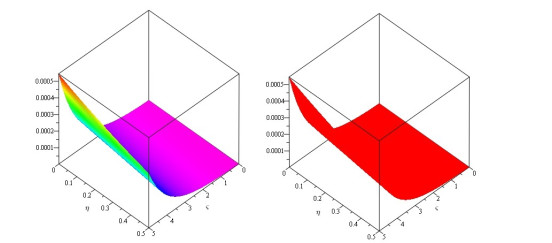
Example 1, displays the evolution of the exact and HPTM solutions at $\alpha = 1$ . shows that the different fractional order at $\alpha = 0.8$ and $0.6$ . In , first graph the fractional order at $\alpha = 0.4$ and second graph show that the various fractional graph of Example 1. In Table 1, the illustrates a computational evaluation of the HPM ^[27] and the HPTM in accordance with absolute error, considering both fractional derivative operators into account.

Figure 1. The exact and analytical solution graph of Example 1.

DownLoad: Full-Size Img PowerPoint

Figure 2. The fractional order graph of

${\alpha} = 0.8$ and

$0.6$ of Example 1.

DownLoad: Full-Size Img PowerPoint

Figure 3. The first graph show that the fractional-order of

$\alpha = 0.4$ and second graph of various fractional

${\alpha}$ of Example 1.

DownLoad: Full-Size Img PowerPoint

Table 1. Comparative analysis of HPM ^[27] and Current method (CM) solution of Example 1.

${\eta}$	${\varsigma}$	$\|Exact-HPM\|$	$\|Exact-HPM\|$	$\|Exact-CM\|$	$\|Exact-CM\|$
		$\alpha=0.6$	$\alpha=1$	$\alpha=0.8$	$\alpha=1$
	0.5	8.48379150 $\times10^{-03}$	1.5700000 $\times10^{-08}$	4.09610800 $\times10^{-04}$	1.5700000 $\times10^{-08}$
	1	1.69675830 $\times10^{-02}$	6.3000000 $\times10^{-08}$	1.63844300 $\times10^{-03}$	6.3000000 $\times10^{-08}$
	1.5	2.54513740 $\times10^{-02}$	1.4100000 $\times10^{-07}$	3.68649700 $\times10^{-03}$	1.4100000 $\times10^{-07}$
	2	3.39351660 $\times10^{-02}$	2.5200000 $\times10^{-07}$	6.55377200 $\times10^{-03}$	2.5200000 $\times10^{-07}$
	2.5	4.24189580 $\times10^{-02}$	3.9300000 $\times10^{-07}$	1.02402690 $\times10^{-02}$	3.9300000 $\times10^{-07}$
0.1	3	5.09027490 $\times10^{-02}$	5.6700000 $\times10^{-07}$	1.47459870 $\times10^{-02}$	5.6700000 $\times10^{-07}$
	3.5	5.93865400 $\times10^{-02}$	7.7000000 $\times10^{-07}$	2.00709300 $\times10^{-02}$	7.7000000 $\times10^{-07}$
	4	6.78703320 $\times10^{-02}$	1.0100000 $\times10^{-06}$	2.62150900 $\times10^{-02}$	1.0100000 $\times10^{-06}$
	4.5	7.63541240 $\times10^{-02}$	1.2700000 $\times10^{-06}$	3.31784700 $\times10^{-02}$	1.2700000 $\times10^{-06}$
	5	8.48379150 $\times10^{-02}$	1.5700000 $\times10^{-06}$	4.09610800 $\times10^{-02}$	1.5700000 $\times10^{-06}$
	0.5	1.27076980 $\times10^{-02}$	6.2500000 $\times10^{-08}$	6.81188800 $\times10^{-04}$	6.2500000 $\times10^{-08}$
	1	2.54153960 $\times10^{-02}$	2.5000000 $\times10^{-07}$	2.72475500 $\times10^{-03}$	2.5000000 $\times10^{-07}$
	1.5	3.81230940 $\times10^{-02}$	5.6300000 $\times10^{-07}$	6.13069900 $\times10^{-03}$	5.6300000 $\times10^{-07}$
	2	5.08307920 $\times10^{-02}$	1.0000000 $\times10^{-06}$	1.08990200 $\times10^{-02}$	1.0000000 $\times10^{-06}$
	2.5	6.35384900 $\times10^{-02}$	1.5630000 $\times10^{-06}$	1.70297190 $\times10^{-02}$	1.5630000 $\times10^{-06}$
0.2	3	7.62461880 $\times10^{-02}$	2.2500000 $\times10^{-06}$	2.45227950 $\times10^{-02}$	2.2500000 $\times10^{-06}$
	3.5	8.89538860 $\times10^{-02}$	3.0600000 $\times10^{-06}$	3.33782500 $\times10^{-02}$	3.0600000 $\times10^{-06}$
	4	1.01661584 $\times10^{-01}$	4.0000000 $\times10^{-06}$	4.35960800 $\times10^{-02}$	4.0000000 $\times10^{-06}$
	4.5	1.14369282 $\times10^{-01}$	5.0600000 $\times10^{-06}$	5.51762900 $\times10^{-02}$	5.0600000 $\times10^{-06}$
	5	1.2707698 $\times10^{-01}$	6.2500000 $\times10^{-06}$	6.81188800 $\times10^{-02}$	6.2500000 $\times10^{-06}$

| Show Table

DownLoad: CSV

4.2. Example

Consider the fractional nonlinear EW equation

$\begin{equation} D_{\eta}^{{\alpha}}\mathbb{V}+3\mathbb{V}^2\mathbb{V}_{\varsigma}-\mathbb{V}_{\varsigma\varsigma\eta} = 0, \ \ \eta > 0, \ \ \varsigma \in R, \ \ 0 < {\alpha}\leq 1, \end{equation}$

(4.10)

with initial condition

$\begin{equation} \mathbb{V}(\varsigma, 0) = \frac{1}{4} sech(\varsigma-30). \end{equation}$

(4.11)

Incorporating Yang transform on (4.10), we get

$\begin{equation} {\mathbb{Y}}\Bigg[\mathbb{V}(\varsigma, \eta)\Bigg] = {v} \mathbb{V}(\varsigma, 0)+(1+{\alpha}(v-1)){\mathbb{Y}}\Bigg[\mathbb{V}_{\varsigma \varsigma \eta}-3\mathbb{V}^2 \mathbb{V}_{\varsigma}\Bigg]. \end{equation}$

(4.12)

Using the initial condition in Eq (4.12), we have

$\begin{equation} {\mathbb{Y}}\Bigg[\mathbb{V}(\varsigma, \eta)\Bigg] = {v} \frac{1}{4} sech(\varsigma-30)+(1+{\alpha}(v-1)){\mathbb{Y}}\Bigg[\mathbb{V}_{\varsigma \varsigma \eta}-3\mathbb{V}^2 \mathbb{V}_{\varsigma}\Bigg]. \end{equation}$

(4.13)

By applying inverse Yang transform, we have

$\begin{equation} \mathbb{V}(\varsigma, \eta) = \frac{1}{4} sech(\varsigma-30)+{\mathbb{Y}}^{-1}\Bigg[(1+{\alpha}(v-1)){\mathbb{Y}}\Bigg\{\mathbb{V}_{\varsigma \varsigma \eta}-3\mathbb{V}^2 \mathbb{V}_{\varsigma}\Bigg\}\Bigg]. \end{equation}$

(4.14)

Now we implemented HPM, we get

$\Sigma_{{\jmath} = 0}^{\infty}p^{\jmath}\mathbb{V}_{\jmath}(\varsigma, \eta) = \frac{1}{4} sech(\varsigma-30)+p\\ \Bigg[{\mathbb{Y}}^{-1}\Bigg\{(1+{\alpha}(v-1)){\mathbb{Y}}\Bigg(\Sigma_{{\jmath} = 0}^{\infty}p^{\jmath}\mathbb{V}_{\jmath}(\varsigma, \eta)_{\varsigma \varsigma \eta}-(\Sigma_{{\jmath} = 0}^{\infty}p^{\jmath} H_{\jmath}(\mathbb{V}))\Bigg)\Bigg\}\Bigg].$

(4.15)

The nonlinear term can be find with the help of He's polynomials

$\begin{equation} \Sigma_{{\jmath} = 0}^{\infty}p^{\jmath} H_{\jmath}(\mathbb{V}) = 3\mathbb{V}^2 \mathbb{V}_{\varsigma}. \end{equation}$

(4.16)

The He's polynomials can be written as

$\begin{equation*} \begin{split} H_0(\mathbb{V})& = 3(\mathbb{V}_{0})^2(\mathbb{V}_0)_{\varsigma}, \\ H_1(\mathbb{V})& = 3(\mathbb{V}_{0})^2(\mathbb{V}_1)_{\varsigma}+6\mathbb{V}_0\mathbb{V}_{1}(\mathbb{V}_0)_{\varsigma}, \\ \vdots \end{split} \end{equation*}$

Coefficients $p$ comparing, we obtain as

$\begin{equation*} \begin{split} p^0:\mathbb{V}_0(\varsigma, \eta)& = \frac{1}{4} sech(\varsigma-30), \\ p^1:\mathbb{V}_1(\varsigma, \eta)& = {\mathbb{Y}}^{-1}\Bigg[(1+{\alpha}(v-1)){\mathbb{Y}}\Bigg\{(\mathbb{V}_0)_{\varsigma \varsigma \eta}-H_0(\mathbb{V})\Bigg\}\Bigg], \\ p^1:\mathbb{V}_1(\varsigma, \eta)& = \frac{3}{64} sech^3(\varsigma-30)\tanh(\varsigma-30)\Big(1+{\alpha}{{\eta}}-{\alpha}\Big), \\ \vdots\\ \end{split} \end{equation*}$

provides the series form solution is

$\begin{equation} \begin{split} \mathbb{V}(\varsigma, \eta)& = \Sigma_{m = 0}^{\infty}\mathbb{V}_m(\varsigma, \eta), \\ \mathbb{V}(\varsigma, \eta)& = \frac{1}{4} sech(\varsigma-30)+\frac{3}{64} sech^3(\varsigma-30)\tanh(\varsigma-30)\Big(1+{\alpha}{{\eta}}-{\alpha}\Big)+\cdots. \end{split} \end{equation}$

(4.17)

The Eq (4.17) put ${\alpha} = 1$ , we obtain the solution of the suggested problem as:

$\begin{equation} \mathbb{V}(\varsigma, \eta) = \frac{1}{4} sech(\varsigma-30)+\frac{3}{64} sech^3(\varsigma-30)\tanh(\varsigma-30)+\cdots. \end{equation}$

(4.18)

The exact result is:

$\begin{equation} \mathbb{V}(\varsigma, \eta) = \frac{1}{4} sech\Bigg(\varsigma-30-\frac{\eta}{4}\Bigg). \end{equation}$

(4.19)

Example 2, displays the evolution of the exact and HPTM solutions at $\alpha = 1$ . shows that the different fractional order at $\alpha = 0.8$ and $0.6$ . In , first graph the fractional order at $\alpha = 0.4$ and second graph show that the various fractional graph of Example 2.

Figure 4. The exact and analytical solution graph of Example 2.

DownLoad: Full-Size Img PowerPoint

Figure 5. The fractional order graph of

${\alpha} = 0.8$ and

$0.6$ of Example 2.

DownLoad: Full-Size Img PowerPoint

Figure 6. The first graph show that the fractional-order of

$\alpha = 0.4$ and second graph of various fractional

${\alpha}$ of Example 2.

DownLoad: Full-Size Img PowerPoint

4.3. Example

Consider the fractional nonlinear EW equation

$\begin{equation} D_{\eta}^{{\alpha}}\mathbb{V}+\frac{12}{7}(\mathbb{V}^6)_\varsigma-\frac{3}{7}(\mathbb{V}^6)_{\varsigma\varsigma\eta} = 0, \ \ \eta > 0, \ \ \varsigma \in R, \ \ 0 < {\alpha}\leq 1, \end{equation}$

(4.20)

with initial condition

$\begin{equation} \mathbb{V}(\varsigma, 0) = \cosh^{\frac{2}{5}}\Bigg(\frac{5\varsigma}{6}\Bigg). \end{equation}$

(4.21)

Using Yang transform on (4.20), we get

$\begin{equation} E\Bigg[\mathbb{V}(\varsigma, \eta)\Bigg] = {v}\mathbb{V}(\varsigma, 0)+(1+{\alpha}(v-1)){\mathbb{Y}}\Bigg[\frac{12}{7}(\mathbb{V}^6)_\varsigma-\frac{3}{7}(\mathbb{V}^6)_{\varsigma\varsigma\eta}\Bigg]. \end{equation}$

(4.22)

Putting the initial condition in the Eq (4.22), we have

$\begin{equation} E\Bigg[\mathbb{V}(\varsigma, \eta)\Bigg] = {v}\cosh^{\frac{2}{5}}\Bigg(\frac{5\varsigma}{6}\Bigg)+(1+{\alpha}(v-1)){\mathbb{Y}}\Bigg[\frac{12}{7}(\mathbb{V}^6)_\varsigma-\frac{3}{7}(\mathbb{V}^6)_{\varsigma\varsigma\eta}\Bigg]. \end{equation}$

(4.23)

By applying inverse Yang transform, we have

$\begin{equation} \mathbb{V}(\varsigma, \eta) = \cosh^{\frac{2}{5}}\Bigg(\frac{5\varsigma}{6}\Bigg)+{\mathbb{Y}}^{-1}\Bigg[(1+{\alpha}(v-1)){\mathbb{Y}}\Bigg\{\frac{12}{7}(\mathbb{V}^6)_\varsigma-\frac{3}{7}(\mathbb{V}^6)_{\varsigma\varsigma\eta}\Bigg\}\Bigg]. \end{equation}$

(4.24)

Now we implemented HPM, we get

$\begin{equation} \Sigma_{{\jmath} = 0}^{\infty}p^{\jmath}\mathbb{V}_{\jmath}(\varsigma, \eta) = \cosh^{\frac{2}{5}}\Bigg(\frac{5\varsigma}{6}\Bigg)+p\Bigg[{\mathbb{Y}}^{-1}\Bigg\{(1+{\alpha}(v-1)){\mathbb{Y}}\Bigg(\Sigma_{{\jmath} = 0}^{\infty}p^{\jmath}\mathbb{V}_{\jmath}(\varsigma, \eta)_{\varsigma \varsigma \eta}\Bigg)\Bigg\}\Bigg]. \end{equation}$

(4.25)

The nonlinear term can be find with the help of He's polynomials

$\begin{equation} \Sigma_{{\jmath} = 0}^{\infty}p^{\jmath} H_{\jmath}(\mathbb{V}) = \frac{12}{7}(\mathbb{V}^6)_\varsigma-\frac{3}{7}(\mathbb{V}^6)_{\varsigma\varsigma\eta}. \end{equation}$

(4.26)

The He's polynomials can be written as

$\begin{equation*} \begin{split} H_0(\mathbb{V}) = \frac{12}{7}(\mathbb{V}_0^6)_\varsigma-\frac{3}{7}(\mathbb{V}_0^6)_{\varsigma\varsigma\eta}\\ \vdots \end{split} \end{equation*}$

Coefficients $p$ comparing, we obtain as

$\begin{equation*} \begin{split} p^0:\mathbb{V}_0(\varsigma, \eta)& = \cosh^{\frac{2}{5}}\Bigg(\frac{5\varsigma}{6}\Bigg), \\ p^1:\mathbb{V}_1(\varsigma, \eta)& = {\mathbb{Y}}^{-1}\Bigg[(1+{\alpha}(v-1)){\mathbb{Y}}\Bigg\{H_0(\mathbb{V})\Bigg\}\Bigg], \\ p^1:\mathbb{V}_1(\varsigma, \eta)& = -\frac{24}{7}\cosh^{\frac{7}{5}}\Bigg(\frac{5\varsigma}{6}\Bigg)\sinh\Bigg(\frac{5\varsigma}{6}\Bigg)\Big(1+{\alpha}{{\eta}}-{\alpha}\Big), \\ \vdots \end{split} \end{equation*}$

The series form solution is

$\begin{equation*} \mathbb{V}(\varsigma, \eta) = \sum\limits_{m = 0}^{\infty}\mathbb{V}_m(\varsigma, \eta) \end{equation*}$

$\begin{equation} \mathbb{V}(\varsigma, \eta) = \cosh^{\frac{2}{5}}\Bigg(\frac{5\varsigma}{6}\Bigg)-\frac{24}{7}\cosh^{\frac{2}{5}}\Bigg(\frac{5\varsigma}{6}\Bigg)\sinh\Bigg(\frac{5\varsigma}{6}\Bigg)\Big(1+{\alpha}{{\eta}}-{\alpha}\Big)+\cdots. \end{equation}$

(4.27)

The Eq (4.27) put ${\alpha} = 1$ , we obtain the solution of the suggested problem as:

$\begin{equation} \mathbb{V}(\varsigma, \eta) = \cosh^{\frac{2}{5}}\Bigg(\frac{5\varsigma}{6}\Bigg)-\frac{24}{7}\cosh^{\frac{7}{5}}\Bigg(\frac{5\varsigma}{6}\Bigg)\sinh\Bigg(\frac{5\varsigma}{6}\Bigg)\eta+\cdots. \end{equation}$

(4.28)

The exact result is:

$\begin{equation} \mathbb{V}(\varsigma, \eta) = \cosh^{\frac{2}{5}}\Bigg\{\frac{5}{6}(\varsigma-\eta)\Bigg\}. \end{equation}$

(4.29)

Example 3, displays the evolution of the exact and HPTM solutions at $\alpha = 1$ . shows that the different fractional order at $\alpha = 0.8$ and $0.6$ . In , first graph the fractional order at $\alpha = 0.4$ and second graph show that the various fractional graph of Example 3.

Figure 7. The exact and analytical solution graph of Example 3.

DownLoad: Full-Size Img PowerPoint

Figure 8. The fractional order graph of

${\alpha} = 0.8$ and

$0.6$ of Example 3.

DownLoad: Full-Size Img PowerPoint

Figure 9. The first graph show that the fractional-order of

$\alpha = 0.4$ and second graph of various fractional

${\alpha}$ of Example 3.

DownLoad: Full-Size Img PowerPoint

5. Conclusions

In this paper, we determined the fractional equal-width equations, applying an homotopy perturbation transform method. The solutions for some problems are investigated applied the given technique. The homotopy perturbation transform method solution is a good agreement with the exact solution of the suggested problems. The present technique are investigated the solutions of fractional-order examples. The figures analysis of the fractional-order results achieved has verify the convergence toward the results of the integer-order. The scheme effective and comprehensive execution is investigated and confirmed in an attempt to display that it may be applicable to other nonlinear evolutionary models that emerge in applied science.

Acknowledgments

The authors are thankful to the Deanship of Scientific Research at Najran University for funding this work under the General Research Funding program grant code (NU/RG/SERC/11/3).

Conflict of interest

The authors declare that there is no conflict of interest.

References

[1]	D. Baleanu, Z. B. Guvenc, J. A. T. Machado, New trends in nanotechnology and fractional calculus applications, New York: Springer, 2010.
[2]	D. Baleanu, J. A. T. Machado, A. C. Luo, Fractional dynamics and control, Springer Science & Business Media, 2011.
[3]	K. M. Saad, A different approach for the fractional chemical model, Rev. Mex. Fis., 68 (2022), 011404. https://doi.org/10.31349/RevMexFis.68.011404 doi: 10.31349/RevMexFis.68.011404
[4]	Y. Talaei, S. Micula, H. Hosseinzadeh, S. Noeiaghdam, A novel algorithm to solve nonlinear fractional quadratic integral equations, AIMS Math., 7 (2022), 13237–13257. https://doi.org/10.3934/math.2022730 doi: 10.3934/math.2022730
[5]	F. Ghomanjani, S. Noeiaghdam, S. Micula, Application of transcendental Bernstein polynomials for solving two-dimensional fractional optimal control problems, Complexity, 2022. https://doi.org/10.1155/2022/4303775
[6]	H. Khan, D. Baleanu, P. Kumam, M. Arif, The analytical investigation of time-fractional multi-dimensional Navier-Stokes equation, Alex. Eng. J., 59 (2020), 2941–2956. https://doi.org/10.1016/j.aej.2020.03.029 doi: 10.1016/j.aej.2020.03.029
[7]	M. Caputo, Linear models of dissipation whose Q is almost frequency independent II, Geophys. J. Int., 13 (1967), 529–539.
[8]	S. Noeiaghdam, S. Micula, J. J. Nieto, A novel technique to control the accuracy of a nonlinear fractional order model of COVID-19: Application of the CESTAC method and the CADNA library, Mathematics, 9 (2021), 1321. https://doi.org/10.1111/j.1365-246X.1967.tb02303.x doi: 10.1111/j.1365-246X.1967.tb02303.x
[9]	K. B. Oldham, J. Spanier, The fractional calculus, New York: Acadamic Press, 1974. https://doi.org/10.3390/math9121321
[10]	H. Mohammadi, S. Kumar, S. Rezapour, S. Etemad, A theoretical study of the Caputo-Fabrizio fractional modeling for hearing loss due to Mumps virus with optimal control, Chaos Soliton. Fract., 144 (2021), 110668. https://doi.org/10.1016/j.chaos.2021.110668 doi: 10.1016/j.chaos.2021.110668
[11]	A. Alshabanat, M. Jleli, S. Kumar, B. Samet, Generalization of Caputo-Fabrizio fractional derivative and applications to electrical circuits, Front. Phys., 8 (2020), 64. https://doi.org/10.3389/fphy.2020.00064 doi: 10.3389/fphy.2020.00064
[12]	S. Kumar, R. Kumar, M. S. Osman, B. Samet, A wavelet based numerical scheme for fractional order SEIR epidemic of measles by using Genocchi polynomials, Numer. Meth. Part. D. E., 37 (2021), 1250–1268. https://doi.org/10.1002/num.22577 doi: 10.1002/num.22577
[13]	S. Kumar, A. Kumar, B. Samet, H. Dutta, A study on fractional host-parasitoid population dynamical model to describe insect species, Numer. Meth. Part. D. E., 37 (2021), 1673–1692. https://doi.org/10.1002/num.22603 doi: 10.1002/num.22603
[14]	X. J. Yang, H. M. Srivastava, J. A. Machado, A new fractional derivative without singular kernel: Application to the modelling of the steady heat flow, Therm. Sci., 20 (2016), 753–756.
[15]	H. Khan, U. Farooq, D. Baleanu, P. Kumam, M. Arif, Analytical solutions of (2+time fractional order) dimensional physical models, using modified decomposition method, Appl. Sci., 10 (2019), 122. https://doi.org/10.2298/TSCI151224222Y doi: 10.2298/TSCI151224222Y
[16]	R. Shah, H. Khan, D. Baleanu, Fractional Whitham-Broer-Kaup equations within modified analytical approaches, Axioms, 8 (2019), 125.
[17]	X. J. Yang, J. T. Machado, A new fractional operator of variable order: Application in the description of anomalous diffusion, Physica A, 481 (2017), 276–283. https://doi.org/10.3390/axioms8040125 doi: 10.3390/axioms8040125
[18]	P. D. Barba, L. Fattorusso, M. Versaci, Electrostatic field in terms of geometric curvature in membrane MEMS devices, Commun. Appl. Ind. Math., 8 (2017), 165–184. https://doi.org/10.1515/caim-2017-0009 doi: 10.1515/caim-2017-0009
[19]	L. Yong, H. N. Wang, X. Chen, X. Yang, Z. P. You, S. Dong, et al., Shear property, high-temperature rheological performance and low-temperature flexibility of asphalt mastics modified with bio-oil, Constr. Build. Mater., 174 (2018), 30–37.
[20]	J. H. He, Homotopy perturbation technique, Comput. Method. Appl. M., 178 (1999), 257–262. https://doi.org/10.1016/S0045-7825(99)00018-3 doi: 10.1016/S0045-7825(99)00018-3
[21]	S. Noeiaghdam, M. A. F. Araghi, D. Sidorov, Dynamical strategy on homotopy perturbation method for solving second kind integral equations using the CESTAC method, J. Comput. Appl. Math., 411 (2022), 114226. https://doi.org/10.1016/j.cam.2022.114226 doi: 10.1016/j.cam.2022.114226
[22]	S. Noeiaghdam, A. Dreglea, J. He, Z. Avazzadeh, M. Suleman, M. A. Fariborzi Araghi, et al., Error estimation of the homotopy perturbation method to solve second kind Volterra integral equations with piecewise smooth kernels: Application of the CADNA library, Symmetry, 12 (2020), 1730. https://doi.org/10.3390/sym12101730 doi: 10.3390/sym12101730
[23]	X. J. Yang, A new integral transform method for solving steady heat-transfer problem, Therm. Sci., 20 (2016), 639–642. https://doi.org/10.2298/TSCI16S3639Y doi: 10.2298/TSCI16S3639Y
[24]	M. Caputo, M. Fabrizio, On the singular kernels for fractional derivatives, some applications to partial differential equations, Progr. Fract. Differ. Appl., 7 (2021), 1–4. http://dx.doi.org/10.18576/pfda/0070201 doi: 10.18576/pfda/0070201
[25]	S. Ahmad, A. Ullah, A. Akgul, M. De la Sen, A novel homotopy perturbation method with applications to nonlinear fractional order KdV and Burger equation with exponential-decay kernel, J. Funct. Space., 2021. https://doi.org/10.1155/2021/8770488
[26]	X. J. Yang, A new integral transform method for solving steady heat-transfer problem, Therm. Sci., 20 (2016), 639–642. https://doi.org/10.2298/TSCI16S3639Y doi: 10.2298/TSCI16S3639Y
[27]	N. A. Shah, I. Dassios, J. D. Chung, Numerical investigation of time-fractional equivalent width equations that describe hydromagnetic waves, Symmetry, 13 (2021), 418. https://doi.org/10.3390/sym13030418 doi: 10.3390/sym13030418

This article has been cited by:

1.	Alexey Zhokh, Peter Strizhak, Zine El Abiddine Fellah, Green’s Functions on Various Time Scales for the Time-Fractional Reaction-Diffusion Equation, 2023, 2023, 1687-9139, 1, 10.1155/2023/6646284
2.	Bibi Fatima, Mati ur Rahman, Saad Althobaiti, Ali Althobaiti, Muhammad Arfan, Analysis of age wise fractional order problems for the Covid-19 under non-singular kernel of Mittag-Leffler law, 2024, 27, 1025-5842, 1303, 10.1080/10255842.2023.2239976
3.	Kashif Ali Abro, Ambreen Siyal, Abdon Atangana, Qasem M. Al-Mdallal, Analytical solution for the dynamics and optimization of fractional Klein–Gordon equation: an application to quantum particle, 2023, 55, 0306-8919, 10.1007/s11082-023-04919-1
4.	Hegagi Ali, Alaa Noreldeen, Ali Ali, A new analytical approximate solution of fractional coupled Korteweg-de Vries system, 2023, 33, 0354-0243, 667, 10.2298/YJOR221215013A

Reader Comments

Your name:*

Email:*
© 2022 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)

通讯作者: 陈斌, bchen63@163.com

1.
沈阳化工大学材料科学与工程学院沈阳 110142

AIMS Mathematics

1.8 3.4

Metrics

Article views(1894) PDF downloads(82) Cited by(4)

Preview PDF

Download XML

Export Citation

Article outline

Show full outline

Figures and Tables

Figures(9) / Tables(1)

AIMS Mathematics

Evaluation of fractional-order equal width equations with the exponential-decay kernel

Related Papers:

Abstract

1. Introduction

2. Preliminaries concepts

3. Methodology of the HPTM

4. Implementation of the technique

4.1. Example

4.2. Example

4.3. Example

5. Conclusions

Acknowledgments

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Catalog

AIMS Mathematics

Evaluation of fractional-order equal width equations with the exponential-decay kernel

Related Papers:

Abstract

1. Introduction

2. Preliminaries concepts

3. Methodology of the HPTM

4. Implementation of the technique

4.1. Example

4.2. Example

4.3. Example

5. Conclusions

Acknowledgments

Conflict of interest

References

This article has been cited by:

Reader Comments

通讯作者: 陈斌, bchen63@163.com

Metrics

Figures and Tables

Other Articles By Authors

Related pages

Tools

Export File

Citation

Format

Content

Catalog