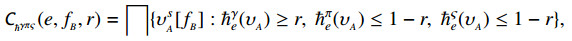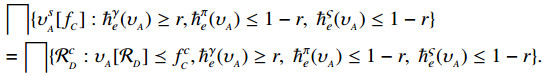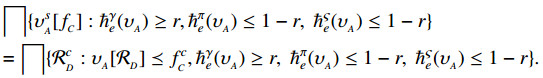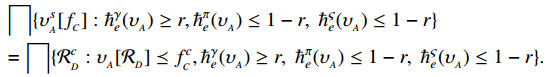On single-valued neutrosophic soft uniform spaces

1.   Introduction

There are many theories that have been suggested for dealing with uncertainties in an efficient way such as the theory of fuzzy sets^[1], the theory of intuitionistic fuzzy sets^[2], the theory of rough sets^[3], and the theory of neutrosophic sets^[4]. However, the idea of fuzzy sets, intuitionistic fuzzy sets, and neutrosophic sets are not sufficient to cope with parametrization tools. In 1999, Molodtsov^[5] proposed the idea of a soft set that has the ability to deal with this difficulty. The idea of fuzzy soft (FS) sets and neutrosophic soft sets was proposed by Maji et al.^[6,7], and some properties of FS sets were discussed by Ahmad and Kharal^[8]. Wang et al. ^[9] proposed the idea of single-valued neutrosophic sets. Saber et al. ^{[10,11,12,13]} introduced several concepts including, r-single-valued neutrosophic compact modulo, and r-single-valued neutrosophic connected sets in single-valued neutrosophic topological spaces, single-valued neutrosophic ideal open local function, single-valued neutrosophic $\theta£$-separated. Single-valued neutrosophic fuzzy set and multi-attribute decision-making were introduced by Sasirekha et al. ^[14]. Masri et al. ^[15] introduced the idea of a single-valued trapezoidal neutrosophic number.

Šostak's single-valued neutrosophic soft topological spaces and single-valued neutrosophic soft sets were constructed by Saber et al. ^[16]. The concept of single-valued neutrosophic soft has been thoroughly explored and advanced by numerous researchers, such as (Shahzadi et al. ^[17], Cano et al. ^[18], Özkan et al. ^[19], Al-Hijjawi et al. ^[20], Jana et al. ^[21] and Kamal et al. ^[22]) There are three alternative approaches to uniformity in the fuzzy case: Lowen's ^[23] entourage approach based on power sets of the form $\zeta^{\mathcal{X}\times\mathcal{X}}$, Kotzé's ^[24] uniform covering approach, and Hutton's ^[25] uniform operator approach.

It is well known that the theory of neutrosophic sets has been regarded as a generalization of the theory of fuzzy sets, the theory of intuitionistic fuzzy sets and the theory of rough sets. Furthermore, this is an important mathematical tool to deal with uncertainty. One of the main contributions of this paper is to introduce the concepts of single-valued neutrosophic uniformity in the sense of entourage, which is a generalization of the concepts introduced in Lowen^[23], Kotzé^[24], Hutton^[25] and Abbas et al. ^[26].

Motivated by the above discussion, the present work deals with the single-valued neutrosophic uniformity in the sense of entourage. We introduce the notions of single-valued neutrosophic soft uniform spaces and single-valued neutrosophic soft uniform bases. The notion of this single-valued neutrosophic soft uniformities to be stratified is ensured. We investigate the relationship among single-valued neutrosophic soft uniformities, single-valued neutrosophic soft topologies and single-valued neutrosophic soft interior operators. We study several single-valued neutrosophic soft topologies induced by a single-valued neutrosophic soft uniform structure. Finally, we introduce the product single-valued neutrosophic soft uniformity of a given family of single-valued neutrosophic soft uniform spaces.

2.   Preliminaries

In this section, we give all the basic definitions and results that we need to go through our work. First, we give the definition of a single-valued neutrosophic set (svn-set) and a single-valued neutrosophic soft set (svns-set). For more details about svn-set theory and svns-set theory, we refer to ^[9,16]. As usual, $\widehat{(\mathcal{X}, \text{E})}$ denotes the family of all svns-sets on $\mathcal{X}$, and $\text{E}$ is the set of all parameters. Additionally, $\mathcal{X}$ indicates an initial universe and $\zeta^{\mathcal{X}}$ are the sets of all svn-sets on $\mathcal{X}$ (where, $\zeta = [0, 1]$ and $\zeta_{0} = (0, 1]$).

Definition 1. ^[4]. Let $\mathcal{X}$ be a universe set. A neutrosophic set (n-set) $\Theta$ on $\mathcal{X}$ defined as

where $\gamma_{_{\Theta}}(y)$, $\pi_{_{\Theta}}(y)$ and $\varsigma_{_{\Theta}}(y)$ are the truth, the indeterminacy, and the falsity membership functions respectively.

Definition 2. ^[9]. Let $\mathcal{X}$ be a non-null set. Then, svn-set $\Theta$ on $\mathcal{X}$ is defined as

where $\gamma_{_{\Theta}}, \pi_{_{\Theta}}, \varsigma_{_{\Theta}}:\mathcal{X}\rightarrow \zeta$ and $0\leq\gamma_{_{\Theta}}(y)+\pi_{_{\Theta}}(y)+\varsigma_{_{\Theta}}(y)\leq3.$

Remark 1. To clarify the relationship between intuitionistic fuzzy sets if-set, neutrosophic sets n-set, and single-valued neutrosophic sets svn-set, let us confirm that both neutrosophic sets and single-valued neutrosophic sets are a generalization of the concept of intuitionistic fuzzy sets, as follows:

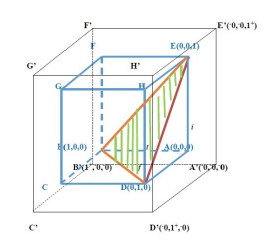
In IFS, paraconsistent, dialtheist and incomplete information cannot be characterized. This most important distinction between if-set and n-set is shown in the below neutrosophic cube A' B' C' D' E' F' G' H' introduced by J. Dezert ^[27].

Because only the classical interval [0, 1]is used as a range for the neutrosophic parameters in technical applications (truth, indeterminacy and falsity), we call the cube ABCDEDGH the technical neutrosophic cube and its extension A' B' C' D' E' F' G' H' the neutrosophic cube or nonstandard neutrosophic cube, used in the fields where we need to differentiate between absolute and relative notions like philosophy.

Definition 3. ^[16]. $f_{_{A}}$ is an svns-set on $\mathcal{X}$, where $f: {E}\rightarrow \zeta^{\mathcal{X}}$; i.e., $f_{e}\triangleq f(e)$ is an svn-set on $\mathcal{X}$, for all $e\in A$ and $f(e) = \langle0, 1, 1\rangle$, if $e\not\in A$.

The svn-set $f(e)$ is termed as an element of the $svns-set \; f_{_{A}}$. Thus, an $svns-set \; f_{ {E}}$ on $\mathcal{X}$ can be defined as:

where $\gamma_{_{f}}: {E}\rightarrow \zeta$ ($\gamma_{_{f}}$ is termed as a membership function), $\pi_{f}: {E}\rightarrow \zeta$ ($\pi_{_{f}}$ is termed as indeterminacy function) and $\varsigma_{_{f}}: {E}\rightarrow \zeta$ ($\varsigma_{_{f}}$ is termed as a nonmembership function) of $svns-set$.

An svns-set $f_{ {E}}$ on $\mathcal{X}$ is termed as a null svns-set (for short, $\widehat{\Phi}$), if $\gamma_{_{f}}(e) = 0, \pi_{_{f}}(e) = 1$ and $\varsigma_{_{f}}(e) = 1$, for any $e\in {E}$.

An $svns-set \; f_{E}$ on $\mathcal{X}$ is termed as an absolute svns-set (for short, $\tilde{\widehat{E}}$), if $\gamma_{_{f}}(e) = 1, \pi_{_{f}}(e) = 0$ and $\varsigma_{_{f}}(e) = 0$, for any $e\in {E}$.

Definition 4. ^[16]. Let $f_{_{A}}, g_{_{B}}\in \widehat{(\mathcal{X}, {E})}$ be an svns-sets on $\mathcal{X}$. Then,

(1) Inclusion of two sets (for short, $f_{_{A}}\preceq g_{_{B}}$) defined as:

(2) The complemented of the set $f_{_{A}}$ denoted by (for short, $f_{_{A}}^{c}$) defined as:

Definition 5. ^[16]. A mapping $\mathfrak{T}^{\gamma}, \mathfrak{T}^{\pi}, \mathfrak{T}^{\varsigma}: {E}\rightarrow \zeta^{\widehat{(\mathcal{X}, {E})}}$ is said to be a single-valued neutrosophic soft topology (svnst) on $\mathcal{X}$ if it meets the next criteria, for every $e\in {E}$:

$(\mathfrak{T}_{1}) \; \mathfrak{T}_{e}^{\gamma}(\widehat{\Phi}) = 1$, $\mathfrak{T}^{\pi}_{e}(\widehat{\Phi}) = 0$, $\mathfrak{T}^{\varsigma}_{e}(\widehat{\Phi}) = 0$ and $\mathfrak{T}^{\gamma}_{e}(\widehat{E}) = 1 \; \mathfrak{T}^{\pi}_{e}(\widehat{E}) = 0$, $\mathfrak{T}^{\varsigma}_{e}(\widehat{E}) = 0$,

$(\mathfrak{T}_{2}) \; \mathfrak{T}_{e}^{\gamma}(f_{_{A}}\sqcap g_{_{B}})\geq \mathfrak{T}_{e}^{\gamma}(f_{A})\wedge\mathfrak{T}_{e}^{\gamma}(g_{_{B}})$, $\mathfrak{T}_{e}^{\pi}(f_{A}\sqcap g_{_{B}})\leq \mathfrak{T}_{e}^{\pi}(f_{_{A}})\vee\mathfrak{T}_{e}^{\pi}(g_{_{B}})$,

$\mathfrak{T}_{e}^{\varsigma}(f_{_{A}}\sqcap g_{_{B}})\leq \mathfrak{T}_{e}^{\varsigma}(f_{_{A}})\vee\mathfrak{T}_{e}^{\varsigma}(g_{_{B}})$,  $\forall \; f_{_{A}}, g_{_{B}}\in \widehat{(\mathcal{X}, {E})}$,

$(\mathfrak{T}_{3}) \; \mathfrak{T}_{e}^{\gamma}(\bigsqcup\limits_{j\in \Gamma}(f_{_{A}})_{j})\geq \bigwedge\limits_{j\in \Gamma} \mathfrak{T}_{e}^{\gamma}((f_{A})_{j})$,   $\mathfrak{T}_{e}^{\pi}(\bigsqcup\limits_{j\in \Gamma}(f_{_{A}})_{j})\leq \bigvee\limits_{j\in \Gamma} \mathfrak{T}_{e}^{\pi}((f_{_{A}})_{j})$,

$\mathfrak{T}_{e}^{\varsigma}(\bigsqcup\limits_{j\in \Gamma}(f_{_{A}})_{j})\leq \bigvee\limits_{j\in \Gamma} \mathfrak{T}_{e}^{\varsigma}((f_{A})_{j})$,   $\forall \; (f_{A})_{j}\in \widehat{(\mathcal{X}, {E})}$, $j\in \Gamma$.

(Note that $\sqcap$ and $\sqcup$ in the definition are clarified in Molodtsov ^[5]). The quadruple $(\mathcal{X}, \mathfrak{T}^{\gamma}, \mathfrak{T}^{\pi}, \mathfrak{T}^{\varsigma})$ is said to be a single-valued neutrosophic soft topological space (svnst-space), where $(\mathfrak{T}_{e}^{\gamma}(f_{_{A}}))$ representing the degree of openness, $(\mathfrak{T}_{e}^{\pi}(f_{_{A}}))$ the degree of indeterminacy and $(\mathfrak{T}_{e}^{\varsigma}(f_{_{A}}))$ the degree of non-openness; of a svns-set with respect to that parameter $e\in {E}$. Sometimes, we will write $\mathfrak{T}^{\gamma\pi\varsigma}$ for $(\mathfrak{T}^{\gamma}, \mathfrak{T}^{\pi}, \mathfrak{T}^{\varsigma})$.

Let $(\mathcal{X}, \mathfrak{T}^{\gamma\pi\varsigma})$ and $(\mathcal{G}, \mathfrak{T}^{\star\gamma\pi\varsigma})$ be svnst-space. An svns-mapping $\psi_{_{\varphi}}:\widehat{(\mathcal{X}, {E})}\rightarrow \widehat{(\mathcal{G}, \mathcal{R})}$ is said to be a single-valued neutrosophic soft continuous mapping (svnsc-map) if

for all $g_{_{B}}\in \widehat{(\mathcal{G}, \mathcal{R})}$ and $e\in {E}$ [Saber et al. (2022)^[16].

Definition 6. A map ${I}: {E}\times\widehat{(\mathcal{X}, {E})}\times \zeta_{0}\rightarrow \widehat{(\mathcal{X}, {E})}$ is said to be single-valued neutrosophic soft interior operator (svnsi-operator) on $\mathcal{X}$ if it meets the next criteria, $\forall$, $e\in {E}$, $f_{_{A}}, g_{_{B}}\in \widehat{(\mathcal{X}, {E})}$ and $r, s\in\zeta$:

(${I}_{1}$) ${I}(e, \widehat{E}, r) = \widehat{E}$,

(${I}_{2}$) ${I}(e, f_{_{A}}, r)\preceq f_{_{A}}$,

(${I}_{3}$) if $f_{_{A}}\preceq g_{_{B}}$ and $r\leq s$ then ${I}(e, f_{_{A}}, r)\preceq{I}(e, g_{_{B}}, s)$,

(${I}_{4}$) ${I}(e), f_{_{A}}\sqcap g_{_{B}}, r\wedge s)\succeq{I}(e, f_{_{A}}, r)\sqcap{I}(e, g_{_{B}}, s)$,

(${I}_{5}$) ${I}(e, {I}(e, f_{_{A}}, r), r) = {I}(e, f_{_{A}}, r)$.

Definition 7. ^[16]. A map ${C}: {E}\times\widehat{(\mathcal{X}, {E})}\times \zeta_{0}\rightarrow \widehat{(\mathcal{X}, {E})}$ is said to be single-valued neutrosophic soft closure operator (svnsc-operator) on $\mathcal{X}$ if it meets the next criteria, $\forall$, $e\in {E}$, $f_{_{A}}, g_{_{B}}\in \widehat{(\mathcal{X}, {E})}$ and $r, s\in\zeta$:

(${C}_{1}$) ${C}(e, \widehat{\Phi}, r) = \widehat{\Phi}$,

(${C}_{2}$) ${C}(e, f_{_{A}}, r)\succeq f_{_{A}}$,

(${C}_{3}$) if $f_{_{A}}\preceq g_{_{B}}$ and $r\leq s$ then ${C}(e, f_{_{A}}, r)\preceq{C}(e, g_{_{B}}, s)$,

(${C}_{4}$) ${C}(e, f_{_{A}}\sqcup g_{_{B}}, r\wedge s)\preceq{C}(e, f_{_{A}}, r)\sqcup{C}(e, g_{_{B}}, s)$,

(${C}_{5}$) ${C}(e, {C}(e, f_{_{A}}, r), r)\preceq{C}(e, f_{_{A}}, r)$,

(${C}_{6}$) ${C}(e, f_{_{A}}, r) = [{I}(e, f^{c}_{_{A}}, r)]^{c}$.

3.   Single-valued neutrosophic soft soft uniform spaces

The main objective of this section is to define and discuss the concepts of single-valued neutrosophic soft uniformity (svns-uniformity), single-valued neutrosophic soft uniform base (svns-uniform base) and stratified single-valued neutrosophic soft uniform space (ssvns-uniform space). Several basic properties and theorems related to these concepts are explored.

In this section, we indicate that $\widehat{(\mathcal{X}\times\mathcal{X}, \text{E})}$ is the family of all svns-sets on $\mathcal{X}\times\mathcal{X}$ and $\zeta^{\mathcal{X}\times\mathcal{X}}$ are the sets of all svn-sets on $\mathcal{X}\times\mathcal{X}$. Additionally, for $\varrho\in\zeta$, $\bar{\varrho}(x, y) = \varrho$ for any $(x, y)\in\mathcal{X}\times\mathcal{X}$.

Definition 8. Let $\mathcal{X}$ be a set. A mappings $£^{\gamma}, £^{\pi}, £^{\varsigma}: {E}\rightarrow \zeta^{\widehat{(\mathcal{X}\times\mathcal{X}, {E})}}$ is called an svns-uniformity on $\mathcal{X}$ if it meets the next criteria:

$(£_{1})$ for any $e\in {E}$, there exists $\upsilon_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, {E})}$ such that $£^{\gamma}_{e}(\upsilon_{_{A}}) = 1$, $£^{\pi}_{e}(\upsilon_{_{A}}) = 0$, $£^{\varsigma}_{e}(\upsilon_{_{A}}) = 0$,

$(£_{2})$ if $\upsilon_{_{A}}\preceq \mu_{_{B}}$, then $£^{\gamma}_{e}(\upsilon_{_{A}})\leq£^{\gamma}_{e}(\mu_{_{B}})$, $£^{\pi}_{e}(\upsilon_{_{A}})\geq£^{\pi}_{e}(\mu_{_{B}})$, $£^{\varsigma}_{e}(\upsilon_{_{A}})\geq£^{\varsigma}_{e}(\mu_{_{B}})$,

$(£_{3})$ for every $\upsilon_{_{A}}, \mu_{_{B}}\in \widehat{(\mathcal{X}\times\mathcal{X}, {E})}$, then

$(£_{4}) \; (\top)_{\texttt{C}}\npreceq\upsilon_{_{A}}$ implies that $£^{\gamma}_{e}(\upsilon_{_{A}}) = 0$, $£^{\pi}_{e}(\upsilon_{_{A}}) = 1$, $£^{\varsigma}_{e}(\upsilon_{_{A}}) = 1$, where, $\forall \; e\in {E}$,

$(£_{5}) \; £^{\gamma}_{e}(\upsilon_{_{A}})\leq£^{\gamma}_{e}(\upsilon^{s}_{_{A}})$, $£^{\pi}_{e}(\upsilon_{_{A}})\geq£^{\pi}_{e}(\upsilon^{s}_{_{A}})$, $£^{\varsigma}_{e}(\upsilon_{_{A}})\geq£^{\varsigma}_{e}(\upsilon^{s}_{_{A}})$, where $\upsilon^{s}_{_{e}}(x, y) = \upsilon_{_{e}}(y, x)$ for every $e\in {E}$,

$(£_{6})$ for each $\upsilon_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, {E})}$, $e\in {E}$,

where $(\upsilon_{_{B}}\circ\mu_{_{B}}) = \bigvee\limits_{z\in\mathcal{X}}\{\upsilon_{e}(x, z)\wedge\mu_{e}(z, y)\}$ for each $x, y\in\mathcal{X}$.

A svns-uniformity $£^{\gamma}, £^{\pi}, £^{\varsigma}: {E}\rightarrow \zeta^{\widehat{(\mathcal{X}\times\mathcal{X}, {E})}}$ is called stratified if

$(£_{st}) \; £^{\gamma}_{e}(\widehat{E}_{\varrho}) = 1$, $£^{\pi}_{e}(\widehat{E}_{\varrho}) = 0$, $£^{\varsigma}_{e}(\widehat{E}_{\varrho}) = 0$, where $\upsilon_{_{ {E}}} = \widehat{E}_{\varrho}$ if $\upsilon_{ {e}} = \bar{\varrho} \; \forall$, $e\in {E}$.

After adding the last condition $(\mathcal{X}, £^{\gamma}, £^{\pi}, £^{\varsigma})$ is called ssvns-uniform space. Sometimes, we will write $£_{ {E}}^{\gamma\pi\varsigma}$ for $(£^{\gamma}, £^{\pi}, £^{\varsigma})$.

Let $£_{_{ {E}}}^{\gamma\pi\varsigma}$ and $£_{_{ {E}}}^{\star\gamma\pi\varsigma}$ be two svns-uniformities on $\mathcal{X}$. $£_{_{ {E}}}^{\gamma\pi\varsigma}$ is finer than $£_{_{ {E}}}^{\star\gamma\pi\varsigma}$ ($£_{_{ {E}}}^{\star\gamma\pi\varsigma}$ is coarser than $£_{_{ {E}}}^{\gamma\pi\varsigma}$), indicated by $£_{_{ {E}}}^{\star\gamma\pi\varsigma}\preceq£_{_{ {E}}}^{\gamma\pi\varsigma}$ provided

Remark 2. Suppose that $(\mathcal{X}, £_{ {E}}^{\gamma\pi\varsigma})$ is an svns-uniform space. Then, by using the two conditions $(£_{1})$ and $(£_{2})$, we obtain, $£^{\gamma}_{e}(\widehat{E}) = 1$, $£^{\pi}_{e}(\widehat{E}) = 0$, $£^{\varsigma}_{e}(\widehat{E}) = 0$ because $\upsilon_{_{A}}\preceq\widehat{E}$ for every $e\in {E}$, $\upsilon_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, {E})}$.

Theorem 1. Let $(\mathcal{X}, £_{ {E}}^{\gamma\pi\varsigma})$ be an svns-uniform space. Define for any $e\in {E}$, $\upsilon_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, {E})}$.

Then, $(£_{st}^{\gamma\pi\varsigma})_{ {E}}$ is the coarsest ssvns-uniformity which is finer than $£^{\gamma\pi\varsigma}_{ {E}}$.

Proof. $(£_{1})$ There exists $\upsilon_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, \text{E})}$ such that $£^{\gamma}_{e}(\upsilon_{_{A}}) = 1$, $£^{\pi}_{e}(\upsilon_{_{A}}) = 0$, $£^{\varsigma}_{e}(\upsilon_{_{A}}) = 0$ for every $e\in \text{E}$. Since $\upsilon_{_{A}}\sqcap\widehat{E}_{\varrho}\preceq\upsilon_{_{A}}, \; then\; (£_{st}^{\gamma})_{e}(\upsilon_{_{A}}) = 1, \; (£_{st}^{\pi})_{e}(\upsilon_{_{A}}) = 0, \; (£_{st}^{\varsigma})_{e}(\upsilon_{_{A}}) = 0$.

$(£_{2})$ Direct from the definition.

$(£_{3})$ Let there exist $(\upsilon_{1})_{_{A}}, (\upsilon_{2})_{_{B}}\in \widehat{(\mathcal{X}\times\mathcal{X}, \text{E})}$ such that for every $e\in \text{E}$,

By using the definition of $(£_{st}^{\gamma\pi\varsigma})_{ \text{E}}$, then there exists $(\mu_{1})_{_{C}}, (\mu_{2})_{_{D}}\in \widehat{(\mathcal{X}\times\mathcal{X}, \text{E})}$, $\varrho_{1}, \varrho_{2}\in\zeta$ with $(\mu_{1})_{_{C}}\sqcap\widehat{E}_{\varrho_{1}}\preceq(\upsilon_{1})_{_{A}}$, $(\mu_{2})_{_{D}}\sqcap\widehat{E}_{\varrho_{2}}\preceq(\upsilon_{2})_{_{B}}$ such that

Otherwise, $(\mu_{1})_{_{C}}\sqcap(\mu_{2})_{_{D}}\sqcap\widehat{E}_{\varrho_{1}}\sqcap\widehat{E}_{\varrho_{2}}\preceq(\upsilon_{1})_{_{A}}\sqcap(\upsilon_{2})_{_{B}}$. Then, we have

This is a contradiction. Consequently, $(£_{3})$ holds.

$(£_{4})$ Direct from the definition.

$(£_{5})$ Let

$\forall$, $e\in \text{E}$, $\upsilon_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, \text{E})}$. By using the definition of $(£_{st}^{\gamma\pi\varsigma})_{ \text{E}}$, there exists $\mu_{_{B}}\in \widehat{(\mathcal{X}\times\mathcal{X}, \text{E})}$, $\varrho\in\zeta$ with $\mu_{_{B}}\sqcap\widehat{E}_{\varrho}\preceq\upsilon_{_{A}}$, such that

Since $£^{\gamma\pi\varsigma}_{ \text{E}}$ is svns-uniformity, then

It follows that

On the other hand, $\mu^{s}_{_{B}}\sqcap\widehat{E}_{\varrho}\preceq\upsilon^{s}_{_{A}}$. Hence, for each $e\in \text{E}$

This is a contradiction. Therefore, $(£_{5})$ holds.

$(£_{6})$ Suppose that

for any $\upsilon_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, \text{E})}$. From the definition of $(£_{st}^{\gamma\pi\varsigma})_{ \text{E}}$, there exists $\mu_{_{B}}\in \widehat{(\mathcal{X}\times\mathcal{X}, \text{E})}$, $\varrho\in\zeta$ with $\mu_{_{B}}\sqcap\widehat{E}_{\varrho}\preceq\upsilon_{_{A}}$ such that

Since $£^{\gamma\pi\varsigma}_{ \text{E}}$ is svns-uniformity on $\mathcal{X}$, then

That means, there is $\sigma_{_{D}}\in \widehat{(\mathcal{X}\times\mathcal{X}, \text{E})}$ such that $\sigma_{D}\sqcap\sigma_{D}\preceq\mu_{_{B}}$ and that

On the other hand,

which means that there is $(\upsilon_{1})_{_{C}} = \sigma_{D}\sqcap\widehat{E}_{\varrho}$ with $(\upsilon_{1})_{_{C}}\circ(\upsilon_{1})_{_{C}}\preceq\upsilon_{_{A}}$,

It is a contradiction. Thus, ($£_{6}$) holds.

($£_{st}$) Since $\widehat{E}_{\varrho}\sqcap\widehat{E}_{1} = \widehat{E}_{\varrho}$ for each $\varrho\in\zeta$, then $(£_{st}^{\gamma})_{\widehat{E}} = 1$, $(£_{st}^{\pi})_{\widehat{E}} = 0$ and $(£_{st}^{\varsigma})_{\widehat{E}} = 0$. Therefore, $(£_{st}^{\gamma\pi\varsigma})_{e}$ is stratified.

For each $\upsilon_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, \text{E})}$, $\upsilon_{_{A}}\sqcap\widehat{E}_{1} = \upsilon_{_{A}}$, we have for each $e\in \text{E}$

Hence, $(£_{st}^{\gamma\pi\varsigma})_{ \text{E}}$ is finer than $£^{\gamma\pi\varsigma}_{ \text{E}}$.

Finally, consider $£^{\star\gamma\pi\varsigma}_{ \text{E}}$ is an ssvns-uniformity finer than $£^{\gamma\pi\varsigma}_{ \text{E}}$. Let there exists $\upsilon_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, \text{E})}$ such that

From the definition of $\left\{(£_{st}^{\gamma})_{e}(\upsilon_{_{A}}), (£_{st}^{\pi})_{e}(\upsilon_{_{A}}), (£_{st}^{\varsigma})_{e}(\upsilon_{_{A}})\right\}$, there exists $\mu_{_{B}}\in \widehat{(\mathcal{X}\times\mathcal{X}, \text{E})}$, $\varrho\in\zeta$ with $\mu_{_{B}}\sqcap\widehat{E}_{\varrho}\preceq\upsilon_{_{A}}$ and

Since $£^{\star\gamma\pi\varsigma}_{ \text{E}}$ is stratified, then

It is a contradiction. Hence,

for each $\upsilon_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, \text{E})}$, $e\in \text{E}$. Hence, $(£_{st}^{\gamma\pi\varsigma})_{ \text{E}}$ is the coarsest ssvns-uniformity which is finer than $£^{\gamma\pi\varsigma}_{ \text{E}}$. □

Remark 3. Let $\hbar^{\gamma}, \hbar^{\pi}, \hbar^{\varsigma}: {E}\rightarrow \zeta^{\widehat{(\mathcal{X}\times\mathcal{X}, {E})}}$ be a mapping and $\upsilon_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, {E})}$. Let us define $\langle\hbar^{\gamma}_{e}\rangle$, $\langle\hbar^{\pi}_{e}\rangle$ and $\langle\hbar^{\varsigma}_{e}\rangle$ as follows for each $e\in {E}$:

Definition 9. A mappings $\hbar^{\gamma}, \hbar^{\pi}, \hbar^{\varsigma}: {E}\rightarrow \zeta^{\widehat{(\mathcal{X}\times\mathcal{X}, {E})}}$ is called a svns-uniform base on $\mathcal{X}$ if it meets the next criteria:

$(\hbar_{1})$ There exists $\upsilon_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, {E})}$ such that $\hbar^{\gamma}_{e}(\upsilon_{_{A}}) = 1$, $\hbar^{\pi}_{e}(\upsilon_{_{A}}) = 0$, $\hbar^{\varsigma}_{e}(\upsilon_{_{A}}) = 0$, for all $e\in {E}$,

$(\hbar_{2})$ for each $\upsilon_{_{A}}, \mu_{_{B}}\in \widehat{(\mathcal{X}\times\mathcal{X}, {E})}$, $e\in {E}$, such that

$(\hbar_{3})$ If $(\top)_{A}\npreceq\upsilon_{_{B}}$, then $\hbar^{\gamma}_{e}(\upsilon_{_{B}}) = 0$, $\hbar^{\pi}_{e}(\upsilon_{_{B}}) = 1$, $\hbar^{\varsigma}_{e}(\upsilon_{_{B}}) = 1$.

$(\hbar_{4})$ For every $\upsilon_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, {E})}$, $\langle\hbar^{\gamma}_{e}\rangle(\upsilon^{s}_{_{A}})\geq\hbar^{\gamma}_{e}(\upsilon_{_{A}})$, $\langle\hbar^{\pi}_{e}\rangle(\upsilon^{s}_{_{A}})\leq\hbar^{\pi}_{e}(\upsilon_{_{A}})$ and $\langle\hbar^{\varsigma}_{e}\rangle(\upsilon^{s}_{_{A}})\leq\hbar^{\varsigma}_{e}(\upsilon_{_{A}})$,

$(\hbar_{5})$ For every $\upsilon_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, {E})}$,

A svns-uniform base $(\hbar^{\gamma}, \hbar^{\pi}, \hbar^{\varsigma})$ is said to be stratified if and only if $(\hbar^{\gamma}, \hbar^{\pi}, \hbar^{\varsigma})$ satisfies

$(\hbar_{st}) \; \hbar^{\gamma}_{e}(\widehat{E}_{\varrho}) = 1$, $\hbar^{\pi}_{e}(\widehat{E}_{\varrho}) = 0$, $\hbar^{\varsigma}_{e}(\widehat{E}_{\varrho}) = 0$, $\forall \; \varrho\in\zeta$, $e\in {E}$.

In this case $(\hbar^{\gamma}, \hbar^{\pi}, \hbar^{\varsigma})$ is stratified single-valued neutrosophic soft uniform base (for short, ssvns-uniform base). Sometimes, we will write $\hbar_{ {E}}^{\gamma\pi\varsigma}$ for $(\hbar^{\gamma}, \hbar^{\pi}, \hbar^{\varsigma})$.

Let $\hbar_{_{ {E}}}^{\gamma\pi\varsigma}$ and $\hbar_{_{ {E}}}^{\star\gamma\pi\varsigma}$ be two svns-uniform bases on $\mathcal{X}$. Then, $\hbar_{_{ {E}}}^{\gamma\pi\varsigma}$ is finer than $\hbar_{_{ {E}}}^{\star\gamma\pi\varsigma}$ ($\hbar_{_{ {E}}}^{\star\gamma\pi\varsigma}$ is coarser than $\hbar_{_{ {E}}}^{\gamma\pi\varsigma}$), denoted by $\hbar_{_{ {E}}}^{\star\gamma\pi\varsigma}\preceq\hbar_{_{ {E}}}^{\gamma\pi\varsigma}$ provided

for each $e\in {E}$, $\upsilon_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, {E})}$. Obviously, all svns-uniformity $£^{\gamma\pi\varsigma}_{ {E}}$ on $\mathcal{X}$ is a svns- uniform base with $\langle£^{\gamma\pi\varsigma}_{ {E}}\rangle = £^{\gamma\pi\varsigma}_{ {E}}$.

Theorem 2. Let $\hbar^{\gamma\pi\varsigma}_{ {E}}$ be a svns-uniform base on $\mathcal{X}$, define the mappings $\hbar^{\gamma}, \hbar^{\pi}, \hbar^{\varsigma}: {E}\rightarrow \zeta^{\widehat{(\mathcal{X}\times\mathcal{X}, {E})}}$, for any $\upsilon_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, {E})}$, $e\in {E}$ as follows:

Then,

$(1) \; (\hbar_{st}^{\gamma\pi\varsigma})_{ {E}}$ is the coarsest ssvns-uniform base which is finer than $\hbar^{\gamma\pi\varsigma}_{ {E}}$,

$(2) \; \langle(\hbar_{st}^{\gamma\pi\varsigma})_{ {E}}\rangle = \langle\hbar_{ {E}}^{\gamma\pi\varsigma}\rangle_{st}$.

Proof. (1) Similar to Theorem 1.

(2) It becomes clear to us from (1), that

Conversely, let

for some $\upsilon_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, \text{E})}$. By the concept of $\langle(\hbar_{st}^{\gamma\pi\varsigma})_{ \text{E}}\rangle$, there exists $\mu_{_{B}}\in \widehat{(\mathcal{X}\times\mathcal{X}, \text{E})}$ with $\mu_{_{B}}\preceq\upsilon_{_{A}}$ such that

By the concept of $\langle\hbar_{ \text{E}}^{\gamma\pi\varsigma}\rangle_{st}$, there exists $\sigma_{_{C}}\in \widehat{(\mathcal{X}\times\mathcal{X}, \text{E})}$, $\varrho\in\zeta$ with $\sigma_{_{C}}\sqcap\widehat{E}_{\varrho}\preceq\mu_{_{B}}$ such that

On the other hand, $\sigma_{_{C}}\sqcap\widehat{E}_{\varrho}\preceq\upsilon_{_{A}}$ implies that

It is a contradiction. Hence, $\langle\hbar_{e}^{\gamma}\rangle_{st}(\upsilon_{_{A}})\geq\langle(\hbar_{st}^{\gamma})_{e}\rangle(\upsilon_{_{A}})$, $\langle\hbar_{e}^{\pi}\rangle_{st}(\upsilon_{_{A}})\leq\langle(\hbar_{st}^{\pi})_{e}\rangle(\upsilon_{_{A}})$, $\langle\hbar_{e}^{\varsigma}\rangle_{st}(\upsilon_{_{A}})\leq\langle(\hbar_{st}^{\varsigma})_{e}\rangle(\upsilon_{_{A}})$, and $\langle(\hbar_{st}^{\gamma\pi\varsigma})_{ \text{E}}\rangle = \langle\hbar_{ \text{E}}^{\gamma\pi\varsigma}\rangle_{st}$. □

Theorem 3. Let $(\mathcal{X}, £^{\gamma\pi\varsigma}_{ {E}})$ be an svns-uniform space. For all $f_{_{B}}\in\widehat{(\mathcal{X}, {E})}$ and $\upsilon_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, {E})}$, the image $\upsilon_{_{A}}[f_{_{B}}]$ of $f_{_{B}}$ with respect to $\upsilon_{_{A}}$ is the svns of $\mathcal{X}$ defined by

For $f_{_{C}}, (f_{_{D}})_{j}\in\widehat{(\mathcal{X}, {E})}$, $\upsilon_{_{A}}, \mu_{_{B}}\in \widehat{(\mathcal{X}\times\mathcal{X}, {E})}$, we have:

$(1) \; f_{_{C}}\preceq\upsilon_{_{A}}[f_{_{C}}]$ whenever $£^{\gamma}_{e}(\upsilon_{_{A}}) > 0$, $£^{\pi}_{e}(\upsilon_{_{A}}) < 1$, $£^{\varsigma}_{e}(\upsilon_{_{A}}) < 1$,

$(2) \; \upsilon_{_{A}}\preceq\upsilon_{_{A}}\circ\upsilon_{_{A}}$ whenever $£^{\gamma}_{e}(\upsilon_{_{A}}) > 0$, $£^{\pi}_{e}(\upsilon_{_{A}}) < 1$, $£^{\varsigma}_{e}(\upsilon_{_{A}}) < 1$,

$(3) \; (\mu_{_{B}}\circ\upsilon_{_{A}})[f_{_{C}}] = \mu_{_{B}}[\upsilon_{_{A}}[f_{_{C}}]]$,

$(4) \; \upsilon_{_{A}}[\bigsqcup\limits_{j}(f_{_{D}})_{j}] = \bigsqcup\limits_{j}\upsilon_{_{A}}[(f_{_{D}})_{j}]$,

$(5) \; (\upsilon_{_{A}}\sqcap\mu_{_{B}})[(f_{_{D}})_{1}\sqcap (f_{_{D}})_{2}]\preceq\upsilon_{_{A}}[(f_{_{D}})_{1}]\sqcap \mu_{_{B}}[(f_{_{D}})_{2}]$,

$(6) \; (\upsilon_{_{A}}\sqcup\mu_{_{B}})[(f_{_{D}})_{1}\sqcup (f_{_{D}})_{2}]\preceq\upsilon_{_{A}}[(f_{_{D}})_{1}]\sqcup \mu_{_{B}}[(f_{_{D}})_{2}]$,

$(7) \; \upsilon_{_{A}}[(\upsilon^{s}_{_{A}}[f_{_{C}}])^{c}]\preceq f^{c}_{_{C}}$.

Proof. Obvious. □

Theorem 4. Let $\hbar^{\gamma\pi\varsigma}_{ {E}}$ be a svns-uniform base on $\mathcal{X}$. define the operator ${I}_{_{\hbar^{\gamma\pi\varsigma}}}: {E}\times\widehat{(\mathcal{X}, {E})}\times \zeta_{_{0}}\rightarrow \widehat{(\mathcal{X}, {E})}$ as next for every $e\in {E}$, $r\in\zeta$, $f_{_{B}}\in \widehat{(\mathcal{X}, {E})}$,

Then, ${I}_{_{\hbar^{\gamma\pi\varsigma}}}$ is an svnsi-operator on $\mathcal{X}$.

Proof. $(\texttt{I}_{1})$ Since $\widehat{E} = \upsilon_{_{E}}[\widehat{E}]$, for all $\hbar^{\gamma}_{e}(\upsilon_{_{E}})\geq r, \; \hbar^{\pi}_{e}(\upsilon_{_{E}})\leq 1-r, \; \hbar^{\varsigma}_{e}(\upsilon_{_{E}})\leq 1-r$, then $\texttt{I}_{_{\hbar^{\gamma\pi\varsigma}}}(e, \widehat{E}, r) = \widehat{E}$.

$(\texttt{I}_{2})$ Whenever $\mathcal{R}_{_{C}}\preceq\upsilon_{_{A}}[\mathcal{R}_{_{C}}]\preceq f_{_{B}}$, $\forall \; \hbar^{\gamma}_{e}(\upsilon_{_{A}})\geq r, \; \hbar^{\pi}_{e}(\upsilon_{_{A}})\leq 1-r, \; \hbar^{\varsigma}_{e}(\upsilon_{_{A}})\leq 1-r$, we get that $\texttt{I}_{_{\hbar^{\gamma\pi\varsigma}}}(e, f_{_{B}}, r)\preceq f_{_{B}}$ for all $f_{_{B}}\in \widehat{(\mathcal{X}, {E})}$.

$(\texttt{I}_{3})$ Clearly, $\texttt{I}_{_{\hbar^{\gamma\pi\varsigma}}}(e, f_{_{B}}, r)\preceq\texttt{I}_{_{\hbar^{\gamma\pi\varsigma}}}(e, \mathcal{R}_{_{D}}, s)$ for every $f_{_{B}}\preceq\mathcal{R}_{_{D}}$, $f_{_{B}}, \mathcal{R}_{_{D}}\in \widehat{(\mathcal{X}, {E})}$ and $r\leq s$.

$(\texttt{I}_{4})$ Assume that

Then, there exists $(\mathcal{R}_{_{D}})_{1}, (\mathcal{R}_{_{D}})_{2}\in \widehat{(\mathcal{X}, {E})}$ with $\upsilon_{_{A}}[(\mathcal{R}_{_{D}})_{1}]\preceq (f_{_{C}})_{1} \; \mu_{_{B}}[(\mathcal{R}_{_{D}})_{2}]\preceq (f_{_{C}})_{2}$ and

such that

Since

we get than

Then,

This is a contradiction. Consequently, $(\texttt{I}_{4})$ holds.

$(\texttt{I}_{5})$ Assume that $\texttt{I}_{_{\hbar^{\gamma\pi\varsigma}}}(e, f_{_{C}}, r)\npreceq\texttt{I}_{_{\hbar^{\gamma\pi\varsigma}}}(e, \texttt{I}_{_{\hbar^{\gamma\pi\varsigma}}}(e, f_{_{C}}, r), r)$. By using the definition of $\texttt{I}_{_{\hbar^{\gamma\pi\varsigma}}}(e, f_{_{C}}, r)$, there exists $\upsilon_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, {E})}$ and $\mathcal{R}_{_{D}}\in \widehat{(\mathcal{X}, {E})}$, such that

and $\mathcal{R}_{_{D}}\npreceq\texttt{I}_{_{\hbar^{\gamma\pi\varsigma}}}(e, \texttt{I}_{_{\hbar^{\gamma\pi\varsigma}}}(e, f_{_{C}}, r), r)$. Otherwise, since

there exists $\mu_{_{B}}\in \widehat{(\mathcal{X}\times\mathcal{X}, \text{E})}$ with $\mu_{_{B}}\circ\mu_{_{B}}\preceq\upsilon_{_{A}}$ such that

By using the definition of $\texttt{I}_{_{\hbar^{\gamma\pi\varsigma}}}(e, f_{_{C}}, r)$, we obtain $\mu_{_{B}}[\mathcal{R}_{_{D}}]\preceq\texttt{I}_{_{\hbar^{\gamma\pi\varsigma}}}(e, f_{_{C}}, r)$. By the concept of $\texttt{I}_{_{\hbar^{\gamma\pi\varsigma}}}(e, \texttt{I}_{_{\hbar^{\gamma\pi\varsigma}}}(e, f_{_{C}}, r), r)$, it follows that

This is a contradiction. Consequently, $(\texttt{I}_{5})$ holds. □

Theorem 5. Let $\hbar^{\gamma\pi\varsigma}_{ {E}}$ be a svns-uniform base on $\mathcal{X}$. Define the operator $\texttt{C}_{_{\hbar^{\gamma\pi\varsigma}}}: {E}\times\widehat{(\mathcal{X}, {E})}\times \zeta_{_{0}}\rightarrow \widehat{(\mathcal{X}, {E})}$ as next for every $e\in {E}$, $f_{_{B}}\in \widehat{(\mathcal{X}, {E})}$, $r\in\zeta$,

Then, $\texttt{C}_{_{\hbar^{\gamma\pi\varsigma}}}$ is a svnsc-operator on $\mathcal{X}$.

Proof. $(\texttt{C}_{1})$ Since $\widehat{\Phi} = \upsilon_{_{A}}[\widehat{\Phi}]$, for all $\hbar^{\gamma}_{e}(\upsilon_{_{A}})\geq r, \; \hbar^{\pi}_{e}(\upsilon_{_{A}})\leq 1-r, \; \hbar^{\varsigma}_{e}(\upsilon_{_{A}})\leq 1-r$, then $\texttt{C}_{_{\hbar^{\gamma\pi\varsigma}}}(e, \widehat{\Phi}, r) = \widehat{\Phi}$.

$(\texttt{C}_{2})$ Whenever $\mathcal{R}_{_{C}}\preceq\upsilon_{_{A}}[\mathcal{R}_{_{C}}]\preceq f_{_{B}}$, for all $\hbar^{\gamma}_{e}(\upsilon_{_{A}})\geq r, \; \hbar^{\pi}_{e}(\upsilon_{_{A}})\leq 1-r, \; \hbar^{\varsigma}_{e}(\upsilon_{_{A}})\leq 1-r$, we get that $\texttt{C}_{_{\hbar^{\gamma\pi\varsigma}}}(e, f_{_{B}}, r)\succeq f_{_{B}}$ for each $f_{_{B}}\in \widehat{(\mathcal{X}, {E})}$.

$(\texttt{C}_{3})$ It is established that $\texttt{C}_{_{\hbar^{\gamma\pi\varsigma}}}(e, f_{_{B}}, r)\preceq\texttt{C}_{_{\hbar^{\gamma\pi\varsigma}}}(e, \mathcal{R}_{_{D}}, s)$ for every $f_{_{B}}\preceq\mathcal{R}_{_{D}}$, $f_{_{B}}, \mathcal{R}_{_{D}}\in \widehat{(\mathcal{X}, {E})}$ and $r\leq s$.

$(\texttt{C}_{4})$ Assume that

Then, there exists $r, s\in\zeta_{_{0}}$, $\upsilon_{_{A}}, \mu_{_{B}}\in\widehat{(\mathcal{X}\times\mathcal{X}, \text{E})}$ with

such that

Since $\hbar^{\gamma}_{e}(\upsilon_{_{A}}\sqcup\mu_{_{B}})\geq\hbar^{\gamma}_{e}(\upsilon_{_{A}})\sqcap\hbar^{\gamma}_{e}(\mu_{_{B}})\geq r\wedge s$, $\hbar^{\pi}_{e}(\upsilon_{_{A}}\sqcup\mu_{_{B}})\leq\hbar^{\pi}_{e}(\upsilon_{_{A}})\sqcup\hbar^{\pi}_{e}(\mu_{_{B}})\leq 1-(r\vee s)$, $\hbar^{\varsigma}_{e}(\upsilon_{_{A}}\sqcup\mu_{_{B}})\leq\hbar^{\varsigma}_{e}(\upsilon_{_{A}})\sqcup\hbar^{\varsigma}_{e}(\mu_{_{B}})\leq 1-(r\vee s)$ and $(\upsilon_{_{A}}\sqcup\mu_{_{B}})^{s}[f_{_{C}}\sqcup\mathcal{R}_{_{D}}]\preceq\upsilon_{_{A}}^{s}[f_{_{C}}]\sqcup\mu_{_{B}}^{s}[\mathcal{R}_{_{D}}]$, then $\texttt{C}_{_{\hbar^{\gamma\pi\varsigma}}}(e, f_{_{C}}\sqcup \mathcal{R}_{_{D}}, r\wedge s)\preceq\upsilon_{_{A}}^{s}[f_{_{C}}]\sqcup\mu_{_{B}}^{s}[\mathcal{R}_{_{D}}]$. It is a contradiction. Thus, $(\texttt{C}_{4})$ holds.

$(\texttt{C}_{5})$ Assume that there exists $r\in\zeta_{0}$, $e\in \text{E}$, $f_{_{C}}\in \widehat{(\mathcal{X}, \text{E})}$, such that

Using the concept of $\texttt{C}_{_{\hbar^{\gamma\pi\varsigma}}}(e, f_{_{C}}, r)$, there exist $\upsilon_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, \text{E})}$ with

such that $\texttt{C}_{_{\hbar^{\gamma\pi\varsigma}}}(e, \texttt{C}_{_{\hbar^{\gamma\pi\varsigma}}}(e, f_{_{C}}, r), r)\npreceq\upsilon_{_{A}}^{s}[f_{_{C}}]$. Otherwise, from $(\hbar_{5})$, we have

which leads to the existence of $\mu_{_{B}}\in\widehat{(\mathcal{X}\times\mathcal{X}, \text{E})}$ with $\mu_{_{B}}\circ\mu_{_{B}}\preceq\upsilon_{_{A}}$ and

It follows that

It is a contradiction. Thus, $(\texttt{C}_{5})$ holds.

$(\texttt{C}_{6})$ We want, for each $e\in \text{E}$, $f_{_{C}}\in \widehat{(\mathcal{X}, \text{E})}$, $r\in\zeta_{0}$, to verify that $\texttt{C}_{_{\hbar^{\gamma\pi\varsigma}}}(e, f_{_{C}}, r) = (\texttt{I}_{_{\hbar^{\gamma\pi\varsigma}}}(e, f^{c}_{_{C}}, r))^{c}$. This means that we need to prove it:

Since $\upsilon_{_{A}}[(\upsilon^{s}_{_{A}}[f_{_{C}}])^{c}]\preceq f_{_{C}}^{c}$, from (7) in Theorem 3, we obtain

Since $(\upsilon_{_{A}}[\mathcal{R}_{_{D}}])^{c}\succeq f_{_{C}}$, we obtain $\upsilon^{s}_{_{A}}[f_{_{C}}]\preceq\upsilon^{s}_{_{A}}[(\upsilon_{_{A}}[\mathcal{R}_{_{D}}])^{c}]$. Then,

Thus, $(\texttt{C}_{6})$ holds. □

4.   Single-valued neutrosophic soft topologies induced by a single-valued neutrosophic soft uniformity

In this section, we study several single-valued neutrosophic soft topologies induced by a single-valued neutrosophic soft uniform structure. We have proved that single-valued neutrosophic soft uniform base and single-valued neutrosophic soft uniform space are single-valued neutrosophic soft topological spaces.

Theorem 6. Let $\hbar^{\gamma\pi\varsigma}_{ {E}}$ be an svns-uniform base on $\mathcal{X}$, define the mappings $\mathfrak{T}_{\hbar}^{\gamma}: {E}\rightarrow \zeta^{\widehat{(\mathcal{X}, {E})}}$, $\mathfrak{T}_{\hbar}^{\pi}: {E}\rightarrow \zeta^{\widehat{(\mathcal{X}, {E})}}$, $\mathfrak{T}_{\hbar}^{\varsigma}: {E}\rightarrow \zeta^{\widehat{(\mathcal{X}, {E})}}$ as follows for each $e\in {E}$, $r\in\zeta_{0}$, $f_{_{A}}\in \widehat{(\mathcal{X}, {E})}$,

Then, $\mathfrak{T}_{\hbar}^{\gamma\pi\varsigma}$ is an svnst on $\mathcal{X}$.

Proof. $(\mathfrak{T}_{1})$ Since $\texttt{I}_{_{\hbar^{\gamma\pi\varsigma}}}(e, \widehat{E}, r) = \widehat{E}$ and $\texttt{I}_{_{\hbar^{\gamma\pi\varsigma}}}(e, \widehat{\Phi}, r) = \widehat{\Phi}$ for each $r\in\zeta_{0}$, $e \in \text{E}$, then

$(\mathfrak{T}_{2})$ To prove the second condition, we follow as follows:

$(\mathfrak{T}_{3})$ Assume that there exists a collection $\{(f_{_{A}})_{j}: j\in\Gamma\}$ such that

For every $j\in\Gamma$, there exist $r_{j}\in\zeta_{0}$ such that $(f_{_{A}})_{j}\preceq\texttt{I}_{_{\hbar^{\gamma\pi\varsigma}}}(e, f_{_{A}}, r)$ and that

Putting $r = \bigwedge\limits_{j\in \Gamma} r_{j}$ and $1-r = \bigvee\limits_{j\in \Gamma} (1-r)_{j}$, from Theorem 4, we get that

It follows that

It is a contradiction. Thus, $\mathfrak{T}_{3}$ holds. □

Definition 10. Let $f_{_{B}}\in \widehat{(\mathcal{X}, {E})}$ and $\upsilon_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, {E})}$. Define $\upsilon^{f_{_{B}}}_{_{A}}\in \widehat{(\mathcal{X}\times\mathcal{X}, {E})}$, for each $e\in A\cap B$ related with $f_{_{B}}$ by

Theorem 7. Let $(\mathcal{X}, £_{ {E}}^{\gamma\pi\varsigma})$ be an svns-uniform space, define the mappings $\mathfrak{T}_{£}^{*\gamma}, \mathfrak{T}_{£}^{*\pi}, \mathfrak{T}_{£}^{*\varsigma}: {E}\rightarrow \zeta^{\widehat{(\mathcal{X}, {E})}}$ as follows:

Then, $\mathfrak{T}_{£}^{*\gamma\pi\varsigma}$ is an svnst on $\mathcal{X}$.

Proof. $({\mathfrak{T}_{1}}) \; (\mathfrak{T}_{£}^{*\gamma})_{e}(\widehat{\Phi}) = 1$, $(\mathfrak{T}_{£}^{*\pi})_{e}(\widehat{\Phi}) = 0$, $(\mathfrak{T}_{£}^{*\varsigma})_{e}(\widehat{\Phi}) = 0$ and $(\mathfrak{T}_{£}^{*\gamma})_{e}(\widehat{E}) = £^{\gamma}_{_{e}}(\upsilon^{\widehat{E}}_{_{e}}) = 1$, $(\mathfrak{T}_{£}^{*\pi})_{e}(\widehat{E}) = £^{\pi}_{_{e}}(\upsilon^{\widehat{E}}_{_{e}}) = 0$, $(\mathfrak{T}_{£}^{*\varsigma})_{e}(\widehat{E}) = £^{\varsigma}_{_{e}}(\upsilon^{\widehat{E}}_{_{e}}) = 0$.

$({\mathfrak{T}_{2}})$ Since $\upsilon^{f_{_{B}}}_{_{A}}\sqcap\upsilon^{\mathcal{R}_{_{C}}}_{_{A}} = \upsilon^{f_{_{B}}\sqcap\mathcal{R}_{_{C}}}_{_{A}}$ for every $f_{_{B}}, \mathcal{R}_{_{C}}\in \widehat{(\mathcal{X}, \text{E})}$, by $(£_{3})$, we have

Thus,

$(\mathfrak{T}_{3})$ Similar to the proof in $(\mathfrak{T}_{3})$ from Theorem 6. □

Theorem 8. Let $(\mathcal{X}, £_{ {E}}^{\gamma\pi\varsigma})$ be a svns-uniform space, define the mappings $\mathfrak{T}_{£}^{\star\star\gamma}, \mathfrak{T}_{£}^{\star\star\pi}, \mathfrak{T}_{£}^{\star\star\varsigma}: {E}\rightarrow \zeta^{\widehat{(\mathcal{X}, {E})}}$ as follows:

Then, $\mathfrak{T}_{£}^{\star\star\gamma\pi\varsigma}$ is an svnst on $\mathcal{X}$, where $(\upsilon_{_{A}}[x])(y)\preceq \upsilon_{_{A}}(y, x)$ for all $e\in A$.

Proof. $(\mathfrak{T}_{1})$ Obvious.

$(\mathfrak{T}_{2})$ Assume that

Then, there exists $\upsilon_{_{A}}, \mu_{_{B}}$ with $\upsilon_{_{A}}[x]\preceq (f_{_{D}})_{_{1}}$, $\mu_{_{B}}[x]\preceq (f_{_{D}})_{_{2}}$ such that

This results in $(\upsilon_{_{A}}\sqcap\mu_{_{B}})[x]\preceq (f_{_{D}})_{_{1}}\sqcap (f_{_{D}})_{_{2}}$ such that

It is a contradiction. Thus,

Likewise, we can establish through a similar line of reasoning that

$(\mathfrak{T}_{3})$ For $e\in \text{E}$

In a similar vein, we can demonstrate through a parallel line of reasoning that

Therefore, $\mathfrak{T}_{£}^{\star\star\gamma\pi\varsigma}$ is an svnst on $\mathcal{X}$. □

5.   Single-valued neutrosophic soft uniformly continuous mappings

In this section, we obtain crucial results in introducing and characterizing single-valued neutrosophic soft uniformly continuous, on single-valued neutrosophic soft uniformly topological spaces. Moreover, the relationship between single-valued neutrosophic soft uniformly continuous and single-valued neutrosophic soft continuous is studied.

Definition 11. Let $(\mathcal{X}, £_{ {E}}^{\gamma\pi\varsigma})$ and $(\mathcal{G}, £_{ {R}}^{\star\gamma\pi\varsigma})$ be two svns-uniform spaces and $\psi:\mathcal{X}\rightarrow \mathcal{G}$ and $\vartheta: {E}\rightarrow \mathcal{R}$ be two mappings. Then, an svns-map $\psi_{_{\vartheta}}:\widehat{(\mathcal{X}\times\mathcal{X}, {E})}\rightarrow \widehat{(\mathcal{G}\times\mathcal{G}, \mathcal{R})}$ is called single-valued neutrosophic soft uniformly continuous (svns-uniformly continuous) if

for each $\mu_{_{B}}\in\widehat{(\mathcal{G}\times\mathcal{G}, \mathcal{R})}$, $e\in {E}$.

Proposition 1. Let $(\mathcal{X}, £_{_{E}}^{\gamma\pi\varsigma})$ and $(\mathcal{G}, \mathcal{F}_{_{R}}^{\gamma\pi\varsigma})$ be svns-uniform spaces. If $\psi_{_{\vartheta}}:(\mathcal{X}, £^{\gamma\pi\varsigma})\rightarrow (\mathcal{G}, \mathcal{F}^{\gamma\pi\varsigma})$ is svns-uniformly continuous, then $\psi_{_{\vartheta}}:(\mathcal{X}, (£_{st}^{\gamma\pi\varsigma})\rightarrow (\mathcal{G}, \mathcal{F}_{st}^{\gamma\pi\varsigma})$ is svns-uniformly continuous.

Proof. To prove this theorem, we need to prove that

for each $\upsilon_{_{A}}\in\widehat{(\mathcal{G}\times\mathcal{G}, \mathcal{R})}$, $e\in \text{E}$.

Assume that

From the concept of $(\mathcal{F}_{st}^{\star\gamma\pi\varsigma})_{_{\vartheta(e)}}(\upsilon_{_{A}})$, there exists $\mu_{_{B}}\in\widehat{(\mathcal{G}\times\mathcal{G}, \mathcal{R})}$, $e\in \text{E}$, $\varrho\in\zeta$ with $\mu_{_{B}}\sqcap\widehat{E}_{\varrho}\preceq\upsilon_{_{A}}$ such that

Since $\psi_{_{\vartheta}}:(\mathcal{X}, (£_{st}^{\gamma\pi\varsigma})\rightarrow (\mathcal{G}, \mathcal{F}_{st}^{\gamma\pi\varsigma})$ is svns-uniformly continuous,

From the concept of $£^{\gamma\pi\varsigma}_{_{e}}((\psi\times\psi)^{-1}_{_{\vartheta}}(\upsilon_{_{A}}))$, we get

This is a conflict with the hypothesis. □

Proposition 2. Let $\psi:\mathcal{X}\rightarrow \mathcal{G}$, and $\vartheta: {E}\rightarrow \mathcal{R}$ be two mappings, and let $f_{_{D}}\in\widehat{(\mathcal{X}, {E})}$, $\upsilon_{_{A}}, \mu_{_{B}}, \kappa_{_{C}}\in\widehat{(\mathcal{G}\times\mathcal{G}, \mathcal{R})}$. Then, the following results hold in general:

$(1) \; \psi^{-1}_{_{\vartheta}}(\upsilon_{_{A}}[\psi_{_{\vartheta}}(f_{_{D}})]) = ((\psi\times\psi)^{-1}_{_{\vartheta}}(\upsilon_{_{A}}))[f_{_{D}}]$,

$(2) \; ((\psi\times\psi)^{-1}_{_{\vartheta}}(\upsilon^{s}_{_{A}}))[f_{_{D}}] = ((\psi\times\psi)^{-1}_{_{\vartheta}}(\upsilon_{_{A}}))^{s}[f_{_{D}}]$,

$(3) \; (\psi\times\psi)^{-1}_{_{\vartheta}}(\upsilon_{_{A}}\sqcap\mu_{_{B}}) = (\psi\times\psi)^{-1}_{_{\vartheta}}(\upsilon_{_{A}})\sqcap(\psi\times\psi)^{-1}_{_{\vartheta}}(\mu_{_{B}})$,

$(4) \; (\psi\times\psi)^{-1}_{_{\vartheta}}(\upsilon_{_{A}})\circ(\psi\times\psi)^{-1}_{_{\vartheta}}(\upsilon_{_{A}})\preceq(\psi\times\psi)^{-1}_{_{\vartheta}}(\upsilon_{_{A}}\circ\upsilon_{_{A}})$.

Proof. (1) For $\omega\in\psi(\text{E})$, we get that

(2) For $\omega\in\psi(\text{E})$, we have

(3) Direct.

(4) For $\omega\in\psi(\text{E})$, we have

□

Theorem 9. Let $(\mathcal{X}, £^{\gamma\pi\varsigma})$ and $(\mathcal{G}, \mathcal{F}^{\gamma\pi\varsigma})$ be svns-uniform spaces, $\psi_{_{\vartheta}}:\widehat{(\mathcal{X}\times\mathcal{X}, {E})}\rightarrow \widehat{(\mathcal{G}\times\mathcal{G}, \mathcal{R})}$ be svns-uniformly continuous. Then, the following results hold in general.

$(1) \; \psi^{-1}_{_{\vartheta}}({I}_{_{\mathcal{F}^{\gamma\pi\varsigma}}}(\omega, f_{_{C}}, r))\preceq{I}_{_{£^{\gamma\pi\varsigma}}}(\vartheta^{-1}(\omega), \psi^{-1}_{_{\vartheta}}(f_{_{C}}), r))$, for each $f_{_{C}}\in\widehat{(\mathcal{G}, \mathcal{R})}$, $r\in\xi$, $\omega\in\mathcal{R}$,

$(2) \; {C}_{_{£^{\gamma\pi\varsigma}}}(\vartheta^{-1}(\omega), \psi^{-1}_{_{\vartheta}}(f_{_{C}}), r))\preceq\psi^{-1}_{_{\vartheta}}({C}_{_{\mathcal{F}^{\gamma\pi\varsigma}}}(\omega, f_{_{C}}, r))$, for each $f_{_{C}}\in\widehat{(\mathcal{G}, \mathcal{R})}$, $r\in\xi$, $\omega\in\mathcal{R}$,

$(3) \; \psi_{_{\vartheta}}({C}_{_{£^{\gamma\pi\varsigma}}}(e, g_{_{D}}, r))\preceq{C}_{_{\mathcal{F}^{\gamma\pi\varsigma}}}(\vartheta(\omega), \psi_{_{\vartheta}}(g_{_{D}}), r))$, for each $g_{_{D}}\in\widehat{(\mathcal{X}, {E})}$, $r\in\xi$, $e\in{E}$.

Proof. (1) For each $\upsilon_{_{A}}, \in\widehat{(\mathcal{G}\times\mathcal{G}, \mathcal{R})}$ and $f_{_{C}}, g_{_{D}}\in\widehat{(\mathcal{G}, \mathcal{R})}$, from Proposition 2, $\upsilon_{_{A}}[f_{_{C}}]\preceq g_{_{D}}$ implies that

Since

for every $\mu_{_{B}}\in(\psi\times\psi)^{-1}_{_{\vartheta}}(\upsilon_{_{A}})$, we obtain

In a similar vein, we can demonstrate (2) and (3) through a parallel line of reasoning. □

Theorem 10. Let $(\mathcal{X}, £_{ {E}}^{\gamma\pi\varsigma})$ and $(\mathcal{G}, \mathcal{F}_{\mathcal{R}}^{\gamma\pi\varsigma})$ be svns-uniform spaces, and $\psi_{_{\vartheta}}:\widehat{(\mathcal{X}, {E})}\rightarrow \widehat{(\mathcal{G}, \mathcal{R})}$ an injective svns-uniformly continuous. Then, $\psi_{_{\vartheta}}:(\mathcal{X}, \mathfrak{T}_{£}^{\star\gamma\pi\varsigma})\rightarrow (\mathcal{G}, \mathfrak{T}_{\mathcal{F}}^{\star\gamma\pi\varsigma})$ is svns-continuous.

Proof. Since $\psi_{_{\vartheta}}$ injective and by applying Theorem 4, we get that:

For each $\upsilon_{_{A}}, \in\widehat{(\mathcal{G}\times\mathcal{G}, \mathcal{R})}$ and $f_{_{B}}\in\widehat{(\mathcal{G}, \mathcal{R})}$, $\omega\in A\cap B$. Then,

Therefore, $\forall \; e\in \text{E}$

□

Theorem 11. Let $(\mathcal{X}, £_{ {E}}^{\gamma\pi\varsigma})$ and $(\mathcal{G}, \mathcal{F}_{\mathcal{R}}^{\gamma\pi\varsigma})$ be two svns-uniform spaces and $\psi_{_{\vartheta}}:\widehat{(\mathcal{X}, {E})}\rightarrow \widehat{(\mathcal{G}, \mathcal{R})}$ be an svns-uniformly continuous mapping. Then, $\psi_{_{\vartheta}}:(\mathcal{X}, \mathfrak{T}_{£}^{\star\star\gamma\pi\varsigma})\rightarrow (\mathcal{G}, \mathfrak{T}_{\mathcal{F}}^{\star\star\gamma\pi\varsigma})$ is svns-continuous.

Proof. Initially, it is clear that $\psi^{-1}_{_{\vartheta}}(\upsilon_{_{A}}[\psi(x)]) = (\psi\times\psi)^{-1}_{_{\vartheta}}(\upsilon_{_{A}}[x])$ from that:

Thus, $\upsilon_{_{A}}[\psi(x)]\preceq f_{_{B}}$ implies that $\psi^{-1}_{_{\vartheta}}(\upsilon_{_{A}}[\psi(x)]) = ((\psi\times\psi)^{-1}_{_{\vartheta}}(\upsilon_{_{A}}))[x]\preceq \psi^{-1}_{_{\vartheta}}(f_{_{B}})$. By applying Theorem 8, we obtain

Likewise, we can establish through a similar line of reasoning that $(\mathfrak{T}_{£}^{\star\star\varsigma})_{\omega}(f_{_{B}})\geq(\mathfrak{T}_{£}^{\star\star\varsigma})_{_{\vartheta^{-1}(\omega)}}(\psi^{-1}_{_{\vartheta}}(f_{_{B}})).$ □

Theorem 12. Let $\{(\mathcal{X}_{_{j}}, (£_{_{j}}^{\gamma\pi\varsigma})_{ {E}_{_{j}}}): j\in\Gamma\}$ be a family of svns- uniform spaces and, for all $j\in\Gamma$, $\psi_{_{j}}:\mathcal{X}\rightarrow \mathcal{X}_{_{j}}$, and $\vartheta_{_{j}}: {E}\rightarrow {E}_{_{j}}$ are mappings. Define $£^{\gamma}: {E}\rightarrow \zeta^{\widehat{(\mathcal{X}\times\mathcal{X}, {E})}}$, $£^{\pi}: {E}\rightarrow \zeta^{\widehat{(\mathcal{X}\times\mathcal{X}, {E})}}$ and $£^{\varsigma}: {E}\rightarrow \zeta^{\widehat{(\mathcal{X}\times\mathcal{X}, {E})}}$ on $\mathcal{X}$ by:

where $\bigvee$is taken over all finite subsets $\Omega = \{\omega_{1}, \omega_{2}, ..., \omega_{n}\}\subseteq\Gamma$. Then,

$(1) \; £^{\gamma\pi\varsigma}$ is the coarsest svns-uniformity on $\mathcal{X}$ for which all $\{(\psi_{\vartheta})_{_{j}}: j\in\Gamma\}$ are svns-uniformly continuous.

$(2)$ A map $\psi_{_{\vartheta}}:(\mathcal{X}^{\star}, £_{_{\mathcal{R}}}^{\gamma\pi\varsigma})\rightarrow (\mathcal{X}, £_{_{ {E}}}^{\gamma\pi\varsigma})$ is svns-uniformly continuous if for all $j\in\Gamma$, $(\psi_{\vartheta})_{_{j}}\circ\psi_{\vartheta}: (\mathcal{X}^{\star}, £_{_{\mathcal{R}}}^{\gamma\pi\varsigma})\rightarrow (\mathcal{X}_{_{j}}, (£_{_{j}}^{\gamma\pi\varsigma})_{_{ {E}_{_{j}}}})$ is svns-uniformly continuous.

Proof. (1) Initially, we indication that $£^{\gamma\pi\varsigma}$ is an svns-uniformity on $\mathcal{X}$ for which all $\{(\psi_{\vartheta})_{_{j}}: j\in\Gamma\}$ are svns-uniformly continuous.

$(£_{_{1}})$ For every $\omega_{_{j}}\in\Omega$, there exists $(\upsilon_{_{A}})_{_{\omega_{_{j}}}}\in(\mathcal{X}_{_{\omega_{_{j}}}}\times\widehat{\mathcal{X}}_{_{\omega_{_{j}}}}, {E}_{_{\omega_{_{j}}}})$ such that, for $e\in {E}$, we obtain that

Put $(\psi_{_{\omega_{_{j}}}}\times\psi_{_{\omega_{_{j}}}})^{-1}_{_{\vartheta_{_{\omega_{_{j}}}}}}((\mu_{_{B}})_{\omega_{_{j}}}) = \upsilon_{_{A}}$. Then, $£^{\gamma}_{_{e}}(\upsilon_{_{A}}) = 1$, $£^{\pi}_{_{e}}(\upsilon_{_{A}}) = 0$ and $£^{\varsigma}_{_{e}}(\upsilon_{_{A}}) = 0$.

$(£_{_{2}})$ It is obvious from the definition of $£^{\gamma\pi\varsigma}$.

$(£_{_{3}})$ For all limited subsets $\Omega = \{\omega_{1}, \omega_{2}, ..., \omega_{n}\}$, $T = \{t_{1}, t_{2}, ..., t_{m}\}$ of $\Gamma$ such that

we have

Moreover, for all $\omega\in\Omega\cap T$ we have

Put $(\psi_{_{m_{j}}}\times\psi_{_{m_{j}}})^{-1}_{_{\vartheta_{_{m_{j}}}}}((\mathcal{W}_{_{C}})_{_{m_{j}}})\preceq\mu_{_{B}}\sqcap\upsilon_{_{A}}$, where

Therefore, we obtain

Taking the supremum on the families $\sqcap^{n}_{j = 1}(\psi_{_{\omega_{_{j}}}}\times\psi_{_{\omega_{_{j}}}})^{-1}_{_{\vartheta_{_{\omega_{_{j}}}}}}((\upsilon_{_{A}})_{\omega_{_{j}}})\preceq\upsilon_{_{A}}$ and $\sqcap^{m}_{j = 1}(\psi_{_{t_{_{j}}}}\times\psi_{_{t_{_{j}}}})^{-1}_{_{\vartheta_{_{t_{_{j}}}}}}((\mu_{_{B}})_{_{t_{_{j}}}})\preceq\mu_{_{B}}$ we obtain

$(£_{_{4}})$ If $£_{_{e}}^{\gamma}(\upsilon_{_{A}})\not = 0$, $£_{_{e}}^{\pi}(\upsilon_{_{A}})\not = 1$ and $£_{_{e}}^{\varsigma}(\upsilon_{_{A}})\not = 1$, then there exists $\Omega = \{\omega_{1}, \omega_{2}, ..., \omega_{p}\}$ of $\Gamma$ with $\sqcap^{p}_{j = 1}(\psi_{_{\omega_{_{j}}}}\times\psi_{_{\omega_{_{j}}}})^{-1}_{_{\vartheta_{_{\omega_{_{j}}}}}}((\mu_{_{B}})_{\omega_{_{j}}})\preceq\upsilon_{_{A}}$ such that

Since, $(£_{_{\omega_{_{j}}}}^{\gamma})_{_{\vartheta_{\omega_{_{j}}}(e)}}((\mu_{_{B}})_{_{\omega_{j}}})\not = 0$, $(£_{_{\omega_{_{j}}}}^{\pi})_{_{\vartheta_{\omega_{_{j}}}(e)}}((\mu_{_{B}})_{_{\omega_{j}}})\not = 1$, $(£_{_{\omega_{_{j}}}}^{\varsigma})_{_{\vartheta_{\omega_{_{j}}}(e)}}((\mu_{_{B}})_{_{\omega_{j}}})\not = 0 \; \forall \; \omega_{_{j}}\in\Omega$, then $(\top)_{C}\npreceq(\upsilon_{_{B}})_{_{\omega_{_{j}}}}$. Thus,

$(£_{_{5}})$ Assume that $£^{\gamma}_{_{e}}(\upsilon_{_{A}}^{s})\ngeq£^{\gamma}_{_{e}}(\upsilon_{_{A}})$, $£^{\pi}_{_{e}}(\upsilon_{_{A}}^{s})\nleq£^{\pi}_{_{e}}(\upsilon_{_{A}})$ and $£^{\varsigma}_{_{e}}(\upsilon_{_{A}}^{s})\nleq£^{\varsigma}_{_{e}}(\upsilon_{_{A}})$. From the concept of $£^{\gamma\pi\varsigma}$, there exists $\Omega = \{\omega_{1}, \omega_{2}, ..., \omega_{p}\}$ of $\Gamma$ with $\sqcap^{p}_{j = 1}(\psi_{_{\omega_{_{j}}}}\times\psi_{_{\omega_{_{j}}}})^{-1}_{_{\vartheta_{_{\omega_{_{j}}}}}}((\mu_{_{B}})_{\omega_{_{j}}})\preceq\upsilon_{_{A}}$ such that

Since $£_{\omega_{_{j}}}^{\gamma\pi\varsigma}$ is an svns-uniformity on $\mathcal{X}$ for each $\omega_{_{j}}$

It follows that

On the other hand,

Hence,

It is a contradiction. Hence, $(£_{_{5}})$ holds.

$(£_{_{6}})$ Suppose that for each $\upsilon_{_{A}}\in\widehat{(\mathcal{X}\times\mathcal{X}, \text{E})}$

By the concept of $£^{\gamma\pi\varsigma}$, there exists $\Omega = \{\omega_{1}, \omega_{2}, ..., \omega_{p}\}$ of $\Gamma$ with $\sqcap^{p}_{j = 1}(\psi_{_{\omega_{_{j}}}}\times\psi_{_{\omega_{_{j}}}})^{-1}_{_{\vartheta_{_{\omega_{_{j}}}}}}((\mu_{_{B}})_{\omega_{_{j}}})\preceq\upsilon_{_{A}}$ such that

Since $£_{\omega_{_{j}}}^{\gamma\pi\varsigma}$ is svns- uniformity on $\mathcal{X}_{_{\omega_{_{j}}}}$ for each $\omega_{_{j}}\in\Omega$

Thus, there exists $\mathcal{W}_{_{C}}\in\widehat{(\mathcal{X}_{_{\omega_{_{j}}}}\times\mathcal{X}_{_{\omega_{_{j}}}}, {E}_{_{\omega_{_{j}}}})}$, $\mathcal{W}_{_{C}}\circ\mathcal{W}_{_{C}}\preceq\upsilon_{_{A}}$ such that

On the other hand,

Therefore, we have

It is a contradiction. Hence, $£_{_{6}}$ holds.

Next by the concept of $£^{\gamma\pi\varsigma}$ it is easily proved that, for each $j\in\Gamma$

Thus, $(\psi_{_{j}})_{\vartheta_{j}}:\mathcal{X}\rightarrow \mathcal{X}_{_{j}}$ is svns-uniformly continuous.

Lastly, let us say that $£^{\star\gamma\pi\varsigma}$ is an svns-uniformity on $\mathcal{X}$ and $(\psi_{_{j}})_{\vartheta_{j}}:(\mathcal{X}, £^{\star\gamma\pi\varsigma})\rightarrow (\mathcal{X}_{_{j}}, £_{j}^{\gamma\pi\varsigma})$ is svns-uniformly continuous, that is, for every $j\in \Gamma$ and $(\mu_{_{B}})_{j}\in\widehat{(\mathcal{X}_{j}\times\mathcal{X}_{j}, \text{E}_{_{j}}})$,

For every finite subset $\Omega = \{\omega_{1}, \omega_{2}, ..., \omega_{p}\}$ of $\Gamma$ with $\sqcap^{p}_{j = 1}(\psi_{_{\omega_{_{j}}}}\times\psi_{_{\omega_{_{j}}}})^{-1}_{_{\vartheta_{_{\omega_{_{j}}}}}}((\mu_{_{B}})_{\omega_{_{j}}})\preceq\upsilon_{_{A}}$, we have

In a similar vein, we can demonstrate through a parallel line of reasoning that $£^{\pi}_{_{e}}(\upsilon_{_{A}})\geq £^{\star\pi}_{_{e}}(\upsilon_{_{A}})$ and $£^{\varsigma}_{_{e}}(\upsilon_{_{A}})\geq £^{\star\varsigma}_{_{e}}(\upsilon_{_{A}})$.

(2) It can be easily proved. □

6.   Conclusions

Many scientists have studied the soft set theory and easily applied it to many problems in social life. In the present work, we defined the single-valued neutrosophic soft uniform spaces and single-valued neutrosophic soft uniform bases. The relationships between them were also investigated. Next, the relationship among single-valued neutrosophic soft uniformities, single-valued neutrosophic soft topologies, and single-valued neutrosophic soft interior operators were introduced and studied. Finally, we proved crucial results in introducing and characterizing single-valued neutrosophic soft uniformly continuous, on single-valued neutrosophic soft uniformly topological spaces. Moreover, the relationship between single-valued neutrosophic soft uniformly continuous and single-valued neutrosophic soft continuous was studied. This paper can form the theoretical basis for further applications of single-valued neutrosophic soft topology, potentially leading to the development of other scientific areas.

Use of AI tools declaration

The authors declare that they have not used Artificial Intelligence tools in the creation of this article.

Acknowledgments

The authors would like to extend their sincere appreciation to Supporting Project number (RSPD2023R860) King Saud University, Riyadh, Saudi Arabia.

Conflict of interest

The authors declare that they have no conflicts of interest.