Optimal investment and reinsurance for the insurer and reinsurer with the joint exponential utility under the CEV model

Ling Chen; Xiang Hu; Mi Chen; Ling Chen; Xiang Hu; Mi Chen

doi:10.3934/math.2023786

AIMS Mathematics

2023, Volume 8, Issue 7: 15383-15410. doi: 10.3934/math.2023786

Previous Article Next Article

Research article

Optimal investment and reinsurance for the insurer and reinsurer with the joint exponential utility under the CEV model

Ling Chen ¹,
Xiang Hu ²,
Mi Chen ^{1
,
,}

1.
School of Mathematics and Statistics & FJKLMAA, Fujian Normal University, Fuzhou 350117, China
2.
School of Finance, Zhongnan University of Economics and Law, Nanhu Road, Wuhan 430073, China

Received: 22 March 2023 Revised: 16 April 2023 Accepted: 20 April 2023 Published: 26 April 2023
MSC : 91B05, 91G05

This paper considers the problem of optimal investment-reinsurance for the insurer and reinsurer under the constant elasticity of variance (CEV) model. It is assumed that the net claims process is approximated by a diffusion process, both the insurer and reinsurer can invest in risk-free assets and risky assets. We use the variance premium principle to calculate the premiums of the insurer and reinsurer, and the reinsurance proportion is constrained by the net profit condition. Our objective is to maximize the joint exponential utility of the insurer and reinsurer's terminal wealth for a fixed time. By solving the HJB equation, we obtain the explicit expressions of the optimal investment-reinsurance strategy and value function. We find that the optimal reinsurance strategy can be divided into many cases and is related to the risk aversion coefficient of the insurer and reinsurer, but independent of the price of risky assets. Furthermore, we give the proof of the verification theorem. Finally, we demonstrate a numerical analysis to explain the results.

Keywords:

Citation: Ling Chen, Xiang Hu, Mi Chen. Optimal investment and reinsurance for the insurer and reinsurer with the joint exponential utility under the CEV model[J]. AIMS Mathematics, 2023, 8(7): 15383-15410. doi: 10.3934/math.2023786

Related Papers:

[1]	Rasool Shah, Abd-Allah Hyder, Naveed Iqbal, Thongchai Botmart . Fractional view evaluation system of Schrödinger-KdV equation by a comparative analysis. AIMS Mathematics, 2022, 7(11): 19846-19864. doi: 10.3934/math.20221087
[2]	Azzh Saad Alshehry, Naila Amir, Naveed Iqbal, Rasool Shah, Kamsing Nonlaopon . On the solution of nonlinear fractional-order shock wave equation via analytical method. AIMS Mathematics, 2022, 7(10): 19325-19343. doi: 10.3934/math.20221061
[3]	Maysaa Al-Qurashi, Saima Rashid, Fahd Jarad, Madeeha Tahir, Abdullah M. Alsharif . New computations for the two-mode version of the fractional Zakharov-Kuznetsov model in plasma fluid by means of the Shehu decomposition method. AIMS Mathematics, 2022, 7(2): 2044-2060. doi: 10.3934/math.2022117
[4]	Ahmad El-Ajou, Rania Saadeh, Moawaih Akhu Dunia, Ahmad Qazza, Zeyad Al-Zhour . A new approach in handling one-dimensional time-fractional Schrödinger equations. AIMS Mathematics, 2024, 9(5): 10536-10560. doi: 10.3934/math.2024515
[5]	Y. Massoun, C. Cesarano, A. K Alomari, A. Said . Numerical study of fractional phi-4 equation. AIMS Mathematics, 2024, 9(4): 8630-8640. doi: 10.3934/math.2024418
[6]	Manal Alqhtani, Khaled M. Saad, Rasool Shah, Thongchai Botmart, Waleed M. Hamanah . Evaluation of fractional-order equal width equations with the exponential-decay kernel. AIMS Mathematics, 2022, 7(9): 17236-17251. doi: 10.3934/math.2022949
[7]	Mamta Kapoor, Nehad Ali Shah, Wajaree Weera . Analytical solution of time-fractional Schrödinger equations via Shehu Adomian Decomposition Method. AIMS Mathematics, 2022, 7(10): 19562-19596. doi: 10.3934/math.20221074
[8]	Yousef Jawarneh, Humaira Yasmin, M. Mossa Al-Sawalha, Rasool Shah, Asfandyar Khan . Numerical analysis of fractional heat transfer and porous media equations within Caputo-Fabrizio operator. AIMS Mathematics, 2023, 8(11): 26543-26560. doi: 10.3934/math.20231356
[9]	Yousef Jawarneh, Humaira Yasmin, M. Mossa Al-Sawalha, Rasool Shah, Asfandyar Khan . Fractional comparative analysis of Camassa-Holm and Degasperis-Procesi equations. AIMS Mathematics, 2023, 8(11): 25845-25862. doi: 10.3934/math.20231318
[10]	Rehana Ashraf, Saima Rashid, Fahd Jarad, Ali Althobaiti . Numerical solutions of fuzzy equal width models via generalized fuzzy fractional derivative operators. AIMS Mathematics, 2022, 7(2): 2695-2728. doi: 10.3934/math.2022152

Abstract

Nomenclature

$\omega:$ Independent variable; $\phi:$ Time; ${\upsilon}({\omega}, {\phi}):$ Dependent function representing the physical quantity; $\alpha:$ Fractional order; $S:$ Shehu transform; $S^{-1}:$ Inverse Shehu transform; $\epsilon:$ Perturbation parameter

1. Introduction

In recent years, the utilization of non-integer order derivatives, also known as fractional derivatives, has gained remarkable traction across various scientific and engineering disciplines due to its ability to model complex phenomena with more accuracy and flexibility ^[1,2]. Fractional calculus provides an extended framework that goes beyond the restrictions of typical integer-order derivatives, allowing for a more comprehensive modeling of processes with memory effects and long-range interactions ^[3,4]. Mathematicians that study fractional calculus extend the idea of integrals and derivatives of integer orders to non-integer orders. The creation of fractional differential equations is the result of this concept's multiple applications in a variety of scientific and technical fields. Differential equations involving fractional derivatives of an unknown function are known as fractional differential equations. Complex processes that cannot be modelled using integer-order differential equations can be described by these equations. Numerous physical, biological, and engineering systems, including viscoelastic materials, diffusion processes, wave propagation, and control systems, have been modelled using them ^[5,6,7].

Nonlinear fractional differential equations are a specific category of fractional differential equations that demonstrate nonlinear characteristics, wherein the unknown function is present in nonlinear expressions ^[1,2]. The resolution of these equations presents a greater level of difficulty compared to their linear equivalents, necessitating the utilisation of advanced analytical and numerical methods ^[3,11]. In recent years, there has been significant interest in the examination of nonlinear fractional differential equations, with numerous academics dedicating their efforts to exploring their properties, solutions, and applications ^[12,13]. Various techniques are employed to solve these equations, encompassing numerical methods, analytical methods such as fractional calculus, and perturbation methods ^[14,15]. In brief, fractional nonlinear differential equations play a crucial role in the modelling of intricate systems that demonstrate nonlinear dynamics. These entities possess a wide range of applications across diverse disciplines and want advanced methodologies for their examination and resolution ^{[4,17,18,19,20,21]}.

The fractional Phi-four equation is a non-linear partial differential equation that characterises the temporal and spatial evolution of a field variable. The equation under consideration can be seen as a fractional extension of the widely recognised Phi-four equation, which holds significant importance in the field of mathematical physics due to its relevance in the analysis of solitons and nonlinear waves. The equation known as the fractional Phi-four equation is characterised by the inclusion of a fractional derivative of the field variable with respect to time, a second-order derivative of the field variable with respect to space, and a cubic nonlinear factor. The inclusion of the fractional derivative in the system introduces a memory component, so enabling the manifestation of non-local characteristics and long-range interactions ^[22].

The fractional Phi-four equation is very important in the study of mathematical physics because of its capacity to demonstrate many dynamics and its wide applicability in many physics disciplines. The equation demonstrates a number of dynamic phenomena that are dependent on the parameters and starting circumstances, such as the formation of solitons, chaotic behaviour, and turbulence. In recent years, there has been a significant amount of study done on the aforementioned equation, owing to its relevance in a variety of areas such as condensed matter physics, statistical mechanics, nonlinear dynamics, and fluid mechanics ^[23,24]. Researchers are presently investigating the equation and its many versions, employing fresh analytical and numerical methodologies to examine its behaviour under various situations ^{[25,26,27,28]}. Furthermore, research into the fractional Phi-four equation has made substantial contributions to the advancement of fractional calculus, a solid mathematical framework used for the analysis of complex systems showing memory and non-local phenomena. Because of its numerous applications, fractional calculus is widely used in a variety of scientific and engineering areas, including physics, chemistry, biology, and finance ^[3]. Depending on the parameter values and beginning conditions, the fractional Phi-four equation exhibits a variety of dynamic phenomena such as soliton production, chaotic behaviour, and turbulence. Several analytical and numerical techniques, such as the homotopy analysis method ^[30] and others ^[1,2] have been developed to explore the dynamics of the fractional Phi-four equation.

In the field of nonlinear differential equation solving, the Homotopy Perturbation Method (HPM) and the Adomian Decomposition Method (ADM) are widely used numerical techniques. Liao proposed the HPM (Homotopy Perturbation Method) as a mathematical technique in 1992 ^[33]. Adomian, on the other hand, developed the ADM (Adomian Decomposition Method) as an alternate approach in 1988 ^[34]. The HPM (Homotopy Perturbation Method) includes creating a homotopy, or continuous transformation, that connects a linear problem with a known solution to the nonlinear problem under inquiry ^{[35,36,37,38]}. Particular advantages of High Performance Computing (HPC) technology may be seen in scientific research. This method works well for handling nonlinear problems with precision while avoiding the requirement for linearization. Utilising a homotopy parameter, the Homotopy Perturbation Method (HPM) simplifies complex nonlinear problems into more manageable linear ones, making it applicable in a variety of situations. It's important to remember that researchers can alter the convergence of solution series, which expands their practical applications. Because of its versatility, HPM may be easily integrated with a variety of methods, which improves its accuracy and effectiveness. The solution is obtained by working through a series of linear issues while progressively raising the homotopy parameter from zero to one. Numerous nonlinear problems, such as Burgers' equation ^[39], heat transport in porous media ^[40], and fractional differential equations ^[41], have been solved using the homotropy perturbation method, or HPM.

Numerous nonlinear issues, such as the nonlinear Schrödinger equation, have been tackled with the ADM approach ^[42]. A useful method for making nonlinear differential equations easier to solve using the HPM and ADM processes is the Shehu Transform. Numerous nonlinear scenarios, including the Duffing equation ^[43], the nonlinear Black-Scholes equation ^[44], and the Boussinesq equation ^[45], have been solved using the Shehu Transform.

This work is summarised in the following. We start Section 2 by providing a definition and description of the Shehu transform, which we employ in this study. The solution mechanism of the Shehu transform decomposition method (STDM) is explained in Section 3. The solution approach for the homotopy perturbation transform technique (HPTM) is explained in Section 4. For our current study, the results of numerical simulations are shown and discussed in Section 5. Lastly, the results of our investigation are presented in Section 6.

2. Basic definitions

Definition 2.1. Fractional derivative of $f \in C_{-1}^n$ is given in the sense of Caputo as the following ^[45,46]:

$D_\phi^\alpha \upsilon(\omega, \phi) = \left\{\begin{array}{cc} \frac{d^n \upsilon(\omega, \phi)}{d \phi^n}, & \alpha = n \in \mathbb{N}, \\ \frac{1}{\Gamma(n-\alpha)} \int_0^\phi(\omega, \phi-\vartheta)^{n-\alpha-1} \upsilon^{(n)}(\omega, \vartheta) d \vartheta, & n-1 < \alpha < n, n \in \mathbb{N} . \end{array}\right.$

Definition 2.2. Shehu transform is defined as follows ^[45,46]:

$S[Q({\phi})] = \int_0^{\infty} \mathrm{e}^{\left(-\frac{{\wp} {\phi}}{{\varpi}}\right)} Q({\phi}) \mathrm{d} {\phi} .$

Shehu transform will be transformed into Laplace transform by considering ${\varpi} = 1$ ,

Shehu transform will be transformed into Yang transformed by considering ${\wp} = 1$ , where $S$ is considered a Shehu Transform operator.

Definition 2.3. The Inverse Shehu transform operator is defined as ^[45,46]

Let $S[Q({\phi})] = J({\wp}, {\varpi})$ and $S^{-1}[J({\wp}, {\varpi})] = Q({\phi})$ ,

then $Q({\phi}) = S^{-1}[J({\wp}, {\varpi})] = \lim_{x\to\infty}\frac{1}{2 \pi i} \int_{\beta+i x}^{\beta- i x} \frac{\mathrm{e}^{-{\wp} {\phi}}}{{\varpi}} J({\wp}, {\varpi}) \mathrm{d} {\wp}$ ,

where ${\wp}$ and ${\varpi}$ are considered as Shehu transform variables and $\beta$ is a real constant.

Lemma 2.4. Linearity property of Shehu transform ^[45,46]:

If $S\left[Q_1({\phi})\right] = J_1({\wp}, {\varpi})$ and $S\left[Q_2({\phi})\right] = J_2({\wp}, {\varpi})$ ,

Then $S\left[\alpha_1 Q_1({\phi})+\alpha_2 Q_2({\phi})\right] = \alpha_1 S\left[Q_1({\phi})\right]+\alpha_2 S\left[Q_2(\omega, {\phi})\right]$ ,

$S\left[\alpha_1 Q_1({\phi})+\alpha_2 Q_2({\phi})\right] = \alpha_1 J_1({\wp}, {\varpi})+\alpha_2 S J_2({\wp}, {\varpi})$ ,

where $\alpha_1$ and $\alpha_2$ are the arbitrary constants.

Lemma 2.5. Linearity property of inverse Shehu transform ^[45,46]:

If $S^{-1}\left[J_1({\wp}, {\varpi})\right] = Q_1({\phi})$ and $S^{-1}\left[J_2({\wp}, {\varpi})\right] = Q_2({\phi})$ , then,

$\begin{aligned} & S^{-1}\left[\alpha_1 J_1({\wp}, {\varpi})+\alpha_2 J_2({\wp}, {\varpi})\right] = \alpha_1 S^{-1}\left[J_1({\wp}, {\varpi})\right]+\alpha_2 S^{-1}\left[J_2({\wp}, {\varpi})\right], \\ & S^{-1}\left[\alpha_1 J_1({\wp}, {\varpi})+\alpha_2 J_2({\wp}, {\varpi})\right] = \alpha_1 Q_1({\phi})+\alpha_2 Q_2({\phi}) . \end{aligned}$

Definition 2.6. Shehu transform of Caputo fractional derivative (C.F.D) ^[45,46]

$S\left[D_{\phi}^\alpha Q\left(\eta_1, {\phi}\right)\right] = \frac{{\wp}^\alpha}{{\varpi}^\alpha} S\left[Q\left(\eta_1, {\phi}\right)\right]-\sum\limits_{r = 0}^{\theta-1}\left(\frac{{\wp}}{{\varpi}}\right)^{\alpha-r-1} Q^r\left(\eta_1, 0\right), \ \ \theta = 1, 2, 3\cdots.$

Definition 2.7. Mittag-Leffler function considered for two parameters ^[45,46]

$E_{\mu, {\varpi}}(n) = \sum\limits_{k = 0}^{\infty} \frac{n^k}{\Gamma(k \mu+{\varpi})},$

where $E_{1, 1}(n) = \exp (n)$ and $E_{2, 1}\left(n^2\right) = \cos (n)$ .

3. Solution procedure of Shehu transform decomposition method (STDM)

In this section, we consider the nonlinear FDEs to demonstrate the basic idea of the projected algorithm as given

$\begin{equation} D_{\phi}^{\alpha}{{\upsilon}({\omega}, {\phi})} = \mathcal{P}_1({\omega}, {\phi})+\mathcal{Q}_1({\omega}, {\phi}), \\ 1 < {\alpha} \leq 2, \end{equation}$

(3.1)

and

${\upsilon}({\omega}, 0) = \xi({\omega}), \ \ \frac{\partial}{\partial{\phi}}{\upsilon}({\omega}, 0) = \zeta({\omega}).$

where $D_{\phi}^{\alpha} = \frac{\partial^{\alpha}}{\partial {\phi}^{\alpha}}$ signifies fractional Caputo operator, $\mathcal{P}_1$ , $\mathcal{Q}_1$ are respectively linear and non-linear operators.

Now we apply ST to obtain

$\begin{equation} \begin{split} &{S}[D_{\phi}^{\alpha}{{\upsilon}({\omega}, {\phi})}] = {S}[\mathcal{P}_1({\omega}, {\phi})+\mathcal{Q}_1({\omega}, {\phi})], \\ &\frac{\wp^\alpha}{\varpi^\alpha} S[{\upsilon}({\omega}, {\phi})]-\sum\limits_{r = 0}^{\theta-1}\left(\frac{\wp}{\varpi}\right)^{\alpha-r-1}{\upsilon}^r({\omega}, 0) = {S}[\mathcal{P}_1({\omega}, {\phi})+\mathcal{Q}_1({\omega}, {\phi})].\\ \end{split} \end{equation}$

(3.2)

Now by employing inverse ST, I get

$\begin{equation} {\upsilon}({\omega}, {\phi}) = \sum\limits_{r = 0}^{\theta-1}\left(\frac{\wp}{\varpi}\right)^{\alpha-r-1}{\upsilon}^r({\omega}, 0)+{S}^{-1}[\frac{\varpi^\alpha}{\wp^\alpha}{S}[\mathcal{P}_1({\omega}, {\phi})+\mathcal{Q}_1({\omega}, {\phi})]. \end{equation}$

(3.3)

Now the solution is as

$\begin{equation} {\upsilon}({\omega}, {\phi}) = \sum\limits_{m = 0}^{\infty}{\upsilon}_m({\omega}, {\phi}). \end{equation}$

(3.4)

The nonlinear terms $\mathcal{Q}_1$ is discarded as

$\begin{equation} \begin{split} &\mathcal{Q}_1({\omega}, {\phi}) = \sum\limits_{m = 0}^{\infty}\mathcal{A}_m, \\ &\mathcal{A}_m = \frac{1}{m!}\left[\frac{\partial^m}{\partial\ell^m}\left\{\mathcal{Q}_1\left(\sum\limits_{k = 0}^{\infty}\ell^k{\omega}_k\right)\right\}\right]_{\ell = 0}.\\ \end{split} \end{equation}$

(3.5)

Using Eqs (3.4) and (3.5) into (3.3), I have

$\begin{equation} \sum\limits_{m = 0}^{\infty}{\upsilon}_m({\omega}, {\phi}) = \sum\limits_{r = 0}^{\theta-1}\left(\frac{\wp}{\varpi}\right)^{\alpha-r-1}{\upsilon}^r({\omega}, 0)+{S}^{-1} \left[\frac{\varpi^\alpha}{\wp^\alpha}{S}\left\{\mathcal{P}_1(\sum\limits_{m = 0}^{\infty}{\omega}_m)+\sum\limits_{m = 0}^{\infty}\mathcal{A}_m\right\}\right]. \end{equation}$

(3.6)

Comparing both sides allows for a straightforward approximation to be obtained.

$\begin{equation*} {\upsilon}_0({\omega}, {\phi}) = \sum\limits_{r = 0}^{\theta-1}\left(\frac{\wp}{\varpi}\right)^{\alpha-r-1}{\upsilon}^r({\omega}, 0), \end{equation*}$

$\begin{equation*} {\upsilon}_1({\omega}, {\phi}) = {S}^{-1}\left[\frac{\varpi^\alpha}{\wp^\alpha} {S}^+\{\mathcal{P}_1({\omega}_0)+\mathcal{A}_0\}\right], \end{equation*}$

The general recursive equation can be derived as follows:

$\begin{equation*} {\upsilon}_{m+1}({\omega}, {\phi}) = {S}^{-1}\left[\frac{\varpi^\alpha}{\wp^\alpha} {S}^+\{\mathcal{P}_1({\omega}_m)+\mathcal{A}_m\}\right].\\ \end{equation*}$

4. Solution procedure of homotopy perturbation transform method (HPTM)

The basic idea of the projected algorithm is demonstrated by considering the nonlinear FDEs in this section.

$\begin{equation} \ D_{{\phi}}^{{\alpha}}{\upsilon}({\omega}, {\phi}) = \mathcal{P}_1[{\omega}]{\upsilon}({\omega}, {\phi})+\mathcal{Q}_1[{\omega}]{\upsilon}({\omega}, {\phi}), \ \ 1 < {\alpha}\leq2, \end{equation}$

(4.1)

and

${\upsilon}({\omega}, 0) = \xi({\omega}), \ \ \frac{\partial}{\partial{\phi}}{\upsilon}({\omega}, 0) = \zeta({\omega}).$

The given expression involves the fractional Caputo operator $D_{{\phi}}^{{\alpha}}$ , where $\alpha$ represents the order of differentiation, and ${\phi}$ represents the variable with respect to which differentiation is performed. Additionally, $\mathcal{P}_1[{\omega}]$ and $\mathcal{Q}_1[{\omega}]$ are the linear and nonlinear operators, respectively.

Using the ST, we obtain

$\begin{equation} \ {S}[D_{{\phi}}^{{\alpha}}{\upsilon}({\omega}, {\phi})] = {S}[\mathcal{P}_1[{\omega}]{\upsilon}({\omega}, {\phi})+\mathcal{Q}_1[{\omega}]{\upsilon}({\omega}, {\phi})], \end{equation}$

(4.2)

$\begin{equation} \frac{\wp^\alpha}{\varpi^\alpha} S[{\upsilon}({\omega}, {\phi})]-\sum\limits_{r = 0}^{\theta-1}\left(\frac{\wp}{\varpi}\right)^{\alpha-r-1}{\upsilon}^r({\omega}, 0) = {S}[\mathcal{P}_1[{\omega}]{\upsilon}({\omega}, {\phi})+\mathcal{Q}_1[{\omega}]{\upsilon}({\omega}, {\phi})]. \end{equation}$

(4.3)

By applying the inverse of ST, we get:

$\begin{equation} {\upsilon}({\omega}, {\phi}) = \sum\limits_{r = 0}^{\theta-1}\left(\frac{\wp}{\varpi}\right)^{\alpha-r-1}{\upsilon}^r({\omega}, 0)+{S}^{-1}[\frac{\varpi^\alpha}{\wp^\alpha}{S}[\mathcal{P}_1[{\omega}]{\upsilon}({\omega}, {\phi})+\mathcal{Q}_1[{\omega}]{\upsilon}({\omega}, {\phi})]]. \end{equation}$

(4.4)

By HPM, we get

$\begin{equation} {\upsilon}({\omega}, {\phi}) = \sum\limits_{k = 0}^{\infty}\epsilon^{k}{\upsilon}_{k}({\omega}, {\phi}). \end{equation}$

(4.5)

The nonlinear terms are neglected, with $\epsilon$ serving as the homotopy parameter ranging from 0 to 1.

$\begin{equation} \ \mathcal{Q}_1[{\omega}]{\upsilon}({\omega}, {\phi}) = \sum\limits_{k = 0}^{\infty}\epsilon^{k}H_{n}({\upsilon}), \end{equation}$

(4.6)

with $H_{k}({\upsilon})$ representing the He's polynomials

$\begin{equation} H_{n}({\upsilon}_{0}, {\upsilon}_{1}, ..., {\upsilon}_{n}) = \frac{1}{\Gamma(n+1)}D_{\epsilon}^{k}\left[\mathcal{Q}_1\left(\sum\limits_{k = 0}^{\infty}\epsilon^{i}{\upsilon}_{i}\right)\right]_{\epsilon = 0}, \end{equation}$

(4.7)

with $D_{\epsilon}^{k} = \frac{\partial^{k}}{\partial \epsilon^{k}}.$

Using Eqs (4.5) and (4.7) in Eq (4.4), we obtain

$\begin{equation} \ \sum\limits_{k = 0}^{\infty}\epsilon^{k}{\upsilon}_{k}({\omega}, {\phi}) = \sum\limits_{r = 0}^{\theta-1}\left(\frac{\wp}{\varpi}\right)^{\alpha-r-1}{\upsilon}^r({\omega}, 0)+\epsilon\times\left({S}^{-1}\left[\frac{\varpi^\alpha}{\wp^\alpha}{S}\{\mathcal{P}_1\sum\limits_{k = 0}^{\infty}\epsilon^{k}{\upsilon}_{k}({\omega}, {\phi})+ \sum\limits_{k = 0}^{\infty}\epsilon^{k}H_{k}({\upsilon})\}\right]\right). \end{equation}$

(4.8)

When we compare the $\epsilon$ coefficients, we arrive at the following conclusion:

$\begin{equation} \ \begin{split} &\epsilon^{0}:{\upsilon}_{0}({\omega}, {\phi}) = {\upsilon}(0)+ {\upsilon}^{'}(0), \\ &\epsilon^{1}:{\upsilon}_{1}({\omega}, {\phi}) = {S}^{-1}\left[\frac{\varpi^\alpha}{\wp^\alpha}{S}(\mathcal{P}_1[{\omega}]{\upsilon}_{0}({\omega}, {\phi})+H_{0}({\upsilon}))\right], \\ &\epsilon^{2}:{\upsilon}_{2}({\omega}, {\phi}) = {S}^{-1}\left[\frac{\varpi^\alpha}{\wp^\alpha}{S}(\mathcal{P}_1[{\omega}]{\upsilon}_{1}({\omega}, {\phi})+H_{1}({\upsilon}))\right], \\ &.\\ &.\\ &.\\ &\epsilon^{k}:{\upsilon}_{k}({\omega}, {\phi}) = {S}^{-1}\left[\frac{\varpi^\alpha}{\wp^\alpha}{S}(\mathcal{P}_1[{\omega}]{\upsilon}_{k-1}({\omega}, {\phi})+H_{k-1}({\upsilon}))\right], \ \ k > 0, k\in N. \end{split} \end{equation}$

(4.9)

Thus, the analytical solution is

$\begin{equation*} \ {\upsilon}({\omega}, {\phi}) = \lim\limits_{M\rightarrow \infty}\sum\limits_{k = 1}^{M}{\upsilon}_{k}({\omega}, {\phi}). \end{equation*}$

Theorem 4.1. Convergence analysis. Let ${X}$ be a Banach space and let $\upsilon_m({\omega}, {\phi})$ and $\upsilon_{(}(\mu, \psi)$ be in ${X}$ . Suppose $\Theta \in(0, 1)$ , then the series solution $\left\{\upsilon_m({\omega}, {\phi})\right\}_{m = 0}^{\infty}$ which is defined from $\sum_{m = 0}^{\infty} \upsilon_m({\omega}, {\phi})$ converges to the solution of Eq. (7) whenever $\upsilon_m({\omega}, {\phi}) \leq \Theta \upsilon_{m-1}({\omega}, {\phi}) \forall m > \mathbb{N}$ , that is for any given $\varepsilon > 0$ there exists a positive number $\mathbb{N}$ such that $\left\|\upsilon_{m+n}({\omega}, {\phi})\right\| \leq \varepsilon \forall m, n > \mathbb{N}$ . Besides, the absolute error is ^[46]

$\left\|\upsilon({\omega}, {\phi})-\sum\limits_{n = 0}^m \upsilon_n({\omega}, {\phi})\right\| \leq \frac{\Theta^{m+1}}{1-\Theta}\left\|\upsilon_0({\omega}, {\phi})\right\|.$

5. Numerical solutions

The phi-four equation, a nonlinear partial differential equation, finds practical applications across physics and mathematics. It is crucial in describing phenomena like phase transitions in particle physics and condensed matter, guiding insights into superfluidity and superconductivity. In fields such as nonlinear optics, it models optical pulse propagation through solitons in optical fibers. Additionally, the equation's relevance extends to fluid dynamics, cosmology, and mathematical modeling, showcasing its versatility in explaining phenomena ranging from wave behavior to early universe processes, making it an invaluable tool in understanding a wide array of complex systems.

5.1. Example 1

Suppose that we consider the Phi-four equation in fractional form ^[22]:

$\begin{equation} \frac{\partial ^{{\alpha} }{{\upsilon}}({{\omega}}, {{\phi}}) }{\partial {\phi} ^{{\alpha} }} = \frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))-\chi_{1}{\upsilon}({\omega}, {\phi})-\chi_{2}{\upsilon}^{3}({\omega}, {\phi}), \quad 1 < {\alpha} \leq2, \end{equation}$

(5.1)

with

$\begin{equation*} {{\upsilon}}({{\omega}}, 0) = \sqrt{\frac{-\chi_{1}^{2}}{\chi_{2}}}\tanh\Bigg(\chi_{1}\sqrt{\frac{1}{2(\rho^{2}-1)}}{\omega}\Bigg), \ \ \frac{\partial}{\partial{\phi}}{\upsilon}({{\omega}}, 0) = -\chi_{1}\rho\sqrt{\frac{-\chi_{1}^{2}}{2\chi_{2}(\rho^{2}-1)}}{\rm{sech}}^{2}\Bigg(\chi_{1}\sqrt{\frac{1}{2(\rho^{2}-1)}}{\omega}\Bigg). \end{equation*}$

Implementation of the HPTM

On taking the ST, we have

$\begin{equation} \ {S}\left(\frac{\partial^{{\alpha}}{\upsilon}}{\partial {\phi}^{{\alpha}}}\right) = {S}\left[\frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))-\chi_{1}{\upsilon}({\omega}, {\phi})-\chi_{2}{\upsilon}^{3}({\omega}, {\phi})\right], \end{equation}$

(5.2)

$\begin{equation} \ \frac{\wp^\alpha}{\varpi^\alpha} S[{\upsilon}({\omega}, {\phi})]-\sum\limits_{r = 0}^{\theta-1}\left(\frac{\wp}{\varpi}\right)^{\alpha-r-1}{\upsilon}^r({\omega}, 0) = {S}\left[\frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))-\chi_{1}{\upsilon}({\omega}, {\phi})-\chi_{2}{\upsilon}^{3}({\omega}, {\phi})\right]. \end{equation}$

(5.3)

Now by employing inverse ST, we get

$\begin{equation} \ \begin{split} &{\upsilon}({\omega}, {\phi}) = {S}^{-1}[\sum\limits_{r = 0}^{\theta-1}\left(\frac{\wp}{\varpi}\right)^{\alpha-r-1}{\upsilon}^r({\omega}, 0)]+{S}^{-1}\left[\frac{\varpi^\alpha}{\wp^\alpha}\left\{{S}\left[\frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))-\chi_{1}{\upsilon}({\omega}, {\phi})-\chi_{2}{\upsilon}^{3}({\omega}, {\phi})\right]\right\}\right], \\ &{\upsilon}({\omega}, {\phi}) = \Bigg(\sqrt{\frac{-\chi_{1}^{2}}{\chi_{2}}}\tanh\Bigg(\chi_{1}\sqrt{\frac{1}{2(\rho^{2}-1)}}{\omega}\Bigg)-{\phi}\chi_{1}\rho\sqrt{\frac{-\chi_{1}^{2}}{2\chi_{2}(\rho^{2}-1)}}{\rm{sech}}^{2}\Bigg(\chi_{1}\sqrt{\frac{1}{2(\rho^{2}-1)}}{\omega}\Bigg)\Bigg)+\\ &{S}^{-1}\left[\frac{\varpi^\alpha}{\wp^\alpha}\left\{{S}\left[\frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))-\chi_{1}{\upsilon}({\omega}, {\phi})-\chi_{2}{\upsilon}^{3}({\omega}, {\phi})\right]\right\}\right].\\ \end{split} \end{equation}$

(5.4)

By HPM, we attain

$\begin{equation} \begin{split} &\sum\limits_{k = 0}^{\infty}\epsilon^{k}{\upsilon}_{k}({\omega}, {\phi}) = \Bigg(\sqrt{\frac{-\chi_{1}^{2}}{\chi_{2}}}\tanh\Bigg(\chi_{1}\sqrt{\frac{1}{2(\rho^{2}-1)}}{\omega}\Bigg)-{\phi}\chi_{1}\rho\sqrt{\frac{-\chi_{1}^{2}}{2\chi_{2}(\rho^{2}-1)}}{\rm{sech}}^{2}\Bigg(\chi_{1}\sqrt{\frac{1}{2(\rho^{2}-1)}}{\omega}\Bigg)\Bigg)+\\ &\epsilon\Bigg({S}^{-1}\Bigg[\frac{\varpi^\alpha}{\wp^\alpha}{S}\Bigg[\Bigg(\sum\limits_{k = 0}^{\infty}\epsilon^{k}{\upsilon}_{k}({\omega}, {\phi})\Bigg)_{{\omega}{\omega}}-\chi_{1}\Bigg(\sum\limits_{k = 0}^{\infty}\epsilon^{k}{\upsilon}_{k}({\omega}, {\phi})\Bigg)-\chi_{2}\Bigg(\sum\limits_{k = 0}^{\infty}\epsilon^{k}H_{k}({\upsilon})\Bigg)+\Bigg]\Bigg]\Bigg).\\ \end{split} \end{equation}$

(5.5)

The polynomial $H_{k}({\upsilon})$ is used to discard the nonlinear terms.

$\begin{equation} \begin{split} &\sum\limits_{k = 0}^{\infty}\epsilon^{k}H_{k}({\upsilon}) = {\upsilon}^{3}.\\ \end{split} \end{equation}$

(5.6)

Certain terms are computed as

$\begin{equation*} \ \begin{split} &H_{0}({\upsilon}) = {\upsilon}_{0}^{3}, \\ &H_{1}({\upsilon}) = 3{\upsilon}_{0}^{2}{\upsilon}_{1}.\\ \end{split} \end{equation*}$

Comparing the $\epsilon$ coefficients, we obtain

$\begin{equation*} \ \begin{split} &\epsilon^{0}:{\upsilon}_{0}({\omega}, {\phi}) = \Bigg(\sqrt{\frac{-\chi_{1}^{2}}{\chi_{2}}}\tanh\Bigg(\chi_{1}\sqrt{\frac{1}{2(\rho^{2}-1)}}{\omega}\Bigg)-{\phi}\chi_{1}\rho\sqrt{\frac{-\chi_{1}^{2}}{2\chi_{2}(\rho^{2}-1)}}{\rm{sech}}^{2}\Bigg(\chi_{1}\sqrt{\frac{1}{2(\rho^{2}-1)}}{\omega}\Bigg)\Bigg), \\ &\epsilon^{1}:{\upsilon}_{1}({\omega}, {\phi}) = \frac{\chi_{1}^{2}\rho^{2}{\phi}^{{\alpha}}}{8(-1+\rho^{2})\Gamma({\alpha}+1)}{\rm{sech}}^{6}\Bigg(\frac{\chi_{1}{\omega}\sqrt{\frac{1}{-1+\rho^{2}}}}{\sqrt{2}}\Bigg)\Bigg(-3\sqrt{2}\chi_{1}\rho{\phi}\sqrt{\frac{\chi_{1}^{2}}{\chi_{2}-\rho^{2}\chi_{2}}}+2\sqrt{2}\chi_{1}^{3}\rho{\phi}^{3}\sqrt{\frac{\chi_{1}^{2}}{\chi_{2}-\rho^{2}\chi_{2}}}+\\ &2\sqrt{2}\chi_{1}\rho{\phi}\sqrt{\frac{\chi_{1}^{2}}{\chi_{2}-\rho^{2}\chi_{2}}}\cosh\Bigg(\sqrt{2}\chi_{1}{\phi}\sqrt{\frac{1}{-1+\rho^{2}}}\Bigg)+\sqrt{2}\chi_{1}\rho{\phi}\sqrt{\frac{\chi_{1}^{2}}{\chi_{2}-\rho^{2}\chi_{2}}}\cosh\Bigg(2\sqrt{2}\chi_{1}{\phi}\sqrt{\frac{1}{-1+\rho^{2}}}\Bigg)\\ &2\sqrt{\frac{-\chi_{1}^{2}}{\chi_{2}}}\sinh\Bigg(\sqrt{2}\chi_{1}\sqrt{\frac{1}{2(-1+\rho^{2})}}{\omega}\Bigg)+6{\phi}^{2}\Bigg(-\frac{\chi_{1}}{\chi_{2}}\Bigg)^{\frac{3}{2}}\chi_{2}\sinh\Bigg(\sqrt{2}\chi_{1}\sqrt{\frac{1}{2(-1+\rho^{2})}}{\omega}\Bigg)\\ &+\sqrt{-\frac{\chi_{1}^{2}}{\chi_{2}}}\sinh\Bigg(2\sqrt{2}\chi_{1}\sqrt{\frac{1}{(-1+\rho^{2})}}{\omega}\Bigg)\Bigg)\\ \vdots \end{split} \end{equation*}$

Therefore, the analytical solution is

$\begin{equation*} \ \begin{split} &{\upsilon}({{\omega}}, {{\phi}}) = {{\upsilon}}_{0}({{\omega}}, {{\phi}})+{{\upsilon}}_{1}({{\omega}}, {{\phi}})+\cdots.\\ &{\upsilon}({\omega}, {\phi}) = \Bigg(\sqrt{\frac{-\chi_{1}^{2}}{\chi_{2}}}\tanh\Bigg(\chi_{1}\sqrt{\frac{1}{2(\rho^{2}-1)}}{\omega}\Bigg)-{\phi}\chi_{1}\rho\sqrt{\frac{-\chi_{1}^{2}}{2\chi_{2}(\rho^{2}-1)}}{\rm{sech}}^{2}\Bigg(\chi_{1}\sqrt{\frac{1}{2(\rho^{2}-1)}}{\omega}\Bigg)\Bigg)+\\ &\frac{\chi_{1}^{2}\rho^{2}{\phi}^{{\alpha}}}{8(-1+\rho^{2})\Gamma({\alpha}+1)}{\rm{sech}}^{6}\Bigg(\frac{\chi_{1}{\omega}\sqrt{\frac{1}{-1+\rho^{2}}}}{\sqrt{2}}\Bigg)\Bigg(-3\sqrt{2}\chi_{1}\rho{\phi}\sqrt{\frac{\chi_{1}^{2}}{\chi_{2}-\rho^{2}\chi_{2}}}+2\sqrt{2}\chi_{1}^{3}\rho{\phi}^{3}\sqrt{\frac{\chi_{1}^{2}}{\chi_{2}-\rho^{2}\chi_{2}}}+\\ &2\sqrt{2}\chi_{1}\rho{\phi}\sqrt{\frac{\chi_{1}^{2}}{\chi_{2}-\rho^{2}\chi_{2}}}\cosh\Bigg(\sqrt{2}\chi_{1}{\phi}\sqrt{\frac{1}{-1+\rho^{2}}}\Bigg)+\sqrt{2}\chi_{1}\rho{\phi}\sqrt{\frac{\chi_{1}^{2}}{\chi_{2}-\rho^{2}\chi_{2}}}\cosh\Bigg(2\sqrt{2}\chi_{1}{\phi}\sqrt{\frac{1}{-1+\rho^{2}}}\Bigg)\\ &2\sqrt{\frac{-\chi_{1}^{2}}{\chi_{2}}}\sinh\Bigg(\sqrt{2}\chi_{1}\sqrt{\frac{1}{2(-1+\rho^{2})}}{\omega}\Bigg)+6{\phi}^{2}\Bigg(-\frac{\chi_{1}}{\chi_{2}}\Bigg)^{\frac{3}{2}}\chi_{2}\sinh\Bigg(\sqrt{2}\chi_{1}\sqrt{\frac{1}{2(-1+\rho^{2})}}{\omega}\Bigg)\\ &+\sqrt{-\frac{\chi_{1}^{2}}{\chi_{2}}}\sinh\Bigg(2\sqrt{2}\chi_{1}\sqrt{\frac{1}{(-1+\rho^{2})}}{\omega}\Bigg)\Bigg)+\cdots.\\ \end{split} \end{equation*}$

Implementation of the STDM

On taking the ST, we have

$\begin{equation} {S}\left\{\frac{\partial^{{\alpha}}{\upsilon}}{\partial {\phi}^{{\alpha}}}\right\} = {S}\left[\frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))-\chi_{1}{\upsilon}({\omega}, {\phi})-\chi_{2}{\upsilon}^{3}({\omega}, {\phi})\right], \\ \end{equation}$

(5.7)

$\begin{equation} \frac{\wp^\alpha}{\varpi^\alpha} S[{\upsilon}({\omega}, {\phi})]-\sum\limits_{r = 0}^{\theta-1}\left(\frac{\wp}{\varpi}\right)^{\alpha-r-1}{\upsilon}^r({\omega}, 0) = {S}\left[\frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))-\chi_{1}{\upsilon}({\omega}, {\phi})-\chi_{2}{\upsilon}^{3}({\omega}, {\phi})\right]. \end{equation}$

(5.8)

Now by employing inverse ST, we get

$\begin{equation} \ \begin{split} &{\upsilon}({\omega}, {\phi}) = {S}^{-1}[\sum\limits_{r = 0}^{\theta-1}\left(\frac{\wp}{\varpi}\right)^{\alpha-r-1}{\upsilon}^r({\omega}, 0)]+{S}^{-1}\left[\frac{\varpi^\alpha}{\wp^\alpha}\left\{{S}\left[\frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))-\chi_{1}{\upsilon}({\omega}, {\phi})-\chi_{2}{\upsilon}^{3}({\omega}, {\phi})\right]\right\}\right], \\ &{\upsilon}({\omega}, {\phi}) = \Bigg(\sqrt{\frac{-\chi_{1}^{2}}{\chi_{2}}}\tanh\Bigg(\chi_{1}\sqrt{\frac{1}{2(\rho^{2}-1)}}{\omega}\Bigg)-{\phi}\chi_{1}\rho\sqrt{\frac{-\chi_{1}^{2}}{2\chi_{2}(\rho^{2}-1)}}{\rm{sech}}^{2}\Bigg(\chi_{1}\sqrt{\frac{1}{2(\rho^{2}-1)}}{\omega}\Bigg)\Bigg)\\ &+{S}^{-1}\left[\frac{\varpi^\alpha}{\wp^\alpha}\left\{{S}\left[\frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))-\chi_{1}{\upsilon}({\omega}, {\phi})-\chi_{2}{\upsilon}^{3}({\omega}, {\phi})\right]\right\}\right].\\ \end{split} \end{equation}$

(5.9)

Now the solution is

$\begin{equation} \ {\upsilon}({\omega}, {\phi}) = \sum\limits_{m = 0}^{\infty}{\upsilon}_m({\omega}, {\phi}). \end{equation}$

(5.10)

The Adomian polynomials are discarded and the nonlinear term ${\upsilon}^{3}$ is expressed as $\sum_{m = 0}^{\infty}\mathcal{A}_m$ .

$\begin{equation} \begin{split} &\sum\limits_{m = 0}^{\infty}{\upsilon}_m({\omega}, {\phi}) = {\upsilon}({\omega}, 0) +{S}^{-1}\left[\frac{\varpi^\alpha}{\wp^\alpha}\left\{{S}\left[\frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))-\chi_{1}{\upsilon}({\omega}, {\phi})-\sum\limits_{m = 0}^{\infty}\mathcal{A}_m\right]\right\}\right], \\ &\sum\limits_{m = 0}^{\infty}{\upsilon}_m({\omega}, {\phi}) = \Bigg(\sqrt{\frac{-\chi_{1}^{2}}{\chi_{2}}}\tanh\Bigg(\chi_{1}\sqrt{\frac{1}{2(\rho^{2}-1)}}{\omega}\Bigg)-{\phi}\chi_{1}\rho\sqrt{\frac{-\chi_{1}^{2}}{2\chi_{2}(\rho^{2}-1)}}{\rm{sech}}^{2}\Bigg(\chi_{1}\sqrt{\frac{1}{2(\rho^{2}-1)}}{\omega}\Bigg)\Bigg)\\ &+{S}^{-1}\left[\frac{\varpi^\alpha}{\wp^\alpha}\left\{{S}\left[\frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))-\chi_{1}{\upsilon}({\omega}, {\phi})-\sum\limits_{m = 0}^{\infty}\mathcal{A}_m\right]\right\}\right].\\ \end{split} \end{equation}$

(5.11)

Some terms are calculated as

$\begin{equation*} \ \begin{split} &\mathcal{A}_0 = {\upsilon}_{0}^{3}, \\ &\mathcal{A}_1 = 3{\upsilon}_{0}^{2}{\upsilon}_{1}.\\ \end{split} \end{equation*}$

We can easily obtain the approximation by comparing both sides

$\begin{equation*} {\upsilon}_0({\omega}, {\phi}) = \Bigg(\sqrt{\frac{-\chi_{1}^{2}}{\chi_{2}}}\tanh\Bigg(\chi_{1}\sqrt{\frac{1}{2(\rho^{2}-1)}}{\omega}\Bigg)-{\phi}\chi_{1}\rho\sqrt{\frac{-\chi_{1}^{2}}{2\chi_{2}(\rho^{2}-1)}}{\rm{sech}}^{2}\Bigg(\chi_{1}\sqrt{\frac{1}{2(\rho^{2}-1)}}{\omega}\Bigg)\Bigg). \end{equation*}$

On $m = 0$

$\begin{equation*} \ \begin{split} &{\upsilon}_{1}({\omega}, {\phi}) = \frac{\chi_{1}^{2}\rho^{2}{\phi}^{{\alpha}}}{8(-1+\rho^{2})\Gamma({\alpha}+1)}{\rm{sech}}^{6}\Bigg(\frac{\chi_{1}{\omega}\sqrt{\frac{1}{-1+\rho^{2}}}}{\sqrt{2}}\Bigg)\Bigg(-3\sqrt{2}\chi_{1}\rho{\phi}\sqrt{\frac{\chi_{1}^{2}}{\chi_{2}-\rho^{2}\chi_{2}}}+2\sqrt{2}\chi_{1}^{3}\rho{\phi}^{3}\sqrt{\frac{\chi_{1}^{2}}{\chi_{2}-\rho^{2}\chi_{2}}}+\\ &2\sqrt{2}\chi_{1}\rho{\phi}\sqrt{\frac{\chi_{1}^{2}}{\chi_{2}-\rho^{2}\chi_{2}}}\cosh\Bigg(\sqrt{2}\chi_{1}{\phi}\sqrt{\frac{1}{-1+\rho^{2}}}\Bigg)+\sqrt{2}\chi_{1}\rho{\phi}\sqrt{\frac{\chi_{1}^{2}}{\chi_{2}-\rho^{2}\chi_{2}}}\cosh\Bigg(2\sqrt{2}\chi_{1}{\phi}\sqrt{\frac{1}{-1+\rho^{2}}}\Bigg)\\ &2\sqrt{\frac{-\chi_{1}^{2}}{\chi_{2}}}\sinh\Bigg(\sqrt{2}\chi_{1}\sqrt{\frac{1}{2(-1+\rho^{2})}}{\omega}\Bigg)+6{\phi}^{2}\Bigg(-\frac{\chi_{1}}{\chi_{2}}\Bigg)^{\frac{3}{2}}\chi_{2}\sinh\Bigg(\sqrt{2}\chi_{1}\sqrt{\frac{1}{2(-1+\rho^{2})}}{\omega}\Bigg)\\ &+\sqrt{-\frac{\chi_{1}^{2}}{\chi_{2}}}\sinh\Bigg(2\sqrt{2}\chi_{1}\sqrt{\frac{1}{(-1+\rho^{2})}}{\omega}\Bigg)\Bigg)\\ \vdots \end{split} \end{equation*}$

The series form STDM solution are as follows:

$\begin{equation*} {\upsilon}({\omega}, {\phi}) = \sum\limits_{m = 0}^{\infty}{\upsilon}_m({\omega}, {\phi}) = {\upsilon}_0({\omega}, {\phi})+{\upsilon}_1({\omega}, {\phi})+\cdots. \end{equation*}$

$\begin{equation*} \begin{split} &{\upsilon}({\omega}, {\phi}) = \Bigg(\sqrt{\frac{-\chi_{1}^{2}}{\chi_{2}}}\tanh\Bigg(\chi_{1}\sqrt{\frac{1}{2(\rho^{2}-1)}}{\omega}\Bigg)-{\phi}\chi_{1}\rho\sqrt{\frac{-\chi_{1}^{2}}{2\chi_{2}(\rho^{2}-1)}}{\rm{sech}}^{2}\Bigg(\chi_{1}\sqrt{\frac{1}{2(\rho^{2}-1)}}{\omega}\Bigg)\Bigg)+\\ &\frac{\chi_{1}^{2}\rho^{2}{\phi}^{{\alpha}}}{8(-1+\rho^{2})\Gamma({\alpha}+1)}{\rm{sech}}^{6}\Bigg(\frac{\chi_{1}{\omega}\sqrt{\frac{1}{-1+\rho^{2}}}}{\sqrt{2}}\Bigg)\Bigg(-3\sqrt{2}\chi_{1}\rho{\phi}\sqrt{\frac{\chi_{1}^{2}}{\chi_{2}-\rho^{2}\chi_{2}}}+2\sqrt{2}\chi_{1}^{3}\rho{\phi}^{3}\sqrt{\frac{\chi_{1}^{2}}{\chi_{2}-\rho^{2}\chi_{2}}}+\\ &2\sqrt{2}\chi_{1}\rho{\phi}\sqrt{\frac{\chi_{1}^{2}}{\chi_{2}-\rho^{2}\chi_{2}}}\cosh\Bigg(\sqrt{2}\chi_{1}{\phi}\sqrt{\frac{1}{-1+\rho^{2}}}\Bigg)+\sqrt{2}\chi_{1}\rho{\phi}\sqrt{\frac{\chi_{1}^{2}}{\chi_{2}-\rho^{2}\chi_{2}}}\cosh\Bigg(2\sqrt{2}\chi_{1}{\phi}\sqrt{\frac{1}{-1+\rho^{2}}}\Bigg)\\ &2\sqrt{\frac{-\chi_{1}^{2}}{\chi_{2}}}\sinh\Bigg(\sqrt{2}\chi_{1}\sqrt{\frac{1}{2(-1+\rho^{2})}}{\omega}\Bigg)+6{\phi}^{2}\Bigg(-\frac{\chi_{1}}{\chi_{2}}\Bigg)^{\frac{3}{2}}\chi_{2}\sinh\Bigg(\sqrt{2}\chi_{1}\sqrt{\frac{1}{2(-1+\rho^{2})}}{\omega}\Bigg)\\ &+\sqrt{-\frac{\chi_{1}^{2}}{\chi_{2}}}\sinh\Bigg(2\sqrt{2}\chi_{1}\sqrt{\frac{1}{(-1+\rho^{2})}}{\omega}\Bigg)\Bigg)+\cdots.\\ \end{split} \end{equation*}$

If we take ${\alpha} = 2$ , we get the exact solution as

$\begin{equation} \ {\upsilon}({\omega}, {\phi}) = \sqrt{\frac{-\chi_{1}^{2}}{\chi_{2}}}\tanh\Bigg(\chi_{1}\sqrt{\frac{1}{2(\rho^{2}-1)}}({\omega}-\rho{\phi})\Bigg). \end{equation}$

(5.12)

5.2. Example 2

Assuming the fractional Phi-four equation with $\chi_1 = 1$ and $\chi_2 = -1$ ^[22]

$\begin{equation} \frac{\partial ^{{\alpha} }{{\upsilon}}({{\omega}}, {{\phi}}) }{\partial {\phi} ^{{\alpha} }} = \frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))+{\upsilon}({\omega}, {\phi})-{\upsilon}^{3}({\omega}, {\phi}), \quad 1 < {\alpha} \leq2, \end{equation}$

(5.13)

with

$\begin{equation*} {{\upsilon}}({{\omega}}, 0) = \tanh\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg), \ \ \frac{\partial}{\partial{\phi}}{\upsilon}({{\omega}}, 0) = \tanh\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg). \end{equation*}$

Implementation of the HPTM

On taking the ST, we have

$\begin{equation} \ {S}\left(\frac{\partial^{{\alpha}}{\upsilon}}{\partial {\phi}^{{\alpha}}}\right) = {S}\left[\frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))+{\upsilon}({\omega}, {\phi})-{\upsilon}^{3}({\omega}, {\phi})\right], \end{equation}$

(5.14)

$\begin{equation} \ \frac{\wp^\alpha}{\varpi^\alpha} S[{\upsilon}({\omega}, {\phi})]-\sum\limits_{r = 0}^{\theta-1}\left(\frac{\wp}{\varpi}\right)^{\alpha-r-1}{\upsilon}^r({\omega}, 0) = {S}\left[\frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))+{\upsilon}({\omega}, {\phi})-{\upsilon}^{3}({\omega}, {\phi})\right]. \end{equation}$

(5.15)

Now by employing inverse ST, we get

$\begin{equation} \ \begin{split} &{\upsilon}({\omega}, {\phi}) = {S}^{-1}[\sum\limits_{r = 0}^{\theta-1}\left(\frac{\wp}{\varpi}\right)^{\alpha-r-1}{\upsilon}^r({\omega}, 0)]+{S}^{-1}\left[\frac{\varpi^\alpha}{\wp^\alpha}\left\{{S}\left[\frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))+{\upsilon}({\omega}, {\phi})-{\upsilon}^{3}({\omega}, {\phi})\right]\right\}\right], \\ &{\upsilon}({\omega}, {\phi}) = (1+{\phi})\tanh\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)+{S}^{-1}\left[\frac{\varpi^\alpha}{\wp^\alpha}\left\{{S}\left[\frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))+{\upsilon}({\omega}, {\phi})-{\upsilon}^{3}({\omega}, {\phi})\right]\right\}\right].\\ \end{split} \end{equation}$

(5.16)

By HPM, we obtain

$\begin{equation} \begin{split} &\sum\limits_{k = 0}^{\infty}\epsilon^{k}{\upsilon}_{k}({\omega}, {\phi}) = (1+{\phi})\Bigg(\tanh\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)\Bigg)+\\ &\epsilon\Bigg({S}^{-1}\Bigg[\frac{\varpi^\alpha}{\wp^\alpha}{S}\Bigg[\Bigg(\sum\limits_{k = 0}^{\infty}\epsilon^{k}{\upsilon}_{k}({\omega}, {\phi})\Bigg)_{{\omega}{\omega}}+\Bigg(\sum\limits_{k = 0}^{\infty}\epsilon^{k}{\upsilon}_{k}({\omega}, {\phi})\Bigg)-\Bigg(\sum\limits_{k = 0}^{\infty}\epsilon^{k}H_{k}({\upsilon})\Bigg)+\Bigg]\Bigg]\Bigg).\\ \end{split} \end{equation}$

(5.17)

The polynomial $H_{k}(\upsilon)$ is used to eliminate the nonlinear terms.

$\begin{equation} \begin{split} &\sum\limits_{k = 0}^{\infty}\epsilon^{k}H_{k}({\upsilon}) = {\upsilon}^{3}.\\ \end{split} \end{equation}$

(5.18)

Certain terms are computed as

$\begin{equation*} \ \begin{split} &H_{0}({\upsilon}) = {\upsilon}_{0}^{3}, \\ &H_{1}({\upsilon}) = 3{\upsilon}_{0}^{2}{\upsilon}_{1}.\\ \end{split} \end{equation*}$

By comparing the $\epsilon$ coefficients, we can derive the following.

$\begin{equation*} \ \begin{split} &\epsilon^{0}:{\upsilon}_{0}({\omega}, {\phi}) = (1+{\phi})\tanh\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg), \\ &\epsilon^{1}:{\upsilon}_{1}({\omega}, {\phi}) = -\frac{{\phi}^{{\alpha}}}{\Gamma({\alpha}+1)}\tanh\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)\Bigg(-1-{\phi}-\frac{(1+{\phi}){\rm{sech}}^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)}{\kappa^{2}-1}\Bigg)+(1+{\phi})^{3}\\ &\tanh^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg), \\ &\epsilon^{2}:{\upsilon}_{2}({\omega}, {\phi}) = \frac{{\phi}^{2{\alpha}}}{\Gamma(2{\alpha}+1)}\tanh\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)(1+{\phi}+\frac{1+{\phi}}{-1+\kappa^{2}}{\rm{sech}}^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)\\ &\frac{2(1+{\phi})(\kappa^{2}(1+{\phi})^{2}-{\phi}(2+{\phi}))}{(-1+\kappa^{2})^{2}}\times{\rm{sech}}^{4}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)-\\ &\frac{(1+{\phi})(\kappa^{2}(1+{\phi})^{2}-{\phi}(2+{\phi}))\Bigg(-2+\cosh\Bigg(\sqrt{2}\sqrt{\frac{1}{1-\kappa^{2}}}\Bigg)\Bigg){\omega}}{(-1+\kappa^{2})^{2}}\\ &{\rm{sech}}^{4}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)-(1+{\phi})^{3}\tanh^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)+\frac{2{\rm{sech}}^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)}{2-2\kappa^{2}}\\ &(-1-{\phi}-\frac{(1+{\phi}){\rm{sech}}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)^{2}}{-1+\kappa^{2}}+(1+{\phi})^{3}\tanh^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg))+3(1+{\phi})^{2}\tanh^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)\\ &(-1-{\phi}-\frac{(1+{\phi}){\rm{sech}}^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)}{-1+\kappa^{2}}+(1+{\phi})^{3}\tanh^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)))\\ \vdots \end{split} \end{equation*}$

Hence, the analytical solution is

$\begin{equation*} \ \begin{split} &{\upsilon}({{\omega}}, {{\phi}}) = {{\upsilon}}_{0}({{\omega}}, {{\phi}})+{{\upsilon}}_{1}({{\omega}}, {{\phi}})+{{\upsilon}}_{2}({{\omega}}, {{\phi}})+\cdots.\\ &{\upsilon}({\omega}, {\phi}) = (1+{\phi})\tanh\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)-\frac{{\phi}^{{\alpha}}}{\Gamma({\alpha}+1)}\tanh\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)\\ &\Bigg(-1-{\phi}-\frac{(1+{\phi}){\rm{sech}}^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)}{\kappa^{2}-1}\Bigg)+(1+{\phi})^{3}\tanh^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)\\ &-\frac{{\phi}^{2{\alpha}}}{\Gamma(2{\alpha}+1)}\tanh\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)(1+{\phi}+\frac{1+{\phi}}{-1+\kappa^{2}}{\rm{sech}}^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)\\ &\frac{2(1+{\phi})(\kappa^{2}(1+{\phi})^{2}-{\phi}(2+{\phi}))}{(-1+\kappa^{2})^{2}}\times{\rm{sech}}^{4}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)-\\ &\frac{(1+{\phi})(\kappa^{2}(1+{\phi})^{2}-{\phi}(2+{\phi}))\Bigg(-2+\cosh\Bigg(\sqrt{2}\sqrt{\frac{1}{1-\kappa^{2}}}\Bigg)\Bigg){\omega}}{(-1+\kappa^{2})^{2}}\\ &{\rm{sech}}^{4}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)-(1+{\phi})^{3}\tanh^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)+\frac{2{\rm{sech}}^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)}{2-2\kappa^{2}}\\ &(-1-{\phi}-\frac{(1+{\phi}){\rm{sech}}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)^{2}}{-1+\kappa^{2}}+(1+{\phi})^{3}\tanh^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg))+3(1+{\phi})^{2}\tanh^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)\\ &(-1-{\phi}-\frac{(1+{\phi}){\rm{sech}}^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)}{-1+\kappa^{2}}+(1+{\phi})^{3}\tanh^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)))\\ \end{split} \end{equation*}$

Implementation of the STDM

On taking the ST, we attain

$\begin{equation} {S}\left\{\frac{\partial^{{\alpha}}{\upsilon}}{\partial {\phi}^{{\alpha}}}\right\} = {S}\left[\frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))+{\upsilon}({\omega}, {\phi})-{\upsilon}^{3}({\omega}, {\phi})\right], \\ \end{equation}$

(5.19)

$\begin{equation} \frac{\wp^\alpha}{\varpi^\alpha} S[{\upsilon}({\omega}, {\phi})]-\sum\limits_{r = 0}^{\theta-1}\left(\frac{\wp}{\varpi}\right)^{\alpha-r-1}{\upsilon}^r({\omega}, 0) = {S}\left[\frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))+{\upsilon}({\omega}, {\phi})-{\upsilon}^{3}({\omega}, {\phi})\right]. \end{equation}$

(5.20)

Now by employing inverse ST, we get

$\begin{equation} \ \begin{split} &{\upsilon}({\omega}, {\phi}) = {S}^{-1}[\sum\limits_{r = 0}^{\theta-1}\left(\frac{\wp}{\varpi}\right)^{\alpha-r-1}{\upsilon}^r({\omega}, 0)]+{S}^{-1}\left[\frac{\varpi^\alpha}{\wp^\alpha}\left\{{S}\left[\frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))+{\upsilon}({\omega}, {\phi})-{\upsilon}^{3}({\omega}, {\phi})\right]\right\}\right], \\ &{\upsilon}({\omega}, {\phi}) = (1+{\phi})\tanh\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)+{S}^{-1}\left[\frac{\varpi^\alpha}{\wp^\alpha}\left\{{S}\left[\frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))+{\upsilon}({\omega}, {\phi})-{\upsilon}^{3}({\omega}, {\phi})\right]\right\}\right].\\ \end{split} \end{equation}$

(5.21)

Now the solution is

$\begin{equation} \ {\upsilon}({\omega}, {\phi}) = \sum\limits_{m = 0}^{\infty}{\upsilon}_m({\omega}, {\phi}). \end{equation}$

(5.22)

The Adomian polynomials are discarded and the nonlinear term ${\upsilon}^{3}$ is expressed as the summation of $\mathcal{A}_m$ from $m = 0$ to $\infty$ .

$\begin{equation} \begin{split} &\sum\limits_{m = 0}^{\infty}{\upsilon}_m({\omega}, {\phi}) = {\upsilon}({\omega}, 0) +{S}^{-1}\left[\frac{\varpi^\alpha}{\wp^\alpha}\left\{{S}\left[\frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))-\chi_{1}{\upsilon}({\omega}, {\phi})-\sum\limits_{m = 0}^{\infty}\mathcal{A}_m\right]\right\}\right], \\ &\sum\limits_{m = 0}^{\infty}{\upsilon}_m({\omega}, {\phi}) = (1+{\phi})\tanh\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)\\ &+{S}^{-1}\left[\frac{\varpi^\alpha}{\wp^\alpha}\left\{{S}\left[\frac{\partial^{2} }{\partial {\omega}^{2} }({\upsilon}({\omega}, {\phi}))-\chi_{1}{\upsilon}({\omega}, {\phi})-\sum\limits_{m = 0}^{\infty}\mathcal{A}_m\right]\right\}\right].\\ \end{split} \end{equation}$

(5.23)

Some terms are calculated as

$\begin{equation*} \ \begin{split} &\mathcal{A}_0 = {\upsilon}_{0}^{3}, \\ &\mathcal{A}_1 = 3{\upsilon}_{0}^{2}{\upsilon}_{1}.\\ \end{split} \end{equation*}$

The approximation can be readily acquired by comparing each side of the equation.

$\begin{equation*} {\upsilon}_0({\omega}, {\phi}) = (1+{\phi})\tanh\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg). \end{equation*}$

On $m = 0$

$\begin{equation*} \begin{split} &{\upsilon}_1({\omega}, {\phi}) = -\frac{{\phi}^{{\alpha}}}{\Gamma({\alpha}+1)}\tanh\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)\Bigg(-1-{\phi}-\frac{(1+{\phi}){\rm{sech}}^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)}{\kappa^{2}-1}\Bigg)+(1+{\phi})^{3}\\ &\tanh^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg).\\ \end{split} \end{equation*}$

On $m = 1$

$\begin{equation*} \begin{split} &{\upsilon}_2({\omega}, {\phi}) = \frac{{\phi}^{2{\alpha}}}{\Gamma(2{\alpha}+1)}\tanh\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)(1+{\phi}+\frac{1+{\phi}}{-1+\kappa^{2}}{\rm{sech}}^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)\\ &\frac{2(1+{\phi})(\kappa^{2}(1+{\phi})^{2}-{\phi}(2+{\phi}))}{(-1+\kappa^{2})^{2}}\times{\rm{sech}}^{4}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)-\\ &\frac{(1+{\phi})(\kappa^{2}(1+{\phi})^{2}-{\phi}(2+{\phi}))\Bigg(-2+\cosh\Bigg(\sqrt{2}\sqrt{\frac{1}{1-\kappa^{2}}}\Bigg)\Bigg){\omega}}{(-1+\kappa^{2})^{2}}\\ &{\rm{sech}}^{4}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)-(1+{\phi})^{3}\tanh^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)+\frac{2{\rm{sech}}^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)}{2-2\kappa^{2}}\\ &(-1-{\phi}-\frac{(1+{\phi}){\rm{sech}}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)^{2}}{-1+\kappa^{2}}+(1+{\phi})^{3}\tanh^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg))+3(1+{\phi})^{2}\tanh^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)\\ &(-1-{\phi}-\frac{(1+{\phi}){\rm{sech}}^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)}{-1+\kappa^{2}}+(1+{\phi})^{3}\tanh^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg))).\\ \end{split} \end{equation*}$

The series form STDM solution are as follows:

$\begin{equation*} {\upsilon}({\omega}, {\phi}) = \sum\limits_{m = 0}^{\infty}{\upsilon}_m({\omega}, {\phi}) = {\upsilon}_0({\omega}, {\phi})+{\upsilon}_1({\omega}, {\phi})+{\upsilon}_2({\omega}, {\phi})+\cdots. \end{equation*}$

$\begin{equation*} \begin{split} &{\upsilon}({\omega}, {\phi}) = (1+{\phi})\tanh\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)-\frac{{\phi}^{{\alpha}}}{\Gamma({\alpha}+1)}\tanh\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)\\ &\Bigg(-1-{\phi}-\frac{(1+{\phi}){\rm{sech}}^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)}{\kappa^{2}-1}\Bigg)+(1+{\phi})^{3}\tanh^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)\\ &-\frac{{\phi}^{2{\alpha}}}{\Gamma(2{\alpha}+1)}\tanh\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)(1+{\phi}+\frac{1+{\phi}}{-1+\kappa^{2}}{\rm{sech}}^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)\\ &\frac{2(1+{\phi})(\kappa^{2}(1+{\phi})^{2}-{\phi}(2+{\phi}))}{(-1+\kappa^{2})^{2}}\times{\rm{sech}}^{4}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)-\\ &\frac{(1+{\phi})(\kappa^{2}(1+{\phi})^{2}-{\phi}(2+{\phi}))\Bigg(-2+\cosh\Bigg(\sqrt{2}\sqrt{\frac{1}{1-\kappa^{2}}}\Bigg)\Bigg){\omega}}{(-1+\kappa^{2})^{2}}\\ &{\rm{sech}}^{4}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)-(1+{\phi})^{3}\tanh^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)+\frac{2{\rm{sech}}^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)}{2-2\kappa^{2}}\\ &(-1-{\phi}-\frac{(1+{\phi}){\rm{sech}}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)^{2}}{-1+\kappa^{2}}+(1+{\phi})^{3}\tanh^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg))+3(1+{\phi})^{2}\tanh^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)\\ &(-1-{\phi}-\frac{(1+{\phi}){\rm{sech}}^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)}{-1+\kappa^{2}}+(1+{\phi})^{3}\tanh^{2}\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}{{\omega}}\Bigg)\Bigg)\Bigg).\\ \end{split} \end{equation*}$

If we take ${\alpha} = 2$ , we get the exact solution as

$\begin{equation} \ {\upsilon}({\omega}, {\phi}) = \tanh\Bigg(\sqrt{\frac{1}{2(1-\kappa^{2})}}({{\omega}}-\kappa{\phi})\Bigg). \end{equation}$

(5.24)

6. Results and discussion

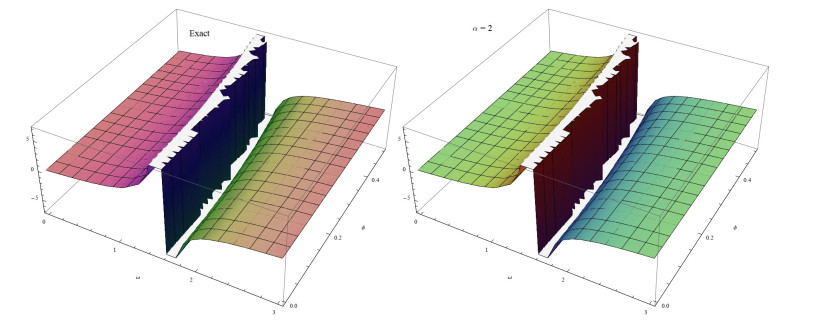
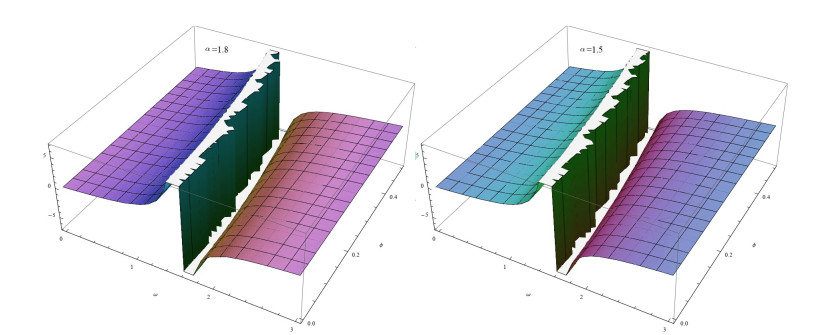
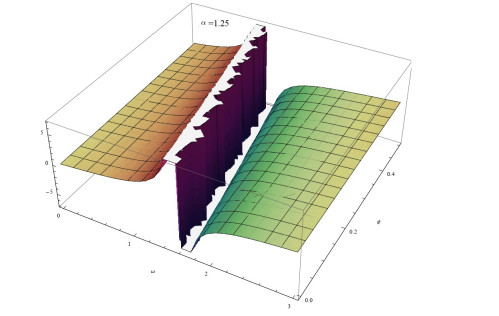
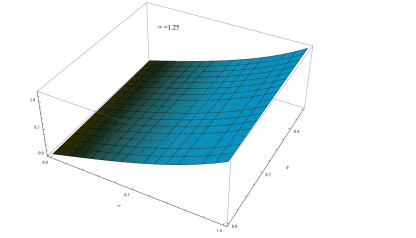
In Example 1 of the Phi-four equation, compares the precise solution to the answer determined by the supplied methodologies for the integer order situation with a value of $\alpha = 2$ at $\chi_1 = 1$ and $\chi_1 = -1$ . depicts this comparison with graphs. The narrative structure allows for a visual assessment of the precision and usefulness of the supplied approaches in approximating the precise solution for this specific situation. The following study focuses on , which depicts the solution produced from the adoption of the suggested approaches for fractional orders. The solutions for fractional orders of $\alpha = 1.8$ and $\alpha = 1.5$ at $\chi_1 = 1$ and $\chi_1 = -1$ are displayed in Example 1's pictures. This comparative research sheds light on how various fractional orders affect the dynamics of the solutions. This allows for the identification of solution trends or variations as the fractional order is changed. A more thorough analysis of the proposed techniques is presented in , which focuses on a fractional order of $\alpha = 1.25$ in Example 1 at $\chi_1 = 1$ and $\chi_1 = -1$ . It is possible to fully assess the accuracy and calibre of the solutions offered by the different approaches in this particular case by concentrating on a single fractional order. displays the absolute error values of the solutions obtained using the suggested techniques in Example 1 for both the integer order $\alpha = 2$ and the multiple fractional orders $\alpha = 1.25$ , $\alpha = 1.5$ , and $\alpha = 1.8$ . The discrepancy between the precise answer and the solutions obtained from the suggested methods is used to gauge how precise the solutions of the supplied strategies are. For every fractional order, the performance and accuracy of the supplied options may be evaluated using the absolute error numbers in Example 1.

Table 1. The absolute error values of the solutions obtained using the proposed methods for different fractional order

$\alpha$ in Example 1.

${\phi}$	${\omega}$	$\alpha={1.25}$	$\alpha={1.5}$	${\alpha}={1.8}$	$\alpha={2}$	$\alpha={2}$ q-HATM ^[22]
0.20	1	$2.2345 \times 10^{-3}$	$1.7601 \times 10^{-5}$	$1.2023 \times 10^{-7}$	$7.8224 \times 10^{-9}$	$7.8224 \times 10^{-9}$
	2	$2.7821 \times 10^{-2}$	$3.3233 \times 10^{-5}$	$2.3367 \times 10^{-6}$	$1.5192 \times 10^{-8}$	$1.5192 \times 10^{-8}$
	3	$1.0279 \times 10^{-2}$	$4.78018 \times 10^{-5}$	$3.3360 \times 10^{-6}$	$2.1684 \times 10^{-8}$	$2.1684 \times 10^{-8}$
	4	$1.7946 \times 10^{-2}$	$6.07350 \times 10^{-5}$	$4.1491 \times 10^{-6}$	$2.6965 \times 10^{-8}$	$2.6965 \times 10^{-8}$
	5	$1.2796 \times 10^{-2}$	$6.84409 \times 10^{-5}$	$4.7420 \times 10^{-6}$	$3.0816 \times 10^{-8}$	$3.0816 \times 10^{-8}$
0.50	1	$1.8597 \times 10^{-3}$	$2.4849 \times 10^{-5}$	$2.0189 \times 10^{-6}$	$1.5626 \times 10^{-8}$	$1.5626 \times 10^{-8}$
	2	$2.7649 \times 10^{-2}$	$4.72858 \times 10^{-5}$	$3.9274 \times 10^{-6}$	$3.0371 \times 10^{-8}$	$3.0371 \times 10^{-8}$
	3	$2.7946 \times 10^{-2}$	$6.8238 \times 10^{-5}$	$5.6089 \times 10^{-6}$	$4.3360 \times 10^{-8}$	$4.3360 \times 10^{-8}$
	4	$1.2379 \times 10^{-2}$	$8.5130 \times 10^{-5}$	$6.9773 \times 10^{-6}$	$5.3928 \times 10^{-8}$	$5.3928 \times 10^{-8}$
	5	$2.8970 \times 10^{-2}$	$9.7255 \times 10^{-5}$	$7.9755 \times 10^{-6}$	$6.1634 \times 10^{-8}$	$6.1634 \times 10^{-8}$
0.80	1	$1.2790 \times 10^{-2}$	$3.0393 \times 10^{-5}$	$2.7327 \times 10^{-6}$	$2.3412 \times 10^{-8}$	$2.3412 \times 10^{-8}$
	2	$1.7462 \times 10^{-2}$	$5.8129 \times 10^{-5}$	$5.3202 \times 10^{-6}$	$4.5535 \times 10^{-8}$	$4.5535 \times 10^{-8}$
	3	$2.2561 \times 10^{-3}$	$8.3556 \times 10^{-5}$	$7.6004 \times 10^{-6}$	$6.5026 \times 10^{-8}$	$6.5026 \times 10^{-8}$
	4	$1.2473 \times 10^{-2}$	$1.0426 \times 10^{-4}$	$9.4564 \times 10^{-7}$	$8.0887 \times 10^{-8}$	$8.0887 \times 10^{-8}$
	5	$1.2479 \times 10^{-2}$	$1.1037 \times 10^{-4}$	$1.0810 \times 10^{-6}$	$9.2455 \times 10^{-8}$	$9.2455 \times 10^{-8}$
1.0	1	$3.9863 \times 10^{-3}$	$3.5056 \times 10^{-5}$	$3.3865 \times 10^{-7}$	$3.1180 \times 10^{-8}$	$3.1180 \times 10^{-8}$
	2	$6.2736 \times 10^{-3}$	$6.7260 \times 10^{-5}$	$6.4868 \times 10^{-7}$	$6.0684 \times 10^{-8}$	$6.0684 \times 10^{-8}$
	3	$9.7456 \times 10^{-3}$	$9.6633 \times 10^{-5}$	$9.3174 \times 10^{-7}$	$8.6683 \times 10^{-8}$	$8.6683 \times 10^{-8}$
	4	$1.5496 \times 10^{-2}$	$1.1255 \times 10^{-4}$	$1.0823 \times 10^{-6}$	$1.0784 \times 10^{-7}$	$1.0784 \times 10^{-7}$
	5	$1.7123 \times 10^{-2}$	$1.2821 \times 10^{-4}$	$1.2314 \times 10^{-6}$	$1.2327 \times 10^{-7}$	$1.2327 \times 10^{-7}$

| Show Table

DownLoad: CSV

Figure 1. The exact solution and the solution obtained using the proposed methods for the integer order

$\alpha = 2$ at

$\chi_{1} = 1$ and

$\chi_{1} = -1$ of Example 1.

DownLoad: Full-Size Img PowerPoint

Figure 2. The solutions obtained using the proposed methods for the fractional orders

$\alpha = 1.8$ and

$1.5$ at

$\chi_{1} = 1$ and

$\chi_{1} = -1$ of Example 1.

DownLoad: Full-Size Img PowerPoint

Figure 3. The solution obtained using the proposed methods for the fractional order

$\alpha = 1.25$ at

$\chi_{1} = 1$ and

$\chi_{1} = -1$ of Example 1.

DownLoad: Full-Size Img PowerPoint

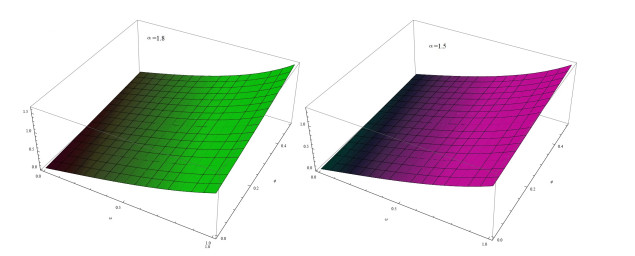
displays the graphs of the precise solution and the solution found using the proposed approaches for the integer order $\alpha = 2$ at $\chi_{1} = 1$ and $\chi_{1} = -1$ in Example 2 of the Phi-four equation. The map facilitates a visual evaluation of the precision and efficacy of the recommended methodologies in approximating the precise solution for this particular case. In , the focus is on the solutions produced using the provided approaches for fractional orders of $\alpha = 1.8$ and $\alpha = 1.5$ at $\chi_{1} = 1$ and $\chi_{1} = -1$ in Example 2. This comparative analysis offers valuable insights into the impact of varying fractional orders on the dynamics of the solutions. This enables the observation of solution variations or trends in response to changes in the fractional order.

Figure 4. The exact solution and the solution obtained using the proposed methods for the integer order

$\alpha = 2$ at

$\chi_{1} = 1$ and

$\chi_{1} = -1$ of Example 2.

DownLoad: Full-Size Img PowerPoint

Figure 5. The solutions obtained using the proposed methods for the fractional orders

$\alpha = 1.8$ and

$1.5$ at

$\chi_{1} = 1$ and

$\chi_{1} = -1$ of Example 2.

DownLoad: Full-Size Img PowerPoint

In Example 2, provides a more detailed analysis of the proposed approaches, specifically focusing on a specific fractional order of $\alpha = 1.25$ at $\chi_{1} = 1$ and $\chi_{1} = -1$ . By directing attention towards a certain fractional order, one may thoroughly examine the precision and attributes of the solutions generated by the suggested methodologies in this specific scenario. The utilisation of numerous graphs depicting different fractional orders enables the examination and juxtaposition of solution behaviour and accuracy in response to alterations in the fractional order.

Figure 6. The solution obtained using the proposed methods for the fractional order

$\alpha = 1.25$ at

$\chi_{1} = 1$ and

$\chi_{1} = -1$ of Example 2.

DownLoad: Full-Size Img PowerPoint

The measurement of the accuracy of the solutions produced through the proposed approaches is achieved by assessing the disparity between the precise solution and the solutions derived from the proposed methods. displays the absolute error values of the solutions derived using the given approaches for several fractional orders, namely $\alpha = 1.25$ , $\alpha = 1.5$ , $\alpha = 1.8$ , and the integer order $\alpha = 2$ , in Example 2. The performance and precision of the offered approaches for each fractional order discussed in Example 2 can be evaluated by analysing the absolute error numbers.

Table 2. The absolute error of the proposed methods of different fractional order of Example 2.

$\phi$	$\omega$	$\alpha=1.25$	$\alpha=1.5$	$\alpha=1.8$	$\alpha=2$
0.20	1	$2.5462 \times 10^{-2}$	$1.7216 \times 10^{-4}$	$6.7701 \times 10^{-8}$	$7.0832 \times 10^{-13}$
	2	$3.5120 \times 10^{-2}$	$2.7439 \times 10^{-4}$	$1.2595 \times 10^{-8}$	$4.4031 \times 10^{-13}$
	3	$4.1456 \times 10^{-2}$	$3.0123 \times 10^{-4}$	$1.7018 \times 10^{-8}$	$1.1304 \times 10^{-13}$
	4	$5.8721 \times 10^{-2}$	$4.5789 \times 10^{-4}$	$1.9756 \times 10^{-8}$	$1.6642 \times 10^{-13}$
	5	$7.2546 \times 10^{-2}$	$4.1587 \times 10^{-4}$	$2.0842 \times 10^{-7}$	$3.3639 \times 10^{-13}$
0.50	1	$1.1596 \times 10^{-2}$	$1.7290 \times 10^{-4}$	$7.5341 \times 10^{-8}$	$1.1326 \times 10^{-11}$
	2	$1.4218 \times 10^{-2}$	$2.5197 \times 10^{-4}$	$1.3864 \times 10^{-8}$	$7.0326 \times 10^{-12}$
	3	$4.2586 \times 10^{-2}$	$3.8712 \times 10^{-4}$	$1.8653 \times 10^{-8}$	$1.7976 \times 10^{-12}$
	4	$5.5200 \times 10^{-2}$	$4.6523 \times 10^{-4}$	$2.1600 \times 10^{-8}$	$2.6707 \times 10^{-12}$
	5	$3.7824 \times 10^{-2}$	$4.8745 \times 10^{-4}$	$2.2749 \times 10^{-7}$	$5.3857 \times 10^{-12}$
0.80	1	$1.1485 \times 10^{-2}$	$1.3578 \times 10^{-4}$	$6.6788 \times 10^{-8}$	$5.7308 \times 10^{-11}$
	2	$3.0148 \times 10^{-2}$	$2.2794 \times 10^{-4}$	$1.2112 \times 10^{-8}$	$3.5546 \times 10^{-11}$
	3	$7.1458 \times 10^{-2}$	$2.7228 \times 10^{-4}$	$1.6203 \times 10^{-8}$	$9.0450 \times 10^{-12}$
	4	$2.7892 \times 10^{-2}$	$3.1272 \times 10^{-4}$	$1.8701 \times 10^{-7}$	$1.3560 \times 10^{-11}$
	5	$2.1755 \times 10^{-2}$	$3.8742 \times 10^{-4}$	$1.9650 \times 10^{-7}$	$2.7284 \times 10^{-11}$
1	1	$7.0178 \times 10^{-2}$	$7.2330 \times 10^{-5}$	$4.8542 \times 10^{-7}$	$1.8101 \times 10^{-10}$
	2	$2.7824 \times 10^{-2}$	$1.28452 \times 10^{-4}$	$8.5846 \times 10^{-7}$	$1.1217 \times 10^{-10}$
	3	$7.0796 \times 10^{-2}$	$1.5424 \times 10^{-4}$	$1.1366 \times 10^{-8}$	$2.8412 \times 10^{-11}$
	4	$2.7021 \times 10^{-2}$	$1.8168 \times 10^{-4}$	$1.3039 \times 10^{-7}$	$4.2984 \times 10^{-11}$
	5	$2.1679 \times 10^{-2}$	$1.8784 \times 10^{-4}$	$1.3644 \times 10^{-7}$	$8.6290 \times 10^{-11}$

| Show Table

DownLoad: CSV

7. Conclusions

In conclusion, my study focused on the nonlinear fractional Phi-four equation, employing the homotopy perturbation and Adomian decomposition methods with the Shehu transform. These analytical techniques are potent tools, particularly for solving fractional differential equations. Comparing the solutions obtained from both methods, we observed a high level of agreement, validating their effectiveness as illustrated in the previous figures and tables. The homotopy Perturbation and Adomian decomposition methods have consistently proven their efficiency and reliability in solving various nonlinear differential equations across disciplines like physics, engineering, and finance. The rising popularity of fractional calculus in modeling complex physical phenomena underscores the significance of the nonlinear fractional Phi-four equation we examined. The solutions obtained by both methods enhance our understanding and predictive capabilities for such systems. In summary, the application of the homotopy perturbation method and the Adomian decomposition method, with the Shehu transform, offers an efficient and accurate approach to solving the Nonlinear fractional Phi-four equation. Furthermore, these methods can be extended to address other nonlinear fractional differential equations, contributing significantly to the advancement of mathematical modeling in science and engineering.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Funding

Princess Nourah bint Abdulrahman University Researchers Supporting Project number (PNURSP2023R183), Princess Nourah bint Abdulrahman University, Riyadh, Saudi Arabia. This work was supported by the Deanship of Scientific Research, the Vice Presidency for Graduate Studies and Scientific Research, King Faisal University, Saudi Arabia (Grant No. 4126).

Acknowledgements

Conflict of interest

The authors declare that they have no competing interests.

References

[1]	S. Browne, Optimal investment policies for a firm with a random risk process: exponential utility and minimizing the probability of ruin, Math. Oper. Res., 20 (1995), 937–958. https://doi.org/10.1287/moor.20.4.937 doi: 10.1287/moor.20.4.937
[2]	J. C. Cox, S. A. Ross, The valuation of options for alternative stochastic processes, J. Financ. Econ., 3 (1976), 145–166. https://doi.org/10.1016/0304-405x(76)90023-4 doi: 10.1016/0304-405x(76)90023-4
[3]	S. Asmussen, M. Taksar, Controlled diffusion models for optimal dividend pay-out, Insur. Math. Econ., 20 (1997), 1–15. https://doi.org/10.1016/s0167-6687(96)00017-0 doi: 10.1016/s0167-6687(96)00017-0
[4]	M. I. Taksar, Optimal risk and dividend distribution control models for an insurance company, Math. Method. Oper. Res., 51 (2000), 1–42. https://doi.org/10.1007/s001860050001 doi: 10.1007/s001860050001
[5]	L. Bai, J. Guo, Optimal proportional reinsurance and investment with multiple risky assets and no-shorting constraint, Insur. Math. Econ., 42 (2008), 968–975. https://doi.org/10.1016/j.insmatheco.2007.11.002 doi: 10.1016/j.insmatheco.2007.11.002
[6]	Z. Sun, K. C. Yuen, J. Guo, A BSDE approach to a class of dependent risk model of mean-variance insurers with stochastic volatility and no-short selling, J. Comput. Appl. Math., 366 (2020), 112413. https://doi.org/10.1016/j.cam.2019.112413 doi: 10.1016/j.cam.2019.112413
[7]	A. Gu, X. Guo, Z. Li, Y. Zeng, Optimal control of excess-of-loss reinsurance and investment for insurers under a CEV model, Insur. Math. Econ., 51 (2012), 674–684. https://doi.org/10.1016/j.insmatheco.2012.09.003 doi: 10.1016/j.insmatheco.2012.09.003
[8]	J. Cao, D. Landriault, B. Li, Optimal reinsurance-investment strategy for a dynamic contagion claim model, Insur. Math. Econ., 93 (2020), 206–215. https://doi.org/10.1016/j.insmatheco.2020.04.013 doi: 10.1016/j.insmatheco.2020.04.013
[9]	X. Jiang, K. C. Yuen, M. Chen, Optimal investment and reinsurance with premium control, J. Ind. Manag. Optim., 16 (2020), 2781–2797. https://doi.org/10.3934/jimo.2019080 doi: 10.3934/jimo.2019080
[10]	L. Xu, D. Yao, G. Cheng, Optimal investment and dividend for an insurer under a Markov regime switching market with high gain tax, J. Ind. Manag. Optim., 16 (2020), 325–356. https://doi.org/10.3934/jimo.2018154 doi: 10.3934/jimo.2018154
[11]	Y. Zhang, P. Zhao, B. Kou, Optimal excess-of-loss reinsurance and investment problem with thinning dependent risks under Heston model, J. Comput. Appl. Math., 382 (2021), 113082. https://doi.org/10.1016/j.cam.2020.113082 doi: 10.1016/j.cam.2020.113082
[12]	Z. Sun, X. Zhang, K. C. Yuen, Mean-variance asset-liability management with affine diffusion factor process and a reinsurance option, Scand. Actuar. J., 2020 (2020), 218–244. https://doi.org/10.1080/03461238.2019.1658619 doi: 10.1080/03461238.2019.1658619
[13]	M. Chen, K. C. Yuen, W. Wang, Optimal reinsurance and dividends with transaction costs and taxes under thinning structure, Scand. Actuar. J., 2021 (2021), 198–217. https://doi.org/10.1080/03461238.2020.1824158 doi: 10.1080/03461238.2020.1824158
[14]	M. Kaluszka, Optimal reinsurance under mean-variance premium principles, Insur. Math. Econ., 28 (2001), 61–67. https://doi.org/10.1016/s0167-6687(00)00066-4 doi: 10.1016/s0167-6687(00)00066-4
[15]	M. Kaluszka, Mean-variance optimal reinsurance arrangements, Scand. Actuar. J., 2004 (2004), 28–41. https://doi.org/10.1080/03461230410019222 doi: 10.1080/03461230410019222
[16]	Z. Liang, K. C. Yuen, Optimal dynamic reinsurance with dependent risks: variance premium principle, Scand. Actuar. J., 2016 (2016), 18–36. https://doi.org/10.1080/03461238.2014.892899 doi: 10.1080/03461238.2014.892899
[17]	X. Zhang, H. Meng, Y. Zeng, Optimal investment and reinsurance strategies for insurers with generalized mean-variance premium principle and no-short selling, Insur. Math. Econ., 67 (2016), 125–132. https://doi.org/10.1016/j.insmatheco.2016.01.001 doi: 10.1016/j.insmatheco.2016.01.001
[18]	X. Liang, Z. Liang, V. R. Young, Optimal reinsurance under the mean-variance premium principle to minimize the probability of ruin, Insur. Math. Econ., 92 (2020), 128–146. https://doi.org/10.1016/j.insmatheco.2020.03.008 doi: 10.1016/j.insmatheco.2020.03.008
[19]	K. Borch, Reciprocal reinsurance treaties, ASTIN Bulletin: The Journal of the IAA, 1 (1960), 170–191. https://doi.org/10.1017/s0515036100009557 doi: 10.1017/s0515036100009557
[20]	V. K. Kaishev, Optimal retention levels, given the joint survival of cedent and reinsurer, Scand. Actuar. J., 2004 (2004), 401–430. https://doi.org/10.1080/03461230410020437 doi: 10.1080/03461230410020437
[21]	V. K. Kaishev, D. S. Dimitrova, Excess of loss reinsurance under joint survival optimality, Insur. Math. Econ., 39 (2006), 376–389. https://doi.org/10.1016/j.insmatheco.2006.05.005 doi: 10.1016/j.insmatheco.2006.05.005
[22]	J. Cai, Y. Fang, Z. Li, G. E. Willmot, Optimal reciprocal reinsurance treaties under the joint survival probability and the joint profitable probability, J. Risk. Insur., 80 (2013), 145–168. https://doi.org/10.1111/j.1539-6975.2012.01462.x doi: 10.1111/j.1539-6975.2012.01462.x
[23]	D. Li, X. Rong, H. Zhao, Optimal reinsurance-investment problem for maximizing the product of the insurer's and the reinsurer's utilities under a CEV model, J. Comput. Appl. Math., 255 (2014), 671–683. https://doi.org/10.1016/j.cam.2013.06.033 doi: 10.1016/j.cam.2013.06.033
[24]	D. Li, X. Rong, H. Zhao, Time-consistent reinsurance-investment strategy for an insurer and a reinsurer with mean-variance criterion under the CEV model, J. Comput. Appl. Math., 283 (2015), 142–162. https://doi.org/10.1016/j.cam.2015.01.038 doi: 10.1016/j.cam.2015.01.038
[25]	H. Zhao, C. Weng, Y. Shen, Y. Zeng, Time-consistent investment-reinsurance strategies towards joint interests of the insurer and the reinsurer under CEV models, Sci. China Math., 60 (2017), 317–344. https://doi.org/10.1007/s11425-015-0542-7 doi: 10.1007/s11425-015-0542-7
[26]	N. Zhang, Z. Jin, L. Qian, R. Wang, Optimal quota-share reinsurance based on the mutual benefit of insurer and reinsurer, J. Comput. Appl. Math., 342 (2018), 337–351. https://doi.org/10.1016/j.cam.2018.04.030 doi: 10.1016/j.cam.2018.04.030
[27]	Y. Bai, Z. Zhou, R. Gao, H. Xiao, Nash equilibrium investment-reinsurance strategies for an insurer and a reinsurer with intertemporal restrictions and common interests, Mathematics, 8 (2020), 139. https://doi.org/10.3390/math8010139 doi: 10.3390/math8010139
[28]	Y. Huang, Y. Ouyang, L. Tang, J. Zhou, Robust optimal investment and reinsurance problem for the product of the insurer's and the reinsurer's utilities, J. Comput. Appl. Math., 344 (2018), 532–552. https://doi.org/10.1016/j.cam.2018.05.060 doi: 10.1016/j.cam.2018.05.060
[29]	J. Grandell, Aspects of risk theory, New York: Springer, 1991. https://doi.org/10.1007/978-1-4613-9058-9
[30]	T. S. Ferguson, Betting systems which minimize the probability of ruin, J. Soc. Indust. Appl. Math., 13 (1965), 795–818. https://doi.org/10.1137/0113051 doi: 10.1137/0113051
[31]	H. U. Gerber, An introduction to mathematical risk theory, New York: R. D. Irwin, 1979.
[32]	W. H. Fleming, H. M. Soner, Controlled Markov processes and viscosity solutions, 2 Eds., New York: Springer, 2006. https://doi.org/10.1007/0-387-31071-1
[33]	X. Zeng, M. Taksar, A stochastic volatility model and optimal portfolio selection, Quant. Financ., 13 (2013), 1547–1558. https://doi.org/10.1080/14697688.2012.740568 doi: 10.1080/14697688.2012.740568

This article has been cited by:

1.	Jamshad Ahmad, Tayyaba Younas, Dynamical behavior of soliton solutions to the fractional phi-four model via two analytical techniques, 2024, 38, 0217-9849, 10.1142/S021798492450310X
2.	M. Mossa Al-Sawalha, Humaira Yasmin, Rasool Shah, Abdul Hamid Ganie, Khaled Moaddy, Unraveling the Dynamics of Singular Stochastic Solitons in Stochastic Fractional Kuramoto–Sivashinsky Equation, 2023, 7, 2504-3110, 753, 10.3390/fractalfract7100753

Reader Comments

Your name:*

Email:*
© 2023 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)