Numerical study of fractional phi-4 equation

1.   Introduction

Over the last two decades, many researches used LKF method to get stability results for time-delay systems ^[1,2]. The LKF method has two important technical steps to reduce the conservatism of the stability conditions. The one is how to construct an appropriate LKF, and the other is how to estimate the derivative of the given LKF. For the first one, several types of LKF are introduced, such as integral delay partitioning method based on LKF ^[3], the simple LKF ^[4,5], delay partitioning based LKF ^[6], polynomial-type LKF ^[7], the augmented LKF ^[8,9,10]. The augmented LKF provides more freedom than the simple LKF in the stability criteria because of introducing several extra matrices. The delay partitioning based LKF method can obtain less conservative results due to introduce several extra matrices and state vectors. For the second step, several integral inequalities have been widely used, such as Jensen inequality ^{[11,12,13,14]}, Wirtinger inequality ^[15,16], free-matrix-based integral inequality ^[17], Bessel-Legendre inequalities ^[18] and the further improvement of Jensen inequality ^{[19,20,21,22,23,24,25]}. The further improvement of Jensen inequality ^[22] is less conservative than other inequalities. However, The interaction between the delay partitioning method and the further improvement of Jensen inequality ^[23] was not considered fully, which may increase conservatism. Thus, there exists room for further improvement.

This paper further researches the stability of distributed time-delay systems and aims to obtain upper bounds of time-delay. A new LKF is introduced via the delay partitioning method. Then, a less conservative stability criterion is obtained by using the further improvement of Jensen inequality ^[22]. Finally, an example is provided to show the advantage of our stability criterion. The contributions of our paper are as follows:

$\bullet$ The integral inequality in ^[23] is more general than previous integral inequality. For $r = 0, 1, 2, 3$, the integral inequality in ^[23] includes those in ^{[12,15,21,22]} as special cases, respectively.

$\bullet$ An augmented LKF which contains general multiple integral terms is introduced to reduce the conservatism via a generalized delay partitioning approach. For example, the $\int_{t-\frac{1}{m}h}^{t} x(s)ds$, $\int_{t-\frac{1}{m}h}^{t}\int_{u_1}^{t} x(s)dsdu_1$, $\cdots$, $\int_{t-\frac{1}{m}h}^{t}\int_{u_1}^{t}\cdots\int_{u_{N-1}}^t x(s)dsdu_{N-1}\cdots d_{u_1}$ are added as state vectors in the LKF, which may reduce the conservatism.

$\bullet$ In this paper, a new LKF is introduced based on the delay interval $[0, h]$ is divided into $m$ segments equally. From the LKF, we can conclude that the relationship among $x(s)$, $x(s-\frac{1}{m}h)$ and $x(s-\frac{m-1}{m}h)$ is considered fully, which may yield less conservative results.

Notation: Throughout this paper, $\mathbb{R}^m$ denotes $m$-dimensional Euclidean space, $A^{\top}$ denotes the transpose of the A matrix, $0$ denotes a zero matrix with appropriate dimensions.

2.   Preliminary

Consider the following time-delay system:

where $x(t)\in R^{n}$ is the state vector, $A, B_1, B_2 \in R^{n\times n}$ are constant matrices. $h > 0$ is a constant time-delay and $\Phi(t)$ is initial condition.

$\bf{Lemma\; 2.1.}$ ^[23] For any matrix $R > 0$ and a differentiable function $x(s), \; s\in [a, b]$, the following inequality holds:

where

$\bf {Remark\; 2.1.}$ The integral inequality in Lemma 2.1 is more general than previous integral inequality. For $r = 0, 1, 2, 3$, the integral inequality (2.3) includes those in ^{[12,15,21,22]} as special cases, respectively.

3.   Results

$\bf{Theorem\; 3.1.}$ For given integers $m > 0, \; N > 0$, scalar $h > 0$, system (2.1) is asymptotically stable, if there exist matrices $P > 0$, $Q > 0$, $R_i > 0, \; i = 1, 2, \cdots, m$, such that

where

Proof. Let an integer $m > 0$, $[0, h]$ can be decomposed into $m$ segments equally, i.e., $[0, h] = \bigcup_{i = 1}^{m}[\frac{i-1}{m}h, \frac{i}{m}h]$. The system (2.1) is transformed into

Then, a new LKF is introduced as follows:

where

The derivative of $V(x_t)$ is given by

Then, one can obtain

By Lemma 2.1, one can obtain

Thus, we have $\dot V(x_t)\le \phi^T(t)\Psi \phi(t)$ by (3.4) and (3.5). We complete the proof.

$\bf {Remark\; 3.1.}$ An augmented LKF which contains general multiple integral terms is introduced to reduce the conservatism via a generalized delay partitioning approach. For example, the $\int_{t-\frac{1}{m}h}^{t} x(s)ds$, $\int_{t-\frac{1}{m}h}^{t}\int_{u_1}^{t} x(s)dsdu_1$, $\cdots$, $\int_{t-\frac{1}{m}h}^{t}\int_{u_1}^{t}\cdots\int_{u_{N-1}}^t x(s)dsdu_{N-1}\cdots d_{u_1}$ are added as state vectors in the LKF, which may reduce the conservatism.

$\bf {Remark\; 3.2.}$ For $r = 0, 1, 2, 3$, the integral inequality (3.5) includes those in ^{[12,15,21,22]} as special cases, respectively. This may yield less conservative results. It is worth noting that the number of variables in our result is less than that in ^[23].

$\bf {Remark\; 3.3.}$ Let $B_2 = 0$, the system (2.1) can reduces to system (1) with $N = 1$ in ^[23]. For $m = 1$, the LKF in this paper can reduces to LKF in ^[23]. So the LKF in our paper is more general than that in ^[23].

4.   Numerical examples

This section gives a numerical example to test merits of our criterion.

Example 4.1. Consider system (2.1) with $m = 2, \; N = 3$ and

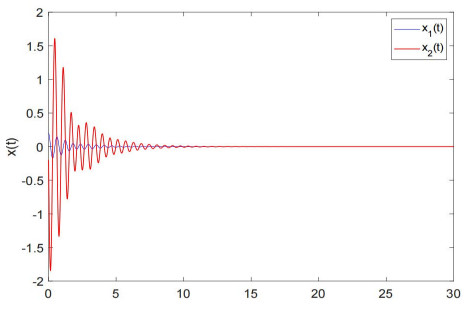
Table 1 lists upper bounds of $h$ by our methods and other methods in ^{[15,20,21,22,23]}. Table 1 shows that our method is more effective than those in ^{[15,20,21,22,23]}. It is worth noting that the number of variables in our result is less than that in ^[23]. Furthermore, let $h = 1.141$ and $x(0) = [0.2, -0.2]^T$, the state responses of system (1) are given in Figure 1. Figure 1 shows the system (2.1) is stable.

Table 1.  $h_{max}$ for different methods.

Methods	$h_{max}$	NoDv
[15]	0.126	16
[20]	0.577	75
[21]	0.675	45
[22]	0.728	45
[23]	0.752	84
Theorem 3.1	1.141	71
Theoretical maximal value	1.463	$-$

---

 | Show Table

DownLoad: CSV

Figure 1.  The state trajectories of the system (2.1) of Example 4.1.

DownLoad: Full-Size Img PowerPoint

5.   Conclusions

In this paper, a new LKF is introduced via the delay partitioning method. Then, a less conservative stability criterion is obtained by using the further improvement of Jensen inequality. Finally, an example is provided to show the advantage of our stability criterion.

Acknowledgments

This work was supported by Basic Research Program of Guizhou Province (Qian Ke He JiChu[2021]YiBan 005); New Academic Talents and Innovation Program of Guizhou Province (Qian Ke He Pingtai Rencai[2017]5727-19); Project of Youth Science and Technology Talents of Guizhou Province (Qian Jiao He KY Zi[2020]095).

Conflict of interest

The authors declare that there are no conflicts of interest.