Complicate dynamical analysis of a discrete predator-prey model with a prey refuge

A. Q. Khan; Ibraheem M. Alsulami; A. Q. Khan; Ibraheem M. Alsulami

doi:10.3934/math.2023768

AIMS Mathematics

2023, Volume 8, Issue 7: 15035-15057. doi: 10.3934/math.2023768

Previous Article Next Article

Research article Special Issues

Complicate dynamical analysis of a discrete predator-prey model with a prey refuge

A. Q. Khan ^{1
,
,},
Ibraheem M. Alsulami ²

1.
Department of Mathematics, University of Azad Jammu and Kashmir, Muzaffarabad 13100, Pakistan
2.
Department of Mathematical Science, Faculty of Applied Science, Umm Al-Qura University, Makkah 21955, Saudi Arabia

Received: 12 January 2023 Revised: 01 April 2023 Accepted: 12 April 2023 Published: 23 April 2023
MSC : 70K50, 92D25, 40A05

In this paper, some complicated dynamic characteristics are formulated for a discrete predator-prey model with a prey refuge. After studying the local dynamical properties about fixed points, our main purpose is to investigate condition(s) for the occurrence of flip and hopf bifurcations, respectively. Further, by the bifurcation theory, we have studied flip bifurcation at boundary fixed point, and flip and hopf bifurcations at interior fixed point of the discrete model. We have also studied chaos by state feedback control strategy. Furthermore, theoretical results are numerically verified. Finally, we have also discussed the influence of prey refuge in the discrete model.

Keywords:

Citation: A. Q. Khan, Ibraheem M. Alsulami. Complicate dynamical analysis of a discrete predator-prey model with a prey refuge[J]. AIMS Mathematics, 2023, 8(7): 15035-15057. doi: 10.3934/math.2023768

Related Papers:

[1]	Cuiying Li, Rui Wu, Ranzhuo Ma . Existence of solutions for Caputo fractional iterative equations under several boundary value conditions. AIMS Mathematics, 2023, 8(1): 317-339. doi: 10.3934/math.2023015
[2]	Ahmed Alsaedi, Fawziah M. Alotaibi, Bashir Ahmad . Analysis of nonlinear coupled Caputo fractional differential equations with boundary conditions in terms of sum and difference of the governing functions. AIMS Mathematics, 2022, 7(5): 8314-8329. doi: 10.3934/math.2022463
[3]	Murat A. Sultanov, Vladimir E. Misilov, Makhmud A. Sadybekov . Numerical method for solving the subdiffusion differential equation with nonlocal boundary conditions. AIMS Mathematics, 2024, 9(12): 36385-36404. doi: 10.3934/math.20241726
[4]	Naimi Abdellouahab, Keltum Bouhali, Loay Alkhalifa, Khaled Zennir . Existence and stability analysis of a problem of the Caputo fractional derivative with mixed conditions. AIMS Mathematics, 2025, 10(3): 6805-6826. doi: 10.3934/math.2025312
[5]	Xinwei Su, Shuqin Zhang, Lixin Zhang . Periodic boundary value problem involving sequential fractional derivatives in Banach space. AIMS Mathematics, 2020, 5(6): 7510-7530. doi: 10.3934/math.2020481
[6]	Ayub Samadi, Chaiyod Kamthorncharoen, Sotiris K. Ntouyas, Jessada Tariboon . Mixed Erdélyi-Kober and Caputo fractional differential equations with nonlocal non-separated boundary conditions. AIMS Mathematics, 2024, 9(11): 32904-32920. doi: 10.3934/math.20241574
[7]	Karim Guida, Lahcen Ibnelazyz, Khalid Hilal, Said Melliani . Existence and uniqueness results for sequential $\psi$ -Hilfer fractional pantograph differential equations with mixed nonlocal boundary conditions. AIMS Mathematics, 2021, 6(8): 8239-8255. doi: 10.3934/math.2021477
[8]	Nichaphat Patanarapeelert, Thanin Sitthiwiratthame . On nonlocal fractional symmetric Hanh integral boundary value problems for fractional symmetric Hahn integrodifference equation. AIMS Mathematics, 2020, 5(4): 3556-3572. doi: 10.3934/math.2020231
[9]	Abdelkader Amara . Existence results for hybrid fractional differential equations with three-point boundary conditions. AIMS Mathematics, 2020, 5(2): 1074-1088. doi: 10.3934/math.2020075
[10]	Yige Zhao, Yibing Sun, Zhi Liu, Yilin Wang . Solvability for boundary value problems of nonlinear fractional differential equations with mixed perturbations of the second type. AIMS Mathematics, 2020, 5(1): 557-567. doi: 10.3934/math.2020037

Abstract

1. Introduction

In graph theory, graph labeling is an assignment of labels or weights to the vertices and edges of a graph. Graph labeling plays an important role in many fields such as computer science, coding theory and physics ^[32]. Baca et al. ^[10] have introduced the definition of an edge irregular total $\ell$ -labeling of any graph as a labeling $\mathcal{L}:V \cup E\to \left\{\mathrm{1, 2}, 3, \dots, \ell\right\}$ in which every two distinct edges $fh$ and ${f}^{*}{h}^{*}$ of a graph $G$ have distinct weights, this means that ${w}_{\mathcal{L}}\left(fh\right)\ne {w}_{\mathcal{L}}\left({f}^{*}{h}^{*}\right)$ where ${w}_{\mathcal{L}}\left(fh\right) = \mathcal{L}\left(f\right)+\mathcal{L}\left(h\right)+\mathcal{L}\left(fh\right)$ . They have deduced inequality which gives a lower bound of $tes\left(G\right)$ for a graph $G$ ,

$tes\left(G\right)\ge max\left\{\left\lceil {\frac{\left|E\left(G\right)\right|+2}{3}} \right\rceil , \left\lceil {\frac{\Delta +1}{2}} \right\rceil \right\}$

(1)

Also, they have introduced the exact value of TEIS, $tes\left(G\right)$ for some families of graphs like fan graph ${F}_{n}$ and wheel graph ${W}_{n}$ ,

$tes\left({F}_{n}\right) = \left\lceil {\frac{3n+2}{3}} \right\rceil \text{, }$

$tes\left({W}_{n}\right) = \left\lceil {\frac{2n+2}{3}} \right\rceil \text{.}$

In ^[15] authors have proved that for any tree $T$

$tes\left(T\right) = max\left\{\left\lceil {\frac{k+1}{3}} \right\rceil , \left\lceil {\frac{\Delta +1}{2}} \right\rceil \right\},$

where $\Delta$ is maximum degree on $k$ vertices. In addition, Salama ^[26] investigated the exact value of TEIS for a polar grid graph,

$tes\left({P}_{m, n}\right) = \left\lceil {\frac{2mn+2}{3}} \right\rceil \text{.}$

Authors in ^[1] determined $\mathrm{T}\mathrm{E}\mathrm{I}\mathrm{S}$ for zigzag graphs. Also, the exact value of TEIS of the generalized web graph ${W}_{n, m}$ and some families has been determined, see ^[14]. Tilukay et al. ^[31] have investigated total irregularity strength for a wheel graph, a fan graph, a triangular Book graph and a friendship graph. On the other hand, in ^{[2,3,8,17,20,24,29]} the total edge irregularity strengths for hexagonal grid graphs, centralized uniform theta graphs, generalized helm graph, series parallel graphs, disjoint union of isomorphic copies of generalized Petersen graph, disjoint union of wheel graphs, subdivision of star ${S}_{n}$ and categorical product of two cycles have been investigated. For more details, see ^{[4,5,6,7,9,11,12,13,16,18,19,21,23,25,27,28,30]}.

A generalized theta graph $\theta ({t}_{1}, {t}_{2}, \dots, {t}_{n})$ is a pair of $n$ internal disjoint paths with lengths at least two joined by end vertices where the end vertices are named south pole $S$ and north pole $N$ and ${t}_{i}$ is the number of vertices in the nth path. Uniform theta graph $\theta (t, m)$ is a generalized theta graph in which all paths have the same numbers of internal vertices, for more details see ^[22].

In this paper, we have defined a new type of family of graph called uniform theta snake graph, ${\theta }_{n}(t, m)$ . Also, the exact value of TEIS for some special types of the new family has been determined.

2. Main results

In the following, we define a new type of graph which is called uniform theta snake graph.

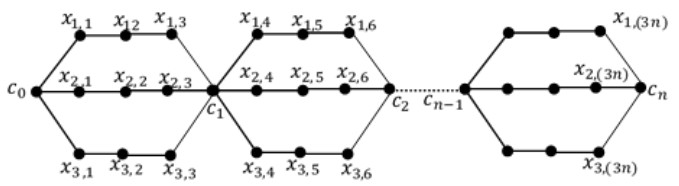
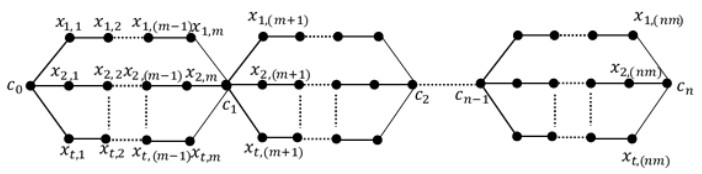
Definition 1. If we replace each edge of a path ${P}_{n}$ by a uniform theta graph $\theta (t, m)$ , we have a uniform theta snake graph ${\theta }_{n}(t, m)$ . See Figure 1.

Figure 1. Uniform theta snake graph θ(t, m).

DownLoad: Full-Size Img PowerPoint

It is clear that for a uniform theta snake graph $\left|\mathrm{E}\left({\mathtt{θ }}_{\mathrm{n}}\right(\mathrm{t}, \mathrm{m}\left)\right)\right| = \mathrm{t}\left(\mathrm{m}+1\right)\mathrm{n}$ and $\left|\mathrm{V}\left({\mathtt{θ }}_{\mathrm{n}}\right(\mathrm{t}, \mathrm{m}\left)\right)\right| = \left(\mathrm{t}\mathrm{m}+1\right)\mathrm{n}+1$ . In this section, we determine the exact value of TEIS for uniform theta snake graph ${\mathtt{θ }}_{\mathrm{n}}\left(\mathrm{3, 3}\right)$ , ${\mathtt{θ }}_{\mathrm{n}}\left(3, \mathrm{m}\right)$ , ${\mathtt{θ }}_{\mathrm{n}}(\mathrm{t}, 3)$ , ${\mathtt{θ }}_{\mathrm{n}}\left(4, \mathrm{m}\right)$ , and ${\mathtt{θ }}_{\mathrm{n}}(\mathrm{t}, 4)$ .

Theorem 1. For a uniform theta snake graph ${\theta }_{n}\left(\mathrm{3, 3}\right)$ with 10 $n+1$ vertices and 12 $n$ edges, we have

$tes\left({\theta }_{n}\left(\mathrm{3, 3}\right)\right) = 4n+1\text{.}$

Proof. Since a uniform theta snake graph ${\theta }_{n}\left(\mathrm{3, 3}\right)$ has 12 $n$ edges and $\left({\theta }_{n}\left(\mathrm{3, 3}\right)\right) = 6$ , then from (1) we have:

$tes\left({\theta }_{n}\left(\mathrm{3, 3}\right)\right)\ge 4n+1\text{.}$

To prove the invers inequality, we show that $ħ-$ labeling is an edge irregular total for ${\theta }_{n}\left(\mathrm{3, 3}\right)$ , see , and $ħ = 4n+1$ . Let $ħ = 4n+1$ and a total $ħ-$ labeling $\alpha :V{(\theta }_{n}\left(\mathrm{3, 3}\right)) \cup E({\theta }_{n}\left(\mathrm{3, 3}\right))\to \left\{\mathrm{1, 2}, 3, \dots, \mathrm{ħ}\right\}$ is defined as:

Figure 2. Uniform theta snake graph θ(3, 3).

DownLoad: Full-Size Img PowerPoint

$\mathtt{α }\left({\mathrm{c}}_{0}\right) = 1,$

$\mathtt{α }\left({\mathrm{c}}_{\mathrm{s}}\right) = 4\mathrm{s}\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }\;\mathrm{f}\mathrm{o}\mathrm{r}\;\mathrm{ }1\le \mathrm{s}\le \mathrm{n}-1$

$\mathtt{α }\left({\mathrm{c}}_{\mathrm{n}}\right) = \mathrm{ħ},$

$\alpha \left({x}_{i, j}\right) = \left\{\begin{array}{cc}j& for\;1\le j\le 3\\ j+1& for\;4\le j\le 6\\ .& .\\ .& .\\ j+n-1& for\;3n-2\le j\le 3n-1\end{array}, i = 1, \mathrm{ }2, \mathrm{ }3\right.\text{, }$

$\alpha \left({x}_{i, 3n}\right) = ħ-1 \; for\; i = 1, 2, 3$

$\alpha \left({c}_{0}{x}_{i, 1}\right) = i \; for\; i = i1, 2, 3$

$\alpha \left({c}_{S}{x}_{i, 3S}\right) = 4S+i \; for\; 1\le S\le n-1, i = 1, 2, 3$

$\alpha \left({c}_{S}{x}_{i, 3S+1}\right) = 4S+i+1 \; for\; 1\le S\le n-1, i = 1, 2, 3$

$\alpha \left({c}_{n}{x}_{i, 3n}\right) = \left\{\begin{array}{cc}ħ-2& for\; i = 1\\ ħ-1& for\; i = 2\\ ħ& for\; i = 3\end{array}\right.\text{, }$

$\alpha \left({{x}_{i, j}x}_{i, j+1i}\right) = \left\{\begin{array}{cc}j+i+1& for\; 1\le j\le 2\\ j+i+2& for\; 4\le j\le 5\\ .& .\\ .& .\\ j+i+n-\mathrm{I}1& for\; 3n-5\le j\le 3n-4\\ ħ+i-3& for\; 3n-2\le j\le 3n-1\end{array}, i = 1, 2, 3\right..$

It is clear that $ħ$ is the greatest used label. The weights of edges of ${\theta }_{n}\left(\mathrm{3, 3}\right)$ are given by:

${w}_{\alpha }\left({c}_{0}{x}_{i, 1}\right) = i+2 \; for\; i = 1, 2, 3\text{, }$

${w}_{\alpha }\left({c}_{S}{x}_{i, 3S}\right) = 12S+i-1 \; for\; 1\le S\le n-1 , i = 1, 2, 3$

${w}_{\alpha }\left({c}_{S}{x}_{i, 3S+1}\right) = 12S+i+2\; for\; 1\le S\le n-1, i = 1, 2, 3,$

${w}_{\alpha }\left({c}_{n}{x}_{i, 3n}\right) = \left\{\begin{array}{cc}3\left(ħ-1\right)& for\; i = 1\\ 3ħ-2& for\; i = 2\\ 3ħ-1& for\; i = 3\end{array}\right.\text{, }$

${w}_{\alpha }\left({{x}_{i, j}x}_{i, j+1}\right) = \left\{\begin{array}{cc}3j+i+2& for\; 1\le j\le 2\\ 3j+i+5& for\; 4\le j\le 5\\ .& .\\ .& .\\ 3j+i+3n-4& for\; 3n-5\le j\le 3n-4\\ 3ħ+i-10& for\; j = 3n-2\\ 3ħ+i-7& for\; j = 3n-1\end{array}, i = 1, 2, 3\right.$

Obviously, the weights of edges are distinct. So $\alpha$ is an edge irregular total $\mathrm{ħ}-$ labeling. Hence

$tes\left({\theta }_{n}\left(\mathrm{3, 3}\right)\right) = 4n+1\text{.}$

Theorem 2. For ${\theta }_{n}\left(3, m\right), m>3$ be a uniform theta snake graph. Then

$tes\left({\theta }_{n}(3, m)\right) = (m+1)n+1\text{.}$

Proof. Since $\left|E\left({\theta }_{n}\right(3, m\left)\right)\right| = 3\left(m+1\right)n$ and $\Delta \left({\theta }_{n}\right(3, m\left)\right) = 6$ . Substituting in (1), we find

$tes\left({\theta }_{n}(3, m)\right)\ge (m+1)n+1\text{.}$

The existence of an edge irregular total $ƛ-$ labeling for ${\theta }_{n}(3, m)$ , See , $m>3$ will be shown, with $ƛ = (m+1)n+1$ . Define a total $ƛ-$ labeling $\beta :V\left({\theta }_{n}(3, m)\right)\cup E\left({\theta }_{n}(3, m)\right)\to \left\{\mathrm{1, 2}, 3, \dots, ƛ\right\}$ for ${\theta }_{n}(3, m)$ as:

Figure 3. Uniform theta snake graph θ(3, m).

DownLoad: Full-Size Img PowerPoint

$\beta \left({c}_{0}\right) = 1,$

$\beta \left({c}_{s}\right) = (m+1)s \; for\; 1\le s\le n-1\text{, }$

$\beta \left({c}_{n}\right) = ƛ$

$\beta \left({x}_{i, j}\right) = \left\{\begin{array}{cc}j& for\; 1\le j\le m\\ j+1& for\; m+1\le j\le 2m\\ .& .\\ .& .\\ j+n-1& for\; m\left(n-1\right)+1\le j\le mn-1\end{array}\right.\text{, }$

$\beta \left({x}_{i, mn}\right) = ƛ-1 \; for\; i = 1, 2, 3$

$\beta \left({c}_{0}{x}_{i, 1}\right) = 1 \; for\; i = 1, 2, 3$

$\beta \left({c}_{S}{x}_{i, mS}\right) = (m+1)S+i \; for\; 1\le S\le n-1, i = 1, 2, 3$

$\beta \left({c}_{S}{x}_{i, mS+1}\right) = (m+1)S+i+1 \; for\; 1\le S\le n-1, i = 1, 2, 3$

$\beta \left({c}_{n}{x}_{i, mn}\right) = \left\{\begin{array}{cc}ƛ-2& for\; i = 1\\ ƛ-1& for\; i = 2\\ ƛ& for\; i = 3\end{array}\right.\text{, }$

$\beta \left({{x}_{i, j}x}_{i, j+1}\right) = \left\{\begin{array}{cc}j+i+1& for\; 1\le j\le m-1\\ j+i+2& for\; m+1\le j\le 2m-1\\ .& .\\ .& .\\ j+i+n& for\; m\left(n-1\right)+1\le j\le mn-2\\ j+i+n-1& for\; j = mn-1\end{array}.\right.$

Clearly, $ƛ$ is the most label of edges and vertices. The edges weights are given as follows:

${w}_{\beta }\left({c}_{0}{x}_{i, 1}\right) = i+2\; for\; i = 1, 2, 3\text{, }$

${w}_{\beta }\left({c}_{S}{x}_{i, mS}\right) = 3\left(m+1\right)S+i-1 \; for\; 1\le S\le n-1, i = 1, 2, 3$

${w}_{\beta }\left({c}_{S}{x}_{i, mS+1}\right) = 3\left(m+1\right)S+i+2 \; for\; 1\le S\le n-1, i = 1, 2, 3\text{, }$

${w}_{\beta }\left({c}_{n}{x}_{i, mn}\right) = \left\{\begin{array}{cc}3ƛ-3& for\; i = 1\\ 3ƛ-2& for\; i = 2\\ 3ƛ-1& for\; i = 3\end{array}\right.\text{, }$

${w}_{\beta }\left({{x}_{i, j}x}_{i, j+1}\right) = \left\{\begin{array}{cc}3j+i+2& for\; 1\le j\le m-1\\ 3j+i+5& for\; m+1\le j\le 2m-1\\ .& .\\ .& .\\ 3j\mathrm{I}+i+3n-1& for\; m\left(n-1\right)+1\le j\le mn-2\\ 3ƛ+i-7& for\; j = mn-1\end{array}, \right.$

It is obvious that the weights of edges are different, thus $\beta$ is an edge irregular total $ƛ-$ labeling of ${\theta }_{n}(3, m)$ . Hence

$tes\left({\theta }_{n}(3, m)\right) = (m+1)n+1\text{.}$

Theorem 3. Let ${\theta }_{n}\left(t, 3\right)$ be a theta snake graph for $t>3$ . Then

$tes\left({\theta }_{n}(t, 3)\right) = \left\lceil {\frac{4tn+2}{3}} \right\rceil \text{.}$

Proof. A size of the graph ${\theta }_{n}(t, 3)$ equals $4tn$ and $\Delta \left({\theta }_{n}\left(t, 3\right)\right) = 2t$ , then from (1) we have

$tes\left({\theta }_{n}(t, 3)\right)\ge \left\lceil {\frac{4tn+2}{3}} \right\rceil \text{.}$

We define an edge irregular total $ħ-$ labeling for ${\theta }_{n}(t, 3)$ to get upper bound. So, let $ħ = \left\lceil {\frac{4tn+2}{3}} \right\rceil$ and a total $ħ-$ labeling $\gamma :V\left({\theta }_{n}(t, 3)\right)\cup E\left({\theta }_{n}(t, 3)\right)\to \left\{\mathrm{1, 2}, 3, \dots, ħ\right\}$ is defined in the following three cases:

Case 1. $4tn+2\equiv 0\left(mod \; 3\right)$

$\gamma$ is defined as:

$\gamma \left({c}_{0}\right) = 1,$

$\gamma \left({c}_{S}\right) = \left(t+1\right)S\; for\; 1\le S\le n-1\text{, }$

$\gamma \left({c}_{n}\right) = ħ$

$\gamma \left({x}_{i, j}\right)\mathrm{I} = \left\{\begin{array}{cc}i& for\; 1\le j\le 3, i = 1, 2, \dots , t\\ i+t+1& for\; 4\le j\le 6, i = 1, 2, \dots , t\\ i+2(t+1)& for\; 7\le j\le 9, i = 1, 2, \dots , t\\ .& .\\ .& .\\ .& .\\ i+(n-1)(t+1)& for\; 3n-5\le j\le 3n-3, i = 1, 2, \dots , t\\ ħ-1& for\; 3n-2\le j\le 3n, i = 1\\ ħ& for\; 3n-2\le j\le 3n, i = 2, 3, \dots , t\end{array}\right.\text{, }$

$\gamma \left({c}_{0}{x}_{i, 1}\right) = 1 \; for\; i = 1, 2, \dots , t$

$\gamma \left({c}_{S}{x}_{i, 3S}\right) = 2St-2S+3 \; for\; 1\le S\le n-1, i = 1, 2, \dots , t$

$\gamma \left({c}_{n}{x}_{i, 3n}\right) = \left\{\begin{array}{cc}ħ-t+2& for\; i = 1\\ ħ-t+i& for\; i = 2, 3, \dots , t\end{array}\right.\text{, }$

$\gamma \left({c}_{S}{x}_{i, 3S+1}\right) = 2St-2S+2 \; for\; 1\le S\le n-1, i = 1, 2, \dots , t$

$\gamma \left({{c}_{n-1}x}_{i, 3n-2}\right) = \left\{\begin{array}{c}\left\{\begin{array}{cc}\left(t+2\right)n-t-5& for\; i = 1\\ \left(t+2\right)n-t+i-7& for\; i = 2, 3, \dots , t\end{array}, n = 2, 3\right.\\ \begin{array}{c}\left\{\begin{array}{cc}\left(t+1\right)n-t-1& for\; i = 1\\ \left(t+1\right)n-t+i-3& for\; i = 2, 3, \dots , t\end{array}, n\ne 2, 3\right.\end{array}\end{array}\right.$

$\gamma \left({{x}_{i, j}x}_{i, j+1}\right) = \left\{\begin{array}{c}\left\{\begin{array}{cc}t+j& for\; 1\le j\le 2\\ 3t+j-5& for\; 4\le j\le 5\\ 5t+j-10& for\; 7\le j\le 8\\ .& .\\ .& .\\ .& .\\ \left(2n-3\right)t+j-5\left(n-2\right)& for\; 3n-5\le j\le 3n-4\end{array}, i = 1, 2, \dots , t\right.\\ \begin{array}{cc}ħ-3\left(t+n\right)+j+5& for\; 3n-2\le j\le 3n-1, i = 1\\ ħ-3\left(t+n\right)+j+5+2(i-2)& for\; 3n-2\le j\le 3n-1, i = 2, 3, \dots , t\end{array}\end{array}\right.$

Obviously, $ħ$ is the greatest label. The edges weights of ${\theta }_{n}(t, 3)$ can be expressed as:

${w}_{\gamma }\left({c}_{0}{x}_{i, 1}\right) = i+2 \; for\; i = 1, 2, \dots , t$

${w}_{\gamma }\left({c}_{S}{x}_{i, 3S}\right) = t\left(4S-1\right)+i+2\; for\; 1\le S\le n-1, i = 1, 2, \dots , t$

${w}_{\gamma }\left({c}_{S}{x}_{i, 3S+1}\right) = 4St+i+2 \; for\; 1\le S\le n-1, i = 1, 2, \dots , t\text{, }$

${w}_{\gamma }\left({c}_{n-1}{x}_{i, 3n-2}\right) = \left\{\begin{array}{cc}2nt+3n-2t+ħ+i-8& for\; n = 2, 3\\ 2nt+2n-2t+ħ+i-4& for\; n\ne 2, 3\end{array}, i = 1, 2, \dots , t\right.$

${w}_{\gamma }\left({{x}_{i, j}x}_{i, j+1}\right) = \left\{\begin{array}{c}\left\{\begin{array}{cc}t+j+2i& for\; 1\le j\le 2\\ 5t+j+2i-4& for\; 4\le j\le 5\\ 9t+j+2i-6& for\; 7\le j\le 8\\ .& .\\ .& .\\ .& .\\ \left(4n-5\right)t+j+2i-3n+8& for\; 3ni-5\le j\le 3n-4\end{array}, i = 1, 2, \dots , t\right.\\ \begin{array}{cc}3ħ-3\left(t+in\right)+j+3& for\; 3n-2\le j\le 3n-1, i = 1\\ 3ħ-3\left(t+in\right)+j+2i+3& for\; 3n-2\le j\le 3n-1, i = 2, 3, \dots , t\end{array}\end{array}\right.$

It implies that the edges weights have distinct values. So $\gamma$ is the desired edge irregular total $ħ-$ labeling, $ħ = \left\lceil {\frac{4tn+2}{3}} \right\rceil$ . Hence

$tes\left({\theta }_{n}(t, 3)\right) = \left\lceil {\frac{4tn+2}{3}} \right\rceil \text{.}$

Case 2. $4tn+2\equiv 1\left(mod \; 3\right)$

$\mathrm{D}\mathrm{e}\mathrm{f}\mathrm{i}\mathrm{n}\mathrm{e}\;\gamma$ as:

$\gamma \left({c}_{0}\right) = 1,$

$\gamma \left({c}_{S}\right) = \left(t+1\right)S \; for\; 1\le S\le n-1\text{, }$

$\gamma \left({c}_{n}\right) = ħ$

$\gamma \left({x}_{i, j}\right) = \left\{\begin{array}{cc}i& for\; 1\le j\le 3, i = 1, 2, \dots , t\\ i+t+1& for\; 4\le j\le 6, i = 1, 2, \dots , t\\ i+2(t+1)& for\; 7\le j\le 9, i = 1, 2, \dots , t\\ .& .\\ .& .\\ .& .\\ i+(n+1)(t+1)& for\; 3n-5\le j\le 3n-3, i = 1, 2, \dots , t\\ ħ-1& for\; 3n-2\le j\le 3n, i = 1\\ ħ& for\; 3n-2\le j\le 3n, i = 2, 3, \dots , t\end{array}\right.\text{, }$

$\gamma \left({c}_{0}{x}_{i, 1}\right) = 1\; for\; i = 1, 2, \dots , t$

$\gamma \left({c}_{S}{x}_{i, 3S}\right) = 2St-2S+3 \; for\; 1\le S\le n-1, i = 1, 2, \dots,\rm{t}$

$\gamma \left({c}_{n}{x}_{i, 3n}\right) = \left\{\begin{array}{cc}ħ-t& for\; i = 1\\ ħ-t+i-2& for\; i = 2, 3, \dots , t\end{array}\right.\text{, }$

$\gamma \left({c}_{S}{x}_{i, 3S+1}\right) = 2St-2S+2 \; for\; 1\le \mathrm{I}S\le n-1, i = 1, 2, \dots t$

$\gamma \left({{c}_{n-1}x}_{i3n-2}\right) = \left\{\begin{array}{c}\left\{\begin{array}{cc}\left(t+2\right)n-t-5& for\; i = 1\\ \left(t+2\right)n-t+i-7& for\; i = 2, 3, \dots , t\end{array}, n = 2, 3\right.\\ \begin{array}{c}\left\{\begin{array}{cc}\left(t+1\right)n-t-1& for\; i = 1\\ \left(\mathrm{I}t+\mathrm{I}1\right)n-t+i-3& for\; i = 2, 3, \dots , t\end{array}, n\ne 2, 3\right.\end{array}\end{array}\right.$

$\gamma \left({{x}_{i, j}x}_{i, j+1}\right) = \left\{\begin{array}{c}\left\{\begin{array}{cc}t+j& for\; 1\le j\le 2\\ 3t+j-5& for\; 4\le j\le 5\\ 5t+j-10& for\; 7\le j\le 8\\ .& .\\ .& .\\ .& .\\ \left(2n-3\right)t+j-5(n-2)& for\; 3n-5\le j\le 3n-4\end{array}, i = 1, 2, \dots , t\right.\\ \begin{array}{cc}ħ-3\left(t+n\right)+j+3& for\; 3n-2\le j\le 3n-1, i = 1\\ ħ-3\left(t+n\right)+j+2(i-2)& for\; 3n-2\le j\le 3n-1, i = 2, 3, \dots , t\end{array}\end{array}\right.$

It is clear that the greatest label is $ħ$ . We define the weights of edges of ${\theta }_{n}(t, 3)$ as:

${w}_{\gamma }\left({c}_{0}{x}_{i, 1}\right) = i+2\; for\; i = 1, 2, \dots , t$

${w}_{\gamma }\left({c}_{S}{x}_{i, 3S}\right) = t\left(4S-1\right)+i+2 \; for\; 1\le S\le n-1, i = 1, 2, \dots , t{w}_{\gamma }\left({c}_{n}{x}_{i, 3n}\right) = 3ħ-t+i-2 \; for\; 1\le S\le n-1, i = 1, 2, \dots , t$

${w}_{\gamma }\left({c}_{S}{x}_{i, 3S+1}\right) = 4St+i+2\; for\; 1\le S\le n-1, i = 1, 2, \dots , t\text{, }$

${w}_{\gamma }\left({c}_{n-1}{x}_{i, 3n-2}\right) = \left\{\begin{array}{cc}2nt+3n-2t+ħ+i-8& for\; n = 2, 3\\ 2nt+2n-2t+ħ+i-4& for\; n\ne 2, 3\end{array}, i = 1, 2, \dots , t\right.$

${w}_{\gamma }\left({{x}_{i, j}x}_{i, j+1}\right) = \left\{\begin{array}{c}\left\{\begin{array}{cc}t+j+2i& for\; 1\le j\le 2\\ 5t+j+2i-4& for\; 4\le j\le 5\\ 9t+j+2i-6& for\; 7\le j\le 8\\ .& .\\ .& .\\ .& .\\ \left(4n-5\right)t+j+2i-3n+8& for\; 3n-5\le j\le 3n-4\end{array}, i = 1, 2, \dots , t\right.\\ \begin{array}{cc}3ħ-3\left(t+n\right)+j+1& for\; 3n-2\le j\le 3n-1, i = 1\\ 3ħ-3\left(t+n\right)+j+2(i-2)& for\; 3n-2\le j\le 3n-1, i = 2, 3, \dots , t\end{array}\end{array}\right.$

It is obvious that the edges weights are different. Then

$tes\left({\theta }_{n}(t, 3)\right) = \left\lceil {\frac{4tn+2}{3}} \right\rceil \text{.}$

Case 3. $4tn+2\equiv 2\left(mod \; 3\right)$

$\gamma$ is defined as follows:

$\gamma \left({c}_{0}\right) = 1,$

$\gamma \left({c}_{S}\right) = \left(t+1\right)S \; for\; 1\le S\le n-1\text{, }$

$\gamma \left({c}_{n}\right) = ħ$

$\gamma \left({x}_{i, j}\right) = \left\{\begin{array}{cc}i& for\; 1\le j\le 3, i = 1, 2, \dots , t\\ i+t+1& for\; 4\le j\le 6, i = 1, 2, \dots , t\\ i+2(t+1)& for\; 7\le j\le 9, i = 1, 2, \dots , t\\ .& .\\ .& .\\ .& .\\ i+\left(n-1\right)\left(t+1\right)& for\; 3n-5\le j\le 3n-3, i = 1, 2, \dots , t\\ ħ-1& for\; 3n-2\le j\le 3n, i = 1\\ ħ& for\; 3n-2\le j\le 3n, i = 2, 3, \dots , t\end{array}, \right.$

$\gamma \left({c}_{0}{x}_{i, 1}\right) = 1\; for\; i = 1, 2, \dots , t$

$\gamma \left({c}_{S}{x}_{i, 3S}\right) = 2St-2S+3 \; for\; 1\le S\le n-1, i = 1, 2, \dots , t$

$\gamma \left({c}_{n}{x}_{i, 3n}\right) = \left\{\begin{array}{cc}ħ-t+1& for\; i = 1\\ ħ-t+i-1& for\; i = 2, 3, \dots , t\end{array}\right.\text{, }$

$\gamma \left({c}_{S}{x}_{i, 3S+1}\right) = 2St-2S+2 \; for\; 1\le S\le n-2, i = 1, 2, \dots , t$

$\gamma \left({{x}_{i, j}x}_{i, j+1}\right) = \left\{\begin{array}{c}\left\{\begin{array}{cc}t+j& for\; 1\le j\le 2\\ 3t+j-5& for\; 4\le j\le 5\\ 5t+j-10& for\; 7\le j\le 8\\ .& .\\ .& .\\ .& .\\ \left(2n-3\right)t+j-5(n-2)& for\; 3n-5\le j\le 3n-4\end{array}, i = 1, 2, \dots t\right.\\ \begin{array}{cc}ħ-3\left(t+i\right)+j+4& for\; 3n-2\le j\le 3ni-1, i = 1\\ ħ-3\left(t+n\right)+j+2i& for\; 3n-2\le j\le 3n-1, i = 2, 3, \dots , t\end{array}\end{array}\right.$

We can see that $ħ$ is the greatest label. For edges weights of ${\theta }_{n}(t, 3)$ , we have

${w}_{\gamma }\left({c}_{0}{x}_{i, 1}\right) = i+2 \; for\; i = 1, 2, \dots , t$

${w}_{\gamma }\left({c}_{0}{x}_{i, 3S}\right) = t\left(4S-1\right)+i+2 \; for\; 1\le S\le n-1, i = 1, 2, \dots , t{w}_{\gamma }\left({c}_{n}{x}_{i, 3n}\right) = 3ħ-t+i-1 \; for\; 1\le S\le n-1, i = 1, 2, \dots , t$

${w}_{\gamma }\left({c}_{S}{x}_{i, 3S+1}\right) = 4St+i+2 \; for\; 1\le S\le n-1, i = 1, 2, \dots , t\text{, }$

${w}_{\gamma }\left({c}_{n}{x}_{i, 3n-2}\right) = \left\{\begin{array}{cc}2nt-3n-2t+ħ+i-8& for\; n = 2, 3\\ 2nt+2n-2t+ħ+i-4& for\; n\ne 2, 3\end{array}, i = 1, 2, \dots , t\right.$

${w}_{\gamma }\left({{x}_{i, j}x}_{i, j+1}\right) = \left\{\begin{array}{c}\left\{\begin{array}{cc}t+j+2i& for\; 1\le j\le 2\\ 5t+j+2i-4& for\; 4\le j\le 5\\ 9t+j+2i-6& for\; 7\le j\le 8\\ .& .\\ .& .\\ .& .\\ \left(4n-5\right)t+j+2i-3n+8& for\; 3n-5\le j\le 3n-4\end{array}, i = 1, 2, \dots , t\right.\\ \begin{array}{cc}3ħ-3\left(t+n\right)+j+2& for\; 3n-2\le j\le 3n-1, i = 1\\ 3ħ-3\left(t+n\right)+j+2i& for\; 3n-2\le j\le 3n-1, i = 2, 3, \dots , t\end{array}\end{array}\right.$

It clears that the edges weights are i distinct. So $\gamma$ is the desired edge irregular total $ħ-$ labeling, $ħ = \left\lceil {\frac{4tn+2}{3}} \right\rceil$ . Hence

$tes\left({\theta }_{n}(t, 3)\right) = \left\lceil {\frac{4tn+2}{3}} \right\rceil \text{.}$

Theorem 4. For ${\theta }_{n}\left(4, m\right)$ be a theta snake graph for $t>3$ . Then

$tes\left({\theta }_{n}(4, m)\right) = \left\lceil {\frac{4(m+1)n+2}{3}} \right\rceil \text{.}$

Proof. Since $\left|E\left({\theta }_{n}\left(4, m\right)\right)\right| = 4(m+1)n$ and $\Delta \left({\theta }_{n}\left(4, m\right)\right) = 8$ , then from (1) we have

$tes\left({\theta }_{n}(4, m)\right)\ge \left\lceil {\frac{4(m+1)n+2}{3}} \right\rceil \text{.}$

The existence of an edge irregular total $ƛ-$ labeling for ${\theta }_{n}(4, m)$ , $m>3$ will be shown, with $ƛ = \left\lceil {\frac{4(m+1)n+2}{3}} \right\rceil$ . Define a total $ƛ-$ labeling $\beta :V\left({\theta }_{n}(4, m)\right)\cup E\left({\theta }_{n}(4, m)\right)\to \left\{\mathrm{1, 2}, 3, \dots, ƛ\right\}$ for ${\theta }_{n}(4, m)$ in the following three cases as:

Case 1. $4\left(m+1\right)n+2\equiv 0\left(mod \; 3\right)$ , $i = 1, 2, \mathrm{3, 4}$

$\beta$ is defined as:

$\beta \left({c}_{s}\right) = \left\{\begin{array}{c}\begin{array}{c}1 \; for\; s = 0\\ \left(m+1\right)s \; for\; 1\le s\le \left\lceil {\frac{n}{2}} \right\rceil \end{array}\\ ƛ+s-n \; for\;\left\lceil {\frac{n}{2}} \right\rceil \le s\le n\end{array}\right.\text{, }$

$\beta \left({x}_{i, j}\right) = \left\{\begin{array}{cc}j& for\; 1\le j\le m\\ j+1& for\; m+1\le j\le 2m\\ .& .\\ .& .\\ \begin{array}{c}j+\left\lceil {\frac{n}{2}} \right\rceil -1\\ ƛ-j+22\\ ƛ\end{array}& \begin{array}{c}for\; m\left(\left\lceil {\frac{n}{2}} \right\rceil -1\right)+1\le j\le m\left\lceil {\frac{n}{2}} \right\rceil +1\\ for\; m\left\lceil {\frac{n}{2}} \right\rceil +2\le j\le m\left(n-1\right)\\ for\; m\left(n-1\right)+1\le j\le mn-1\end{array}\end{array}, \right.$

$\beta \left({c}_{0}{x}_{i, 1}\right) = 1 \; for\; i = 1, 2, \mathrm{3, 4}$

$\beta \left({c}_{S}{x}_{i, mS}\right) = \left\{\begin{array}{c}{2c}_{S}+i-1\; for\; 1\le S\le \left\lceil {\frac{n}{2}} \right\rceil -1\\ \begin{array}{c}{c}_{S}+i-4\left(m+1\right)for\;\left\lceil {\frac{n}{2}} \right\rceil \le s\le n-1\\ ƛ-4+i \; for\; s = n\end{array}\end{array}\right., i = 1, 2, \mathrm{3, 4}$

$\beta \left({c}_{S}{x}_{i, mS+1}\right) = \left\{\begin{array}{c}{2c}_{S}+i+1 \; for\; 1\le S\le \left\lceil {\frac{n}{2}} \right\rceil , i = 1, 2, \mathrm{3, 4}\\ {c}_{S}+i-4\left(m+1\right)+2 \; for\;\left\lceil {\frac{n}{2}} \right\rceil +1\le s\le n-1\end{array}\right.$

$\beta \left({c}_{n}{x}_{i, mn}\right) = \left\{\begin{array}{cc}ƛ-3& for\; i = 1\\ ƛ-2& for\; i = 2\\ \begin{array}{c}ƛ-1\\ ƛ\end{array}& \begin{array}{c}for\; i = 3\\ for\; i = 4\end{array}\end{array}\right.\text{, }$

$\beta \left({{x}_{i, j}x}_{i, j+1}\right) = \left\{\begin{array}{cc}j+i+1& for\; 1\le j\le m-1\\ j+i+2& for\; m+1\le j\le 2m-1\\ .& .\\ .& .\\ j+i+\left\lceil {\frac{n}{2}} \right\rceil & for\; j = m\left(\left\lceil {\frac{n}{2}} \right\rceil -1\right)+1\\ 2j+i-2[nm\left(\left\lceil {\frac{n}{2}} \right\rceil -1\right)+1]& for\; m\left(\left\lceil {\frac{n}{2}} \right\rceil -1\right)+2\le j\le mn-1\end{array}.\right.$

It is clear that $ƛ$ is the greatest used label. The weights of edges of ${\theta }_{n}(4, m)$ are given by:

${w}_{\beta }\left({c}_{0}{x}_{i, 1}\right) = i+2 \; for\; i = 1, 2, \mathrm{3, 4}\text{, }$

${w}_{\beta }\left({c}_{S}{x}_{i, mS}\right) = \left\{\begin{array}{c}{2ms+s+2c}_{S}+i-1 \; for\; 1\le S\le \left\lceil {\frac{n}{2}} \right\rceil -1, \\ \begin{array}{c}{c}_{S}+i+ƛ+\left(s-4\right)\left(m+1\right)-n+\left\lceil {\frac{n}{2}} \right\rceil -1\; for\;\left\lceil {\frac{n}{2}} \right\rceil \le s\le n-1\\ 3ƛ-4+i+s-n\; for\; s = n\end{array}\end{array}\right., i = 1, \mathrm{2, 3}, 4$

${w}_{\beta }\left({c}_{S}{x}_{i, mS+1}\right) = \left\{\begin{array}{c}\left(2m+1\right)s+{2c}_{S}+i+1 \; for\; 1\le S\le \left\lceil {\frac{n}{2}} \right\rceil, \\ 2ƛ+s-n+{c}_{S}+i-4\left(m+1\right)+2 \;for\; \left\lceil {\frac{n}{2}} \right\rceil \le s\le n-1\end{array}\right.i = 1, 2, \mathrm{3, 4},$

${w}_{\beta }\left({c}_{n}{x}_{i, mn}\right) = \left\{\begin{array}{cc}3ƛ+s-n-3& for\; i = 1\\ 3ƛ+s-n-2& for\; i = 2\\ \begin{array}{c}3ƛ+s-n-1\\ 3ƛ+s-n\end{array}& \begin{array}{c}for\; i = 3\\ for\; i = 4\end{array}\end{array}\right.\text{, }$

${w}_{\beta }\left({{x}_{i, j}x}_{i, j+1}\right) = \left\{\begin{array}{cc}3j+i+2& for\; 1\le j\le m-1\\ 3j+i+4& for\; m+1\le j\le 2m-1\\ .& .\\ .& .\\ 3j+i+3\left\lceil {\frac{n}{2}} \right\rceil -1& for\; j = m\left(\left\lceil {\frac{n}{2}} \right\rceil -1\right)+1\\ \begin{array}{c}4j+2ƛ+45+i-2[nm\left(\left\lceil {\frac{n}{2}} \right\rceil -1\right)+1]\\ 2j+2ƛ+i-2[nm\left(\left\lceil {\frac{n}{2}} \right\rceil -1\right)+1]\end{array}& \begin{array}{c}for\; m\left\lceil {\frac{n}{2}} \right\rceil +2\le j\le m\left(n-1\right)\\ for\; m\left(n-1\right)+1\le j\le mn-1\end{array}\end{array}, \right.$

It is obvious that the weights of edges are different, thus $\beta$ is an edge irregular total $ƛ-$ labeling of ${\theta }_{n}(4, m)$ . Hence

$tes\left({\theta }_{n}(4, m)\right) = \left\lceil {\frac{4(m+1)n+2}{3}} \right\rceil \text{.}$

Case 2. $4\left(m+1\right)n+2\equiv 1\left(mod \; 3\right)$ , $i = 1, 2, \mathrm{3, 4}$

$\beta$ is defined as:

$\beta \left({c}_{s}\right) = \left\{\begin{array}{c}\begin{array}{c}1\; for\; s = 0\\ \left(m+1\right)s \; for\; 1\le s\le \left\lceil {\frac{n}{2}} \right\rceil \end{array}\\ ƛ+s-n \; for\;\left\lceil {\frac{n}{2}} \right\rceil \le s\le n\end{array}\right.\text{, }$

$\beta \left({x}_{i, j}\right) = \left\{\begin{array}{cc}j& for\; 1\le j\le m\\ j+1& for\; m+1\le j\le 2m\\ .& .\\ .& .\\ \begin{array}{c}j+\left\lceil {\frac{n}{2}} \right\rceil -1\\ ƛ-j+22\\ ƛ\end{array}& \begin{array}{c}for\; m\left(\left\lceil {\frac{n}{2}} \right\rceil -1\right)+1\le j\le m\left\lceil {\frac{n}{2}} \right\rceil \\ for\; m\left\lceil {\frac{n}{2}} \right\rceil +1\le j\le m\left(n-1\right)\\ for\; m\left(n-1\right)+1\le j\le mn-1\end{array}\end{array}, \right.$

$\beta \left({c}_{0}{x}_{i, 1}\right) = 1 \; for\; i = 1, 2, \mathrm{3, 4}$

$\beta \left({c}_{S}{x}_{i, mS}\right) = \left\{\begin{array}{c}{2c}_{S}+i-1 \; for\; 1\le S\le \left\lceil {\frac{n}{2}} \right\rceil -1, \\ \begin{array}{c}\begin{array}{c}ƛ-7+i \; for\; s = \left\lceil {\frac{n}{2}} \right\rceil \\ {c}_{S}+i-4m-2 \; for\;\left\lceil {\frac{n}{2}} \right\rceil +1\le s\le n-1\end{array}\\ ƛ-6+i \; for\; s = n\end{array}\end{array}\right., i = 1, 2, \mathrm{3, 4}$

$\beta \left({c}_{S}{x}_{i, mS+1}\right) = \left\{\begin{array}{c}{2c}_{S}+i+1 \; for\; 1\le S\le \left\lceil {\frac{n}{2}} \right\rceil \\ {c}_{S}+i-4m \; for\;\left\lceil {\frac{n}{2}} \right\rceil \le s\le n-1\end{array}, i = 1, 2, \mathrm{3, 4}\right.$

$\beta \left({c}_{n}{x}_{i, mn}\right) = \left\{\begin{array}{cc}ƛ-5& for\; i = 1\\ ƛ-4& for\; i = 2\\ \begin{array}{c}ƛ-3\\ ƛ-2\end{array}& \begin{array}{c}for\; i = 3\\ for\; i = 4\end{array}\end{array}\right.\text{, }$

It is clear that $ƛ$ is the greatest used label. The weights of edges of ${\theta }_{n}(4, m)$ are given by:

${w}_{\beta }\left({c}_{0}{x}_{i, 1}\right) = i+2\; for\; i = 1, 2, \mathrm{3, 4}\text{, }$

${w}_{\beta }\left({c}_{S}{x}_{i, mS}\right) = \left\{\begin{array}{c}\begin{array}{c}{2ms+s+2c}_{S}+i-1 \; for\; 1\le S\le \left\lceil {\frac{n}{2}} \right\rceil -1, \\ 2ƛ-m\left\lceil {\frac{n}{2}} \right\rceil +\left(m+1\right)s+i+15 \; for\; s = \left\lceil {\frac{n}{2}} \right\rceil \end{array}\\ \begin{array}{c}{c}_{S}+i+ƛ+(s-4)\left(m+1\right)-n+\left\lceil {\frac{n}{2}} \right\rceil -1 \; for\;\left\lceil {\frac{n}{2}} \right\rceil \le s\le n-1\\ 3ƛ-4+i+s-n \; for\; s = n\end{array}\end{array}\right.$

${w}_{\beta }\left({c}_{S}{x}_{i, mS+1}\right) = \left\{\begin{array}{c}\left(2m+1\right)s+{2c}_{S}+i+1\; for\; 1\le S\le \left\lceil {\frac{n}{2}} \right\rceil , i = 1, 2, \mathrm{3, 4}\\ 2ƛ+s-n+{c}_{S}+i-4m\; for\;\left\lceil {\frac{n}{2}} \right\rceil \le s\le n-1\end{array}\right.i = 1, 2, \mathrm{3, 4},$

${w}_{\beta }\left({c}_{n}{x}_{i, mn}\right) = \left\{\begin{array}{cc}3ƛ+s-n-5& for\; i = 1\\ 3ƛ+s-n-4& for\; i = 2\\ \begin{array}{c}3ƛ+s-n-3\\ 3ƛ+s-n-2\end{array}& \begin{array}{c}for\; i = 3\\ for\; i = 4\end{array}\end{array}\right.\text{, }$

It is obvious that the weights of edges are different, thus $\beta$ is an edge irregular total $ƛ-$ labeling of ${\theta }_{n}(4, m)$ . Hence

$tes\left({\theta }_{n}(4, m)\right) = \left\lceil {\frac{4(m+1)n+2}{3}} \right\rceil \text{.}$

Case 3. $4\left(m+1\right)n+2\equiv 2\left(mod \; 3\right)$ , $i = 1, 2, \mathrm{3, 4}$

$\beta$ is defined as:

$\beta \left({x}_{i, j}\right) = \left\{\begin{array}{cc}j& for\; 1\le j\le m\\ j+1& for\; m+1\le j\le 2m\\ .& .\\ .& .\\ \begin{array}{c}j+\left\lceil {\frac{n}{2}} \right\rceil -1\\ ƛ-j+22\\ ƛ\end{array}& \begin{array}{c}for\; m\left(\left\lceil {\frac{n}{2}} \right\rceil -2\right)+1\le j\le m\left(\left\lceil {\frac{n}{2}} \right\rceil -1\right)\\ for\; m\left(\left\lceil {\frac{n}{2}} \right\rceil -1\right)+1\le j\le m\left(n-1\right)\\ for\; m\left(n-1\right)+1\le j\le mn-1\end{array}\end{array}, \right.$

$\beta \left({c}_{0}{x}_{i, 1}\right) = 1 \; for\; i = 1, 2, \mathrm{3, 4}$

$\beta \left({c}_{S}{x}_{i, mS}\right) = \left\{\begin{array}{c}{2c}_{S}+i-1 \; for\; 1\le S\le \left\lceil {\frac{n}{2}} \right\rceil -1, i = 1, 2, \mathrm{3, 4}\\ \begin{array}{c}\begin{array}{c}ƛ-7+i \; for\; s = \left\lceil {\frac{n}{2}} \right\rceil \\ {c}_{S}+i-4m-2 \; for\;\left\lceil {\frac{n}{2}} \right\rceil +1\le s\le n-1\end{array}\\ ƛ-5+i \; for\; s = n\end{array}\end{array}\right.$

$\beta \left({c}_{S}{x}_{i, mS+1}\right) = \left\{\begin{array}{c}{2c}_{S}+i+1 \; for\; 1\le S\le \left\lceil {\frac{n}{2}} \right\rceil -1, i = 1, 2, \mathrm{3, 4}\\ \begin{array}{c}{c}_{S}+1+i \; for\; s = \left\lceil {\frac{n}{2}} \right\rceil \\ {c}_{S}+i-4m+1 \; for\;\left\lceil {\frac{n}{2}} \right\rceil +1\le s\le n-1\end{array}\end{array}\right.$

$\beta \left({c}_{n}{x}_{i, mn}\right) = \left\{\begin{array}{cc}ƛ-4& for\; i = 1\\ ƛ-3& for\; i = 2\\ \begin{array}{c}ƛ-2\\ ƛ-1\end{array}& \begin{array}{c}for\; i = 3\\ for\; i = 4\end{array}\end{array}\right.\text{, }$

$\beta \left({{x}_{i, j}x}_{i, j+1}\right) = \left\{\begin{array}{cc}j+i+1& for\; 1\le j\le m-1\\ j+i+2& for\; m+1\le j\le 2m-1\\ .& .\\ .& .\\ j+i+\left\lceil {\frac{n}{2}} \right\rceil & for\; j = m\left(\left\lceil {\frac{n}{2}} \right\rceil -1\right)+1\\ 2j+i-2\left[nm\left(\left\lceil {\frac{n}{2}} \right\rceil -1\right)+1\right]+1& for\; m\left(\left\lceil {\frac{n}{2}} \right\rceil -1\right)+2\le j\le mn-1\end{array}.\right.$

It is clear that $ƛ$ is the greatest used label. The weights of edges of ${\theta }_{n}(4, m)$ are given by:

${w}_{\beta }\left({c}_{0}{x}_{i, 1}\right) = i+2 \; for\; i = 1, 2, \mathrm{3, 4}\text{, }$

${w}_{\beta }\left({c}_{S}{x}_{i, mS}\right) = \left\{\begin{array}{c}\begin{array}{c}{2ms+s+2c}_{S}+i-1 \; for\; 1\le S\le \left\lceil {\frac{n}{2}} \right\rceil -1, \\ 2ƛ-m\left\lceil {\frac{n}{2}} \right\rceil +\left(m+1\right)s+i+15 \; for\; s = \left\lceil {\frac{n}{2}} \right\rceil \end{array}\\ \begin{array}{c}{c}_{S}+i+ƛ+(s-4)\left(m+1\right)-n+\left\lceil {\frac{n}{2}} \right\rceil -1 \; for\;\left\lceil {\frac{n}{2}} \right\rceil \le s\le n-1\\ 3ƛ-3+i+s-n \; for\; s = n\end{array}\end{array}\right.$

${w}_{\beta }\left({c}_{S}{x}_{i, mS+1}\right) = \left\{\begin{array}{c}\left(2m+1\right)s+{2c}_{S}+i+1 \; for\; 1\le S\le \left\lceil {\frac{n}{2}} \right\rceil, i = 1, 2, \mathrm{3, 4}\\ \begin{array}{c}\\ 2ƛ+s-n+{c}_{S}+i-4m+1\; for\; \left\lceil {\frac{n}{2}} \right\rceil \le s\le n-1\end{array}\end{array}\right.,$

It is obvious that the weights of edges are different, thus $\beta$ is an edge irregular total $ƛ-$ labeling of ${\theta }_{n}(4, m)$ . Hence

$tes\left({\theta }_{n}(4, m)\right) = \left\lceil {\frac{4(m+1)n+2}{3}} \right\rceil$

Theorem 5. If ${\theta }_{n}\left(t, 4\right)$ is theta snake graph for $t>3$ . Then

$tes\left({\theta }_{n}(t, 4)\right) = \left\lceil {\frac{5tn+2}{3}} \right\rceil \text{.}$

Proof. Since $\left|E\left({\theta }_{n}\right(t, 4\left)\right)\right| = 5tn$ and $\Delta \left({\theta }_{n}\right(t, 4\left)\right) = 2t$ . Substituting in (1), we have

$tes\left({\theta }_{n}(t, 4)\right)\ge \left\lceil {\frac{5tn+2}{3}} \right\rceil \text{.}$

We define an edge irregular total $ħ-$ labeling for ${\theta }_{n}(t, 4)$ to get upper bound. Let $ħ = \left\lceil {\frac{5tn+2}{3}} \right\rceil$ and a total $ħ-$ labeling $\gamma :V\left({\theta }_{n}(t, 4)\right)\cup E\left({\theta }_{n}(t, 4)\right)\to \left\{\mathrm{1, 2}, 3, \dots, ħ\right\}$ is defined in the following three cases:

Case 1. $5tn+2\equiv 0\left(mod \; 3\right)$

$\mathrm{D}\mathrm{e}\mathrm{f}\mathrm{i}\mathrm{n}\mathrm{e}\; \gamma$ as:

$\gamma \left({c}_{0}\right) = 1,$

$\gamma \left({c}_{S}\right) = \left(t+1\right)S \; for\; 1\le S\le n-1\text{, }$

$\gamma \left({c}_{n}\right) = ħ$

$\gamma \left({x}_{i, j}\right) = \left\{\begin{array}{cc}i& for\; 1\le j\le 4, i = 1, 2, \dots , t\\ i+t+1& for\; 5\le j\le 8, i = 1, 2, \dots , t\\ i+2(t+1)& for\; 9\le j\le 12, i = 1, 2, \dots , t\\ .& .\\ .& .\\ .& .\\ i+(n-1)(t+1)& for\; 4n-7\le j\le 4n-4, i = 1, 2, \dots , t\\ ħ-1& for\; 4n-3\le j\le 4n, i = 1\\ ħ& for\; 4n-3\le j\le 4n, i = 2, 3, \dots , t\end{array}\right.\text{, }$

$\gamma \left({c}_{0}{x}_{i, 1}\right) = 1 \; for\; i = 1, 2, \dots , t$

$\gamma \left({c}_{S}{x}_{i, 4S}\right) = 3St-2S+3 \; for\; 1\le S\le n-1, i = 1, 2, \dots , t$

$\gamma \left({c}_{n}{x}_{i, 4n}\right) = \left\{\begin{array}{cc}ħ-t+2& for\; i = 1\\ ħ-t+i& for\; i = 2, 3, \dots , t\end{array}\right.\text{, }$

$\gamma \left({c}_{S}{x}_{i, 4S+1}\right) = 3St-2S+2 \; for\; 1\le S\le n-1, i = 1, 2, \dots , t$

$\gamma \left({{c}_{n-1}x}_{i, 4n-3}\right) = \left\{\begin{array}{c}\left\{\begin{array}{cc}\left(t+2\right)n-t-5& for\; i = 1\\ \left(t+2\right)n-t+i-7& for\; i = 2, 3, \dots , t\end{array}, n = 2, 3\right.\\ \left(t+1\right)n-t+i-3 \; for\; i = 2, 3, \dots , t , n\ne 2, 3\end{array}\right.$

$\gamma \left({{x}_{i, j}x}_{i, j+1}\right) = \left\{\begin{array}{c}\left\{\begin{array}{cc}t+j& for\; 1\le j\le 2\\ 3t+j-5& for\; 4\le j\le 5\\ 5t+j-10& for\; 7\le j\le 8\\ .& .\\ .& .\\ .& .\\ \left(2n-3\right)t+j-5\left(n-2\right)& for\; 4n-5\le j\le 4n-4\end{array}, i = 1, 2, \dots , t\right.\\ \begin{array}{cc}ħ-3\left(t+n\right)+j+5& for\; 4n-2\le j\le 4n, i = 1\\ ħ-3\left(t+n\right)+j+5+2(i-2)& for\; 4n-2\le j\le 4n, i = 2, 3, \dots , t\end{array}\end{array}\right.$

It is clear that, $ħ$ is the greatest label. The edges weights of ${\theta }_{n}(t, 4)$ can be expressed as:

${w}_{\gamma }\left({c}_{0}{x}_{i, 1}\right) = i+2 \; for\; i = 1, 2, \dots , t$

${w}_{\gamma }\left({c}_{S}{x}_{i, 4S}\right) = t\left(5S-1\right)+i+2 \; for\; 1\le S\le n-1, i = 1, 2, \dots , t{w}_{\gamma }\left({c}_{n}{x}_{i, 4n}\right) = 3ħ-t+i \; for\; i = 1, 2, \dots , t$

${w}_{\gamma }\left({c}_{S}{x}_{i, 4S+1}\right) = 5St+i+2 \; for\; 1\le S\le n-1, i = 1, 2, \dots , t\text{, }$

${w}_{\gamma }\left({c}_{n-1}{x}_{i, 4n-2}\right) = \left\{\begin{array}{cc}2nt+3n-2t+ħ+i-8& for\; n = 2, 3\\ 2nt+2n-2t+ħ+i-6& for\; n\ne 2, 3\end{array}, i = 1, 2, \dots , t\right.$

${w}_{\gamma }\left({{x}_{i, j}x}_{i, j+1}\right) = \left\{\begin{array}{c}\left\{\begin{array}{cc}t+j+2i& for\; 1\le j\le 2\\ 5t+j+2i-4& for\; 4\le j\le 5\\ 9t+j+2i-6& for\; 7\le j\le 8\\ .& .\\ .& .\\ .& .\\ \left(4n-5\right)t+j+2i-3n+8& for\; 4n-5\le j\le 4n-4\end{array}, i = 1, 2, \dots , t\right.\\ \begin{array}{cc}3ħ-3\left(t+n\right)+j+3& for\; 4n-2\le j\le 4n-1, i = 1\\ 3ħ-3\left(t+n\right)+j+2i+3& for\; 4n-2\le j\le 4n-1, i = 2, 3, \dots , t\end{array}\end{array}\right.$

It implies that the edges weights have distinct values. So $\gamma$ is the desired edge irregular total $ħ-$ labeling, $ħ = \left\lceil {\frac{5tn+2}{3}} \right\rceil$ . Hence

$tes\left({\theta }_{n}(t, 4)\right) = \left\lceil {\frac{5tn+2}{3}} \right\rceil \text{.}$

Case 2. $5tn+2\equiv 1\left(mod \; 3\right)$

$\mathrm{D}\mathrm{e}\mathrm{f}\mathrm{i}\mathrm{n}\mathrm{e}\; \gamma$ as:

$\gamma \left({c}_{0}\right) = 1,$

$\gamma \left({c}_{S}\right) = \left(t+1\right)S \; for\; 1\le S\le n-1\text{, }$

$\gamma \left({c}_{n}\right) = ħ$

$\gamma \left({x}_{i, j}\right) = \left\{\begin{array}{cc}i& for\; 1\le j\le 4, i = 1, 2, \dots , t\\ i+t+1& for\; 5\le j\le 8, i = 1, 2, \dots , t\\ i+2(t+1)& for\; 9\le j\le 12, i = 1, 2, \dots , t\\ .& .\\ .& .\\ .& .\\ i+(n+1)(t+1)& for\; 4n-7\le j\le 4n-4, i = 1, 2, \dots , t\\ ħ-1& for\; 4n-3\le j\le 4n, i = 1\\ ħ& for\; 4n-3\le j\le 4n, i = 2, 3, \dots , t\end{array}\right.\text{, }$

$\gamma \left({c}_{0}{x}_{i, 1}\right) = 1 \; for\; i = 1, 2, \dots , t$

$\gamma \left({c}_{S}{x}_{i, 4S}\right) = 3St-2S+3 \; for\; 1\le S\le n-1, i = 1, 2, \dots, \rm{t}$

$\gamma \left({c}_{n}{x}_{i, 4n}\right) = \left\{\begin{array}{cc}ħ-t& for\; i = 1\\ ħ-t+i-2& for\; i = 2, 3, \dots , t\end{array}\right.\text{, }$

$\gamma \left({c}_{S}{x}_{i, 4S+1}\right) = 3St-2S+2$

$for\; 1\le S\le n-1, i = 1, 2, \dots t$

$\gamma \left({{c}_{n-1}x}_{i, 4n-3}\right) = \left\{\begin{array}{c}\left\{\begin{array}{cc}\left(t+2\right)n-t-5& for\; i = 1\\ \left(t+2\right)n-t+i-7& for\; i = 2, 3, \dots , t\end{array}, n = 2, 3\right.\\ \begin{array}{c}\left\{\begin{array}{cc}\left(t+1\right)n-t-1& for\; i = 1\\ \left(t+1\right)n-t+i-3& for\; i = 2, 3, \dots , t\end{array}, n\ne 2, 3\right.\end{array}\end{array}\right.$

$\gamma \left({{x}_{i, j}x}_{i, j+1}\right) = \left\{\begin{array}{c}\left\{\begin{array}{cc}t+j& for\; 1\le j\le 3\\ 3t+j-5& for\; 5\le j\le 7\\ 5t+j-10& for\; 9\le j\le 11\\ .& .\\ .& .\\ .& .\\ \left(2n-3\right)t+j-5(n-2)& for\; 4n-7\le j\le 4n-5\end{array}, i = 1, 2, \dots , t\right.\\ \begin{array}{cc}ħ-4\left(t+n\right)+j+3& for\; 4n-3\le j\le 4n-1, i = 1\\ ħ-4\left(t+n\right)+j+2(i-2)& for\; 4n-3\le j\le 4n-1, i = 2, 3, \dots , t\end{array}\end{array}\right.$

It is clear that the i greatest label is $ħ$ . We define the weights of edges of ${\theta }_{n}(t, 4)$ as:

${w}_{\gamma }\left({c}_{0}{x}_{i, 1}\right) = i+2\; for\; i = 1, 2, \dots , t$

${w}_{\gamma }\left({c}_{S}{x}_{i, 4S}\right) = t\left(5S-1\right)+i+2\; for\; 1\le S\le n-1, i = 1, 2, \dots , t{w}_{\gamma }\left({c}_{n}{x}_{i, 4n}\right) = 3ħ-t+i-2 \; for\; 1\le S\le n-1, i = 1, 2, \dots , t$

${w}_{\gamma }\left({c}_{S}{x}_{i, 4S+1}\right) = 5St+i+2 \; for\; 1\le S\le n-1, i = 1, 2, \dots , t\text{, }$

${w}_{\gamma }\left({c}_{n-1}{x}_{i, 4n-3}\right) = \left\{\begin{array}{cc}3nt+3n-2t+ħ+i-8& for\; n = 2, 3\\ 3nt+2n-2t+ħ+i-6& for\; n\ne 2, 3\end{array}, i = 1, 2, \dots , t\right.$

${w}_{\gamma }\left({{x}_{i, j}x}_{i, j+1}\right) = \left\{\begin{array}{c}\left\{\begin{array}{cc}t+j+2i& for\; 1\le j\le 3\\ 5t+j+2i-4& for\;i 5\le j\le 7\\ 9t+j+2i-6& for\; 9\le j\le 11\\ .& .\\ .& .\\ .& .\\ \left(4n-5\right)t+j+2i-3n+8& for\; 4n-7\le j\le 4n-5\end{array}, i = 1, 2, \dots , t\right.\\ \begin{array}{cc}3ħ-4\left(t+n\right)+j+1& for\; 4n-3\le j\le 4n-1, i = 1\\ 3ħ-4\left(t+n\right)+j+2(i-2)& for\; 4n-3\le j\le 4n-1, i = 2, 3, \dots , t\end{array}\end{array}\right.$

It is obvious that the edges weights are different. Then

$tes\left({\theta }_{n}(t, 4)\right) = \left\lceil {\frac{5tn+2}{3}} \right\rceil \text{.}$

Case 3. $5tn+2\equiv 2\left(mod \; 3\right)$

$\mathrm{D}\mathrm{e}\mathrm{f}\mathrm{i}\mathrm{n}\mathrm{e} \; \gamma$ as:

$\gamma \left({c}_{0}\right) = 1,$

$\gamma \left({c}_{S}\right) = \left(t+1\right)S \; for\; 1\le S\le n-1\text{, }$

$\gamma \left({c}_{n}\right) = ħ$

$\gamma \left({x}_{i, j}\right) = \left\{\begin{array}{cc}i& for\; 1\le j\le 4, i = 1, 2, \dots , t\\ i+t+1& for\; 5\le j\le 8, i = 1, 2, \dots , t\\ i+2(t+1)& for\; 9\le j\le 12, i = 1, 2, \dots , t\\ .& .\\ .& .\\ .& .\\ i+(in-1)(t+1)& for\; 4n-7\le j\le 4n-4, i = 1, 2, \dots , t\\ ħ-1& for\; 4n-3\le j\le 4n, i = 1\\ ħ& for\; 4n-3\le j\le 4n, i = 2, 3, \dots , t\end{array}, \right.$

$\gamma \left({c}_{0}{x}_{i, 1}\right) = 1 \; for\; i = 1, 2, \dots , t$

$\gamma \left({c}_{S}{x}_{i, 4S}\right) = 3St-2S+3 \; for\; 1\le S\le n-1, i = 1, 2, \dots , t$

$\gamma \left({c}_{n}{x}_{i, 4n}\right) = \left\{\begin{array}{cc}ħ-t+1& for\; i = 1\\ ħ-t+i-1& for\; i = 2, 3, \dots , t\end{array}\right.\text{, }$

$\gamma \left({c}_{S}{x}_{i, 4S+1}\right) = 3St-2S+2 \; for\; 1\le S\le n-2, i = 1, 2, \dots , t$

$\gamma \left({{c}_{n-1}x}_{i, 4n-3}\right) = \left\{\begin{array}{c}\left\{\begin{array}{cc}\left(t+2\right)n-t-5& for\; i = 1\\ \left(t+2\right)n-t+i-7& for\; i = 2, 3, \dots , t\end{array}, n = 2, 3\right.\\ \begin{array}{c}\left\{\begin{array}{cc}\left(t+1\right)n-t-1& for\; i = 1\\ \left(t+1\right)n-t+i-3& for\; i = 2, 3, \dots , t\end{array}, n\ne 2, 3\right.\end{array}\end{array}\right.$

$\gamma \left({{x}_{i, j}x}_{i, j+1}\right) = \left\{\begin{array}{c}\left\{\begin{array}{cc}t+j& for\; 1\le j\le 3\\ 3t+j-5& for\; 5\le j\le 7\\ 5t+j-10& for\; 9\le j\le 11\\ .& .\\ .& .\\ .& .\\ \left(2n-3\right)t+j-5(n-2)& for\; 4n-7\le j\le 4n-5\end{array}, i = 1, 2, \dots t\right.\\ \begin{array}{cc}ħ-4\left(t+n\right)+j+4& for\; 4n-3\le j\le 4n-1, i = 1\\ ħ-4\left(t+n\right)+j+2i& for\; 4n-3\le j\le 4n-1, i = 2, 3, \dots , t\end{array}\end{array}\right.$

We can see that $ħ$ is the greatest label. For edges weights of ${\theta }_{n}(t, 4)$ , we have:

${w}_{\gamma }\left({c}_{0}{x}_{i, 1}\right) = i+2\; for\; i = 1, 2, \dots , t$

${w}_{\gamma }\left({c}_{0}{x}_{i, 4S}\right) = t\left(5S-1\right)+i+2 \; for\; 1\le S\le n-1, i = 1, 2, \dots , t{w}_{\gamma }\left({c}_{n}{x}_{i, 4n}\right) = 3ħ-t+i-1 \; for\; 1\le S\le in-1, i = 1, 2, \dots , t$

${w}_{\gamma }\left({c}_{S}{x}_{i, 4S+1}\right) = 5St+i+2 \; for\; 1\le S\le n-1, i = 1, 2, \dots , t\text{, }$

${w}_{\gamma }\left({c}_{n}{x}_{i, 4n-3}\right) = \left\{\begin{array}{cc}2nt-3n-2t+ħ+i-8& for\; n = 2, 3\\ 2nt+2n-2t+ħ+i-6& for\; n\ne 2, 3\end{array}, i = 1, 2, \dots , t\right.$

${w}_{\gamma }\left({{x}_{i, j}x}_{i, j+1}\right) = \left\{\begin{array}{c}\left\{\begin{array}{cc}t+j+2i& for\; 1\le j\le 3\\ 5t+j+2i-4& for\; 5\le j\le 7\\ 9t+j+2i-6& for\; 9\le j\le 11\\ .& .\\ .& .\\ .& .\\ \left(4n-5\right)t+j+2i-3n+8& for\; 4n-7\le j\le 4n-5\end{array}, i = 1, 2, \dots , t\right.\\ \begin{array}{cc}3ħ-4\left(t+n\right)+j+2& for\; 4n-3\le j\le 3n-1, i = 1\\ 3ħ-4\left(t+n\right)+j+2i& for\; 4n-3\le j\le 4n-1, i = 2, 3, \dots , t\end{array}\end{array}\right.$

It is obvious that the edges weights are distinct. So $\gamma$ is the desired edge irregular total $ħ-$ labeling, $ħ = \; \left\lceil {\frac{5tn+2}{3}} \right\rceil$ . Hence

$tes\left({\theta }_{n}(t, 4)\right) = \left\lceil {\frac{5tn+2}{3}} \right\rceil \text{.}$

The previous results lead us to introduce the following conjecture for a general case of a uniform theta snake graph ${\theta }_{n}(t, m)$ .

Conjecture. For uniform theta snake graph ${\theta }_{n}(t, m)$ , $n\ge 2, t\ge 3, and m\ge 3$ we have

$tes\left({\theta }_{n}(t, m)\right) = \left\lceil {\frac{(m+1)tn+2}{3}} \right\rceil \text{.}$

3. Conclusions

In the current paper, we have defined a new type of a family of graph called uniform theta snake graph, ${\theta }_{n}(t, m)$ . Also, the exact i value of TEISs for ${\mathtt{θ }}_{\mathrm{n}}\left(\mathrm{3, 3}\right)$ , ${\mathtt{θ }}_{\mathrm{n}}(3, \mathrm{m})$ and ${\mathtt{θ }}_{\mathrm{n}}(\mathrm{t}, 3)$ has been determined. Finally, we have generalized for t, m and found TEIS of a uniform theta snake graph ${\mathtt{θ }}_{\mathrm{n}}(\mathrm{t}, \mathrm{m})$ for $\mathrm{m}\ge 3$ , $\mathrm{t}\ge 3$ .

$tes\left({\theta }_{n}\left(\mathrm{3, 3}\right)\right) = 4n+1\text{.}$

$tes\left({\theta }_{n}(3, im)\right) = (im+1)in+1\text{.}$

$tes\left({\theta }_{n}(t, 3)\right) = \left\lceil {\frac{4tn+2}{3}} \right\rceil$

$tes\left({\theta }_{n}(t, m)\right) = \left\lceil {\frac{(m+1)tn+2}{3}} \right\rceil \text{.}$

Conflict of interest

All authors declare no conflict of interest in this paper.

Acknowledgment

We are so grateful to the reviewer for his many valuable suggestions and comments that significantly improved the paper.

References

[1]	M. Onana, B. Mewoli, J. J. Tewa, Hopf bifurcation analysis in a delayed Leslie-Gower predator-prey model incorporating additional food for predators, refuge and threshold harvesting of preys, Nonlinear Dyn., 100 (2020), 3007–3028. https://doi.org/10.1007/s11071-020-05659-7 doi: 10.1007/s11071-020-05659-7
[2]	E. González-Olivares, R. Ramos-Jiliberto, Dynamic consequences of prey refuges in a simple model system: more prey, fewer predators and enhanced stability, Ecol. Model., 166 (2003), 135–146. https://doi.org/10.1016/S0304-3800(03)00131-5 doi: 10.1016/S0304-3800(03)00131-5
[3]	L. Chen, F. Chen, L. Chen, Qualitative analysis of a predator-prey model with Holling type Ⅱ functional response incorporating a constant prey refuge, Nonlinear Anal.: Real World Appl., 11 (2010), 246–252. https://doi.org/10.1016/j.nonrwa.2008.10.056 doi: 10.1016/j.nonrwa.2008.10.056
[4]	V. Křivan, Effects of optimal antipredator behavior of prey on predator-prey dynamics: the role of refuges, Theor. Popul. Biol., 53 (1998), 131–142. https://doi.org/10.1006/tpbi.1998.1351 doi: 10.1006/tpbi.1998.1351
[5]	P. H. Leslie, Some further notes on the use of matrices in population mathematics, Biometrika, 35 (1948), 213–245. https://doi.org/10.2307/2332342 doi: 10.2307/2332342
[6]	P. H. Leslie, A stochastic model for studying the properties of certain biological systems by numerical methods, Biometrika, 45 (1958), 16–31. https://doi.org/10.2307/2333042 doi: 10.2307/2333042
[7]	F. Chen, L. Chen, X. Xie, On a Leslie-Gower predator-prey model incorporating a prey refuge, Nonlinear Anal.: Real World Appl., 10 (2009), 2905–2908. https://doi.org/10.1016/j.nonrwa.2008.09.009 doi: 10.1016/j.nonrwa.2008.09.009
[8]	J. D. Murray, Mathematical biology Ⅱ: spatial models and biomedical applications, New York: Springer, 2001.
[9]	R. P. Agarwal, P. J. Wong, Advanced topics in difference equations, Springer Science and Business Media, 2013.
[10]	C. Celik, O. Duman, Allee effect in a discrete-time predator-prey system, Chaos Solitons Fract., 40 (2009), 1956–1962. https://doi.org/10.1016/j.chaos.2007.09.077 doi: 10.1016/j.chaos.2007.09.077
[11]	W. Ko, K. Ryu, Qualitative analysis of a predator-prey model with Holling type Ⅱ functional response incorporating a prey refuge, J. Differ. Equ., 231 (2006), 534–550. https://doi.org/10.1016/j.jde.2006.08.001 doi: 10.1016/j.jde.2006.08.001
[12]	T. K. Kar, Stability analysis of a prey-predator model incorporating a prey refuge, Commun. Nonlinear Sci. Numer. Simul., 10 (2005), 681–691. https://doi.org/10.1016/j.cnsns.2003.08.006 doi: 10.1016/j.cnsns.2003.08.006
[13]	Y. Huang, F. Chen, L. Zhong, Stability analysis of a prey-predator model with Holling type Ⅲ response function incorporating a prey refuge, Appl. Math. Comput., 182 (2006), 672–683. https://doi.org/10.1016/j.amc.2006.04.030 doi: 10.1016/j.amc.2006.04.030
[14]	S. M. S. Rana, Chaotic dynamics and control of discrete ratio-dependent predator-prey system, Discrete Dyn. Nat. Soc., 2017 (2017), 1–13. https://doi.org/10.1155/2017/4537450 doi: 10.1155/2017/4537450
[15]	K. S. Al-Basyouni, A. Q. Khan, Discrete-time predator-prey model with bifurcations and chaos, Math. Probl. Eng., 2020 (2020), 1–14. https://doi.org/10.1155/2020/8845926 doi: 10.1155/2020/8845926
[16]	O. Mehrjooee, S. Fathollahi Dehkordi, M. Habibnejad Korayem, Dynamic modeling and extended bifurcation analysis of flexible-link manipulator, Mech. Based Des. Struct. Mach., 48 (2020), 87–110. https://doi.org/10.1080/15397734.2019.1665542 doi: 10.1080/15397734.2019.1665542
[17]	P. Chakraborty, U. Ghosh, S. Sarkar, Stability and bifurcation analysis of a discrete prey-predator model with square-root functional response and optimal harvesting, J. Biol. Syst., 28 (2020), 91–110. https://doi.org/10.1142/S0218339020500047 doi: 10.1142/S0218339020500047
[18]	W. Liu, D. Cai, Bifurcation, chaos analysis and control in a discrete-time predator-prey system, Adv. Differ. Equ., 2019 (2019), 1–22. https://doi.org/10.1186/s13662-019-1950-6 doi: 10.1186/s13662-019-1950-6
[19]	J. R. Beddington, C. A. Free, J. H. Lawton, Dynamic complexity in predator-prey models framed in difference equations, Nature, 255 (1975), 58–60. https://doi.org/10.1038/255058a0 doi: 10.1038/255058a0
[20]	F. Chen, Permanence and global attractivity of a discrete multispecies Lotka-Volterra competition predator-prey systems, Appl. Math. Comput., 182 (2006), 3–12. https://doi.org/10.1016/j.amc.2006.03.026 doi: 10.1016/j.amc.2006.03.026
[21]	Q. Fang, X. Li, M. Cao, Dynamics of a discrete predator-prey system with Beddington-DeAngelis function response, Appl. Math., 3 (2012), 389–394. https://doi.org/10.4236/am.2012.34060 doi: 10.4236/am.2012.34060
[22]	H. N. Agiza, E. M. Elabbasy, H. El-Metwally, A. A. Elsadany, Chaotic dynamics of a discrete prey-predator model with Holling type Ⅱ, Nonlinear Anal.: Real World Appl., 10 (2009), 116–129. https://doi.org/10.1016/j.nonrwa.2007.08.029 doi: 10.1016/j.nonrwa.2007.08.029
[23]	K. Zhuang, Z. Wen, Dynamical behaviors in a discrete predator-prey model with a prey refuge, Int. J. Math. Comput. Sci., 5 (2011), 1149–1151.
[24]	E. A. Grove, G. Ladas, Periodicities in nonlinear difference equations, Chapman and Hall/CRC, 2004. https://doi.org/10.1201/9781420037722
[25]	A. Wikan, Discrete dynamical systems with an introduction to discrete optimization problems, London, UK, 2013.
[26]	M. R. S. Kulenović, G. Ladas, Dynamics of second order rational difference equations: with open problems and conjectures, Chapman and Hall/CRC, 2001.
[27]	E. Camouzis, G. Ladas, Dynamics of third-order rational difference equations with open problems and conjectures, CRC Press, 2007.
[28]	J. Guckenheimer, P. Holmes, Nonlinear oscillations, dynamical systems and bifurcation of vector fields, New York: Springer, 1983. https://doi.org/10.1007/978-1-4612-1140-2
[29]	Y. A. Kuznetsov, Elements of applied bifurcation theorey, New York: Springer, 2004. https://doi.org/10.1007/978-1-4757-3978-7
[30]	W. B. Zhang, Discrete dynamical systems, bifurcations and chaos in economics, Elsevier, 2006.
[31]	C. Lei, X. Han, W. Wang, Bifurcation analysis and chaos control of a discrete-time prey-predator model with fear factor, Math. Biosci. Eng., 19 (2022), 6659–6679. https://doi.org/10.3934/mbe.2022313 doi: 10.3934/mbe.2022313
[32]	X. Han, C. Lei, Bifurcation and turing instability analysis for a space-and time-discrete predator-prey system with smith growth function, Chaos Solitons Fract., 166 (2023), 112910. https://doi.org/10.1016/j.chaos.2022.112910 doi: 10.1016/j.chaos.2022.112910
[33]	S. N. Elaydi, An introduction to difference equations, New York: Springer, 1996. https://doi.org/10.1007/978-1-4757-9168-6
[34]	S. Lynch, Dynamical systems with applications using Mathematica, Boston: Birkhäuser, 2007.
[35]	D. Auerbach, C. Grebogi, E. Ott, J. A. Yorke, Controlling chaos in high dimensional systems, Phys. Rev. Lett., 69 (1992), 3479. https://doi.org/10.1103/PhysRevLett.69.3479 doi: 10.1103/PhysRevLett.69.3479
[36]	F. J. Romeiras, C. Grebogi, E. Ott, W. P. Dayawansa, Controlling chaotic dynamical systems, Phys. D: Nonlinear Phenom., 58 (1992), 165–192. https://doi.org/10.1016/0167-2789(92)90107-X doi: 10.1016/0167-2789(92)90107-X
[37]	X. S. Luo, G. Chen, B. H. Wang, J. Q. Fang, Hybrid control of period-doubling bifurcation and chaos in discrete nonlinear dynamical systems, Chaos Solitons Fract., 18 (2003), 775–783. https://doi.org/10.1016/S0960-0779(03)00028-6 doi: 10.1016/S0960-0779(03)00028-6

Reader Comments

Your name:*

Email:*
© 2023 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)