1.
Introduction
In recent years, fractional calculus (FC) has been successfully applied to describe the mathematical issues in real materials [1,2]. For instance, [3] provided the representation of the constitutive relationship (RCR) of the fractional mechanical element with fractional derivative (FD) of the Riemann-Liouville type, and [4] presented its generalised form. In [5], the RCR with FC of the Liouville-Caputo type was taken into consideration. It has been demonstrated that the local fractional derivative is a wonderful mathematical tool for addressing fractal issues and some challenging natural phenomena [6,7]. The Caputo-Fabrizio type's RCR through fractional derivative was introduced in [8]. The study of fractional differential equations has expanded owing to the Caputo-Fabrizio fractional derivative. The nonsingular kernel of the new derivative [9], is what makes it so beautiful. The Caputo-Fabrizio derivative has the same additional motivating characteristics of heterogeneity and configuration [10,11] with different scales as it does in the Caputo and Riemann-Liouville fractional derivatives despite being created through the convolution of an ordinary derivative and an exponential function. In the past two years, numerous studies related to the the new Caputo-Fabrizio fractional derivative have been published. It has been shown that modelling using the fractional derivative of the Atangana-Baleanu has a brief random walk. Additionally, it has been found that the Mittag-Leffler function is a more significant and practical filter tool than the power and exponential law functions, making the Atangana-Baleanu fractional derivative in the sense of Caputo an effective mathematical tool for simulating more difficult real-world problems [12,13].
Using partial differential equations, it is possible to express a specific relationship between its partial derivatives (PDEs) and an unknown function. PDEs may be found in almost every field of engineering and research. In recent years, the application of PDEs in fields such as finance, biology, image graphics and processing, and social sciences has risen. As a result, when certain independent variables interact with one another in each of the above-mentioned fields, appropriate functions in these variables may be established, allowing for the modelling of a variety of processes via the use of equations for the related functions [14,15,16]. The study of PDEs has various elements. The traditional method, which dominated the nineteenth century, was to develop procedures for identifying explicit answers [17,18,19]. Theoretical PDE analysis offers a wide range of applications. It's worth noting that there are certain really difficult equations that even supercomputers can't solve. All one can do in these situations is attempt to get qualitative data. Moreover, the formulation of the equation, as well as its associated side conditions, is significant. A model of a physical or technical issue is frequently used to generate the equation. It is not immediately clear that the model is continuous in the view that it results to a solved PDE [20,21,22]. Furthermore, in most circumstances, it is preferable that the answer be one of a kind and stable under minor data disruptions. Theoretical comprehension of the equation aids in determining whether or not these requirements are satisfied. Several methods for solving classical PDEs have already been suggested, and several solutions have been discovered [23,24,25,26,27,28,29].
The propagation of shallow water is represented by numerous well-known integral models, such as the Boussinesq equation, KdV equation, Whitham-Broer-Kaup equation, and others. Whitham, Broer, and Kaup [30,31,32,33,34] created non-linear Whitham-Broer-Kaup equations employing the Boussinesq approximation
where ψ=ψ(υ,τ),φ=φ(υ,τ) represents the velocity of horizontal and fluid height, which fluctuates substantially from equilibriums, and q,p are constants made up of many diffusion power. Wang and Zheng [32] implemented riccati sub-equation method to obtain the result of fractional order Whitham-Broer-Kaup equations. The analytical and numerical methods have been applied to find the solutions of Whitham-Broer-Kaup equations, such as reduced differential transform method, residual power series method (1.1), finite element method [35], power-series method[36], the finite difference approach [37], the exponential-function technique [38], the variation iteration technique, the homotopy perturbation technique, the homotopy analysis technique and others [39,40,41].
Adomian introduced the Adomian decomposition approach in 1980, which is an effective approach for locating numeric and explicit solutions to a wide variety of differential equations that describe physical conditions. This method is applicable to initial value problems, boundary value problems, partial and ordinary differential equations, including linear and nonlinear equations, and stochastic systems. The Natural transform decomposition method is constructed by combining the Adomian decomposition method with the Natural transform method (NTDM). NTDM has also been used to analyze fractional-order non-linear partial differential equations numerically in a number of articles [42,43,44].
In this research, we use NTDM in combination with two different derivatives to investigate the general as well as numerical solution of the coupled system of Whitham-Broer-Kaup equations of fractional order, as inspired by the above papers. NTDM is a simple and effective technique that does not require any perturbation. We compare our suggested method's results to those of other well-known approaches like the variational iteration method, Adomain decomposition method, and Optimal homoptoy analysis method. We can observe that the proposed method is superior to the previously discussed methods for finding nonlinear fractional order partial differential equation solutions. We use Maple to perform the calculations. The suggested techniques' convergence is also ensured by extending the concept mentioned in [45,46].
2.
Basic definitions
Fractional derivatives and integrals have a great number of properties and definitions. We propose changes to some basic fractional calculus definitions and preliminaries used in this study.
Definition 2.1. The Riemann-Liouville integral of order fraction for a function j∈C℘,℘≥−1, is given as [47,48,49]
Definition 2.2. The derivative with order fraction for j(η) in Caputo manner is stated as [47,48,49]
for m−1<ϱ≤m, m∈N, η>0,j∈Cm℘,℘≥−1.
Definition 2.3. The derivative with order fraction for j(η) in CF sense is stated as [47]
having 0<ϱ<1 and F(ϱ) is a normalization function having F(0)=F(1)=1.
Definition 2.4. The derivative with order fraction for j(η) in ABC sense is stated as [47]
having 0<ϱ<1, B(ϱ) is normalization function and Eϱ(z)=∑∞m=0zmΓ(mϱ+1) is the Mittag-Leffler function.
Definition 2.5. For a function ψ(τ), the natural transform (NT) is stated as
and for τ∈(0,∞), the NT of ψ(τ) is stated as
where H(τ) is the Heaviside function.
Definition 2.6. For the function ψ(κ,ℓ), the inverse NT is stated as
Lemma 2.7. If the NT of ψ1(τ) and ψ2(τ) are U1(κ,ℓ) and U2(κ,ℓ) respectively, so
having c1 and c2 constants.
Lemma 2.8. If ψ1(τ) and ψ2(τ) are the inverse NT of ψ1(κ,ℓ) and ψ2(κ,ℓ) respectively, so
having c1 and c2 constants.
Definition 2.9. The NT of Dϱτψ(τ) in Caputo sense is stated as [47]
Definition 2.10. The NT of Dϱτψ(τ) in CF sense is stated as [47]
Definition 2.11. The NT of Dϱτψ(τ) in ABC sense is stated as [47]
with M[ϱ] denoting a normalization function.
3.
General implementation of method
Consider the fractional partial differential equation
with the initial condition
which L is linear, N non-linear functions and sources function h(υ,τ).
3.1. Case I (NTDMCF)
The fractional CF derivative and natural transformation, Eq (3.1) can be stated as,
with
On employing natural inverse transformation, we get
Thus, for ψ(υ,τ), the series type solution is given as
and N(ψ(υ,τ)) can be decomposed as
the Adomian polynomials Ai is given as
Putting Eqs (3.7) and (3.6) into (3.5), we obtain
From (3.8), we get,
Thus, we obtain the result of (3.1) by putting (3.9) into (3.6) applying NTDMCF,
3.2. Case II (NTDMABC)
The fractional ABC derivative and natural transformation, Eq (3.1) is given as,
with
On employing natural inverse transformation, we get
The Adomain decomposition, we obtain as
From (3.8), we get,
Thus, we obtain the result of (3.1), by applying NTDMABC
4.
Convergence analysis
In this part, we give the uniqueness and convergence of the NTDMABC and NTDMCF.
Theorem 4.1. Let |L(ψ)−L(ψ∗)|<γ1|ψ−ψ∗| and |N(ψ)−N(ψ∗)|<γ2|ψ−ψ∗|, where ψ:=ψ(℘,τ) and ψ∗:=ψ∗(℘,τ) are values of two different functions and γ1, γ2 are Lipschitz constants.
L and N are the operators stated in (3.1). Then for NTDMCF the solution of (3.1) is unique when 0<(γ1+γ2)(1−ϱ+ϱτ)<1 for all τ.
Proof. Let H=(C[J],||.||) having norm ||ϕ(τ)||=maxτ∈J|ϕ(τ)| is the Banach space, ∀ continuous function over the interval J=[0,T]. Let I:H→H a non-linear mapping, with
So, I is contraction as 0<(γ1+γ2)(1−ϱ+ϱτ)<1. The solution of (3.1) is unique as according to Banach fixed point theorem.
Theorem 4.2. As according to the above theorem, the solution of (3.1) is unique for NTDMABC when 0<(γ1+γ2)(1−ϱ+ϱτϱΓ(ϱ+1))<1 for all τ.
Proof. As from above theorem let H=(C[J],||.||) be the Banach space, ∀ continuous function over the interval J. Let I:H→H is non-linear mapping, with
So, I is contraction as 0<(γ1+γ2)(1−ϱ+ϱτϱΓ(ϱ+1))<1. The solution of (3.1) is unique as according to Banach fixed point theorem.
Theorem 4.3. Let L and N are a Lipschitz functions as stated in the above theorems, then the NTDMCF solution of (3.1) is convergent.
Proof. Suppose H be the Banach space determined above and let ψm=∑mr=0ψr(℘,τ). To prove that ψm is a Cauchy sequence in H. Let,
Let m=n+1, then
where γ=(γ1+γ2)(1−ϱ+ϱτ). Thus, we get
As 0<γ<1, we have 1−γm−n<1. Thus,
Since ||ψ1||<∞, ||ψm−ψn||→0 when n→∞. As a result, ψm is a Cauchy sequence in H, stated that the series ψm is convergent.
Theorem 4.4. Let L and N are a Lipschitz functions as stated in the above theorems, then the NTDMABC solution of (3.1) is convergent.
Proof. Suppose ψm=∑mr=0ψr(℘,τ). To prove that ψm is a Cauchy sequence in H. Let,
Suppose m=n+1, thus
with γ=(γ1+γ2)(1−ϱ+ϱτϱΓ(ϱ+1)). Thus, we get
As 0<γ<1, we have 1−γm−n<1. Thus,
Since ||ψ1||<∞, ||ψm−ψn||→0 when n→∞. As a result, ψm is a Cauchy sequence in H, stated that the series ψm is convergent.
5.
Applications
Example 1. Consider the fractional-order system of Whitham-Broer-Kaup equations is given as
the initial condition
On employing natural transform, we have
Thus, we have
On simplification we have
Using the inverse natural transform, we get
5.1. NTDMCF solution
The series form solution for the unknown term ψ(υ,τ) and φ(υ,τ) is given as
The non-linear functions define by Adomian polynomials are given as ψψυ=∑∞m=0Am, ψφυ=∑∞m=0Bm and φψυ=∑∞m=0Cm, thus by means of these functions Eq (5.6) can be calculated as
Both side comparison of Eq (5.8), we achieve
Thus, for ψl and φl with (l≥3) the remaining terms are simply calculate. So, the result in series type is as
5.2. NDMABC solution
The series form solution for the unknown term ψ(υ,τ) and φ(υ,τ) is given as
The non-linear functions by mean of Adomian polynomials is given as ψψυ=∑∞l=0Al and ψ2ψυ=∑∞l=0Bl, thus by mean of these functions Eq (5.6) can be calculated as
Both side comparison of Eq (5.13), we get
Thus, for ψl with (l≥3) the remaining component are simply calculated. So, the series form solution is given as
The exact result is
Example 2. Consider the fractional-order system of Whitham-Broer-Kaup equations is given as
with the initial condition
On employing natural transform, we have
Thus, we have
On simplification we have
Using the inverse natural transform, we have
5.3. NDMCF solution
The series form solution for the unknown term ψ(υ,τ) and φ(υ,τ) is given as
The non-linear functions by Adomian polynomials is given as ψψυ=∑∞m=0Am, ψφυ=∑∞m=0Bm and φψυ=∑∞m=0Cm, thus by mean of these functions Eq (5.23) can be calculated as
Both sides comparisons of Eq (5.25), we achieve
Thus, for ψl and φl with (l≥3) the remaining terms are simply calculate. So, the series form solution is as
5.4. NDMABC solution
The series form solution for the unknown term ψ(υ,τ) and φ(υ,τ) is given as
The non-linear function by mean of Adomian polynomials are define as ψψυ=∑∞l=0Al and ψ2ψυ=∑∞l=0Bl, thus by mean of these function Eq (5.23) can be calculated as
Both side comparison of Eq (5.30), we achieve
Thus, for ψl and φl with (l≥3), the remaining terms are simply calculated. So, the series form solution is as
We achieve the series type result at integer-order ϱ=1,κ=0.1, λ=0.005, θ=10, as
The exact result of Eq (5.18) at ϱ=1, and taking κ=0.1, λ=0.005, θ=10,
6.
Numerical results and discussion
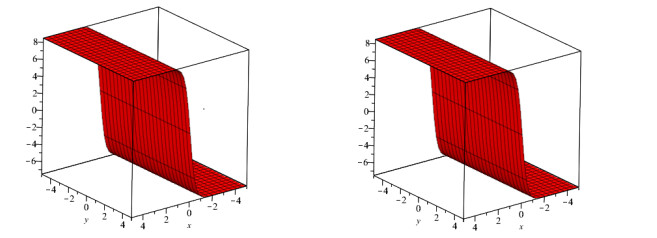
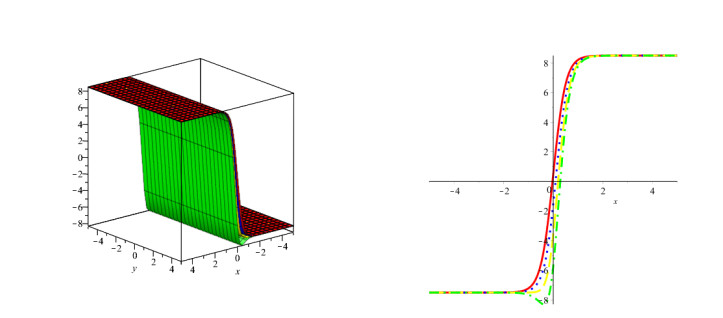
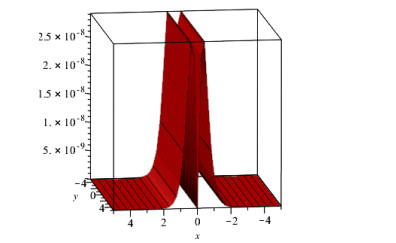
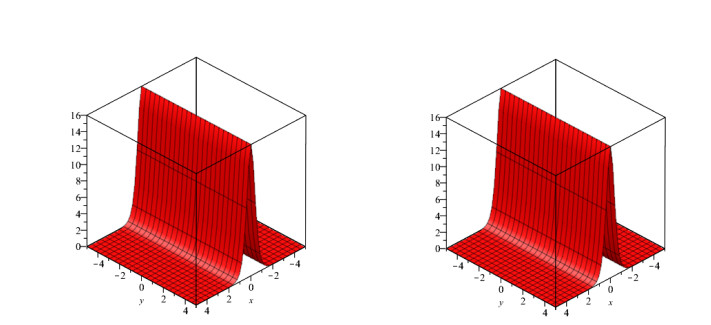
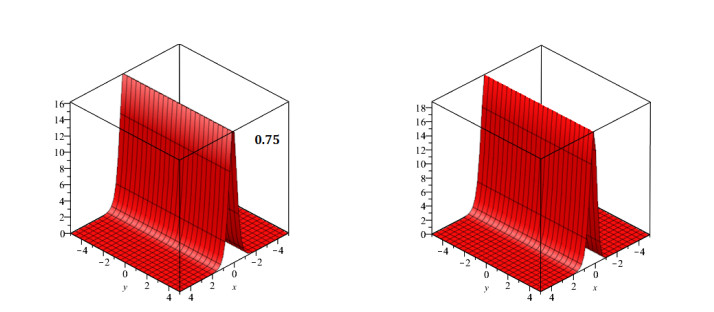
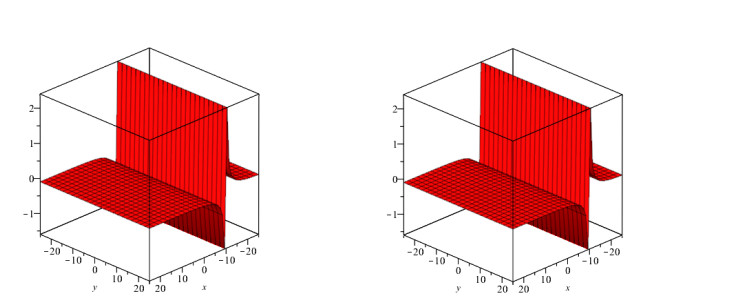
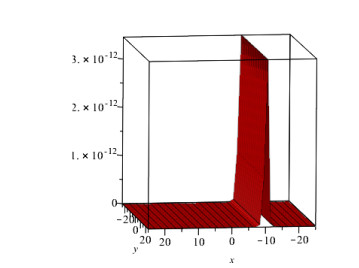
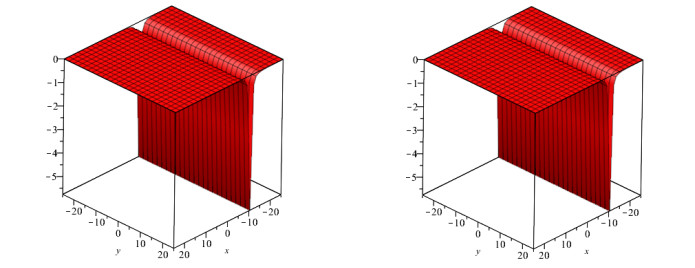
In this study, we investigated the numerical solution of coupled fractional Whitham-Broer-Kaup equation systems using two novel approaches. For any order and different values of the space and time variables, numerical data for the coupled fractional Whitham-Broer-Kaup equations can be found using Maple. We create numerical simulations for system 1 at various υ and τ values in Tables 1 and 2. The Adomian decomposition approach, variational iteration method, optimal homotopy asymptotic method, and natural decomposition method are numerically compared in Tables 3 and 4 in terms of absolute error. Tables 5 and 6 display the results of calculations performed for the coupled system taken into account in Problem 2. We can conclude that the Natural decomposition approach yields more accurate results based on the data in the tables above. For ψ(υ,τ) of Problem 1, Figure 1 shows the behavior of the exact and Natural decomposition approach result, while Figure 2 shows the behavior of the analytical result at various fractional-orders of ϱ. Figure 3 shows the solution to the equation ψ(υ,τ) in various fractional orders, whereas Figure 4 shows the absolute error. The behavior of the exact and analytical solutions for φ(υ,τ) is shown in Figure 5, while the behavior of the analytical result at various fractional orders of ϱ is shown in Figures 6 and 7. The behavior of the exact and analytical solution for ψ(υ,τ) is depicted in Figure 8, while the absolute error for Problem 2 is shown in Figure 9. Similarly, the behavior of the exact and analytical solution for φ(υ,τ) is depicted in Figure 10, while the absolute error is shown in Figure 11 for Problem 2.
7.
Conclusions
The Atangana-Baleanu and Caputo-Fabrizio operators are used in this work to attempt a semi-analytic solution of fractional Whitham-Broer-Kaup equations. To show and confirm the efficiency of the recommended technique, two examples are solved. The numerical results show that the proposed method for solving time-fractional Whitham-Broer-Kaup equations is quite effective and accurate. According to numerical data, the method is very effective and reliable for getting close solutions for nonlinear fractional partial differential equations. Compared to other analytical methods, the proposed method is a quick and easy way to look into the numerical solution of nonlinear coupled systems of fractional partial differential equations. The proposed method gives solutions in the form of a series that is more accurate and take less time to figure out. The calculated results have been displayed graphically and in tables. For both considerably coupled systems, computations were done to determine the absolute error. Several computational solutions are contrasted with well-known analytical methods and the precise results at ϱ=1. Fewer calculations and more precision are two advantages of the present approaches. Lastly, we can say that the proposed approaches are very effective and useful and that they can be used to study any nonlinear problems that come up in complex phenomena.
Conflict of interest
The authors declare that they have no competing interests.









 DownLoad:
DownLoad: