1.
Introduction
The primary and most notable application of linear programming problems is in the field of transportation [25]. It has a wide range of applications in inventory control, supply management, logistics systems, and production planning, among other areas. The dimensions of the classic transportation problem are cost, supply, and demand. However, in light of the current intensity of market rivalry, it is conceivable that the criteria may not be articulated with utmost precision. The price of the product may fluctuate periodically or be contingent upon the manufacturing process. Furthermore, the lack of relevant information on the shipment of products may result in an uncertain and unclear relationship between supply and demand. In response to these factors and with the aim of addressing indeterminate data, [28] established the concept of fuzziness.
Decision-making is of paramount importance in several disciplines such as Economics, Psychology, Philosophy, Mathematics, and Statistics. The indispensability of transportation as a component of distribution networks must be recognized. The primary goal of the transportation problem (TP) is to minimize the cost associated with the movement of commodities from producers to consumers, hence facilitating the fulfillment of customer requests by manufacturers. The transportation problem (TP) involves considerations of price, supply, and demand. Goods can be transported from origins to destinations using various modes of transportation within a transportation system, enabling cost savings and meeting deadlines. The first foundational theoretical principles (TPs) were formulated by Hitchcock [12] and subsequently extensively debated by several writers, as documented in the literature.
The solid transportation problem (STP), also known as the three-dimensional TP, is an enhanced iteration of the renowned TP first formulated by Haley [10]. The primary goal of the process known as STP is to efficiently convey uniform commodities from their point of origin to their destination using transportation methods in order to decrease the overall expense of transportation. Key components of a three-dimensional TP include product availability at source locations, demand at destination locations, and the capacity of transportation modes (such as trucks, cargo flights, goods trains, and ships) used to transport products from sources to destinations. The parameters of the problem are non-deterministic in reality owing to the existence of several elements such as machine breakdowns and labor problems in production, market circumstances, road conditions, and weather conditions during transportation. Random variables are often used to characterize these uncertainties, especially those that are stochastic. When developing a practical SPP (Shortest Path Problem), it is crucial to consider optimizing many objectives, such as reducing transportation time, limiting loss during transit, and lowering transportation expenses. We must consider a stochastic multi-objective STP based on this understanding. The STP has great importance as a research field, both in terms of theory and practical application. Many scholars in this discipline have made significant contributions. The study presents an expanded modi-method to solve a multi-index transportation problem involving three indices [10].
A pressing problem in the transportation industry that requires immediate attention and resolution is the solid transportation problem. Inadequate transportation infrastructure can lead to delays, congestion, or limited connectivity. For example, if a city's public transportation infrastructure is antiquated and incapable of accommodating the increasing demand, the city may face a significant transportation problem. Consequently, travelers may encounter crowded buses, prolonged waiting times, and feelings of annoyance. In order to facilitate the seamless and effective transportation of people and goods, addressing substantial transportation challenges requires meticulous strategic planning, substantial investments in infrastructure development, and proficient administration.
Most real-world decision-making problems are often represented using several options. Multi-choice optimization approaches have become more significant in several domains, such as technology, business, transportation, and military applications. The price indices are the objective function's focus. In this context, the variable cijk denotes the cost associated with transporting a unit of production from a specific source i to a designated destination j using a particular mode of conveyance k. We propose using a multiple-choice format for the cost coefficients of the goal function in the transportation problem, in response to the increase in fuel costs and other important factors. To accurately capture market price fluctuations for all goods, multi-choice supply and demand factors are essential. Healy [11] first created multiple choice programming as a technique for tackling linear programming problems with zero-one variables.
Mathematicians and computer scientists use Newton's split difference interpolation as a computational method to estimate a function based on a set of data points. This method enables the generation of a polynomial function that interpolates the given data points. The use of Newton's Divided Difference Interpolation allows for the resolution of a multi-choice fractional stochastic transportation problem by converting it into a deterministic model [1]. The MCFS-MOTPs may be solved by using a technique that involves interpolating multi-choice parameters, changing probabilistic constraints, linearizing the problem, and applying fuzzy goal programming and the ϵ-constraint method. This approach was proposed by Sayed and Baky [24]. The MOTPs may be effectively addressed by treating it as a chance-constrained programming problem and using the global criteria technique and fuzzy goal programming methodology. This approach allows for the identification of optimal solutions within a realistic timeframe [6]. A novel methodology for analysing Shortest Path Problems (SPP) is proposed, which integrates multi-choice programming with stochastic programming. Additionally, a transformation technique is used to identify the best solution [22]. The study conducted by Nomani et al. [19] proposes a weighted goal programming method to address multi-objective transportation challenges. This methodology allows for the generation of compromise solutions that align with the decision-maker's preferences. The authors, Joshi et al. [13] propose a weighted goal programming method to tackle multi-objective transportation problems. This technique aims to identify compromise solutions based on the decision-maker's preferences. The authors include a numerical example to demonstrate the use of their method.
The study by Pradhan and Biswal [20] proposes an approach for solving linear programming models with multi-choice parameters. This technique involves changing the model into a non-linear mixed integer probabilistic programming problem and using chance constrained programming. The article presents a method for resolving a challenging transportation problem using an objective function that is the quotient of two linear functions [3]. The new goal programming method handles "more/better" and "less/better" aspirations in complex decision problems [4]. The authors propose an alternative method to formulate multi-choice aspiration levels in goal programming, which is easier to understand and can be solved by common linear programming packages [5]. The use of an innovative conic scalarizing function in a recently introduced multi-choice goal programming formulation enables improved adaptability, effectiveness, and dependability in the decision-making process [26]. The researchers address the optimization of transportation problem that include uncertainty and various objectives, specifically focusing on the ratios of costs. They the use of techniques such as weighted sum and fuzzy programming to identify the most favourable trade-offs [23]. They use neutrosophic sets to represent uncertain multi-objective transportation problems and utilizes neutrosophic compromise programming to determine optimum solutions [14]. A novel approach addresses multi-objective transportation problem by considering goals as ratios of linear functions, such as cost per unit carried. This technique builds upon earlier research and has been confirmed by numerical examples provided by Joshi and Saini [15]. The problem of uncertainty in supply and demand for transportation is addressed using a fractional target (cost per unit) and utilizing a range-based strategy for generating robust decisions [16].
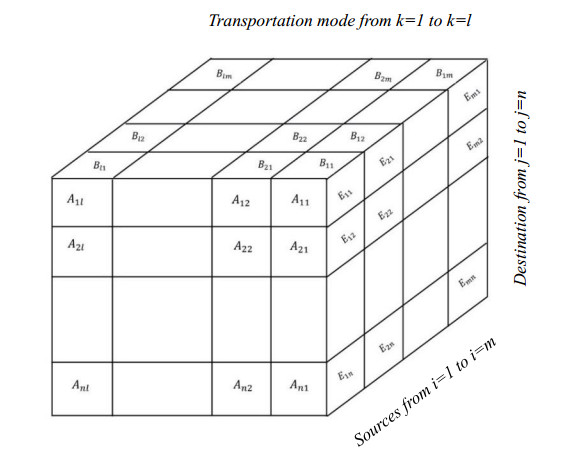
The Solid Fractional Transportation Problem (SFTP) is an extended formulation that incorporates the features of both the FTP and the STP. Within the context of SFTP, each item being carried is evaluated based on three properties: the source, the destination, and a third feature often known as the "mode of transport" or "conveyance". The third feature might denote the mode or means used for conveying the merchandise, so introducing an additional level of intricacy to the problem. Quadratic fractional solid transportation problem was proposed by Basu and Acharya [2]. The SFTP entails optimizing the allocation of items from different suppliers to diverse customers, taking into account the fractional amounts and the unique transportation method for each item. This problem scenario arises in situations when things may be separated into fractional pieces and the transportation procedure requires several techniques, routes, or vehicles. To solve the SFTP, one must address both fractional amounts and the method of transport's associated characteristic. Advanced optimization approaches, such as mixed-integer linear programming or specialized algorithms, are often used to discover optimum or nearly optimal solutions. The SFTP has practical applications in many real-world situations, including Multi-Modal Transportation, Supply Chain with Divisible Goods, Logistics Planning, Emergency Response, and Infrastructure Development. The inclusion of fractional numbers and the mode of transport feature in the SFTP (Figure 1) demonstrates the complexities of real-world transportation situations, resulting in a more precise and complete method for allocating and distributing resources.
In order to solve the SFTP, a minimum of mnl−(m−1)(n−1)(l−1) variables xijk (Figure 2) must have nonzero values in a basic feasible solution. While a fundamental feasible solution to the transportation problem is always present, it may not always be the case for the solid problem. However, it can be demonstrated that there always a solution with the appropriate number of nonzero variables that satisfies all constrains except for the nonnegative ones. Such a solution will be termed a 'basic' solution. Only mn+nl+lm−m−n−l+1 equations (nonzero xijk's) so m+n+l−1 are set at zero [10].
The proposed method addresses the multi-objective capacitated transportation problem (MOCTP) by converting uncertain input data into a deterministic format. The resulting MOCTP is then solved to get a compromise solution, as described by Healy [11]. The use of Fuzzy AI in manufacturing enables the resolution of decision-making challenges arising from uncertainties in transportation. It produces customized solutions with associated confidence levels, facilitating the selection of optimal alternatives that satisfy multiple objectives [17]. This method solves fuzzy linear programming problems with trapezoidal fuzzy numbers for imprecise data using multi-objective programming with different solution approaches [27]. A new fuzzy linear programming method with trapezoidal fuzzy numbers considers decision-maker' risk tolerance and solves problems using goal programming [7]. Furthermore, a two-stage method for fuzzy multi-objective linear programs (such as engineering project selection) uses fuzzy numbers and TOPSIS to find the best project portfolio while considering decision-maker preferences [8]. This research proposes a new method to address uncertainties in transportation problems with multiple objectives [18].
We delved into the multi-choice solid fractional multi-objective transportation problem (MCSF-MOTP) with the factors supply, demand, and conveyance capacity. The coefficients of the objective functions are of the multi-choice type due to inherent uncertainty. To mitigate this uncertainty, the proposed model utilizes the Newton divided difference interpolation polynomial method to condense the multi-option parameters into a single choice. This ensures that the final solution either achieves the ideal or approaches it as closely as possible. Furthermore, we conduct a comparison with other studies to demonstrate the superiority of our model, which exhibits significant proximity to the ideal value. The effectiveness of this model is validated through a numerical illustration employing a ranking approach. Comparison of the approaches are given in Table 1.
The paper is structured in the following manner: Section 1 provides a comprehensive overview of the relevant literature and serves as an introduction. The fundamental terms relevant to this study are presented in Section 2. The notation of this article is discussed in Section 3. The comprehensive problem statement is outlined in Section 4. The solution methodology for the given issue is shown in Section 5. Section 6 introduces a novel approach solution process. Section 7 assesses the effectiveness of the suggested solution approach using a series of numerical examples. Section 8 explores the theoretical and practical consequences of the suggested approach. The work is concluded in Section 9, which also provides suggestions for future research topics.
2.
Basic definitions
In this section, we present basic definitions of various types of solutions to optimization problems.
2.1. Optimal solution
An optimal solution is a result that achieves the best possible outcome based on the given criteria or objectives. It is like finding the most favourable answer among all the available choices.
2.2. Ideal solution
When a problem involves minimization, the ideal solution is one in which each objective function achieves its optimal minimum.
2.3. Anti-ideal solution
When a problem involves minimization, the anti-ideal solution is one in which each objective function achieves its maximum value.
2.4. Feasible solution
A feasible solution to an optimization problem is a set of values for the decision variables that satisfies all of the constraints of the problem.
2.5. Pareto optimal solution
If the objective function cannot be enhanced without decreasing one or more other objective values. A feasible option is referred to as a Pareto optimum solution (Figure 3).
3.
List of notation used in this study
In this section, we provide a list of the notation that will be utilized throughout this research, ensuring clarity and consistency in our presentation and analysis.
● R: number of objective functions.
● m: number of supply sources.
● n: number of demand destinations.
● l: number of conveyances.
● xijk: The number of shipment units from ith supply source to jth demand destination using kth transportation mode.
● Zr: rth objective function.
● cijk(r): The cost of transporting one unit of the shipment from ith supply source to jth demand destination using kth transportation mode of rth objective function.
● dijk(r): The profit of transporting one unit of the shipment from ith supply source to jth demand destination using kth transportation mode of rth objective function.
● Ajk: Required number of units using kth transportation mode to be send to destination j.
● Bki: Required number of units using kth transportation mode to be send from source i.
● Eij: Number of available units at source i demanded by destination j.
● ZUr: Upper value of rth objective function.
● ZLr: Lower value of rth objective function.
● Ur: Upper bound of the rth objective function.
● Lr: Lower bound of the rth objective function.
● gr: the aspiration or target level for the rth goal.
● η: Satisfaction level of the decision maker.
● ρ: The general deviational variable for all objectives.
● μr(Zr(x)): Linear membership function for the rth objective function.
● Wr: The weight assigned to the rth objective function.
● Z∗r: The ideal objective.
4.
Problem statement
A transportation company needs to transport its goods from various manufacturing sites to different retail locations. In this scenario, where a uniform product is transported from the ith manufacturing facility to the jth retail store using the kth vehicle, there are m manufacturing facilities, n retail stores, and l vehicles. Let xijk represent the quantity of the product in units.
The mathematical formulation of the problem is as follows, with solid fractional transportation cost functions assumed to be of the multi-choice random parameters, meaning their values are not always fixed.
Model 1:
Where cijk(r)=(c1ijk(r),…,cs−1ijk(r)).
Subject to
The MCFS-MOTP is a complex optimization problem encompassing multiple factors in real-world transportation scenarios. Application of MCFS-MOTP are as follows
 Supply chain management: In supply chain management, the transportation of goods from multiple sources to multiple destinations often involves multiple objectives such as cost minimization, time minimization, and carbon footprint reduction. The multi-choice solid fractional multi-objective transportation problem can help optimize the allocation of goods, considering multiple objectives simultaneously.
Supply chain management: In supply chain management, the transportation of goods from multiple sources to multiple destinations often involves multiple objectives such as cost minimization, time minimization, and carbon footprint reduction. The multi-choice solid fractional multi-objective transportation problem can help optimize the allocation of goods, considering multiple objectives simultaneously.
● Container shipping: Choosing the most cost-effective and environmentally friendly route for containerized goods, considering multiple carriers and partial shipments.
● Air cargo transportation: Optimizing air cargo delivery, balancing cost, delivery time, and carbon emissions while dealing with discrete aircraft capacity and potentially splitting shipments.
● Oil and gas pipeline transportation: Choosing optimal pumping schedules and flow rates to minimize operational costs and energy consumption while satisfying demand at various delivery points.
 Emergency services planning: During emergencies, such as natural disasters or accidents, emergency services need to be efficiently deployed to affected areas. The multi-choice solid fractional multi-objective transportation problem can aid in determining the optimal allocation of emergency resources, considering factors like response time, cost, and the number of affected individuals.
Emergency services planning: During emergencies, such as natural disasters or accidents, emergency services need to be efficiently deployed to affected areas. The multi-choice solid fractional multi-objective transportation problem can aid in determining the optimal allocation of emergency resources, considering factors like response time, cost, and the number of affected individuals.
 Urban transportation planning: In order to enhance urban transportation systems, policymakers must take into account various objectives, including the minimization of travel time, the reduction of congestion, and the maximization of passenger satisfaction. The multi-choice solid fractional multi-objective transportation problem can be utilized to optimize the allocation of transportation resources, such as buses or trains, in order to achieve these objectives.
Urban transportation planning: In order to enhance urban transportation systems, policymakers must take into account various objectives, including the minimization of travel time, the reduction of congestion, and the maximization of passenger satisfaction. The multi-choice solid fractional multi-objective transportation problem can be utilized to optimize the allocation of transportation resources, such as buses or trains, in order to achieve these objectives.
 Waste management: Waste disposal and recycling involve transportation of waste materials from various collection points to treatment or recycling facilities. The multi-choice solid fractional multi-objective transportation problem can help in optimizing the transportation routes and selection of vehicles to minimize costs, reduce environmental impact, and maximize recycling efficiency.
Waste management: Waste disposal and recycling involve transportation of waste materials from various collection points to treatment or recycling facilities. The multi-choice solid fractional multi-objective transportation problem can help in optimizing the transportation routes and selection of vehicles to minimize costs, reduce environmental impact, and maximize recycling efficiency.
 Healthcare logistics: Within the healthcare industry, the delivery of medical supplies, blood, organs, and drugs frequently serves numerous purposes. The objectives may encompass the minimization of transportation expenses, the reduction of delivery duration, and the assurance of the quality and safety of the transported goods. The utilization of the multi-choice solid fractional multi-objective transportation problem can optimize medical logistics and guarantee the efficient distribution of healthcare resources.
Healthcare logistics: Within the healthcare industry, the delivery of medical supplies, blood, organs, and drugs frequently serves numerous purposes. The objectives may encompass the minimization of transportation expenses, the reduction of delivery duration, and the assurance of the quality and safety of the transported goods. The utilization of the multi-choice solid fractional multi-objective transportation problem can optimize medical logistics and guarantee the efficient distribution of healthcare resources.
These examples illustrate the practical applications of the multi-choice solid fractional multi-objective transportation problem. The problem's capacity to concurrently assess several objectives renders it a valuable instrument for decision-making and optimization across many industries.
5.
Solutions methodology
This section outlines the methodology for addressing the transportation problem, which encompasses a multitude of solutions and diverse objectives. Initially, the multi-choice parameter undergoes modification by the utilization of Newton's divided difference interpolation. Next, the probabilistic restrictions are transformed into their deterministic form by the utilization of the chance constrained technique.
5.1. Newton's divided difference interpolating polynomial for multi-choice parameters
The best option is obtained by transforming a multi-choice parameter using Newton's divided difference Interpolation approach. To allow the definition of the interpolating polynomial for each choice in a multi-choice parameter, declare an integer variable. Given that there are s possible cost alternatives in the current context, the integer variables wtcijk(t=0,1,…,s−1) are employed.
Every multiple-choice parameter has a unique divided difference that is specified by the alternatives that are offered. Using the data provided in Table 2, which outlines the diverse orders of divided differences, the Newton's divided difference (NDD) interpolating polynomial is obtained for the cost parameter cijk(r)=(c1ijk(r),…,cs−1ijk(r)) in Eq (7). The notation cijk=(c1ijk,…,cs−1ijk) will be employed in order to maintain generality.
Therefore, the interpolating polynomial along with these values is
where
Similarly, for the profit parameter dijk, define integer values ytijk and compute the interpolating polynomial. The software replaces the multi-choice parameters with an interpolating polynomial. This allows the mathematical model to be formed.
Subject to the constraints (1) to (6).
5.2. Fuzzy linear membership function [9]
The fuzzy linear membership function of the MOTP measures how well a given solution fits the objective functions. Based on the degree of fulfillment or relative relevance of each goal, the mathematical function assigns a membership value to each one, which ranges from 0 to 1. It is possible to define the fuzzy linear membership function exactly as follows:
The membership value of the objective function is denoted as μ(x), where x is the value of the objective function, and a and b are the lower and upper bounds, respectively, of the objective function. The fuzzy linear membership function measures the degree to which a specific objective function value falls within the desired range. Values that are in close proximity to the lower limit exhibit a membership value that is nearly 0, indicating a diminished level of contentment. In contrast, values that are closer to the upper limit exhibit a membership value that is almost 1, signifying a higher degree of pleasure. Intermediate values possess membership values that span from 0 to 1, denoting the degree of contentment or importance. The application of the fuzzy linear membership function to each objective function in the Multi-Objective Transportation Problem (MOTP) allows decision makers (DMs) to assess the degree to which various solutions fulfill their intended results. This enables them to make informed assessments by taking into account the specific objectives and their related levels of membership.
The mathematical model may vary depending on the specific problem formulation, as well as the objectives and constraints involved. Linear programming, genetic programming, fuzzy programming, and evolutionary algorithms can be employed to tackle the multi-objective transportation problem (MOTP) and offer optimal or near-optimal solutions that successfully balance the conflicting objectives. To successfully navigate the domain of the MOTP, one must use a complex mathematical model that incorporates decision factors, goals, and limitations. The model aims to find a compromise between reducing the highest possible values and maximizing the closest possible values for each objective function as commodities move from their origins to their destinations.
5.3. Proposed method
In the context of MOTP, the fuzzy linear membership function, denoted as μr(Zr(x)), establishes a connection between the lower bound Lr and upper bound Ur of each objective function Zr(x). To obtain the upper bound and lower bound value, we have to find the optimum (ideal value) and worst (anti ideal) values of the corresponding Zr(x). The determination of the degree of membership or satisfaction for a certain solution x is based on the given range between Lr and Ur.
whenever Lr≠Ur, and if Lr=Ur, then, 0≤μr(Zr(x))≤1∀r,r=1,2,…,R.
Within the context of real-world MOTPs, some goals possess a higher level of significance compared to others, considering the existing limitations. Although there are several methods that provide varied compromise solutions, decision makers (DMs) often have their own distinct priority system that is based on individual requirements. Our novel approach not only provides a middle ground solution when preferences are not clearly specified, but it also consistently produces outcomes that are in line with various desires. Goal weights are used to indicate preferences, facilitating efficient goal management throughout the problem-solving process.
Various methodologies are documented in the existing body of literature, with a selection of them being elaborated upon in Subsection 5.4. These methodologies aim to transform multi-objective optimization issues into single-objective optimization problems. The multi-objective optimization problem is transformed into a new single-objective transportation problem in the proposed model, with the objective being to Maximizeρ′=∑Rr=1ηρ(1−Wr), where ρ and η represent the general deviational variable and the decision maker's satisfaction level for all objectives, respectively, and Wr is the weight assigned to the rth objective. The constraints for each objective are converted into Z∗r+ρ(1−Wr)(ZUr−ZLr), where Z∗r (the ideal objective) is achieved by solving the optimization problem mentioned above for each objective Zr(x),r=1,2,…,R regardless of the other objectives.
The problem with many objectives (9) can be simplified to the single-objective problem as follows:
Model 2:
Subject to
Instead of utilizing a deviational variable, we have implemented a deviational function ρ(1−Wr) in this model. However, it is possible to argue that the weights (Wr) allocated to the rth objective are determined by multiplying (1−Wr) by ρ. However, it should be noted that a higher value of Wr in this function will lead to a lower value of the deviational function. By allocating a higher weight, we will bring our objective closer to the ideal objective. This method can identify a mutually agreeable solution without any bias, as well as in cases where preferences are explicitly stated. Based on the aforementioned analysis, it can be inferred that this approach is universally applicable to many forms of multi-objective optimization problems.
This model introduces a factor 1(ZUr−ZLr) in addition to the current ρ(1−Wr). The variables ZUr and ZLr denote the upper and lower limits within which the compromised solution will be situated. The solution must not surpass this specified range. The optimum allocation can be used to obtain this range for a rth aim. The upper bound, denoted as max, is achieved by substituting the allocation of other objectives into the rth objective. On the other hand, the lower bound, represented by the lower bound, corresponds to the ideal solution Z∗r.
This underscores the necessity of setting different solutions in order of priority due to the wide variety of approaches available to deal with multi-objective transportation problems. To address this problem, a tool that facilitates the assessment and choice of the best course of action must be used. It is advantageous to use the Technique for Order of Preference by Similarity to Ideal Solution (TOPSIS) [21]. The TOPSIS approach facilitates the ranking of several methods by evaluating the performance of their optimum solutions. Within this particular framework, the alternatives represent the most favourable resolutions, while the criteria correspond to the objective functions. Essentially, TOPSIS assists in identifying the most efficient technique for attaining optimum solutions to the given challenge.
5.4. Other approaches for solving the MCSF-MOTP
Goal programming and improved multi-choice goal programming are used to address the Multiple Objective Traveling Salesman Problem (MOTP), and their definitions are provided thereafter.
5.4.1. Goal programming (GP) approach [4]
Now, let us quickly examine the goal programming strategy to solve MC-MOTP. Additionally, let d+r=max(0,Zr−gr) represent positive deviations and d−r=max(0,gr−Zr) represent negative deviations associated with the rth aim of the objective function. The mathematical model is defined as follows:
Subject to
and the constraints (1) to (6).
The rth aspiration level, yr, is a continuous variable that falls between the upper bound (gr,max) and lower bound (gr,min).
5.4.2. Revised multi-choice goal programming (RMCGP) approach [5]
The Revised multi-choice goal programming approach is implemented as a solution to address the MOTP by assuming that the objective functions take into account various objectives, which will be referred to as:
Subject to
and the constraints (1) to (6).
Here, e+r and e−r represent positive and negative deviations associated with the rth objective of |yr−gr,max|, while αr denotes the weight assigned to the total of the deviations of |yr−gr,max|.
5.4.3. Conic scalarization approach (CSA) [26]
Mathematical formulation of CSA is given by
Subject to
and the constraints (1) to (6).
5.4.4. Weighted sum method (WSM)
The approach of the weighted sum is often used to solve a Multiple Objective Linear Transportation Problem (MOLTP), yielding diverse outcomes based on the assigned weights. The fundamental concept of this approach is assigning a non-negative weight, denoted as Wr, to each objective function Zr. The aim is to minimize the new objective function, represented as ∑Rr=1WrZr(x), while considering the constraints of the task. While this strategy may be user-friendly, it is crucial to pre-determine the weights that the decision maker (DM) will apply, since they significantly impact the outcome. The application of the weighted sum approach results in the following normalized single-objective optimization problem:
Subject to:
The weights Wr,r=1,2,…,R associated with the objective function must meet the following requirements. ∑Rr=1Wr=1,0≤Wr≤1,r=1,2,…,R. Using the aforementioned approach, distinct solution points are derived for varying weights, which accurately represent the decision-maker's preferences. This approach becomes ineffective when the decision maker lacks knowledge or understanding of the individual's preferences.
6.
Proposed model solution process
Step by step procedure of the proposed algorithm is as follows (Figure 4):
Step 1: Apply the Newton's divided difference formula to transform the Multichoice parameters into a single choice.
Step 2: Construct the MCSF-MOTP by using the single option parameter obtained from step 1.
Step 3: Optimize the MCSF-MOTP treating it as a single goal transportation problem, without considering any other objectives. Perform this process R times for each of the R goals. If the solution is identical, proceed to step 7 if not proceed to step 4.
Step 4: Compute the linear membership function, denoted as μr(Zr(x)) for the rth objective function.
Step 5: Assign a distinct weight to each target to determine its level of importance. The weight assigned to this factor influences the decision-making process in order to align with the priorities of the DMs.
Step 6: Using the model 2 given in Section 5.3, transform the imprecise model that was created in Step 3 into a precise model.
Step 7: Show the decision makers the possible solution. Continue to Step 8 if the DM is satisfied. If not, go back to Steps 5 and continue assessing each goal until a compromise is struck.
Step 8: Utilize the TOPSIS approach to assess solutions and get a ranked list of optimal alternatives for various transportation problem.
Step 9: Stop.
7.
Numerical example
In this part, we use our suggested approach to demonstrate the following MCSF-MOTP instances. Consider the following problem (Tables 3 and 4) with integer variables wijk,yijk,uijk, and vijk related to cijk and dijk for the first and second objective function Z1 and Z2, respectively. The mathematical formulation of the numerical example is given in the appendix.
8.
Results and discussion
The multi-objective function, including restrictions, is represented in solid fractional form and illustrated by numerical examples. The cost and profit are considered as random variables with many possible values. The solutions were derived using the LINGO 18.0 software (Table 5).
The comparison presented in Table 6 reveals that the objective values of the weighted sum method (WSM), Goal Programming (GP), Revised multi-choice goal programming (RMCGP), and Conic scalarization approach (CSA) exhibit slight variations across different weights. However, the proposed method consistently yields compromise optimal solutions that are closer to the ideal value for various weights. Consequently, it can be concluded that the proposed method demonstrates greater consistency and provides solutions that are closer to the ideal when compared to the WSM, GP, RMCGP, and CSA.
Based on the provided description, it is evident that the method suggested in the paper exhibits a high level of proximity to the ideal value, indicating its exceptional performance and near-equivalence to the optimal conclusion. The proximity of the proposed approach to the ideal value suggests that the proposed method exhibits a high level of effectiveness. Furthermore, the statement suggests that the proposed method can be considered useful for DMs who strive to make optimal choices. Given the tight alignment between the suggested approach and the ideal value, it can be inferred that the method has the potential to assist decision makers in making optimal choices. The persistent superiority of the suggested method in terms of objective values suggests its potential as a beneficial tool for decision-makers to enhance their decision-making process by providing more informed and optimal options. Decision makers can anticipate achieving outcomes that are better aligned with their aims and objectives by implementing the proposed strategy, thus enhancing decision-making processes and results.
These graphs Figures 5 and 6 show comparison of the two objective functions Z1 and Z2 solutions in the mentioned methods which shows that the proposed method has better achievement result.
The graph in Figure 7 depicting the comparative analysis of the weighted sum, GP, RMCGP, Conic, and suggested technique with respect the ranking. The superiority of the suggested technique over the weighted sum, GP, RMCGP, and Conic is evident. It may be compared to a race, where our approach is achieving success by being more aligned with our desired outcome. The graph serves as a narrative device, conveying that the suggested technique is adept at identifying accurate solutions. The graph represents a solid fractional transportation problem with many objectives.
9.
Conclusions
We explore the MCSF-MOTP. Random multi-choice factors constitute the cost and profit parameters. Newton's Divided Difference Interpolation was used to reduce the multi-option parameters to a single choice, ensuring that the final solution either becomes ideal or gets as close to it as possible. When compared to other studies, our model emerges as superior and is quite close to the ideal value. The suggested model, employing the TOPSIS approach, achieves outstanding performance and ranking results, placing it close to the ideal value. The model can assist decision makers in making better decisions, as evidenced by the close approximation to the optimal value. By enhancing performance and empowering manufacturers to improve their decision-making processes, our study significantly advances the field by providing a dependable and effective solution for MCSF-MOTPs. There is no suitable algorithm for discovering MCSF-MOTP compromise solutions. In addition to comparing our method with the conic, GP, RMCGP, and weighted sum approaches, Table 7 presents rankings of the compromise options. LINGO 18.0 software is used to address the MCSF-MOTP mentioned above.
Further research incorporating multiple-choice or unknown aspects pertaining to conveyance characteristics, supply, and demand can enhance the model's validity. Researchers can also investigate the model's effectiveness in other fields, such supply chain management and economics. We present a novel approach to addressing MCSF-MOTPs, making it an invaluable resource for decision-makers in the industrial sector. Because modern manufacturing is dynamic, new approaches to decision-making are required for success in an increasingly globalized world.
Author contributions
Vishwas Deep Joshi: Writing–review and editing, Writing–original draft, Visualization, Validation, Supervision, Software, Resources, Project administration, Methodology, Investigation, Formal Analysis, Data curation, Conceptualization.
Medha Sharma: Writing–review and editing, Visualization, Validation, Software, Resources, Methodology, Investigation, Formal Analysis.
Huda Alsaud: Writing–review and editing, Visualization, Validation, Supervision, Resources, Formal Analysis, Funding acquisition.
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Acknowledgments
The authors would like to extend their sincere appreciation to Researchers Supporting Project number (RSP2024R472), King Saud University, Riyadh, Saudi Arabia.
Conflict of interest
The authors declare no conflicts of interest.
Appendix
Mathematical formulation of the numerical example discussed in Section 7:
and
Where
Subject to
Where the values of Ajk,Bki,Eij, and the transportation cost cijk and profit dijk for the two objective functions are given in the Tables 3 and 4.









 DownLoad:
DownLoad:


 Supply chain management: In supply chain management, the transportation of goods from multiple sources to multiple destinations often involves multiple objectives such as cost minimization, time minimization, and carbon footprint reduction. The multi-choice solid fractional multi-objective transportation problem can help optimize the allocation of goods, considering multiple objectives simultaneously.
Supply chain management: In supply chain management, the transportation of goods from multiple sources to multiple destinations often involves multiple objectives such as cost minimization, time minimization, and carbon footprint reduction. The multi-choice solid fractional multi-objective transportation problem can help optimize the allocation of goods, considering multiple objectives simultaneously.






