1.
Introduction
During the quick cycle of the twenty-first century, people faced various challenges, like the terrorist attacks of 9/11 in 2001, the Catalina typhoon in 2005, the Pakistan earthquake in 2005, the Wenchuan earthquake in Sichuan, China (2008); and the explosion accident at Port Group in Tianjin, China (2015). The corona virus is spreading like wildfire over the world, and it has affected over 200 countries. The virus was discovered and reported for the first time in December 2019 in Wuhan, China. On February 11, 2020, the World Health Organization (WHO) designated the SARS-CoV-2 infection sickness caused as 2019 noval coronavirus (Covid-19). Many individuals are currently suffering from the Covid-19 outbreak all around the world. The lives of people on Earth are extremely difficult under the current situation owing to the lockdown. The WHO announced a global pandemic and designated Covid-19 an epidemic. To avoid continued aggravation of the situation, decision makers (DMs) or the emergency management agency should establish the rules or utilize an effective crisis approach in an emergency. Making a rapid and reasonable decision in an urgent situation is a problem for the different management. In many areas, emergency decision making is an essential element of emergency responders and an area of research in learning environments. People are often constrained theoretically instead of totally logical when taking decision in this circumstance [25,30,35]. As a result, it is critical to develop decision making techniques that comprehend the behavior of people in order to give effective methods for Covid-19 personnel to handle in emergency scenarios.
The current Covid-19 outbreak has had a severe significant influence on individual development and economic progress. In the following the end of this kind of disastrous emergency, it is critical that efficient and appropriate measures are implemented to observe the worsening and rapidly deteriorating of the scenario (e.g., medical management, first aid learning, enhanced necessary safety devices, constrained mass transit, and so on). Therefore, an important study topic for emergency planning is to somehow behave in an efficient and simple way, or how to select a suitable option in the early phases of a catastrophe, and several experts have addressed these problems. In [35], proposed an interval dynamic reference point method (IDRPM) that was focused mostly with inappropriate or insufficient data in emergency scenarios and selecting best emergency possibilities, while neglecting DMs' mental behaviour in emergency cases.
The resistance to diffusion of conservation of the mean implies an aversion to risk. In general decision-making theories, spread aversion preserved by the mean, commonly known as serious risk rejection, is very constraining than weak risk aversion. The control and prevention of the coronavirus, as well as the prevention of disease spread, should indicate risk aversion based on the situation. The proposed invention provides a variety of Covid-19 preventive and control options as well as risk avoidance. We provide a high aversion to risk as a basis for individuals to become secure and avoid the effects of Covid-19, like as immunisation and hospital treatment. Developing online first-aid training, separating the infected individual from other normal individuals, and training experts, among other things. In [19,32], used cumulative prospect theory to define the requirements for loss aversion. Since a social disruptions of Covid-19 have expanded over the world, many people have asked about the role of policymakers and probabilistic reasoning. Even though decision makers and probabilistic reasoning are important when dealing with such pentamic scenarios. Each company follow three phases (shown in Figure 1) to solve any problem: Planning, implementing, and finally making a decision.
Problems involving unclear conditions happen often in DMs, however they are difficult to solve due to the difficulty of modelling and the scenario regulating these ambiguities. In [3], proposed intuitionistic fuzzy set (IFS) as an expansion of FS [49] by addition nonmembership grade (NMG) to membership grade (MG), with the constraint that the combination of MG and NMG bounds with one. IF elements' geometric perspective was provided by [4]. Afterwards, In [5], developed the interval-valued IFS (IVIFS), that is distinguished by an interval-valued MG and NMG. Weighted geometric aggregation operators for IFNs were presented by [43]. These investigations can deal with unclear information that has been evaluated. The linguistic-intuitionist fuzzy set (LIFS) was defined by [50]. The IFS failed because space was limited for MG and NMG, Yager introduced an innovative method known as the Pythagorean fuzzy set (PFS).
The Pythagorean fuzzy set (PFS) is an extention of the IFS concept that fulfills the constraint that the square combination of its MG and NMG be less than or equal to one were presented by [44,45]. For example, Multi-parametric similarity measures on PFS were investigated by [28]. Moreover, Certain aggregation operators (AOs) in the PFS surrounding, were presented by [45], whereas in [18], extended PFS to novel logarithmic operating rules and related aggregation operators. In [8], introduced and addressed the innovative idea of a Pythagorean Probabilistic Hesitant Fuzzy Set in decision making. Einstein t-norm functions for PFNs were implemented by [13,14]. Symmetric PF AOs were developed by [27]. Under the PF paradigm, In [15], provided consider all possible DM approaches for solving MCDM scenarios with instant chance. In [16], suddenly introduced a Linguistic PFS and offered a DMP solutions predicted by the linear software system are given in [17].
Some scholars have explored another notion known as q-rung orthopair fuzzy set (q-ROFS) [1,21] to broaden the IFS and PFS field. Regardless of the fact that IFS and PFS have been broadly researched and utilised in a number of fields, their range for offering data remains restricted. To address the issue, qROFS are a more effective technique of evaluating different ambiguity than IFS and PFS were originally developed by [46]. The q-ROFSs are also characterised as two level information, such as C and Y, with qth power limitation i.e. 0≤Cq+Yq≤1,q≥1. It is noticeable that q-ROFSs are very specific than IFS and PFS, thus putting q = 1 and q = 2 reduces the collection to IFS and PFS. There are three types of q-ROFSs: The first and most significant component is basic operating laws. In [11], demonstrated the mathematical procedures associated with generalised OFS. The concept of the continuities and diferentials of q-ROF processes was explained by [12]. For q-ROFS were suggested by [47]. In [29], expressed concern over q-ROFN. The second component is the AOs, which are a useful instrument when utilizing DMP. In [26] provided an overview. q-ROFS were described by [42]. Several different forms of q-ROFN have been discussed, such as the weighted Heronian mean (HM) by [36], the weighted Maclaurin symmetric mean (MSM) by [37], the weighted power partioned MSM by [7], and the weighted Bonferroni mean (WBM) by [20] for aggregating the DM data provided by reseachers. The third component of the decision maker's role is to evaluate the choices.
Although the ideas of IFS, PFS, and q-ROFS have a broad variety of applications in numerous real-world sectors, each has its own set of constraints that are pertinent to MG and NMG. In [31], developed the innovative notion of linear diophantine fuzzy set (LDFS) with the adding of reference parameters (RP) to eliminate these restrictions. Due to the usage of RP, the LDFS method is more efficient and adaptable than other techniques. By filling holes left by current methods and adding RPs, this collection enlarged the space for MG and NMG. LDFSs also provided the two levels of data, the combination of which is restricted by one, and the combination of its factors is also limited by one, for example, the combination of RPs with MG and NMG is less than or equal to one.
As a consequence, in such real-life scenarios, the combination of the RPs that an option meets in order to satisfy the feature gained by DM is frequently larger than 1, suggesting that LDFS didn't gain his goal with respect to RPs. When it relates to RP, the LDFS has its own number of constraints. As a consequence, we present the q-LDFS, an innovative notion (q-LDFS). The reference parameter's qth value is added in q-LDFS to accommodate the area of structural components as well as the area of MG and NMG. Across the article, the reason for the suggested paradigm is investigated intensively. Now we'll go into some of the important themes of the paper.
Sometimes in real-life environments, the combination of MG and NMG in any kind of FS may be larger than one, for instance, 0.8+0.7>1, and the square combination may also be larger than one, for instance, (0.82+0.72>1). In several instances, both IFS and PFS have failed. To account for these shortcomings, the constraints on MG and NMG are altered in 0≤Cq+Yq≤1 in the situation of q-ROFS. We can solve MG and NMG even for absolutely enormous values of q. In certain real cases, if both MG C and NMG Y are equal to 1 (i.e. C=Y=1) then we find 1q+1q>1, that exceeds the q-ROFS constraint. To address this constraints, in [31], presented the notion of LDFS, that includes the function of RPs, that satisfy the restriction 0≤(a)C+(b)Y≤1∀h∈M with 0≤a+b≤1. Although, the combination of the RP's values provided by DM may be greater than one, i.e. a+b>1, which violates the LDFS limitation. As a consequence, in accordance of RPs, LDFS failed of his target. It restricts the MADM's options and impacts the optimal decision. To resolve this conflict, we present the innovative notion of the non-LDFS, which can cope with such scenarios.
The main contributions of the paper are as follows:
● An extended version of MABAC method for group decision-making under a non-linear diophantine fuzzy environment is proposed.
● The objective is to implement the function of qth power of reference parameters (RPs) in non-LDFS while IFSs, PyFSs, q-ROFSs and LDFSs cannot handle qth parameters.
● Our third aim is to build close relationships between the present study and problems with the MADM. We established two major algorithms to handle the data in a parametric manner with multi-attribute complexities.
● We introduce various score functions and accuracy functions in the ranking of feasible alternatives in the MCDM approach.
● The main goal of the presented work is to create a solution technique for covid-19 by introducing MABAC method under non-linear diophantine fuzzy set (non-LDFS).
This paper is structured as follows: Section 2 introduces the fundamental ideas of FS, IFS, PFS, q-ROFS, and LDFS. In Section 3, We explain the innovative idea of q-LDFS and provide an example to explain several q-LDFS operations. The notion of q-LDFSs is introduced in Section 4 for the Non-LDFWAA, Non-LDFOWAA, and Non-LDFHWAA operators. Section 5 provides the concept of q-LDFSs for defining Non-LDFWGA, Non-LDFOWGA, and Non-LDFHWGA operators, as well as providing different score and accuracy for comparing Non-LDFNs with various orders. Section 6 introduces the MADM idea using the Non-LDFWAA and Non-LDFWGA operators. In Section 7, a case study on Coronavirus disease 2019 (Covid-19) in Wuhan, China, in December 2019 is presented to demonstrate the applicability of the proposed method by utilizing MABAC method in a Non-LDF environment and their score function to determine how to reduce the disease's preventive alternative. In Section 8, we compare the proposed technique to current methods in detail and examine the aggregated findings as the influence of score functions on the final selection. Section. 9 outlines the conclusion of the research.
2.
Preliminaries
Within that part, we explain the fundamental definitions related to FS, IFS, PFS, and q-ROFS, etc. To comprehend the notion, we study the basic concept and characteristics.
2.1. Fuzzy set (FS)
In [49], a fuzzy set ϝ on δ is stated as follows:
where the transformation C: δ → [0, 1] indicates the membership grade (MG) of each η ∈ δ. In [5], proposed the notion of MG and NMG on the condition that the addition of MG and NMG bounded with unity.
2.2. Intuitionistic fuzzy set (IFS)
In [3], an intuitionistic fuzzy set ℑ on δ is stated as follows:
where the mappings C:δ → [0, 1] indicates the MG and Y:δ → [0, 1] indicates the NMG of each η∈δ, respectively. Such that
The indeterminacy or hesitation element can be denoted as
In many real world situations, IFS cannot solve when
To remove this drawback, In [44,45], proposed the PFS, which is an expand the idea of IFS by [3].
2.3. Pythagorean fuzzy set (PFS)
In [44,45], a Pythagorean fuzzy set (PFS) ℑp on δ is expressed in the form:
where Cℑp(η)∈[0,1] and Yℑp(η)∈[0,1] indicates the MG and NMG for each η∈δ, respectively and the inequality
The hesitant part is formulated as
The IFS and PFS cannot deal in the situation when the addition of MG and NMG and the addition of square are also greater than one, therefore the q-ROFS proposed by [46] which is the extended form of IFS and PFS.
2.4. q-rung orthopair fuzzy set (q-ROFS)
In [45], a q-rung orthopair fuzzy set O on δ is given by the following:
where Cδ, Yδ:δ → [0, 1] represents the MG and NMG of the option η∈δ and for every η we have
In general,
is called indeterminacy degree of η∈δ
2.5. Linear diophantine fuzzy set (LDFS)
In [31], a linear diophantine fuzzy set HD on δ is specified as follows:
where, CD(η),YD(η),a,b∈[0,1] are denoted by ML, NML, and the RPs respectively, and satisfy the constraint
with 0≤a+b≤1. Such RPs may assist in the description or identification of a certain framework. The level of indeterminacy is specifically defined:
where χ is the RPs associated with the level of indeterminacy.
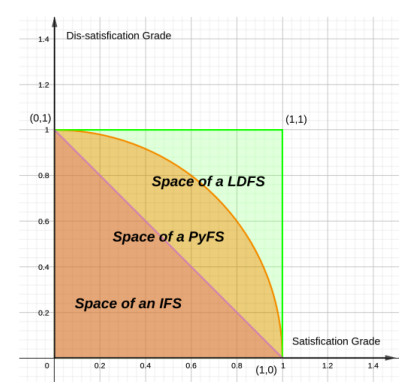
The graphical representation of the comparison spaces of the IFS, PFS, and LDFS are given in Figure 2.
However, in some practical situations, the combination of RPs that satisfy the attribute of DM might be greater than one. Therefore, LDFS has not satisfied its RP objective. To remove this drawbacks, we suggest the novel notion of q-linear diophantine fuzzy set (q-LDFS), which can be solved in such circumstances.
3.
q-Linear diophantine fuzzy set (q-LDFS)
In [2], let δ represent the non-empty set. A q-LDFS ŁDq on δ is generally defined:
where, CDq(η),YDq(η),a,b∈[0,1] are MG, NMG and RPs respectively. These functions fulfill the restriction;
with 0≤aq+bq≤1. These RPs may assist in the description or identifying of the given framework. The component of the hesitation that may be computed is as follows:
where ξ are the RPs related to the grade of indeterminacy or hesitation. A specific system is categorized and described by RP which also modify the physical interpretation of the framework. They increase the level space utilised in q-LDFS and remove restrictions on them. We generalise the concept of LDFS in q-LDFS by expanding the RP and classifying it as; the addition of the qth power of the RPs is limited by one, and the qth power of RPs with MG or NMG is added that fulfil the absence of RP.
3.1. q-linear diophantine fuzzy nnumber (q-LDFN)
A q-linear diophantine fuzzy number (q-LDFN) is a set of fuzzy numbers.
where ρ is the q-linear diophantine fuzzy numbers with requirements.
● 0≤(a)q+(b)q≤1,q≥1.
● 0≤(a)qCDq(η)+(b)qYDq(η)≤1.
● 0≤(a),CDq(η),(b),YDq(η)≤1.
The following definitions are for absolute q-linear diophantine fuzzy set (Absolute q-LDFS) and null or empty q-linear diophantine fuzzy set (q-LDFS).
3.2. Absolute q-LDFS
Absolute q-LDFS on M is defined as
3.3. Empty or null q-LDFS
Empty or null q-LDFS on M is defined as,
As we know that,
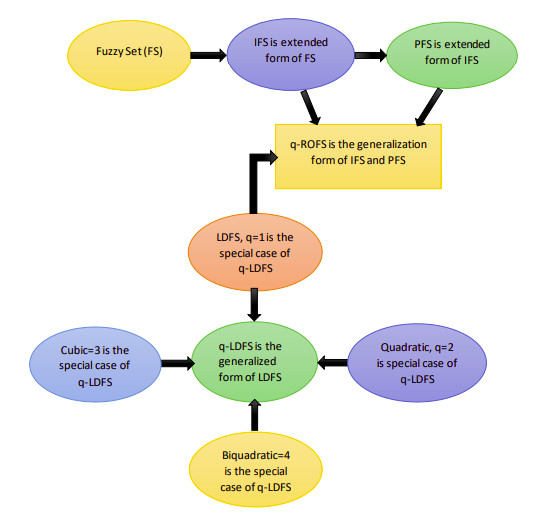
● q-LDFS is reduced to LDFS when we enter q = 1 in Definition 6.
● q-LDFS is reduced to quadratic DFS when we enter q = 2 in Definition 6.
● q-LDFS is reduced to cubic DFS when we enter q = 3 in Definition 6.
● q-LDFS is reduced to bi-quadratic DFS when we enter q = 4 in Definition 6, and so on as illustrated in Figure 3.
Which are the benefits of q-LDFS to fluctuations in q values. It should be observed that as the rung q increases, the diophantine area extends, and thus the border restrictions have a wider area that can transmit a wider range of fuzzy data.
As illustrated in Figure 3, Atanassov's IFS are q-ROFs when q = 1 and Yager's Pythagorean fuzzy sets are q-ROFs when q = 2.
(1) For any q≥1, a q-ROF is any intuitionistic fuzzy set.
(2) A PFS is an intuitionistic fuzzy set.
(3) For q≥2, any PFS subset is a q-ROF.
LDFS, q-ROFS, PyFS, IFS, and FS are all special instances of q-LDFS, according to the above observations.
3.4. Buying of a mobile
Consider this: If a person wants to acquire a mobile phone from the top companies, he has various options, including Samsung, Huawei, LG, Oppo, Vivo, and so on. For purchasing a mobile, the individual chose from a list of four companies: Samsung, Huawei, LG, and Oppo. He is having difficulty deciding which company is the best. Let A=η1,η2,η3,η4 represent a collection with some well companies, where η1 = Samsung, η2 = Huawei, η3 = LG and η4 = Oppo.
As a result, we may simply construct the source data in the way of q-LDFS, where MG ad NMG ⟨C,Y⟩ indicate company satisfaction and dissatisfaction, and RPs ⟨a,b⟩ represent "highly best technology" and "not so best technology", respectively. These parameters frequently relate to linguistic phrases and frequently relate to traits or characteristics. We may modify the physical way of such RPs based on our preferences or the needs of the situation. If we modify the way of the RPs, it will be as follows: a = cheap and b = costly, or a = use less electricity and b = use more electricity, and so on. The beauty of these qth power of RPs is that they increase MG and NMG area and enhance the parameterizations range of the problems. The q-LDFS to buy best company mobile of such RPs is represented in Tables 1–3.
4.
Non-linear diophantine fuzzy weighted averaging aggregation (Non-LDFWAA) operator
In [2], Non-LDFWAA operator, Non-LDFOWAA operator, and Non-LDFHWAA operator are mentioned as follows.
4.1. Non-Linear diophantine fuzzy weighted averaging aggregation (Non-LDFWAA) aggregation operator
Let
represent the collection of Non-LDFNs on the set Q and ζ=(ζ1,ζ2,...ζn)q be the weight vector with ∑nδ=1ζδ=1, then the transformation
is also known as the non-linear diophantine fuzzy weighted averaging aggregation (Non-LDFWAA) operator and described as:
4.2. Non-linear diophantine fuzzy ordered weighted averaging aggregation (Non-LDFOWAA) operator
Let
represent the collection of Non-LDFNs on the set Q and ζ=(ζ1,ζ2,...ζn)q be the weight vector with ∑nδ=1ζδ=1, then the transformation
is also known as the non-linear diophantine fuzzy ordered weighted averaging aggregation (Non-LDFOWAA) operator and described as:
where (φ(1),φ(2),φ(3),...,φ(n)) is the arrangement of (δ∈N), for which HDq(φ)(δ=1)≥HDq(φ)∀(δ∈N).
4.3. Non-linear diophantine fuzzy hybrid weighted averaging aggregation (Non-LDFHWAA) operator
Let
represent the collection of Non-LDFNs on the set Q and ζ=(ζ1,ζ2,...ζn)q be the weight vector with ∑nδ=1ζδ=1, then the transformation
is also known as the non-linear diophantine fuzzy hybrid weighted averaging aggregation (Non-LDFHWA) operator and described as:
where H⋄Dq(φ) is the φth biggest weighted Non-LDF values H⋄Dq(i)(H⋄Dq(φ)=H⋄Dq(φ))nζφ,φ∈N)) and ζ=(ζ1,ζ2,...ζn)T be the weight of (H⋄Dq(φ) by means of ζ=1 with ∑nδ=1ζδ=1.
If ζ=(1ζ,1ζ...,1ζ) then the Non-LDFWAA Operator and Non-LDFOWAA Operator are defined particular cases of Non-LDFHWAA Operator. As a result, the Non-LDFHWAA Operator is the generalised form of the Non-LDFWAA Operator and Non-LDFOWAA Operator.
5.
Non-linear diophantine fuzzy weighted geometric aggregation (Non-LDFWGAA) operator
This section introduces the Non-linear diophantine fuzzy weighted geometric aggregation (Non-LDFWGA) Operator, Non-LDFOWGA Operator, and Non-LDFHWGA Operator. We also develop specific scoring functions (SFs) and accuracy functions (AFs) for Non-LDFN comparison analysis in MADM. In [10], established the concept of an IFS scoring function. The definition may be extended to include fuzzy number hybrid framework and Non-LDFNs. We broaden the idea of scoring function introduced by [10,33] by including the qth power of RPs. There are several mappings to determine the value due to the varied approaches of various aggregation methods utilised in the method. We explain multiple score functions in this paper and then compare their findings to determine the operations of Non-LDFNs under the effect of these scoring functions.
5.1. Non-linear diophantine fuzzy weighted geometric aggregation (Non-LDFWGA) operator
Let
represent the collection of Non-LDFNs on the set Q and ζ=(ζ1,ζ2,...ζn)q be the weight vector with ∑nδ=1ζδ=1, then the transformation
is also known as the Non-linear diophantine fuzzy weighted geometric aggregation (Non-LDFWGA) operator and described as:
5.2. Non-linear diophantine fuzzy ordered weighted geometric aggregation (Non-LDFOWGA) operator
Let
represent the collection of Non-LDFNs on the reference set Q and ζ=(ζ1,ζ2,...ζn)q be the weight vector with ∑nδ=1ζδ=1, then the transformation
is also known as the non-linear diophantine fuzzy ordered weighted geometric aggregation (Non-LDFOWGA) operator and described as:
where (φ(1),φ(2),φ(3),...,φ(n)) is the arrangement of (δ∈N), for which HDq(φ)(δ=1)≥HDq(φ)∀(δ∈N).
5.3. Non-linear diophantine fuzzy hybrid weighted geometric aggregation (Non-LDFHWGA) operator
Let
represent the collection of Non-LDFNs on the set Q and ζ=(ζ1,ζ2,...ζn)q be the weight vector with ∑nδ=1ζδ=1, then the transformation
is also known as the non-linear diophantine fuzzy hybrid weighted geometric aggregation (Non-LDFHWGA) operator and described as:
where H⋄Dq(φ) is the φth biggest weighted Non-LDF values H⋄Dq(i)(H⋄Dq(φ)=H⋄Dq(φ))nζφ,φ∈N)) and ζ=(ζ1,ζ2,...ζn)T be the weight of (H⋄Dq(φ) by means of ζ=1 with ∑nδ=1ζδ=1.
If ζ=(1ζ,1ζ...,1ζ) then the Non-LDFWGA Operator and Non-LDFOWGA Operator are defined particular cases of Non-LDFHWGA Operator. As a result, the Non-LDFHWGA Operator is the generalised form of the Non-LDFWGA Operator and Non-LDFOWGA Operator.
5.4. Score function (SF)
In [2], let HDq=(⟨CDq,YDq⟩,⟨a,b⟩) be a Non-LDFN, then scoring function (SF) on HDq can be defined by the mapping B:Non−LDFN(Q)→[−1,1] and is stated as follows:
where Non-LDFN(Q) is the collection of Non-LDFNs on Q.
5.5. Accuracy function (AF)
In [2], the accuracy function (AF) can be described by the mapping ψ:Non−LDFN(Q)→[0,1] and is stated as follows:
5.6. Quadratic score function (QSF)
The quadratic score function (QSF) for Non-LDFN is a mapping κ:Non−LDFN(Q)→[−1,1] and is stated as follows:
5.7. Quadratic accuracy function (QAF)
The quadratic accuracy function (QAF) for Non-LDFN is a mapping τ:Non−LDFN(M)→[0,1] and is stated as follows:
5.8. Expectation score function (ESF)
Let HDq=(⟨CDq,YDq⟩,⟨a,b⟩) be a Non-LDFN, then expectation score function (ESF) on JDq can be defined by the transformation ϑ:Non−LDFN(Q)→[0,1] and is stated as follows:
Besides [-1, 1], the ESF elements are limited by [0, 1]. The ESF is a broader definition of SF.
5.9. Non-linear diophantine fuzzy normalized hamming distance (Non-LDFNHD)
Let HD1=(⟨CD1,YD1⟩,⟨a1,b1⟩) and JD2=(⟨CD2,YD2⟩,⟨a2,b2⟩) be two Non-LDFNs, then we obtain the Non-linear diophantine fuzzy normalized hamming distance (Non-LDFNHD) as given by the equation
6.
Modal for MADM using MABAC method Non-LDF information
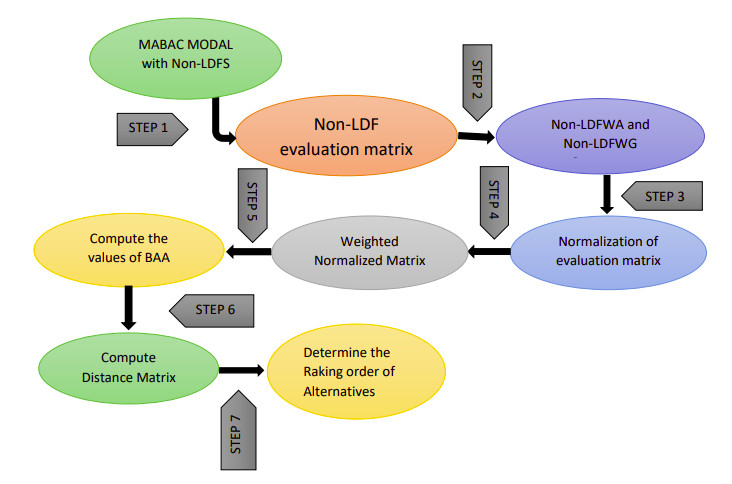
Throughout this section, we provide a novel strategy to MADM in the Non-LDFS environment, which includes the Non-LDFWAA, Non-LDFOWAA, and Non-LDFHWAA operator, as well as Non-LDFWGA, Non-LDFOWGA, and Non-LDFHWGA operator. As a result, our proposed method for the same numerical model, obtain preventative and control rankings for the Covid-19 outbreak utilizing various forms of SFs.
6.1. The conventional MABAC model
In [34], consider there are u alternatives {ˆZ1,ˆZ2,...ˆZu} v attributes/criteria {ˆG1,ˆG2,....ˆGv} with weighting vector be ζh(1,2,...v) and experts {e1,e2,...eλ} with weighting vector be {ζ1,ζ2,...ζλ}, then the decision-making process of the conventional MABAC model are as follows.
Step 1. Establish the evaluation matrix
as below
where
denotes the assessment data of options ˆAf(f=1,2,...,u) on criteria ˆGh=(h=1,2,...,v) by experts dλ.
Step 2. we can use general ˆAλfh to ˆAfh, according to several aggregation operators.
Step 3. Using the given formula, normalized the matrix
depending on the type of each criteria.
For benefit criteria:
For cost criteria:
Step 4. According to the normalized matrix ˆNfh(f=1,2,...,u,h=1,2,...v) and the weights of criteria ζh(h=1,2,...v) the weighted normalized matrix ˆWˆNfh,f=1,2,...,u,h=1,2,...,v can be calculated as:
(f=1,2,...,u,h=1,2,...,v).
Step 5. Compute the values of border approximation area (BAA) and the BAA matrix ˆG=[ˊgh]1×v can be established in the following
(f=1,2,...,u,h=1,2,...,v).
Step 6. Determine the distance D=[dfh]u×v between alternatives and the BAA using the given formula
where d(ˆW,ˊgh) means the distance d from ˆW to ˊgh. According to the values ofdfh, we can get:
(1) If dfh is greater than zero, the alternatives correspond to the upper approximation area ˆG+(UAA).
(2) If dfh is equal to zero, the alternatives correspond to the upper approximation area ˆG(UAA).
(3) If dfh is less than zero, the alternatives correspond to the upper approximation area ˆG−(UAA).
Therefore the greatest alternative are shown in ˆG+(UAA) and the lowest alternative are shown in ˆG−(LAA).
Step 7. By using the given formula, add the values of each alternative's dfh.
The ranking of all options can be evaluated from the thorough assessment outcome ˘Sf obviously, the higher the thorough assessment outcome ˘Sf, the better the option.
6.2. The MABAC model with Non-LDFNs
Despite the fact that the IFS and PFS have been employed in various domains of decision making difficulties, there are certain particular circumstances that the IFS and PFS cannot handle. For example, if the fuzzy data provided by experts is presented as (0.8, 0.7), indicating that the MG is 0.8 and NMG is 0.7, both IFS and PFS cannot fulfil this condition. Because it is an extension of the two fuzzy sets, Non-LDFS is more efficient and logical in solving more unstable and unpredictable issues. Based on the conventional MABAC technique and the Non-linear diophantine fuzzy data, we may develop the Non-linear diophantine fuzzy MABAC model, in which all assessment data is represented by Non-LDFNs. Consider there are u alternatives {ˆZ1,ˆZ2,...ˆZu} attributes {ˆG1,ˆG2,....ˆGv} with weighting vector be ζh(1,2,...v) and experts {e1,e2,...eλ} with weighting vector be {ζ1,ζ2,...ζλ} given the q-linear diophantine fuzzy evaluation matrix ˆR=[ˆAλfh]u×v=(⟨CλD,YλD⟩,⟨aλ,bλ⟩) indicated the MG and NMG, then the decision making steps of Non-linear diophantine fuzzy MABAC approach can be given below.
Step 1. construct the Non-linear diophantine fuzzy evaluation matrix
as below
where [ˆAλfh]u×v=(⟨CλD,YλD⟩,⟨aλ,bλ⟩) indicates the Non-linear diophantine fuzzy data of alternative ˆAf(f=1,2,...,u) on attribute ˆGh=(h=1,2,...,v) by experts dλ.
Step 2. We may use general ˆAλfh to ˆAfh according to the Non-LDFWA or Non-LDFWG aggregation operator, the fused Non-LDFNs matrix r=[ˆAfh]u×v shown as below:
where [ˆAfh]u×v=(⟨CD,YD⟩,⟨a,b⟩) represents the fused Non- linear diophantine fuzzy data of alternative ˆAf(f=1,2,...,u) on attribute ˆGh=(h=1,2,...,v).
Step 3. Using the given formula, normalized the matrix
depending on the type of each criteria.
For benefit criteria:
f = 1, 2, ..., u, h = 1, 2, ..., v.
For cost criteria:
f = 1, 2, ..., u, h = 1, 2, ..., v. If all attributes are benefit in this scenario, we do not need to normalize. In our provided situation, all choices and criteria are advantageous.
Step 4. Calculate the weighted matrix ˆW. The criterion weighting vector is denoted as ζh(h=1,2,...,v), and the weighted evaluating matrix
may be computed as,
(f = 1, 2, ..., u, h = 1, 2, ..., v).
Step 5. Determine the values of border approximation area (BAA) and the BAA matrix ˆG=[ˊgh]1×v can be established in the following:
Step 6. Determine the distance D=[dfh]u×v between alternatives and the BAA using the given formula
where d(ˆW,ˊgh) means the distance d from ˆW to ˊgh, which may be computed using Eq (5.9).
Step 7. By using the given formula, add the values of each alternative's dfh.
The ranking of all options can be evaluated from the thorough assessment outcome ˘Sf obviously, the higher the thorough assessment outcome ˘Sf, the better the option.
7.
Case study
To highlight the effectiveness of the suggested approach, this part explains a specific scenario of emergency decision making in public health. To select the optimal criteria under q-LDF data, we offer a unique Covid-19 infectious disease solution [6,9,38,39,40,41,48]. The world, s papulation has incurred heavy casualties as a result of the recent outbreak of emergencies. Whenever an incident occurs, rapid monitoring and execution of one of the emergency alternatives is essential. Depending on the findings of the preceding study, a q-LDF contingency decision making framework is used to describe the Covid-19 emergency decision making process. In addition, research into coronavirus diagnosis and treatment is being conducted in order to resist pandemic spread.
Discuss the problems: The new corona virus compelled the Chinese government to impose the world's biggest quarantine order at the start of 2020, impacting around 45 millions of people. The virus has been provisionally designated as the new coronavirus of 2019. (2019nCoV). In December 2019, the coronavirus syndrome caused by the Covid-19 viral infection was discovered for the first time in Wuhan, China. The recent pandemic was proclaimed a worldwide a public-health crisis by the Director General of the WHO (World Health Organization) on 30th January, 2020. More than 1,518,614 occurrences (Covid-19 Worldwide Occurrences (JHU) 2020) were reported in 209 countries / territories on 8th April, 2020, including 48,079 (Covid-19 Worldwide Occurrences (JHU) 2020) defined as severe. At least 88,480 (Covid-19 Worldwide Emergencies (JHU) 2020) fatalities have been ascribed to the virus, with the global active in mainland China, Italy, Iran, the United States, the United Kingdom, and other countries, with over 1,000 people dying in many of these countries. More than 330,357 patients have saved (2020 worldwide Covid-19 (JHU) occurrences). The danger of continued rise is extremely large. Without a doubt, this sickness has resulted in tremendous financial loss, ecological pollution, and insufficient PPE (personal protective equipment); PPE contains sunglasses, health care face mask, rubber gloves, or other safety. Furthermore, there is insufficient capability to grow PPE manufacturing, and world production for breathing and masks cannot be satisfied, particularly if PPE is widely used and misused. WHO collaborates with worldwide expert networks and organizations in the areas of labs, preventive medicine, care services, and computational analysis.
In such cases, strong emergency procedures are required to avoid further deaths and preserve people. In both healthcare and social contexts, preventative and controlling actions are essential. Here's how we're putting our novel Non-LDFS system to work for the DM. We present a way for a decision maker to choose the optimum solution with Non-LDF data depending on the Non-LDFWAA and Non-LDFWGA operators. Table 4 summarises the many options to prevent and control the Corona virus.
To reduce the overall danger of this sickness, there are five main public-health crises aspects to be considered. The foregoing are the most appropriate society safety precautions:
ˆG1: Clinical management: Vaccination is a very effective way to prevent some infectious diseases once the virus has spread. Vaccines are usually very safe and severe negative impacts are uncommon. At the moment, there are no Covid-19-specific effective medicines. Clinical management consists adopting suggested infection prevention and control measures as quickly as feasible, as well as medical intervention for complications, including advanced organ function if necessary.
ˆG2: Improved personal protective equipment or first-aid training: Because the sickness grows fast, the effective way to reduce it is to prevent persons who have been trained or who have indications of this infection. To understanding what to do in an emergency situations, it is actually recommended that individuals take a properly regulated online first-aid treatment. The lack of test kits is the current problem. Rising production of test kits, a shortage of verification requirements, and the local government's decision to warn and, ultimately, isolating all infected patients will all help to improve the situation. Each area will receive masks, gloves, respirators, and gowns. Face masks offer minimal protection against the spread of the disease. As a result, basic personal care is the most effective strategy to avoid the spread. Consequently, labelling worldwide PPE is a significant challenge.
ˆG3: Trained technician: Because the virus's genetic composition is rapidly spreading, the rest of the world will start to develop specific screenings and work on possible vaccinations.
ˆG4: Banned intra city transportation: The virus-caused illness is dangerous. The local government must take steps for the safety of the locals or declare that inter-city travel is restricted to force patients to go to the nearest society clinic. As a result, all aircrafts and subway service from and to Wuhan were suspended, and so have their lunar New Year festivities, and everyone between you and somebody coughing or sneezing must maintain a distance of at least 1 meter (3 feet).
ˆG5: Global uncertainty: The economic consequences of the Corona virus: In the first quarter, the dramatic fall in transport facilities and tourism will have an impact on the global economy, as well as consumer and commerce. It is likely to have a substantial effect on the overall economy of the country. It has global consequences, not just for China. The nation's economy is dependent on China's expansion. The corona virus has had a detrimental effect on global oil demand. The closure of the plant is delaying the procurement of goods and parts from China, which is having an impact on companies around the world, including Apple and Nissan.
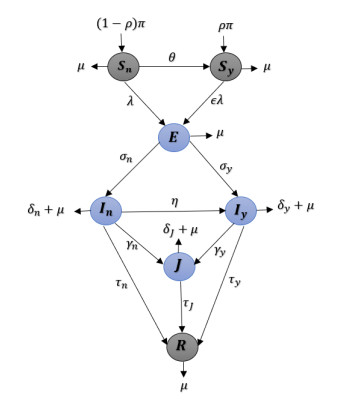
Figure 4 represented the flow chart of the proposed method. We now construct the data input in the Non-LDFS structure, where positively and negatively membership functions inform us about the level of satisfaction of ideal attributes, and reference parameters symbolise the "most critical public health emergency service" and "less critical public health emergency management". We may now gather information on the critical aspects in determining emergency management demands for public health using Non-LDFSs.
7.1. Illustrative example
This section contains a real-world decision making application that use the Non-linear diophantine fuzzy MABAC model to choose the optimal option for Corona virus prevention and control. Consider there are four emergency alternatives, each of which is denoted by ˆZf=(f=1,2,3,4). Three medical authorities, eλ(λ=1,2,3), who manage the entire public health crisis management program, are assigned to evaluate emergency options based on five factors/criteria of ˆGh(h=1,2,3,4,5). Let ζ=(ζ1,ζ2,...,ζv) be the weight of the attribute/criteria ζδ(δ=1,2,...,v) such that ζδ>0 with ∑vδ=1wδ=1. The suggested method addresses the challenges associated with emergency decision-making in order to obtain the most effective option. Using Non-LDFSs, we can now gather information on the critical aspects of assessing emergency management objectives for population health. Assume the expert has a weighted vector of (0.4,0.35,0.25) and the attribute has a weighting vector of (0.32,0.27,0.17,0.14,0.1). In Tables 5–7, we select three medical authorities to handle the whole crisis/disaster assessment process, and then we have three decision maker for the source data [2].
Step 1. Given the Non-linear diophantine fuzzy evaluation matrix
as given in Tables 5–7.
Step 2. we can use genareal ˆAλfh to ˆAfh according to the Non-LDFWA or Non-LDFWG aggregation operator, the fused Non-LDFNs matrix r=[ˆAfh]u×v where
We may obtain calculating outcome, therefore all of them are shown in Table 8.
Step 3. There is no need to normalise in this instance because all attributes are benefit.
Step 4. Calculate the weighted matrix ˆW. The criterion weight vector is represented by ζ=(ζ1,ζ2,,ζv) and ∑vh=1wh=1 we can obtain the weighted evaluating matrix
where
We may obtain computing outcomes, therefore all of them are shown in Table 9.
Step 5. Determine the BAA matrix ˆG=[ˊgh]1×v, where
compute the values of BAA can be constructed as shown in Table 10.
Step 6. Using the values of Tables 9 and 10 in Eqs (5.4) and (6.13) to find the score values as well as the distance matrix D=[dfh]u×v between the options and the BAA, the obtained results are given in Tables 11 and 12 respectively.
Step 7. By using the given formula, add the values of each alternative's dfh.
As a result, we obtain different computation outcomes. Table 13 lists all of the outcomes. Using Non-LDFWA, Non-LDFOWA, Non-LDFHWA, Non-LDFWG, Non-LDFOWG, and Non-LDFHWG operators to find the final ranking of outcomes given in Tables 14 and 15, the obtained results are displayed in Tables 16 and 17.
8.
Discussion and comparison analysis
This part compares the proposed MABAC approach to existing operators presented in [31], demonstrating their ability to address real-life decision-making issues (DMIs) with uncertainties. Because of the qth power of RPs, this idea covers the assessment spaces of IFS, PFS, q-ROFS, and LDFS. Tables 18 and 19 show the ranking results of four options based on existing techniques and suggested concepts. Table 19 shows that the proposed method's medical authorities' opinion on the control and prevention of the Corona virus is the same as existing methods, that is descriptive in and of itself and verifies the validity and reliability of the proposed technique, as well as demonstrating that medical assistance is needed in the preventative measures of virus infection (Covid-19). To avoid the transmission of the Corona virus, people should follow doctor's advice, such as wearing a surgical mask at all times. The proposed technique and the existing technique yield somewhat different ranking outcomes, but the optimal and first options are the same in both ways. Tables 19 and 20 illustrate the comparing findings. Now we'll discuss about the accuracy and adaptability of the developed technique for dealing with various two signals.
8.1. Validity and simplicity of the proposed technique
To avoid the transmission of the Corona virus, people should follow their doctor's advice, such as wearing a surgical mask at all times. The proposed technique and the existing technique yield somewhat different ranking outcomes, but the optimal and first options are the same in both ways. Tables 19 and 20 illustrate the compared findings. Now we'll discuss the accuracy and adaptability of the developed technique for dealing with various signals.
8.2. The Influence of score function
We generalised and then implemented the previously presented three kinds of scoring functions, SF, QSF, and ESF, as well as related accuracy functions (AFs). Allow for a slightly different effect because each SF has its own observations and sorting techniques. Tables 19 and 20 show that the rankings for the SF, ESF, and QSF varied significantly from one another. It is worth noting, however, that the final result for all score functions is nearly the same when the MABAC approach is used.
8.3. Flexibility of aggregation with different inputs and outputs
Because the qth power of RPs increases the level area and varies depending on the scenario in MADM methods, this approach is more flexible than others. It may also be used for a number of different signal conditions.
8.4. Sensitivity analysis
The sensitivity analysis of the proposed strategies can be seen in Tables 16 and 17. Differences in score functions cause changes in alternative rankings, but the optimal result remains the same. As a result, it is clear that the MABAC approach is simply sensitive to the score's functions.
8.5. Superiority and comparison of proposed method with existing approaches
Because q-LDFS handles qth parameterizations, it takes up a lot of space when compared to IFS, PFS, q-ROFS, and LDFS. In [31], established LDFSs with RP additions, although LDFSs have certain limitations on reference parameters and cannot handle qth parameterizations. To fill this knowledge gap, we demonstrated the concept of LDFSs and proposed q-LDFSs. The suggested approach and MADM difficulties are inextricably linked. In this technique, the qth power of RPs is essential.
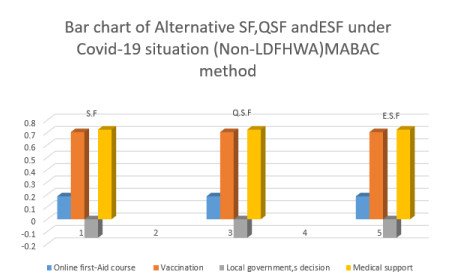
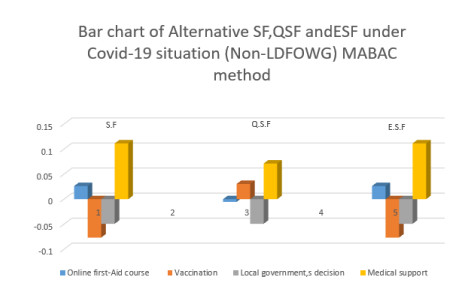
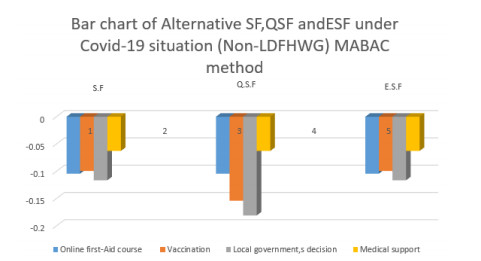
Table 21 illustrates the ranking of corona virus preventive and control approaches. The Bar chart of score, Quadratic and Expectation score functions values based on Non-LDFWA, Non-LDFOWA, Non-LDFHWA, Non-LDFWG, Non-LDFOWG and Non-LDFHWG aggregation operators is given in Table 18, Figures 5–10 respectively.
9.
Conclusions
With rising human influence and tremendous economic growth in today's world, emergency incidents have become more common, presenting a direct threat to people's lives and property. Emergency decision making plays an important role in reducing fatalities and has aroused the public's attention. In [31], created the notion of LDFS in order to remove such restrictions, in which they added the role of RPs, that fulfill the conditions 0≤aCD(η)+bYD(η)≤1 ∀ς∈δ with 0≤a+b≤1 However, the total of the RPs values provided by DM may be greater than one, i.e. a+b>1, that contradicts the LDFS requirements. As a result, LDFS failed to fulfill his RPs goal. It limits the MADM's options and influences the best decision. To resolve this contradictions, we offer and describe the innovative notion of the q-LDFS, that is able to deal with these scenarios. We developed the q-LDF emergency DM problem in this manuscript based on the present pandemic of Covid-19. The provided method assisted us in determining how to avoid Covid-19. FS extensions that have been studied include IFSs, PFSs, q-ROFSs, and LDFSs. We created q-LDFS, an innovative version of FS which is more effective and versatile in dealing with uncertainty. In the presence of uncertainty, the concept of the qth power of RPs can provide a very reliable and efficient structure for fuzzy system modelling and decision making. We presented Non-LDFS' geometric and averaging features in order to compare it to other established FSs extensions. We used multiple SFs and AFs to compare Non-LDFNs, then extended these functions to get an additional scoring function. The concept of Non-LDFS has been extended to Non-LDFWAA and Non-LDFWGAA operator. In addition, the decision processes described in the research are easily applicable to real-life group decision making situations involving both Non-LDFWAA and Non-LDFWGA information. We offered an application of the recommended MADM approaches for the control and prevention of corona virus using a case study. Finally, we compared the suggested method to some other methods that were already in use. The results obtained demonstrated the advantages and usability of the suggested approach.
We will extend this work in the future to other generalised theories of fuzzy environments, such as hesitant fuzzy sets [23], Extended cumulative residual entropy [24], probabilistic hesitant fuzzy [22], and so on. Also, the suggested method will be used to address practical MAGDM problems such as technology advancements, uncertain decision-making, program installation, and site analysis, and etc.
Conflict of interest
The authors declare that they have no conflicts of interest.










 DownLoad:
DownLoad: