1.
Introduction
In the past few decades, the demand for lithium-ion batteries (LIBs) has increased sharply. According to a recent analysis [1], the global LIB market size is expected to grow at a compound annual growth rate (CAGR) of 13.0% and reach USD 87.5 billion by 2027. The market growth can be attributed to the growing demand for portable consumer electronics and electric vehicles (EVs). Due to their lightweight, high energy density, long cycle, and low self-discharge rate, LIBs have been used extensively in consumer electronics such as smartphones, laptops, and digital cameras. In 2020, consumer electronics was the most dominant LIB market segment with a 36.1% global market share [2]. After consumer electronics, the automotive industry is the most revenue-generating segment in the global LIB market. Globally, the automotive segment accounted for 17.6% of the total revenue in 2019 [1]. However, the demand for LIBs in the automotive industry is likely to surge in the next few decades due to recent movements against climate change. New environmental regulations and government subsidies have spurred restructuring of the technology and manufacturing processes employed by the auto industry with the aim of accommodating EV manufacturing. In January 2021, General Motors, a giant automaker company in the United States, announced that the company would sell only zero-emission vehicles by 2035. Other large automakers like Ford are spending billions of dollars on introducing battery driven EVs to the market [3]. LIBs are currently the dominant battery technology for EVs [4], and are predicted to remain so for the foreseeable future. Due to the significant market demand, LIB production is increasing at a phenomenal rate. The global LIB production capacity is expected to grow from 455 GWh in 2020 to 1447 GWh in 2025, at a CAGR of 26%. However, as of 2020, China alone accounts for 77% of total LIB production capacity [5]. The near-monopolistic supply creates potential supply disruption risks and vulnerability to the rest of the world. Moreover, an upscaling of LIB production will also increase the burden on the geological reserves of metals (such as cobalt, lithium, manganese, and nickel) found in a LIB [6]. A study by Yan et al. [7] indicated potential supply risk and shortages of lithium in the 2030-time frame. The same has been predicted for cobalt and nickel [8]. However, value recovery from spent LIBs is a promising strategy for reducing the gap between supply and demand for LIB materials. A lifespan of approximately ten years is expected for LIBs in automotive applications [9]. Globally, 700,000 metric tons of LIBs are expected to reach the end of their useful life by the end of 2025 [10]. Recovering valuable metals from numerous spent batteries can be an essential measure in mitigating future supply risks and reducing the consumption rate of geological reserves.
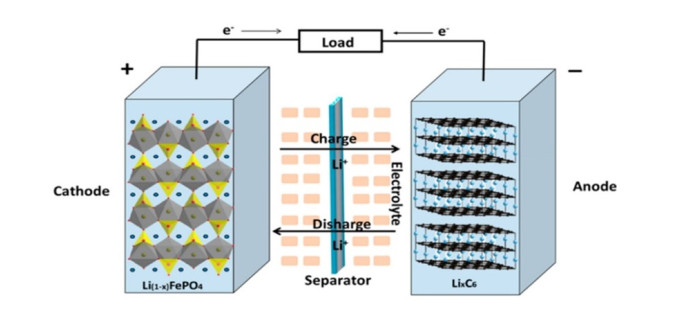
A LIB is composed of four main components: cathode (positive electrode), anode (negative electrode), separator, and electrolyte (Figure 1). The cathode is composed of aluminum foil coated with a metal oxide layer. Similarly, the anode is composed primarily of graphite, binder, and additives coated on copper foil. These two electrodes are isolated by a separator, which consists of microporous polymeric materials. The separator is moistened with electrolytes, allowing the flow of lithium ions between the cathode and anode. The electrolyte is a lithium salt in the organic solvent, which is harmful to the environment. If the organic electrolyte and heavy metals in LIBs are exposed to the environment without suitable treatment, they may cause serious soil and land pollution [12]. In contrast, the cathode material in a LIB is rich in valuable metals, including critical materials such as cobalt, lithium, manganese, and nickel. The intensity of these raw materials varies depending on the cathode chemistry. Table 1 provides a brief overview of some popular chemistries that are used to develop cathode materials. According to a Roskill report [13], LCO type batteries dominate the current end-of-life (EOL) LIB market due to their long-standing applications in portable electronics. The forecast suggests that the share of LCO batteries will remain almost constant in the future, while the NMC chemistry will continue to increase in share due to its dominant applications in the EV industry. LFP is projected to be the most common cathode material reaching the end of life after 2024.
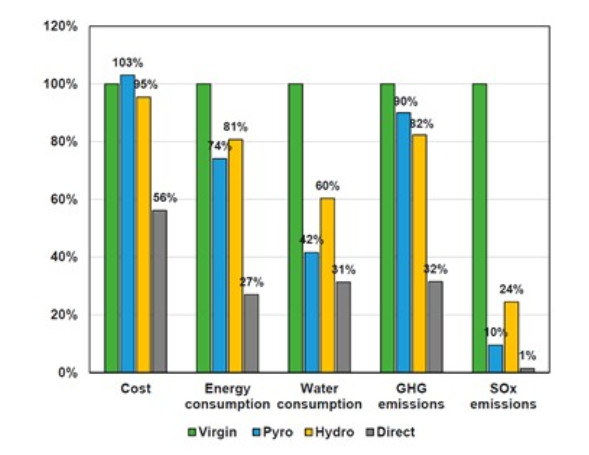
LIB cathodes have been the major target for recycling due to their critical material content and high economic value. A LIB cathode contains lithium, cobalt, manganese, and nickel, depending on the cathode type, as shown in Table 1. Proper recovery of these valuable materials could enhance environmental sustainability by reducing energy consumption, water consumption, and SOx emissions by 82%, 77%, and 91%, respectively, compared with the virgin-production levels of these critical materials [21,22]. Moreover, cathodes constitute the highest cost of EV LIB cells (i.e., 42–50% [22]), representing an economic incentive to recycle [23].
The state-of-the-art recycling technologies can be classified into three main categories: direct recycling, pyrometallurgy, and hydrometallurgy. Direct recycling is the recovery, regeneration, and reuse of battery components directly without breaking down the chemical structure. Consequently, the recovered materials are reusable with minimum treatment. The pyrometallurgy technique uses high temperatures to reduce the oxides present in the cathode material to their metallic form. A mixed alloy of metals is generated by the pyrometallurgical process, and the metals can be separated through hydrometallurgical refining. The hydrometallurgy technique uses mineral or organic acids to dissolve the metal ions from the cathode material into an aqueous solution. Different separation techniques such as solvent extraction, chemical precipitation, and ion exchange are used to purify the individual metals from the leachate solution [24].
However, recycling is not the only available for a spent LIB. If a battery is in good shape and requires no further treatment, then this battery may be suitable for reuse. In some cases, reuse is impossible due to the loss in the capacity below a specific threshold. For example, a spent LIB collected from an EV may not be reused if it loses more than 20% of its capacity after the first use [25]. In those scenarios, the EOL battery may be used in other applications outside the automotive industry, but may be subjected to a refurbishment process before its second use. Recycling should be the last option, as it requires extensive chemicals and/or energy to recover value at the materials level, which increases the cost and environmental burden. Therefore, a spent LIB should be carefully inspected to identify the most favorable recovery option.
The number of publications in the field of spent LIB recovery has grown steadily in recent years. As the battery chemistry and relevant technologies are changing rapidly, this paper reviews recent articles (published between 2014 and 2021) and older articles are excluded from this investigation. Section 2 shows a detailed review of different LIB recovery technologies, their commercial applications, and the advantages and disadvantages of each method. Section 3 shows the economic and environmental impacts of these technologies and the associated reverse supply chain optimization strategies for maximizing the economic and environmental benefits. In addition, section 4 summarizes the key challenges and future work for sustainable value recovery from spent LIBs.
2.
Value recovery technologies
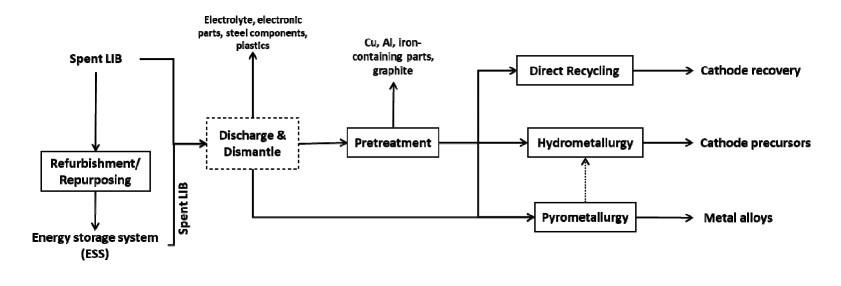
Repurposing, direct recycling, pyrometallurgical processing, and hydrometallurgical processing are the most common methods of LIB recovery. Figure 2 shows a general flowsheet of these four LIB recovery pathways. To avoid the risk of fire and explosion, spent LIBs are discharged, as cutting cell cases may result in self-ignition due to internal short circuits (cathode and anode come into contact) [26]. Dismantling and skinning may occur, followed by crushing the components into smaller pieces for materials recycling [27,28]. Afterward, direct recycling, pyrometallurgy, and/or hydrometallurgy are employed for recycling of the spent LIBs.
2.1. Refurbishment and repurposing
LIBs from EOL EVs may retain 70–80% of the original capacity and can be directly reused in EVs (i.e., refurbishment) or repurposed for grid energy storage and other niche applications for an additional 10 years before eventually being recycled [29,30,31,32]. Therefore, spent LIBs should be tested for their state of health to determine reusability. Refurbishment operations include testing, disassembly, diagnosis on different product/component levels (i.e., pack, module, or cell), reconfiguration based on the second-life application, and reassembly.
Disassembly Planning is an essential step in the recovery process of spent LIBs. The EverBatt model developed by Argonne National Laboratory estimated that disassembly could constitute the largest cost in LIB recycling [20,33]. The battery system can be disassembled up to battery cells for reuse or recycling. Figure 3 shows the general structure of a LIB pack.
Due to the high costs of disassembly, disassembly planning has emerged as an essential research topic in recent decades. Alfaro and Ramirez [34] proposed a model for designing the disassembly process of an EV battery pack, which provides an optimal disassembly level a.k.a. "stopping point" as a trade-off decision between no disassembly and complete disassembly. The model also suggests an efficient recovery option (e.g., reuse, remanufacturing, recycling, or disposal) for each disassembled component, ensuring the highest profitability and minimum environmental impact. The result of their study indicated that the profits are highly dependent on the state of the modules. According to the case study performed on an Audi A3 Sportback e-tron Hybrid Li-ion Battery Pack, when all modules are ready for reuse, complete disassembly of the pack will return the highest profit and environmental benefit; otherwise, partial disassembly is recommended with the optimal disassembly level identified based on the number of reusable modules. Gentilini et al. [35] proposed a mathematical model that determines the optimal disassembly sequence for minimizing the exposure time of an operator to hazardous voltages. Their safety-oriented disassembly strategy reduced the hazard risk by more than 50% compared with the time-oriented disassembly model that minimized disassembly time. However, the study only considered the pack-to-modules disassembly phase and ignored more subtle risks relevant to the module-to-cells phase. The model also assumes that the operators are exposed to risks only when performing a task; therefore, no setup times or preparation tasks are included in the model.
It is worth noting that the battery pack design varies significantly depending on the model. Therefore, it is often difficult to conduct a study for LIB disassembly that applies to all models. A few research works tried to provide valuable insight on LIB disassembly based on specific models. Rallo et al. [36] investigated the economics of disassembly operations by analyzing the Smart ForFour Li-ion battery. They found that battery pack level disassembly is more cost effective than module or cell level disassembly and is less time-consuming. However, compared with other disassembly levels, cell-level disassembly increases the chance of recovering more material for second use and could benefit the environment. Wegener et al. [37] identified the disassembly steps for Audi Q5 Hybrid System battery priority matrix and disassembly graph. They also investigated the automation potential of disassembly operations. Complete automation is both expensive and infeasible due to the complexities present in the disassembly process of the battery system. Therefore, Herrmann et al. [38] suggested automating only the risky and unproductive tasks associated with manual disassembly, e.g., the extraction of modules from the system, removing cells from the modules, and loosening the screws and nuts.
Overall, disassembly planning for LIB packs has rarely been investigated. Future research could employ multi-objective models that simultaneously minimize disassembly time, exposure risk, and environmental impact, while maximizing the recovery profit. In addition, real-time planning of the disassembly process can also be studied to account for process uncertainties and the quality of disassembled components. Due to the significant difference in battery pack design, it is challenging to plan a disassembly process that works for all the battery models. The cost and time to disassemble a battery pack also vary depending on the model, which are important input parameters to the optimal disassembly planning models. Therefore, developing inventory databases and classification technologies to quickly identify battery pack design and the constituent materials will help optimize the disassembly process, cost, and time. Examples of such an approach are (1) automated disassembly of hard disk drives (HDDs) by utilizing a database containing disassembly information of different HDD models and scanning the barcodes of HDDs to retrieve disassembly information for rapid fastener removal [39] and (2) application of machine learning and artificial intelligence for municipal solid waste identification, characterization, and sortation [40]. Enhanced labeling requirement (e.g., QR code and/or color coding of batteries) for battery identification and their removability from equipment, as was proposed in the new EU Battery Regulation, could also help [41].
2.2. Materials recycling
For LIBs not reused, mechanical pretreatment such as crushing, magnetic separation, and screening may be applied to obtain cathode containing powder (referred to as black mass) for further recycling. For example, Zheng et al. [42] used wind sieving to recover separators by exploiting the weight difference between electrode materials and battery shells. Rothermel et al. [28] utilized magnetic separation to recover iron-containing parts after size reduction. To recover graphite, they proposed a thermal treatment followed by mechanical separation using an air-jet. Liang et al. [43] stirred anode powder collected from the dismantling phase in absolute ethanol and centrifuged this powder repeatedly prior to thermal treatment to recover graphite. After the pretreatment process, direct recycling, hydrometallurgical processes, and pyrometallurgical processes could be employed to recover valuable materials from spent LIBs.
2.2.1. Direct recycling
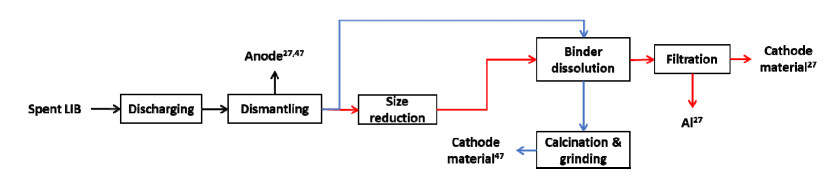
Direct recycling aims to separate cathode materials from black mass without changing the cathode crystal structure [17]. Indeed, the primary distinction between direct recycling (also known as cathode-to-cathode recycling) and pyro-and hydro-processes is the ability to recover the cathode intact, i.e., without decomposition to its constituent materials [44]. The recovered cathode is directly reusable if sufficient capacity is retained or minimal additional treatment is performed [45]. For example, Zhang et al. [27] used trifluoroacetic acid to dissolve organic binder and detach cathode materials from aluminum foil (Eq 1). Figure 4 summarizes the cathode material recovery process shown in the papers mentioned above.
For a more detailed review on direct recycling methods (than that presented here), please refer to the paper by Ji et al. [46] published in the same Journal of Clean Technologies and Recycling.
2.2.2. Pyrometallurgy
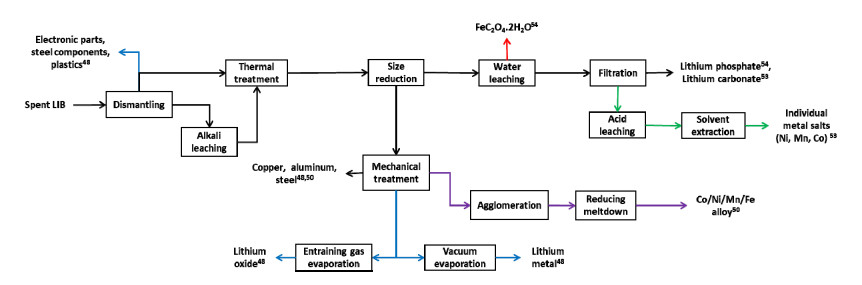
Pyrometallurgical recycling employs high-temperature thermal treatments, which can discharge battery cells, remove flammable electrolytes, decompose organics, and remove the separator layer [48]. Industries also use low-temperature thermal treatment (<500℃) for removing binder and electrolyte without affecting the recovery of cathode and anode active materials [49]. For example, Accurec Recycling GmbH applies a low-temperature thermal treatment (250℃) to remove electrolyte, solvent, and hydrocarbons, which is followed by size reduction, a series of mechanical treatments (i.e., vibrating screening, magnetic separation, and zig-zag classification), agglomeration, and a high-temperature thermal treatment (>800℃) for reducing meltdown to recover Co/Ni/Mn/Fe alloy [50,51]. Träger et al. [48] proposed EcoBatRec, a joint mechanical-pyrometallurgical process starting with disassembly of spent LIB packs into modules. The pyrometallurgical process was used to discharge batteries and remove flammable electrolytes and the separator layer. Deactivated cells were subjected to a series of mechanical processes including crushing, shredding, and sieving to remove aluminum, copper, and steel from the electrode powder. The concentrated electrode powder was either directly vacuum evaporated to recover metallic lithium or entraining gas evaporated to recover lithium oxide.
Due to high energy consumption and the emission of hazardous gases, the typical pyrometallurgical process may be unsuitable for handling the growing number of spent LIBs in the future [11,52]. As a result, several studies have attempted to combine this technique with a hydrometallurgical process in order to boost efficiency and minimize environmental impact. Hu et al. [53] recovered lithium carbonate and other individual metal salts through a combined pyro- and hydro-process. Black mass was alkali leached to remove aluminum and then a three-hour thermal treatment was performed at 650℃. Carbonated water leaching was carried out to transform lithium carbonate into more soluble lithium bicarbonate (Eq 2), and the pH of the solution was adjusted to 3.5 to remove iron. The solid and liquid were then separated via filtering. Lithium carbonate was recovered from the liquid solution through evaporative crystallization at 100℃ for 0.5 h (Eq 3), whereas the solid residue was acid leached and solvent extracted to recover nickel, cobalt, and manganese.
Another study by Fan et al. [54] discussed the effect of wet mechanochemical treatment applied to LFP cathode materials. Spent LIBs were discharged, dismantled, and alkali leached for aluminum removal. After drying and calcination of the residue at 700℃ for 5 h to remove organic impurities, the feedstock was wet ball-milled with oxalic acid to reduce the particle size and solubilize lithium as shown in Eq 4. The materials were soaked in water, and the pH was adjusted to 4 to further remove iron. Lithium phosphate was precipitated after further pH adjustment to 8, filtration, and drying. Figure 5 shows a flowsheet of the aforementioned pyro-processes.
2.2.3. Hydrometallurgy
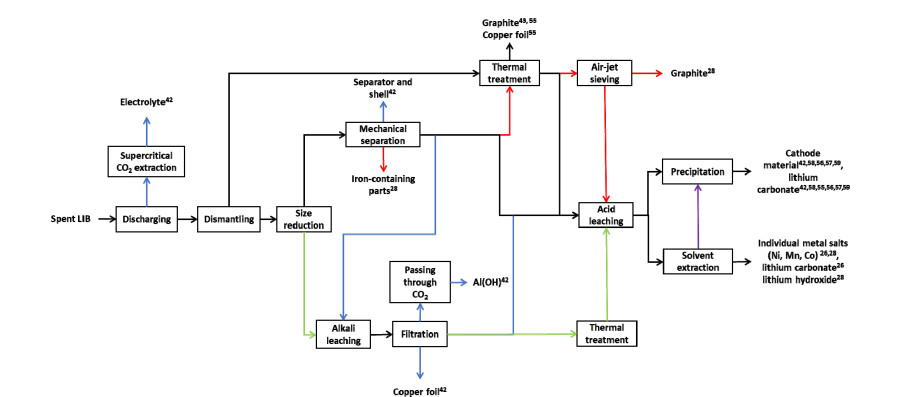
Hydrometallurgical recycling has been widely used on an industrial scale throughout the world. This process has several advantages over pyro-processes, including lower energy consumption and potentially smaller environmental footprint [11]. Generally, a hydrometallurgical process begins in one of two possible ways. In the first route, materials are subjected to the previously described pyrometallurgical processes, and the resulting slag is leached and further processed for value recovery. Yang et al. [55] obtained anode current collectors in the dismantling step. They separated graphite from copper foils using a thermal treatment and then leached the materials to recover lithium through sodium carbonate precipitation. Wang et al. [56] performed deep dismantling to extract cathode materials after discharging. After washing and drying the spent cathode materials, thermal treatment was used to decompose the organic binder. The obtained materials were leached in acid and precipitated to recover cathode materials. Yang et al. [57] retrieved cathode materials from spent LIBs by discharging, dismantling, and thermally treating for aluminum foil separation. Sulfuric acid was used to leach the spent cathode materials in the presence of hydrogen peroxide. Impurities were precipitated out from the leachate by adjusting the pH to 4.8 using sodium hydroxide and further removed via solvent extraction. After solvent extraction, cathode materials (i.e., Ni, Mn, Co) were co-precipitated, and lithium carbonate was precipitated from raffinate.
The second route involves the direct leaching of cathode materials obtained through mechanical pretreatment. Zheng et al. [42] developed an alkali leaching process by adding NaOH to dismantled and crushed LIBs that contain aluminum (Eq 5), and then filtering the leaching liquor. Passing CO2 into the liquor recovered Al(OH)3 (Eq 6). Moreover, the solid residue from alkali leaching was subjected to sulfuric acid leaching with hydrogen peroxide as a reducing agent that solubilizes Li, Co, Ni, and Mn from mixed cathode materials containing LCO, LMO, and NMC (Eqs 7–9). Ammonium hydroxide precipitation was used to remove iron. In addition, copper extractants and phosphate ester were used to remove copper, iron, and residual aluminum. New copper sulfate, nickel sulfate, and manganese sulfate were then added, and NixCoyMn(1-x-y)(OH)2 was recovered via hydroxide precipitation.
Nayaka et al. [58] dissolved aluminum foil using alkali leaching with NaOH, immediately after size reduction of LCO-type LIBs. Subsequently, the solid materials were heated to burn off the organics including carbon and PVDF. The obtained powder was then leached using nitrilotriacetic acid and adipic acid with ascorbic acid as a reducing agent. Gao et al. [59] recovered lithium carbonate and other separated individual metals from the cathode scrap. They cut the cathode scraps into small pieces, which were then leached with formic acid and hydrogen peroxide, a reducing agent. Hydroxide precipitation was applied to recover Ni, Co, and Mn from the leachate, and the raffinate was pH adjusted and sodium carbonate was added to recover lithium carbonate. Figure 6 shows the overall flowsheet of the hydrometallurgical processes discussed above.
Optimizing LIB leaching through design of experiments. Regarding process optimization, the existing literature focused on optimizing LIB leaching conditions such as acid concentration, solid/liquid (S/L) ratio, temperature, and leaching time to maximize metal extraction efficiency [60]. Design of experiments (DOE) is an efficient technique for identifying the impact of parameters on a desired output. These techniques can also be used for parameter optimization by modeling the relationship between processing parameters and response variables and subsequently predicting pathways for optimum response values. This section reviews articles that applied DOE methods to the optimization of the leaching process employed for spent LIB cathode materials.
Table S1 in the Supplementary shows research studies where mineral acids were used as leaching agents, and the leaching conditions were optimized for maximum metal extraction. Sulfuric acid has been the most widely used leaching agent with the help of hydrogen peroxide as a reducing agent for improving the solubility of cobalt, manganese, and nickel present in the cathode material. However, Ghassa et al. [61] used iron scrap as a reductant for sulfuric acid leaching of mixed cathode materials because of its lower environmental impact than hydrogen peroxide. They found a satisfactory leaching efficiency under the optimal condition (1 mol/L H2SO4, 25 g/L iron scrap, 10 g/L solid to liquid ratio, 150 min of leaching time, and agitation speed of 500 rpm). However, additional downstream process (i.e., adding NaOH) was required to remove iron from the leachate.
Most studies have confined the scope of experiments to leachate production in their DOE, and only a few studies [24,62] have considered downstream processes such as chemical precipitation, flotation, and solvent extraction. Huang et al. [24] used a stepwise recovery process to recover lithium, iron, and manganese after leaching a hybrid powder of LiFePO4 and LiMn2O4 in the presence of HCl and H2O. They used 12 g/L [Hbet][Tf2N] and 9 g/L n-butyl xanthate for separating Fe3+ ions in the first stage. Afterward, 0.35 molL−1 KMnO4 and 0.20 molL−1 Na3PO4 were added to the Mn, Li-enriched solution for sequential recovery of Mn and Li through precipitation. Wang et al. [62] used solvent extraction to recover metals from the leachate. D2EHPA was used to extract copper and manganese, while PC-88A was used to separate nickel and cobalt. Chan et al. [63] studied a closed-loop recycling process of cathode materials, where the materials were regenerated from the leaching solution. The new LIBs containing the regenerated cathode materials yielded similar or even better performance in terms of specific capacity, rate capability, and cycling performance than the initial LIBs.
While mineral acids provide high leaching efficiency, some disadvantages can limit their use in practical applications. First, hazardous gases such as Cl2 and SO3 are produced in the leaching process and negatively affect both the environment and human health. Moreover, wastewater containing strong acid from the leaching process requires further treatment before being disposed of in the environment. These factors have motivated many studies focused on organic acids. The leaching process of spent LIBs using organic acid has been extensively investigated and DOE techniques have been used to optimize the leaching experiment parameters (see Table S2 in the Supplementary). Golmohammadzadeh et al. [60] explored the leaching of an LCO cathode material using four organic acids: citric acid, D-malic acid, oxalic acid, and acetic acid. They found that citric acid was the best leaching reagent, owing to its high H+ ion production rate, which plays a crucial role in recovering lithium and cobalt. Zeng et al. [64] developed a novel recovery process that uses acid leaching for direct separation of cobalt and lithium in an LCO cathode. The authors used 1 M oxalic acid (H2C2O4), which acted as leaching and precipitating reagent. After leaching, the obtained solution contained the precipitated CoC2O4 and soluble Li2C2O4 or LiHC2O4. The solution was filtered to separate CoC2O4 from the lithium-rich solution. NMC is one of the most studied cathode chemistries in the literature. Li et al. [65] proposed closed-loop recycling of an NMC cathode using lactic acid as the leaching and chelating reagent. Li et al. [66] compared the performance of NMC cathode materials synthesized from acetic acid leachate (NCM-Ac) and maleic acid leachate (NCM-Ma). The authors found that NCM-Ma provided higher capacity and better cycling performance than NCM-Ac. Ning et al. [67] used ultrasound-assisted malic acid to leach an NMC cathode, which reduced the viscosity of the solution by increasing the reaction area. Consequently, the metal ions diffused easily, thereby accelerating the reaction rate. In some studies [24,68,69,70], mixed cathode materials were used in the leaching experiments, representing a more practical feedstock (than non-mixed materials) in the absence of battery chemistry classification.
Tables S1 and S2 in the Supplementary show that fractional factorial design is the most popular DOE method applied to the leaching of LIB materials, as it requires the least number of experiments for a given set of design parameters. Factorial or fractional factorial design is used to screen factors and model (with a first-order model) the relationship of independent variables and their interactions to the response variable. A more complex technique referred to as response surface methodology (RSM) aims to optimize the response through a sequential procedure that could employ multiple procedures. These include (fractional) factorial design, steepest ascent (or descent) method, central composite design for a second-order model, and ridge analysis for continuous exploration of the optimal region [71,72]. The existing RSM studies have utilized Minitab Response Surface Optimizer or the Process Optimization function of Design Expert to identify the single 'optimal' condition through a one-step process. In some cases, experimental verification of the response value(s) associated with the optimal condition was lacking.
2.3. Recycling technology comparison
The previous sub-sections discussed three primary methods of recycling spent LIBs—direct recycling, pyrometallurgy, and hydrometallurgy. These technologies require different levels of pre-treatments to reduce impurities for downstream processes and maximize the value recovered from spent LIBs. Disassembly for separating components such as steel and plastics, thereby reducing contamination in the subsequent steps of battery recycling, was required in most studies [47,48,56]. Pretreatments are labor- and chemical-intensive, adding costs to LIB recycling. Direct recycling is capable of recycling all the battery cathode materials including cobalt, nickel, manganese, and lithium, while preserving the cathode morphology. This feature is critical for countries such as the United States that lack a domestic cathode powder manufacturer. However, this process requires the pre-sorting of battery types, and the performance of the recovered cathode materials may be inferior to that of virgin materials. Isolating the electrodes, which is accomplished through the use of organic solvents or thermal decomposition, represents another significant challenge of direct recycling [44]. Solvents present toxicity when released to the atmosphere, and thermal decomposition may release hazardous HF. Pyrometallurgy is the least complex process of the three and can be applied to any battery. The pyrometallurgical process is an energy-intensive process with toxic gas emissions. Although the pyro-process can effectively recover cobalt, nickel, and copper, aluminum and lithium are often lost in the slag [52]. The hydrometallurgical method outperforms the pyrometallurgical method in terms of energy consumption, toxic air emissions, and material recovery; nevertheless, hydro-processes are typically more complex and require additional pretreatment as well as many chemical reagents. Table 2 summarizes the pros and cons of the different aforementioned recycling technologies.
In terms of technology development and adoption trend, pyrometallurgical techniques are generally considered as established processes for metal alloy recovery, whereas hydrometallurgical techniques are emerging for recovery of cathode precursors [50]. The transition is partly due to the higher energy consumption and higher material losses associated with pyrometallurgical processes, whereas the more complex processes of mechanical pretreatment combined with hydrometallurgy are able to recover a broader range of cathode precursor materials and thus more desirable for closing the material loops in the LIB industry [50]. A more recent advancement is direct recycling (e.g., technologies are being developed by OnTo Technology, ReCell Center) which aims to recover cathode materials for direct reuse in LIBs, while reducing the energy and chemical consumptions compared with the pyro- and hydro- processes [17,50]. The direct recycling methods are currently in the early stage of development, mostly based on laboratory scale experiments [17].
Industries have adopted different recovery technologies, pyro-, hydro-, and a hybrid of these two processes. By filing the first patents in 2007, GEM and Brunp are the pioneers of LIB recycling in China [13]. These companies have expanded throughout the country, owing to the steady stream of EOL LIBs, established collection system, and high demand [13]. GEM recycles more than 10% of discarded electronics and scrapped batteries in China, and the recycled cobalt exceeds virgin production from mining in China [75]. Brunp has a processing capacity of 120,000 tons per year and a metal recovery rate of ∼99 percent for nickel, cobalt, and manganese [76]. Both companies employ hydrometallurgical processes and are capable of producing commodities and refined products such as precursors and ternary cathode materials (i.e., cathode materials containing Ni, Co, and Mn). Table 3 lists the names of other prominent recycling companies located outside of China. Due to the large number of companies involved, only those having an annual capacity higher than 1,000 tons are shown. For a more comprehensive list of LIB recycling companies in the North America, readers may refer to the NAATBatt LIB Supply Chain Database [77].
3.
Environmental impacts, economics, policies, and reverse supply chain optimization to support sustainable LIB recovery[17,50]
While spent LIBs can be environmentally hazardous upon disposal [72], valuable materials could be recovered through a reverse supply chain to generate revenue and substitute virgin materials for environmental credits. This section will review literature on life cycle assessment and techno-economic analysis for sustainable LIB recycling. In addition, the relevant policies and reverse logistics optimization that support the technology implementation and commercialization will be discussed.
3.1. Life cycle assessment
Although LIBs are essential to zero-emission vehicles, their production process and EOL terms can impose substantial environmental burdens. Cobalt production is particularly challenging, as Congo supplies ∼70% of the global cobalt [81] and 20–60% of the export has come from artisanal mining in the last decade [82,83]. The existing literature has focused on developing LIB recycling technologies, and only a few studies attempted to quantify the environmental impacts of various LIB recovery options through life cycle assessment (LCA).
Repurposing: Ahmadi et al. [29] investigated the environmental impacts of repurposing spent EV LIB packs for stationary ESS (e.g., solar energy storage), using the ReCiPe method [84]. They concluded that repurposing offers a significant environmental benefit by extending the life of LIBs and thus delaying LIB manufacturing with minor added impacts from remanufacturing. Moreover, they showed that the global warming potential (GWP) of an LFP battery pack over its life cycle is ∼0.25 kg CO2 equivalent (eq.) per kWh battery capacity. Battery manufacturing dominates the life cycle GWP, contributing ∼40% of the GWP. Within LIB manufacturing, cathode production is the primary source of GWP, consistent with the research efforts on cathode recovery. Another study by Richa et al. [85] conducted an LCA on cascaded reuse of EV LIBs with LMO cathodes in stationary ESS. This study demonstrated that reusing LIBs in ESS could reduce the net cumulative energy demand (CED) and GWP by 15–70% compared with using lead-acid (PbA) batteries.
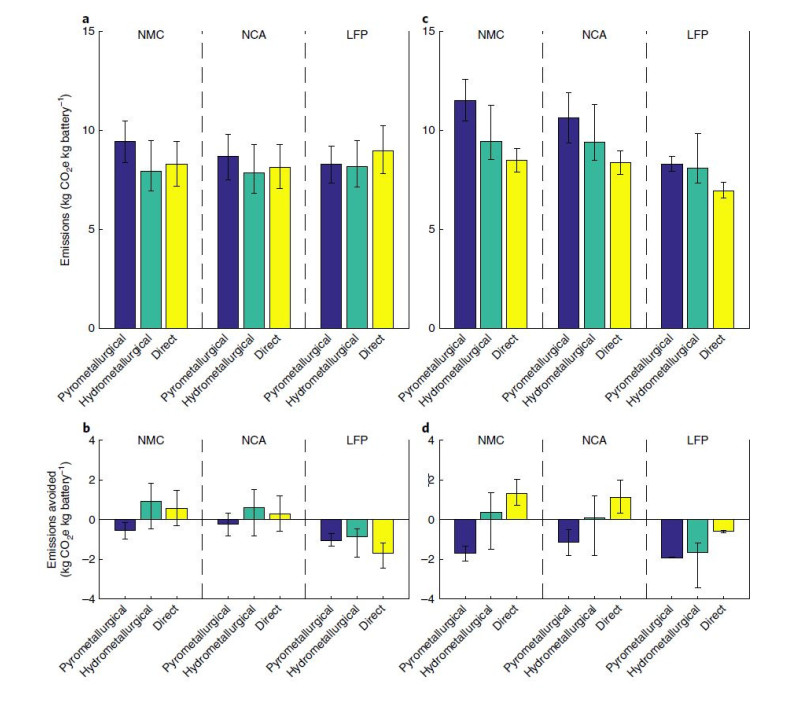
Metals Recycling: Ceiz and Whitacre [86] examined the environmental impacts of pyrometallurgical, hydrometallurgical, and direct recycling of NMC, NCA, and LFP batteries, using Greenhouse gases, Regulated Emissions, and Energy use in Technologies (GREET) Model 2016 (Figure 7). This study showed that, except for LFP, the greenhouse gas (GHG) emissions generated by recycling LIBs using hydrometallurgical or direct recycling were lower than those resulting from new LIB production. Direct recycling showed better environmental performance, as high CO2 eq. emissions (compared with those of the hydrometallurgical process) were avoided by preserving the cathode material structure. For LFP cells, the iron precursor materials used in LFP cathodes are more energetically efficient to produce and emit lower GHGs than nickel, cobalt, and manganese precursors used in the other cathodes. Therefore, the net GHG saving from recycling LFP batteries was lower (resulting primarily from avoiding the burden of new cathode manufacturing) than that resulting from the other cathode chemistries.
Gaines et al. [17] compared the costs and environmental impacts associated with producing 1 kg of NMC111 from virgin materials and three recycling techniques — hydrometallurgical, pyrometallurgical, and direct recycling (Figure 8). The EverBatt model, an Excel-based software developed by Argonne National Laboratory, was used to estimate the process costs and impacts. Direct recycling generated the lowest cost and environmental impacts [86]. The superior economic and environmental performance of direct recycling (compared with that of other techniques) resulted from the low temperature/energy consumption and cathode material structure preservation aimed at avoiding virgin production.
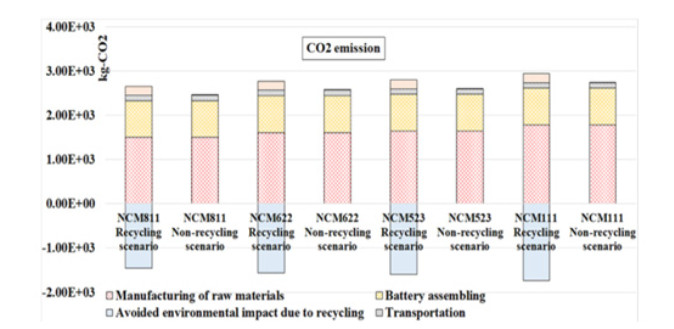
Wang and Yu [87] conducted LCA on NMC-type EV batteries in China and considered two scenarios—landfilling vs. recycling—using MiLCA software. The system boundary included dismantling of spent LIBs, alkali leaching of electrodes, and acid leaching of cathode materials. Figure 9 shows the environmental benefit of recycling over landfilling in terms of the carbon footprint. An important finding is that the evolution of cathodes toward lower cobalt content (i.e., from NMC111 to NMC811) reduced the carbon footprint of cathode manufacturing. However, this reduction also lowered the benefit of recycling the cathode materials, which was manifested as avoidance of the carbon footprint resulting from recycling. Therefore, the overall cradle-to-cradle impacts of the recycling scenario were similar across different NMC chemistries.
Cascaded reuse, repurposing, and recycling. Richa et al. [88] studied the environmental impacts of four different waste management strategies for LIBs: (1) closed-loop direct reuse in EVs, (2) open-loop cascaded use in stationary ESS, (3) recycling, and (4) landfills. The results showed that the environmental benefits from reuse and recycling outweigh the environmental burden from the value recovery activities (e.g., testing, cell replacement, and metal recycling). However, the benefit from closed-loop reuse of LIBs in EVs is minimal, owing primarily to the fact that increasing energy losses from aging batteries, results in declining travel efficiency. Cascaded use in stationary ESS yields the most significant environmental benefits (e.g., net CED saving of 1,330 MJ/kWh) by avoiding (1) the landfilling of spent LIBs and (2) production and use of lead-acid batteries, which are popular in stationary ESS applications. Regarding recycling methods, the hydrometallurgical process was 25% better in terms of CED than the pyrometallurgical process due to the lower energy input. The last option, landfilling, is associated with landfill leaching and eco-toxicity, in particular from manganese.
Limitations and future work. First, the inclusion of value recovery supply chain and transportation-related impacts has rarely been reported in the LCA literature. The existing LCA tools such as the EverBatt model can be used to calculate the transportation impacts, and the users need to define the transportation mode and distance that reflect the current LIB supply chain in their LCA studies. In the papers we have reviewed, the transportation distance was assumed without any elaboration on the connection to the LIB supply chain, and some papers did not discuss the transportation mode or distance in their LCA. As the LIB recycling business is at an early stage of development in the US and LIB manufacturing infrastructure is heavily concentrated in Asia, the recycled black mass or battery precursors may need to be transported overseas to close the materials loop. As a relevant example, recovering NdFeB magnet assembly from hard drives collected in the US entailed transportation of the EOL hard drives to Thailand due to lack of disassembly and manufacturing facilities in the US, and the transportation mode (i.e., air vs. sea) played a decisive role in the environmental benefit of the recovery strategy [89]. Transportation would therefore contribute significantly to the environmental impact of LIB recycling. Second, variations in functional unit definition (e.g., 1 kg of cathode materials vs. 1 kWh of battery capacity vs. 1 kWh of energy delivered) and LCA methods adopted (e.g., ReCiPe vs. GREET) were commonplace. These variations led to heterogeneous results that were difficult to integrate or compare. In addition, life cycle inventory data was not disclosed in some LCA studies, challenging the reliability, transparency, and repeatability of the analysis. Therefore, future studies could explore the supply chain implications of the recycled LIB materials, standardize the functional unit and LCA method, and disclose the full life cycle inventory data in order to facilitate data interpretation.
3.2. Techno-economic assessment
Techno-economic assessment is an important tool for evaluating and improving the economic feasibility of implementing LIB recycling technologies on a commercial scale. The primary focus of LIB recycling was to recover cobalt, due to its high price, and the recovery of other elements was considered the secondary objective. However, the evolution of cathode chemistries toward less cobalt [90] has reduced the revenue from LIB recycling and increased interests in recovering additional elements such as lithium, manganese, and nickel. Similar to the LCA results, LIB reuse was generally more favorable than metals recycling from spent LIBs. Richa et al. [88] proposed a waste management hierarchy for EV LIBs and estimated the economic benefit from different value recovery pathways, including direct reuse, cascaded use, and recycling. The purchasing price of a new EV LIB was assumed to be $125/kWh, and users could purchase a refurbished one for $38/kWh, which was 30% of the new LIB purchasing cost. For the recycling pathway, they assumed that LIB waste streams were processed either by means of a pyrometallurgical or hydrometallurgical process. A maximum recycling capacity of 34,000 tons/year was assumed (fixed cost: $1,000,000/year and variable cost: $1,100/ton). Their results showed that all value recovery pathways resulted in economic savings. In fact, the pathways could be listed as follows, cascaded reuse in stationary ESS ($590/LIB pack) > direct reuse in EV ($480/LIB pack) > and recycling ($50/LIB pack), i.e., in descending order of the savings generated.
Wang et al. [87] investigated the economic impact of recycling spent EV batteries with different cobalt concentrations. A hydrometallurgical process, using sodium hydroxide, acetic acid, and acetone solutions, was adopted for recycling NMC batteries in China. Table 4 shows the detailed cost estimates and those from three other studies, which indicate that the major cost driver is the waste LIB purchasing cost. The results showed that the recycling profit decreased from 10,603 CNY/kg to 8,242 CNY/kg when the cobalt content was reduced from NMC111 to NMC811.
Wang et al. [94] developed an optimization model for identifying the minimum break-even recycling volume of LIB scraps by changing the share of LCO, LFP, and LMO batteries in the mixed LIB scraps. They concluded that the minimum recycling volume should be 170 tons per year based on three major assumptions: (1) all the LIB feedstocks should be of LCO cathode type, the most valuable one among the three alternatives, (2) the variable cost was $2,800 per ton LIB, and (3) the fixed cost was $1 M at a processing capacity of 34,000 tons of LIB per year. Revenue from recycling LCO batteries was seven times higher than that from LFP batteries and ten times higher than that from LMO batteries, owing primarily to the higher cobalt price. As the LIB industry moves toward reducing the amount of cobalt in cathodes, the economic incentives for recycling will decrease.
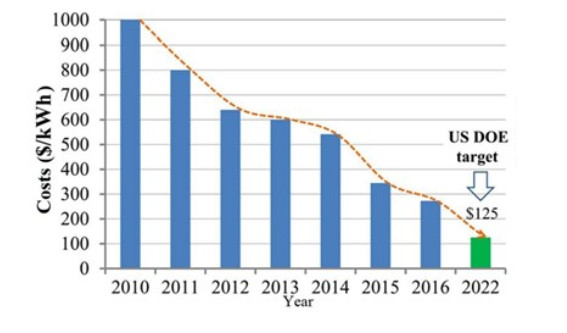
Future research direction: Ding et al. [18] and Bloomberg New Energy Finance [95] projected that the cost of LIB manufacturing will decrease continuously, as shown in Figure 10, through new technology development (e.g., new battery chemistries—NMC811 and Si anode) and product design optimization (e.g., cell design). Consequently, the LIB recovery technologies are challenged to be robust to process diverse incoming EOL LIB designs and chemistries and yet lower the cost continuously to compete with virgin LIB production. For instance, recycling of LFP and LMO cathodes through pyro- or hydro- processes could not pay back the recovery cost due to the low value of the constituent materials, rendering direct recycling the potential solution [23]. Another benefit of direct recycling is the streamlined process that reduces the processing steps and transportation distances among various stakeholders of the supply chain, since it could directly recover cathode materials as opposed to the other techniques recovering metal alloys or cathode precursors [23,49]. Therefore, future studies may focus on addressing the limitations associated with direct recycling shown in Table 2 for industry adoption of this new technology. For example, development of cost-effective sortation technologies would be desirable for separating batteries with different cathodes. Another way to address the mixed cathodes problem is to develop recycling methods that enable the production of high-value products, such as cathode, directly from mixed feed without breaking down the cathode structures (e.g., application to a mixture of NMC cathodes) [23]. In addition, policy incentives that promote LIB recovery may help the economic viability of LIB recycling, as will be shown in the next section.
3.3. Battery recycling policies
The EU Battery Directive has established regulations for the proper recycling and disposal of EOL batteries [90]. The EOL Vehicle Directive in the EU also provided guidelines for collecting and managing EOL vehicles and their components [96]. The US classified mercury-based, nickel-cadmium, and PbA batteries as hazardous wastes that fall under the standards for universal waste management (i.e., Mercury-Containing and Rechargeable Battery Management Act of 1996) [97]. However, LIBs are overlooked by the Act, and national-level recycling mandates for LIBs in the US are lacking. Only three states have implemented regulations that prohibit landfilling of LIBs and mandated (via law) that retailers take back LIBs. These regulations are referred to as California's rechargeable battery recycling [98], New York state rechargeable Act of 2006 [99], and Minnesota rechargeable battery law of 1994 [100].
Table 5 shows the global policies and recommendations for LIBs in different life cycle phases [88]. For example, the EOL Vehicle Directive (ELV) prohibits hazardous material usage in battery manufacturing and recommends a design for dismantling aimed at facilitating battery removal. Labeling of batteries is also important for identifying hazardous material content and facilitating the subsequent EOL treatment. The EU Battery Directive (BD) and ELV facilitate the collection of EOL batteries through Extended Producer Responsibility (EPR). EPR is also practiced in three US states wherein manufacturers must allow free return of EOL batteries by consumers. New York and California regulations for battery collection are limited to consumer electronics (Call2Recycle [101]) and exclude large vehicle batteries. Domestic and international transportation of LIBs should meet specific safety requirements, as LIBs are classified as class 9 miscellaneous hazardous materials [102].
The European Commission proposed a new battery regulation in December 2020 to establish requirements for sustainable and competitive battery value chains, which include carbon footprint declaration, minimum recycled content, and labelling with more information (see Supplementary Table S3) [41,103]. Updates on the California's Rechargeable Battery Recycling Act are also underway.
Financial strategies may create additional incentives for recycling batteries. For example, the state of New York imposed civil penalties of up to $200, $500, and $5,000 for consumers, manufacturers, and retailers, respectively, for improper disposal of batteries [99]. Consumer depositing of funds at the time of purchase may also promote the collection of spent batteries. The EPR policies shown in Table 5 also reduce the collection cost burden from LIB recyclers and improve economies of scale with increased LIB collection volume.
3.4. Reverse supply chain optimization
To implement the LIB recovery technologies, reverse logistics deal with the collection of EOL products from end-users and the transport of such products to downstream processors for reuse, remanufacturing, and recycling. Spent LIBs are class 9 hazardous materials, and the transportation cost in the US could constitute 27% of the total recycling cost, which is four times higher than the recycling materials cost [20,33]. Optimizing the reverse logistics network will reduce the cost and environmental impact associated with transportation while maximizing the service level (i.e., percentage of delivered product relative to the customer demand). However, only a few research articles have been published on the strategic design of a reverse logistics network for spent LIBs. Table 6 summarizes the relevant studies in this area.
To minimize operations cost and GHG emissions, Wang et al. [25] designed an optimal reverse logistics network for spent LIBs. Their model considered three potential strategies for handling spent batteries of different qualities: reuse, remanufacturing, and recycling. The optimal configuration of the recycling network is affected mainly by transportation costs, the carbon tax, and the number of used electric vehicle batteries. Although the processing cost constituted a major cost, changes in the processing cost had no effect on the optimal network design. Li et al. [104] integrated remanufacturing and recycling into an existing forward supply chain network of LIBs, which could increase the total profit by 31%. The cost savings associated with the integrated supply chain resulted mainly from the reduced transportation cost, raw material purchasing cost, and processing cost. Sensitivity analysis revealed that the profit was mainly influenced by the processing cost of collection centers, highlighting the importance of optimizing collection facility operations (e.g., dismantling). Hoyer et al. [105] developed an optimization model that allowed a sequential deployment of recycling plants over time. The strategy was found to return a higher net present value than establishing all the plants at the beginning of the planning horizon because future developments in the LIB market are particularly uncertain and dynamic. Tadaros et al. [106] designed a future supply chain network for spent LIBs in Sweden. They emphasized the importance of increasing the initial capacity of inspection sites and recycling centers compared with that of the status-quo and opening new facilities over the years to accommodate the increasing volume of spent LIBs. Hendrickson et al. [107] applied geospatial modeling to determine the optimal facility locations for LIB recycling in California with the goal of minimizing the cost and GHG emissions. The results showed that, compared with using air transport, using rail and truck transportation could substantially reduce transportation-related GHG emissions (23–45%). The authors also used LCA to compare LIB virgin production with pyrometallurgical recycling. Material recovery from pyro-process was found to lower the primary energy demand by 6–56% and decrease GHG emissions by 23% compared to virgin production.
With the limited literature on optimizing LIB reverse logistics, numerous opportunities are available for future research in this area.
● The objective functions could be expanded to cover all three dimensions of sustainability—economic, environmental, and social. Although a few studies have assessed the environmental impacts, these assessments focused on only GHG emissions. Future research could broaden the range of environmental impact categories (to include (for example) CED and water consumption), thereby facilitating informed decision-making for sustainable operations.
● Each study has overlooked uncertainty in the mathematical models employed. However, supply chain operations can be highly sensitive to uncertain parameters such as the purchasing cost of spent LIBs, return quantity, time of return, market price, and demand for recycled products. Therefore, advanced mathematical modeling techniques such as stochastic programming or robust optimization could be applied in future studies to design a resilient LIB reverse supply chain. Furthermore, different risk mitigation strategies (including (among others) alternative sourcing [108], backup suppliers [109], spare capacity [110], buffer inventory [111], facility fortification [112], and substitute products [113]) could be tested.
● Optimization models will be tailored to the promising technologies and supply chain infrastructure. Li-Cycle, North America's largest LIB recycling company, patented a spoke and hub technology that segregates collection and pre-processing facilities (i.e., spokes) and downstream recycling facilities (i.e., hubs) for efficient LIB recovery infrastructure [114]. This technology was claimed to be suitable for all battery chemistries and able to extract high-grade materials for battery reproduction at a cost lower than virgin battery materials. The reverse logistics network shall be optimized to enable introduction of these emerging technologies to the market.
● Future research shall delve into the vertical integration of the entire LIB reverse supply chain to close the material loop. Wang et al. [25] assumed that reusable battery materials are transported to new LIB manufacturing plants in China, which dominates the current LIB production market. For LIB recycling in other countries (e.g., Sweden, Germany, and USA; see Table 6), export of the recycled materials overseas for further processing may be required. This additional step shall be accounted for in the reverse logistics design to help optimize the overall supply chain impact.
4.
Conclusions
This paper reviewed LIB value recovery technologies and the associated environmental impacts, economics, policies, and supply chain optimization strategies. Emerging technologies developed for LIB recycling employ hydrometallurgical, pyrometallurgical, and direct recycling. However, their commercial viability is challenged by the high recycling cost and changes in battery chemistry toward less valuable metals (e.g., lower cobalt content) reduce the economic and environmental incentives for recycling. Optimizing the disassembly process, material recycling technologies, and reverse logistics would contribute to sustainable value recovery from LIBs.
General recommendations for future studies include:
● Prioritizing reuse and direct recycling over hydrometallurgical and pyrometallurgical processes to preserve the functional value of LIBs and cathode materials, respectively, and eliminate the need for harsh chemical- or energy-intensive processes.
● Developing value recovery technologies for the emerging LIB cathode materials (e.g., LFP, NMC811) that contain less valuable materials (but would increase in market share) than conventional cathode materials.
● Evaluating different policies and their impacts on LIB recycling to help identify the most effective strategies for incentivizing technology development and commercialization that exert the minimum environmental impacts.
● Incorporating the impact of reverse logistics in the economic and environmental analysis to account for the current limitations of the LIB supply chain (e.g., manufacturing facilities concentrated in Asia) and vertically integrate recovery processes to close the material loop.
● Designing a reverse supply chain that strategically optimizes the economic, environmental, and uncertainty aspects of LIB value recovery at the outset of process development and commercialization efforts.
Acknowledgments
This work was supported through a sub-contract from the Ames Laboratory with funding from the Department of Energy-Energy Efficiency and Renewable Energy under contract No. DE-AC02-07CH11358; Agreement No. 26110-AMES-CMI.
Conflict of interest
All authors declare no conflict of interest in this paper.









 DownLoad:
DownLoad: