1.
Introduction
Fréshet [1] introduced the concept of a distance between two abstract objects. A well known Banach contraction principle [2] in metric spaces has been extended in various directions [3,4,5,6,7]. Zadeh [8] introduced the concept of a fuzzy set, back in 1965, as an extension of a crisp set where each element of a set has some membership values between [0,1]. Since then, several mathematical structures have been transformed to fuzzy sets, see [9,10,11,12,13,14,15]. Kramosil and Michálek [16] applied this theory to metric spaces and defined fuzzy metric space which could be viewed as a reformulation of statistical metric spaces [17]. Grabiec [18] first defined the concept of convergence of a sequence and a Cauchy sequence and then proved the Banach and Edelstein contraction principles in fuzzy metric space. In 1994, George and Veeramani [19] modified the definition of a fuzzy metric space given in [16]. This generalized definition of a fuzzy metric space is now being used widely [20,21,22,23,24,25,26].
Bakhtin [27] introduced the concept of a b-metric space which generalizes the notion of metric space. In 1993, Czerwik [28] studied some contractive mappings in b-metric space. Branciari [29] introduced rectangular metric spaces and proved Banach-Caccippoli type fixed point result in this new setup. In 2016, Roshan et al. [30] extended the notion of a rectangular metric space by introducing b-Branciari or b-rectangular metric space. Nǎdǎban [31] generalized the notion of a fuzzy metric space in the sense of Kramosil and Michálek by introducing fuzzy b-metric space. He also introduced the concept of fuzzy quasi b-metric space as the generalization of a fuzzy quasi metric space. In [32], authors replaced the constant b in the definition of b-metric space with some function θ and introduced the extended b-metric space, while in [33] the definition of a fuzzy b-metric space was generalized to extended fuzzy b-metric space. The generalization of a fuzzy rectangular metric space to fuzzy rectangular b-metric space is given in [34]. Asim et al. [35] proved fixed point results for contractive mapping in extended rectangular b-metric space. Sezen [36] introduced the concept of controlled fuzzy metric space by using a controlled function λ and proved some interesting fixed point results in this space. Mani [23] et al. introduced the concept of a fuzzy triple controlled bipolar metric spaces and proved the existence and uniqueness of the solution of an integral equation.
In this paper, we follow the definition of George and Veeramani and define extended rectangular fuzzy b-metric space as an extension of fuzzy rectangular b-metric space, fuzzy rectangular metric space, extended fuzzy b-metric space, fuzzy b−metric space and fuzzy metric space. We generalize the Banach contraction principle in the framework of extended rectangular fuzzy b-metric space.
2.
Preliminaries
Definition 2.1. [37] A binary operation ∗:[0,1]×[0,1]⟶[0,1] is called a continuous t-norm if
1) ∗ is continuous, commutative and associative,
2) a∗1=a for all a∈[0,1],
3) a∗b≤c∗d for all a,b,c,d∈[0,1] provided that a≤c,b≤d.
Definition 2.2. [38] Let X be a non-empty set, ∗ be a continuous t-norm. A fuzzy set Mr:X×X×(0,∞)⟶[0,1] is called a fuzzy rectangular metric on X, if for distinct u,x,y,z∈X and t,s,w>0, following conditions hold:
(Mr1) Mr(x,y,t)=0,
(Mr2) Mr(x,y,t)=1 for all t>0 if and only if x=y,
(Mr3) Mr(x,y,t)=Mr(y,x,t),
(Mr4) Mr(x,z,(t+s+w))≥Mr(x,y,t)∗Mr(y,u,s)∗Mr(u,z,w),
(Mr5) Mr(x,y,⋅):(0,∞)⟶[0,1] is left continuous and limt⟶∞Mr(x,y,t)=1.
The triplet (X, Mr, ∗) is called a fuzzy rectangular metric space.
Definition 2.3. [34] Let X be a non-empty set, ∗ be a continuous t-norm and b≥1 be a real number. A fuzzy set Mrb:X×X×(0,∞)⟶[0,1] is called a fuzzy rectangular b-metric on X, if for distinct u,x,y,z∈X and t,s,w>0, following conditions hold:
(Mrb1) Mrb(x,y,t)=0,
(Mrb2) Mrb(x,y,t)=1 for all t>0 if and only if x=y,
(Mrb3) Mrb(x,y,t)=M(y,x,t),
(Mrb4) Mrb(x,z,b(t+s+w))≥Mrb(x,y,t)∗Mrb(y,u,s)∗Mrb(u,z,w),
(Mrb5) Mrb(x,y,⋅):(0,∞)⟶[0,1] is left continuous and limt⟶∞Mrb(x,y,t)=1.
The triplet (X,Mrb,∗) is called a fuzzy rectangular b-metric space.
3.
Main results
In this section we will define the notion of an extended rectangular fuzzy b-metric space that generalizes many fuzzy metric spaces in the literature.
Definition 3.1. Let X be a non-empty set, ∗ be a continuous t-norm and α:X×X⟶[1,∞). A fuzzy set M:X×X×(0,∞)⟶[0,1] is called an extended rectangular fuzzy b-metric on X, if for distinct u,x,y,z∈X and t,s,w>0, following conditions hold:
(M1) M(x,y,t)>0,
(M2) M(x,y,t)=1 for all t>0 if and only if x=y,
(M3) M(x,y,t)=M(y,x,t),
(M4) M(x,z,α(x,z)(t+s+w))≥M(x,y,t)∗M(y,u,s)∗M(u,z,w),
(M5) M(x,y,⋅):(0,∞)⟶[0,1] is continuous.
The triplet (X,M,∗) is called an extended rectangular fuzzy b-metric space.
Remark 3.1. (i) Taking α(x,z)=b≥1 in (M4), then an extended rectangular fuzzy b-metric space reduces to a fuzzy rectangular b-metric space [34].
(ii) Taking α(x,z)=1 in ([M4]), then an extended rectangular fuzzy b-metric space reduces to a fuzzy rectangular metric space [38].
(iii) Taking u=z and s+w=t′ in ([M4]), then an extended rectangular fuzzy b-metric space reduces to extended fuzzy b-metric space [33].
(iv) Taking u=z and s+w=t′ and α(x,z)=b≥1 in ([M4]), then an extended rectangular fuzzy b-metric space reduces to fuzzy b-metric space [31].
(iv) Taking u=z and s+w=t′ and α(x,z)=1 in ([M4]), then an extended rectangular fuzzy b-metric space reduces to fuzzy metric space [19].
We now give an example of an extended rectangular fuzzy b-metric space.
Example 3.1. Let X={1,2,3,4}. If we define d:X×X⟶[0,∞) by d(x,y)=(x−y)2 for all x,y in X and α:X×X→[1,∞) by α(x,y)=x2+y2+1. Then M:X×X×(0,∞)→[0,1] given by
is an extended rectangular fuzzy b-metric on X provided that ∗ is a minimum t-norm, that is, a∗b=min{a,b} for all a,b∈[0,1].
Clearly,
and
Note that first three axioms (M1–M3) clearly hold. To prove the axiom (M4), we discuss the following cases:
Case 3.1. Let x=1,z=4. Then, we have
Also,
Note that
Thus,
similarly, we have
and
Hence
Case 3.2. Let x=2, and z=4. Then
and
Now
implies that
Similarly, we obtain that
and
Hence
Case 3.3. If x=3, and z=4, then we have
Also,
Now
implies that
Similarly,
Now
gives that
Hence
Thus in each case, we have,
for all distinct u,x,y,z∈X and t,s,w>0. Hence (X,M,∗) is an extended rectangular fuzzy b-metric space.
We now give an example of extended rectangular fuzzy b-metric space with product t-norm but is not an extended rectangular fuzzy b-metric space with minimum t-norm.
Example 3.2. Let X={1,2,3,4}. Define α:X×X⟶[1,∞) by
Define a mapping M:X×X×(0,∞)⟶[0,1] by
Then (X,M,∗) is an extended rectangular fuzzy b-metric space with product t-norm, that is, a∗b=ab for all a,b∈[0,1].
Note that,
It is straight forward to verify axioms (M1) to (M3). We verify axiom (M4). For this, we consider the following cases:
Case 3.4. If x=1, and z=4, then either y=2 and u=3 or y=3 and u=2. We prove the case for y=2 and u=3. Note that
implies that
The proof of the case for y=3 and u=2 is similar. Similarly, one can verify the remaining cases. Hence (X,M,∗) is an extended rectangular fuzzy b-metric space with product t-norm. However, M(x,y,∗) is not an extended rectangular fuzzy b-metric space with respect to minimum t-norm. For instance, if we take
then
Clearly,
Example 3.3. Let X=N. Define
for all t>0 with t1∗t2=t1t2 and α(x1,x2)=3(x1+x2). Then (X,M,∗) is an extended rectangular fuzzy b-metric space but not a rectangular fuzzy metric space. Let x1,x2,x3,x4 be distinct elements in X and t,s,w>0. As
we have
Hence (X,M,∗) is an extended rectangular fuzzy b-metric space.
Definition 3.2. Let X be a non-empty set and α:X×X⟶[1,∞). A function f:R⟶R is called an α-non-decreasing if for t<s, we have f(t)≤f(α(x,y)s). If α(x,y)=b≥1, then f is called b-non-decreasing. if we take α(x,y)=1, then f is called non-decreasing.
Lemma 3.1. Let (X,M,∗) be an extended rectangular fuzzy b-metric space and α:X×X⟶[1,∞) be a continuous function, then M(x,y,.) is α-non-decreasing for all x,y∈X.
Proof. Let 0<t<s, then
Hence M(x,y,sα(x,y))≥M(x,y,t) which shows that M(x,y,.) is α-non-decreasing for all x,y∈X.
On the basis of Lemma 1, we have following observations.
Remark 3.2. (i) An extended rectangular fuzzy b-metric is not increasing.
(ii) If we choose α(x,y)=b≥1, then every rectangular fuzzy b-metric is b-non-decreasing.
(iii) Every extended fuzzy b-metric is an α-non-decreasing, where α:X×X⟶[1,∞).
(iv) If we take α(x,y)=1, then every rectangular fuzzy metric is non-decreasing.
Definition 3.3. Let (X,M,∗) be an extended rectangular fuzzy b-metric space, x∈X and r∈(0,1). An open ball with center x and radius r is denoted by B(x,r,t) and is defined as:
where, t>0.
In the following example, we show that an extended rectangular fuzzy b-metric space is not Hausdorff.
Example 3.4. Consider an extended rectangular fuzzy b-metric space as given in Example 3.1. Consider the open ball with center x1=1, radius r1=0.5 and t=7 as
Now
Hence,
Now consider the open ball with center x2=2, radius r2=0.45 and t=7 as
Now
Hence,
Note that B1(1,0.5,7)∩B2(2,0.45,7)={1,2,3}∩{1,2,3,4}={1,2,3}≠∅. Which shows (X,M,∗) is not Hausdorff.
Analogous to the concepts of Cauchy sequence and the convergence of sequences given in [19], we have the following definition in the settings of an extended rectangular fuzzy b-metric space:
Definition 3.4. Let (X,M,∗) be an extended rectangular fuzzy b-metric space. Then a sequence {xn} in X is called:
1) convergent sequence if there exists x∈X such that,
2) Cauchy sequence if and only if,
An extended rectangular fuzzy b-metric space is complete if every Cauchy sequence in X is convergent in X.
Lemma 3.2. Let {xn} be a Cauchy sequence in an extended rectangular fuzzy b-metric space (X,M,∗) such thatxm≠xn whenever m≠n,m,n∈N. Then {xn} converges to at most one point in X.
Proof. On the contrary suppose that xn⟶x and xn⟶y for x≠y. Then limn⟶∞M(xn,x,t)=1 and limn⟶∞M(xn,y,t)=1 for all t>0.
Consider
as n,m⟶∞. So M(x,y,t)=1, a contradiction as x≠y.
Following lemma is needed in the proof of our main results.
Lemma 3.3. [39] Let x,y be any two points in a fuzzy metric space (X,M,∗). If for any k∈(0,1), we have
then x=y.
Definition 3.5. Let (X,M,∗) be an extended rectangular fuzzy b-metric space. A mapping T:X⟶X satisfying
is called Ćirić type fuzzy contraction, where
Now, we prove our main result in the settings of an extended rectangular fuzzy b-metric space.
Theorem 3.1. Let (X,M,∗) be a complete extended rectangular fuzzy b-metric space, α:X×X⟶[1,1k) be a continuous function with k∈(0,1) such that
and T:X⟶X be a Ćirić type fuzzy contraction. Then T has a unique fixed point.
Proof. Let a0∈X be an arbitrary point. If Ta0=a0, then a0 is the required fixed point. If Ta0≠a0, then there exists a1∈X such that Ta0=a1. Continuing in this way, we define {an} in X such that
Note that
where
If min{M(an,an+1,3t),M(an−1,an,t),1}=M(an,an+1,3t) or 1, then M(an,an+1,kt)≥M(an,an+1,3t) or 1 and hence by Lemma 3, we have an=an+1. If an≠an+1 and min{M(an,an+1,3t),M(an−1,an,t),1}=M(an,an+1,3t) or 1, then we have a contradiction because M(x,y,.) is α-non-decreasing. Now if min{M(an,an+1,t),M(an−1,an,t),1}=M(an−1,an,t), then we obtain that M(an,an+1,kt)≥M(an−1,an,t). Similarly, we have M(an−1,an,t)≥M(an−2,an−1,tk) and M(an−2,an−1,tk)≥M(an−3,an−2,tk2) and so on. Hence,
which implies that
Consider the following two cases:
Case 3.5. If p is odd (say) p=2m+1 for m≥1, then
By (3.2), the right hand side of the above inequality becomes,
Case 3.6. If p=2m, m∈N, that is, p is even, then
From (3.2), the right hand side of the above inequality becomes,
Thus,
Which show that {an} is a Cauchy sequence in X. As X is complete, there exists some a∈X such that
We now prove that a is the fixed point of T. For this, consider
Now
On taking limit as n⟶∞, we have
If limn→∞C(an,a,t3kα(a,Ta))=1, then M(a,Ta,t)≥1∗1∗1 and hence a=Ta. If limn→∞C(an,a,t3kα(a,Ta))=M(a,Ta,tkα(a,Ta)), then we have M(a,Ta,t)≥1∗1∗M(a,Ta,tkα(a,Ta))=M(a,Ta,tkα(a,Ta)). Now α(a,Ta)<1k implies that 1<1kα(a,Ta), that is, t<tkα(a,Ta) and hence by Lemma 3.3, we have a=Ta. To prove the uniqueness of a fixed point, let a′ be another fixed point of T. Note that
where,
If C(a,a′,tk)=1, then a=a′. If C(a,a′,tk)=M(a,a′,tk), then M(a,a′,t)≥M(a,a′,tk) and hence by Lemma 3.3, we have a=a′. If C(a,a′,tk)=21+M(a,a′,tk), then we obtain that M(a,a′,t)≥21+M(a,a′,tk). As M(a,a′,tk)≤1, 21+M(a,a′,tk)≥1 and M(a,a′,t)≥1. Now 1≥M(a,a′,t)≥1 implies that M(a,a′,t)=1 which further implies that a=a′.
Following example is to support our main result.
Example 3.5. Let X={0,1,2,3}, define a complete extended rectangular fuzzy b-metric space (X,M,∗) with α(x,y)=3(x2+y2+1) and
under product t-norm t1∗t2=t1t2. Let T:X⟶X be a mapping defined as Tx=x2, for all x∈X. To satisfy the contractive condition 3.1 used in Theorem 3.1, we have the following cases:
Case 3.7. If x=0,y=1, then
Clearly, C(0,1,t)=e−1t. Also,
Hence, M(T0,T1,kt)>C(0,1,t).
Case 3.8. If x=0,y=2, then
Clearly, C(0,2,t)=e−4t. Also,
Hence, M(T0,T2,kt)>C(0,2,t). Similarly, for the other cases, we can calculate C(x,y,t) for all x,y∈X as:
C(0,3,t)=C(3,0,t)=e−9t, C(0,0,t)=1,C(1,0,t)=C(1,2,t)=C(2,1,t)=C(2,2,t)=e−1t, C(1,1,t)=C(2,0,t)=C(2,3,t)=C(3,1,t)=C(3,3,t)=e−4t, C(1,3,t)=e−254t, C(3,2,t)=e−94t.
Observe that M(Tx,Ty,kt)=M(Ty,Tx,kt) and M(Tx,Tx,kt)=1 for all x,y∈X, after simple calculation, we have the following observations:
M(T0,T3,kt)=e−94kt, M(T1,T0,kt)=M(T1,T2,kt)=M(T2,T3,kt)=e−14kt, M(T1,T3,kt)=e−1t, M(T2,T0,kt)=e−1kt. Since
where C(x,y,t)=min{M(y,Ty,3t)[1+M(x,Tx,t)]1+M(x,y,t),M(x,Tx,t),M(y,Ty,3t),M(y,Tx,t),M(x,y,t)}holds for k∈(14,1) and for all x,y∈X. All conditions of the Theorem 3.1 are satisfied, hence 0 is the unique fixed point of T in X.
If we take C(x,y,t)=M(x,y,t) in the Círić type contraction (3.1), then we have the following Banach contraction principle.
Theorem 3.2. Let (X,M,∗) be a complete extended rectangular fuzzy b-metric space such that
and α:X×X⟶[1,1k),wherek∈(0,1). If T:X⟶Xis a mapping such that for allx,y∈X, we have
Then T has a unique fixed point.
Proof. By taking C(x,y,t)=M(x,y,t) in Theorem 3.1, the proof follows on the same lines.
We now present an example to support the above result.
Example 3.6. Let I=[0,1] and T:I⟶I a mapping given by
Define M:I×I×(0,∞)⟶I by
Note that M(x,y,t) is a complete extended rectangular fuzzy b-metric space and
Thus all the conditions of Theorem 3.2 are satisfied. Moreover, x=0 is a unique fixed point of T.
Theorem 3.2 generalizes Theorem 2.1 in [34] in the following way.
Corollary 3.1. Let (X,M,∗) be a complete fuzzy rectangular b-metric space such that
If T:X⟶X is a mapping such that for k∈(0,1b) and for all x,y∈X, we have
Then T has a unique fixed point.
Proof. Choose α(x,y)=b≥1 and k∈(0,1b) in Theorem 3.2, rest of the proof follows on the same lines.
Remark 3.3. By taking α(x,y)=b≥1 in Theorem 3.2, our main result reduces to the main results in [34].
Corollary 3.2. Let (X,M,∗) be a complete fuzzy rectangularmetric space such that limt→∞M(x,y,t)=1. IfT:X⟶X is a mappingsuch that for all x,y∈X, we have
where k∈(0,1). Then T has a unique fixed point.
Proof. Take α(x,y)=1 in Theorem 3.2 remaining proof will follow on the same lines.
Remark 3.4. If we take α(x,y)=1, then Theorem 3.2 generalizes the Banach contraction theorem for fuzzy rectangular metric space [38].
4.
Application
In this section, we consider the following integral equation
where s∈I=[0,1]. Define a complete extended rectangular fuzzy b-metric space with α(x,y)=3(x+y+1) and for all t>0, x,y∈C(I,R) as:
with product t-norm, where C(I,R) is the space of all continuous functions defined on I.
Theorem 4.1. Consider an integral operator defined on C(I,R) as follows:
where F satisfies the conditions:
there exists f:I×I⟶[0,∞] such that f∈L1(I,R) and for all x,y∈C(I,R) and r,s∈I, we have
where
Then the integral Eq (4.1) has a unique solution.
Proof. Let x,y∈C([0,a],R),k∈(0,1). Note that
Thus M(Tx(s),Ty(s),kt)≥M(x,y,t), for all x,y∈C([0,a],R). Since all the conditions of Theorem 3.2 are satisfied so the integral Eq (4.1) has a unique solution.
Example 4.1. Consider the differential equation
which gives the following integral equation
Here, F(s,r,y(r))=(s−r)y(r). Note that
Take, f(s,r)=(s−r). Clearly sups∈I∫0sf2(s,r)dr≤1. Note that
Thus M(Tx(s),Ty(s),kt)≥M(x,y,t), for all x,y∈C([0,a],R). Since all conditions of Theorem 3.2 are satisfied so the integral Eq (4.1) has a unique solution.
5.
Conclusions
In this article, we introduced the concept of an extended rectangular fuzzy b-metric space which generalizes rectangular fuzzy b-metric space, fuzzy rectangular metric space, extended fuzzy b-metric space, fuzzy b-metric, fuzzy metric space and proved Ćirić type contraction results in such spaces. Our result extended the Banach fixed point theorem to fuzzy rectangular metric space [38] and to fuzzy rectangular b-metric space [34]. We studied the condition under which Cauchy sequence in an extended rectangular fuzzy b-metric space can have at most one limit point. We also provided an example to show that an extended rectangular fuzzy b-metric space is not Hausdorff. A result is presented to show that an extended rectangular fuzzy b-metric space is α-non-decreasing. An application to integral equation is presented here to support our main results.
The concepts presented in this paper could be employed to extend and unify the results in [40,41,42,43,44,45].
Conflict of interest
The authors declare to have no conflict of interest.












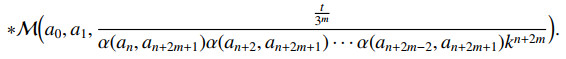




 DownLoad:
DownLoad: