Structure of a chain ring as a ring of matrices over a Galois ring

1.   Introduction

Fractional differential equations (FDEs) are precision tools to describe many nonlinear phenomena from porous media to other areas of scientific disciplines. Researchers have used different local and nonlocal fractional derivatives to model the phenomena around them. For example, Yang et al. ^[1] considered an advection-dispersion equation with the conformable derivative and obtained its analytical solutions using the Fourier transform. Hosseini et al. ^[2] studied the BiswasArshed model involving the beta derivative and derived its soliton waves through the Jacobi and Kudryashov techniques. In ^[3], the authors steered an analytical study on a Caputo time-fractional equation using a capable analytic scheme. In another paper, Sulaiman et al. ^[4] explored the coupled Burgers system involving the Mittag-Leffler kernel through the Laplace homotopy perturbation method. Generally, the most widely used fractional derivatives that have been adopted by many authors are the conformable derivative ^[5,6,7,8], the beta derivative ^[9,10,11,12], the Caputo derivative ^{[13,14,15,16]}, and the Atangana-Baleanu derivative ^{[17,18,19,20]}. For more information about the fractional derivatives, see ^{[21,22,23,24,25,26,27,28,29,30]}.

The $M$-fractional derivative is another type of fractional derivatives that lies in the class of the local fractional derivatives (Compared to the nonlocal fractional derivatives such as the Caputo fractional derivative). This local fractional derivative is a generalization of other local fractional derivatives like the conformable fractional derivative. The $M$-fractional derivative was first proposed by Sousa and Oliveira in ^[31] that encompasses a number of ordinary derivative properties such as linearity, product rule, etc. Sousa and Oliveira ^[31] also developed a series of classical results from the Rolle's theorem to other theorems in the $M$ sense. Such results led to the use of this well-behaved derivative in the studies of many researchers. In this respect, Yusuf et al. ^[32] gained solitons of the Ginzburg-Landau equation involving the $M$-fractional derivative using the generalized Bernoulli method. Özkan ^[33] used the simplest equation approach to derive exact solutions of Biswas-Arshed equation with the $M$-fractional derivatives. Tariq et al. ^[34] found optical solitons of Schrödinger-Hirota equation involving the $M$-fractional derivative through the Fan's method. Zafar et al. ^[35] tried to acquire optical solitons of Biswas-Arshed model with the $M$-fractional derivative using the sinh-Gordon method.

For $f:[0, \infty)\rightarrow \mathbb{R}$, the $M$-fractional derivative of $f$ of order $\alpha$ is given by ^[31]

where $x > 0$ and $\alpha \in (0, 1)$. Here, ${}_{i}{{E}_{\beta }}(.), \; \; \beta > 0$ is the Mittag-Leffler function ^[36]. If the $M$-fractional derivative of $f$ of order $\alpha$ exists, then it is said that $f$ is $\alpha$-differentiable. Note that for the $\alpha$-differentiable function $f$, one can define

provided that

exists.

It can be readily shown that for the $\alpha$-differentiable functions, the $M$-fractional derivative satisfies the following features ^[31]:

A. $_{i}D_{M}^{\alpha, \beta }\left(af+bg \right) = a\left(_{i}D_{M}^{\alpha, \beta }f \right)+b\; \left(_{i}D_{M}^{\alpha, \beta }g \right), \; a, b\in \mathbb{R}$.

B. $_{i}D_{M}^{\alpha, \beta }{{x}^{p}} = \frac{p}{\rm{ }\!\!\Gamma\!\!\rm{ (}\beta +1\rm{)}}{{x}^{p-\alpha }}, \; p\in \mathbb{R}$.

C. If $f(x) = c$, then $_{i}D_{M}^{\alpha, \beta }f = 0$.

D. $_{i}D_{M}^{\alpha, \beta }(fg) = g\left(_{i}D_{M}^{\alpha, \beta }f \right)+f\left(_{i}D_{M}^{\alpha, \beta }g \right)$.

E. $_{i}D_{M}^{\alpha, \beta }\left(\frac{f}{g} \right) = \frac{g\left(_{i}D_{M}^{\alpha, \beta }f \right)-f\left(_{i}D_{M}^{\alpha, \beta }g \right)}{{{g}^{2}}}$.

F. $_{i}D_{M}^{\alpha, \beta }\left(fog \right)(x) = {f}'\Big(g(x) \Big)_{i}D_{M}^{\alpha, \beta }g(x)$, where $f$ is differentiable at $g(x)$.

G. $_{i}D_{M}^{\alpha, \beta }f(x) = \frac{{{x}^{1-\alpha }}}{\rm{ }\!\!\Gamma\!\!\rm{ (}\beta +1\rm{)}}\frac{df}{dx}$.

H. If $a > 0$ and $f:[a, b]\to \mathbb{R}$ is continuous and $\alpha$-differentiable for some $\alpha \in (0, 1)$, then there is $c\in (a, b)$ such that

Abdeljawad ^[37] introduced the conformable power series and applied such a representaion for a group of certain functions. The main aim of the current paper is to introduce the power series based on the $M$-fractional derivative and prove some new theorems and corollaries regarding it.

The next sections of the present paper are as follows: In Section 2, the power series based on the $M$-fractional derivative is introduced. More peciesely, the Taylor and Maclaurin expansions are generalized for fractional-order differentiable functions in accordance with the $M$-fractional derivative. Furthermore, some new definitions, theorems, and corollaries regarding the power series in the $M$ sense are presented and formally proved, in this section. In Section 3, a number of ODEs with the $M$-fractional derivative are solved to examine the validity of the results presented. The paper totalizes the outcomes in Section 4.

2.   New definitions, theorems and corollaries

In the current section, some new definitions, theorems, and corollaries regarding the power series in the $M$ sense are presented and formally proved.

Definition 2.1. An infinite series

is called an $\alpha$-power series in ${{x}^{\alpha }}$. Additionally, the series

is known as an $\alpha$-power series in ${{x}^{\alpha }}-x_{0}^{\alpha }$ which is more general than the previous one.

Definition 2.2. An infinite $\alpha$-power series

is referred to as the $\alpha$-Taylor expansion of the function $f$ at $x_0$ provided that $f$ is infinitely $\alpha$-differentiable at $x_0$.

Definition 2.3. An infinite $\alpha$-power series

is known as the $\alpha$-Maclaurin expansion of the function $f$ provided that $f$ is infinitely $\alpha$-differentiable at ${{x}_{0}} = 0$.

Definition 2.4. A sequence $\{{{f}_{n}}\}$ is called convergent uniformly to $f$ on the set $E\subseteq \mathbb{R}$, if for every $\varepsilon > 0$, there exists an $N\in \mathbb{N}$ such that for all $n\ge N$ and all $x\in E$, $|{f}_{n}(x)-f(x)| < \varepsilon$.

Theorem 2.5. Assume $\{{{f}_{n}}\}$ converges uniformly to f on the set $E\subseteq \mathbb{R}$. Let $x$ be a limit point of $E$ and let $\lim\limits_{t\to x}\, {{f}_{n}}(t) = {{l}_{n}}$. Then, $\{{{l}_{n}}\}$ converges and $\lim\limits_{t\to x}\, f(t) = \lim\limits_{n\to \infty }\, {{l}_{n}}$. Particularly

For the proof, see ^[38,39].

Theorem 2.6. (Uniform convergence and the truncated $M$-fractional derivative) Let $0 < \alpha < 1$ and $a\ge 0$. Suppose $\{{{f}_{n}}\}$ is $M$-fractional differentiable on $(a, b)$ such that $\{{{f}_{n}}({{x}_{0}})\}$ converges for some point ${{x}_{0}}$ on $(a, b)$. If $\{{}_{i}D_{M}^{\alpha, \beta }{{f}_{n}}\}$ converges uniformly on $(a, b)$, then $\{{{f}_{n}}\}$ converges uniformly on $(a, b)$ to a function $f$ and for every $x\in (a, b)$, we have

Proof. Suppose $\varepsilon > 0$ and consider ${{N}_{1}}\in \mathbb{N}$ such that $m, n\ge {{N}_{1}}$. Now, $t\in (a, b)$ implies

and

If we apply the mean value theorem (H) to the function ${{f}_{m}}-{{f}_{n}}$ where $m, n\ge {{N}_{1}}$, from (2.1), we find

for every $x, t\in (a, b)$, where $z$ is a point between $x$ and $t$. Thus, for every $x\in (a, b)$ and $m, n\ge {{N}_{1}}$, the following

implies that $\{{{f}_{n}}\}$ converges uniformly on $(a, b)$. Let $f(x) = \lim\limits_{n\to \infty }\, {{f}_{n}}(x)$ and $x\in (a, b)$.

Let us fix a point $c$ on $(a, b)$. Suppose that

then

Thus, there exists a positive number $\gamma$ such that

for $0 < |\lambda | < \gamma$. Furthermore, there exists ${{N}_{2}}\in \mathbb{N}$ such that $m, n\ge {{N}_{2}}$. Now, $x\in (a, b)$ implies

Now, for $0 < |\lambda | < \gamma$, one can define

Since $\lim\limits_{n\to \infty }\, {{g}_{n}}(\lambda) = g(\lambda)$ and $\lim\limits_{\lambda \to 0}\, {{g}_{n}}(\lambda) = {}_{i}D_{M}^{\alpha, \beta }{{f}_{n}}(c)$, for $m, n\ge {{N}_{2}}$, we have

where $z$ is a point between $0$ and $\lambda$. This shows $\{{{g}_{n}}\}$ converges uniformly to $g$ on $0 < |\lambda | < \gamma$. Theorem 1 implies that $\lim\limits_{\lambda \to 0}\, g(\lambda)$ exists and $\lim\limits_{\lambda \to 0}\, g(\lambda) = \lim\limits_{n\to \infty }\, \lim\limits_{\lambda \to 0}\, {{g}_{n}}(\lambda)$. This means that ${}_{i}D_{M}^{\alpha, \beta }f(c)$ exists and

Corollary 2.7. In Theorem 2, if for every $n\in \mathbb{N}$, ${{f}_{n}}$ is differentiable in the usual context, then property (G) implies

for all $x\in (a, b)$.

Theorem 2.8. Suppose that $0 < \alpha < 1$, $0 < R\le x_{0}^{\alpha }$ and the $\alpha$-power series

converges on $I=\Big({{\Big(x_{0}^{\alpha }-R\Big)}^{\frac{1}{\alpha }}}, {{\Big(x_{0}^{\alpha }+R\Big)}^{\frac{1}{\alpha }}}\Big)$, and $f(x)=\sum\limits_{n=0}^{\infty }{{{a}_{n}}{{({{x}^{\alpha }}-x_{0}^{\alpha })}^{n}}}$ for $x\in I$. Then the series

converges uniformly on every closed interval of $I$. The function $f$ is continuous and $\alpha$-differentiable in $I$, and

Proof. Suppose $[a, b]\subseteq I$ and $p$ is a point in $[a, b]$ such that for every $x\in [a, b]$, $|{{x}^{\alpha }}-x_{0}^{\alpha }|\le |{{p}^{\alpha }}-x_{0}^{\alpha }|$. Then,

for all $x\in [a, b]$. Since

converges absolutely, the Weierstrass $M$-test yields the uniform convergence of the series

on $[a, b]$. Since

the series

and

have a similar interval of convergence. Accordingly

converges uniformly on every $[a, b]\subseteq I$. Now, if

then

Since the sequences $\{{{s}_{n}}\}$ and $\{{}_{i}D_{M}^{\alpha, \beta }{{s}_{n}}\}$ converge uniformly on $[a, b]$, they also converge uniformly on $(a, b)$. Therefore, Theorem 2 and its corollary imply that ${}_{i}D_{M}^{\alpha, \beta }f(x)$ exists on $(a, b)$ and

But, for any $x$ which $x\in I$, there exists a closed interval $[a, b]$ such that $x\in (a, b)\subseteq [a, b]\subseteq I$. This reveals that ${}_{i}D_{M}^{\alpha, \beta }f(x)$ exists for any $x\in I$ and

The continuity of $f$ is yielded from the existence of ${}_{i}D_{M}^{\alpha, \beta }f$.

Corollary 2.9. Under the hypotheses of Theorem 3, $f$ has $M$-fractional derivatives of all orders in

which are given by

In particular

Corollary 2.10. Suppose $0 < \alpha < 1$, $R > 0$, and the $\alpha$-power series

converges on $\left(0, {{R}^{\frac{1}{\alpha }}}\right)$, and $f(x) = \sum\limits_{n = 0}^{\infty }{{{a}_{n}}{{x}^{n\alpha }}}$, where $0 < x < {{R}^{\frac{1}{\alpha }}}$. Then, the series

converges uniformly on every closed interval of $\left(0, {{R}^{\frac{1}{\alpha }}}\right)$. The function $f$ is continuous and $\alpha$-differentiable in $\left(0, {{R}^{\frac{1}{\alpha }}}\right)$, and

It is easy to show that

Since $\lim\limits_{x\to {{0}^{+}}}\, {}_{i}^{k}D_{M}^{\alpha, \beta }f(x)$ exists for $k = 0, 1, \cdots$,

Corollary 2.11. If two $\alpha$-power series

represent the same function in a neighborhood, then ${{a}_{n}} = {{b}_{n}}$ for all $n$. This means that the $\alpha$-power series expansion of a function about a given point is uniquely determined.

3.   Applications

In this section, by using the $\alpha$-power series method, several linear and nonlinear ODEs with the $M$-fractional derivative are solved to examine the validity of the results presented in the current study.

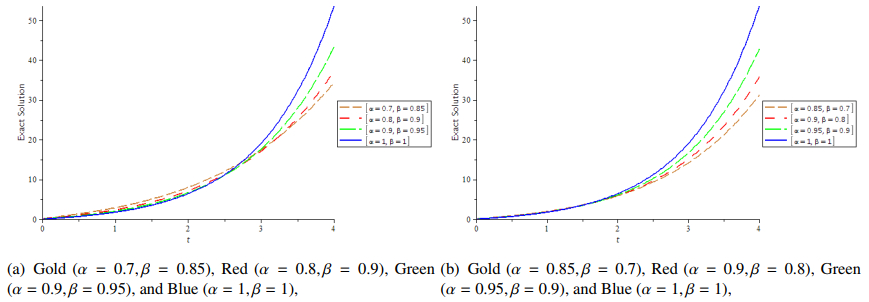
Example 3.1. Firstly, we deal with a problem involving the $M$-fractional derivative as ^[40]

where the exact solution of Eq (3.1) is

According to Section 2, we adopt a solution for Eq (3.1) as

By substituting Eq (3.2) into (3.1) and simplifying the resulting expression, we get

and so

By performing some simple operations, we achieve

From (3.3), it is clear that

By applying the above coefficients in Eq (3.2), the solution of Eq (3.1) is derived as

or

converging to

The exact solution of Eq (3.1) for different sets of $\alpha$ and $\beta$ has been plotted in Figure 1.

Example 3.2. Secondly, we want to deal with a problem with the $M$-fractional derivative as

where the exact solution of Eq (3.4) is

Based on Section 2, the solution of Eq (3.4) is assumed to be

By setting Eq (3.5) in (3.4) and simplifying the resulting expression, we find

or

Applying some simple operations, we obtain

From (3.6), it is found that

Inserting the above coefficients into Eq (3.5) leads to

or

The exact solution of Eq (3.4) for different values of $\beta$ has been portrayed in Figure 2.

Example 3.3. Thirdly, we deal with a problem involving the $M$-fractional derivative as

which has the following exact solution

According to Section 2, we adopt a solution for Eq (3.7) as

By substituting Eq (3.8) into (3.7) and simplifying the resulting expression, we get

or

By performing some simple operations, we achieve

From (3.9), it is clear that

By applying the above coefficients in Eq (3.8), the solution of Eq (3.7) is derived as

converging to

The exact solution of Eq (3.7) for different sets of $\alpha$ and $\beta$ has been plotted in Figure 3.

Example 3.4. In the end, we will deal with a problem with the $M$-fractional derivative as

which has the following exact solution

Based on Section 2, the solution of Eq (3.10) is supposed to be

By setting Eq (3.11) in (3.10) and simplifying the resulting expression, we find

or

Through applying some simple operations, we obtain

From (3.12), it is found that

Inserting the above coefficients into Eq (3.11) leads to

or

The exact solution of Eq (3.10) for different sets of $\alpha$ and $\beta$ has been portrayed in Figure 4.

4.   Conclusions

The key goal of the current paper was to conduct a new investigation on ordinary differential equations involving the $M$-fractional derivative. In this respect, first, the $\alpha$-Taylor expansion and the $\alpha$-Maclaurin expansion were established based on the $M$-fractional derivative. Then, several definitions, theorems, and corollaries regarding the power series in the $M$ sense were given and successfully proved. To examine the effectiveness of the results provided in the present work, some ordinary differential equations involving the $M$-fractional derivative were solved. The Maple package as a worthwhile tool was formally adopted to deal with symbolic computations. As a possible future work, the authors will apply the power series in the $M$ sense to solve other well-known ODEs involving the $M$-fractional derivative.

Conflict of interest

All authors declare no conflicts of interest in this paper.