1.
Introduction
In today's world mathematics is necessary in all fields. It is an essential instrument for comprehension around us. It covers all the facts of life. Mathematics is the branch of science that deals with the reasoning of figures, numbers and order. In our daily life, we use mathematics in our routine work in various forms. There are many branches of mathematics like algebra, geometry, arithmetic, trigonometry, analysis and many other theories. Graph theory is the study of mathematical objects known as graph, which consist of vertices connected by edges. It is the mathematical theory which deals with the properties and applications of graphs. When we apply graph theory on chemistry then it is called chemical graph theory. In mathematical models graph theory is used to get a deep understanding of the physical properties of these chemical compounds. Some physical properties such as boiling point, melting point, density are associated to geometrical structure of the compound. Now a days, several ways are used in mathematical chemistry to understand chemical structure which are existing behind the chemical concepts, to create and inquire novel mathematical representation. In complete history of chemistry certain scientist, usually contemplates connections between mathematics with chemistry and the possibility of using mathematics to analyze and predict new chemical concepts. Mufti defined sanskruti and harmonic indices of certain graph structure [25]. Babujee calculated topological indices and new graph structures in 2012 [5]. Farahani worked on a new version of zagreb index of circumcoronene series of benzenoid in 2013 [7]. Hayat defined some degree based topological indices of certain nanotubes [8]. Imran worked on topological indices of certain interconnection networks in 2014 [14]. In 2016, Siddiqui computed zagreb indices and zagreb polynomials of some nanostars dendrimers [27]. Saleem computed retractions and homomorphisms on some operations of graphs [28]. Iqbal calculated eccentricity based topological indices of some benzenoid structures [12]. Yang examined two-point resistances and random walks on stellated regular graphs [29]. Islam defined M-polynomial and entropy of paraline graph of Napthalene in 2019 [11]. Iqbal worked on topological indices of subdivided and line graph of subdivided friendship graph [13]. In 2020, Afzal examined M-polynomial and topological indices of zigzag edge coronoid fused by starphene [1]. Archdeacon defined the medial graph and voltage-current duality [2]. Munir computed M-polynomial and degree-based topological indices of polyhex nanotubes [22]. Iqbal calculated ve topological indices of tickysim spinnaker model [15]. Azhar examined a note on valency dependence invariants of L(G(K)) Graph [4]. Jamil defined the first general zagreb eccentricity index [18]. Iqbal defined ABC4 and GA5 index of subdivided and line graph of subdivided dutch windmill graph [16]. Maji worked on the first entire zagreb index of various corona products and their bounds [23]. Imran describe computation of topological indices of NEPS of graphs [17]. Hayat computed topological indices for networks derived by applying graph operations from honeycomb structures [9], based on this idea we have computed the topological indices for transformed structures. Next we have few definitions [3,9]:
Definition 1:
Let K be a simple connected graph its M-polynomial is defined as;
where: S = Min{dβ|β∈V(K)}, T = Max{dβ|β∈V(K)}, and mab(K) is the number of edges γβ∈E(K) such that {dγ, dβ} = {a,b}.
Definition 2:
When we place a new vertex in each face of a planar graph G, and attach that vertex with all the vertices of the respective face of G, we get the stellation of G and denote it as St(G).
Definition 3:
We introduce a node in each edge of the graph and join the nodes if their corresponding edges are adjacent and is denoted by Md(G).
Definition 4:
We introduce a node in each bounded faces of the graph and join the nodes by an edge if the faces share an edge in graph it is called bounded dual. It is represented as Bdu(G).
T. Réti introduced First and Second Zagreb indices are [26],
Second modified Zagreb index is defined as [10],
Symmetric division and reciprocal general Randic index is [19],
Whereas, the General Randic index defined as [24],
where α is an arbitrary real number.
In 1998, the Atomic Bond Connectivity Index is defined as [6],
Geometric-Arithmetic index is [30],
In 2015, the General Version of Harmonic Index is defined [31],
First and Second Gourava Indices were introduced by Kalli which is defined as, respectively [20],
Kalli introduced the First and Second Hyper-Gourava Indices which is defined as, respectively [21],
2.
Methodology
At first, we obtain transformed pattern of molecular structures zigzag and triangular benzenoid named as T1 and T2. Next we define M-polynomial and topological indices of these networks by applying the stellation, medial and bounded dual. Further we define edge partition depending upon degree based vertices of T1 and T2 and then calculate the indices.
3.
Results and discussion
3.1. M-polynomial and degree based topological indices of T1 structure
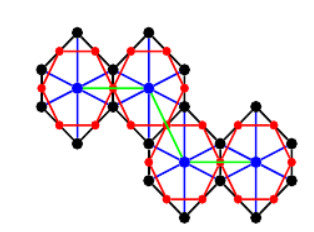
Now we will construct the stellation, medial and bounded dual operations on simple and undirected zigzag benzenoid structure. We get a new transformed network (say) T1, shown in Figure 1 for n = 2. After this we will compute M-polynomial and degree based topological indices of our newly obtained network. Where, blue is stellation, red is medial, green is bounded dual operations applied on T1.
Following Table 3 shows the types of edges and their count for network T1; n≥2. Now, we will calculate the M-polynomial and vertex degree based topological indices namely first Zagreb index, second Zagreb index, second modified index, symmetric division index, general Randic index, reciprocal general Randic index, atomic bond connectivity index, geometric arithmetic index, general version of harmonic index, first and second gourava indices, first and second hyper gourava indices.
3.1.1. Theorem
Let T1, be the transformed network then its M-polynomial is
Proof:
Using definition of M-polynomial and information from Table 3, we have
3.1.2. Theorem
Let T1, be the transformed network and
be its M-polynomial. Then, the first Zagreb index M1(T1), the second Zagreb index M2(T1), the second modified Zagreb index mM2(T1), the general Randic index Rα(T1), where α ϵ N, reciprocal general Randic index RRα(T1), where α ϵ N, and the symmetric division degree index SDD(T1) obtained from M-polynomial are as follows:
Proof: Let f(x,y) = M(T1;x,y) be the M-polynomial of the transformed network T1. Then
Now, the required partial derivatives and integrals are obtained as:
By using the information given in Table 3 and formulas for Table 1;
Consequently,
3.1.3. Theorem
For T1, the Atomic Bond Connectivity, Geometric Arithematic Index and General Harmonic Index are as follows, respectively.
Proof:
1). According to Eq (1.7)
By using the information given in Table 3.
2). According to Eq (1.8)
By using the information given in Table 3.
3). According to Eq (1.9)
By using the information given in Table 3.
3.1.4. Theorem
For T1, the First, Second Gourava Indices and the First, Second Hyper Gourava Indices are as follows, respectively.
Proof:
1). According to Eq (1.10)
By using the information given in Table 3.
2). According to Eq (1.11)
By using the information given in Table 3.
3). According to Eq (1.12)
By using the information given in Table 3.
4). According to Eq (1.13)
By using the information given in Table 3.
3.2. M-polynomial and degree based topological indices of T2 structure
Now we will construct the stellation, medial and bounded dual operations on simple and undirected triangular benzenoid structure. We get a new transformed network (say) T2, shown in Figure 3 for n = 5. After this we will compute M-polynomial and degree based topological indices of our newly obtained network. Where, blue is stellation, red is medial, green is bounded dual operations applied on T2.
Following Table 4 shows the types of edges and their count for network T2; n≥5. Now, we will calculate the different M-polynomial and vertex degree based topological indices namely first Zagreb index, second Zagreb index, second modified index, symmetric division index, general Randic index, reciprocal general Randic index, atomic bond connectivity index, geometric arithmetic index, general version of harmonic index, first and second gourava indices, first and second hyper gourava indices.
3.2.1. Theorem
Let T2, be the transformed network then its M-polynomial is
Proof: Using definition of M-polynomial and information from Table 4, we have
3.2.2. Theorem
Let T2, be the transformed network and
be its M-polynomial. Then, the first Zagreb index M1(T2), the second Zagreb index M2(T2), the second modified Zagreb mM2(T2), the general Randic index Rα(T2), where α ϵ N, reciprocal general Randic index RRα(T2), where α ϵ N, and the symmetric division degree index SDD(T2) obtained from M-polynomial are as follows:
Proof: Let f(x,y) = M(T2;x,y) be the M-polynomial of the transformed network T2. Then
Now, the required partial derivatives and integrals are obtained.
By using the information given in Table 4 and formulas for Table 1:
Consequently,
3.2.3. Theorem
For T2, the Atomic Bond Connectivity, Geometric Arithmetic Index and General Harmonic Index are as follows, respectively.
Proof: Using Eqs 1.7–1.9 and Table 4, we get the results 1–3.
3.2.4. Theorem
For T2, the First, Second Gourava Indices and the First, Second Hyper Gourava Indices are as follows, respectively.
Proof: Using Eqs 1.10–1.13 and Table 4 we get the above results 1–4.
4.
Conclusions
In this paper, we defined M-polynomial of the transformed zigzag benzenoid and transformed triangular benzenoid structures by applying stellation, medial and bounded dual operations to get networks named as T1 and T2. With the help of M-polynomial, we computed certain degree-based topological indices such as first Zagreb index, second Zagreb index, second modified Zagreb index, general Randic index, reciprocal general Randic index, symmetric division degree index. We also computed atomic bound connectivity index, geometric arithmetic index, general harmonic index, first and second gourava indices, first and second hyper gourava indices. M-polynomial is used to calculate the certain degree based topological indices as a latest developed instrument in the chemical graph theory. In future, we can compute other indices on these structures and some additional transformed structures can be studied for a variety of topological indices to have an insight about their properties.
Acknowledgments
This work was supported by the Key teaching research project of quality engineering in Colleges and universities in Anhui Province. Anhui Vocational college of Electronics and Information Technology Guangzhou Zhuoya Education Investment Co., Ltd. Practical Education Base (subject No: 2020sjjd093). The authors are very thankful for this research funding. We are also very grateful to the reviewers for their valuable suggestions.
Conflict of interest
The authors declare no conflict of interest.









 DownLoad:
DownLoad:







