The finite time blow-up for Caputo-Hadamard fractional diffusion equation involving nonlinear memory

1.   Introduction

The main purpose of this paper is to study the finite time blow-up to time-space fractional partial differential equation in the following form

where $d\in \mathbb{N}$, $0 < \alpha < \gamma < 1$, $0 < s < 1$, $p > 1$, the operators $_{CH}{\rm{D}}_{a, t}^{\alpha}$, $(-\Delta)^{s}$, and $_{H}{\rm{D}}^{-(1-\gamma)}_{a, t}$ respectively denote the Caputo-Hadamard fractional derivative, fractional Laplacian, and Hadamard fractional integral, and the initial value $u_{a}({\rm{x}})\in C_{0}(\mathbb{R}^{d})$, where $C_{0}(\mathbb{R}^{d}) = \{\upsilon\in C(\mathbb{R}^{d})\, |\, \lim\limits_{|{\rm{x}}|\rightarrow \infty}\upsilon({\rm{x}}) = 0\}$.

Fractional calculus has attracted considerable attention during recent years because of its widespread applications in science and engineering fields such as physics, chemistry, biology, anomalous diffusion, control theory of dynamical systems, etc., see ^[1,2,3,4]. It has been found that Hadamard-type fractional calculus had many potential applications ^{[5,6,7,8,9,10,11]}, for example, the ultraslowly diffusive process such as Sinai diffusion ^[5], fractal analysis ^[8], the Lomnitz logarithmic creep law in rheology ^[9], and some studies in this respect have been available ^{[12,13,14,15,16,17,18,19]}. The fractional Laplacian is a typically nonlocal pseudo-differential operator, which appears in different disciplines of mathematics and various applications, see ^{[20,21,22,23]} and the list of references therein.

We next recall some pioneering work on the blow-up problem for fractional diffusion equation, here we only mention the results related to our studies.

In the 1960s, Fujita ^[24] first considered the following semilinear heat equation

where $\alpha > 0$ and $u_{0}({\rm{x}})\geq 0$. In that paper, the author shown that: If $u_{0}({\rm{x}})\not\equiv 0$ and $0 < \alpha < \frac{2}{d}$ then the solution of (1.2) blows up in finite time; If $\alpha > \frac{2}{d}$ and the initial value $u_{0}({\rm{x}})$ can be bounded by sufficiently small Gaussian then the solution of (1.2) exists globally. As for the critical case $\alpha = \frac{2}{d}$, Weissler ^[25] proved that (1.2) has a global solution when $||u_{0}||_{L^{\frac{\alpha d}{2}}(\mathbb{R}^{d})}$ is sufficiently small.

Later, Cazenave et al. ^[26] studied the following Cauchy problem of heat equation with nonlinear memory

where $p > 1, 0\leq \gamma < 1$, and $u_{0}\in C_{0}(\mathbb{R}^{d})$. Let $p_{\gamma} = \frac{1+2(2-\gamma)}{(d-2+2\gamma)_{+}}$ with $(d-2+2\gamma)_{+} = \max\{0, d-2+2\gamma\}$. They proved that: If $\gamma \neq 0, p\leq \max\{\frac{1}{\gamma}, p_{\gamma}\}, u_{0}\geq 0$, and $u_{0}\not\equiv 0$, then the solution of (1.3) blows up in finite time; if $\gamma \neq 0, p > \max\{\frac{1}{\gamma}, p_{\gamma}\}$ and $||u_{0}||_{L^{q_{sc}}(\mathbb{R}^{d})}$ is sufficiently small with $q_{sc} = \frac{d(p-1)}{4-2\gamma}$, then (1.3) has global solution. In the case with $\gamma = 0$, every nontrivial positive solution of (1.3) will blow up ^[27].

In ^[28], Fino and Kirane further investigated the equation involving fractional Laplacian with nonlinear memory

where $0 < \beta \leq 2, 0 < \gamma < 1, p > 1$, and $u_{0}\in C_{0}(\mathbb{R}^{d})$. They derived that: If $u_{0}\geq 0, u_{0}\not\equiv 0$, and $p\leq \max\{1+\frac{\beta(2-\gamma)}{(d-\beta+\beta\gamma)_{+}}, \frac{1}{\gamma}\}$, then the solution of (1.4) will blow up in finite time; if $p > \max\{1+\frac{\beta(2-\gamma)}{(d-\beta+\beta\gamma)_{+}}, \frac{1}{\gamma}\}$ and $||u_{0}||_{L^{p_{sc}}(\mathbb{R}^{d})}$ is very small with $p_{sc} = \frac{d(p-1)}{\beta(2-\gamma)}$, then (1.4) exists global solution.

Shortly after, Li and Zhang ^[29] discussed the following time fractional diffusion equation involving Caputo derivative with nonlinear memory

where $0 < \alpha < \gamma < 1$, $p > 1$, and $u_{0}\in C_{0}(\mathbb{R}^{d})$. They proved that: If $1 < p < p^{*} = \max\{1+\frac{1-\gamma}{\alpha}, 1+\frac{2(1+\alpha-\gamma)}{\alpha d}\}$ and $u_{0}\geq 0$ with $u_{0}\not\equiv 0$, then the solution of (1.5) will blow up in finite time; if $d < \frac{2(1+\alpha-\gamma)}{1-\gamma}$ with $p\geq p^{*}$ or $d\geq \frac{2(1+\alpha-\gamma)}{1-\gamma}$ with $p > p^{*}$, and $||u_{0}||_{L^{q_{c}}(\mathbb{R}^{d})}$ is small enough, where $q_{c} = \frac{\alpha d(p-1)}{2(1+\alpha-\gamma)}$, then (1.5) has global solution.

Recently, Li and Li ^[16] investigated the semilinear time-space fractional diffusion equation involving Caputo-Hadamard derivative and fractional Laplacian,

where $0 < \alpha < 1$, $0 < s < 1$, $p > 1$, and $u_{a}\in C_{0}(\mathbb{R}^{d})$. They obtained that: If $1 < p < \frac{2s}{d}$ and $u_{a}\geq 0$ with $u_{a}\not\equiv 0$, then the solution of (1.6) will blow up in finite time; Conversely, if $p\geq 1+\frac{2s}{d}$ and $||u_{a}||_{L^{q{*}}(\mathbb{R}^{d})}$ is sufficiently small, where $q_{*} = \frac{d(p-1)}{2s}$, then (1.6) has a global solution.

Motivated mathematically by the results and methods in ^[16], this paper will further study the blow-up property and global solution to time-space fractional diffusion equation (1.1) with nonlinear memory. The main result is displayed in the following theorem.

Theorem 1.1. Let $d\in \mathbb{N}$, $0 < \alpha < \gamma < 1$, $0 < s < 1$, and $p > 1$.Assume that $u_{a}\in C_{0}(\mathbb{R}^{d})$ and $u_{a}\geq 0$ with $u_{a}\not\equiv 0$.

(1) If $1 < p < \widetilde{p} = \max\{1+\frac{1-\gamma}{\alpha}, 1+\frac{2s(1+\alpha-\gamma)}{\alpha d}\}$, then the mild solution of Eq (1.1) will blow up in finite time.

(2) If $d < \frac{2s(1+\alpha-\gamma)}{1-\gamma}, p\geq \widetilde{p}$ or $d\geq \frac{2s(1+\alpha-\gamma)}{1-\gamma}, p > \widetilde{p}$, and $||u_{a}||_{L^{p^{*}}(\mathbb{R}^{d})}$ is small enough with $p^{*} = \frac{\alpha d(p-1)}{2s(1+\alpha-\gamma)}$, then Eq (1.1) exists global solution.

The organization of this paper is as follows. Section 2 recalls some basic definitions and presents several important lemmas. In Section 3, we define a mild solution to Eq (1.1) and then prove the local existence and uniqueness of the mild solution. Then, a weak solution of Eq (1.1) is introduced and the mild solution is actually proved to be a weak solution. Next, we show the finite time blow-up and global existence of the solution to Eq (1.1) in Section 4. Finally, an illustrative example is provided to verify the blow-up of solution in finite time in Section 5. The conclusions are given in the last section. Throughout the paper, we use the letter $C$ to denote a generic positive constant which may take different values at different places.

2.   Preliminaries

Let us recall some basic definitions and several important lemmas, which will be applied in the next sections.

Definition 2.1. ^[4,30] Let a function $f(t)$ be defined on the interval $(a, b)\, (0\leq a < b\leq +\infty)$ and $\alpha > 0$. The left- and right- sided Hadamard fractional integrals of the function $f(t)$ with order $\alpha$ are given by

and

where the Gamma function $\Gamma(\xi) = \int_{0}^{\infty}e^{-t}t^{\xi-1}{\rm{d}}t$.

Definition 2.2. ^[4,31] Let a function $f(t)$ be defined on the interval $(a, b)\, (0\leq a < b\leq +\infty)$ and $n-1 < \alpha < n\in \mathbb{N}$. The left- and right- sided Caputo-Hadamard fractional derivative of the function $f(t)$ with order $\alpha$ can be written as

and

where $\delta^{n}f(t) = \left(t\frac{{\rm{d}}}{{\rm{d}}t}\right)^n f(t)$.

Definition 2.3. ^[2,20,30] The fractional Laplacian $(-\Delta)^{s}$ with $s\in (0, 1)$ is defined by

where P.V. denotes the Cauchy principle value and the constant

for any ${\rm{y}} = (y_{1}, y_{2}, \cdots, y_{d})\in \mathbb{R}^{d}$.

To define a mild solution of Eq (1.1), let us consider the following linear equation,

whose solution is expressed by ^[14]

where $G_{a}({\rm{x}}, t)$ and $G_{f}({\rm{x}}, t)$ are the fundamental solutions given by

and

The special function $H_{23}^{21}(z)$ in the above equalities is the Fox $H$-function and some details regarding this function can be found in ^[4,32,33].

In the sequel, we list some properties of the functions $G_{a}({\rm{x}}, t)$ and $G_{f}({\rm{x}}, t)$.

Lemma 2.1. ^[16] Let $d\in \mathbb{N}$, $0 < \alpha < 1$, and $0 < s < 1$. Thenthe functions $G_{a}({\rm{x}}, t)$ and $G_{f}({\rm{x}}, t)$ in Eqs (2.8) and (2.9) have the following properties.

(1) $G_{a}({\rm{x}}, t) > 0$, $G_{f}({\rm{x}}, t) > 0$.

(2) $\int_{\mathbb{R}^{d}}G_{a}({\rm{x}}, t){\rm{d}}{\rm{x}} = 1$, $\int_{\mathbb{R}^{d}}G_{f}({\rm{x}}, t){\rm{d}}{\rm{x}} = \frac{1}{\Gamma(\alpha)}\left(\log \frac{t}{a}\right)^{\alpha-1}$. (3) $_{H}{\rm{D}}^{-(1-\alpha)}_{a, t}G_{f}({\rm{x}}, t) = G_{a}({\rm{x}}, t)$.

Lemma 2.2. ^[16] Let $d\in \mathbb{N}$, $0 < \alpha < 1$, and $0 < s < 1$.If $u_{a}({\rm{x}})\geq 0$ and $u_{a}({\rm{x}})\not\equiv 0$, then we have $G_{a}({\rm{x}}, t)*u_{a}({\rm{x}}) > 0$ and $||G_{a}({\rm{x}}, t)*u_{a}({\rm{x}})||_{L^{1}(\mathbb{R}^{d})} = ||u_{a}({\rm{x}})||_{L^{1}(\mathbb{R}^{d})}$.Furthermore, when $1\leq r\leq q\leq +\infty$ and $\frac{1}{r}-\frac{1}{q} < \min\{1, \frac{2s}{d}\}$, it holds that

Lemma 2.3. ^[16] Let $d\in \mathbb{N}$, $0 < \alpha < 1$, and $0 < s < 1$.If $u_{a}({\rm{x}})\geq 0$ and $u_{a}({\rm{x}})\not\equiv 0$, then we have $G_{f}({\rm{x}}, t)*u_{a}({\rm{x}}) > 0$ and $||G_{f}({\rm{x}}, t)*u_{a}({\rm{x}})||_{L^{1}(\mathbb{R}^{d})} = \frac{1}{\Gamma(\alpha)}\left(\log\frac{t}{a}\right)^{\alpha-1}||u_{a}({\rm{x}})||_{L^{1}(\mathbb{R}^{d})}$.Furthermore, when $1\leq r\leq q\leq +\infty$ and $\frac{1}{r}-\frac{1}{q} < \min\{1, \frac{4s}{d}\}$, it holds that

Lemma 2.4. ^[16] Let $d\in \mathbb{N}$, $0 < \alpha < 1$, and $0 < s < 1$. Assume $u_{a}({\rm{x}})\in C_{0}(\mathbb{R}^{d})$. Then for $t > a > 0$, we have $G_{a}({\rm{x}}, t)*u_{a}({\rm{x}})\in C_{0}(\mathbb{R}^{d})$ and

And there exists a constant $C > 0$ such that

For simplicity of representation, from now on, we denote $G_{a}(t) = G_{a}({\rm{x}}, t)$, $G_{f}(t) = G_{f}({\rm{x}}, t)$, and so on.

Lemma 2.5. ^[16] Let $d\in \mathbb{N}$, $0 < \alpha < 1$, $0 < s < 1$, and $T > a > 0$.Let also $f\in L^{q}((a, T), C_{0}(\mathbb{R}^{d}))$ with $q > 1$ and

Then we have

Furthermore, one has $\theta(t)\in C([a, T], C_{0}(\mathbb{R}^{d}))$ provided that $q\alpha > 1$.

3.   The mild solution and weak solution

In this part, we first define a mild solution of Eq (1.1) and then prove the local existence and uniqueness of the mild solution in terms of the contraction mapping principle. Next, the definition of a weak solution is introduced to Eq (1.1). We can also prove that the mild solution is just a weak solution. Let us begin by introducing the definition of a mild solution to Eq (1.1).

Definition 3.1. Let $d\in \mathbb{N}$, $0 < \alpha < \gamma < 1$, $0 < s < 1$, $p > 1$ and $T > a > 0$. Let $u_{a}\in C_{0}(\mathbb{R}^{d})$. Then a mild solution $u\in C([a, T], C_{0}(\mathbb{R}^{d}))$ of Eq (1.1) is given by

Theorem 3.1. Let $d\in \mathbb{N}$, $0 < \alpha < \gamma < 1$, $0 < s < 1$, $p > 1$, and $T > a > 0$.Let $u_{a}\in C_{0}(\mathbb{R}^{d})$. Then there isa maximal time $T_{\max} > a$ such that Eq (1.1) hasa unique mild solution $u\in C([a, T_{\max}), C_{0}(\mathbb{R}^{d}))$, where, either $T_{\max} = \infty$ or $T_{\max} < \infty$ and $||u||_{L^{\infty}((a, T), L^{\infty}(\mathbb{R}^{d})}\rightarrow \infty$ as $t\rightarrow T_{\max}^{-}$. Moreover, if $u_{a}\geq 0$ and $u_{a}\not\equiv 0$, then $u(t) > 0$ for any $a < t < T_{\max}$. Besides, if $u_{a}\in L^{r}(\mathbb{R}^{d})$ for $1\leq r < \infty$, then one has $u\in C([a, T_{\max}), L^{r}(\mathbb{R}^{d}))$.

Proof. For given $T > a > 0$ and $u_{a}\in C_{0}(\mathbb{R}^{d})$, let

and

Obviously, $(E_{a, T}, d)$ is a complete metric space. By means of the fundamental solutions $G_{a}(t)$ and $G_{f}(t)$, we define the following operator $\mathscr{F}$ on the metric space $(E_{a, T}, d)$,

It follows from Lemma 2.5 that $\mathscr{F}(u)\in C([a, T], C_{0}(\mathbb{R}^{d}))$.

We next show that $\mathscr{F}: E_{a, T}\rightarrow E_{a, T}$. For $u\in E_{a, T}$ and $t\in [a, T]$, by Definition 2.1 and Lemma 2.1, we get

Choosing $T > a$ sufficiently close to $a$ such that

then we obtain $||\mathscr{F}(u)||_{L^{\infty}((a, T), L^{\infty}(\mathbb{R}^{d}))} \leq 2||u_{a}||_{L^{\infty}(\mathbb{R}^{d})}$ and $\mathscr{F}(u)\in E_{a, T}$, viz., the operator $\mathscr{F}$ maps $E_{a, T}$ into itself.

We need to show that the operator $\mathscr{F}$ is contractive on $E_{a, T}$. For $u, v\in E_{a, T}$ and $t\in [a, T]$, one can deduce that

Taking $T > a$ sufficiently close to $a$ gives rise to

which means $||\mathscr{F}(u)(t)-\mathscr{F}(v)(t)||_{L^{\infty}(\mathbb{R}^{d})} \leq \frac{1}{2}||u-v||_{L^{\infty}((a, T), C_{0}(\mathbb{R}^{d}))}$. This illustrates the operator $\mathscr{F}$ is contractive on $E_{a, T}$ and thus it has a fixed point $u\in E_{a, T}$ by the contraction mapping principle. Moreover, using Gronwall inequality immediately knows the uniqueness of the mild solutions to Eq (1.1) holds.

In view of the uniqueness, there is a maximal time $T_{\max} > a$ such that the solution of Eq (1.1) exists on the interval $[a, T_{\max})$, where

Next, we show $||u||_{L^{\infty}((a, T), L^{\infty}(\mathbb{R}^{d}))}\rightarrow \infty$ as $t\rightarrow T_{\max}^{-}$ provided that $T_{\max} < \infty$. If $T_{\max} < \infty$ and there is $M > 0$ satisfying $||u(t)||_{L^{\infty}(\mathbb{R}^{d})}\leq M$ for $t\in [a, T_{\max})$, then we have for $a < \xi < \eta < T_{\max}$,

which implies $\lim\limits_{t\rightarrow T_{\max}^{-}}u(t)$ exists in $C_{0}(\mathbb{R}^{d})$.

Now we define $\lim\limits_{t\rightarrow T_{\max}^{-}}u(t) = u_{T_{\max}}$. Therefore one gets $u\in C([a, T_{\max}], C_{0}(\mathbb{R}^{d}))$. Furthermore, using Lemma 2.5 yields that

For $h > 0$ and $\sigma > 0$, consider a set

equipped with

Then the metric space $(\widetilde{E}_{h, \sigma}, d)$ is complete.

On the space $(\widetilde{E}_{h, \sigma}, d)$, define an operator $\mathscr{Q}$ as follows,

It is easy to see that $\mathscr{Q}(v)\in C([T_{\max}, T_{\max}+h], C_{0}(\mathbb{R}^{d}))$ and $\mathscr{Q}(v)(T_{\max}) = u_{T_{\max}}$.

We first prove $\mathscr{Q}(v)\in \widetilde{E}_{h, \sigma}$ for $v\in \widetilde{E}_{h, \sigma}$. As a matter of fact, if $t\in [T_{\max}, T_{\max}+h]$, then

By taking sufficiently small $h$, we arrive at

In regard to $||J_{2}||_{L^{\infty}(\mathbb{R}^{d})}$, one has

for $t\in [T_{\max}, T_{\max}+h]$ and $h$ small enough. Therefore, there holds $||\mathscr{Q}(v)(t)-u_{T_{\max}}||_{L^{\infty}(\mathbb{R}^{d})}\leq \sigma$, i.e., $d(\mathscr{Q}(v), u_{T_{\max}})\leq \sigma$ for $t\in [T_{\max}, T_{\max}+h]$.

We next show that the operator $\mathscr{Q}$ is contractive on $\widetilde{E}_{h, \sigma}$. Assume that $v, w\in \widetilde{E}_{h, \sigma}$ and $t\in [T_{\max}, T_{\max}+h]$, it follows that

In this case, for $t\in [T_{\max}, T_{\max}+h]$, one may take very small $h$ such that

which suggests the operator $\mathscr{Q}$ is contractive on $\widetilde{E}_{h, \sigma}$ and thus it has a fixed point $v\in \widetilde{E}_{h, \sigma}$. In view of $v(T_{\max}) = \mathscr{Q}(v)(T_{\max}) = u(T_{\max})$, we set

such that $\overline{u}(t)\in C([a, T_{\max}+h], C_{0}(\mathbb{R}^{d}))$ and

which means $\overline{u}(t)$ is indeed a mild solution of Eq (1.1). Recalling the definition of $T_{\max}$, this yields a contradiction.

The proof of the remainder of this theorem follows that of Theorem 3.2 in ^[16] and so is omitted. The proof is thus complete.

In the following, we present the definition of a weak solution to Eq (1.1) and show that the mild solution given by Definition 3.1 is a weak solution.

Definition 3.2. Let $d\in \mathbb{N}$, $0 < \alpha < \gamma < 1$, $0 < s < 1$, $p > 1$, and $T > a > 0$. For given $u_{a}\in L_{Loc}^{\infty}(\mathbb{R}^{d})$, a function $u$ is said to be a weak solution of Eq (1.1) if $u\in L^{p}((a, T), L_{Loc}^{\infty}(\mathbb{R}^{d}))$ and

for any test function $\varphi\in C_{{\rm{x}}, t}^{2, 1}(\mathbb{R}^{d}\times[a, T])$ satisfying $\mathop{\rm{supp}}\limits_{{\rm{x}}}\varphi\subset\subset \mathbb{R}^{d}$ and $\varphi(\cdot, T) = 0$.

Theorem 3.2. Let $d\in \mathbb{N}$, $0 < \alpha < \gamma < 1$, $0 < s < 1$, $p > 1$, and $T > a > 0$.If the initial value $u_{a}\in C_{0}(\mathbb{R}^{d})$, thenthe mild solution $u\in C([a, T], C_{0}(\mathbb{R}^{d}))$ of Eq (1.1) is also its weak solution.

Proof. Assume that $u\in C([a, T], C_{0}(\mathbb{R}^{d}))$ is a mild solution to Eq (1.1). Then Definition 3.1 gives

Use Lemma 2.5 to get

Therefore, for every $\varphi\in C_{{\rm{x}}, t}^{2, 1}(\mathbb{R}^{d}\times[a, T])$ satisfying $\mathop{\rm{supp}}\limits_{{\rm{x}}}\varphi \subset\subset \mathbb{R}^{d}$ and $\varphi(\cdot, T) = 0$, there holds

For $I_{1}$, an application of Lemma 2.4 leads to

To estimate $I_{2}$, we set $h > 0$, $t\in[a, T)$ and $t+h\leq T$, then

Applying the mean value theorem yields that

and

Consequently,

Combining (3.3) and (3.4), we obtain

which is the desired result and the proof is now ended.

4.   Proof of main result

Proof of Theorem 1.1.

(1) We consider two cases: (i) $1 < p < \widetilde{p} = 1+\frac{1-\gamma}{\alpha}$. (ii) $1 < p < \widetilde{p} = 1+\frac{2s(1+\alpha-\gamma)}{\alpha d}$.

(i) Assume that $1 < p < \widetilde{p} = 1+\frac{1-\gamma}{\alpha}$. Let

and the function $\Phi$ satisfy

Thanks to Theorem 3.2, we may take $\varphi_{1}({\rm{x}}) = \omega({\rm{x}}) \Phi_{n}({\rm{x}})$ with $\Phi_{n}({\rm{x}}) = \Phi(|{\rm{x}}|/n), n = 1, 2, \ldots$, and $\varphi_{2}(t) = \left(1-\frac{\log (t/a)}{\log (T/a)}\right)^{m}$ for $t\in[a, T]$, where $m\geq \max\{2, \frac{p(1+\alpha-\gamma)}{p-1}\}$. Now we set $\varphi({\rm{x}}, t) = \, _{CH}{\rm{D}}^{1-\gamma}_{t, T} (\varphi_{1}({\rm{x}})\varphi_{2}(t))$. From Definition 3.2 of the weak solution, one has

Furthermore, it follows that

According to the inequality $(-\Delta)^{s}\omega({\rm{x}})\leq \omega({\rm{x}})$ in ^[16] and the Lebesgue dominated convergence theorem, we have with $n\rightarrow \infty$ in (4.2),

Using Jensen's inequality in (4.3) gives

Denoting $f(t) = \int_{\mathbb{R}^{d}}u\, \omega\, {\rm{d}}{\rm{x}}$, it is easy to see that $f(t)\geq 0$ and $f(a) > 0$. In view of inequality (4.4), Hölder inequality and Young's inequality, we obtain

Hence there holds

Then we get

If Eq (1.1) has a global solution, we know that $f(a) = 0$ as $T\rightarrow \infty$ in (4.5) by $0 < \alpha < \gamma < 1$ and $p < 1+\frac{1-\gamma}{\alpha}$, which is inconsistent with $f(a) > 0$. Hence, the mild solution of Eq (1.1) blows up in finite time.

(ii) Suppose that $1 < p < \widetilde{p} = 1+\frac{2s(1+\alpha-\gamma)}{\alpha d}$. For $t\in [a, T]$ with $T > a > 0$, we take

with $m\geq \max\{2, \frac{p(1+\alpha-\gamma)}{p-1}\}$, and $\varphi({\rm{x}}, t) = \, _{CH}{\rm{D}}^{1-\gamma}_{t, T}(\varphi_{1}({\rm{x}})\varphi_{2}(t))$.

Let $u$ be a mild solution of Eq (1.1), then Theorem 3.2 implies

Note that the fact

and

where the positive constants $C_{1}$ and $C_{2}$ are independent of $T$.

According to (4.6)–(4.8), together with Young's inequality and Hölder inequality, it holds that

As a result,

i.e.,

The condition $1 < p < 1+\frac{2s(1+\alpha-\gamma)}{\alpha d}$ indicates $1+\alpha-\gamma+\frac{\alpha d}{2s}-\frac{p(1+\alpha-\gamma)}{p-1} < 0$. If Eq (1.1) has a global solution, then $\int_{\mathbb{R}^{d}}u_{a}\varphi{\rm{d}}{\rm{x}} = 0$ as $T\rightarrow \infty$, that is $u_{a}\equiv 0$, which makes a contradiction with the assumption $u_{a}\not\equiv 0$. Therefore, blowup of the mild solution $u$ of Eq (1.1) occurs in finite time.

(2) Based on the fixed point principle, we demonstrate the required result by constructing the global solution of Eq (1.1). Firstly, the condition $p\geq 1+\frac{2s(1+\alpha-\gamma)}{\alpha d}$ implies that

where $(p\alpha-p\gamma+1)_{+} = \max\{0, p\alpha-p\gamma+1\}$. If $d < \frac{2s(1+\alpha-\gamma)}{1-\gamma}$, one has $p\geq \widetilde{p} = 1+\frac{2s(1+\alpha-\gamma)}{\alpha d}$, and if $d\geq\frac{2s(1+\alpha-\gamma)}{1-\gamma}$, one gets $p > \widetilde{p} = 1+\frac{1-\gamma}{\alpha}$. In either case, we obtain

In addition, by $p > 1+\frac{1-\gamma}{\alpha} > \frac{1}{\gamma}$, it follows that

and

Hence, taking (4.12)–(4.15) into account, we can choose $q > p$ such that

and

Let

Then (4.16) gives

If the initial value $u_{a}$ satisfies

then $\vartheta < +\infty$ by (4.18) and (2.10) provided that $u_{a}\in L^{p^{*}}(\mathbb{R}^{d})$ with $p^{*} = \frac{\alpha d(p-1)}{2s(1+\alpha-\gamma)}$.

Next we use the contractive mapping principle to obtain result. To this end, we denote

and

Define

By $q > p$ and $q > \frac{d(p-1)}{4s}$, one gets $\frac{p}{q}-\frac{1}{q} < \min\{1, \frac{4s}{d}\}$. Thus, for any $u, v\in E_{K}$ and $t > a$, it follows that

By taking $K$ small enough such that

which yields $||\Psi(u)-\Psi(v)||_{E}\leq \frac{1}{2}||u-v||_{E}$ by Eq (4.21).

A similar calculation as (4.21) results in

Choose sufficiently small $\vartheta$ and $K$ such that

This implies $\Psi(u)\in E_{K}$ and thus $\Psi$ has a fixed point $u\in E_{K}$ by the contractive mapping principle.

Finally, We need to prove $u\in C([a, \infty), C_{0}(\mathbb{R}^{d}))$. For a $T$ sufficiently close to $a$, let

As demonstrated before, it is known that there is a unique solution $u$ on $E_{K, T}$. It follows from Theorem 3.1 and the initial value $u_{a}\in C_{0}(\mathbb{R}^{d})\cap L^{q}(\mathbb{R}^{d})$ that there exists a unique solution $\widetilde{u}\in C([a, T], C_{0}(\mathbb{R}^{d}))\cap C([a, T], L^{q}(\mathbb{R}^{d}))$ for $T$ sufficiently close to $a$. Hence, for $T$ sufficiently close to $a$, one has $\sup\limits_{a < t < T}\left(\log\frac{t}{a}\right)^{\beta} ||\widetilde{u}(t)||_{L^{q}(\mathbb{R}^{d})}\leq K$. This means that $u = \widetilde{u}$ for $t\in [a, T]$ from the uniqueness of solution and thus $u\in C([a, T], C_{0}(\mathbb{R}^{d}))\cap C([a, T], L^{q}(\mathbb{R}^{d}))$.

Our purpose is to prove $u\in C([a, \infty), C_{0}(\mathbb{R}^{d}))$. In fact, for $t > T$, it holds that

Using the fact $u\in C([a, T], C_{0}(\mathbb{R}^{d}))$, one obtains

For any $\widetilde{T} > T$, it can be easily find that $u^{p}\in L^{\infty} ((T, \widetilde{T}), L^{q/p}(\mathbb{R}^{d}))$ and $_{H}{\rm{D}}^{-(1-\gamma)}_{a, \tau}u^{p}\in L^{\infty}((T, \widetilde{T}), L^{q/p}(\mathbb{R}^{d}))$. On the other hand, the condition $q > \frac{d(p-1)}{2s}$ indicates that we may choose $r > q$ such that $\frac{d}{2s}(\frac{p}{q}-\frac{1}{r}) < 1$. As what we have proved in Lemma 2.5, it is obvious that $I_{2}\in C([T, \widetilde{T}], L^{r}(\mathbb{R}^{d}))$. By the arbitrariness of $\widetilde{T}$, we see that $I_{2}\in C([T, \infty), L^{r}(\mathbb{R}^{d}))$ and thus $u\in C([T, \infty), L^{r}(\mathbb{R}^{d}))$.

Let $r = q\lambda^{n}$ and $\lambda > 1$ satisfy

then $u\in C([T, \infty), L^{q\lambda^{n}}(\mathbb{R}^{d}))$. After finite steps, one has $\frac{p}{q\lambda^{n}} < \frac{2s}{d}$. In other words, we show $u\in C([a, \infty), C_{0}(\mathbb{R}^{d}))$. This concludes the proof of the theorem.

Remark 4.1. It is worth noticing that, according to Theorem 1.1, the Fujita critical exponent to Eq (1.1) is the number $\widetilde{p} = \max\{1+\frac{1-\gamma}{\alpha}, 1+\frac{2s(1+\alpha-\gamma)}{\alpha d}\}$.

Remark 4.2. In the Eq (1.1) , we consider the case $0 < \alpha < \gamma < 1$ and prove the main result, i.e., Theorem 1.1. If $\gamma\geq \alpha$ with $0 < \alpha < 1$ and $0\leq \gamma < 1$, then it is easy to verify that Theorems 3.1 and 3.2 are still valid provided that a mild solution and a weak solution are defined as Definitions 3.1 and 3.2. However, compared with Theorem 1.1, we see that the main conclusions are very different. In fact, we can derive the following result whose proof is similar to that of Theorem 1.1 or can also refer to the proof of Theorem 1 in ^[34].

Theorem 4.1. Let $d\in \mathbb{N}$, $0 < \alpha < 1$, $0\leq\gamma < 1$, $\gamma\leq \alpha$, $0 < s < 1$, and $p > 1$.Assume that $u_{a}\in C_{0}(\mathbb{R}^{d})$ and $u_{a}\geq 0$ with $u_{a}\not\equiv 0$.

(1) If $1 < p\leq\overline{p} = \max\{\frac{1}{\gamma}, 1+\frac{2s(1+\alpha-\gamma)}{(2+\alpha d-2s(1+\alpha-\gamma))_{+}}\}$, then the mild solution of Eq (1.1) will blow up in finite time.

(2) If $p > \overline{p}$ and $||u_{a}||_{L^{p^{*}}(\mathbb{R}^{d})}$ is small enough with $p^{*} = \frac{\alpha d(p-1)}{2s(1+\alpha-\gamma)}$, then Eq (1.1) exists global solution.

Remark 4.3. From Theorem 4.1, we remark that the Fujita critical exponent is $\overline{p} = \max\{\frac{1}{\gamma}, 1+\frac{2s(1+\alpha-\gamma)}{(2+\alpha d-2s(1+\alpha-\gamma))_{+}}\}$ when $\gamma\leq \alpha$ for $0 < \alpha < 1$ and $0\leq\gamma < 1$.

5.   Numerical simulations

In this section, we show the finite time blow-up of the solution to Eq (1.1) by numerical simulation. For this purpose, we have to approximate the Caputo-Hadamard derivative, fractional Laplacian and Hadamard fractional integral in Eq (1.1), respectively. We shall use formulaes (3.2) and (3.3) in ^[35] to discretize the Caputo-Hadamard derivative of order $\alpha\in (0, 1)$ and apply formula (2.9) in ^[36] to approximate the fractional Laplacian of order $s\in (0, 1)$. For the right sided Hadamard fractional integral of order $1-\gamma\, (\gamma\in (0, 1))$ in Eq (1.1), we present the following discrete scheme.

Let $a = t_{0} < t_{1} < \ldots < t_{k} < \ldots < t_{N} = T$ be a partition of the interval $[a, T]$ with $N\in \mathbb{N}$ and some positive number $T > a$. Then the Hadamard fractional integral with order $1-\gamma\, (\gamma\in (0, 1))$ can be approximated by, for $t = t_{k}, 1\leq k\leq N$,

where

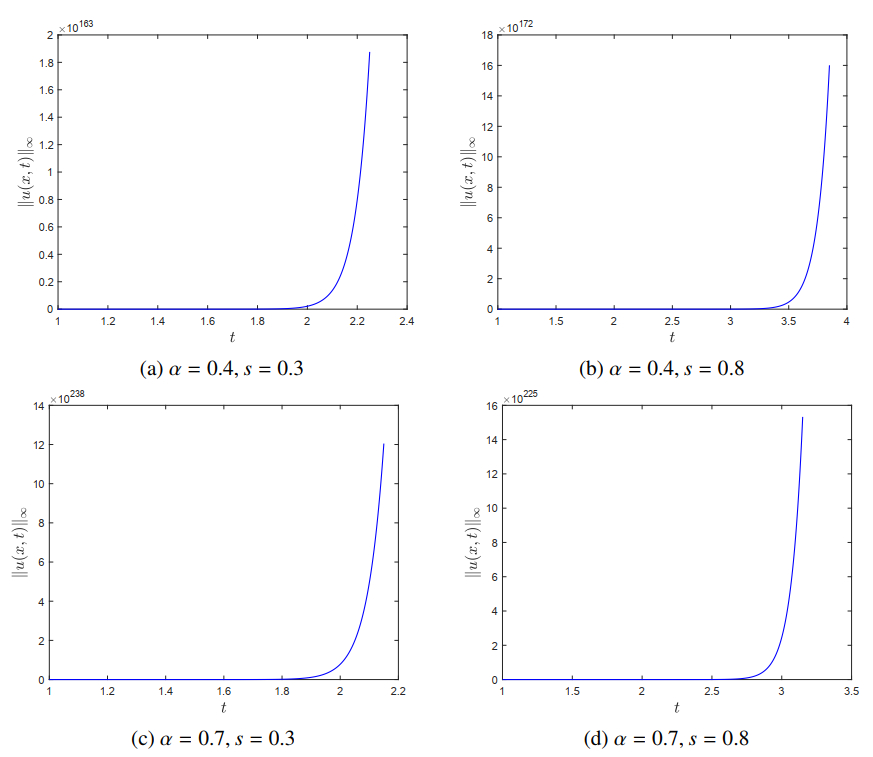
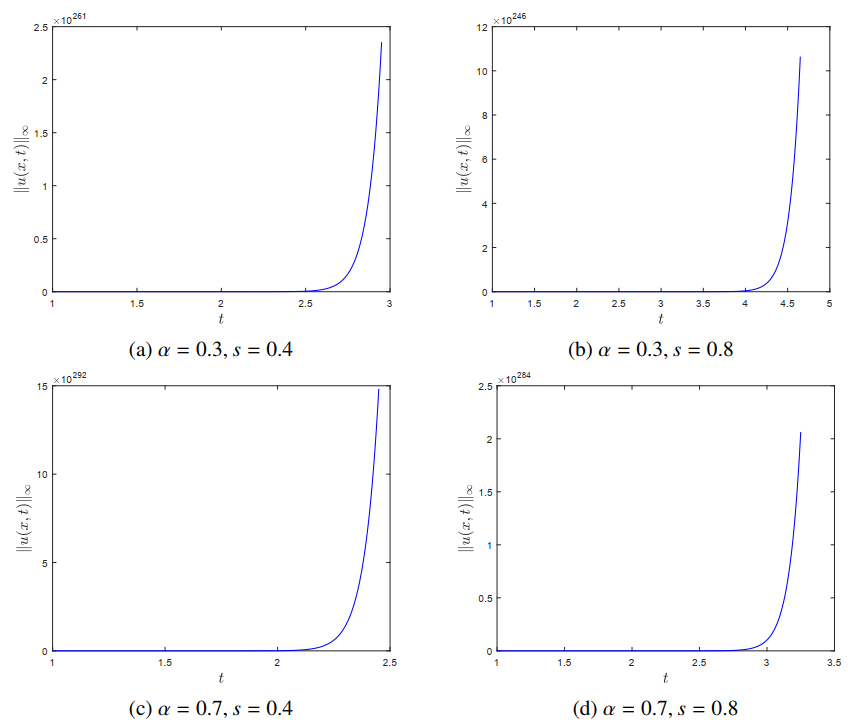
Based on these results, we obtain a numerical scheme to Eq (1.1). For simplicity, we now take $d = 1, a = 1, p = 2$ and $u_{a} = 10$ in Eq (1.1). Figure 1 depicts the curves of the solution to Eq (1.1) when the parameters $\alpha$ and $s$ choose different values and $\gamma = 0.8$, which displays the finite time blow-up of solution of Eq (1.1) and thus shows the effectiveness of the results in Theorem 1.1. Similarly, Figure 2 presents the curves of the solution to Eq (1.1) in the case $\gamma\leq \alpha$ and illustrates the validity of the results given by Theorem 4.1.

6.   Conclusions

In this paper, we study the blow-up and global existence of solution of the Cauchy problem to time-space fractional partial differential Eq (1.1) with nonlinear memory. A mild solution and a weak solution are introduced to Eq (1.1) and the mild solution is actually shown to be the weak solution. We next prove the local existence and uniqueness of the mild solution of Eq (1.1) by using the fixed point argument. Finally, the finite time blow-up and global solution of Eq (1.1) are established and the Fujita critical exponent is also determined, where the blowing-up character of the solution in a finite time is verified by numerical simulations.

Acknowledgements

The work was partially supported by the Scientific and Technological Innovation Programs of Higher Education Institutions in Shanxi, China (No. 2021L573).

Conflicts of interest

The author declare no conflict of interest.