1.
Introduction
Infectious diseases have consistently posed a significant challenge to public health globally. Historically, pandemics such as the plague, black death, and the 1918 Spanish influenza have led to the loss of millions, even tens of millions, deeply affecting the political, social, and economic structures of societies. Today, despite major advancements in medical and healthcare sciences, outbreaks like SARS, H1N1, Ebola, and COVID-19 continue to threaten global health.
In the fight against infectious diseases, mathematical modeling has become an indispensable tool, offering insights into the study, understanding, and management of disease spread [1,2,3,4,5,6,7,8,9,10]. By establishing appropriate mathematical models, we can analyze the effectiveness of control measures quantitatively or qualitatively. Among these models, the state-dependent pulse model is particularly noteworthy for its ability to effectively describe infectious disease control [11,12]. The model operates under the assumption that no control measures are implemented when the number of susceptible populations is within a certain range. However, comprehensive measures, including immunization of susceptible populations and treatment of infected individuals, are implemented when the size of the susceptible population reaches or exceeds the control threshold. Subsequently, scholars have developed numerous state-dependent pulse models tailored to different types of infectious disease characteristics, delving into the impact of state-dependent pulse control strategies on dynamic behaviors, such as disease elimination and epidemics.
Nevertheless, many existing mathematical models of infectious diseases assume continuous interventions like vaccination, drug treatment, or isolation. These models frequently overlook real-world challenges, including resource constraints, high costs, and the impracticality of sustained interventions. Addressing these issues, pulse control strategies, involving periodic interventions like vaccination or patient isolation, have garnered significant attention in the field of mathematical modeling. Pulse control proves particularly beneficial in resource-limited scenarios and is extensively researched for its potential in infectious disease control [13,14,15,16,17,18,19,20]. Furthermore, state-dependent feedback control, as represented by pulse semi-dynamic systems, finds application across various fields and sciences, such as fisheries harvesting [21,22,23], integrated pest management [24,25,26], and interactions among biological populations [27,28,29].
In the realm of infectious disease modeling, the selection of the ratio-dependent action threshold method is driven by a meticulous consideration of the limitations inherent in traditional control strategies and the imperative need for a more realistic representation of disease dynamics. The foundational premise of triggering interventions based on a fixed population density threshold, a cornerstone of state-dependent feedback control, tends to oversimplify the intricate dynamics of real-world scenarios. This conventional approach may overlook crucial factors, such as situations where the transmission rate remains high despite a low population density or vice versa. In both scenarios, a high population density may not necessarily warrant immediate control intervention if the transmission rate remains low. To address this limitation, the adoption of a more effective control strategy becomes imperative. This strategy considers both population density and its rate of change, leading to the formulation of a nonlinear threshold control policy known as the "ratio-dependent action threshold". In this approach, the decision to implement control measures hinges on the ratio of population density to its rate of change, providing a more adaptive and realistic representation of infectious disease scenarios.
The significance of the ratio-dependent action threshold method lies in its capacity to capture the nuanced dynamics of infectious diseases. Recent research has delved into nonlinear threshold strategies, revealing diverse and dynamic behaviors in various contexts [30,31,32,33,34,35,36]. This method not only challenges prevailing assumptions in pulse control models, where the rate of change is assumed to directly correlate with population density following the introduction of control measures but also offers a more accurate representation by acknowledging inherent constraints in this relationship. Furthermore, the adoption of a saturated form that includes a nonlinear function enhances the realism of infectious disease models. This nuanced approach considers the complexities of disease dynamics, contributing to a more comprehensive understanding of infectious diseases and facilitating the development of intervention strategies aligned with the dynamic nature of disease transmission. In summary, the ratio-dependent action threshold method is chosen for its ability to address real-world complexities, offering a more accurate and adaptive representation of infectious disease dynamics in modeling and control strategies.
Our efforts aim to integrate a nonlinear threshold strategy within an infectious disease transmission model with a saturated incidence rate. In Section 2, we introduce an SIR state-dependent pulse model with a saturated incidence rate and adopt a rate-related nonlinear action threshold. Section 3 delves into constructing the corresponding Poincaré map by defining the pulse set and phase set range and discussing the essential attributes of the Poincaré map. In Section 4, conditions for the existence and stability of 1st order periodic solutions are established. Section 5 analyzes the bifurcation of the discrete mapping's one-parameter family concerning a critical parameter near the trivial fixed point when the model possesses a local disease equilibrium. Sensitivity analysis of the model is conducted in Section 6, where variations in parameters lead to complex dynamics. The analysis of the intricate interplay remains a challenging and underexplored frontier in this domain. Finally, a comprehensive conclusion and detailed discussions wrap up our study.
2.
Model
In our current study, we have chosen an SIR model with a saturated incidence rate, and extended the model presented in [37] by considering the density of susceptible individuals and their rate of change as action thresholds. The model is given by the following equation:
Here, S(t) and I(t) denote the numbers of susceptible and infected individuals, respectively. Λ is the birth rate, δ is the mortality rate, γ indicates the rate of recovery, β is the rate at which transmission occurs, and α is the half-saturation constant. Additionally, we define q as q=γ+δ. The parameters u1, v1, and ET are all positive constants, and to simplify the model, we set u1+v1=1. When the population density of susceptible individuals reaches the action threshold given by
control measures will be implemented to update the numbers of susceptible individuals and infected individuals as follows: (1−η1)S(t) and (1−η2)I(t). In this context, η1 and η2 represent the maximum vaccination rate and maximum treatment rate, respectively. The parameters u1, v1, ET, η1, and η2 are pivotal factors within the control of decision-makers, intricately associated with infectious disease control measures. The values of these parameters can be effectively determined through practical applications in the field of infectious disease control.
The dynamic analysis of the non-pulsed model in [37] has been extremely valuable for our research. We define the non-pulsed model as M0, and, based on [37], it is evident that M0 possesses a disease-free equilibrium point, denoted as E0(K,0), where E0 is stable when R0<1, and it becomes unstable when R0>1, where
Furthermore, M0 has an endemic equilibrium point denoted as E∗(S∗,I∗) for R0>1, where
In cases where Δ≥0, E(S,I∗) is a stable node, while for Δ<0, it behaves as a stable focus, where
It follows from
that we have
and we can define the impulsive curve as
Since
we can define the corresponding phase space as
According to dSdt=0, we can denote the vertical isocline as
There is an intersection point between L1 and LM for
where
and the intersection point denote as
where
and
If K>ETu1, then there is a unique intersection PET where all coordinates are positive. However, when u1=0, the inequality LM(S)<L1(S) always holds, indicating that lines LM and L1 do not intersect.
3.
Poincaré map for R0<1
The trajectory starting from any point P+k=(S+k,I+k) on LN will definitely reach LM after finite time t, and its intersection with LM is
and it will pulse to point P+k+1⊂LN. Therefore, we can denote
with
and we define the Poincaré map PM as
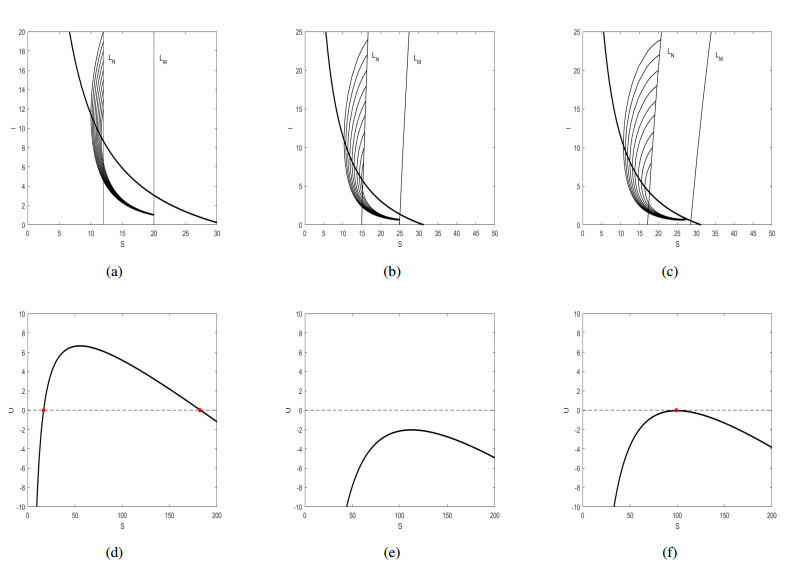
There exists a point on LN, denoted as PT=(ST,IT). The trajectory starting from PT reaches LM at point PT1=(ST1,IT1) (see Figure 1). Therefore, we define the impulsive set and phase set as
We denote continuous functions Q1(S(t),I(t)) and Q2(S(t),I(t)) as
so we consider the following scalar differential equation:
G(S,I) is a continuously differentiable function. Now we denote S+0=Sc, I+0=Y with (S+0,I+0)∈N. Then, we have
and
where the value of S is between the LM and the LN.
Therefore, the Poincaré map PM has the following form
Theorem 1. For model (1) with R0≤1, the Poincaré map PM exhibits the subsequent characteristics:
(i) PM has a domain spanning [0,+∞) and encompasses a range of [0,PM(IT)].
(ii) Within the interval [0,IT], PM exhibits an upward trend, but shows a decline for (IT,+∞). It remains continuous and exhibits a concave nature over [0,IT].
(iii) The singular stable fixed point for PM is at I=0. Hence, model (1) possesses a stable disease-free periodic solution (DFPS).
Proof. It follows from the first function of system (2) that we have
where A=Λ−δS, B=1+αS, C=βS, and
Given that S<K and R0≤1, it follows that A>0 and C−qB<0, and for I<IT, D>0, whereas for I>IT, D<0. From these observations, it is clear that both
hold true for every I<IT.
From the principles of the Cauchy-Lipschitz theorem applied to the scalar differential equation, we can deduce that
and
Moreover, according to
we have
and
Given that (1−η2)>0, for Y∈(0,I(T)], it is evident that
This suggests that PM(Y) is both continuously differentiable and concave within the interval (0,IT]. Additionally, PM(Y) consistently rises within (0,IT] and falls in the range (IT,+∞).
Therefore, when R0≤1, and taking into account that ETu1<K, it follows that for every Y in the sets (0,IT] and (IT,K), the inequality Y≥I(S,Y)>PM(Y) holds true. This implies that the Poincaré map PM exhibits a singular fixed point which is globally stable. As a result, model (1) maintains a distinct DFPS that demonstrates global stability. □
4.
The existence and stability of boundary order-1 periodic solution
If we define the function
solving LM=0 yields the intersection points of LM with the x-axis, which is equivalent to solving f(S)=0. When I=0, the model can be simplified as follows:
Furthermore, it follows from f(S)=0 that we have
so model (3) is equivalent to
Denoting the initial value S+Z=(1−η1)SZ, we have the following results:
Theorem 2. If R0≤1, then system (1) has a globally stable boundary order-1 limit cycle (ST(t),0) with period T, where
and
Proof. Based on the premise that the solution S(t) of the system moves from the initial value S0 to SZ within a finite time T, by solving the system with initial value S0=S+Z, we can conclude that the periodic solution
and
Furthermore, it follows from in [38, Lemma A.1] that we have
where
By simple calculations, we have
and
which yields
Moreover, we can obtain
where
and
Therefore,
so we can know that μ2<1 for R0<1, which means that the boundary order-1 limit cycle (ST(t),0) is orbitally asymptotically stable.
Once it is established that the boundary order-1 limit cycle (ST(t),0) is globally attractive, it is tantamount to demonstrating its global stability. Thus, we make the assumption that the pulse point sequence I+k for k≥0 lies entirely on the line LN and I+k∈[0,(1−η2)IT1]. Given that R0≤1, we can deduce that dIdt<0 for S<K. Consequently, we can ascertain that I+k forms a strictly decreasing sequence, with
Additionally, the monotonicity of I(t), as depicted in Figure 2a, dictates that I0=0. As a result, the boundary order-1 limit cycle (ST(t),0) for model (1) is globally attractive. This completes the proof of Theorem 2. □
5.
Bifurcations for R0>1
5.1. Transcritical bifurcations for ET
According to
taking the derivative of μ2 with respect to ET as
where
taking the derivative of g(S) with respect to S yields
where
By simple calculations, we know that g2(S) has a minimum value (−αδ(S∗)2+(αΛ−δ)S∗+Λ) for S=S∗, and if
then
and then g′(S)>0.
Based on the above discussion, if
then
and
If
then
and
Moreover, it is clear that
as SZ approaches S∗, and
as SZ approaches K. According to the monotonicity of ∫SZS+Zg(S)dS, it can be inferred that there is an
such that μ2=1.
It is clear that
and it follows from the definition of PM that we have
According to the monotonicity of g(S), it can be known that
To simplify symbols, now we denote
By simple calculations, we have
For convenience, we derive the derivative of E1(x) with respect to x as
Therefore, through inequality A, we can obtain
According to the monotonicity of E1(x), we know that the function E2(x) is monotonically decreasing for x∈[S+Z,S∗] and monotonically increasing for x∈[S∗,SZ]. Thus, if ET=ET∗, then
which indicates that E2(S∗)<E2(x)≤1 for all x∈[S+Z,SZ]. Moreover, we have
and thus, E4(x)>0 is monotonically increasing on [S+Z,SZ]. Furthermore, we have
By simple calculations, we have
which means that
The above calculations demonstrate that the conditions of in [38, Lemma A.2] are satisfied. Therefore, we have the following conclusion:
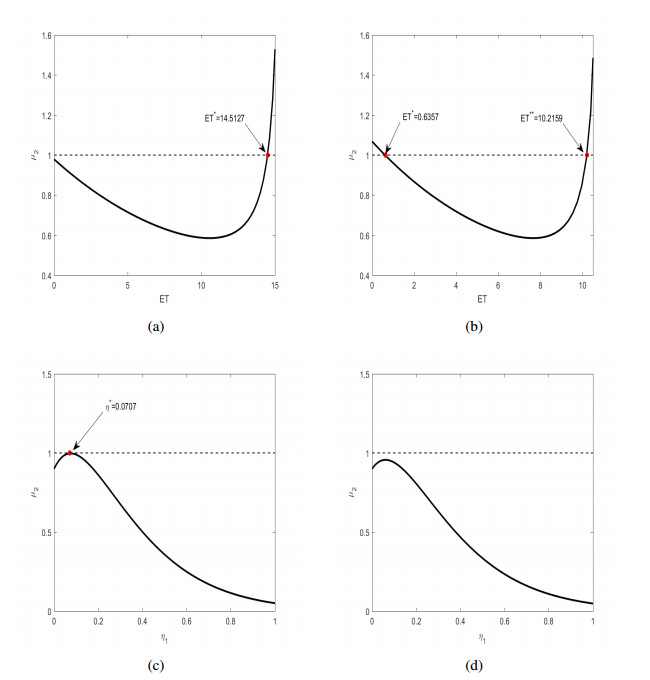
Theorem 3. Given that S∗<SZ<K and R0>1, PM(Y,ET) undergoes a transcritical bifurcation at ET=ET∗. This indicates the emergence of an unstable positive fixed point for PM(Y,ET) as ET transitions across ET∗ from the right to the left side. Consequently, we posit that for some sufficiently small value ϵ>0, system (1) exhibits an unstable positive periodic solution when ET lies in the interval (ET∗−ϵ,ET∗).
The emergence of a positive fixed point as elucidated in Theorem 3 indicates an unstable positive periodic solution for system (1). This suggests a concurrent local stability of the internal equilibrium (S∗,I∗) and the DFPS in system (1), leading to what we refer to as a bistable impulsive system, as shown Figure 2b. A more precise description is provided below.
Theorem 4. Given conditions S∗<SZ<K, R0>1, and ET∈(ET∗−ϵ,ET∗), where ϵ>0 is sufficiently small, it can be concluded that system (1) exhibits bistability.
5.2. Transcritical bifurcations for η1
Here we take η1 as the key factor for bifurcation. Thus, we have
By simple calculation, we have
and solving
yields a unique root S+Z=S∗, i.e.,
where
From the given information, for η1 in the range (0,¯η1), it is evident that
Conversely, for η1 in the interval (¯η1,1), we observe that
According to the monotonicity of dμ2(η1)dη1, it is clear that the value of μ2(0) is less than that of μ2(¯η1), denoting that μ2(η1) shows an increasing trend in the interval [0,¯η1] and a decreasing trend when η1 lies between (¯η1,1). Moreover, guided by the trend in the rate of change, if μ2(¯η1)>1, a particular value η∗1 exists in the interval (0,¯η1) for which μ2(η∗1)=1. Similarly, if μ2(1)<1, there exists a singular value η∗∗1 in the range (¯η1,1) such that μ2(η∗∗1)=1. If η1=¯η1, it follows from
and
that we have
If
then
and then μ2(¯η1)>1.
If η1=1, then S+Z=0. It follows from
and
that we have
If
then
and then μ2(1)<1.
Therefore, from the above discussion, we can derive the following results according to in [38, Lemma A.2].
Theorem 5. Given the conditions S∗<SZ<K and μ2(¯η1)>1>μ2(1), there exist transcritical bifurcation points denoted by η1=η∗1 and η1=η∗∗1 for PM(Y,η1). This indicates that, as the parameter η1 surpasses η∗1 in the decreasing direction, or goes beyond η∗∗1 in the increasing direction, PM(Y,η1) establishes an unstable positive equilibrium point. Under the presumption of a sufficiently diminutive value ϵ>0, system (1) demonstrates an unstable positive periodic solution when η1 is situated in either the interval (η∗1−ϵ,η∗1) or (η∗∗1,η∗∗1+ϵ).
Proof. It is obvious that
It follows from the proof of Theorem 1 that we have
Thus,
and we have
which means that
and
Through the similarity method in the proof process of Theorem 3, we have
Consequently, under the conditions S∗<SZ<K, μ2(¯η1)>1, and 1>μ2(1), PM(Y,η1) results in an unstable fixed point when η1 transitions past η∗1 in a right-to-left direction or η∗∗1 in a left-to-right direction. Moreover, given η1∈(η∗1−ϵ,η∗1) or η1∈(η∗∗1,η∗∗1+ϵ), where ϵ>0 is sufficiently small, system (1) has an unstable positive periodic solution. This concludes our demonstration. □
6.
Numerical simulation and analysis
We observe the extent to which the model is affected by varying the weighted coefficients, u1 and v1, of the action threshold. When we set u1 and v1, as shown in Figure 3a, LM and LN become two lines perpendicular to the x-axis. At this point, the model is simplified to a pulse system with the threshold based solely on the density of susceptible individuals. Such systems have been extensively studied and widely applied in infectious diseases and population dynamics.
However, when the rate of change is introduced into the action threshold, LM and LN become two curves, and the curvature of these curves varies with changes in u1 and v1, Figure 3b, c highlights the influence of the rate of change of susceptible individuals in the implementation of a comprehensive control strategy. Properly choosing the coefficients can lead to the virus becoming extinct after a finite number of control measures.
When v1 is non-zero, LM and LN represent two curves, and there is a possibility of intersection between them. We define U=LM−LN. By varying the value of ET, we can observe whether there are intersections between the pulse set and phase set. Figure 3d–f shows that as ET increases, the number of intersections decreases from 2 to 0. The presence of intersections poses challenges in the analysis of Poincaré maps, leading to highly complex dynamics. This complexity is a primary focus of our ongoing research.
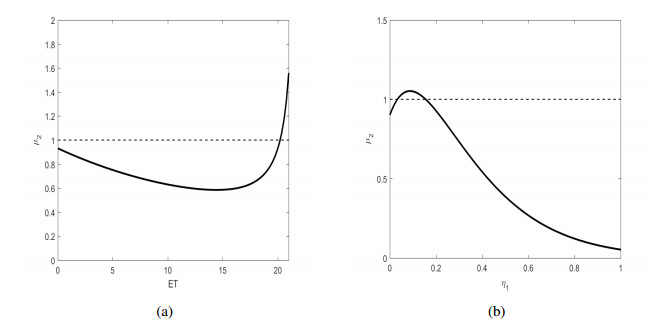
In previous sections, we explored the possibility of bifurcations with respect to individual parameters, as depicted in Figure 4. To examine the sensitivity of the model, we further investigated the impact of different weighted coefficients for the action threshold as influencing factors on bifurcations.
In Figure 5a, b, as the parameter v1 increases, the trajectories of μ2 concerning ET intersect with the line μ2=1 at two distinct points. Specifically, with v1 rising from 0.5 to 0.65, two intersections with the line μ2=1 are highlighted. However, Figure 5c, d depict that, as v1 decreases, the peak values of μ2's trajectories in relation to η1 diminish, eventually dropping below 1. Collectively, these patterns suggest that considering the rate of change in the action threshold is a more comprehensive approach for managing infectious diseases, emphasizing the importance of monitoring not just the current thresholds, but their evolution over time.
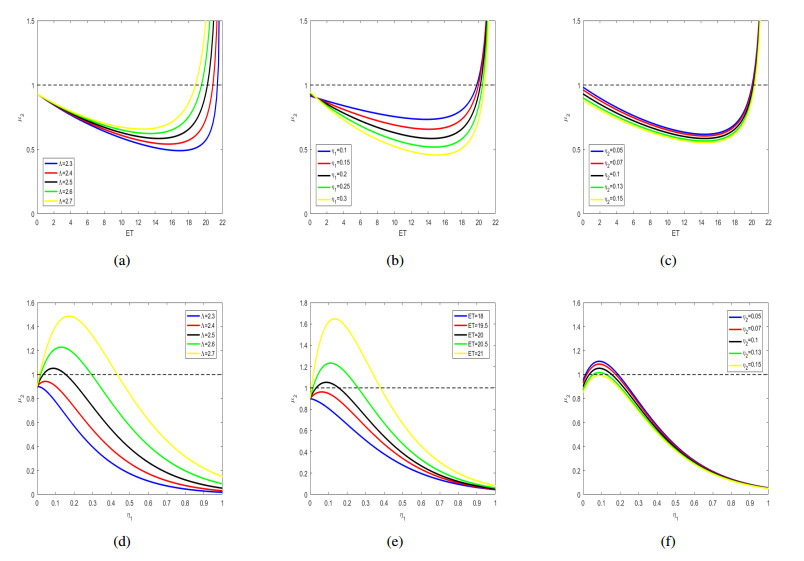
In Figure 6a–c, the float multiplier μ2 response to the action threshold ET is closely related to multiple parameters. First, with an increase in the birth rate, Λ, the initial value of μ2 in the low ET region rises, suggesting that a higher threshold might be needed to maintain stability of μ2 at a higher birth rate. Further considering the maximum vaccination rate η1, its increase results in a slight rise in the initial point of μ2 at low ET, indicating an enhanced sensitivity of μ2 to the threshold under high vaccination scenarios. Additionally, for the maximum treatment rate η2, similar μ2 trajectories are given at low ET values, but with a higher value of η2, the decline trend of μ2 is more rapid. This reveals that, in a high treatment rate environment, μ2 responds more quickly to threshold changes. In summary, these analyses present how the relationship between μ2 and ET is influenced by the three parameters Λ, η1, and η2.
On the other hand, in Figure 6d–f, the relationship between μ2 and the maximum vaccination rate η1 is significantly affected by different parameters. For instance, when the birth rate Λ rises from 2.3 to 2.7, the peak value of μ2 consistently increases and shifts to the right. For scenarios where η1 is close to 1, all μ2 trajectories are approximately 0. When the focus is on the maximum treatment rate η2, even though peak positions of the trajectories are similar, with the growth of η2, the peak gradually decreases; especially in parts where η1 is larger, the decline of μ2 is more pronounced. For the threshold ET, as it increases from 18 to 21, the maximum value of μ2 seems to decrease, and its peak position shifts to the right. This aligns with the observation of μ2 trajectories approaching 0 when η1 is near 1. These shifts provide a comprehensive view of how the system adjusts based on different parameter values.
7.
Conclusions and discussion
In conclusion, state-dependent impulsive semi-dynamic systems represent a category of highly discontinuous and non-smooth systems. These systems have been applied in various fields, including integrated pest management, viral dynamical systems, and diabetes treatment [26,39,40,41]. In recent years, significant progress has been made in the research of such systems, encompassing analytical techniques, qualitative analyses, and practical applications [30,42]. Notably, the Poincaré map serves as a mathematical tool promoting comprehensive exploration of impulsive systems with nonlinear impulsive functions, enabling the study of the existence and global stability of k-order periodic solutions.
In our research, we examined a state-dependent impulsive model, which incorporates a saturation incidence rate and describes the interaction between susceptibles and infectives. A highlight is that the action threshold in our model is determined by the density of susceptibles and its rate of change. This differentiates our work from previous literature [37,38], which primarily operated under the assumption of a fixed action threshold. Our innovation lies in introducing an action threshold determined by the density of the affected susceptibles and its rate of change, thereby translating the impulse set and phase set into more intricate nonlinear curves.
By analyzing the various positional relationships between system trajectories and equilibrium points, we derived the respective impulse and phase sets, as depicted in Figure 1. Utilizing these conditions, we constructed the Poincaré map and studied the conditions for the emergence of a first-order periodic solution within the system framework. Furthermore, we undertook a thorough examination of the stability of these first-order periodic solutions. Our theoretical findings were corroborated by numerical simulations, confirming the existence of the first-order periodic solution. Additionally, based on the results of the single parameter's influence on μ2, we conducted a sensitivity analysis by fixing other parameters and selecting one parameter as a benchmark to observe the trajectory changes of μ2, as shown in Figure 4. Under certain conditions, the threshold parameter ET or vaccination rate η1 can induce a transcritical bifurcation, leading to unstable positive periodic solutions. Boundary periodic solutions and equilibrium points of the impulse-free system can coexist, suggesting the possibility of backward bifurcations. Importantly, when the action threshold ET is weighted by the density of susceptible individuals and its rate of change, the impulse curve and phase curve become nonlinear, as shown in Figure 3. The system's impulse curve, phase curve, and the intricate and mutable positional relationships between them and two isoclines introduce complexity, making the precise definition of the boundaries of impulse and phase sets challenging. Furthermore, based on Figure 5, variations in the weighted values of the susceptible individual density and its rate of change also impact the bifurcation results of the model. According to Figure 6, μ2 responds to the action threshold ET based on several parameters, including birth rate, maximum vaccination rate, and maximum treatment rate. Among them, the relationship between μ2 and vaccination rate η1 is also significantly influenced by the birth rate, maximum treatment rate, and action threshold.
Finally, we emphasize the critical role of mathematical models and control strategies in the field of infectious disease control. Future research directions may delve deeper into considering the growth rate of infected individuals as a threshold for studying disease control strategies, thereby advancing the development of more complex nonlinear pulse control methods. The further application of this technology holds the potential to have a profound impact on infectious disease control and other related fields, providing valuable directions for future research and application.
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Acknowledgments
This work is supported by the National Natural Science Foundation of China (No. 12261033).
Conflict of interest
The authors declare no conflicts of interest.










 DownLoad:
DownLoad: