Epidemiological analysis of fractional order COVID-19 model with Mittag-Leffler kernel

1.   Introduction

Coronavirus (COVID-19) is a new phenomenon in recent days, which indulged the whole world in an emergency situation. According to reports, it's originated from Wuhan city of China ^[1]. In December 2019, the first case of novel coronavirus was reported. The symptoms of coronavirus are dry cough, fever, fatigue, and severe cases of acute respiratory syndrome that appears in 2–10 days and further cause pneumonia, Kidney failure, and even death ^[2]. Between March and April, coronavirus became a global phenomenon, and the whole globe faced an emergency situation. Initial cases were reported in the wet seafood market in Wuhan, China ^[3]. That's why some researchers thought that it's transmitted in humans through animals. This virus is transmitted from one person to another through physical contact, droplets during sneezing and coughing ^[4]. Researchers of the field of epidemiology and other fields of biology are trying hard to develop the cure based on ongoing clinical trials, but different researching companies of different countries have developed the vaccine of COVID-19. If we mention here, then China, the USA, England and Russia have developed the vaccine. In most countries of the globe, people receiving doses of vaccines. According to WHO, at 4:53 pm CET, 18 March 2021, there were 120,915,219 confirmed cases reported globally, including 2,674,078 deaths and a total of 364,184,603 vaccine doses were administered ^[5]. Developed countries like the USA, UK, Italy, Spain, and many others are affected very badly; most of the global deaths are reported from these countries ^[6]. Some precautionary measures for COVID-19 are wearing a face mask, maintaining a 6feet distance, coughing and sneezing in the elbow, and washing your hands minimally 30 seconds. Almost all countries' governments have enforced non-medical interventions such as social distancing, self-quarantine, isolation, wearing a face mask, protecting gears for medical staff, and travel restrictions to control the spread of disease. Mathematical modelling is used to understand the dynamics and behaviour of disease and then develop the procedures for the treatment of disease. For this purpose, many researchers developed the COVID-19 models (see ^[7,8,9,10]). The reproductive number has a notable role in the analysis of mathematical models. Reproductive number explains the behaviour of the simulation of COVID-19.

In Pakistan, 739,818 confirmed cases had been reported, including 15,872 deaths out of over 220 million population to date ^[11,12]. On 26 February 2020, the first case of COVID-19 was reported in Karachi, Pakistan's economic hub. Nowadays, Pakistan has been facing the third wave of COVID-19, which is at its peak. The government is not in the right of Strick lockdown because most of the people are a daily wager; that's why the government has been implementing smart lockdown. There are many mathematical models provided for more insight into how to control the spread of Covid-19 to health authorities ^[13,14,15]. In three highly affected countries, the transmission pattern of COVID-19 was studied by Fanelli and Piazza ^[16]. To explain the simulation of COVID-19 transmission ^[17,18] are used and explain the natural fact of fractional-order mathematical models in a systematic way as in ^[19,20]. The fractional-order models are more effective than classical integer models in analyzing the dynamics and behaviour of infectious diseases ^[21,22]. The fractional-order models give better results to the real data. Some fractional operators are given in ^[23,24], and applications of these fractional operators are given in ^[25,26]. The investigations of some other infectious disease mathematical models have been studied in ^{[27,28,29,30,31]}. Studied the outcome of an antiviral drug on the system to obstruct the contact between epithelial cells and SARS-CoV-2 to restrict the COVID-19 disease in ^[37,38]. Results have good accuracy, and the method is valid for the fuzzy system of fractional ODEs COVID-19. Also, the random COVID-19 model described by a system of random differential equations was presented in ^{[39,40,41,42,43]}, and some application of fractional order for the real-life problem was also given in ^{[44,45,46,47,48]}.

In this paper, we proposed a fractional-order COVID-19 model with Atangana-Baleanu, Atangan-Tufik scheme and fractal fractional-order derivative. In Section 1, we construct the introduction with the literature review of COVID-19 and fractional calculus. Section 2 has some basic definitions which are helpful for analysis and simulation if the model. In Section 3, the mathematical model of COVID-19 is present with disuses the boundedness and positivity of the model. In Section 4, using fixed point theory and an iterative method, the existence and uniqueness of the system of solutions for the model have been made. In Sections 5 and 6, new numerical scheme and fractal fractional-order derivative construct with Atanga-Tufik method for real data of Wuhan China. In Section 7, we describe the numerical simulation of the proposed scheme with real data and best-fitted parameter substitution. We give the conclusions and perspectives in Section 8.

2.   Basic concepts of fractional operators

Definition 2.1. For a function $y\left(t\right)\in {W}_{2}^{1}\left(\mathrm{0, 1}\right), b>a \;and\;\mathrm{\Theta }\in \left[\mathrm{0, 1}\right],$ the definition of Atangana-Baleanu derivative in the Caputo sense is given by

where

By using the Sumudu transform (ST) for (1), we obtain

Definition 2.2. The Laplace transform of the Caputo fractional derivative of a function $y\left(t\right)$ of order $\Theta >0$ is defined as

Definition 2.3. The Laplace transform of the function ${t}^{{\varTheta }_{1}-1}{E}_{\varTheta, {\varTheta }_{1}}(\pm \mu {t}^{\varTheta })$ is defined as

where ${E}_{\varTheta, {\varTheta }_{1}}$ is the two-parameter Mittag-Leffler function with $\varTheta, {\varTheta }_{1}>0.$ Further, the Mittag-Leffler function satisfies the following equation ^[32].

Definition 2.4. Suppose that $y\left(t\right)$ is continuous on an open interval $(a, b),$ then the fractal-fractional integral of $y\left(t\right)$ of order $\mathrm{\Theta }$ having Mittag-Leffler type kernel and given by

3.   Formulation of COVID-19 model

This section considers the novel coronavirus (COVID-19) disease model developed by Yang and Wang ^[33]. In this model total human population is divided into five classes, namely, susceptible individuals are represented by ${S}_{c}$, ${E}_{c}$ represents exposed individuals, who are infected but not infectious as yet, ${I}_{c}$ represents infected population, those individuals in which symptoms have shown strongly and can spread infection by contact with susceptible individuals, ${R}_{c}$represents the individuals who have no symptoms and they have recovered after receiving treatment and the concentration of virus is represented by ${V}_{c}$.

In the above system parameters are defined as the influx of population is denoted by ${\mathrm{\Pi }}_{\mathrm{c}}$, µ_c represents natural death rate, (α_c)⁻¹ represents quarantine period of the infected individuals, rate of recovery is denoted by γ_c, The exposed and infected people which contributing the coronavirus in the surrounding is represented by ψ_1c, ψ_2c respectively, disease induced death rate is represented by ω_c and τ_c represents removal rate. ${\mathtt{β }}_{{\mathrm{E}}_{\mathrm{c}}}$ represents the rate of human to human transmission of virus between exposed and susceptible people, The rate of human to human transmission between infected and susceptible people are represented by ${\mathtt{β }}_{{\mathrm{I}}_{\mathrm{c}}}$ and ${\mathtt{β }}_{{\mathrm{V}}_{\mathrm{c}}}$ denotes the rate of transmission due to environmental contact to human. We suppose that given all functions ${\mathtt{β }}_{{\mathrm{E}}_{\mathrm{c}}}, {\mathtt{β }}_{{\mathrm{I}}_{\mathrm{c}}}\;\mathrm{a}\mathrm{n}\mathrm{d}\;{\mathtt{β }}_{{\mathrm{V}}_{\mathrm{c}}}$ are non-negative and non-increasing. By applying Atangana-Baleanu fractional derivative (ABC) of order $\mathrm{\Theta }$ and $\mathrm{\Theta }\in \left(\left.\mathrm{0, 1}\right]\right.$, then the system (7) becomes

Initial conditions are

Equilibrium points

In this section, we will discuss the equilibrium points of the given COVID-19 model (8). Equilibrium points have two types, namely disease-free equilibrium and endemic equilibrium. We obtained these points by putting the Right-hand side of the system (7) is zero. We suppose that ${\mathrm{E}}^{0}$ represents disease free equilibrium and endemic equilibrium is represented by ${\mathrm{E}}^{\mathrm{*}}$, we have

We obtain the basic reproductive number ${R}_{0}$ by ^[34], we have

We consider the following parameters values and initial conditions ^[34] for our simulations:

Theorem 3.1. The solution of the proposed fractional-order model $\left(8\right)$ along initial conditions (9) is unique and bounded in ${R}_{+}^{5}.$

Proof.

The existence and uniqueness of the solution of system $\left(8\right)$ on the time interval $(0, \mathrm{\infty })$ can be obtained by the process discussed in the work of Lin ^[36]. Subsequently, we have to explain the non-negative region ${\mathrm{R}}_{+}^{5}$ is positively invariant region. From model $\left(8\right),$ we find

If $\left({S}_{c}\left(0\right), {E}_{c}\left(0\right), {I}_{c}\left(0\right), {R}_{c}\left(0\right), {V}_{c}\left(0\right)\right)\in {R}_{+}^{5},$ the solution $\left[{S}_{c}\left(t\right), {E}_{c}\left(t\right), {I}_{c}\left(t\right), {R}_{c}\left(t\right), {V}_{c}\left(t\right)\right]$ cannot escape from the hyperplanes ${S}_{c} = 0, {E}_{c} = 0, {I}_{c} = 0, {R}_{c} = 0\; and\;{V}_{c} = 0$. Also, on each hyperplane bounding the non-negative orthant, the vector field points into ${R}_{+}^{5}$, i.e., the domain ${R}_{+}^{5}$ is a positively invariant set.

In the next theorem, we will show the boundedness of the solution to the proposed model $\left(8\right)$.

Theorem 3.2. The region $A = \left\{\right({S}_{c}\left(t\right), {E}_{c}\left(t\right), {I}_{c}\left(t\right), {R}_{c}\left(t\right), {V}_{c}\left(t\right))\in {R}_{+}^{5}|0\le {S}_{c}\left(t\right)+{E}_{c}\left(t\right)+{I}_{c}\left(t\right)+{R}_{c}\left(t\right)+{V}_{c}\left(t\right)\le \frac{{\mathrm{\Pi }}_{c}}{{\mu }_{c}}\}$ is a positive invariant set for system $\left(8\right)$.

Proof. For the proof of the theorem, we have from system $\left(8\right)$

where

Since ${\psi }_{1c}{E}_{c}, {\psi }_{2c}{I}_{c}, {\omega }_{c}{I}_{c}, {\tau }_{c}{V}_{c}$ are positive parameters, then

Applying the Laplace transform to above equation, we get

which further gives

From Eqs (3) and (4) we infer that $if\; ({S}_{{c}_{0}}, {E}_{{c}_{0}}, {I}_{{c}_{0}}, {R}_{{c}_{0}}, {V}_{{c}_{0}})\in {R}_{+}^{5},$ then

This shows that the total population $N\left(t\right),$ i.e., the subpopulations $S\left(t\right), H\left(t\right), I\left(t\right)$ and $Q\left(t\right),$ are bounded. This proves the boundedness of the solution of system $\left(8\right).$

4.   COVID-19 model with the Mittag-Leffler kernel

By using the Sumudu transform on the system $\left(8\right),$ we get

Rearranging, we get

By using the inverse Sumudu transform of system (11), we have

Therefore the following recursive formula is obtained.

And the solution of (13) is provided by

Stability analysis of model by using fixed-point theory

Theorem 4.1. Suppose that $\left(Y, \left|.\right|\right)$ as a Banach space and H a self-map of $Y$ satisfying

$\forall y, r\in Y,$ and $0\le \theta < 1.$ Assume that H is Picard H-Stable. Suppose that from system (10), we have

Equation (14) is a Lagrange multiplier.

Proof. Suppose K as a self-map is given as

By taking the norm and also using the triangular inequality, we obtain

Hence K satisfied all the conditions of Theorem (4.1) while

This shows that K is Picard K-Stable.

Theorem 4.2. Prove that system (8) has special unique solution.

Proof. Let Hilbert space $H = {L}^{2}\left(\left(a, b\right)\times \left(0, T\right)\right)$ which is given as

Suppose that

We show that

Where $\left({S}_{{c}_{11}}-{S}_{{c}_{12}}, {E}_{{c}_{21}}-{E}_{{c}_{22}}, {I}_{{c}_{31}}-{I}_{{c}_{32}}, {R}_{{c}_{41}}-{R}_{{c}_{42}}, {V}_{{c}_{51}}-{V}_{{c}_{52}}\right),$ represents the special solutions of system. We use the correspondence between norm and the inner product, we write the equation as

Due to large number of ${e}_{1}, {e}_{2}, {e}_{3}, {e}_{4}\; and\; {e}_{5},$ both solutions converge to the exact solution. Applying the topological idea, we have the very small positive five parameters $\left({\chi }_{{e}_{1}}, {\chi }_{{e}_{2}}, {\chi }_{{e}_{3}}{\chi }_{{e}_{4}}\; and\; {\chi }_{{e}_{5}}\right).$

Where

But, it is obvious that

Where $‖{W}_{1}‖, ‖{W}_{2}‖, ‖{W}_{3}‖, ‖{W}_{4}‖, ‖{W}_{5}‖\ne 0$

Therefore, we have

which yields that

This shows that, the special solution is unique.

5.   New numerical scheme

We define the Atanagana-Tufik proposed scheme for fractional derivative model (8) for the COVID-19 epidemic ^[35]. For this purpose, we suppose that

We express the Eq (17) in the form of fractional integral equation by applying fundamental theorem of fractional calculus.

At a given point ${t}_{n+1}, n = \mathrm{0, 1}, \mathrm{2, 3}, \dots,$ the above equation is reformulated as

Within the interval $\left[{t}_{j}, {t}_{j+1}\right],$ the function $g\left(\tau, x\left(\tau \right)\right),$ using the two-steps Lagrange polynomial interpolation, can be approximate as follows:

The above approximation can therefore be included in Eq (19) to produce

For simplicity, we let

and also

where

By using Eqs $\left(22\right)$ and $\left(23\right),$ we obtain

We obtain the following for model $\left(8\right).$

6.   Fractal fractional order model

We present the COVID-19 model $\left(7\right)$ using fractal-fractional Atangana-Baleanu derivative. We have the following model:

In order to present the numerical algorithm for the fractal-fractional COVID-19 model (26), we first describe the general system and present the steps by considering the Cauchy problem below:

The following is obtained by integrating the above equation:

Let $k\left(t, x\left(t\right)\right) = {\varTheta }_{1}{t}^{{\varTheta }_{1}-1}\mathrm{\Phi }\left(t, x\left(t\right)\right),$ then system $\left(26\right)$ becomes

At ${t}_{n+1} = \left(n+1\right)∆t,$ we have

Also, we have

Approximating the function $k\left(t, x\left(t\right)\right),$ using the Newton polynomial, we have

Using Eq $\left(31\right)$ into system $\left(30\right),$ we have

Rearranging the above system, we have

Now, calculating the integrals in system $\left(33\right),$ we get

Inserting them into system $\left(33\right),$ we get

Finally, we have the following approximation:

We obtain the following for system $\left(26\right)$

7.   Result and discussion

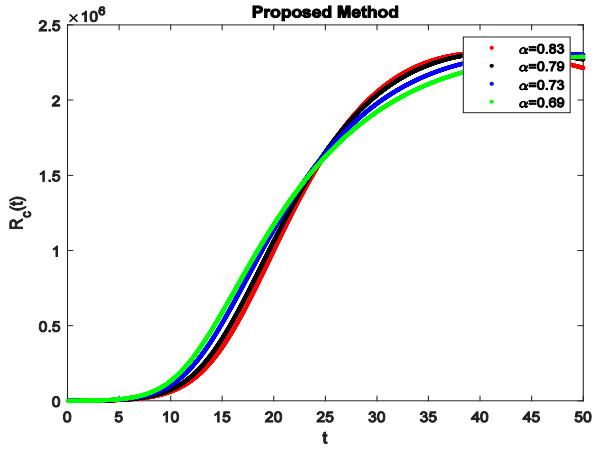
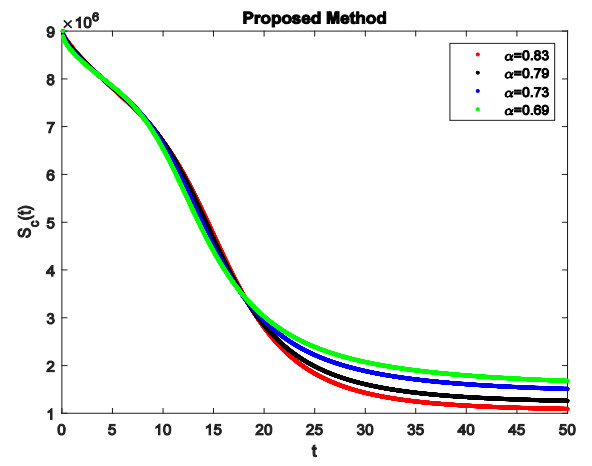
To identify the potential effectiveness of Coronavirus disease transmission in the Community, the COVID-19 fractional-order model in the case of Wuhan, China, is presented to analyze with simulations. That's why; we have used Atangana-Baleanu in Caputo sense with Mittag-Leffler law, new Atangana Toufik scheme and fractal fractional derivative model of the COVID-19 in the case of Wuhan China with the initial conditions are provided. Details of the parameters of real data are Π_c = 8859.23 × 10⁴, β_Ec = 6.11 × 10⁻⁸, β_Ic = 2.62 × 10⁻⁸, β_Vc = 3.03 × 10⁻⁸, µ_c = 3.01 × 10⁻², α_c = 0.143, ω_c = 0.01, γ_c = 0.67, ψ_1c = 0.06, τ_c = 2.0. The various numerical methods identify the mechanical features of the fractional-order model with the time-fractional parameters. The dynamics of the model has changed, and simulations have been divulged. The results of the nonlinear system memory were also detected with the help of fractional values. Figures 1–5 represents the simulations obtained by ABC method and Figures 6–10 is obtained with fractal fractional derivative. It is easily observed that in Figures 1–5, all compartments starts increasing by decreasing the fractional values which converge to steady state. Similar behavior can be seen in Figures 6–10 but converge rapidly. In Figures 1 and 3, we will see that the concentration of virus and infection rate is directly proportional to each other in Figures 6 and 8. In Figures 1 and 5, we will see that the concentration of the virus and the rate of susceptibility are inversely proportional to each other. It has been shown that physical processes are better explained using the fractional-order derivatives, which are the most notable and reliable component compared to the classical-order case. Existing non-integer-order models are less profitable compared to those operators. The behaviors of the dynamics found in the various fractional orders are shown in the form of numerical results that have been reported.

8.   Conclusions

In this paper, Atangana-Baleanu in Caputo sense, Atangana-Toufik and Fractal fractional Atangana-Baleanu differential equation model for COVID-19 disease in case of Wuhan China has been investigated. The uniqueness and stability results of the COVID-19 model are investigated by applying the fixed point theory and the iterative method. The boundedness and positivity of the given model also have been investigated. The arbitrary derivative of fractional order has been taken in the Atangana-Baleanu Caputo sense and Fractal fractional Atangana-Baleanu with Mittag-Leffler kernel. Non-linear fractional differential equations were raised from the derivative with the help of a non-singular and non-local kernel within the fractional derivative framework. Atangana-Baleanu with Smudu transform, Atangana-Toufik and Fractal fractional Atangana-Baleanu is used to obtain the derived fraction order COVID-19 model results. Comparison has been made between Atangana-Toufik and Fractal fractional Atangana-Baleanu to verify the efficiency of results. Some theoretical results are also discussed for the fractional-order model. Simulations are carried out to check the actual behaviour of COVID-19 in society. We observe that obtained results are effective for the proposed fractional-order model, which will be helpful in future to analyze the COVID-19 and for control strategies. To control the transmission of COVID-19, stay at home and putting COVID-19 positive individuals into quarantine.

Acknowledgment

The authors are thankful to the Institute of Research and Consulting Studies at King Khalid University for supporting this research through grant number # 35-69-S-2020.

Conflict of interest

The authors declare no conflict of interest.