1.
Introduction
Fractional calculus represents a significant extension of classical calculus, enabling the analysis of phenomena that cannot be adequately described by integer-order derivatives and integrals. Over the centuries, mathematicians like Euler, Laplace, Riemann, and Liouville contributed to its development. However, it was not until the 20th century that fractional calculus started gaining significant attention and applications. Fractional calculus often provides more accurate and flexible models for real-world phenomena. It can capture memory effects, non-locality, and complex dynamics that traditional integer-order calculus might miss. It has numerous applications in various fields of science and engineering, including: It is applied in the analysis of electromagnetic fields and waves, where fractional derivatives help model complex behaviors. Fractional calculus is utilized in control systems to model and analyze dynamical systems, enhancing the design of controllers for systems with memory effects. In materials science, fractional calculus models the behavior of viscoelastic materials, which exhibit both viscous and elastic characteristics, allowing for a more accurate representation of their stress-strain relationships. For some other applications in various domains, check [1,2,3,4] and the references therein.
Mathematical inequalities provide a foundational framework for understanding the behavior of functions under integration, leading to significant applications in both theoretical and applied mathematics. Convex integral inequalities are a powerful tool in mathematical analysis, providing relationships between integrals of convex functions and their values at specific points. They find applications in various fields, including probability theory, information theory, and optimization (see [5,6,7,8]). These inequalities are crucial for numerical methods, especially for estimating the error bounds in numerical integration techniques like the trapezoidal rule, Simpson's rule, and others. Several notable integral inequalities have been documented in the literature, including Hermite-Hadamard [9], Newton [10], Simpson [11], Bullen [12], and others.
Through the use of the different classes of generalized convex mappings, authors have interpreted the double Hermite and Hadamard (H-H) inequality in various ways. The double inequality was proposed by Hermite (1822–1901) and Hadamard (1865–1963). In addition to their contributions to number theory, nonlinear analysis, and complex analysis, Hermite and Hadamard have made significant contributions to other fields as well. To learn more about their contributions, see [13]. This inequality is a significant discovery in convex analysis and is widely used in various fields of applied analysis, particularly optimality analysis. Let us describe it as below.
Suppose that ψ:Ω⊆R→R is a convex mapping defined over the interval Ω of real numbers, as well as ω1,ω2∈Ω together with ω1≠ω2. Then one has (see [14]):
This inequality is applied in geometric contexts to determine correlations between a function's value at the midpoint of an interval and its average over that interval. In information theory, the inequality has been used to set boundaries and estimations, especially in relation to quantum integral inequalities and quantum calculus. An effective tool for examining a variety of economic phenomena involving convex functions, such as asset pricing and optimization as well as income distribution and production, is due to Hermite-Hadamard inequality (see [15]).
The bidimensional convex function is primarily used to prove that all convex mappings are convex over their coordinates. Additionally, there are bidimensional convex functions that are not convex (see, for instance, [16]). Hermite-Hadamard type inequality of the following kind was established for convex co-ordinated mappings on R2, that is:
Let a function ψ:[ω1,ω2]×[ω3,ω4]⊆R2→R is convex across its coordinate plane. Then, we have the following double inequalities (see [17]):
First, the authors in [18] employed inclusion order and interval-valued functions for two-dimensional double inequality and proposed a number of innovative variations of inequalities in terms of inclusions. They further show that when the interval is wrapped, these results generalize many earlier discoveries. In [19], the authors provide several new refinements and reversals for bidimensional double inequality using s-convex mappings on the rectangular plane. Furthermore, this inequality has been investigated using different types of convex mappings as well as integral operators. For example, in [20], the authors used different types of convex, continuous, and differentiable mappings and found various bounds of double inequalities. In [21], the authors define two variable logarithmic convex mappings and develop Hermite-Hadamard inequality with applications. In [22], the authors define two-dimensional preinvex type mappings and develop various types of Hermite-Hadamard inequalities. In [23], the authors exploited the q-jackson quantum double integral on the plane to established the double inequality with its fascinating applications in quantum calculus. Kalsoom et al. [24] used quantum integrals to established the Fejer and Pachapatte type inequalities utilizing two different forms of invex mappings. In [25], authors introduced a new type of fractional integral operators with singular kernels to develop Hermite-Hadamard inequality by employing several types of integral identities. In the realm of interval maps, Shi et al. [26] created multiple new bounds for double Hermite-Hadamard inequality using two types of generalized convex mappings. Zareen et al. [27] exploited generalized double fractional integrals and generated numerous novel Hermite-Hadamard type inequalities with coordinated convex mappings. Saeed et al. [28] employed generalized double fractional integrals to establish numerous novel Hermite-Hadamard type inequalities over convex set relevant to fuzzy-number-valued settings using coordinated convex mappings. In [29], the authors refined the Hadamard inequality for coordinated convex functions and explored their applications. Afzal et al. [30] exploited coordinated convex mappings to produce Hermite-Hadamard, Pachpatte, and Fejer type integral inequalities using innovative fractional integral operators via fully interval-order relations. For some further recent advancements for coordinated convex mappings, we refer to [31,32,33,34] and the references therein.
Since this article mainly deals with a cr-interval order relations, we should recollect recent advancements focusing on center-radius order relations using some other form of convex mapping. The concept of cr-order was introduced for the first time by the authors in [35] in 2014. This relation is more compatible than other order relations and has several additional properties that other interval order relations do not have. Based on their work, authors in [36] defined a new type of convex mapping for convex optimizing problems in the realm of cr-order. Liu et al. [37,38] first created discrete versions of Jensen and Hermite-Hadamard inequalities utilizing two distinct kinds of generalized convex mappings employing cr-order as a result of these discoveries. Khan and Saad [39] created several novel bounds for various kinds of double inequalities by utilizing superquadratic functions in the relam of fractional frame of reference via cr-order relation. Fahad et al. [40] used geometric and arithmetic-cr-convex functions to explore characteristics and several applications related to entropy and means. Using the concepts of cr-order and various classes of convex and Godunova-Levin functions, the authors of [41,42,43,44] generated a number of novel inequalities connected to these findings with applications. For more recent developments on comparable results using different types of related convex classes, see [45,46,47,48,49] and the references therein.
"Order relations" and "convex mappings" are the basic concepts for adjusting inequalities within interval set-valued mappings. Despite that, authors have extensively used the interval partial order relation "⊆p" to develop different types of inequalities and analyze that this type of order relation though it is not very suitable for adjusting inequalities in interval maps because there are some inequalities that are not adjusted under the same assumptions. For instance, please see reference [50] in which several major results are not developed in interval maps. To overcome this limitation, the authors established a new sort of order relation known as cr-order, whose definition is now standard. This is considered a natural generalization of all recently developed order relations such as inclusion, left-right, pseudo, up and down, and various others. Furthermore, the cr-order is full order, which means it possesses all relational features between intervals, including reflexivity, anti-symmetry, transitivity, and comparability, but the inclusion partial order relation lacks comparability between two intervals.
Inspired by well built appropriate literature, particularly these works [18,24,39,40,41], we derived a novel and improved form of inequalities employing cr-interval order. This paper is organized into five sections, beginning with an introduction and fundamental discussion of the subject connected to preliminary. In Section 3, we first show that double integral preserves cr-order, and then we show that the newly developed mappings after we apply cr-order, including midpoint and center, are both convex in nature. Next, we developed different variants of double inequalities that generalize various previous findings. Finally, in Section 4, we provide a precise conclusion and some future prospects.
2.
Preliminaries
In this section, we discuss some basic concepts related to fractional and interval calculus. Further some key concepts are not thoroughly discussed here, thus we refer to [39].
● Ri: intervals in R;
● ψ_=¯ψ: interval maps become dysfunctional;
● ⊆: inclusion interval order;
● ⪯cr: cr-interval order;
● ≤: basic order;
● ivfs: interval-valued functions.
2.1. Interval operations
Let R be the one-dimensional Euclidean space, and consider Rc the family of all non-empty compact convex subsets of R, that is
To define the Hausdorff metric in Rc, use this formula:
where d(ω1,ω2)=supν∈ω1d(ν,ω2), and d(ν,ω2)=minμ∈ω2d(ν,μ)=minμ∈ω2|ν−μ|.
Remark 2.1. The Hausdorff metric described in (2.1) is alternatively represented in the following manner:
In interval space, we call this the Moore metric.
For instance, if H1=[ω1_,¯ω1] and H2=[ω2_,¯ω2] are two closed intervals, then the Minkowski sum, scalar multiplication, and difference are defined as follows:
and
with the product
and the division
where 0∉H2.
The order relation that permeates our primary findings is outlined by Bhunia and Samanta [51]; it is commonly referred to as center-radius order.
Definition 2.1. [41] For any two intervals the center-radius order relation is defined as H1=[ω1_,¯ω2]=⟨ωc,ωr⟩=⟨ω1_+¯ω22,¯ω2−ω1_2⟩, H2=[Ω1_,¯Ω2]=⟨Ωc,Ωr⟩=⟨Ω1_+¯Ω22,¯Ω2−Ω1_2⟩, where
The relation ⪯cr satisfies the following relational properties for any three intervals H1=[ω1_,¯ω2]=⟨ωc,ωr⟩, H2=[Ω1_,¯Ω2]=⟨Ωc,Ωr⟩ and H3=[η1_,¯η2]=⟨ηc,ηr⟩: Reflexivity: H1⪯crH1. Anti-symmetry: H1⪯crH2 and H2⪯crH1. Transitivity: H1⪯crH2 and H2⪯crH3, then H1⪯crH3. Comparability: H2⪯crH3 or H3⪯crH2.
Theorem 2.1. [42] Let ψ:[ω1,ω2]→Rc be an ivfs represented by ψ(ω)=[ψ_(ω),¯ψ(ω)]. ψ∈IR([ω1,ω2]), iff ψ_(ω),¯ψ(ω)∈R([ω1,ω2]) and
Theorem 2.2. [41] Let ψ,χ:[ω1,ω2]→Rc be an ivfs defined by χ=[χ_,¯χ],ψ=[ψ_,¯ψ]. If ψ(ω)⪯crχ(ω) for all ω∈[ω1,ω2], then
Theorem 2.3. [18] Let Δ=[ω1,ω2]×[ω3,ω4]. If ψ:Δ→Rc is UD-integrable over Δ, then we have
Using Zhao et al. [18] concept of interval-valued double integrals, we provide the following definitions for Hadamard and Katugampola integrals as follows:
Definition 2.2. Let ψ∈IR([ω1,ω2]×[ω3,ω4]). For bidimensional interval-valued functions, the Hadamard integrals of order θ1,θ2>0 with ω1,ω3≥0 are represented as
and
Definition 2.3. Let ψ∈IR([ω1,ω2]×[ω3,ω4]). For bidimensional interval-valued functions, the Katugampola fractional integrals are defined as
and
We have now established a new type of double fractional integral operators under cr-order that generalize various existing operators by specifying different sorts of functions.
Definition 2.4. Let ζ:[ω1,ω2]→R be a increasing and positive monotone function on (ω1,ω2], having a continuous derivative on (ω1,ω2), and let κ:[ω3,ω4]→R be a increasing and positive monotone function on (ω3,ω4], having a continuous derivative on (ω3,ω4). Let ψ∈IR([ω1,ω2]×[ω3,ω4]). The fractional integral operators for interval-valued functions of two variables are defined by
and
for θ1,θ2>0.
Similar to the preceding definitions, we can provide the following integrals:
and
Next, according to the definition provided by the authors [17,18], we discuss the bidimensional convexity via classical and interval order relation.
Definition 2.5. [17] Let ψ:Ω=[ω1,ω2]×[ω3,ω4]⊆R2→R+ be a two-variable convex function if
holds true for every (ω1,ω2),(ω3,ω4)∈Ω along with r1,s1∈[0,1].
Definition 2.6. [18] Let ψ:Ω=[ω1,ω2]×[ω3,ω4]⊆R2→Ri+ be a two-variable set-valued convex mapping defined as ψ=[ψ_(ω,Ω),¯ψ(ω,Ω)] with 0≤ω1<ω2,0≤ω3<ω4, then we have
holds true for every (ω1,ω2),(ω3,ω4)∈Ω along with r1,s1∈[0,1].
Definition 2.7. [52] Let ψ:Ω=[ω1,ω2]×[ω3,ω4]⊆R2→Ri+ be a two-variable set-valued strongly convex mapping defined as ψ=[ψ_(ω,Ω),¯ψ(ω,Ω)] with 0≤ω1<ω2,0≤ω3<ω4 and κ1,κ2 are positive real numbers, then we have
holds true for every (ω1,ω2),(ω3,ω4)∈Ω along with r1,s1∈[0,1].
Definition 2.8. [46] Let ψ:Ω=[ω1,ω2]→Ri+ be a ivfs defined as ψ=[ψ_(ω),¯ψ(ω)] with 0≤ω1<ω2. Then ψ is cr-convex if
holds true for every (ω1,ω2)∈Ω along with r1∈[0,1].
Motivated by the aforementioned definitions, we now effectively extend Definition 2.8 into R2 by the use of cr-order.
Definition 2.9. Let ψ:Ω=[ω1,ω2]×[ω3,ω4]⊆R2→Ri+ be a two-variable set-valued cr convex mapping defined as ψ=[ψ_(ω,Ω),¯ψ(ω,Ω)] with 0≤ω1<ω2,0≤ω3<ω4, then we have
holds true for every (ω1,ω2),(ω3,ω4)∈Ω along with r1,s1∈[0,1].
Remark 2.2. (1) If ψ_≠¯ψ, we have Definition 2, as stated by Zhao et al. in [18] and Definition 6, as stated by Khan et al. in [53].
(2) If ψ_=¯ψ, we have Definition 2.1, as stated by Silvestru Sever in [17].
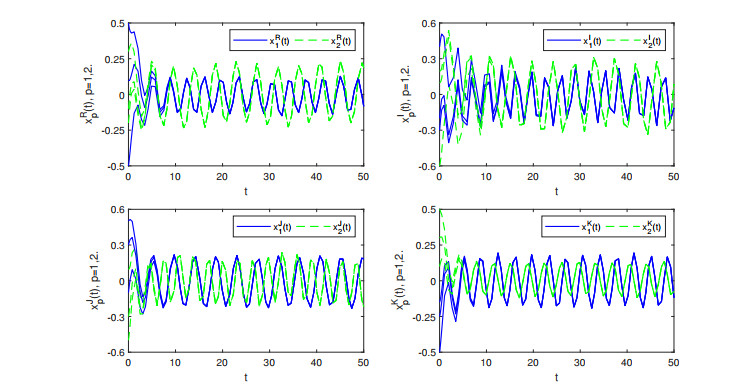
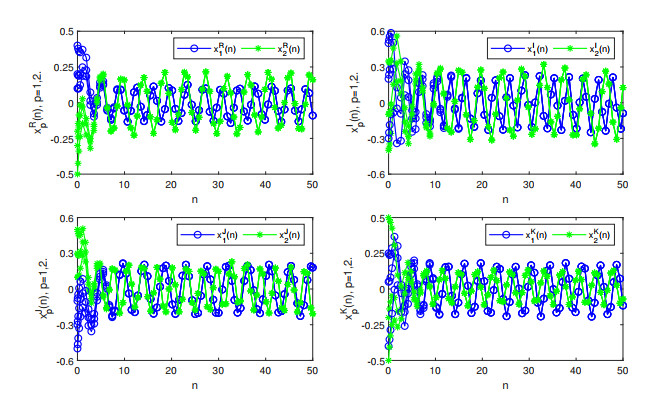
Example 2.1. Let ψ:[ω1,ω2]×[ω3,ω4]⊆R2→Ri+ be a two variable set-valued function defined as (see Figure 1)
Then (see Figure 2),
3.
The major results
In this section, we first show that double integral preserves cr-order, and then we show that the newly developed mappings after we apply cr-order, including midpoint and center, are both convex in nature. Finally, we developed different variants of double inequalities that generalize various previous findings.
Theorem 3.1. Let ψ,ϕ:[ω1,ω2]×[ω3,ω4]→Rc given by ϕ=[ϕ_(ω,Ω),¯ϕ(ω,Ω)], and ψ=[ψ_(ω,Ω),¯ψ(ω,Ω)]. If ψ,ϕ∈ IR([ω1,ω2]×[ω3,ω4]), and ψ(ω,Ω)⪯crϕ(ω,Ω) for all ω,Ω∈[ω1,ω2]×[ω3,ω4]→Rc, then
Proof. Since ψ(ω,Ω)⪯crϕ(ω,Ω) for all ω,Ω∈[ω1,ω2]×[ω3,ω4]→Rc, then we have
Since ψ,ϕ∈ IR([ω1,ω2]×[ω3,ω4]), by Theorem 2.1, we have ψ_(ω,Ω),¯ψ(ω,Ω),ϕ_(ω,Ω),¯ϕ(ω,Ω)∈IR([ω1,ω2]×[ω3,ω4]). When ψc(ω,Ω)≠ϕc(ω,Ω),∀ω,Ω∈[ω1,ω2]×[ω3,ω4], then
That is
When ψc(ω,Ω)=ϕc(ω,Ω),∀ω,Ω∈[ω1,ω2]×[ω3,ω4], then
That is
This completes the proof.□
Now, we have defined a coordinated strongly convex mapping utilizing the center-radius ordering relation.
Definition 3.1. Let ψ:Ω=[ω1,ω2]×[ω3,ω4]⊆R2→Ri+ be a two-variable cr set-valued strongly convex mapping defined as ψ=[ψ_(ω,Ω),¯ψ(ω,Ω)] with 0≤ω1<ω2,0≤ω3<ω4 and κ1,κ2 are positive real numbers, then we have
holds true for every (ω1,ω2),(ω3,ω4)∈Ω along with r1,s1∈[0,1].
Proposition 3.1. Let ψ:Ω=[ω1,ω2]×[ω3,ω4]⊆R2→Ri+ be a two-variable set-valued function represented as ψ=[ψ_(ω,Ω),¯ψ(ω,Ω)] with 0≤ω1<ω2,0≤ω3<ω4 and κ1,κ2. Then ψ is set-valued cr-strongly convex iff ψc and ψr are strongly convex functions.
Proof. As ψc and ψr are strongly coordinated convex in nature, then for all (ω1,ω2),(ω3,ω4)∈[0,1]×[0,1], we have
and
Now, if
This implies
Otherwise, we have
This implies
This can be summed up as follows using the Definition 3.1 and the previously mentioned results:
This concludes the proof. □
3.1. Hermite-Hadamard type inequalities using coordinated center-radius order relations
In this section, we employ coordinated center and radius order relations to create various new bounds for double inequality. Let ψ∈IR([ω1,ω2]×[ω3,ω4]). First, we specify the functions that will be utilized frequently:
Theorem 3.2. Let ζ:[ω1,ω2]→R be a increasing and positive monotone function on (ω1,ω2], having a continuous derivative on (ω1,ω2), and let κ:[ω3,ω4]→R be a increasing and positive monotone function on (ω3,ω4], having a continuous derivative on (ω3,ω4). Let ψ∈IR([ω1,ω2]×[ω3,ω4]), then for θ1,θ2>0 the following Hermite-Hadamard-type relation hold:
Proof. As ψ represents a set-valued coordinated cr-convex mapping on Δ, we have
for (κ1,κ3),(κ2,κ4)∈Δ. Now, for t,s∈[0,1], let κ1=tω1+(1−t)ω2,κ2=(1−t)ω1+tω2,κ3=ω3s+(1−s)ω4, and κ4=(1−s)ω3+sω4. Then, we have
Multiply the above relation by ζ′((1−t)ω1+tω2)[ζ(ω2)−ζ((1−t)ω1+tω2)]1−θ1κ′((1−s)ω3+sω4)[κ(ω4)−κ((1−s)ω3+sω4)]1−θ2, then integrate the resulting relation with respect to t,s over [0,1]×[0,1], we get
Using basic computation, we have
Making use of the variable change τ=(1−t)ω1+tω2 and η=(1−s)ω3+sω4, we obtain
That is, we have
In a similar manner, multiplying (3.3) on both sides by
and by integrating the above relation across [0,1]×[0,1], we get
Moreover, multiplying both sides of (3.3) by
and
then incorporating the aforementioned relation with reference to t,s across [0,1]×[0,1], we get
and
respectively.
Summing the relations (3.5) to (3.8), we have
This concludes the demonstration of the first part of relation in (3.1). To prove the second part in (3.1), again taking into account bidimensional convex mappings, we have
Multiplying both sides of (3.10) by
then incorporating the aforementioned relation with reference to t,s across [0,1]×[0,1], we get
Then, we get
that is,
Similarly, multiplying both sides of (3.10) by
and
and then incorporating the aforementioned relation with reference to t,s across [0,1]×[0,1], we get
and
By adding the relations (3.12) to (3.14), we have
Dividing both sides of relation (3.15) by 16 yields the second relation in (3.1). This concludes the proof.
Remark 3.1. The relationship for set-valued Riemann-Liouville integrals is as follows if we take into account ζ(ω)=ω and κ(Ω)=Ω with ψ_≠¯ψ in Theorem 3.2:
which was proved by the authors in [54].
Remark 3.2. The relationship for Riemann integrals is as follows if we take into account ζ(ω)=ω and κ(Ω)=Ω,θ1=θ2=1 with ψ_≠¯ψ in Theorem 3.2:
which was proved by the authors in [18].
Remark 3.3. The relationship for Riemann-Liouville integrals is as follows if we take into account ζ(ω)=ω and κ(Ω)=Ω with ψ_=¯ψ in Theorem 3.2:
which was proved by the authors in [55].
Remark 3.4. The relationship for fractional integrals is as follows if we take into account ψ_=¯ψ in Theorem 3.2:
which was proved by the authors in [34].
Remark 3.5. The relationship for Riemann integrals is as follows if we take into account ζ(ω)=ω and κ(Ω)=Ω,θ1=θ2=1 with ψ_≠¯ψ in Theorem 3.2, which was proved by the authors in [53].
Corollary 3.1. The relationship for Hadamard fractional integrals is as follows if we take into account ζ(ω)=lnω and κ(Ω)=lnΩ in Theorem 3.2:
Corollary 3.2. The relationship for Katugampola fractional integrals is as follows if we take into account ζ(ω)=ωpη and κ(Ω)=Ωσσ,η,σ>0 in Theorem 3.2:
Example 3.1. Let ψ(ω,Ω)=[24eω+24eΩ+4eΩω+144,216eω+216eΩ+36eΩω+1296],[ω1,ω2]=[0,1],[ω3,ω4]=[0,1],θ1=θ2=1, ζ(ω)=lnω and κ(Ω)=lnΩ, then we have
Thus,
As a result, Theorem 3.2 holds true.
Theorem 3.3. Considering the same hypotheses that were considered in Theorem 3.2, we get the following center-radius order relationships:
Proof. As \psi is a bidimesional convex on \Delta , if we have the mapping \sigma_{\mathtt{x}}^1:[\omega_3, \omega_4] \rightarrow \mathsf{R}, \sigma_{\mathtt{x}}^1(\mathtt{y}) = \psi(\mathtt{x}, \mathtt{y}) , then \sigma_{\mathtt{x}}^1(\mathtt{y}) is convex \forall x \in[\omega_1, \omega_2] and \mathcal{H}_{\mathtt{x}}^1(\mathtt{y}) = \sigma_{\mathtt{x}}^1(\mathtt{y})+\widetilde{\sigma_{\mathtt{x}}^1}(\mathtt{y}) = \psi(\mathtt{x}, \mathtt{y})+\psi_2(\mathtt{x}, \mathtt{y}) = \mathcal{G}(\mathtt{x}, \mathtt{y}), then for the convex mapping \sigma_{\mathtt{x}}^1(\mathtt{y}) , we have
that is,
Multiplying the relation (3.17) by
and
Next, we obtain by integrating the given results from \omega_1 to \omega_2 with regard to \mathtt{x} .
and
respectively.
Also, if we define another mapping \sigma_{\mathtt{x}}^2:[\omega_3, \omega_4] \rightarrow \mathsf{R}, \sigma_{\mathtt{x}}^2(\mathtt{y}) = \psi_1(\mathtt{x, y}) , then \sigma_{\mathtt{x}}^2(\mathtt{y}) is convex \forall x \in[\omega_1, \omega_2] and \mathcal{H}_\mathtt{x}^2(\mathtt{y}) = \psi_1(\mathtt{x, y})+\psi_3(\mathtt{x, y}) = \mathcal{K}(\mathtt{x, y}) , then for the convex function \sigma_{\mathtt{x}}^2(\mathtt{y}) , we have
that is,
Similarly, multiplying the relation (3.20) by
and
Therefore, from \omega_1 to \omega_2 , integrating the acquired output with reference to \mathtt{x} , we obtain
and
respectively.
Moreover, if we have mapping {\sigma_{\mathtt{y}}}^1:[\omega_1, \omega_2] \rightarrow \mathsf{R}, {\sigma_{\mathtt{y}}}^1(\mathtt{x}) = \psi(\mathtt{x, y}) , then {\sigma_{\mathtt{y}}}^1(\mathtt{x}) is convex for all \forall \mathtt{y}\in[\omega_3, \omega_4] and \mathcal{H}_y^1(\mathtt{x}) = {\sigma_{\mathtt{y}}}^1(\mathtt{x})+\widetilde{{\sigma_{\mathtt{y}}}^1}(\mathtt{x}) = \psi(\mathtt{x, y})+\psi_1(\mathtt{x, y}) = \mathcal{H}(\mathtt{x, y}) , then for the convex function {\sigma_{\mathtt{y}}}^1(\mathtt{x}) , we have
that is,
Multiplying the relation (3.23) by
and
Next, by integrating the known findings from \omega_3 to \omega_4 with respect to \mathtt{y} , we derive the subsequent relation:
and
respectively.
Furthermore, if we have mapping {\sigma_{\mathtt{y}}}^2:[\omega_1, \omega_2] \rightarrow \mathsf{R}, {\sigma_{\mathtt{y}}}^2(\mathtt{x}) = \psi_2(\mathtt{x}, \mathtt{y}) , then {\sigma_{\mathtt{y}}}^2(\mathtt{x}) is convex for all \mathtt{y}\in[\omega_3, \omega_4] and \mathcal{H}_{\mathtt{y}}^2(\mathtt{x}) = {\sigma_{\mathtt{y}}}^2(\mathtt{x})+\widetilde{{\sigma_{\mathtt{y}}}^2}(\mathtt{x}) = \psi_2(\mathtt{x}, \mathtt{y})+\psi_3(\mathtt{x}, \mathtt{y}) = \mathcal{L}(\mathtt{x}, \mathtt{y}) , the for the convex mapping {\sigma_{\mathtt{y}}}^2(\mathtt{x}) , we have
that is,
Multiplying the relations (3.28) by
and
subsequently integrating the established findings from \omega_3 to \omega_4 with respect to \mathtt{y} , we derive the following relations:
and
respectively.
Again, if we define the mapping {\sigma_{\mathtt{y}}}^2:[\omega_1, \omega_2] \rightarrow \mathsf{R}, {\sigma_{\mathtt{y}}}^2(\mathtt{x}) = \psi_2(\mathtt{x}, \mathtt{y}) , then {\sigma_{\mathtt{y}}}^2(\mathtt{x}) is convex \forall \mathtt{y} \in[\omega_3, \omega_4] and \mathcal{H}_{\mathtt{y}}^2(\mathtt{x}) = {\sigma_{\mathtt{y}}}^2(\mathtt{x})+\widetilde{{\sigma_{\mathtt{y}}}^2}(\mathtt{x}) = \psi_2(\mathtt{x}, \mathtt{y})+\psi_3(\mathtt{x}, \mathtt{y}) = \mathcal{L}(\mathtt{x}, \mathtt{y}) , then for the convex mapping {\sigma_{\mathtt{y}}}^2(\mathtt{x}) , we have
that is,
Similarly, multiplying the relations (3.31) by
and
upon integrating the acquired outcomes concerning \mathtt{y} between \omega_3 and \omega_4 , we derive the subsequent relationships:
and
respectively.
Summing the relations (3.18), (3.19), (3.21), (3.22), (3.26), (3.27)–(3.30) and (3.33), we have the following result:
That is, we have
This concludes the verification of relations second and third in (3.16). On the other hand, for symmetric function, we have the following relation:
As \psi is set-valued bidimensional convex on \Delta , by using relation (3.34), we obtain
and similarly, we have
Combining the relations (3.35) and (3.36), we obtain the first relation in (3.16). For the second relation, we following double relation:
By using relation (3.37), we obtain the following relations:
and
Combining the relations (3.38) to (3.39), we obtain the last relation in (3.16). This completes the proof. □
Remark 3.6. If we consider \zeta(\mathtt{t}) = \mathtt{t} and \kappa(\mathtt{s}) = \mathtt{s} in Theorem 3.3, then we obtain the following relationship for Riemann-Liouville integrals:
Corollary 3.3. If we consider \zeta(\mathtt{t}) = \mathtt{t} and \kappa(\mathtt{s}) = \mathtt{s} in Theorem 3.3, then we obtain the following relationship for Hadamard integrals:
Corollary 3.4. If we consider \zeta(\mathtt{t}) = \frac{{\mathtt{t}}^\eta}{\eta} and \kappa(\mathtt{s}) = \frac{{\mathtt{s}}^\sigma}{\sigma} in Theorem 3.3, then we obtain the following relationship for Katugampola integrals:
4.
Discussion and conclusions with future remarks
Coordinated convex functions apply the concept of convexity to functions defined as a product of intervals, allowing for more detailed analysis in multivariable settings. The conclusion of these functions focuses on their integral inequalities and applications in mathematical analysis. These functions enable the application of mathematical inequalities that are essential for analyzing and ensuring the stability, optimality, and control of complex systems. In this paper, we develop various novel bounds and refinements for weighted Hermite-Hadamard inequalities as well as their product form by employing new types of fractional integral operators under a cr-order relation. Additionally, we demonstrate that by means of coordinated center-radius order relations for these integral operators, various new findings can be obtained for Katugampola and Hadamard integrals operators. Furthermore, we show that this type of order relation preserves the integral structure. In addition, as a distinct characteristic from pictorial view, we show that this order is convex in nature, whereas inclusion order is non-convex. A number of interesting examples are presented in support of the major findings. It will be interesting in the future if readers take motivation from these findings and construct results using Itô's lemma as well as quantum calculas, multiplicative calculas, fuzzy order relations, and various other fractional integrals.
Additionally, in a very recent study, Afzal et al. [56,57] developed results using a new approach; more specifically, they used tensor Hilbert spaces and variable exponent spaces, which is an extremely new approach to Hermite-Hadamard inequality and its related results. We also suggest readers extend these results in the sense of these spaces that utilize a variety of convex mappings under norm structures and modular function spaces that have not been initiated yet for coordinated convexity of any kind.
Author's contributions
W. Afzal: Conceptualization, Data curation, Writing-original draft, Funding acquisition, Investigation, Visualization; M. Abbas: Conceptualization, Formal analysis, Writing-original draft, Supervision, Validation, Writing-review & editing; J. Ro: Investigation, Project administration, Visualization; K. H. Hakami: Data curation, Formal analysis, Methodology; H. Zogan: Methodology, Project administration, Software. All authors have read and approved the final version of the manuscript for publication.
Acknowledgements
This work was supported by the National Research Foundation of Korea (NRF) grant funded by the Korea government (MSIT) (No. NRF-2022R1A2C2004874), and the Korea Institute of Energy Technology Evaluation and Planning (KETEP) and the Ministry of Trade, Industry & Energy (MOTIE) of the Republic of Korea (No. 20214000000280).
Conflict of interest
The authors declare that they have no competing interests.










 DownLoad:
DownLoad: