Search Advanced

AIMS Mathematics

2021, Volume 6, Issue 9: 10055-10069. doi: 10.3934/math.2021584
Previous Article Next Article
Research article Special Issues

Computation of traveling wave solution for nonlinear variable-order fractional model of modified equal width equation

  • 1.
    Department of Applied Mathematics and Statistics, Institute of Space Technology, P.O. Box 2750, Islamabad 44000, Pakistan
  • 2.
    Institute of Mathematical Science, Faculty of Science, University of Malaya, Kuala Lumpur 50603, Malaysia
  • 3.
    Department of Basic Science and Related Studies, Quaid e Awam University of Engineering Science and Technology (Campus), Larkana 77150, Pakistan
  • 4.
    Department of Mathematics, Faculty of Science, Jiangsu University, Zhenjiang 212013, China
  • 5.
    Department of Mathematics, Obour Institutes, Cairo 11828, Egypt
  • Received: 06 April 2021 Accepted: 28 June 2021 Published: 07 July 2021

  • MSC : 35C07, 35C08, 35K05, 35P99

  • The Variable-order fractional operators (VO-FO) have considered mathematically formalized recently. The opportunity of verbalizing evolutionary leading equations has led to the effective application to the modeling of composite physical problems ranging from mechanics to transport processes, to control theory, to biology. In this paper, find the closed form traveling wave solutions for nonlinear variable-order fractional evolution equations reveal in all fields of sciences and engineering. The variable-order evolution equation is an impressive mathematical model describes the complex dynamical problems. Here, we discuss space-time variable-order fractional modified equal width equation (MEWE) and used exp $ (-\phi(\xi)) $ method in the sense of Caputo fractional-order derivative. Based on variable order derivative and traveling wave transformation convert equation into nonlinear ordinary differential equation (ODE). As a result, constructed new exact solutions for nonlinear space-time variable-order fractional MEWE. It clearly shows that the nonlinear variable-order evolution equations are somewhat natural and efficient in mathematical physics.

    Citation: Umair Ali, Sanaullah Mastoi, Wan Ainun Mior Othman, Mostafa M. A Khater, Muhammad Sohail. Computation of traveling wave solution for nonlinear variable-order fractional model of modified equal width equation[J]. AIMS Mathematics, 2021, 6(9): 10055-10069. doi: 10.3934/math.2021584

    Related Papers:

  • The Variable-order fractional operators (VO-FO) have considered mathematically formalized recently. The opportunity of verbalizing evolutionary leading equations has led to the effective application to the modeling of composite physical problems ranging from mechanics to transport processes, to control theory, to biology. In this paper, find the closed form traveling wave solutions for nonlinear variable-order fractional evolution equations reveal in all fields of sciences and engineering. The variable-order evolution equation is an impressive mathematical model describes the complex dynamical problems. Here, we discuss space-time variable-order fractional modified equal width equation (MEWE) and used exp $ (-\phi(\xi)) $ method in the sense of Caputo fractional-order derivative. Based on variable order derivative and traveling wave transformation convert equation into nonlinear ordinary differential equation (ODE). As a result, constructed new exact solutions for nonlinear space-time variable-order fractional MEWE. It clearly shows that the nonlinear variable-order evolution equations are somewhat natural and efficient in mathematical physics.


    加载中


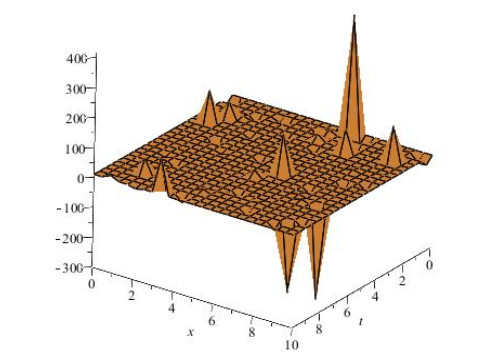
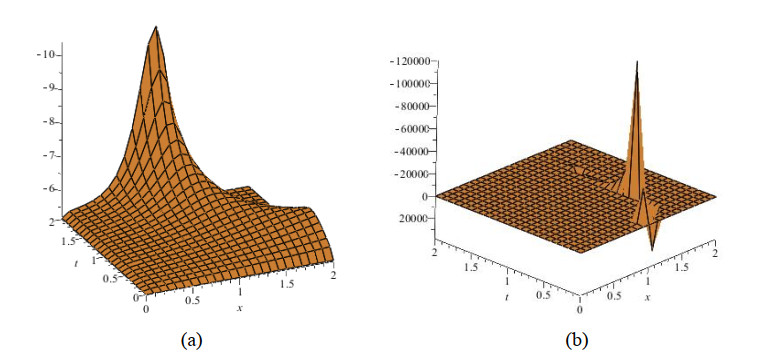
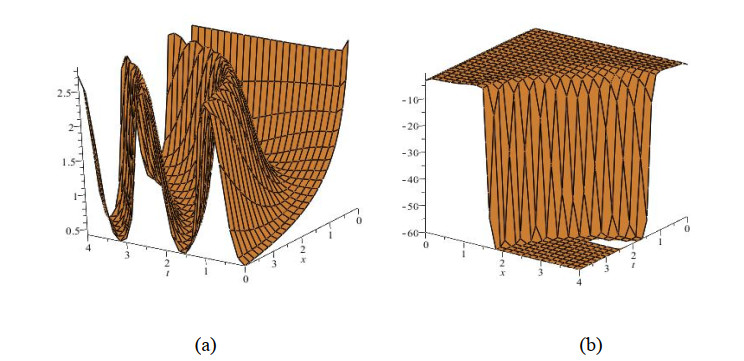
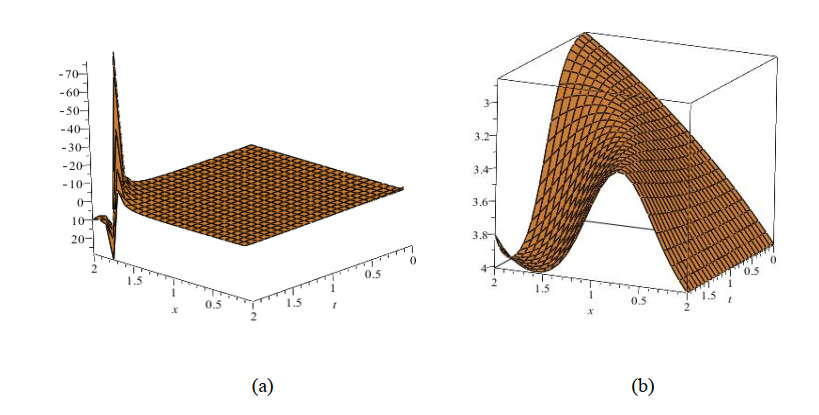
    [1] A. Bekir, Ö. Güner, The G' G-expansion method using modified Riemann-Liouville derivative for some space-time fractional differential equations, Ain Shams Eng. J., 5 (2014), 959-965.
    [2] Z. Bin, (G'/G)-expansion method for solving fractional partial differential equations in the theory of mathematical physics, Commun. Theor. Phys., 58 (2012), 623. doi: 10.1088/0253-6102/58/5/02
    [3] U. Ali, M. Sohail, M. Usman, F. A. Abdullah, I. Khan, K. S. Nisar, Fourth-order difference approximation for time-fractional modified sub-diffusion equation, Symmetry, 12 (2020), 691. doi: 10.3390/sym12050691
    [4] U. Ali, F. A. Abdullah, A. I. Ismail, Crank-Nicolson finite difference method for two-dimensional fractional sub-diffusion equation, J. Interpolation Approximation Sci. Comput., (2017), 18-29.
    [5] Y. Jiang, J. Ma, High-order finite element methods for time-fractional partial differential equations, J. Comput. Appl. Math., 235 (2011), 3285-3290. doi: 10.1016/j.cam.2011.01.011
    [6] M. H. Srivastava, H. Ahmad, I. Ahmad, P. Thounthong, N. M. Khan, Numerical simulation of three-dimensional fractional-order convection-diffusion PDEs by a local meshless method, Therm. Sci., 25 (2021), 347-358. doi: 10.2298/TSCI200225210S
    [7] P. Zhuang, F. Liu, Finite difference approximation for two-dimensional time fractional diffusion equation, J. Algorithms Comput. Technol., 1 (2007), 1-16. doi: 10.1260/174830107780122667
    [8] H. Ahmad, T. A. Khan, S. W. Yao, An efficient approach for the numerical solution of fifth order KdV equations, Open Math., 18 (2020), 738-748. doi: 10.1515/math-2020-0036
    [9] M. Cui, Compact alternating direction implicit method for two-dimensional time fractional diffusion equation, J. Comput. Phys., 231 (2012), 2621-2633. doi: 10.1016/j.jcp.2011.12.010
    [10] Y. Jiang, J. Ma, High-order finite element methods for time-fractional partial differential equations, J. Comput. Appl. Math., 235 (2011), 3285-3290. doi: 10.1016/j.cam.2011.01.011
    [11] U. Ali, F. A. Abdullah, Explicit Saul'yev finite difference approximation for two-dimensional fractional sub-diffusion equation, AIP Conference Proceedings, 1974 (2018), 020111. doi: 10.1063/1.5041642
    [12] I. Ahmad, A. Abouelregal, H. Ahmad, P. Thounthong, M. Abdel-Aty, A new analyzing method for hyperbolic telegraph equation, Authorea, 2020.
    [13] A. T. Balasim, N. H. M. Ali, A comparative study of the point implicit schemes on solving the 2D time fractional cable equation, AIP Conference Proceedings, 1870 (2017), 040050. doi: 10.1063/1.4995882
    [14] A. Akgül, A novel method for a fractional derivative with non-local and non-singular kernel, Chaos, Solitons Fractals, 114 (2018), 478-482. doi: 10.1016/j.chaos.2018.07.032
    [15] A. Akgül, S. Ahmad, A. Ullah, D. Baleanu, E. K. Akgül, A novel method for analysing the fractal fractional integrator circuit, Alexandria Eng. J., 60 (2021), 3721-3729. doi: 10.1016/j.aej.2021.01.061
    [16] A. Akgül, D. Baleanu, Analysis and applications of the proportional Caputo derivative, Adv. Differ. Equations, 2021 (2021), 1-12. doi: 10.1186/s13662-020-03162-2
    [17] A. Akgül, Analysis and new applications of fractal fractional differential equations with power law kernel, Discrete Contin. Dyn. Syst.-S, 2020.
    [18] N. Shang, B. Zheng, Exact solutions for three fractional partial differential equations by the (G'/G) method, Int. J. Appl. Math., 43 (2013), 114-119.
    [19] A. Yokus, H. Durur, H. Ahmad, P. Thounthong, Y. F. Zhang, Construction of exact traveling wave solutions of the Bogoyavlenskii equation by (G'/G, 1/G)-expansion and (1/G')-expansion techniques, Results Phys., (2020), 103409.
    [20] H. K. Barman, A. R. Seadawy, M. A. Akbar, D. Baleanu, Competent closed form soliton solutions to the Riemann wave equation and the Novikov-Veselov equation, Results Phys., (2020), 103-131.
    [21] A. J. A. M. Jawad, M. D. Petković, A. Biswas, Modified simple equation method for nonlinear evolution equations, Appl. Math. Comput., 217 (2010), 869-877.
    [22] Y. Zhang, Solving STO and KD equations with modified Riemann-Liouville derivative using improved (G'/G)-expansion function method, IAENG Int. J. Appl. Math., 45 (2015), 16-22.
    [23] T. Islam, M. A. Akbar, A. K. Azad, Traveling wave solutions to some nonlinear fractional partial differential equations through the rational (G'/G)-expansion method, J. Ocean Eng. Sci., 3 (2018), 76-81. doi: 10.1016/j.joes.2017.12.003
    [24] A. R. Seadawy, D. Yaro, D. Lu, Propagation of nonlinear waves with a weak dispersion via coupled (2+1)-dimensional Konopelchenko-Dubrovsky dynamical equation, Pramana, 94 (2020), 17. doi: 10.1007/s12043-019-1879-z
    [25] A. Başhan, N. M. Yağmurlu, Y. Uçar, A. Esen, Finite difference method combined with differential quadrature method for numerical computation of the modified equal width wave equation, Numer. Methods Partial Differ. Equations, 37 (2021), 690-706. doi: 10.1002/num.22547
    [26] A. Başhan, N. M. Yağmurlu, Y. Uçar, A. Esen, An effective approach to numerical soliton solutions for the Schrödinger equation via modified cubic B-spline differential quadrature method, Chaos, Solitons Fractals, 100 (2017), 45-56. doi: 10.1016/j.chaos.2017.04.038
    [27] A. Başhan, N. M. Yağmurlu, Y. Uçar, A. Esen, A new perspective for the numerical solution of the modified equal width wave equation, Math. Methods Appl. Sci., 2021.
    [28] A. Başhan, A. Esen, Single soliton and double soliton solutions of the quadratic‐nonlinear Korteweg‐de Vries equation for small and long‐times, Numer. Methods Partial Differ. Equations, 37 (2021), 1561-1582. doi: 10.1002/num.22597
    [29] A. Başhan, Y. Uçar, N. M. Yağmurlu, A. Esen, A new perspective for quintic B-spline based Crank-Nicolson-differential quadrature method algorithm for numerical solutions of the nonlinear Schrödinger equation, Eur. Phys. J. Plus, 133 (2018), 1-15. doi: 10.1140/epjp/i2018-11804-8
    [30] A. Başhan, A mixed methods approach to Schrödinger equation: Finite difference method and quartic B-spline based differential quadrature method, Int. J. Optim. Control: Theor. Appl. (IJOCTA), 9 (2019), 223-235. doi: 10.11121/ijocta.01.2019.00709
    [31] A. M. Wazwaz, The Hirota's direct method for multiple-soliton solutions for three model equations of shallow water waves, Appl. Math. Comput., 201 (2008), 489-503.
    [32] K. Hosseini, A. R. Seadawy, M. Mirzazadeh, M. Eslami, S. Radmehr, D. Baleanu, Multiwave, multicomplexiton, and positive multicomplexiton solutions to a (3+1)-dimensional generalized breaking soliton equation, Alexandria Eng. J., 59 (2020), 3473-3479.
    [33] U. Ali, M. Sohail, F. A. Abdullah, An efficient numerical scheme for variable-order fractional sub-diffusion equation, Symmetry, 12 (2020), 1437. doi: 10.3390/sym12091437
    [34] W. G. Glöckle, T. F. Nonnenmacher, A fractional calculus approach to self-similar protein dynamics, Biophys. J., 68 (1995), 46-53. doi: 10.1016/S0006-3495(95)80157-8
    [35] H. Sun, A. Chang, Y. Zhang, W. Chen, A review on variable-order fractional differential equations: Mathematical foundations, physical models, numerical methods, and applications, Fractional Calculus Appl. Anal., 22 (2019), 27-59.
    [36] Y. Shekari, A. Tayebi, M. H. Heydari, A meshfree approach for solving 2D variable-order fractional nonlinear diffusion-wave equation, Comput. Methods Appl. Mech. Eng., 350 (2019), 154-168. doi: 10.1016/j.cma.2019.02.035
    [37] C. M. Chen, F. Liu, I. Turner, V. Anh, Numerical methods with fourth-order spatial accuracy for variable-order nonlinear Stokes' first problem for a heated generalized second grade fluid, Comput. Math. Appl., 62 (2011), 971-986. doi: 10.1016/j.camwa.2011.03.065
    [38] P. Zhuang, F. Liu, V. Anh, I. Turner, Numerical methods for the variable-order fractional advection-diffusion equation with a nonlinear source term, SIAM J. Numer. Anal., 47 (2009), 1760-1781. doi: 10.1137/080730597
    [39] C. M. Chen, F. Liu, V. Anh, I. Turner, Numerical schemes with high spatial accuracy for a variable-order anomalous subdiffusion equation, SIAM J. Scientific Comput., 32 (2010), 1740-1760. doi: 10.1137/090771715
    [40] J. T. Katsikadelis, Numerical solution of variable order fractional differential equations, 2018. Available from: https: //arXiv.org/abs/1802.00519.
    [41] H. Sun, A. Chang, Y. Zhang, W. Chen, A review on variable-order fractional differential equations: Mathematical foundations, physical models, numerical methods and applications, Fractional Calculus Appl. Anal., 22 (2019), 27-59.
    [42] U. Ali, Numerical solutions for two-dimensional time-fractional differential sub-diffusion equation, Ph.D. Thesis, University Sains Malaysia, Penang, Malaysia, 2019.
    [43] S. G. Samko, B. Ross, Integration and differentiation to a variable fractional order, Integr. Transforms Spec. Funct., 1 (1993), 277-300. doi: 10.1080/10652469308819027
    [44] U. Ali, F. A. Abdullah, S. T. Mohyud-Din, Modified implicit fractional difference scheme for 2D modified anomalous fractional sub-diffusion equation, Adv. Differ. Equations, 2017 (2017), 1-14.
    [45] S. Bibi, S. T. Mohyud-Din, U. Khan, N. Ahmed, Khater method for nonlinear Sharma Tasso-Olever (STO) equation of fractional order, Results Phys., 7 (2017), 4440-4450. doi: 10.1016/j.rinp.2017.11.008
    [46] A. Coronel-Escamilla, J. F. Gómez-Aguilar, L. Torres, R. F. Escobar-Jiménez, M. Valtierra-Rodríguez, Synchronization of chaotic systems involving fractional operators of Liouville-Caputo type with variable-order, Phys. A: Stat. Mech. Appl., 487 (2017), 1-21.
    [47] J. F. Gómez-Aguilar, Chaos in a nonlinear Bloch system with Atangana-Baleanu fractional derivatives, Numer. Methods Partial Differ. Equations, 34 (2018), 1716-1738.
    [48] C. J. Zúñiga-Aguilar, H. M. Romero-Ugalde, J. F. Gómez-Aguilar, R. F. Escobar-Jiménez, M. Valtierra-Rodríguez, Solving fractional differential equations of variable-order involving operators with Mittag-Leffler kernel using artificial neural networks, Chaos, Solitons Fractals, 103 (2017), 382-403.
    [49] A. Coronel-Escamilla, J. F. Gómez-Aguilar, L. Torres, M. Valtierra-Rodriguez, R. F. Escobar-Jiménez, Design of a state observer to approximate signals by using the concept of fractional variable-order derivative, Digital Signal Process., 69 (2017), 127-139.
    [50] K. D. Dwivedi, S. Das, J. F. Gomez-Aguilar, Finite difference/collocation method to solve multi term variable‐order fractional reaction-advection-diffusion equation in heterogeneous medium, Numer. Methods Partial Differ. Equations, 37 (2021), 2031-2045.
    [51] C. J. Zúñiga-Aguilar, J. F. Gómez-Aguilar, H. M. Romero-Ugalde, R. F. Escobar-Jiménez, G. Fernández-Anaya, F. E. Alsaadi, Numerical solution of fractal-fractional Mittag-Leffler differential equations with variable-order using artificial neural networks, Eng. Comput., (2021), 1-14.
    [52] J. F. Li, H. Jahanshahi, S. Kacar, Y. M. Chu, J. F. Gómez-Aguilar, N. D. Alotaibi, et al, On the variable-order fractional memristor oscillator: Data security applications and synchronization using a type-2 fuzzy disturbance observer-based robust control, Chaos, Solitons Fractals, 145 (2021), 110681.
    [53] A. H. Khater, D. K. Callebaut, W. Malfliet, A. R. Seadawy, Nonlinear dispersive Rayleigh-Taylor instabilities in magnetohydrodynamic flows, Phys. Scripta, 64 (2001), 533. doi: 10.1238/Physica.Regular.064a00533
    [54] A. H. Khater, D. K. Callebaut, A. R. Seadawy, Nonlinear dispersive instabilities in Kelvin-Helmholtz magnetohydrodynamic flows, Phys. Scr., 67 (2003), 340. doi: 10.1238/Physica.Regular.067a00340
    [55] M. A. Helal, A. R. Seadawy, Variational method for the derivative nonlinear Schrödinger equation with computational applications, Phys. Scr., 80 (2009), 035004. doi: 10.1088/0031-8949/80/03/035004
    [56] M. A. Helal, A. R. Seadawy, Exact soliton solutions of a D-dimensional nonlinear Schrödinger equation with damping and diffusive terms, Z. Angew. Math. Phys., 62 (2011), 839. doi: 10.1007/s00033-011-0117-4
    [57] R. Aly, Exact solutions of a two-dimensional nonlinear Schrödinger equation, Appl. Math. Lett., 25 (2012), 687-691. doi: 10.1016/j.aml.2011.09.030
    [58] A. R. Seadawy, Fractional solitary wave solutions of the nonlinear higher-order extended KdV equation in a stratified shear flow: Part I, Comput. Math. Appl., 70 (2015), 345-352. doi: 10.1016/j.camwa.2015.04.015
    [59] A. R. Seadawy, Approximation solutions of derivative nonlinear Schrödinger equation with computational applications by variational method, Eur. Phys. J. Plus, 130 (2015), 1-10. doi: 10.1140/epjp/i2015-15001-1
    [60] A. R. Seadawy, Three-dimensional nonlinear modified Zakharov-Kuznetsov equation of ion-acoustic waves in a magnetized plasma, Comput. Math. Appl., 71 (2016), 201-212. doi: 10.1016/j.camwa.2015.11.006
    [61] M. Bilal, A. R. Seadawy, M. Younis, S. T. R. Rizvi, K. El-Rashidy, S. F. Mahmoud, Analytical wave structures in plasma physics modelled by Gilson-Pickering equation by two integration norms, Results Phys., 23 (2021), 103959. doi: 10.1016/j.rinp.2021.103959
    [62] A. R. Seadawy, M. Bilal, M. Younis, S. T. R. Rizvi, S. Althobaiti, M. M. Makhlouf, Analytical mathematical approaches for the double-chain model of DNA by a novel computational technique, Chaos, Solitons Fractals, 144 (2021), 110669. doi: 10.1016/j.chaos.2021.110669
    [63] A. Ali, A. R. Seadawy, D. Lu, Dispersive analytical soliton solutions of some nonlinear waves dynamical models via modified mathematical methods, Adv. Differ. Equations, 2018 (2018), 1-20. doi: 10.1186/s13662-017-1452-3
    [64] M. Arshad, A. R. Seadawy, D. Lu, Bright-dark solitary wave solutions of generalized higher-order nonlinear Schrödinger equation and its applications in optics, J. Electromagn. Waves Appl., 31 (2017), 1711-1721. doi: 10.1080/09205071.2017.1362361
    [65] S. T. R. Rizvi, A. R. Seadawy, F. Ashraf, M. Younis, H. Iqbal, D. Baleanu, Lump and interaction solutions of a geophysical Korteweg-de Vries equation, Results Phys., 19 (2020), 103661. doi: 10.1016/j.rinp.2020.103661
    [66] A. R. Seadawy, D. Kumar, K. Hosseini, F. Samadani, The system of equations for the ion sound and Langmuir waves and its new exact solutions, Results Phys., 9 (2018), 1631-1634. doi: 10.1016/j.rinp.2018.04.064
    [67] N. Cheemaa, A. R. Seadawy, S. Chen, More general families of exact solitary wave solutions of the nonlinear Schrödinger equation with their applications in nonlinear optics, Eur. Phys. J. Plus, 133 (2018), 1-9. doi: 10.1140/epjp/i2018-11804-8
    [68] N. Cheemaa, A. R. Seadawy, S. Chen, Some new families of solitary wave solutions of the generalized Schamel equation and their applications in plasma physics, Eur. Phys. J. Plus, 134 (2019), 117. doi: 10.1140/epjp/i2019-12467-7
    [69] Y. S. Özkan, E. Yaşar, A. R. Seadawy, On the multi-waves, interaction and Peregrine-like rational solutions of perturbed Radhakrishnan-Kundu-Lakshmanan equation, Phys. Scr., 95 (2020), 085205.
    [70] A. R. Seadawy, N. Cheemaa, Some new families of spiky solitary waves of one-dimensional higher-order K-dV equation with power law nonlinearity in plasma physics, Indian J. Phys., 94 (2020), 117-126. doi: 10.1007/s12648-019-01442-6
    [71] D. Lu, A. R. Seadawy, A. Ali, Dispersive traveling wave solutions of the equal-width and modified equal-width equations via mathematical methods and its applications, Results Phys., 9 (2018), 313-320. doi: 10.1016/j.rinp.2018.02.036
  • Reader Comments
  • © 2021 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)
通讯作者: 陈斌, bchen63@163.com
  • 1. 

    沈阳化工大学材料科学与工程学院 沈阳 110142

  1. 本站搜索
  2. 百度学术搜索
  3. 万方数据库搜索
  4. CNKI搜索
AIMS Mathematics
1.8 3.4

Metrics

Article views(3353) PDF downloads(235) Cited by(19)

Preview PDF
Download XML
Export Citation
Article outline

Figures and Tables

Figures(5)

Other Articles By Authors

Related pages

Tools

/

DownLoad:  Full-Size Img  PowerPoint
Return
Return

Catalog