General equilibrium of Bertrand game: A spatial computational approach

1.   Introduction

After all these years the equilibrium and stability of Bertrand ^[1] (price) competition in an identical product market has been a topic of extensively study. Although the cases where the number and stability of equilibrium points have been thoroughly discussed, the case where the number and stability of general equilibrium points of n firms based on space agglomeration has not been analyzed. Our purpose of the present paper is to fill this gap.

For price equilibrium. Since 1883, Bertrand's comment of Cournot's oligopoly turned out to the widely used model ^[2]. To this day, the Bertrand oligopoly model is one of the foundations of game theory and microeconomics. It has been a topic of extensively study of the equilibrium of Bertrand. Dastidar ^[3,4] detected that there exists a Bertrand's equilibrium in a duopoly competition with a homogeneous product when the cost function is completely superadditive on [0, $\infty$). The result showed that the values of equilibrium price are much between both firms, and it exists a non-degenerate mixed equilibrium involving the prices (Hoernig, ^[5]). Sekiguchi et al. ^[6] showed, on quantum version in duopoly market, the equilibrium of the model of price competition also existed. Ogawa and Kato ^[7] revealed that the equilibrium price under two firms setting those prices simultaneously has a range and it equals to the study of Dastidar ^[3]. With the increasing of speed of adjustment, under bounded rationality (Zhang et al., ^[8]; Bertrand, ^[9]), the stability of Nash equilibrium point of a duopoly game of Bertrand model will change and cause bifurcation and chaos to occur (Ahmed et al., ^[10]; Ma et al., ^[11]). In an asymmetric triopoly, a continuum of equilibria may exist (Hirata, ^[12]), and the motion of the system is chaotic (Rofin and Mahanty, ^[13]). In the dynamical system of four heterogeneous firms, the existence and stability of fixed points were investigated, and it showed the chaotic behavior of the game (Elsadany et al., ^[14]). Ania ^[15] revealed a characterization of the set of evolutionarily stable prices existed under Bertrand oligopoly with homogeneous product by evolutionary game theory. Although an equilibria exists in a conspecific product market, the competitive price equilibrium is the only steady one. In the long run, the prices of Bertrand competition under decreasing returns will converge to the Walrasian outcome (Abbink and Brandts, ^[16]; Carlos et al., ^[17]). Specifically, as long as the equilibrium identity is nonblack, the equilibrium price shrinks to the competitive price (Hirata and Matsumura, ^[18]). When there are more than five oligarchs, the equilibrium price become more chaosic and may not be derived. Yu and Yu ^[19] found Nash equilibrium only existed in the stability region. Sun and Ma ^[20] further detected local stable region of Nash equilibrium point for dynamical behaviors of the system. Using converse Lyapunov theorems, Kebriaei and Rahimi-Kian ^[21] found the equilibrium region that belongs to the basin of attraction. In an n-person game, Ezro ^[22] prove the existence of an equilibrium point. Barthel and Hoffmann ^[23] analyzed the existence and stability of Nash equilibria in N-firm Cournot-Bertrand oligopolies. From all of the above, the equilibrium of price competition is discussed on some aspect of duopoly, triopoly, four firms, five firms and n firms. However, Li and Kendall ^[24] pointed out that exact solutions to the replicator equation are difficult to obtain. This paper aims to obtain general equilibrium solutions for Bertrand model.

For the equilibrium from the spatial agglomeration. Hamilton et al. ^[25] and Anderson and Neven ^[26] showed spatial agglomeration in equilibrium. Hotelling ^[27] revealed that the duopoly firms will cluster in the middle of the line model (Pal, ^[28]), in middle, there's only one equilibrium. However, centrally clustered equilibrium is only a special case (Mayer, ^[29]). D'Aspremont ^[30] revised a little for Hotelling's model, there isn't only a location for the solution of a price equilibrium. Takanori ^[31] extended spatial agglomeration to a model with multiple cities, the result showed firms can agglomerate at a point. Matsushima ^[32] further modified spatial gathering based on a circular city, firms will gather at two points. Moreover, other researchers (Anderson and Neven, ^[26]; Gupta et al., ^[33]; Sarkar et al., ^[34]; Takanori, ^[35]; Benassi, ^[36]; Gao and Du, ^[37]) mainly concentrate on spatial agglomeration of quantity competition. Whether price competitive equilibrium or quantity competitive equilibrium, for two bargainors, the system solution is always dynamically stable (THeocharis, ^[38]); if the number is three, the result makes it clear that we shall get finite oscillations about the equilibrium district; if the number is more than three, the agglomeration is not so robust (Picard and Tabuchi, ^[39]), the competitive state will always be unstable. That is to say, when there are many firms in the identical market, general equilibrium points are difficult to be derived. Yu ^[40] showed whether it is Bertrand competition or Cournot competition, there exist many equilibrium points, the equilibrium place shows spatial gathering by pairs (Yu and Lai, ^[41]), and in equilibrium place, the enterprises can obtain a more higher price, occasionally save a "sale", and the prices are without any regular pattern (Pitchik, ^[42]).

However, to this days, the general equilibrium points of Bertrand game have not been investigated. When the number of competitors is more than four, it is very difficult to derive the value of the equilibrium points. This paper aims to detect the general competitive equilibrium points for Bertrand game, which is studied from spatial agglomeration with mean value theorem.

In this framework, Section 2 discusses a dynamic price game with demand function and utility function. With the parameters changing, the bifurcation of equilibrium points appears and gradually becomes chaotic. Section 3 considered in a congeneric price oligopoly where there are n firms, along with identical constant cost functions. The mean value theorem is employed. A discrete general equilibrium price choice in a homogeneous price oligopoly is explored. Section 4 further considered the number and stability of general equilibrium points of Bertrand. The celestial body motion is introduced. The final section is concluding remarks of the paper.

2.   Model analysis

A Bertrand duopoly game is considered with demand function. Each firm j chooses a real number $p_{j}$, which is the price of product to be priced by her. The payoff of j'th firm is formulated as follows:

where $C_{j}\left(p_{j}, p_{-j}\right)$ is the cost function. The standard notation ${p}_{-j}$ is used to indicate the vector ${p}$ with the component $p_{j}$ omitted. The optimal price of the firms could be calculated by solving the following problem,

For maximizing profits for the firm, this paper puts a partial derivative $\pi_{j}\left(p_{j}, p_{-j}\right)$ with respect to $p_{j}$ equal to zero,

For the discrete dynamical system each firm can determine the corresponding profit-maximizing price with expectation of the rival's price. The relative profit maximization will be discussed in the next section. The utility function of the representative consumer in one market is given by:

The demand functions come from the maximization of the utility function of the representative consumer, are the following:

$q_{1} = a-p_{1}-b p_{2}$, and

$q_{2} = a-p_{2}-b p_{1}$

where $p_{1}$ and $p_{2}$ are the prices, $q_{1}$ and $q_{2}$ are the outputs, respectively. The parameter b is used to measure the degree of products differentiation. As b is one, the products is interacting, and as b is zero, the products is non-interacting. To improve the game of this work, a cost functions is considered:

$C_{i}\left(p_{i}\right) = c_{i} p_{i}+r_{i} p_{i} p_{j} \quad i, j = 1, 2, \quad i \neq j$

$c_{i}$ is the marginal cost.

In a game, there are two players, they produce differentiated goods of kinds 1 and 2. With the previous assumptions, the absolute profits of each firm are seen below respectively:

The difference between the absolute profit is measured by the relative profit. The relative profits of firm 1 is defined by $R_{1}$ and that of firm 2 by $R_{2}$. The relative profits of the two firms are derived by:

Firm 1 chooses her action through maximizing her relative profit with respect to $p_{1}$, firm 2 chooses her action through maximizing her relative profit with respect to $p_{2}$,

$a-c_{1}-2 p_{1}-\left(b_{1}-b_{2}\right) p_{2} = 0$, and

$a-c_{2}-2 p_{2}-\left(b_{2}-b_{1}\right) p_{1} = 0$

where $b_{1} = b+r_{1}$ and $b_{2} = b+r_{2}$.

Two firms adjustment mechanism is considered, that is,

$p_{i}(t+1) = p_{i}(t)+\alpha_{i} p_{i} \Phi_{i}\left(p_{i}\right), \quad i = 1, 2$

where $\Phi_{i}\left(p_{i}\right)$ is marginal relative profit of ith firm. $\alpha_{i}$ is the speed of adjustment mechanism. The discrete dynamical system of prices can be given as:

where c marginal cost of industry. $\alpha_{1}$, $\alpha_{2}$ adjustment coefficient. b coefficient of association.

In order to study the price behavior of the solutions of the nonlinear dynamic system, the equilibrium points are taken as nonnegative fixed points. It is clear that the system (1) has four fixed points in the following form:

Obviously, $E_0$, $E_1$ and $E_2$ are boundary equilibrium points and they are unstable. $E_3$ is a Nash equilibrium point and it is stable. Zhao ^[43] further discussed the instability of the boundary equilibrium point and the stability condition of the internal equilibrium.

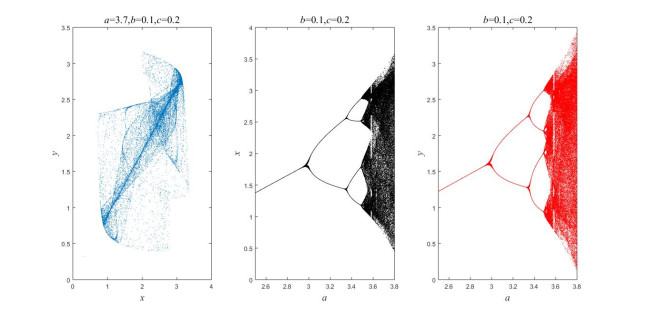
When $\alpha$ = 3.7, b = 0.1 and c = 0.2, the bifurcation appears and gradually becomes chaotic in the system (1). Such as Figure 1.

where x denotes $p_{1}$, y denotes $p_{2}$. The left diagram of Figure 1 shows the equilibrium points of $p_{1}$ and $p_{2}$ are much and chaotic. The middle diagram shows the values of $p_{1}$ become chaotic with $\alpha$. The right diagram shows the values of $p_{2}$ become chaotic with $\alpha$. That is to say, it is very difficult to find optimal equilibrium value. In this paper, we further detect a general equilibrium point for Bertrand model when there are n firms. A differentiation theorem is tried to be introduced to handle this question.

3.   Equilibrium point exploring

Now, we suppose that there are n ($n\geqslant 3$) firms in one market. The cost function is identical or similar for each firm, and without loss of generality, the market demand function is defined:

where $Q_i$ is defined as the quantity, $\alpha$ is constant, $\beta$ is correlation coefficient, $p_i$ is assumed to be the price.

When i = 1, only a firm exists in one market. The scenario is called the monopoly. The profit of a firm can be denoted:

$\pi\left(p \right)$ is defined as the profit of a firm and taken partial derivative:

The optimal price can be obtained from formula (4):

parameter

When i = n, the market belongs to a perfect competition. The price equals the marginal cost $p = c(p)$.

Define 1. ${{p_m}}$ is one of convex points, and presumed one of general equilibrium points of the Bertrand model.

Imperfect competition is between monopoly and perfect competition. A space gathering concept model illuminates imperfectly competitive market. Such as Figure 2. The region is taken as a chaotic area between m and n. The general equilibrium points $P_{1}$, $P_{2}$, $\cdots$, $P_{n}$ move around the field.

The m endpoint is defined as the monopoly market, and the n endpoint represents the perfectly competitive market.

Assumption 1. ${\rm{m}} = \frac{{\alpha + c }}{{2}}$, ${n = c(p)}$. Based on the mean-value theorem, at each point of an open interval $\left({m}, {n}\right)$, there is a point z in (m, n) such that

Then, a general equilibrium point is derived for Bertrand model,

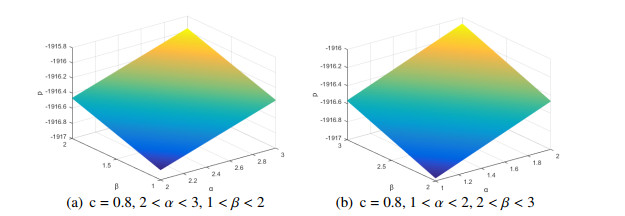
With gradually developing of the market, the oligarch enterprises may aggregate, the economic system will become stable. As Figure 3, the solutions of system form a lower convex surface. With the changing of parameters of $\alpha$ and $\beta$, the surface of solutions don't change. It is clearly that the stable situation of ${p_m}$ is a complicated surface. The convexity is called attractor. The points are defined as the general equilibrium points. It will not become unstable with the increasing number of firms.

From (2) and (7), a general equilibrium production can be derived,

From (3), (7) and (8), the profit of equilibrium is calculated,

If there exists only a firm in one market, a monopoly market emerges, the price and production can be derived,

$\pi _{monopoly}$ is defined as the profit of monopoly,

From above the value of (10)$>$(7), the value of (12)$>$(9). It is clear that the equilibrium situation of the Bertrand can bring about lower price than monopoly. For profit, monopoly structure can generate more profit than any other market structure. The scenario is consistent with reality.

4.   Number and stability of equilibrium points

In one market, many of the similar or homogenous firms try their best to achieve profit, market share and so on. They are aggregated by a market attractive force in one market. On the influence of attraction, the firms can produce more goods. The price of goods fluctuates with the equilibrium price in the market. Many years later, quantity supplied is roughly equal to quantity demanded, the market will gradually become stable. We take the phenomenon of stable market as the motion of the celestial body. Such as the earth, moon and sun, they keep balance with attractive force. Being similar to the celestial bodies, a market remains stable with market attractiveness.

Assumption 2. In a dynamic economics system, there exist two market, K1 and K2. K1 is treated as the monopoly market. K2 is taken as the perfectly competitive market. Imperfect competition locates between K1 and K2.

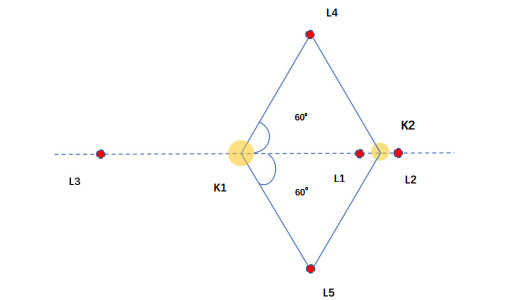
As from Figure 4, based on Lagrangian points and market attractiveness, there exist five general equilibrium points, L1, L2, L3, L4 and L5 respectively. K1, K2, L1, L2 and L3 lie on the same line. The points of L4 and L5 lie in the third corners of the two equilateral triangles and stable. L1, L2 and L3 are unstable points.

Find the solution of L1 in the Eq (13)

The relationship between L1, K1 and K2 defined ${r_1}$. R is indicated as structure coefficient between K1 and K2. ${r_1}$ can be derived from the formula (13),

Find the solution of L2 in the Eq (14):

The relationship between L2, K1 and K2 defined ${r_2}$.

Find the solution of L3 in the Eq (15):

The relationship between L3, K1 and K2 defined ${r_3}$.

The solutions of L4 and L5 are symmetric. It is easy to derive respectively.

Assumption 3. Making K1 = p = $\frac{{\alpha + c }}{{2}}$, and K2 = p = c.

The general equilibrium points of Bertrand model can be derived.

Obviously, the value of $E_3$ belongs to L4. the value of $E_1$ belongs to L1 or L2. It shows that the general equilibrium points for n ($n\geqslant 3$) competitors of the Bertrand model were detected with celestial bodies motion.

5.   Concluding remarks

In this paper, the competitive equilibrium of the Bertrand game is discussed with bounded rationality and the utility function. The chaotic region of the Bertrand model is illustrated. A market structure model of space gathering is established. Further analyzing uniform linear demand of the Bertrand model, we calculate the price of monopoly and that of perfect competition respectively. The general equilibrium points are derived by the mean value theorem. At the same time, the number and stability of equilibrium points are discussed on the basis of the celestial motion theory. The results show that only five general equilibrium points exist in the Bertrand model, three of them are unstable, others are stable. The research generalized the result of the equilibrium points of dynamics system of Bertrand model. Numerical computation and simulation are given to support the consequences.

In addition, the exploration provides additional information of competitive equilibrium for Bertrand (price) competition. When one firm will enter into a relative market, according to the industry marginal cost and demand function, at least a general equilibrium price can be calculated, as the firm may obtain competitive advantage.

Acknowledgments

The authors are grateful for the editor and the anonymous reviewers considering our manuscript. This work is supported in part by Program for Applied Economics Foundation of Yuncheng University (Grant number XK-2020015) and Program for Doctor scientific research of Yuncheng University (Grant number YQ-2021016).

Conflict of interest

The authors declare that there is no conflicts of interest in this paper.