Motivated by cost control and information guidance, in this work, we study a multiple vacations retrial queue with N-policy and breakdowns. This service system has the characteristics that there is no waiting space in front of the server and the waiting list is virtual. If the arriving customer finds that the system is available, he immediately receives the complete service. Otherwise, the customer leaves the system or joins the orbit (virtual waiting list). For cost control, the system is activated only when the current vacation is completed and at least N customers are waiting in the system, otherwise, the server continues to the next vacation until the number of customers in the system is not less than N. Two types of customer joining cases apply to this paper, i.e., non-cooperative customers aim to optimize individual interests, and the social planner in the cooperative case considers the profit of the whole service system. The equilibrium joining strategy for the non-cooperative case and the socially optimal joining strategy for the cooperative case are determined. Since it is difficult to obtain analytical characterization, an improved particle swarm optimization (PSO) algorithm is used to explore the impact of system parameters on the profit of the service provider. At the same time, a large number of numerical experiments visualize the influence of parameters on the system.
Citation: Zhen Wang, Liwei Liu, Yuanfu Shao, Yiqiang Q. Zhao. Joining strategies under two kinds of games for a multiple vacations retrial queue with N-policy and breakdowns[J]. AIMS Mathematics, 2021, 6(8): 9075-9099. doi: 10.3934/math.2021527
| [1] | S. Sundarapandiyan, S. Nandhini . Sensitivity analysis of a non-Markovian feedback retrial queue, reneging, delayed repair with working vacation subject to server breakdown. AIMS Mathematics, 2024, 9(8): 21025-21052. doi: 10.3934/math.20241022 |
| [2] | Bharathy Shanmugam, Mookkaiyah Chandran Saravanarajan . Unreliable retrial queueing system with working vacation. AIMS Mathematics, 2023, 8(10): 24196-24224. doi: 10.3934/math.20231234 |
| [3] | K. Jeganathan, S. Selvakumar, N. Anbazhagan, S. Amutha, Porpattama Hammachukiattikul . Stochastic modeling on M/M/1/N inventory system with queue-dependent service rate and retrial facility. AIMS Mathematics, 2021, 6(7): 7386-7420. doi: 10.3934/math.2021433 |
| [4] | Rani Rajendiran, Indhira Kandaiyan . Transient scrutiny of $ M^X/G(a, b)/1 $ queueing system with feedback, balking and two phase of service subject to server failure under Bernoulli vacation. AIMS Mathematics, 2023, 8(3): 5391-5412. doi: 10.3934/math.2023271 |
| [5] | Jahfar T K, Chithra A V . Central vertex join and central edge join of two graphs. AIMS Mathematics, 2020, 5(6): 7214-7233. doi: 10.3934/math.2020461 |
| [6] | YanLing Li, GenQi Xu, Hao Chen . Analysis of two components parallel repairable degenerate system with vacation. AIMS Mathematics, 2021, 6(10): 10602-10619. doi: 10.3934/math.2021616 |
| [7] | Shaojun Lan, Yinghui Tang . An unreliable discrete-time retrial queue with probabilistic preemptive priority, balking customers and replacements of repair times. AIMS Mathematics, 2020, 5(5): 4322-4344. doi: 10.3934/math.2020276 |
| [8] | Yuejiao Wang, Chenguang Cai . Equilibrium strategies of customers and optimal inventory levels in a make-to-stock retrial queueing system. AIMS Mathematics, 2024, 9(5): 12211-12224. doi: 10.3934/math.2024596 |
| [9] | Muhammad Zeeshan Hanif, Naveed Yaqoob, Muhammad Riaz, Muhammad Aslam . Linear Diophantine fuzzy graphs with new decision-making approach. AIMS Mathematics, 2022, 7(8): 14532-14556. doi: 10.3934/math.2022801 |
| [10] | Sara Pouyandeh, Amirhossein Morovati Moez, Ali Zeydi Abdian . The spectral determinations of connected multicone graphs Kw ▽ mCP(n). AIMS Mathematics, 2019, 4(5): 1348-1356. doi: 10.3934/math.2019.5.1348 |
Motivated by cost control and information guidance, in this work, we study a multiple vacations retrial queue with N-policy and breakdowns. This service system has the characteristics that there is no waiting space in front of the server and the waiting list is virtual. If the arriving customer finds that the system is available, he immediately receives the complete service. Otherwise, the customer leaves the system or joins the orbit (virtual waiting list). For cost control, the system is activated only when the current vacation is completed and at least N customers are waiting in the system, otherwise, the server continues to the next vacation until the number of customers in the system is not less than N. Two types of customer joining cases apply to this paper, i.e., non-cooperative customers aim to optimize individual interests, and the social planner in the cooperative case considers the profit of the whole service system. The equilibrium joining strategy for the non-cooperative case and the socially optimal joining strategy for the cooperative case are determined. Since it is difficult to obtain analytical characterization, an improved particle swarm optimization (PSO) algorithm is used to explore the impact of system parameters on the profit of the service provider. At the same time, a large number of numerical experiments visualize the influence of parameters on the system.
In many service systems, behavioral analysis of the arriving customers' decision whether to join an unavailable system (where service seats are occupied) or leaving forever has become a hot topic in economics in recent years. More specifically, the introduction of customers' strategic behavior in the queuing system makes the analysis of performance indicators more meaningful. Although it is not very difficult to deal with the performance analysis of various M/M/1 systems, it is still difficult to conduct game theory analysis in specific such systems. In fact, the analysis of customer game behavior requires some characteristics of the system, so it is usually very difficult for the Markov process. Furthermore, considering the customer's strategic behavior in the service system can make the customer's subjective behavior theoretical and logical. The joining strategy of customers was first studied by Naor [1]. He studied the M/M/1 system under the observable case, in which the individual's equilibrium joining strategy and social optimal joining strategy were confirmed. Subsequently, the unobservable case of Nash's work was supplemented by Edelson and Hildebrand [2]. In recent years, the research of customer joining strategy in queuing systems has been widely expanded and applied. Such as Shi and Lian [3] presented the joining strategy and socially optimal welfare of passenger-taxi in both observable and unobservable cases, catastrophe or clearing systems with equilibrium [4,5], and queueing systems with setup or vacation [6,7,8]. More specifically, the monograph of Hassin and Haviv [9] summarized a large number of related studies on joining strategies and optimal social welfare.
In many queuing service systems, their default rule is that the customers leave the system once they balk and cannot join the system again. Obviously, this rule is often not suitable for the actual service system. For example, when the customer calls the call center for the first time and encounters a busy line, the customer will try again after a random length of time. The retrial queuing system widely exists in other fields such as communication, information processing, storage management, and epidemic situation control. Kosten [10] illustrated the necessity of retrial queuing through practical applications. Jiang [11] applied a tail asymptotics approach to a matching service voting system. Phung-Duc [12] presented an M/G/1 single server retrial queue with setup time. However, there are still a few works of literature on the joining strategy and social welfare of various retrial queue systems. Economou and Kanta [13] studied joining strategies and social welfare in the M/M/1 queue with retrial. Wang and Li [14] proposed a class of cognitive radio networks with retrial behavior. Wang and Zhang [15] studied price strategy and equilibrium of a local area networking retrial queue with delayed vacations.
The conservation and effective use of resources and energy is the key to sustainable economic growth. Barroso and Holzle [16] pointed out that the proportion of energy consumed by standby equipment is also very high, it can reach 60 percent of the normal operation equipment. In the queuing service system, it is necessary to take some measures to control operating costs and labor costs, which can effectively promote the growth of social welfare. For example, threshold strategy, multiple vacations, and adjusted maintenance times are often applied to queuing systems with detailed services. Wang et al. [17] studied a batch service queuing system with finite capacity and gated policy. Guo and Hassin [18] discussed joining strategy and social optimization of a single server queueing system with N-policy. Wang et al. [19] studied customers' strategic behavior and social optimization in a constant retrial queue with the N -policy. Sun et al. [20] presented the strategies of joining and optimal balking in a Markovian queue with multiple vacations. Ye [21] presented a discrete-time Geom/Geom/1 queue with single working vacation and multiple vacations. Gao [22] considered an unreliable queuing system that adjusts maintenance time and limits idle period. Unlike the previously mentioned literature, the retrial queuing system studied in this paper takes into account the N-policy and multiple vacations. More specifically, this system has the following features: The system is activated only when the current vacation is completed and at least N customers are waiting in the system, otherwise, the server continues to the next vacation until the number of customers in the system is not less than N. Generally, in actual service systems, the server cannot immediately check the system status during the vacation state; or when the number of customers reaches the desired threshold, the server cannot immediately switch to the working state; or when the server ends the vacation, there are no (or few) customers who reach the system. At the same time, it is necessary to consider the operating cycle (lifetime) of the server for the overall benefit of the system. Therefore, the multiple vacations retrial queue with N-policy and breakdowns is considered in this paper; it has a wide range of applications in actual service systems.
There are the cooperative case and the non-cooperative case for the customers to join the system with each other. In the non-cooperative case, the goal of each individual is to obtain their own maximum expected net benefit based on the reward-cost structure. Obviously, if the server is unavailable upon arrival, the customers need to decide whether to join the system or leave forever. If the customer's expected net benefit is positive, then the customer will not hesitate to join the system, otherwise, the customer chooses to balk. If the expected net benefit equals zero, the customer is indifferent to join the orbit or balk. In the cooperative case, the social planner wants the customers to cooperate with each other in order to get the best social welfare for the whole system. Some existing research results ([7,13,19]) indicate that the optimal social joining probability does not exceed the individual's equilibrium joining probability, or the optimal social arrival rate does not exceed the individual's equilibrium arrival rate, i.e., the customers find the server unavailable upon their arrival, the customers in the non-cooperative case are more willing to join the system than the customers in the cooperative case. The result is that social benefits do not reach the expected maximum by the social planner in the non-cooperative case.
The proposed model has a potential application in an order-based production system. In order to avoid equipment wear and tear and unprofitable production, the factory's production lines will only be activated after signing the order quantity sufficient to cover all expenses. A production line is usually in four periods: Vacation, busy, idle, and repair. The vacation period is usually used to accumulate enough orders to cover all expenses, so the production line will not be activated when the order quantity does not accumulate to a certain amount. The idle period is used to prepare production materials for signing orders. The busy period is used to produce orders signed. In the process of production order, equipment may break down due to parts wear or service life. If the equipment breakdown occurs, the equipment is immediately sent for repair, the order which has interrupted service due to breakdown, continues to receive the remaining service after the equipment is repaired. If an idle production line's surplus material can make a new arrival order, the production line can directly complete the order. New orders that arrive during the busy period and the repair period can be joined the waiting list or abandon the factory contract. The new order is independent of other orders and is completed on the first-come, first-served (FCFS) discipline. In this scenario, orders, waiting list, the new order is independent of other orders and is completed on the FCFS principle, equipment breakdown, equipment repair time, the production line of the factory, and order quantity sufficient to cover all expenses corresponding to the customers, the orbit, retrial policy, server breakdown, repair time, the server, and N-policy, respectively, in the queueing terminology. The characteristics and contributions of this model considered in this paper are as follows:
● This article considers a multiple vacations retrial queue with N-policy and breakdowns, it coincides with the actual service system.
● Two types of customer joining cases apply to this paper, i.e., non-cooperative customers aim to optimize individual interests, and the social planner in the cooperative case considers the profit of the whole service system.
● The equilibrium joining strategy for the non-cooperative case and the socially optimal joining strategy for the cooperative case are presented in this paper.
● The improved PSO algorithm is used to visualize the impact of system parameters on the profit of service providers.
● Both the model itself and the numerical experiment have certain guiding significance for the actual service system, this model is suitable for emergency disaster or epidemic control.
The rest of this paper is organized as follows. Section 2 gives a detailed description and parameter representation of this model. The steady-state distribution of the system and the mean orbit sizes are determined in Section 3. Section 4 obtains equilibrium joining strategies in the non-cooperative case and optimal joining strategies in the cooperative case. Section 5 focuses on the profit of the service provider and gives a simple description of PSO algorithms. Section 6 uses a large number of numerical experiments to show the influence of parameters on the two joining probabilities, social welfare, and the profit of the service provider. Section 7 presents the discussion and further study.
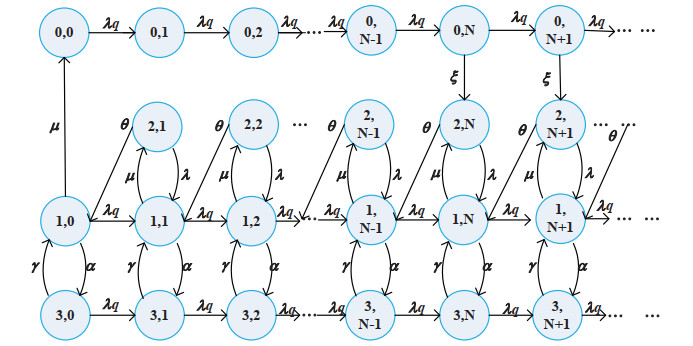
In this paper we consider a constant retrial queuing system with multiple vacations, N-policy, and breakdowns. Assumes the arrival of potential customers according to the Poisson process with rate λ. There is no waiting area in front of the server. Upon arrival, if the server is available (idle state), the arriving customer will immediately receive the service and leaves the system upon the completion of the service. Otherwise, if the server is unavailable (vacation state or busy state or repair state), the arriving customer would enter an infinite capacity orbit with probability q or leave the system forever with probability ¯q=1−q, this virtual orbit is similar to a waiting list. The first customer in the orbit asks for service according to the first-come, first-served (FCFS) discipline with a Poisson flow of retrials of rate θ. The idle server will take an exponential time of rate θ to access (or search) the list customer to provide the service. However, if a new customer arrives during the search process, the server will abandon the search and immediately serves this new customer. The server may breakdown due to parts wear or service life during the service of customers. The life time of the server is exponentially distributed with rate α. If the server breakdown occurs, the server is immediately sent for repair, and the repair time follows an exponential distribution with rate γ. The customer who have interrupted service due to breakdown, continue to receive remaining service after the server is repaired. The service time for all (new or repeated) customers is exponentially distributed with the common rate μ, and all service times are independent.
On the other hand, the server in vacation state does not provide any services to arriving customers, and will not be activated until there are no less than N customers in the orbit after completing the current vacation. If the server finds less than N customers in the orbit at the end of the current vacation, the server will start another new vacation until no less than N customers in the orbit. Once all customers are served, or the system becomes empty, the server starts a vacation of random length V, which is assumed to be exponentially distributed with rate ξ. So we can define this type of queue system as M/M/1/MV constant retrial queueing system with N-policy and breakdowns. Once the server is activated, all customers in the system receive the complete service, and the server turns to vacation state after serving all customers.
Let S(t) be the state of the server at time t,
| S(t)={0,vacation;1,busy;2,idle,3,repair, |
and let N(t) denotes the number of customers in the orbit at time t. Obviously, the stochastic process {(S(t),N(t)),t≥0} is a continuous-time Markov chain with state space Ω={(0,i),i≥0;(1,j),j≥0,(2,k),k≥1;(3,h),h≥0}. The corresponding transition rate diagram of the Markov chain is shown in Figure 1.
Remark 1. It is worthwhile to mention that if q=0, then the model degenerates to a system with a single state (0,0). In this case, all customers will balk. Also, according to the above description, N should be at least equal to 1. Otherwise, the system will be the degenerated one too.
We consider every customer has a reward-cost structure. When a customer arrives, the customer can decide whether to join the system according to the reward-cost structure. It is assumed that the customers are the same except that the arrival time is unexpected. With this structure, every customer received a reward of R units upon the completion of the service. However, a waiting cost of C per unit time is charged for the waiting in the orbit for each customer. In addition, the server imposes a price p, announced to all incoming customers, for each customer to enter the system. Assume that customers are risk neutral and want to maximize their expected benefits. Upon arrival, the customer has to weigh the difference between gain and expenditures (waiting cost and announced price), the customers can evaluate the value and decide whether to enter the system or not. Obviously, the customer is more willing to join the system when all the expenses are less than the reward, while all the expenses are more than the reward, the customer does not enter the system. On the other hand, the customers are indifferent if the reward is equal to all expenses. In addition, in a sense, the customer's decision is immutable, which means that customers who join the system cannot exit, and customers who balked can not retry. So, this system can be regarded as a game between the customers. The server pre-announces the price of p in order to know how it affects the customer's behavior. For our analysis to be meaningful, we assume that
| R>p, | (2.1) |
which ensures that the customers alway join the system if they find that the server is idle upon their arrivals. We can refer to this literature [13] to get the necessary and sufficient stability condition of this system as follows
| λq(λ+θ)(α+γ)θμγ<1, | (2.2) |
which is assumed throughout the paper.
To study the joining strategies of two kindle of games, we first need to study the performance of the system. If the arriving customer chooses to join the system with probability q when finding the server is unavailable.
Let {p(0,i),i≥0;p(1,j),j≥0;p(2,k),k≥1;p(3,h),h≥0} be the steady-state probability distribution of the Markov chain {(S(t),N(t)),t≥0}, and let Pi(z), i=0,1,2,3 be the partial generating functions, defined by
| P0(z)=∞∑n=0znp(0,n),P1(z)=∞∑n=0znp(1,n),P2(z)=∞∑n=1znp(2,n),P3(z)=∞∑n=0znp(3,n),|z|≤1. |
Then, we have the following theorem.
Theorem 1. For the M/M/1/MV constant retrial queueing system with N-policy and breakdowns in the steady state, the probabilities that the server is vacation, busy, idle and repair are given by, respectively,
| P0(1)=λq+Nξξp(0,0), | (3.1) |
| P1(1)=λqγ(λ+θ)(λq+Nξ)ξ(θμγ−λq(λ+θ)(α+γ))p(0,0), | (3.2) |
| P2(1)=λqμγ(λq+Nξ)ξ(θμγ−λq(λ+θ)(α+γ))p(0,0), | (3.3) |
| P3(1)=λqα(λ+θ)(λq+Nξ)ξ(θμγ−λq(λ+θ)(α+γ))p(0,0), | (3.4) |
where p(0,0) can be obtained by the normalization condition P0(1)+P1(1)+P2(1)+P3(1)=1, given by,
| p(0,0)=ξ(θμγ−λq(λ+θ)(α+γ))μγ(λq+θ)(λq+Nξ). | (3.5) |
Proof From Figure 1, the balance equations of steady-state distribution are given as follows,
| λqp(0,0)=μp(1,0), | (3.6) |
| λqp(0,n)=λqp(0,n−1),1≤n≤N−1, | (3.7) |
| (λq+ξ)p(0,n)=λqp(0,n−1),n≥N, | (3.8) |
| (λq+μ+α)p(1,0)=θp(2,1)+γp(3,0), | (3.9) |
| (λq+μ+α)p(1,n)=λqp(1,n−1)+λp(2,n)+θp(2,n+1)+γp(3,n),n≥1, | (3.10) |
| (λ+θ)p(2,n)=μp(1,n),1≤n≤N−1, | (3.11) |
| (λ+θ)p(2,n)=ξp(0,n)+μp(1,n),n≥N | (3.12) |
| (λq+γ)p(3,0)=αp(1,0), | (3.13) |
| (λq+γ)p(3,n)=αp(1,n)+λqp(3,n−1),n≥1. | (3.14) |
From (3.7), we can get
| p(0,N−1)=⋯=p(0,1)=p(0,0), | (3.15) |
which leads to
| N−1∑n=0znp(0,n)=N−1∑n=0znp(0,0)=1−zN1−zp(0,0). | (3.16) |
By (3.8) and (3.12), we get
| p(0,n)=p(0,N−1)(λqλq+ξ)n−N+1=p(0,0)(λqλq+ξ)n−N+1,n≥N. | (3.17) |
So,
| ∞∑n=Nznp(0,n)=∞∑n=Nznp(0,0)(λqλq+ξ)n−N+1=λqzNλq(1−z)+ξp(0,0). | (3.18) |
Therefore, P0(z) can be obtained as follows,
| P0(z)=∞∑n=0znp(0,n)=N−1∑n=0znp(0,n)+∞∑n=Nznp(0,n)=λq(1−z)+ξ(1−zN)(1−z)(λq(1−z)+ξ)p(0,0). | (3.19) |
Then we consider the partial generating function P1(z). Multiplying equations (3.9)–(3.14) by zn and summing up over n, we have that
| (λq+μ+α−λqz)P1(z)=(λ+θz)P2(z)+γP3(z), | (3.20) |
| (λ+θ)P2(z)=μP1(z)−μp(1,0)+ξ∞∑n=Nznp(0,n), | (3.21) |
| (λq+γ−λqz)P3(z)=αP1(z). | (3.22) |
From (3.22), we have
| P3(z)=αλq(1−z)+γP1(z). | (3.23) |
Substituting (3.23) into (3.20) yields
| P2(z)=λ2q2(1−z)2+λq(1−z)(α+μ+γ)+μγ(λq(1−z)+γ)(λ+θz)P1(z). | (3.24) |
Substituting (3.6) and (3.18) into (3.21) yields
| (λ+θ)P2(z)=μP1(z)−λqp(0,0)+λqξzNλq(1−z)+ξp(0,0). | (3.25) |
So, substituting (3.24) into (3.25) yields P1(z) as follows
| P1(z)=λq(ξzN−λq(1−z)−ξ)(λq(1−z)+γ)(λ+θz)(λq(1−z)+ξ)⋅Ap(0,0), | (3.26) |
where
| A=(λ+θ)(λ2q2(1−z)2+λq(1−z)(α+μ+γ)+μγ)−μ(λq(1−z)+γ)(λ+θz). | (3.27) |
Substituting (3.26) into (3.24) yields P2(z) as follows
| P2(z)=λq(ξzN−λq(1−z)−ξ)(λ2q2(1−z)2+λq(1−z)(α+μ+γ)+μγ)(λq(1−z)+ξ)⋅Ap(0,0). | (3.28) |
Substituting (3.26) into (3.23) yields P3(z) as follows
| P3(z)=λqα(ξzN−λq(1−z)−ξ)(λ+θz)(λq(1−z)+ξ)⋅Ap(0,0). | (3.29) |
Let z=1 in (3.19), (3.26), (3.28) and (3.29), we can get the results (3.1)–(3.4), where p(0,0) can be obtained by the normalization condition P0(1)+P1(1)+P2(1)+P3(1)=1, that is (3.5).
Let Mi be the mean orbit sizes when the server in state i (i=0,1,2,3). From (3.19), (3.26), (3.28) and (3.29), by using Mi=ddzPi(z)|z=1, and after some algebraic manipulations, we have the following Theorem 2.
Theorem 2. For the M/M/1/MV constant retrial queueing system with N-policy and breakdowns in the steady state, the mean orbit sizes when the server is on vacation, busy, idle and repair, respectively, are given by
| M0=(N(N−1)2+λq(λq+Nξ)ξ2)p(0,0), | (3.30) |
| M1=λqp(0,0)(N(N−1)γ(λ+θ)2(θμγ−λq(λ+θ)(α+γ))+γ(λq(λ+θ)+λξ)(λq+Nξ)(θμγ−λq(λ+θ)(α+γ))ξ2+λq(γ(α+γ)+λqα)(λ+θ)2(λq+Nξ)(θμγ−λq(λ+θ)(α+γ))2ξ), | (3.31) |
| M2=λqp(0,0)(μγN(N−1)2(θμγ−λq(λ+θ)(α+γ))+μγ(λq+ξ)(λq+Nξ)(θμγ−λq(λ+θ)(α+γ))ξ2+λq(λq+Nξ)(λq(λ+θ)(αμ+(α+γ)2)+λμγ(α+γ))(θμγ−λq(λ+θ)(α+γ))2ξ), | (3.32) |
| M3=λqαp(0,0)(N(N−1)(λ+θ)2(θμγ−λq(λ+θ)(α+γ))+(λq(λ+θ)+λξ)(λq+Nξ)(θμγ−λq(λ+θ)(α+γ))ξ2+λqθμ(λ+θ)(λq+Nξ)(θμγ−λq(λ+θ)(α+γ))2ξ+λq(α+γ−λq)(λ+θ)2(λq+Nξ)(θμγ−λq(λ+θ)(α+γ))2ξ), | (3.33) |
where p(0,0) is given in (3.5).
If the server is unavailable upon arrival, each customer needs to decide whether to join the system based on the reward-cost structure. If the arriving customer is against the will of the social planner, maximizes the individual's expected net benefit, rather than maximizing the overall benefit from the perspective of the social planner, then the customers are in the non-cooperative case. However, the social planner often wants to encourage customers to cooperate with each other to maximize the social welfare in cooperative case. In this section, we consider the joining strategies of the customers in two kinds of game cases, respectively.
In the non-cooperative case, the arriving customer is based on a reward-cost structure with the goal of maximizing the individual benefit, when an arriving customer finds that the server is unavailable, he needs to decide whether to enter the system or not. Actually, if the expected net benefit is greater than zero, the customers are more willing to join the orbit; the customers prefer to balk if expected net benefit is less than zero; if the customers' expected net benefit equals zero, they are indifferent to join the orbit or balk. Each customer's different decisions have different impacts on other customers, and it also affects the performance measures of the system. Next, our goal is to explore the symmetric equilibrium joining strategy of the non-cooperative game between these customers.
The expected waiting time of a tagged customer who finds the server is unavailable and decides to join the orbit upon his arrival, denoted by T(q). Then we can give the following Theorem 3 about T(q).
Theorem 3. For the M/M/1/MV constant retrial queueing system with N-policy and breakdowns in the steady state, the expected waiting time of a tagged customer in the orbit, given that he finds the server is unavailable and decide to join orbit upon his arrival, T(q) is given by
| T(q)=ξN(N−1)(λq+θ)2λqθ(λq+Nξ)+μγ(λq+θ+ξ)+λξ(α+γ)μγθξ+Φ(q)θμγ(θμγ−λq(λ+θ)(α+γ)), | (4.1) |
where
| Φ(q)=λ2q2(λ+θ)(αμ+(α+γ)2)+λq(α+γ)2(λ+θ)2+λqμ(λγ(α+γ)+αθ(λ+θ)). | (4.2) |
Proof According to the model description, it is only available when the server is idle upon customers arrivals. Therefore, according to the Poisson arrivals see time average (PASTA) property and Theorem 1, the server unavailable probability Pun upon arrival of a customer is given by
| Pun=P0(1)+P1(1)+P3(1)=θ(λq+θ). | (4.3) |
Pun is the probability of the server unavailable upon arrival of a customer. So, the total arrival rate of the customers in the orbit can be determined as follows
| λun=λqPun=λqθ(λq+θ). | (4.4) |
From Theorem 2, the customers' mean number in the orbit, denoted by M, then
| M=M0+M1+M2+M3. | (4.5) |
By using Little' formula, we can get
| T(q)=Mλun. | (4.6) |
Hence, substituting (4.4) and (4.5) into (4.6), (4.1) can be obtained.
Now, we obtain the expected waiting time T(q) of a tagged customer who finds the server is unavailable and decides to join orbit upon his arrival. Based on the reward-cost structure previously mentioned, the expected net benefit of a tagged customer, who finds that the server is unavailable upon arrival and decides to join the orbit, denoted by Se(q), it can be obtained as follows
| Se(q)=R−p−CT(q), | (4.7) |
where T(q) is given in (4.1), and the second-order derivative of T(q) in q, given by,
| T″(q)=N(N−1)ξ(λ2q2(λq+3θ)+3Nλqθξ+N2θξ2)θλq3(λq+Nξ)3+2λ2(λ+θ)((λ+θ)2(α+γ)3+(λ+θ)(α+γ)(μγ(α+γ)+θμγ)+θμ2αγ)(θμγ−λq(λ+θ)(α+γ))3. | (4.8) |
Obviously, T″(q) is positive under the condition that the system is stable. So T(q) is strictly convex in the interval 0≤q<θμγλ(λ+θ)(α+γ). Therefore, there is a unique global minimal point qmin∈[0,θμγλ(λ+θ)(α+γ)), which makes T(q) achieve a global minimum in this interval. It follows from (4.7) that Se(q) has a unique global maximal value in this range of q (q∈[0,θμγλ(λ+θ)(α+γ)).
Remark 2. Because T(q) is strictly convex under the stable condition, i.e., q∈[0,θμγλ(λ+θ)(α+γ)), and q should satisfy 0≤q≤1. Defined by the set Q as follows,
| Q={Q1=[0,1],ifθμγλ(λ+θ)(α+γ)>1;Q2=[0,θμγλ(λ+θ)(α+γ)),ifθμγλ(λ+θ)(α+γ)≤1. | (4.9) |
So in the following theorems, we will only study the range of q∈Q.
We are ready to describe the equilibrium joining strategies for the non-cooperative case.
Theorem 4. For the M/M/1/MV constant retrial queueing system with N-policy and breakdowns in the steady state, for any given price p<R, there exists an Nash equilibrium mixed joining strategy, i.e., the arriving customers joining the orbit of the unavailable system with probability qe, where qe have the following cases:
Case I: R−pC<T(qmin). In this case, a unique equilibrium strategy qe exists: qe=0.
Case II: R−pC=T(qmin). In this case, the equilibrium strategy is given by
Case II (a): q∈Q1 and qmin>1. Then, a unique equilibrium strategy qe exists: qe=0.
Case II (b): q∈Q1 and qmin≤1. Then, two equilibrium strategies qe exist: qe=0 and qe=qmin.
Case II (c): q∈Q2. Then, two equilibrium strategies qe exist: qe=0 and qe=qmin.
Case III: R−pC>T(qmin). Let q1<q2 be the roots of Se(q)=0 (there are two roots because of the strict concavity of Se(q) in q∈Q2).
Case III (a): q∈Q1 and q1>1. Then, a unique equilibrium strategy qe exists: qe=0.
Case III (b): q∈Q1 and q1=1. Then, two equilibrium strategies qe exist: qe=0 and qe=1.
Case III (c): q∈Q1 and q1<1. Then, three equilibrium strategies qe exist: qe=0, qe=q1 and qe= min (q2,1).
Case III (d): q∈Q2. Then, three equilibrium strategies qe exist: qe=0, qe=q1 and qe=q2.
Proof According to the model assumption, the arriving customer encounters the idle state, who accepts the service directly, because the customer who arrives at the idle state does not generate waiting time. However, when a customer arrives and finds that the system is not available (vacation or busy or repair), he needs to decide whether to join the system or not. We have proved the strict concavity of Se(q) of q∈[0,θμγλ(λ+θ)(α+γ)) in the previously, and let qmin (qmin∈[0,θμγλ(λ+θ)(α+γ))) be the unique point that globally minimizes T(q) in q∈[0,θμγλ(λ+θ)(α+γ)), i.e., qmin be the unique point that globally maximizes Se(q) in q∈[0,θμγλ(λ+θ)(α+γ)). Obviously, the strategy qe=0 of balking is always an equilibrium strategy. The reason is that all customers balk, a tagged customer also prefers to balk; otherwise this tagged customer will never get service. In fact, if q=0, the model degenerates to a system with a single state of (0,0), then the system will never be activated. Specifically, we have the following results:
(I) When R−pC<T(qmin), i.e., Se(qmin)<0, which implies Se(q) is negative for every q∈Q. In this case, the best response for the tagged customer is balking, i.e., qe=0. Thus we have the Case I.
(II) When R−pC=T(qmin), i.e., Se(qmin)=0, which implies Se(q) is negative for every q≠qmin. There are three cases, (a): if q∈Q1 and qmin>1, which implies qmin∉Q, the equilibrium strategy is qe=0 of balking because of Se(q)<0 for every q in this subcase. (b): if q∈Q1 and qmin≤1, qmin is the unique solution of the equation Se(q)=0 in this subcase, i.e., qmin is a proper mixed strategy q∈Q. (c): if q∈Q2, according to the previous assumption, qmin must belong to the interval Q2, so qmin is the unique solution of the equation Se(q)=0 in this subcase, i.e., qmin is a proper mixed strategy q∈Q. Thus we have the Case II.
(III) When R−pC>T(qmin), i.e., Se(qmin)>0. We notice that the two limits lim, {\lim _{q \to {{(\theta \mu \gamma /\lambda (\lambda + \theta)(\alpha + \gamma))}^ - }}}{S_e}(q) = - \infty and S_{e}(q) is strict concavity in q\in [0, \frac{{\theta\mu \gamma}}{\lambda(\lambda+\theta)(\alpha + \gamma)}) , so the equation S_{e}(q) = 0 must have two roots q_{1} , q_{2} ( q_{1} < q_{2} ) \in [0, \frac{{\theta\mu \gamma}}{\lambda(\lambda+\theta)(\alpha + \gamma)}) . According to the definition of set Q , there are four cases. (a): if q\in Q_{1} and q_{1} > 1 , which implies that the equation S_{e}(q) = 0 has no solution in the interval Q_{1} , so the equilibrium strategy is q_e = 0 of balking. (b): if q\in Q_{1} and q_{1} = 1 , q = 1 is the unique solution of the equation S_{e}(q) = 0 , so q_e = 1 is the equilibrium strategy in this subcase. (c): if q\in Q_{1} and q_{1} < 1 , similar to the discussion of Case III (b), q_{1} is a mixed equilibrium strategy in this subcase because of S_{e}(q_{1}) = 0 . Obviously, if q_{2} < 1 , q_{e} = q_{2} is also a mixed equilibrium strategy in this case; if q_{2} \geq 1 , q_{2} is not the solution of the equation S_{e}(q) = 0 , but q = 1 is a equilibrium strategy in this subcase because of S_{e}(1) > 0 . (d): if q\in Q_{2} , according to the previous description, q_{1} and q_{2} must belong to Q_{2} , so q_{1} and q_{2} are mixed equilibrium strategies because of S_{e}(q_{1}) = 0 and S_{e}(q_{2}) = 0 . Thus we have the Case III.
The customers in the non-cooperative case gain the individual's best benefit by selfish means. However, the joining strategy of the non-cooperative case does not match the wishes of social planners. The intention of the social planner is to promote mutual cooperation between customers in order to maximize the social benefits, i.e., the cooperative case. In this subsection, we turn our interest to the analysis of the socially optimal joining strategy in the cooperative case, in which, the social planner maximizes the social welfare through adopting the socially optimal joining probability q^{*} .
For a given pricing p and joining probability q , the customers join the orbit with probability q when they find the server unavailable upon their arrivals. Social welfare (social net benefit) is the sum of the welfare of customers and server, the benefit of the customers per unit time, denoted by C(q) , it is as follows
| \begin{equation} C(q) = \lambda q({P_0}(1) + {P_1}(1) + {P_3}(1))(R - p - CT(q)) + \lambda {P_2}(1)(R - p), \end{equation} | (4.10) |
and the benefit of the server per unit time, denoted by S(q) , it is as follows
| \begin{equation} S(q) = (\lambda q({P_0}(1) + {P_1}(1) + {P_3}(1)) + \lambda {P_2}(1))p. \end{equation} | (4.11) |
So, social welfare is the sum of the welfare for all customers and also the server, denoted by SW(q) , it is as follows
| \begin{align} SW(q) & = C(q) + S(q) \\ & = (\lambda q({P_0}(1) + {P_1}(1) + {P_3}(1)) + \lambda {P_2}(1))R - C\lambda q({P_0}(1) + {P_1}(1) + {P_3}(1))T(q)\\ & = {\lambda ^*}R - C{\lambda _{un}}T(q), \end{align} | (4.12) |
where
| \begin{equation} {\lambda ^*} = \lambda q({P_0}(1) + {P_1}(1) + {P_3}(1)) + \lambda {P_2}(1) \end{equation} | (4.13) |
is the total arrival rate of the system, and
| \begin{equation} \lambda_{un} = \lambda q({P_0}(1) + {P_1}(1)+ {P_3}(1)) = \frac{\lambda q \theta}{\lambda q + \theta} \end{equation} | (4.14) |
is the total arrival rate to the system when the server is unavailable. By using (4.1), (4.13) and (4.14), we can obtain that
| \begin{align} SW(q) & = \frac{{\lambda q(\lambda + \theta )}}{{\lambda q + \theta }}R - C\left( {\frac{{\xi N(N - 1)}}{{2(\lambda q + N\xi )}} + } \right.\frac{{\lambda q\theta \mu \gamma (\lambda q + \theta + \xi ) + \lambda q\theta \lambda \xi (\alpha + \gamma )}}{{\theta \mu \gamma \xi (\lambda q + \theta )}} \\ & + \left. {\frac{{\lambda q\theta \Phi (q)}}{{\theta \mu \gamma (\lambda q + \theta )(\theta \mu \gamma - \lambda q(\lambda + \theta )(\alpha + \gamma ))}}} \right), \end{align} | (4.15) |
where \Phi (q) is given in (4.2). Further, the second-order derivative of SW(q) in q as follows,
| \begin{equation} SW''(q) = - \frac{{2{\lambda ^2}\theta ((\lambda + \theta )R - C)}}{{{{(\lambda q + \theta )}^3}}} - C\left( {\frac{{{\lambda ^2}\xi N(N - 1)}}{{{{(\lambda q + N\xi )}^3}}} + \frac{{2{\lambda ^2}\theta \mu \gamma (\lambda + \theta )((\lambda + \theta ){{(\alpha + \gamma )}^2} + \theta \mu \alpha )}}{{{{(\theta \mu \gamma - \lambda q(\lambda + \theta )(\alpha + \gamma ))}^3}}}} \right). \end{equation} | (4.16) |
Obviously, under the stability condition 0 \leq q < \frac{{\theta\mu\gamma}}{\lambda(\lambda+\theta)(\alpha+\gamma)} (it is also set Q_{2} ), if (\lambda + \theta)R - C > 0 , i.e., \frac{R}{C} > \frac{1}{{\lambda + \theta }} , then SW''(q) is negative, and it can be further obtained that SW(q) is strictly concave with respect to q in this range (i.e. Q_{2} ) and its maximum q_{max} is unique. From Remark 2, we need to discuss whether q belongs to the set Q_{1} or set Q_{2} . Specifically, the socially optimal joining strategy for the cooperative case is given in Theorem 5.
Remark 3. If (\lambda + \theta)R - C < 0 , i.e., \frac{R}{C} < \frac{1}{{\lambda + \theta }} , then SW''(q) is positive under the stability condition 0 \leq q < \frac{{\theta\mu\gamma}}{\lambda(\lambda+\theta)(\alpha+\gamma)} , it is difficult to get the maximum value of SW(q) under the stable condition. So in the following theorem, we will only study the range of (\lambda + \theta)R - C > 0 , i.e., \frac{R}{C} > \frac{1}{{\lambda + \theta }} .
Theorem 5. For the M/M/1/MV constant retrial queueing system with N -policy and breakdowns in the steady state. For \frac{R}{C} > \frac{1}{{\lambda + \theta }} and any given price p < R , the number and the type of socially optimal joining strategy for cooperative case depend on the values of q_{max} , and the set Q . Specifically, the customers join the orbit with probability q^{*} upon their arrival by seeing an unavailable server, the joining probability q^{*} maximizes social welfare, where q^{*} is given by:
Case I: \frac{R}{C} < \frac{\theta}{\lambda+\theta} T(q_{max}) . In this case, the socially optimal strategy is q^{*} = 0 .
Case II: \frac{R}{C} = \frac{\theta}{\lambda+\theta} T(q_{max}) .
Case II (a): q\in Q_{1} and q_{max} > 1 . Then, the socially optimal strategy is q^{*} = 0 .
Case II (b): q\in Q_{1} and q_{max} = 1 . Then, the socially optimal strategy is q^{*} = 1 .
Case II (c): q\in Q_{1} and q_{max} < 1 . Then, the socially optimal strategy is q^{*} = q_{max} .
Case II (d): q\in Q_{2} . Then, the socially optimal strategy is q^{*} = q_{max} .
Case III: \frac{R}{C} > \frac{\theta}{\lambda+\theta} T(q_{max}) . Then, the socially optimal strategy is q^{*} = min (q_{max}, 1) .
Proof According to the previous description, under the stability condition ( 0 \leq q < \frac{{\theta\mu\gamma}}{\lambda(\lambda+\theta)(\alpha+\gamma)} ), if (\lambda + \theta)R - C > 0 , SW''(q) is strictly concave for q \in [0, \frac{{\theta\mu\gamma}}{\lambda(\lambda+\theta)(\alpha+\gamma)}) and its maximum q_{max} is unique in this interval (i.e. Q_{2} ). According to the reward-cost structure, we have the following results:
(I) When \frac{R}{C} < \frac{\theta}{\lambda+\theta} T(q_{max}) , which implies SW(q_{max}) < 0 , then SW(q) is negative for every q ( q \in Q ). So, it has no positive social welfare for any q . In this case, the socially optimal strategy q^{*} = 0 (balking). Thus we have the Case I.
(II) When \frac{R}{C} = \frac{\theta}{\lambda+\theta} T(q_{max}) , which implies SW(q_{max}) = 0 , then SW(q) \leq 0 of q\in Q . So, SW(q) is negative for each q \ne q_{max} . There are four cases, (a): if q\in Q_{1} and q_{max} > 1 , which implies SW(q) < 0 for every q\in Q because of q_{max} \notin Q , i.e., there is no point such that SW(q) = 0 . In this subcase, the socially optimal strategy q^{*} = 0 (balking). (b): if q\in Q_{1} and q_{max} = 1 , since SW(1) = 0 , we have that the maximum value of SW(q) in q \in Q = Q_{1} is 0, so the socially optimal strategy is attained q^{*} = 1 . (c): if q\in Q_{1} and q_{max} < 1 , similar to the discussion of Case II (b), the socially optimal strategy is attained q^{*} = q_{max} . (d): if q\in Q_{2} , since q_{max} must belong to the set Q_{2} , and SW(q_{max}) = 0 , so a unique socially optimal strategy is attained at q^{*} = q_{max} , there is no other point such that SW(q) = 0 of q\in Q = Q_{2} . Thus we have the Case II.
(III) When \frac{R}{C} > \frac{\theta}{\lambda+\theta} T(q_{max}) , which implies SW(q_{max}) > 0 , i.e. the maximum value of SW(q) in q \in [0, \frac{{\theta\mu\gamma}}{\lambda(\lambda+\theta)(\alpha+\gamma)})) is attained at some point. Since the strict concavity of SW(q) as a function of q \in [0, \frac{{\theta\mu\gamma}}{\lambda(\lambda+\theta)(\alpha+\gamma)})) , we can get the maximum value q_{max} is unique. When q \leq q_{max} , SW(q) is strictly increasing; while SW(q) is strictly decreasing for q \geq q_{max} . So the maximum value of SW(q) is obtained at q^{*} = q_{max} , if q_{max} \leq 1 , otherwise the maximum value of SW(q) is obtained at q^{*} = 1 . Thus we have the Case III.
The problem of maximizing the profit of the server is also considered in the actual service system. As mentioned before, all customers who join the system are charged an admission fee p (announced price, i.e., service price) by the server. On the other hand, running the server will generate consumption costs. In fact, the profit of the server is dynamic, and the server can maximize the profit by adjusting the admission fee p and the service rate \mu .
Assume that the server has no consumption cost in the vacation state and repair state. However, the consumption costs of the server in idle and busy states are C_{i} and C_{b} , respectively. Then, the profit of the service provider per time unit under equilibrium is given by
| \begin{equation} S(\mu, p) = (\lambda{q _e}(P_{0}(1)+P_{1}(1)+P_{3}(1))+\lambda P_{2}(1))p - ({C_b}{P_1}(1) + {C_i}{P_2}(1)), \end{equation} | (5.1) |
where q_{e} is the equilibrium joining probability, which is given in Theorem 4, P_{0}(1) , P_{1}(1) , P_{2}(1) and P_{3}(1) are given in Theorem 1.
The expression S(\mu, p) of the profit of the service provider per time unit under equilibrium is complex, so it is usually difficult to obtain analytical characterization. Therefore, we can give some numerical analysis by Particle Swarm Optimization (PSO) algorithm to overcome the difficulty of obtaining analytical characterization.
PSO algorithm is a global random search algorithm based on swarm intelligence proposed by [23], inspired by the results of artificial life research, by simulating the migration and swarming behavior of birds swarm foraging. The basic idea of the PSO algorithm comes from mutual cooperation and information sharing between groups to obtain the optimal solution. Because of its simple operation and fast convergence speed, the PSO algorithm has been widely used in many fields such as function optimization, image processing, and geodesy.
The PSO algorithm is first initialized as a group of random particles (random solution), and then the optimal solution is found through multiple iterations. In each iteration, the particles continuously update the optimal values of velocity V_{id} and position X_{id} and finally obtain the global optimum through multiple iterations, where velocity V_{id} represents the speed of movement, position X_{id} represents the direction of movement, the number of particles represents the dimension of the solutions. The position of each particle represents a potential optimal solution. V_{id} and X_{id} are updated by the following formulas,
| \begin{equation} {V_{id}} = \omega *{V_{id}} + {c_1}*rand()*(pBes{t_i} - {X_{id}}) + {c_2}*rand()*(gBes{t_i} - {X_{id}}), \end{equation} | (5.2) |
and
| \begin{equation} {X_{id}} = {X_{id}} + {V_{id}}, \end{equation} | (5.3) |
where i is the number of particles, d is dimension, rand() is a random number in (0, 1) , c_{1} and c_{2} are learning factor and \omega is inertia factor.
In this section, the theoretical knowledge we have obtained based on the previous research contains many parameters, so we explore the impact of these parameters on the two joining strategies ( q_{e} and q^{*} ) and social welfare SW(q) through a series of numerical experiments. On the other hand, due to the complexity of the expression S(\mu, p) of the profit of the service provider per time unit under equilibrium, we numerically analyze how the service provider sets up the price p and service rate \mu to maximize its own benefit. To address these issues, through a large number of numerical experiments on various parameters, regardless of the choice of the parameters, we find that the qualitative results of the numerical experiments are similar. So we present some typical numerical scenarios to illustrate the findings of these numerical experiments. The first set of numerical experiments presents the trends of the two joining strategies ( q_{e} and q^{*} ) on the parameters \lambda , \mu , \theta , \xi , \alpha , \gamma and N , respectively, in Figures 2–5, respectively.
Figure 2 (a) shows that q_{e} and q^{*} have a downward trend with arrival rate \lambda , and q_{e} is always above q^{*} . The reason is that as \lambda increases, more and more customers join the system, and the orbit becomes more crowded, the waiting cost of the later arriving customers is increasing, which will lead to a gradual decline in the enthusiasm of customers to join the system. However, as the service rate \mu increases, q_{e} and q^{*} show an increasing trend as shown in Figure 2 (b). This is because as the service rate \mu increases, the customers on the orbit get fast service, and waiting costs will be reduced. Therefore, no matter the cooperation case or non-cooperation case, the arriving customers are more willing to join the unavailable system.
Figure 3 (a) indicates that q_{e} and q^{*} increase as \xi increases, but the growth trend is not very obvious. Since as the vacation time becomes shorter, the number of customers accumulating on the orbit is reduced, so the arriving customers are more likely to join the system when it is unavailable. Figure 3 (b) shows that both q_{e} and q^{*} increase with the retrial rate \theta . The reason is that as the retrial rate continues to increase, the customers accumulating in the orbit get fast service, which leads to the newly arriving customers who are more willing to join the unavailable system.
Obviously, the life cycle of the server effectively affects the joining probability of the arriving customers in the cooperation case and non-cooperation case. In Figure 4 (a), q_{e} and q^{*} show a downward trend with the increase of \alpha . This is because the shortened lifetime of the server leads to an increase in the number of server repairs within a certain period, which will increase the waiting cost of customers in the orbit. So the newly arriving customers are more reluctant to join the system when they find it unavailable. However, the shortening of the server maintenance time, i.e., increase \gamma , it will effectively increase the joining probability of the customers in the two cases, which can be shown in Figure 4 (b). This is exactly the opposite of Figure 4 (a) because the increase in \gamma reduces the waiting costs of the customers in the orbit.
Figure 5 (a) indicates that q_{e} and q^{*} are inconsistent as functions of the threshold N . This is because as N increases, the enthusiasm of selfish customers for joining the system gradually decreases, i.e., q_{e} decreases with N . However, as N increases, accumulating more customers on the waiting list is completely coincide with the wishes of the social planner. Figure 5 (b) shows that the increase in announcement price p leads to a gradual decline in the enthusiasm of selfish customers, i.e., the customers in non-cooperative case, but p has no effect on the customers in the cooperation case. Obviously, as the service price p increases, the probability q_{e} of the arriving customers (the customers in non-cooperative case) to join the unavailable system must be reduced. However, as discussed in Theorem 5, since the service price p is only a transfer relationship between the customers and the server or the service provider, so q^{*} remains unchanged.
Social planners are usually more concerned about the social welfare of the service system, especially the maximum social welfare. This multiple vacation retrial queue with N -policy and breakdowns studied in this paper has more parameters, which effectively affect the trend of social welfare. Through a large number of numerical experiments, we find that the qualitative results of the influence of these parameters on social welfare are similar. Figures 6–8 present typical numerical experiments illustrating the respective effects of parameters R , C , \lambda , \mu , \theta , N , \alpha , \gamma and \xi on the maximum social welfare SW(q^{*}) of this service system.


From Figure 6 (a), we can see that the social planner increases the reward R of the customers will promote the growth of maximum social welfare, because as R increases, it will encourage more and more customers to join the unavailable system, which has a positive stimulating effect on the net profit of this service system. Conversely, increasing waiting cost of C per unit time will reduce the enthusiasm of customers to join the unavailable system, which will lead to a decrease in the maximum social welfare as the waiting cost of C per unit time increases, which as shown in Figure 6 (a).
Figure 6 (b) shows that the growth of both \lambda and \mu can increase the maximum social welfare. Although the probabilities q_{e} and q^{*} of the two joining cases decrease as the arrival rate \lambda increases, which can be seen in Figure 2 (a), the maximum social welfare SW(q^{*}) may not be significantly affected. This is because when the arriving customers find that the system is available, the arriving customers quickly join the system with a probability of 1, which effectively promotes the increase in social welfare. The increase in service rate \mu allows the customers in the orbit to get fast service, and the waiting costs of the customers are reduced. As shown in Figure 2 (b), the customers are more willing to join the unavailable system. Therefore, the increase in service rate \mu effectively promotes the growth of social welfare.
As shown in Figure 4 (a), the increase of \alpha shortens the available time of the server, which leads to a decrease in the probabilities of q_{e} and q^{*} of the two joining cases. As a result, social welfare must decline, which can be seen in Figure 7 (a). On the contrary, as shown in Figure 4 (b), the shortened repair time increases the available time of the server, and the customers under the two joining cases are more willing to join the unavailable system. As a result, Figure 7 (a) shows that the maximum social welfare increases as \gamma increases. The social planner hopes to reduce the server's vacation time to reduce the number of customers on the waiting list, and the probability q_{e} and q^{*} of the customers also conform to that shown in Figure 3 (a). As shown in Figure 7 (b), this will contribute to the growth of social welfare.
Figure 8 (a) illustrates that the maximum social welfare SW(q^{*}) is an increasing function of the retrial rate \theta . As shown in Figure 3 (b), as the retrial rate \theta increases, the retrial frequency of the customers in the orbit is accelerated, and the arriving customers are more willing to join the system when the system is unavailable. Because the waiting cost of the customers is reduced, which encourages more customers to join the unavailable service system, which promotes the growth of maximum social welfare SW(q^{*}) . In Figure 8 (b), the social welfare SW(q^{*}) decreases with N . This is because, with the increase of N , the more customers accumulate in the vacation state, the customers who later arrive at the system are more reluctant to join the system, which ultimately leads to a decline in the net income of the whole service system.
From the discussion in Section 5, we know that the profit of the service provider is dynamic, and the server can obtain the maximum profit by adjusting the entrance fee p and the service rate \mu . It is difficult for us to obtain an explicit or closed-form representation of S(\mu, p) , so we use the PSO algorithm introduced earlier to explore how the server adjusts the price p and service rate \mu to obtain maximum profit under equilibrium. We have run a large number of numerical experiments to find that their qualitative results are similar, so we illustrate the results through typical numerical scenarios. The key procedure of applying the PSO algorithm to find an optimal solution ( S(\mu, p) ) is illustrated in Algorithm 1, where the velocity {V_{id}} and position {X_{id}} are given in (5.2) and (5.3), respectively. Specifically, Figure 9 gives an example of finding the optimal solution of S(\mu, p) through the improved PSO algorithm.
| Algorithm 1 A example of searching for the maximum profit S(\theta, p) of the server |
|
Require: R , C , \lambda , \xi , N , \theta , \alpha , \gamma , C_{b} , C_{i} ; Ensure: \mu , p , S(\theta, p) ; 1: for each particle i 2: Initializing velocity {V_{id}} and position {X_{id}} for each particle i 3: Evaluating particle i and setting pBest_{i}={X_{id}} 4: end for 5: gBest_{i} =min \{ pBest_{i} \} 6: while not stop 7: for i =1 to M 8: Updating the velocity and position of particle i 9: Evaluating particle i 10: if fit ({X_{id}}) < fit (pBest_{i}) 11: pBest_{i} = {X_{id}} 12: if fit (pBest_{i}) < fit (gBest_{i}) 13: gBest_{i} = pBest_{i} 14: end for 15: end while 16: print gBest_{i} 17: end |
Now, we apply the PSO algorithm to explore how the server adjusts the service price p and service rate \mu to maximize profit under the case of q_{e} \neq 0 and \mu \neq 0 . On the basis of the service price p and service rate \mu as control variables, as shown in Figure 10 (a), the profit S(\mu, p) of the service provider decreases with respect to the arrival rate \lambda . This is because as the arrival rate \lambda increases, more and more customers accumulate in the orbit. At this time, the server needs to increase the service rate \mu to reduce congestion, and the increase in service price p is used to reduce service costs. Ultimately, because of the increase in the arrival rate \lambda , the congestion of the system, and the increase in waiting costs have led to a decline in the profit S(\mu, p) of the server. However, as shown in Figure 10 (b), the profit S(\mu, p) of the service provider increases with respect to the retrial rate \theta . The reason is that the increase in the retrial rate \theta helps reduce the length of the orbit, which promotes the rapid operation of the system, the result is an increase in the profits of the service provider.

Figure 11 (a) illustrates that the profit S(\mu, p) of the server decreases with respect to the consumption cost C_{i} of the server per time unit in the idle state. This is because as the increase of C_{i} , the unit time consumption cost of the idle state keeps increasing, and the social planner will shorten the idle time of the server to reduce the consumption cost. The shortening of idle time reduces the number of customers who join the system with probability 1 when the system is available. In the case of increased costs and a decrease in the number of customers who join the system with probability 1, the profit of the service provider is ultimately reduced. Similarly, as shown in Figure 11 (b), with the increase of C_{b} , the unit time consumption of the busy state will continue to increase. In order to reduce costs, the server will shorten the working time of the server, which will cause more and more customers to accumulate in the orbit, the customers who arrive later are more reluctant to join the system, which will eventually lead to a decrease in the effective arrival rate, which will result in a decrease in the server profit S(\mu, p) .

Motivated by the cost control of service and information, a multiple vacations retrial queue with N -policy and breakdowns is studied in this paper. Two types of customer joining cases apply to this paper, i.e., the non-cooperative customers aim to optimize individual interests, and the social planner in the cooperative case consider the profit of the whole service system. For this service system, we presented the equilibrium joining strategy for the non-cooperative case and the socially optimal joining strategy for the cooperative case.
For the maximum profit of the server, it is difficult for us to obtain an explicit or closed-form representation of S(\mu, p) , so we use the improved PSO algorithm to explore how the server obtains maximum profit. A large number of numerical experiments show that the qualitative results of the parameters are similar to the system, so we use some typical numerical scenarios to explain the influence of the parameters on the two joining probabilities, and how the parameters affect the changing trend of maximum social welfare. Both the model itself and the numerical experiment have certain guiding significance for the actual service system. Because there is no waiting space in front of the server and the virtuality of the waiting list, this model is suitable for emergency disaster or epidemic control. Changes in the parameters assumed by the model, resulting in changes in the joining strategy are interesting topics for future research.
This work is supported, in part, by The National Natural Science Foundation of China (No. 61773014), The National Natural Science Foundation of China (No. 11861027), the Research Fund for the Postgraduate Research and Practice Innovation Program of Jiangsu Province (No. KYCX20_0240), and the Natural Sciences and Engineering Research Council of Canada (No. 315660).
No potential conflict of interest was reported by the authors.
| [1] |
P. Naor, The Regulation of Queue Size by Levying Tolls, Econometrica, 37 (1969), 15–24. doi: 10.2307/1909200

|
| [2] |
N. Edelson, D. Hilderbrand, Congestion tolls for Poisson queuing processes, Econometrica, 43 (1975), 81–92. doi: 10.2307/1913415

|
| [3] |
Y. Shi, Z. Lian, Optimization and strategic behavior in a passenger–taxi service system, Eur. J. Oper. Res., 249 (2016), 1024–1032. doi: 10.1016/j.ejor.2015.07.031

|
| [4] |
O, Boudali, A. Economou, Optimal and equilibrium balking strategies in the single server markovian queue with catastrophes, Eur. J. Oper. Res., 218 (2012), 708–715. doi: 10.1016/j.ejor.2011.11.043

|
| [5] |
A. Economou, A. Manou, Equilibrium balking strategies for a clearing queueing system in alternating environment, Ann. Oper. Res., 208 (2013), 489–514. doi: 10.1007/s10479-011-1025-x

|
| [6] |
A. Burnetas, A. Economou, Equilibrium customer strategies in a single server markovian queue with setup times, Queueing Syst., 56 (2007), 213–228. doi: 10.1007/s11134-007-9036-7

|
| [7] |
Y. Zhang, J. Wang, Equilibrium pricing in an M/G/1 retrial queue with reserved idle time and setup time, Appl. Math. Model., 49 (2017), 514–530. doi: 10.1016/j.apm.2017.05.017

|
| [8] |
J. Chang, J. Wang, Unreliable M/M/1/1 retrial queues with set-up time, Qual. Technol. Quantit. M., 15 (2018), 589–601. doi: 10.1080/16843703.2017.1320459

|
| [9] | R. Hassin, M. Haviv, To Queue or Not to Queue: Equilibrium Behavior in Queueing Systems, Kluwer Academic Publishers, Boston, 2003. |
| [10] | L. Kosten, Stochastic theory of service systems, Pergamon Press, University of California, 1973. |
| [11] |
T. Jiang, Tail asymptotics for a batch service polling system with retrials and nonpersistent customers, J. Math. Anal. Appl., 459 (2018), 893–905. doi: 10.1016/j.jmaa.2017.11.023

|
| [12] | T. Phung-Duc, Single server retrial queues with setup time, J. Ind. Manag. Optim., 13 (2017), 75–78. |
| [13] |
A. Economou, S. Kanta, Equilibrium customer strategies and social–profit maximization in the single-server constant retrial queue, Nav. Res. Log., 58 (2011), 107–122. doi: 10.1002/nav.20444

|
| [14] | J. Wang, W. Li, Noncooperative and cooperative joining strategies in cognitive radio networks with random access, IEEE T. Veh. Technol., 65 (2015), 5624–5636. |
| [15] |
J. Wang, F. Zhang, Monopoly pricing in a retrial queue with delayed vacations for local area network applications, IMA J. Manag. Math., 27 (2016), 315–334. doi: 10.1093/imaman/dpu025

|
| [16] | L. Barroso, U. Holzle, The case for energy-proportional computing, Computer, 40 (2007), 33–37. |
| [17] |
Z. Wang, L. Liu, Y. Shao, X. Chai, B. Chang, Equilibrium joining strategy in a batch transfer queuing system with gated policy, Methodol. Comput. Appl., 22 (2020), 75–99. doi: 10.1007/s11009-018-9687-3

|
| [18] |
P. Guo, R, Hassin, Strategic behavior and social optimization in markovian vacation queues, Oper. Res., 59 (2011), 986–997. doi: 10.1287/opre.1100.0907

|
| [19] |
J. Wang, X. Zhang, P. Huang, Strategic behavior and social optimization in a constant retrial queue with the n-policy, Eur. J. Oper. Res., 256 (2017), 841–849. doi: 10.1016/j.ejor.2016.06.034

|
| [20] |
W. Sun, S. Li, E. Guo, Equilibrium and optimal balking strategies of customers in markovian queues with multiple vacations and N-policy, Appl. Math. Model., 40 (2016), 284–301. doi: 10.1016/j.apm.2015.04.045

|
| [21] |
Q. Ye, L. Liu, The analysis of discrete time Geom/Geom/1 queue with single working vacation and multiple vacations (geom/geom/1/swv+ mv), RAIRO-Operations Res., 52 (2018), 95–117. doi: 10.1051/ro/2017079

|
| [22] | S. Gao, H. Dong, X. Wang, Equilibrium and pricing analysis for an unreliable retrial queue with limited idle period and single vacation, Oper. Res., (2018), 1–23. |
| [23] | R. Eberhart, J. Kennedy, A new optimizer using particle swarm theory, MHS'95. Proceedings of the Sixth International Symposium on Micro Machine and Human Science, (1995), 39–43. |
| 1. | Wojciech M. Kempa, Dariusz Kurzyk, On Transient Queue-Size Distribution in a Model of WSN Node with Threshold-Type Power-Saving Algorithm, 2022, 22, 1424-8220, 9285, 10.3390/s22239285 | |
| 2. | Wojciech M. Kempa, Rafał Marjasz, Study on Transient Queue-Size Distribution in the Finite-Buffer Model with Batch Arrivals and Multiple Vacation Policy, 2021, 23, 1099-4300, 1410, 10.3390/e23111410 | |
| 3. | Madhu Jain, Sibasish Dhibar, ANFIS and metaheuristic optimization for strategic joining policy with re-attempt and vacation, 2023, 03784754, 10.1016/j.matcom.2023.03.024 | |
| 4. | Wei Sun, Xumeng Xie, Zhiyuan Zhang, Shiyong Li, Equilibrium balking behavior of customers and regulation measures in a multi-server queue with threshold policy, 2024, 1684-3703, 1, 10.1080/16843703.2024.2358613 | |
| 5. | Sibasish Dhibar, Madhu Jain, ANFIS simulation integrated in FM/FM/1/(CV + WV) queue with Bernoulli service interruption and metaheuristic optimization for mathematical model, 2025, 81, 0920-8542, 10.1007/s11227-024-06481-3 | |
| 6. | Sibasish Dhibar, Madhu Jain, Metaheuristics and strategic behavior of markovian retrial queue under breakdown, vacation and bernoulli feedback, 2025, 55, 0924-669X, 10.1007/s10489-024-05978-x |
| Algorithm 1 A example of searching for the maximum profit S(\theta, p) of the server |
|
Require: R , C , \lambda , \xi , N , \theta , \alpha , \gamma , C_{b} , C_{i} ; Ensure: \mu , p , S(\theta, p) ; 1: for each particle i 2: Initializing velocity {V_{id}} and position {X_{id}} for each particle i 3: Evaluating particle i and setting pBest_{i}={X_{id}} 4: end for 5: gBest_{i} =min \{ pBest_{i} \} 6: while not stop 7: for i =1 to M 8: Updating the velocity and position of particle i 9: Evaluating particle i 10: if fit ({X_{id}}) < fit (pBest_{i}) 11: pBest_{i} = {X_{id}} 12: if fit (pBest_{i}) < fit (gBest_{i}) 13: gBest_{i} = pBest_{i} 14: end for 15: end while 16: print gBest_{i} 17: end |
| Algorithm 1 A example of searching for the maximum profit S(\theta, p) of the server |
|
Require: R , C , \lambda , \xi , N , \theta , \alpha , \gamma , C_{b} , C_{i} ; Ensure: \mu , p , S(\theta, p) ; 1: for each particle i 2: Initializing velocity {V_{id}} and position {X_{id}} for each particle i 3: Evaluating particle i and setting pBest_{i}={X_{id}} 4: end for 5: gBest_{i} =min \{ pBest_{i} \} 6: while not stop 7: for i =1 to M 8: Updating the velocity and position of particle i 9: Evaluating particle i 10: if fit ({X_{id}}) < fit (pBest_{i}) 11: pBest_{i} = {X_{id}} 12: if fit (pBest_{i}) < fit (gBest_{i}) 13: gBest_{i} = pBest_{i} 14: end for 15: end while 16: print gBest_{i} 17: end |