Numerous graph energies of regular subdivision graph and complete graph

1.   Introduction

Consider a simple connected graph $G = (V(G), E(G))$ having $|V(G)| = p$ vertices and $E(G) = q$ edges. Number of edges in the neighborhood of a vertex $x$ in a graph $G$ is named as degree of that vertex and is denoted by $d_x$ or $d(x)$. If the number of edges in the neighborhood of each vertex in a graph are same say $s$ then graph is said to be a $s$-regular graph.

Adjacency matrix is a $p\times{p}$ matrix having entries $a_{xy}$ such that

The eigenvalues of a graph are actually eigenvalues of its $A(G)$. The set which is constructed from eigenvalues of $G$ with their multiplicities is known as spectrum of $G$.

In Mathematics, the graph energy was firstly introduced by Ivan Gutman in 1978. Graph energy is built upon eigenvalues of $A(G)$. It is sum of absolute values of elements of spectrum of $G$. For a $p$-vertex graph $G$ with eigenvalues $\beta_k$ in non-increasing order for $k = 1, 2, 3, ..., p$,

The impression which is stated in Eq (1.1) is associated with computational chemistry. If, in a conjugated hydrocarbon system, the eigenvalues of a molecular graph are $\alpha_1, \alpha_2, \alpha_3, ..., \alpha_p$ and are in non-increasing order. Then Hückel molecular orbital approximation calculated the total $\Pi$-electron energy $E_{\Pi}$ as

for $p$ is even and

for $p$ is odd with $\gamma$ and $\delta$ are constants.

A large number of research papers have been published on graph energy. The thesis of Siraj ^[1] contains some elementary determinations of graph energy.

This paper include upper bounds of different graph energies of subdivision graph $S(G)$ of $s$-regular graph $G$ containing $p$ vertices and $q$ edges. Also various graph energies of complete graph are explored in this paper.

Based on eigenvalues of different graph matrices, several energies of a graph have been such as maximum degree energy, seidel energy, sum-connectivity energy etc. These energies depends upon eigenvalues of their corresponding energy matrices, see ^[2,3,4].

2.   Energies of $s$-regular subdivision graph

First we define subdivision graph.

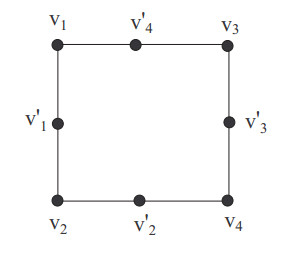
Definition 2.1 (Subdivision graph). The subdivision graph $S(G)$ of a graph $G$ is acquire by dividing each edge of $G$ into two edges with the help of a vertex of degree $2$ on every edge. Thus $|V(S(G))| = |V(G)|+|E(G)|$ and $|E(S(G))| = 2|E(G)|$. The graph of subdivision of cycle $C_4$ is shown in Figure 1.

2.1.   Degree energies

In this section, we present bounds of maximum degree energy, minimum degree energy, Randić energy, sum-connectivity energy and first and second Zagreb energies. Firstly, we define these energies.

Definition 2.2 (Maximum degree energy). ^[5] The maximum degree energy $E_{M}$ of a simple graph $G$ is define as the sum of the absolute eigenvalues of its maximum degree matrix $M(G)$ where $M(G)$ has $(i, j)$th entry $max(d_j, d_j)$ if $v_iv_j\in{E(G)}$ and $0$ elsewhere.

Definition 2.3 (Minimum degree energy). ^[6] The minimum degree energy $E_{m}$ of a simple connected graph $G$ is define as the sum of the absolute eigenvalues of minimum degree matrix $m(G)$ of a graph $G$ where $(i, j)$th entry of $m(G)$ is $min(d_i, d_j)$ if $v_iv_j\in{E(G)}$ and $0$ otherwise.

Definition 2.4 (Randi$\acute{c}$ energy). ^[7] The randi$\acute{c}$ energy $E_{R}$ of a simple connected graph $G$ is the sum of the absolute eigenvalues of the randi$\acute{c}$ matrix $R(G)$ where if $v_iv_j\in{E(G)}$ then $(i, j)$th entry of $R(G)$ is $\frac{1}{\sqrt{d_{i}d_{j}}}$ and $0$ elsewhere.

Definition 2.5 (Sum-connectivity energy). ^[8] The sum-connectivity energy $E_{SC}$ of a simple connected graph $G$ is define as the sum of the absolute eigenvalues of the sum-connectivity matrix $SC(G)$ where $(i, j)$th entry of $SC(G)$ is $\frac{1}{\sqrt{d_{i}+d_{j}}}$ if $v_iv_j\in{E(G)}$ and $0$ otherwise.

Definition 2.6 (First Zagreb energy). ^[9] The first Zagreb energy $ZE_{1}$ of a simple connected graph $G$ is define as the sum of the absolute eigenvalues of first Zagreb matrix $Z^{(1)}(G)$ of $G$ where $Z^{(1)}(G)$ has $(i, j)$th entry $d_i+d_j$ if $v_iv_j\in{E(G)}$ and $0$ otherwise.

Definition 2.7 (Second Zagreb energy). ^[9] The second Zagreb energy $ZE_{2}$ of a simple connected graph $G$ is define as the sum of the absolute eigenvalues of second Zagreb matrix $Z^{(2)}(G)$ of $G$ where $Z^{(2)}(G)$ has $(i, j)$th entry $d_i.d_j$ if $v_iv_j\in{E(G)}$ and $0$ otherwise.

In the following theorem, we give bounds of all above defined degree energies;

Theorem 2.8. Let $p$ and $q$ be vertices and edges of a regular graph $G$. Then

1. for maximum degree energy, we have $E_M(S(G))\leq{2s\sqrt{2pq}};$

2. for minimum degree energy, we have $E_m(S(G))\leq{4\sqrt{2pq}},$

3. for Randi$\acute{c}$ energy, we have $E_R(S(G))\leq{\sqrt{\frac{4pq}{s}}},$

4. for sum-connectivity energy, we have $E_{SC}(S(G))\leq{2\sqrt{\frac{2pq}{2+s}}},$

5. for first Zagreb energy, we have $ZE_1(S(G))\leq{2(s+2)\sqrt{2pq}},$

6. for second Zagreb energy, we have $ZE_2(S(G))\leq{4s\sqrt{2pq}}.$

Proof. Let the incidence matrix of $G$ is $C(G)$. Note that the degree matrix of the subdivision graph $S(G)$ can be stated as:

1. By taking $t = s$ in Eq (2.1), we have following computations for the maximum degree energy of the subdivision graph $S(G)$;

As in ^[12] $CC^{T} = L^{+}(G)$, we have

where $L^{+}(G)$ is signless Laplacian matrix and $\nu_j^+$ are eigenvalues of $L^+(G)$. Thus by Cauchy Schawaz inequality

Hence,

2. By taking $t = 2$ in Eq (2.1), we have following computations for the minimum degree energy of the subdivision graph $S(G)$;

Since,

Therefore,

3. By taking $t = \frac{1}{\sqrt{2s}}$ in Eq (2.1), we have following computations for the Randić energy of the subdivision graph $S(G)$;

As

Therefore,

4. By taking $t = \frac{1}{\sqrt{2+s}}$ in Eq (2.1), we have following computations for the sum-connectivity energy of the subdivision graph $S(G)$;

As

Hence,

5. By taking $t = s+2$ in Eq (2.1), we have following computations for the first Zagreb energy of the subdivision graph $S(G)$;

as

Hence,

6. By taking $t = 2s$ in Eq (2.1), we have following computations for the second Zagreb energy energy of the subdivision graph $S(G)$;

where

Hence,

2.2.   Seidel energy

Definition 2.9 (Seidel energy). ^[10] The Seidel energy $E_{SE}$ of a simple connected graph $G$ as the sum of the absolute eigenvalues of the seidel matrix $SE(G)$ of $G$. Here $SE(G) = [s_{ij}]$ where

Theorem 2.10. For an s-regular $(p, q)$ graph $G$,

Proof. Let $u_1, u_2, u_3, ..., u_p$ be vertices of an s-regular graph $G$ and let $u_j'$ for $1\leq{j}\leq{q}$ be vertices that are added at all edges of $G$ to gain $S(G)$. Note that $SE(S(G))$ is given as:

where $E_{p\times{q}} = [e_{pq}]$ such that

Therefore

As

and

Hence,

2.3.   Degree sum energy

Definition 2.11 (Degree sum energy). ^[11] The degree sum energy $E_{DS}$ of a simple connected graph $G$ is define as the sum of the absolute eigenvalues of the degree sum matrix $DS(G)$ of $G$ where $DS(G)$ has $(i, j)$th entry $d_i+d_j$ if $i\neq{j}$ and $0$ otherwise.

Theorem 2.12. For a s-regular graph G having p and q as order and size respectively,

Proof. Let $G$ has vertices $w_1, w_2, w_3, ..., w_p$ and $w_j'$ for $1\leq{j}\leq{q}$ be the vertices that are added at every edge of $G$ to acquire $S(G)$. Then

Or

Therefore

Hence,

2.4.   Degree square sum energy

Definition 2.13 (Degree square sum energy). ^[12] The degree square sum energy $E_{DSS}$ of a simple connected graph $G$ is define as the sum of the absolute eigenvalues of the degree square sum matrix $DSS(G)$ of $G$ where $DSS(G)$ has $(i, j)$th entry $d_i^2+d_j^2$ if $i\neq{j}$ and $0$ otherwise.

Theorem 2.14. For an s-regular graph $G$

Proof. Let for $1\leq{j}\leq{p}$, $u_j$ be vertices of $G$ and $u_k'$ for $1\leq{k}\leq{q}$ be the vertices that are added in $G$ to get $S(G)$. Note that the degree square sum matrix of $S(G)$ is denoted by $DSS(S(G))$ and is given as:

Or

Therefore

Or

Hence,

3.   Energies of complete graph

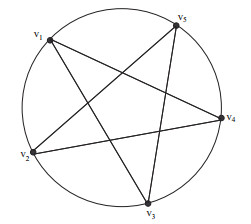
A complete graph denoted by $K_p$ is a simple undirected graph in which every pair of distinct vertices is connected by a unique edge. $K_5$ is shown in Figure 2.

We have following trivial results about energies of complete graphs.

Theorem 3.1. For the complete graph $K_p$, the maximum degree energy is

Theorem 3.2. For the complete graph $K_p$, the minimum degree energy is

Theorem 3.3. For the complete graph $K_p$, the Randić energy is

Theorem 3.4. For the complete graph $K_p$, the Seidel energy is

Theorem 3.5. For the complete graph $K_p$, the sum-connectivity energy is

Theorem 3.6. For the complete graph $K_p$, the degree sum energy is

Theorem 3.7. For the complete graph $K_p$, the degree square sum energy is

Theorem 3.8. For the complete graph $K_p$, the first Zagreb energy is

Theorem 3.9. For the complete graph $K_p$, the second Zagreb energy is

4.   Conclusions

In this paper we gave bounds of maximum degree energy, Randi$\acute{c}$ energy, sum-connectivity energy etc of $s$-regular subdivision graph $S(G)$. Also various graph energies of complete graph are mentioned in this article. In future, we aim to study graph energies for the other families of graphs.

Conflict of interest

Authors do not have any competing interests.