1.
Introduction
Let G be a connected graph with vertex set V(G) and edge set E(G). Two adjacent vertices w,z∈V(G) are denoted by wz (or zw). The order (resp. size) of G is |V(G)| (resp. |E(G)|). The distance between w and z in G is the length of a shortest path connecting w and z and is denoted by dG(w,z). For a vertex w∈V(G), its eccentricity is defined as eG(w)=max{dG(w,z)∣z∈V(G)}. For convenience, we can denote the eccentricity of w∈G by e(w) if no ambiguity occurs. Furthermore, the minimum eccentricity over all the vertices of a graph G is called its radius of G denoted by r(G). Similarly, the maximum eccentricity over all the vertices of a graph G is called diameter of G denoted by d(G). A vertex w∈V(G) is said to be diametrical (resp. central) vertex of G if e(w)=d(G) (resp. e(w)=r(G)). Moreover, the periphery P(G) of a graph G is defined as
Whereas, center of a graph G is the subgraph induced by the central vertices of G. The neighborhood NG(w) of a vertex w∈V(G) is defined as NG(w)={z∈V(G)∣wz∈E(G)}. The degree deg(w) of a vertex w in G is the number |NG(w)|. A connected graph is called self-centered if the eccentricities of all the vertices are equal; otherwise, G is called a non-self-centered graph, shown in Figure 1.
A molecular graph G represents a chemical compound. A topological index is a numerical parameter of a molecular graph G which characterizes the properties of G. Topological indices can be divided into many classes; some of them are degree-based, eccentricity-based, and distance-degree-based indices.
Recently, Xu et al. [7] introduced a new graphic invariant for indicating the non-self centrality of the graph more efficiently. This invariant was named as non-self centrality number (henceforth, NSC) of a graph G, which is given by
where sum is over all vertex pair of vertices of a graph G. For non-self-centered graph G, the formula (1.1) was further simplified using the eccentricity sequence of the graph. If the eccentricity ei appears li≥1 times in G we write, elii in short. Let us assume that e1>e2>⋯>ek be the distinct eccentricities of G with l1,l2,...,lk be their respective multiplicities. Then the eccentricity sequence is ζ(G)={el11,el22,…,elkk}. Therefore, the NSC number of G can be written as
We refer [4] to the readers for some more results on NSC number. By graph structure, we can easily calculate the eccentricities of its vertices. When the eccentricities of vertices of a graph are known, the eccentricity sequence can easily be obtained. Therefore, we will write directly the eccentricity sequences of graphs in this paper.
Ashrafi et al. [3] computed the eccentric-connectivity index of TUC4C8 nanotubes and nanotori. Also Kwun et al. [5] computed M-polynomials and topological indices like Zagreb indices of V-phenylenic nanotubes and nanotori. For further details, we refer [1,2,6]. Heretofore, NSC number is considered for finite family of graphs [7,4]. It motivates us to consider NSC number of infinite family of graphs. In this paper, we calculate NSC number of TUC4C8 and V-phenylenic nanotubes.
2.
V-phenylenic nanotubes
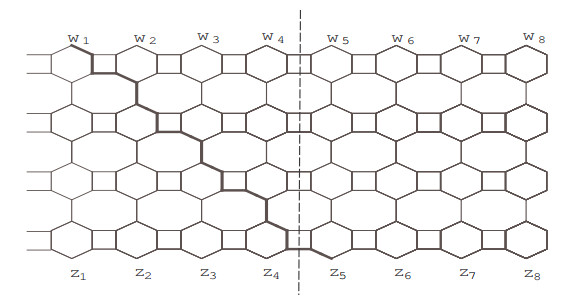
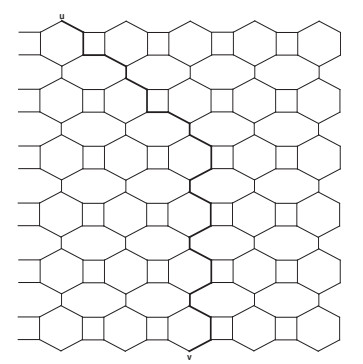
Here we compute the non-self centrality number of V-phenylenic nanotubes. An infinite structure of V-phenylenic nanotubes and nanotori is made by alternating C4, C6 and C8 cycles. The arrangement of C4, C6 and C8 cycles in V-phenylenic structure is such that C4 ring is attached to two C6 rings and also each C4 is attached to two C8 rings. We will denote the V-phenylenic nanotubes by G[h,f], where f and h are the number of columns and rows, respectively, as shown in the Figures 2 and 3. Consider
and
Then |X|=2h and |Y|=6hf−2h. From the structure of G[h,f], we notice that there are two types of edges given by
and
It is easy to see that |E1|=4h and |E2|=h(9f−5). Hence, |E|=|E1|+|E2|=h(9f−1) is the number of total edges in G[h,f].
Before moving to the main results, it is important to know that structure of V-phenylenic nanotube is symmetric and hence it can be divided into two halves as shown in Figure 2. Whereas, we can also divide the structure into two different classes depending on h and f. When h<2f we get a vertical structure shown in Figure 3, while we obtain a horizontal structure for h>2f. Now let us begin with the following result:
Lemma 1. For f≥2 the diameter of G[h,f] is
Proof. Let wi and zj, 1≤i,j≤h, be the peripheral vertices in G[h,f], as shown in Figure 2. We can partition E(G[h,f]) into three classes; horizontal, oblique and vertical edges. Now if h≤2f and h is an even integer then, to find the diameter we have to find the path's length that connects two vertices of X. Let wi be the vertex with an eccentric vertex zj in G[h,f], shown in Figure 2. For j<(h2+i), length of a shortest wi,zj-path contains 2f oblique, (2f−1) vertical and j−1 horizontal edges. Therefore,
Similarly, for j=(h2+i), the length of a shortest wi,zj-path contains exactly 2f−1 vertical, 2f oblique and h2 horizontal edges. Therefore,
Now for j>(h2+i), the length of the shortest wi,zj-path contains 2f oblique, (2f−1) vertical and h−j horizontal edges. Therefore,
From the above discussion and Figure 2, it is observed that dG[h,f](wi,zj)=h2+4f−1 is the maximum distance. Therefore, d(G[h,f])=h2+4f−1.
Similarly, in case of odd h, we choose wi and zj, where zj∈X∪Y, such that dG[h,f](wi,zj) is maximum. We need 2f oblique edges, 2f−1 vertical edges and h−12 horizontal edges to connect wi and zj. Hence, the diameter is given by d(G[h,f])=h−32+4f. In similar manner, we can find the diameter of G[h,f] when h<2f.
Theorem 2. For f≥2, we have
Proof. We discuss five possible cases:
Case 1: h>2f−1 and h is even.
Here we have
Using formula (1.2), the NSC number of G[h,f] is given by
After simplification, we get
Case 2: h>2f−1 and h is odd.
In this case, we have
Using (1.2), the NSC number of T[h,f] is given by
Case 3: h∈{2f−2,2f−1}.
The eccentricity sequence of G[h,f] in this case is given by
Using formula (1.2), the NSC number of G[h,f] is given by
After simplification, we obtain:
Case 4: h<2f−2 and f is even.
In this case, for G[h,f] we have
Using formula (1.2), the NSC number of G[h,f] is given by
After simplification we get
Case 5: h<2f−2 and f is odd.
In this case, the eccentricity sequence of G[h,f] is given by
By formula (1.2), the NSC number of G[h,f] is given by
Simplifying above, we obtain
This completes the proof.
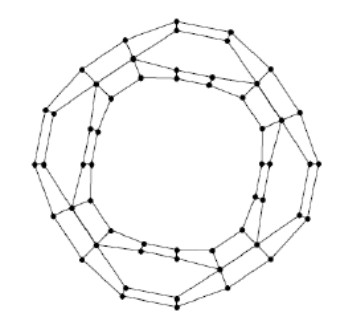
Remark. For V-phenylenic nanotori, the graph become self-centered. Therefore non-self-centrality number of V-phenylenic nanotori is zero.
3.
TUC4C8 nanotubes
In this section, we compute the non-self centrality number of TUC4C8 nanotubes. In the structure of TUC4C8 nanotube, every C4 cycle is adjacent to four C8 cycles. We will denote TUC4C8 nanotube by G[u,v], where s is the number of octagons in a fixed row and t denotes the sum of C4 and C8 cycles in a fixed column (see Figure 4).
Consider
Then |X|=4s and |Y|=4st. Also
Remark. The graph G[s,t] becomes self-centered for t=1. Therefore the non-self-centrality number of G[s,1] is zero.
Theorem 3. Assume that s≥2 and t is an odd integer. Then the NSC number of TUC4C8 nanotube is given by
Proof. The eccentricity sequence of G[s,t] is given by
Using formula (1.2) the NSC of G[s,t] is given by
This completes the proof.
Remark. The graph of TUC4C8 nanotori, shown in Figure 5, is self-centered. Therefore non-self-centrality number of nanotori is zero.
4.
Conclusions
In this paper, we computed general formulas for the non-self-centrality number of V-Phenylenic and TUC4C8(R) nanotubes. For future study, we can calculate the non-self-centrality number of other molecular structures such as α-Boron nanotubes, some layer structures and dendrimers.
Conflict of interest
The authors have no conflict of interest to declare.










 DownLoad:
DownLoad: